Computational Study of the Propeller Position Effects in Wing-Mounted, Distributed Electric Propulsion with Boundary Layer Ingestion in a 25 kg Remotely Piloted Aircraft
Abstract
:1. Introduction
2. Design and Component Selection
3. Methods
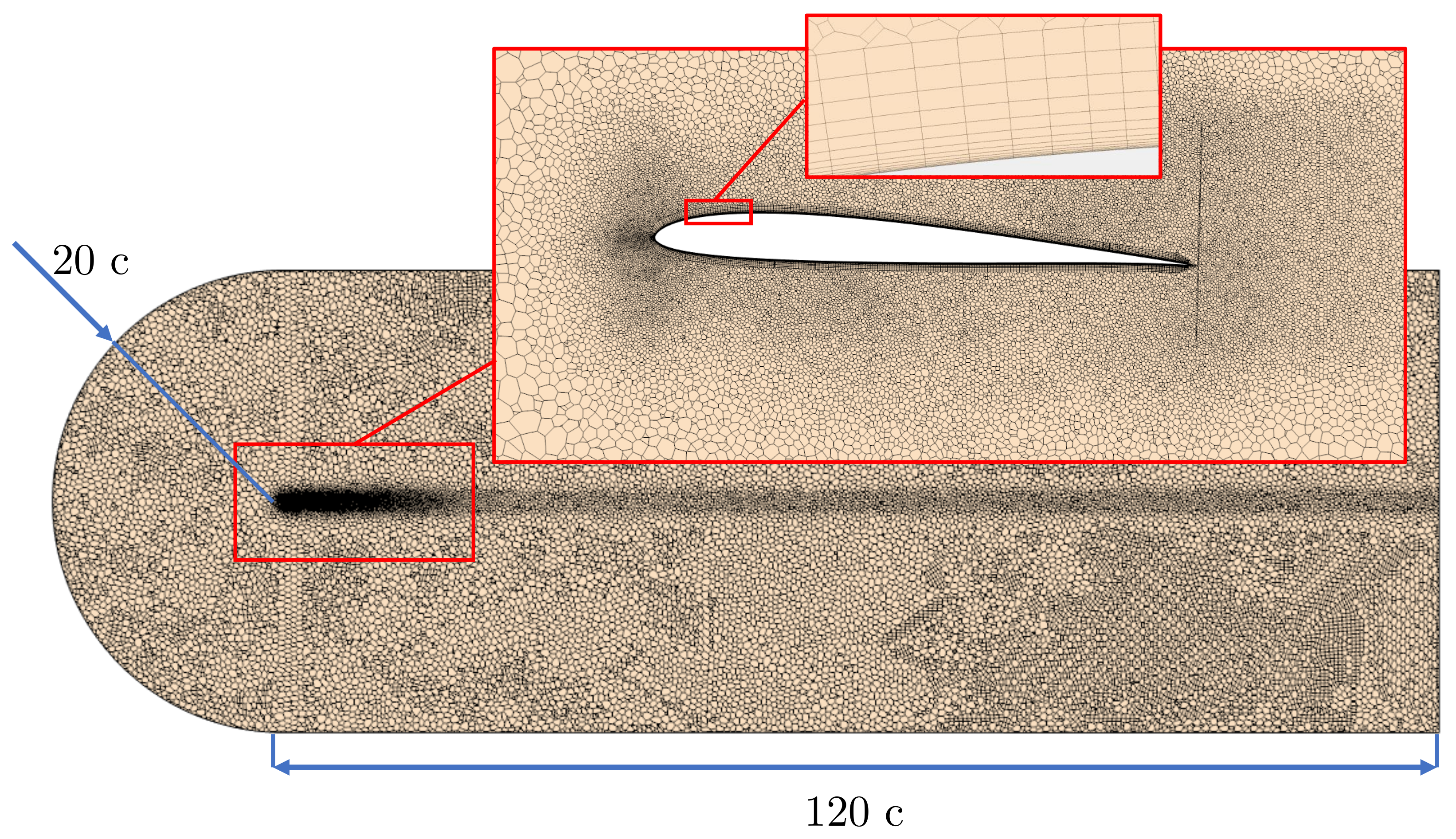
3.1. Computational Setup
3.2. CFD Methodology
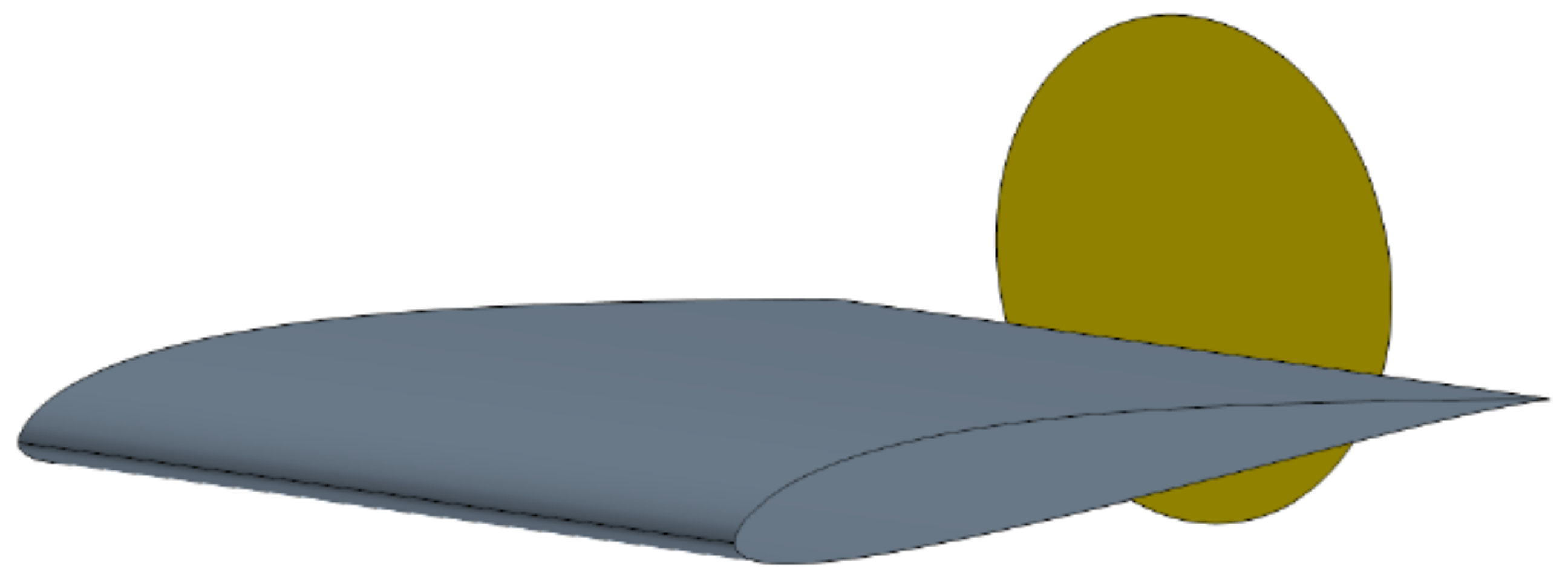
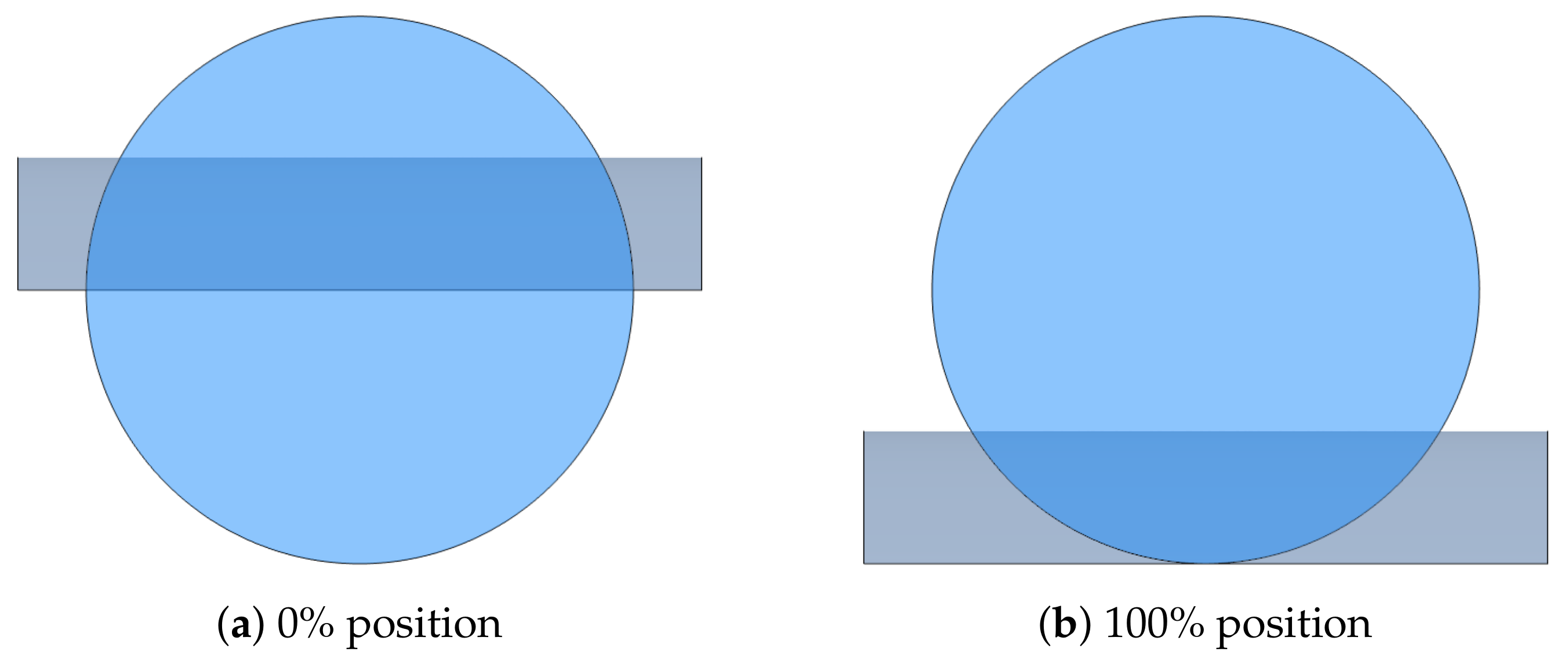
3.3. Actuator Disc Setup
4. Results and Discussion
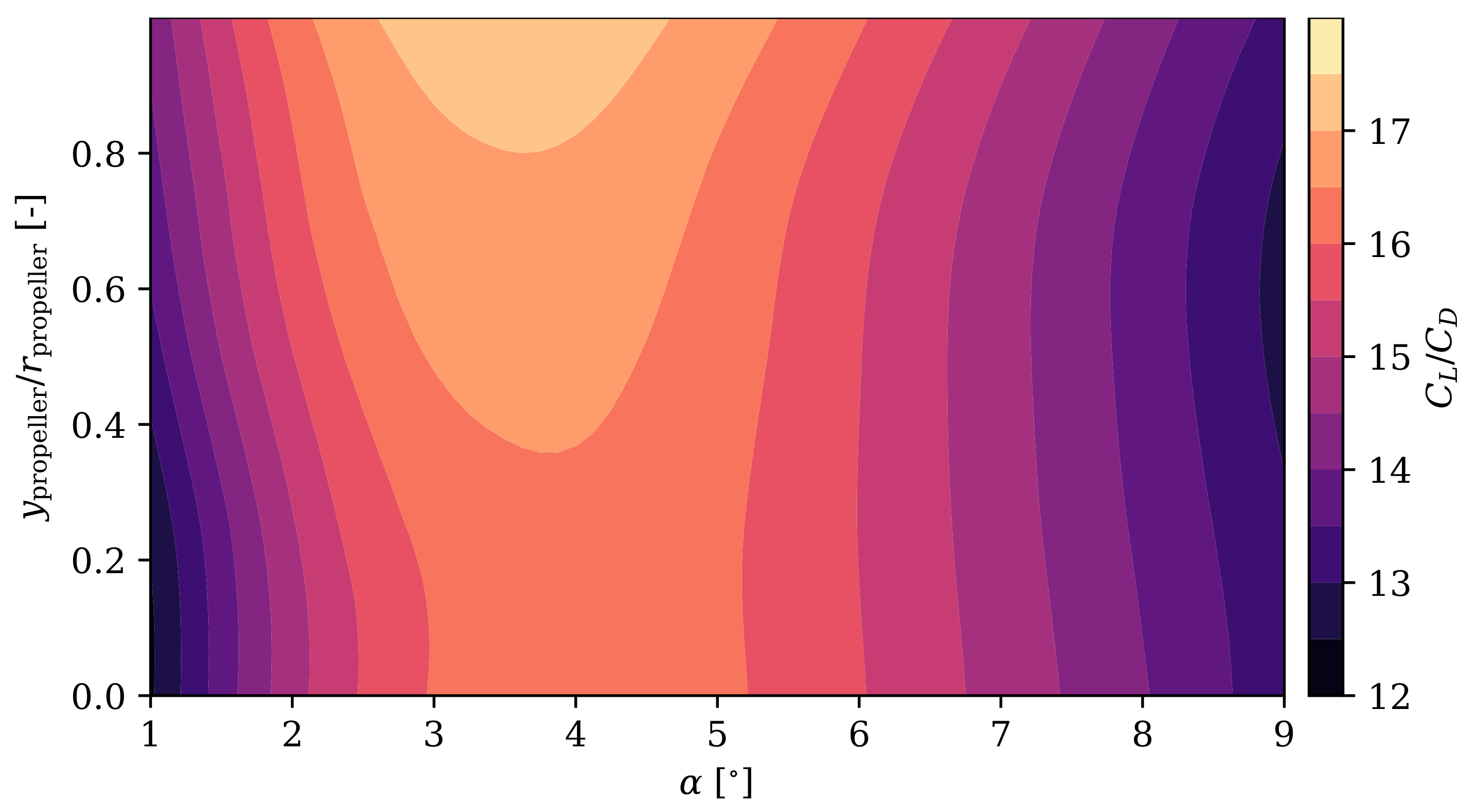
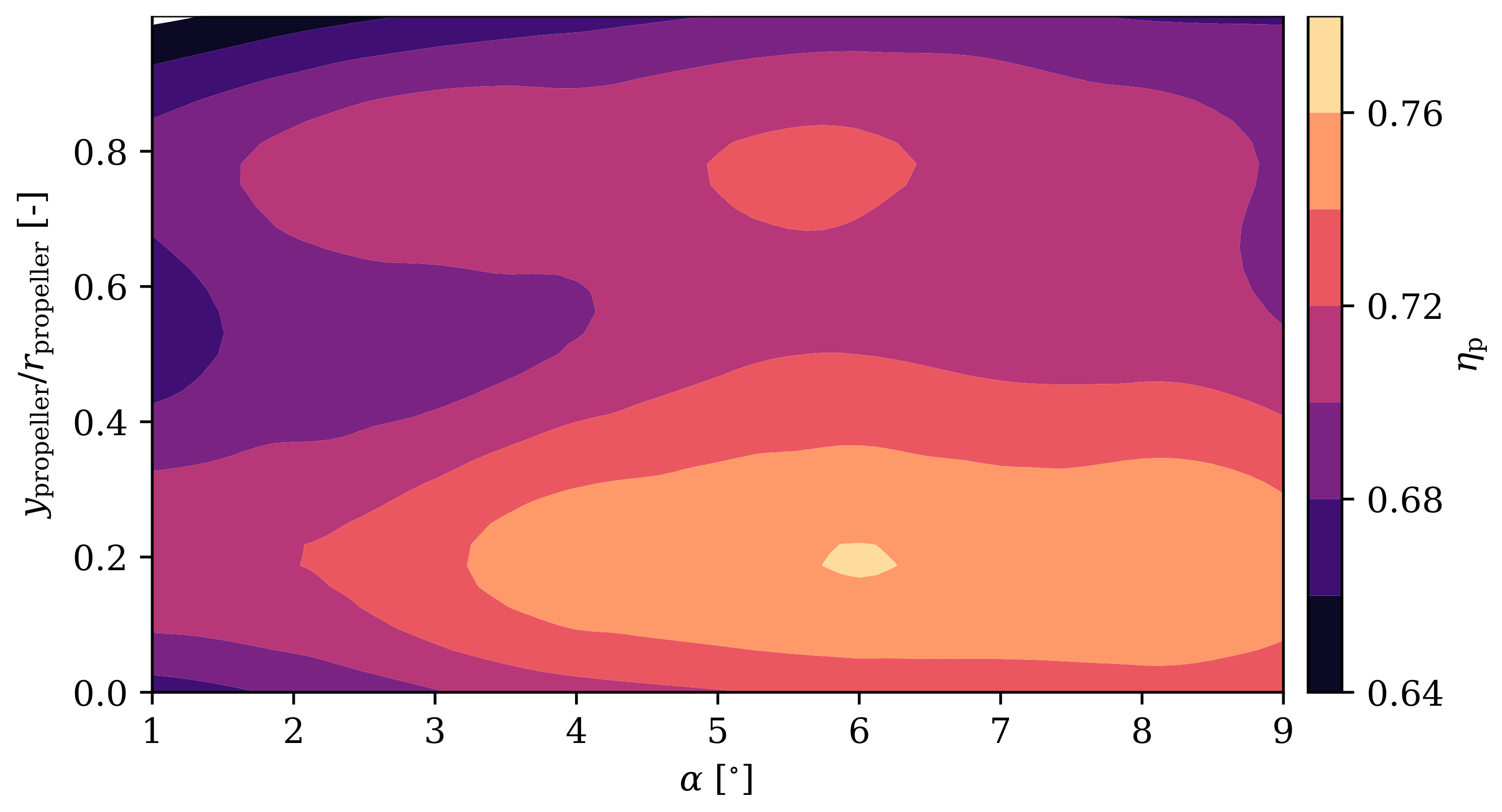
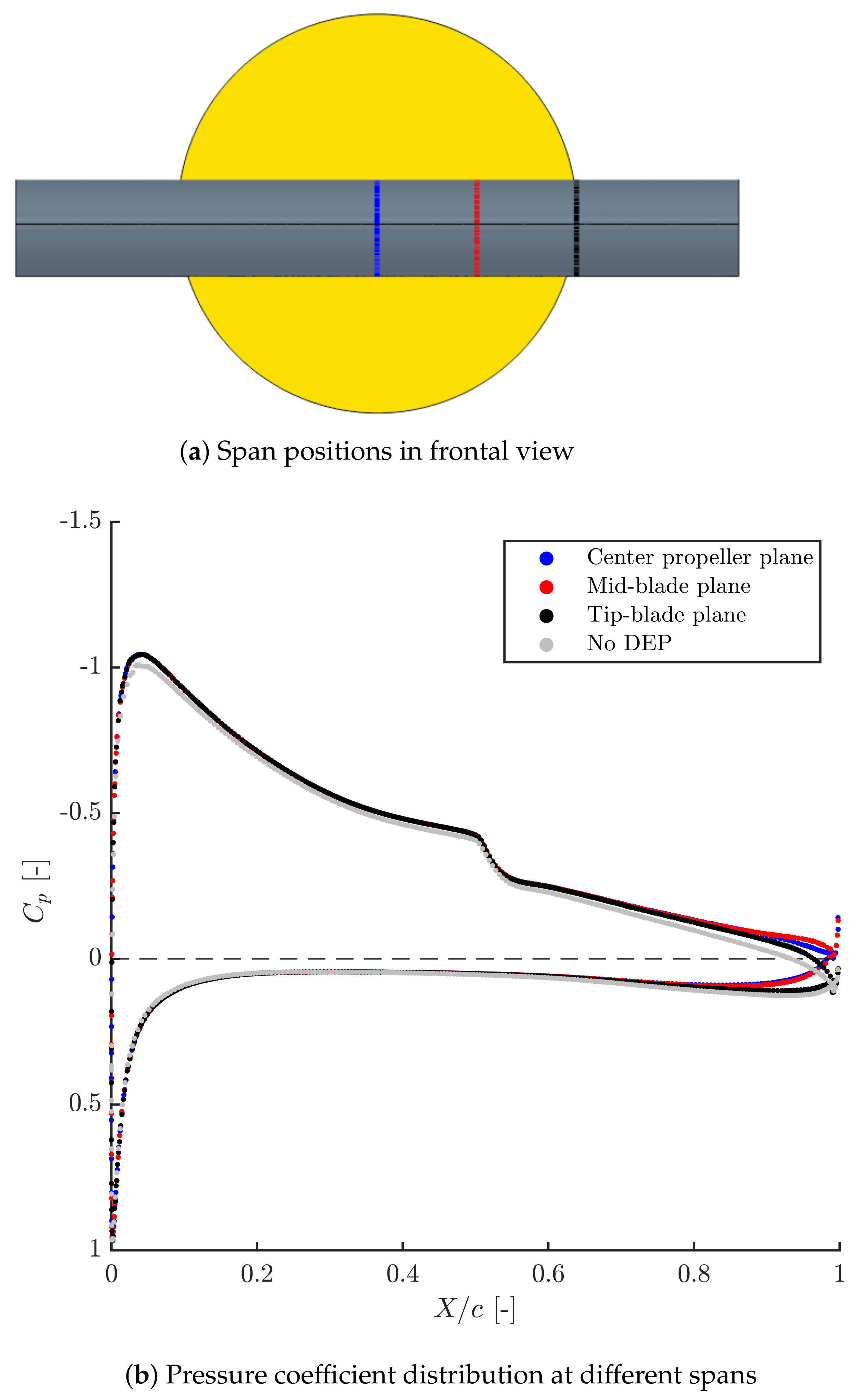
4.1. Propeller Position Cfd Analysis
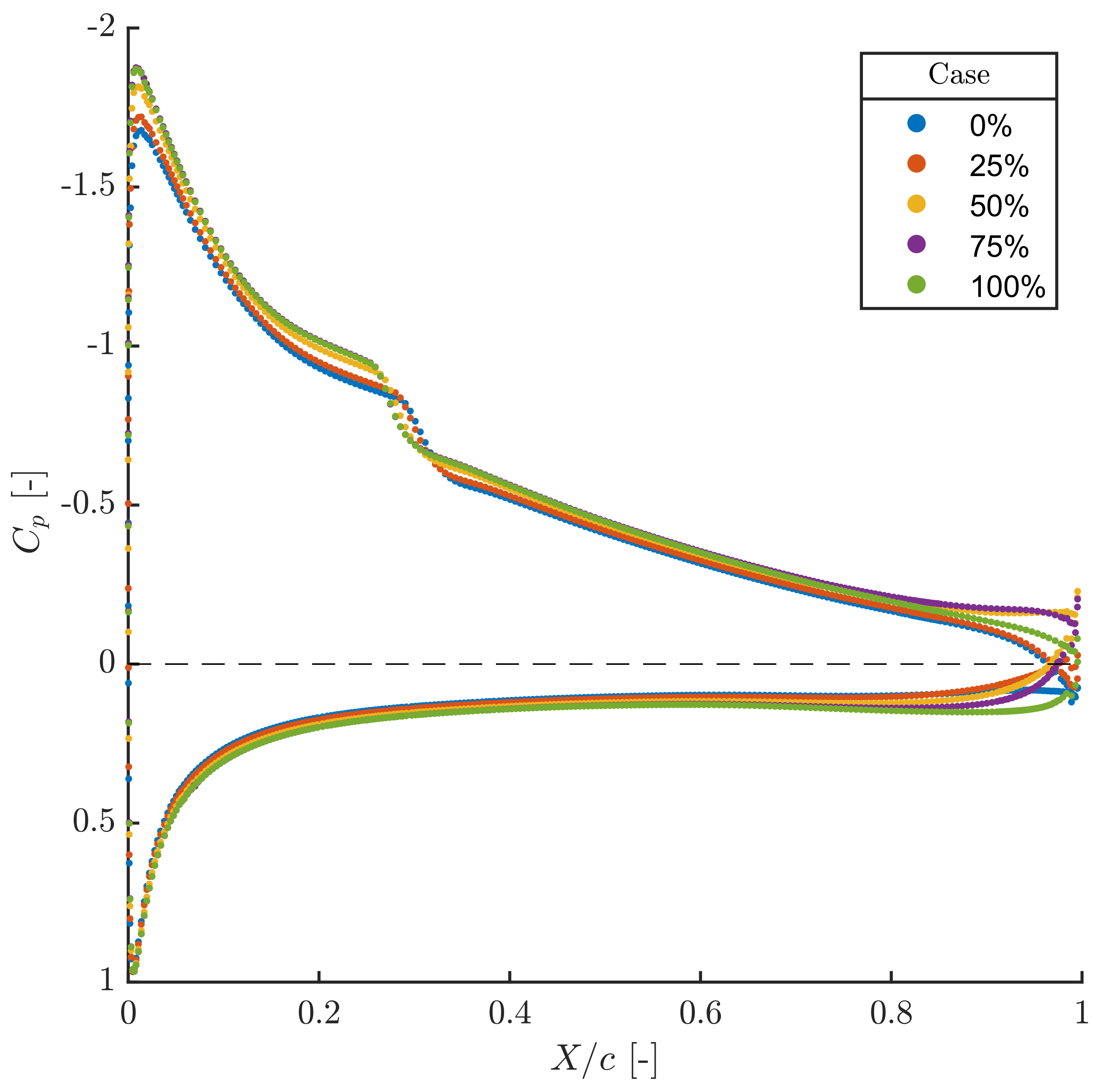
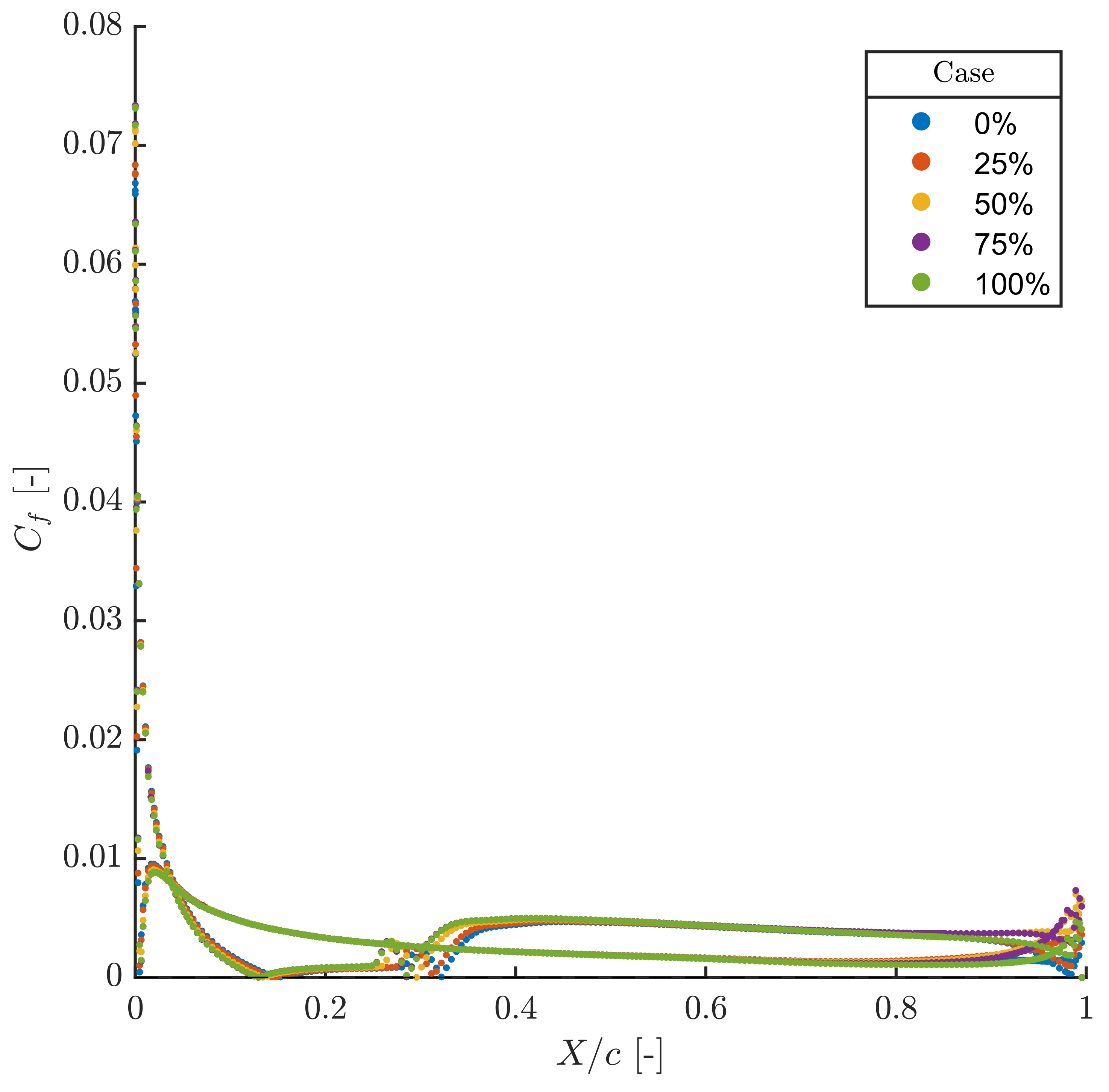
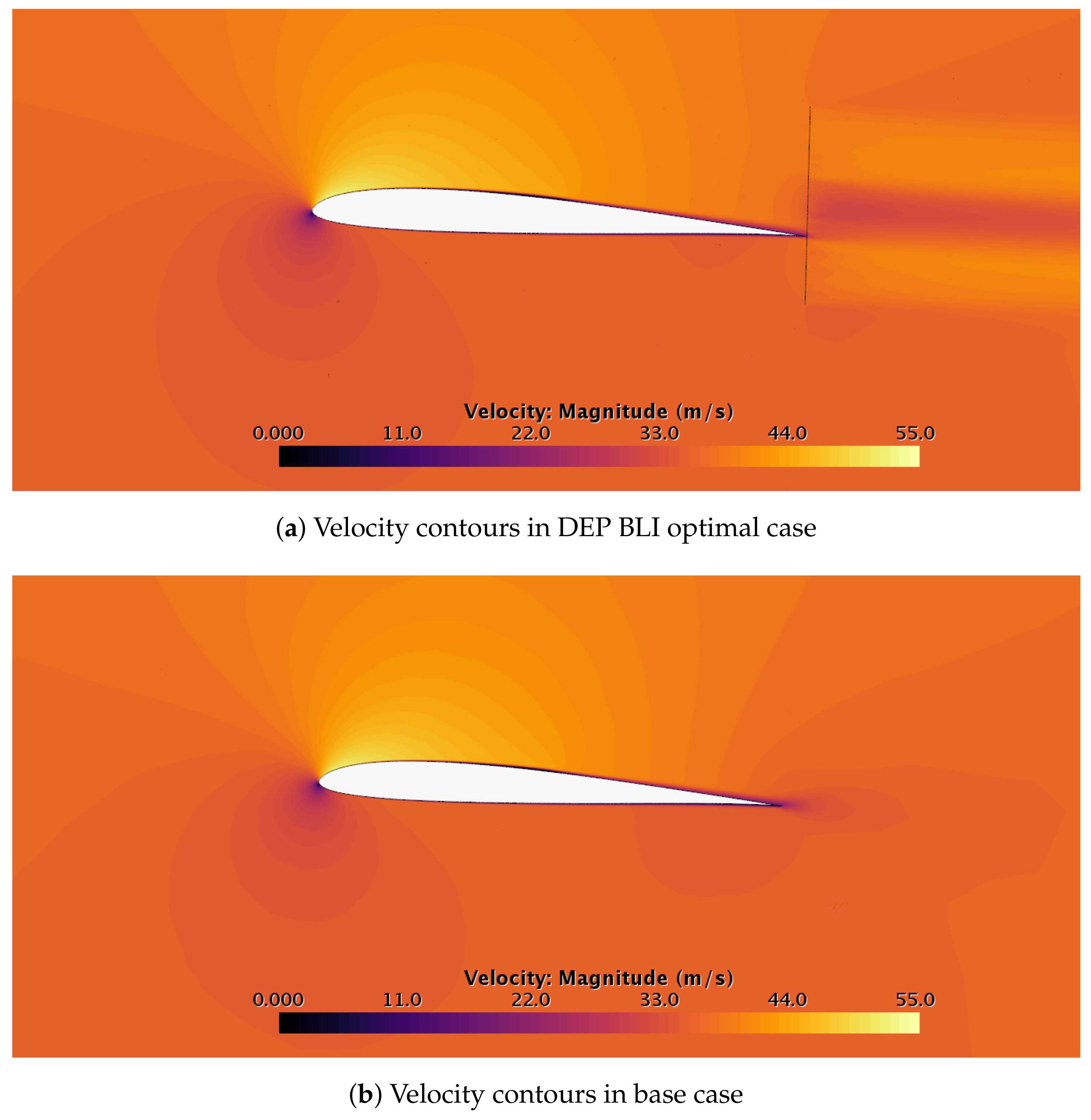
4.2. Best Case Cfd Analysis
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Abbreviations | |
| BLI | Boundary layer ingestion |
| BEMT | Blade Element Model Theory |
| Brake-specific fuel consumption | |
| CFD | Computational fluid dynamics |
| DEP | Distributed electrical propulsion |
| EASA | European Union Aviation Safety Agency |
| ERA | Environmentally Responsible Aviation |
| HE | Hybrid electric |
| ICE | Internal combustion engine |
| ITDS | Information Technology Development Solutions |
| LSB | Laminar separation bubble |
| NASA | National Aeronautics and Space Administration |
| RANS | Reynols-averaged Navier-Stokes |
| RPAS | Remotely piloted aircraft system |
| SESAR | Single European Sky ATM Research |
| TE | Trailing edge |
| UAV | Unmanned aerial vehicle |
| Roman letters | |
| Aspect ratio | |
| b | Wingspan |
| c | Chord |
| Lift coefficient | |
| Drag coefficient | |
| Parasitic drag coefficient of the aircraft without the wing | |
| Parasitic drag coefficient of the wing | |
| Pressure coefficient | |
| Friction coefficient | |
| D | Drag |
| e | Oswald efficiency factor |
| J | Advance ratio |
| n | Rotational speed |
| P | Power |
| Propeller radius | |
| Reynolds | |
| S | Wing surface |
| T | Thrust |
| Air speed | |
| X | Position across the chord |
| Position of the propeller shaft above the trailing edge | |
| Greek letters | |
| Angle of attack | |
| Propulsion efficiency | |
| Density | |
References
- EASA Agency. Study on the Societal Acceptance of Urban Air Mobility in Europe. 2021. Available online: https://www.easa.europa.eu/sites/default/files/dfu/uam-full-report.pdf (accessed on 18 June 2021).
- Single European Sky ATM Research. European Drones Outlook Study. 2016. Available online: http://www.sesarju.eu/sites/default/files/documents/reports/European_Drones_Outlook_Study_2016.pdf (accessed on 10 March 2021).
- Nickol, C.L.; Haller, W.J. Assessment of the performance potential of advanced subsonic transport concepts for NASA’s environmentally responsible aviation project. In Proceedings of the 54th AIAA Aerospace Sciences Meeting, San Diego, CA, USA, 4–8 January 2016; pp. 1–21. [Google Scholar] [CrossRef] [Green Version]
- Ausserer, J.K.; Harmon, F.G. Integration, validation, and testing of a hybrid-electric propulsion system for a small remotely-piloted aircraft. In Proceedings of the 10th Annual International Energy Conversion Engineering Conference, IECEC 2012, Atlanta, GA, USA, 29 July–1 August 2012; pp. 1–11. [Google Scholar] [CrossRef]
- Harmon, F.G.; Frank, A.A.; Chattot, J.J. Conceptual design and simulation of a small hybrid-electric unmanned aerial vehicle. J. Aircr. 2006, 43, 1490–1498. [Google Scholar] [CrossRef]
- Kim, C.; Namgoong, E.; Lee, S.; Kim, T.; Kim, H. Fuel economy optimization for parallel hybrid vehicles with CVT. In SAE Technical Papers; SAE: Warrendale, PA, USA, 1999. [Google Scholar] [CrossRef]
- Stoll, A.M.; Bevirt, J.; Moore, M.D.; Fredericks, W.J.; Borer, N.K. Drag Reduction Through Distributed Electric Propulsion. In Proceedings of the 14th AIAA Aviation Technology, Integration, and Operations Conference, Atlanta, GA, USA, 16–20 June 2014; pp. 22–26. [Google Scholar] [CrossRef] [Green Version]
- Stoll, A.M. Comparison of CFD and experimental results of the leap tech distributed electric propulsion blown wing. In Proceedings of the 15th AIAA Aviation Technology, Integration, and Operations Conference, Dallas, TX, USA, 22–26 June 2015; pp. 22–26. [Google Scholar] [CrossRef] [Green Version]
- Lv, P.; Ragni, D.; Hartuc, T.; Veldhuis, L.; Rao, A.G. Experimental investigation of the flow mechanisms associated with a wake-ingesting propulsor. AIAA J. 2017, 55, 1332–1342. [Google Scholar] [CrossRef]
- Leifsson, L.; Ko, A.; Mason, W.H.; Schetz, J.A.; Grossman, B.; Haftka, R.T. Multidisciplinary design optimization of blended-wing-body transport aircraft with distributed propulsion. Aerosp. Sci. Technol. 2013, 25, 16–28. [Google Scholar] [CrossRef] [Green Version]
- Felder, J.L.; Kim, H.D.; Brown, G.V. Turboelectric distributed propulsion engine cycle analysis for hybrid-wing-body aircraft. In Proceedings of the 47th AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition, Orlando, FL, USA, 5–8 January 2009; pp. 1–25. [Google Scholar] [CrossRef] [Green Version]
- Budziszewski, N.; Friedrichs, J. Modelling of a boundary layer ingesting propulsor. Energies 2018, 11, 708. [Google Scholar] [CrossRef] [Green Version]
- Hall, D.K.; Huang, A.C.; Uranga, A.; Greitzer, E.M.; Drela, M.; Sato, S. Boundary layer ingestion propulsion benefit for transport aircraft. J. Propuls. Power 2017, 33, 1118–1129. [Google Scholar] [CrossRef]
- Teperin, L. Investigation on Boundary Layer Ingestion Propulsion for UAVs. In Proceedings of the International Micro Air Vehicle Conference and Flight Competition (IMAV), Toulouse, France, 18–21 September 2017; pp. 293–300. [Google Scholar]
- Elsalamony, M.; Teperin, L. 2D Numerical Investigation of Boundary Layer Ingestion Propulsor on Airfoil. In Proceedings of the 7th European Conference for Aeronautics and Space Sciences (EUCASS), Milan, Italy, 3–6 July 2017; pp. 1–11. [Google Scholar] [CrossRef]
- Martínez Fernández, A.; Smith, H. Effect of a fuselage boundary layer ingesting propulsor on airframe forces and moments. Aerosp. Sci. Technol. 2020, 100, 105808. [Google Scholar] [CrossRef]
- Goldberg, C.; Nalianda, D.; MacManus, D.; Pilidis, P.; Felder, J. Installed performance assessment of a boundary layer ingesting distributed propulsion system at design point. In Proceedings of the 52nd AIAA/SAE/ASEE Joint Propulsion Conference, Salt Lake City, UT, USA, 25–27 July 2016; Volume 52, pp. 1–22. [Google Scholar] [CrossRef] [Green Version]
- Government of Spain. Real Decreto 1036/2017. In Boletín Oficial del Estado, BOE-A-2017-15721; Agencia Estatal Boletín del Estado: Madrid, Spain, 2017. [Google Scholar]
- UAV Factory USA LLC. Penguin C UAS. Available online: https://www.uavfactory.com (accessed on 10 March 2021).
- AERTEC Solutions. RPAS TARSIS 25. Available online: https://aertecsolutions.com/rpas/rpas-sistema-aereos-tripulados-remotamente/rpas-tarsis25/ (accessed on 27 May 2021).
- Lyon, C.A.; Broeren, A.P.; Giguere, P.; Gopalarathnam, A.; Selig, M.S. Summary of Low-Speed Airfoil Data; SOARTECH Publications: Ann Arbor, MI, USA, 1997; Volume 3, p. 315. [Google Scholar]
- Selig, M.S.; Donovan, J.F.; Fraser, D.B. Airfoils at Low Speeds; H.A. Stokely: Virginia Beach, VA, USA, 1989. [Google Scholar]
- Selig, M.S. Low Reynolds Number Airfoil Design Lecture Notes—Various Approaches to Airfoil Design. In VKI Lecture Series; The von Karman Institute for Fluid Dynamics and NATO-RTO/AVT: Urbana, IL, USA, 2003; pp. 24–28. [Google Scholar]
- Sutton, D.M. Experimental Characterization of the Effects of Freestream Turbulence Intensity on the SD7003 Airfoil at Low Reynolds Numbers. Ph.D. Thesis, University of Toronto, Toronto, ON, Canada, 2015; pp. 1–87. [Google Scholar]
- Hoerner, S. Fluid-Dynamic Drag; Hoerner Fluid Dynamics: Bakersfield, CA, USA, 1965. [Google Scholar]
- Niţă, M.; Scholz, D. Estimating the Oswald Factor from Basic Aircraft Geometrical Parameters. In Deutsche Gesellschaft für Luft-und Raumfahrt-Lilienthal-Oberth eV; Hamburg University Of Applied Sciences: Hamburg, Germany, 2012. [Google Scholar]
- Ananda, G.K.; Sukumar, P.P.; Selig, M.S. Measured aerodynamic characteristics of wings at low Reynolds numbers. Aerosp. Sci. Technol. 2015, 42, 392–406. [Google Scholar] [CrossRef]
- Smith, L.H. Wake ingestion propulsion benefit. J. Propuls. Power 1993, 9, 74–82. [Google Scholar] [CrossRef]
- Blumenthal, B.T.; Elmiligui, A.A.; Geiselhart, K.A.; Campbell, R.L.; Maughmer, M.D.; Schmitz, S. Computational investigation of a boundary-layer-ingestion propulsion system. J. Aircr. 2018, 55, 1141–1153. [Google Scholar] [CrossRef] [PubMed]
- Roache, P.J. Perspective: A Method for Uniform Reporting of Grid Refinement Studies. J. Fluids Eng. 1994, 116, 405–413. [Google Scholar] [CrossRef]
- Gur, O.; Rosen, A. Comparison between blade-element models of propellers. Aeronaut. J. 2008, 112, 689–704. [Google Scholar] [CrossRef]
- Deters, R.W.; Ananda, G.K.; Selig, M.S. Reynolds number effects on the performance of small-scale propellers. In Proceedings of the 32nd AIAA Applied Aerodynamics Conference, Atlanta, GA, USA, 16–20 June 2014; pp. 1–43. [Google Scholar] [CrossRef] [Green Version]
- XFLR5. Available online: http://www.xflr5.tech/xflr5.htm (accessed on 10 March 2021).
- Drela, M. XFOIL Subsonic Airfoil Development System. Available online: https://web.mit.edu/drela/Public/web/xfoil/ (accessed on 10 March 2021).




| Design Parameters | |
|---|---|
| Aspect ratio | 10 |
| Wing area | |
| Wingspan | 2 |
| Wing chord | |
| Maximum takeoff mass | 25 |
| Aerodynamic parameters | |
| (fuselage, empennage, others) | 0.011 |
| Oswald efficiency factor (e) | 0.8 |
| Base case | 0.484 | 0.00769 | 17.280 | 0.692 | 11.963 |
| DEP BLI case | 0.505 | 0.00841 | 17.080 | 0.748 | 12.773 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Serrano, J.R.; Tiseira, A.O.; García-Cuevas, L.M.; Varela, P. Computational Study of the Propeller Position Effects in Wing-Mounted, Distributed Electric Propulsion with Boundary Layer Ingestion in a 25 kg Remotely Piloted Aircraft. Drones 2021, 5, 56. https://doi.org/10.3390/drones5030056
Serrano JR, Tiseira AO, García-Cuevas LM, Varela P. Computational Study of the Propeller Position Effects in Wing-Mounted, Distributed Electric Propulsion with Boundary Layer Ingestion in a 25 kg Remotely Piloted Aircraft. Drones. 2021; 5(3):56. https://doi.org/10.3390/drones5030056
Chicago/Turabian StyleSerrano, José Ramón, Andrés Omar Tiseira, Luis Miguel García-Cuevas, and Pau Varela. 2021. "Computational Study of the Propeller Position Effects in Wing-Mounted, Distributed Electric Propulsion with Boundary Layer Ingestion in a 25 kg Remotely Piloted Aircraft" Drones 5, no. 3: 56. https://doi.org/10.3390/drones5030056
APA StyleSerrano, J. R., Tiseira, A. O., García-Cuevas, L. M., & Varela, P. (2021). Computational Study of the Propeller Position Effects in Wing-Mounted, Distributed Electric Propulsion with Boundary Layer Ingestion in a 25 kg Remotely Piloted Aircraft. Drones, 5(3), 56. https://doi.org/10.3390/drones5030056








