Author Contributions
Conceptualisation, V.S. and A.T.; methodology, A.T. and P.J.; validation, A.T. and P.J.; formal analysis, P.J.; investigation, P.J.; resources, A.T. and M.V.; data curation, P.J.; writing—original draft preparation, P.J.; writing—review and editing, A.T., V.S., M.V. and P.J.; visualisation, P.J.; supervision, A.T.; project administration, A.T. and M.V.; funding acquisition, A.T. and V.S. All authors have read and agreed to the published version of the manuscript.
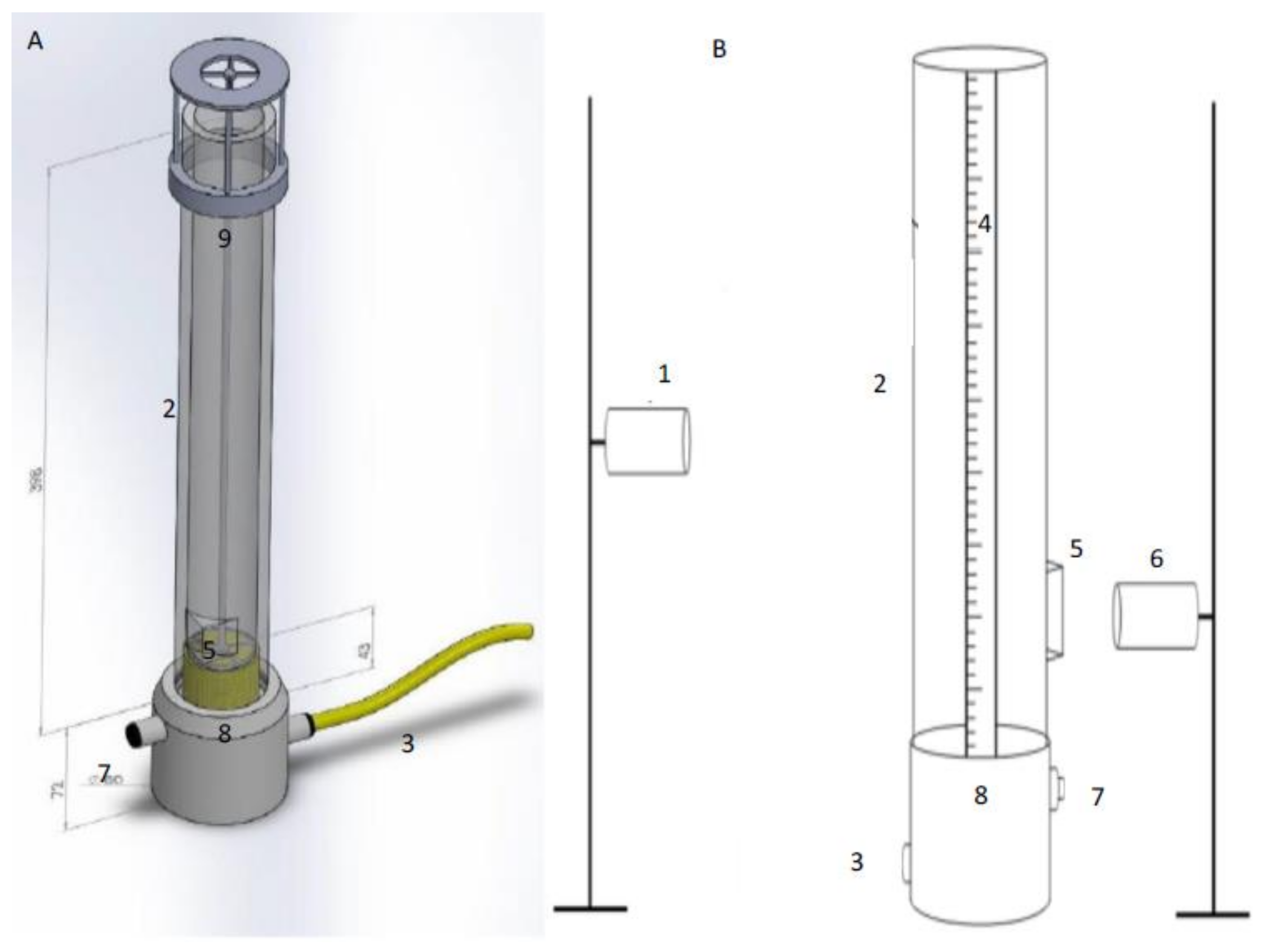
Figure 1.
Drawing of the foam column used in the experiments. (A) shows a depiction of how the column will look when porous media is used instead of capillaries; a metal holder has to be present to prevent unwanted ejection of the media from the column, due to pressure build-up beneath the media. (B) shows a schematic of the experimental setup.
Figure 1.
Drawing of the foam column used in the experiments. (A) shows a depiction of how the column will look when porous media is used instead of capillaries; a metal holder has to be present to prevent unwanted ejection of the media from the column, due to pressure build-up beneath the media. (B) shows a schematic of the experimental setup.
Figure 2.
The six capillary substrates considered for the project to model incompressible porous substrates of varying pore size and pore arrangements. 1. shows 0.1 mm small arrangement of capillaries, 2. shows 0.25 mm small arrangement of capillaries, 3. shows 0.88 mm small arrangement of capillaries, 4. shows 0.1 mm large arrangement of capillaries, 5. shows 0.25 mm large arrangement of capillaries, and 6. shows 0.88 mm large arrangement of capillaries.
Figure 2.
The six capillary substrates considered for the project to model incompressible porous substrates of varying pore size and pore arrangements. 1. shows 0.1 mm small arrangement of capillaries, 2. shows 0.25 mm small arrangement of capillaries, 3. shows 0.88 mm small arrangement of capillaries, 4. shows 0.1 mm large arrangement of capillaries, 5. shows 0.25 mm large arrangement of capillaries, and 6. shows 0.88 mm large arrangement of capillaries.
Figure 3.
The bubble diameter of 118 bubbles along with the cumulative average, indicating that the minimum sample size that can be used is 50 bubbles.
Figure 3.
The bubble diameter of 118 bubbles along with the cumulative average, indicating that the minimum sample size that can be used is 50 bubbles.
Figure 4.
Schematic diagram of the homemade dynamic foam analyser, where the first ohm-meter is positioned close to the bottom and the other electrodes are positioned at 10 cm, 17.5 cm, and 32.5 cm above this point. 1. is the acrylic column, 2. scale, 3. electrodes which are located on each side of the column to allow the resistance and hence the conductivity across the foam to measured, 4. ohmmeter used to obtain the resistance across the foam, 5. gas inlet, and 6. liquid outlet which is plugged during the experiment and is used to allow the easy drainage of the liquid out of the column.
Figure 4.
Schematic diagram of the homemade dynamic foam analyser, where the first ohm-meter is positioned close to the bottom and the other electrodes are positioned at 10 cm, 17.5 cm, and 32.5 cm above this point. 1. is the acrylic column, 2. scale, 3. electrodes which are located on each side of the column to allow the resistance and hence the conductivity across the foam to measured, 4. ohmmeter used to obtain the resistance across the foam, 5. gas inlet, and 6. liquid outlet which is plugged during the experiment and is used to allow the easy drainage of the liquid out of the column.
Figure 5.
The average bubble diameters for each substrate and surfactant type for concentrations 0.5–50 CMC, where SF stands for small formation and LF stands for large formation.
Figure 5.
The average bubble diameters for each substrate and surfactant type for concentrations 0.5–50 CMC, where SF stands for small formation and LF stands for large formation.
Figure 6.
Comparison of average bubble diameter under different conditions at concentrations: 0.5, 1, 10, 20, and 50 critical micelle concentration (CMC).
Figure 6.
Comparison of average bubble diameter under different conditions at concentrations: 0.5, 1, 10, 20, and 50 critical micelle concentration (CMC).
Figure 7.
Foam produced by sodium dodecyl sulphate (SDS) solutions diluted by hard water at 20 CMC using (a) capillaries and (b) dishwasher sponge.
Figure 7.
Foam produced by sodium dodecyl sulphate (SDS) solutions diluted by hard water at 20 CMC using (a) capillaries and (b) dishwasher sponge.
Figure 8.
Rate of foam drainage on capillaries (cap), capillaries, and dishwasher sponge system and foam formed by dishwasher sponge. (a) SDS solutions with hard water at all five concentrations and (b) Tween-20 solutions at 0.5 and 50 CMC.
Figure 8.
Rate of foam drainage on capillaries (cap), capillaries, and dishwasher sponge system and foam formed by dishwasher sponge. (a) SDS solutions with hard water at all five concentrations and (b) Tween-20 solutions at 0.5 and 50 CMC.
Figure 9.
Examples of splitting caused by coalescing at (a) 1 CMC SDS diluted with distilled water with capillaries and sponge and (b) 10 CMC SDS diluted with distilled water with capillaries. The splitting is indicated by the arrow showing the points where the foam has separated.
Figure 9.
Examples of splitting caused by coalescing at (a) 1 CMC SDS diluted with distilled water with capillaries and sponge and (b) 10 CMC SDS diluted with distilled water with capillaries. The splitting is indicated by the arrow showing the points where the foam has separated.
Figure 10.
Dependency of liquid volume fraction with time of foam at each height for SDS solutions diluted with distilled water with concentrations (a) 0.5 CMC, (b) 1 CMC, (c) 10 CMC, (d) 20 CMC, and (e) 50 CMC. The fitting is according to Equation (3).
Figure 10.
Dependency of liquid volume fraction with time of foam at each height for SDS solutions diluted with distilled water with concentrations (a) 0.5 CMC, (b) 1 CMC, (c) 10 CMC, (d) 20 CMC, and (e) 50 CMC. The fitting is according to Equation (3).
Figure 11.
Dependency of liquid volume fraction with time of foam at each height for SDS solutions diluted with distilled 15dH hard water with concentrations (a) 0.5 CMC, (b) 1 CMC, (c) 10 CMC, (d) 20 CMC, and (e) 50 CMC. The fitting is according to Equation (3).
Figure 11.
Dependency of liquid volume fraction with time of foam at each height for SDS solutions diluted with distilled 15dH hard water with concentrations (a) 0.5 CMC, (b) 1 CMC, (c) 10 CMC, (d) 20 CMC, and (e) 50 CMC. The fitting is according to Equation (3).
Figure 12.
Average liquid volume fraction for whole foam against time for each concentration of SDS, where (a) is distilled water solutions and (b) is 15 dH hard water solutions. The fitting is according to Equation (3).
Figure 12.
Average liquid volume fraction for whole foam against time for each concentration of SDS, where (a) is distilled water solutions and (b) is 15 dH hard water solutions. The fitting is according to Equation (3).
Table 1.
Properties of sponge samples found using an SEM device.
Table 1.
Properties of sponge samples found using an SEM device.
| Sponge Type | Pore Size (mm) | Porosity |
|---|
| Dishwasher | 0.302 ± 0.072 | 0.689 |
| Audio | 0.093 ± 0.028 | 0.692 |
| Car | 0.295 ± 0.070 | 0.694 |
Table 2.
The salts and amount in grams used to make 2 litres of 15 dH hard water.
Table 2.
The salts and amount in grams used to make 2 litres of 15 dH hard water.
| Salt | Mass (g) |
|---|
| CaCl12∙2H2O | 0.564 |
| MgCl2∙6H2O | 0.300 |
| NaHCO3 | 0.276 |
Table 3.
Time surpassed for the initial liquid level to be reached for each experimental parameter.
Table 3.
Time surpassed for the initial liquid level to be reached for each experimental parameter.
| | Time to Reach Final Liquid Level (min) |
|---|
| SDS (Distilled Water) | SDS (Hard Water) | Tween-20 |
|---|
| Conc (CMC) | Capillaries | Cap & Sponge | Sponge | Capillaries | Cap & Sponge | Sponge | Capillaries | Cap & Sponge | Sponge |
|---|
| 0.5 | 6 | 0.3 | 6 | 5 | 10 | 8 | 0.3 | 0.1 | 0.1 |
| 1 | 8 | 2 | 14 | 7 | 11 | 15 | 0.1 | 0.1 | 0.3 |
| 10 | 3 | 17 | 10 | 9 | 13 | 26 | 0.5 | 0.3 | 0.7 |
| 20 | 7 | 17 | 14 | 9 | 14 | 28 | 2 | 0.3 | 8 |
| 50 | 5 | 17 | 10 | 10 | 20 | 30 | 2 | 0.3 | 8 |
Table 4.
The characteristic time scale obtained using Equation (4) for foams produced with SDS solutions with distilled water for each of the heights investigated.
Table 4.
The characteristic time scale obtained using Equation (4) for foams produced with SDS solutions with distilled water for each of the heights investigated.
| Concentration (CMC) | Height (cm) | Alpha (1/min) | 1/Alpha (min) | 1/Alpha (s) |
|---|
| 50 | 10 | 0.1736 | 5.7604 | 346 |
| 17.5 | 0.2175 | 4.5977 | 276 |
| 32.5 | 0.2255 | 4.4346 | 266 |
| 20 | 10 | 0.164 | 6.0976 | 366 |
| 17.5 | 0.2242 | 4.4603 | 268 |
| 32.5 | 0.2069 | 4.8333 | 290 |
| 10 | 10 | 0.2 | 5.0000 | 300 |
| 17.5 | 0.1741 | 5.7438 | 345 |
| 32.5 | 0.3505 | 2.8531 | 171 |
| 1 | 10 | 0.3811 | 2.6240 | 157 |
| 17.5 | 0.5632 | 1.7756 | 107 |
| 32.5 | 0.262 | 3.8168 | 229 |
| 0.5 | 10 | 0.5737 | 1.7431 | 105 |
| 17.5 | 0.4816 | 2.0764 | 125 |
| 32.5 | 0.2908 | 3.4388 | 206 |
Table 5.
The characteristic time scale obtained using Equation (4) for foams produced with SDS solutions with 15 dH hard water for each of the heights investigated.
Table 5.
The characteristic time scale obtained using Equation (4) for foams produced with SDS solutions with 15 dH hard water for each of the heights investigated.
| Concentration (CMC) | Height (cm) | Alpha (1/min) | 1/Alpha (min) | 1/Alpha (s) |
|---|
| 50 | 10 | 0.0842 | 11.8765 | 713 |
| 17.5 | 0.0854 | 11.7096 | 703 |
| 32.5 | 0.1436 | 6.9638 | 418 |
| 20 | 10 | 0.1864 | 5.3648 | 322 |
| 17.5 | 0.1804 | 5.5432 | 333 |
| 32.5 | 0.1864 | 5.3648 | 322 |
| 10 | 10 | 0.2605 | 3.8388 | 230 |
| 17.5 | 0.1759 | 5.6850 | 341 |
| 32.5 | 0.2963 | 3.3750 | 202 |
| 1 | 10 | 0.3302 | 3.0285 | 182 |
| 17.5 | 0.3603 | 2.7755 | 167 |
| 32.5 | 0.2714 | 3.6846 | 221 |
| 0.5 | 10 | 0.2634 | 3.7965 | 228 |
| 17.5 | 0.313 | 3.1949 | 192 |
| 32.5 | 0.2632 | 3.7994 | 228 |
Table 6.
The characteristic time scale obtained using Equation (4) for foams produced with SDS solutions with distilled water.
Table 6.
The characteristic time scale obtained using Equation (4) for foams produced with SDS solutions with distilled water.
| Concentration (CMC) | Alpha (1/min) | 1/Alpha (min) | 1/Alpha (s) |
|---|
| 50 | 0.2175 | 4.5977 | 276 |
| 20 | 0.2024 | 4.9407 | 296 |
| 10 | 0.2165 | 4.6189 | 277 |
| 1 | 0.3329 | 3.0039 | 180 |
| 0.5 | 0.25 | 4.0000 | 240 |
Table 7.
The characteristic time scale obtained using Equation (4) for foams produced using SDS solutions with 15 dH hard water.
Table 7.
The characteristic time scale obtained using Equation (4) for foams produced using SDS solutions with 15 dH hard water.
| Concentration (CMC) | Alpha (1/min) | 1/Alpha (min) | 1/Alpha (s) |
|---|
| 50 | 0.1027 | 9.7371 | 584 |
| 20 | 0.1879 | 5.3220 | 319 |
| 10 | 0.2069 | 4.8333 | 290 |
| 1 | 0.3302 | 3.0285 | 182 |
| 0.5 | 0.2516 | 3.9746 | 238 |