Simulation and Experimental Analysis of Microalgae and Membrane Surface Interaction
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Microalgae Cultivation
2.3. Characterization of Microalgae
2.4. Atomic Force Microscope (AFM) Analysis
2.5. Contact Angle of the Algae-Coated Surface
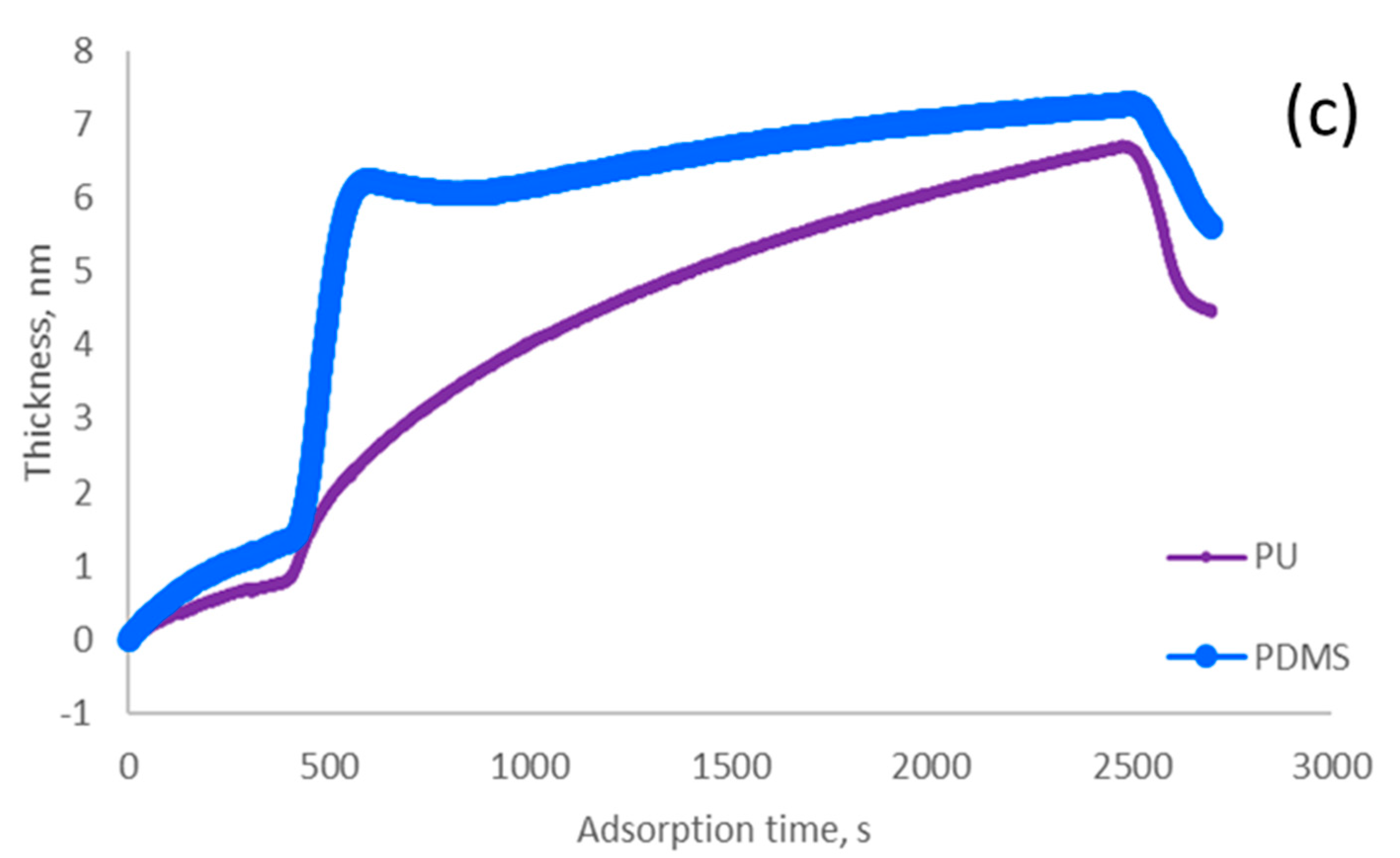
2.6. Adsorption of Algae on the Sensor Surface
2.7. Simulation of Circular Microalgae Interacted with Planar Membrane
2.8. Assessment of Interaction Energy
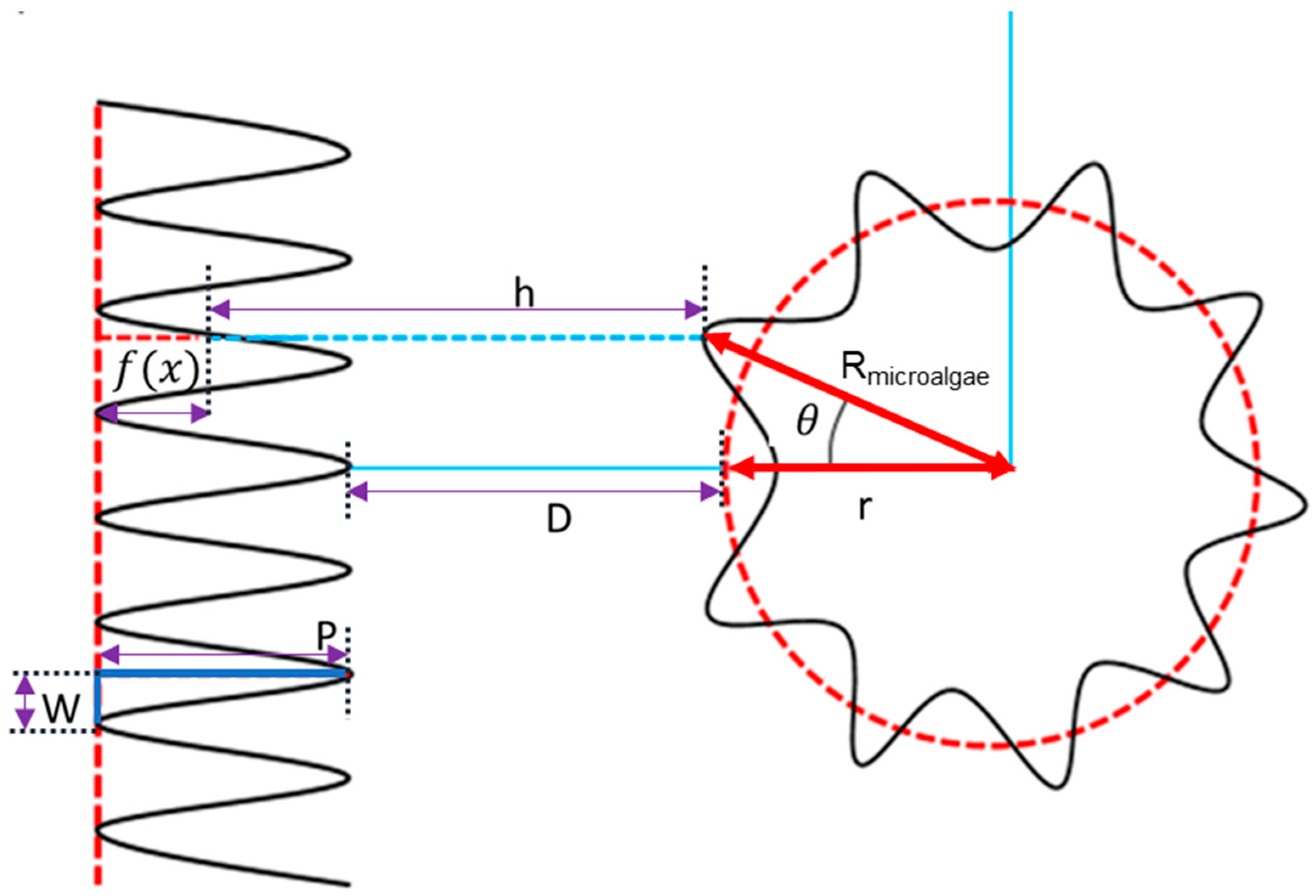
2.9. Asperity Frequency
2.10. Asperity Amplitude
2.11. Particle Radius
2.12. Asperity Height
2.13. Asperity Width
3. Results and Discussion
3.1. Microalgae Cell Characterization
3.2. Contact Angle and Surface Tension of Algae-Coated Surface
3.3. Surface Roughness Characterization
3.4. Adsorption of Algae on Surface
3.5. Modeling Analysis
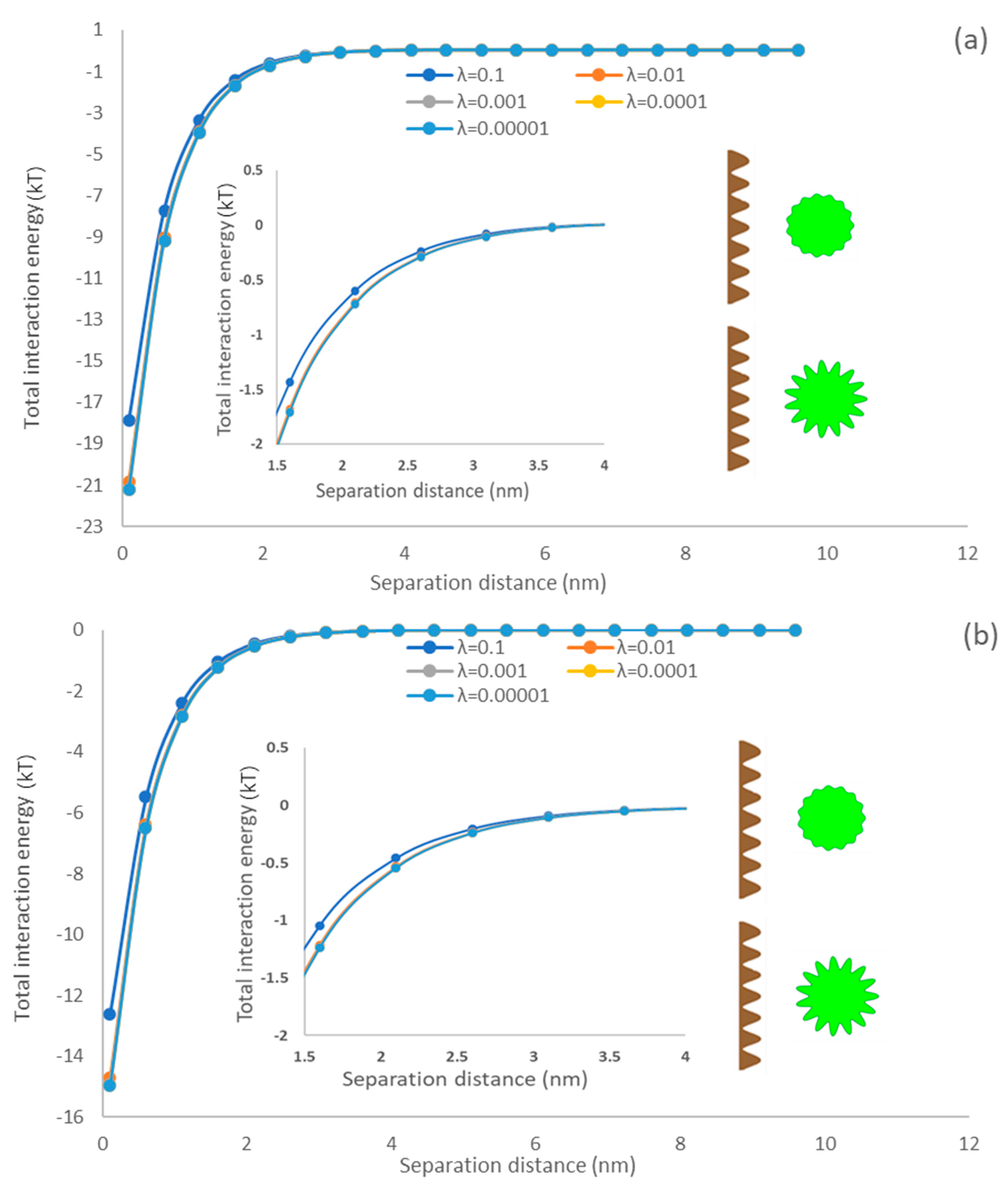
3.5.1. Particle Size Effect
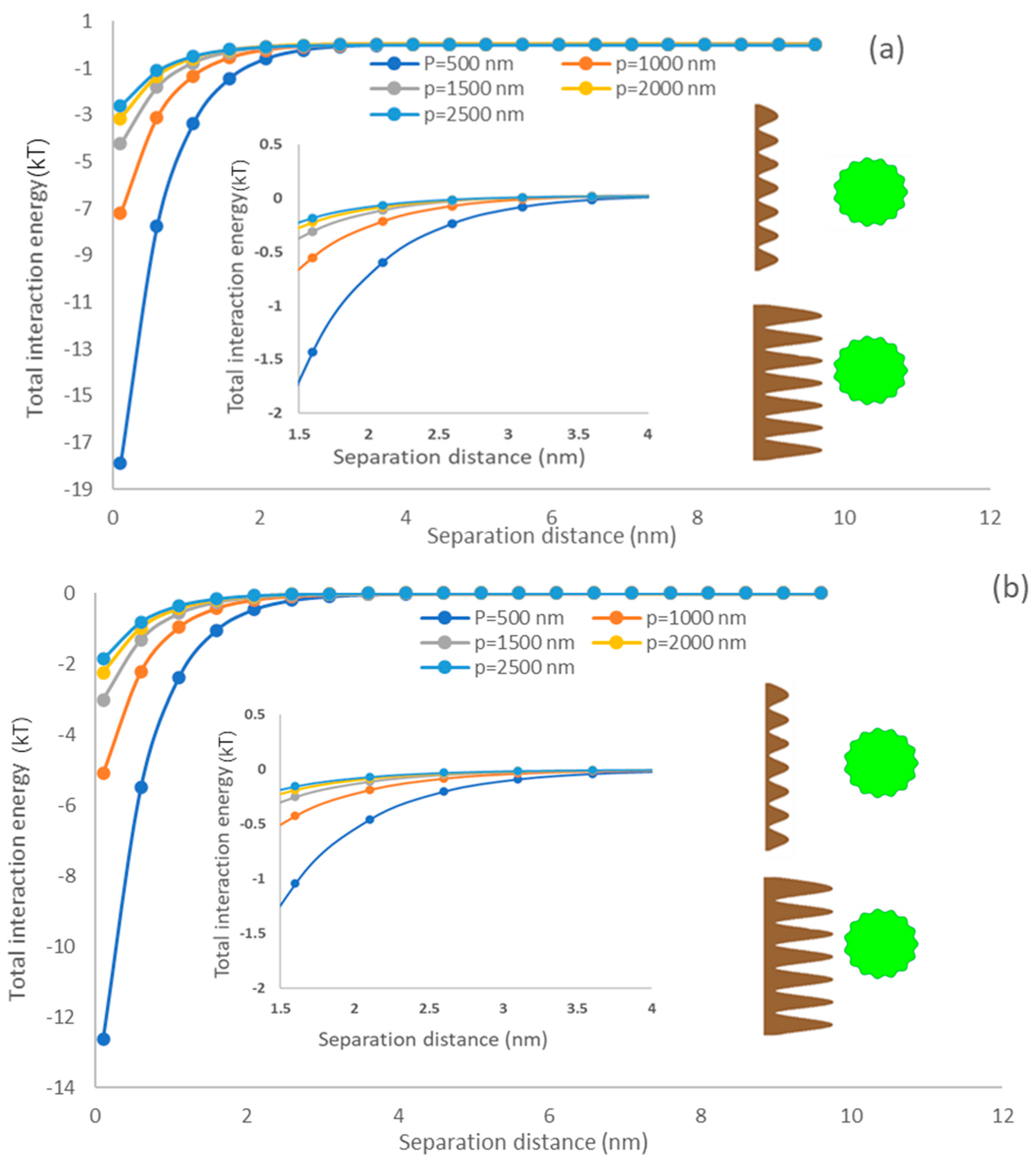
3.5.2. Asperity Frequency Effects
3.5.3. Asperity Amplitude Effects
3.5.4. Asperity Height of the Membrane
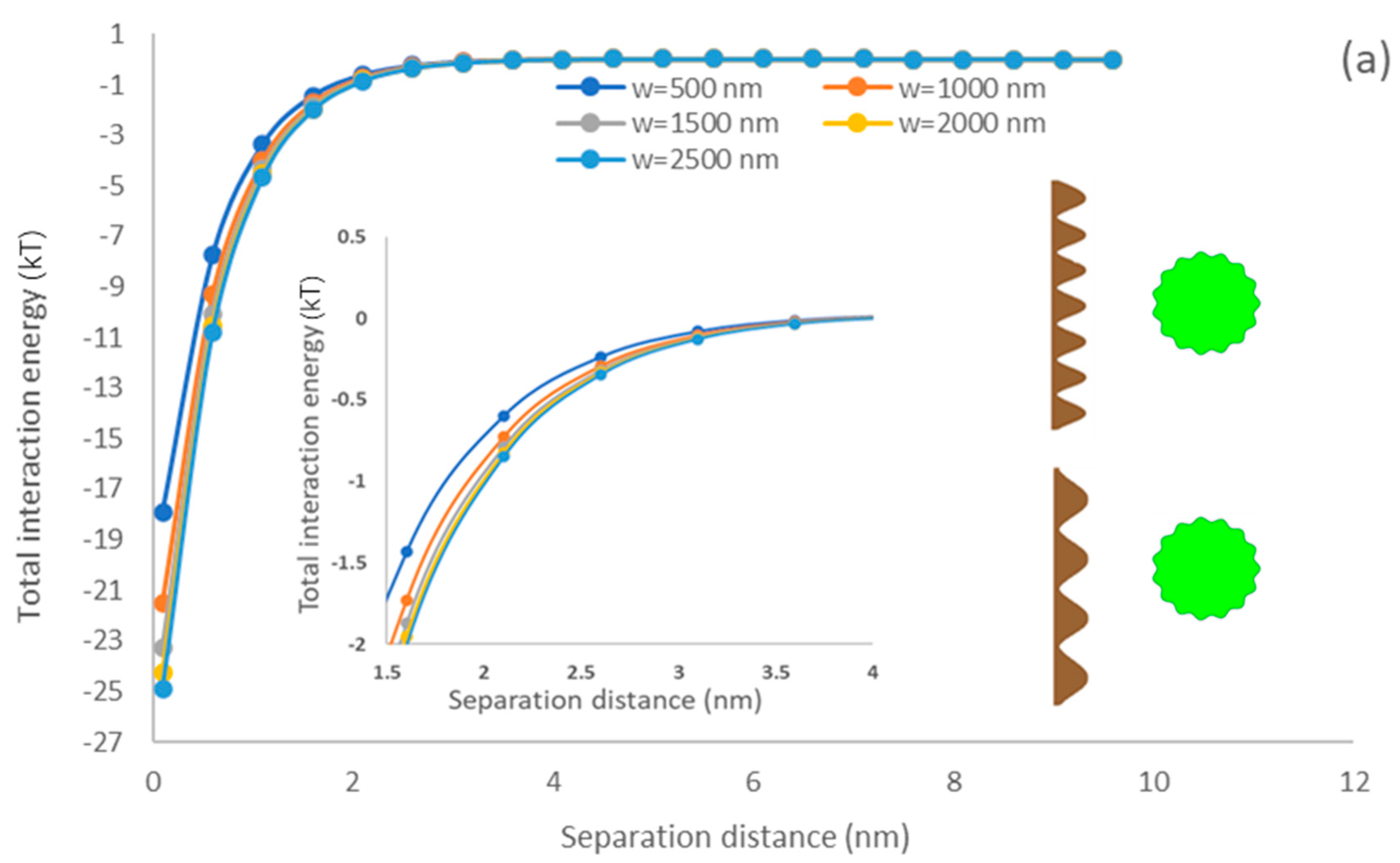
3.5.5. Membrane Asperity Width
3.5.6. Identifying the Most Influential Parameter in Interfacial Interactions
3.5.7. Modeling Validation
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| AH | |
| D | the closest distance between the two-particle surface (nm) |
| the separation distance between two planar surfaces (nm) | |
| interaction energy per unit area (mJ/m2) | |
| the radius of element (smooth) ellipsoidal particle (nm) | |
| asperity ratio | |
| asperity number | |
| asperity height | |
| asperity width | |
| the radius of rough ellipsoidal particle (nm) | |
| the interaction energy between the membrane surface and particle (kT) | |
| surface tension parameter (mJ/m2) | |
| the permittivity of the suspending liquid (C/Vm) | |
| ζ | zeta potential (mV) |
| reciprocal Debye screening length (1/nm) | |
| angle coordinate in the spherical coordinate system | |
| decay length of AB interactions in water (0.6 nm) | |
| minimum equilibrium cut-off distance (0.158 nm) | |
| Superscripts | |
| AB | Lewis acid–base |
| EL | electrostatic double layer |
| LW | Lifshitz-van der Waals |
| Total | total |
| + | electron acceptor |
| − | electron donor |
| Subscripts | |
| liquid | |
| water | |
| microalgae surface | |
| membrane surface | |
| describing particle one and two (i = 1, 2) | |
References
- Wollmann, F.; Dietze, S.; Ackermann, J.U.; Bley, T.; Walther, T.; Steingroewer, J.; Krujatz, F. Microalgae wastewater treatment: Biological and technological approaches. Eng. Life Sci. 2019, 19, 860–871. [Google Scholar] [CrossRef]
- Muñoz, I.; Gómez-Ramos, M.J.; Agüera, A.; Fernández-Alba, A.R.; García-Reyes, J.F.; Molina-Díaz, A. Chemical evaluation of contaminants in wastewater effluents and the environmental risk of reusing effluents in agriculture. TrAC Trends Anal. Chem. 2009, 28, 676–694. [Google Scholar] [CrossRef]
- Sousa, J.C.; Ribeiro, A.R.; Barbosa, M.O.; Pereira, M.F.R.; Silva, A.M. A review on environmental monitoring of water organic pollutants identified by EU guidelines. J. Hazard. Mater. 2018, 344, 146–162. [Google Scholar] [CrossRef]
- Abinandan, S.; Perera, I.A.; Subashchandrabose, S.R.; Venkateswarlu, K.; Cole, N.; Megharaj, M. Acid-adapted microalgae exhibit phenotypic changes for their survival in acid mine drainage samples. FEMS Microbiol. Ecol. 2020, 96, fiaa113. [Google Scholar] [CrossRef]
- Mata, T.M.; Martins, A.A.; Caetano, N.S. Microalgae for biodiesel production and other applications: A review. Renew. Sustain. Energy Rev. 2010, 14, 217–232. [Google Scholar] [CrossRef]
- Spolaore, P.; Joannis-Cassan, C.; Duran, E.; Isambert, A. Commercial applications of microalgae. J. Biosci. Bioeng. 2006, 101, 87–96. [Google Scholar] [CrossRef]
- Tang, J.; Liu, B.; Gao, L.; Wang, W.; Liu, T.; Su, G. Impacts of surface wettability and roughness of styrene-acrylic resin films on adhesion behavior of microalgae Chlorella sp. Colloids Surf. B Biointerfaces 2021, 199, 111522. [Google Scholar] [CrossRef]
- Hu, J.; Nagarajan, D.; Zhang, Q.; Chang, J.-S.; Lee, D.-J. Heterotrophic cultivation of microalgae for pigment production: A review. Biotechnol. Adv. 2018, 36, 54–67. [Google Scholar] [CrossRef] [PubMed]
- Subashchandrabose, S.R.; Ramakrishnan, B.; Megharaj, M.; Venkateswarlu, K.; Naidu, R. Mixotrophic cyanobacteria and microalgae as distinctive biological agents for organic pollutant degradation. Environ. Int. 2013, 51, 59–72. [Google Scholar] [CrossRef] [PubMed]
- Ríos, S.D.; Salvadó, J.; Farriol, X.; Torras, C. Antifouling microfiltration strategies to harvest microalgae for biofuel. Bioresour. Technol. 2012, 119, 406–418. [Google Scholar] [CrossRef] [PubMed]
- Hu, W.; Yin, J.; Deng, B.; Hu, Z. Application of nano TiO2 modified hollow fiber membranes in algal membrane bioreactors for high-density algae cultivation and wastewater polishing. Bioresour. Technol. 2015, 193, 135–141. [Google Scholar] [CrossRef] [PubMed]
- Drexler, I.L.; Yeh, D.H. Membrane applications for microalgae cultivation and harvesting: A review. Rev. Environ. Sci. Bio/Technol. 2014, 13, 487–504. [Google Scholar] [CrossRef]
- Bilad, M.; Arafat, H.A.; Vankelecom, I.F. Membrane technology in microalgae cultivation and harvesting: A review. Biotechnol. Adv. 2014, 32, 1283–1300. [Google Scholar] [CrossRef]
- Ting, H.; Haifeng, L.; Shanshan, M.; Zhang, Y.; Zhidan, L.; Na, D. Progress in microalgae cultivation photobioreactors and applications in wastewater treatment: A review. Int. J. Agric. Biol. Eng. 2017, 10, 1–29. [Google Scholar]
- Carbone, D.A.; Gargano, I.; Pinto, G.; De Natale, A.; Pollio, A. Evaluating microalgae attachment to surfaces: A first approach towards a laboratory integrated assessment. Chem. Eng. Trans 2017, 57, 73–78. [Google Scholar]
- Gao, F.; Yang, Z.-H.; Li, C.; Zeng, G.-M.; Ma, D.-H.; Zhou, L. A novel algal biofilm membrane photobioreactor for attached microalgae growth and nutrients removal from secondary effluent. Bioresour. Technol. 2015, 179, 8–12. [Google Scholar] [CrossRef]
- Zhao, Z.; Li, Y.; Muylaert, K.; Vankelecom, I.F. Synergy between membrane filtration and flocculation for harvesting microalgae. Sep. Purif. Technol. 2020, 240, 116603. [Google Scholar] [CrossRef]
- Gao, F.; Peng, Y.-Y.; Li, C.; Cui, W.; Yang, Z.-H.; Zeng, G.-M. Coupled nutrient removal from secondary effluent and algal biomass production in membrane photobioreactor (MPBR): Effect of HRT and long-term operation. Chem. Eng. J. 2018, 335, 169–175. [Google Scholar] [CrossRef]
- Liao, Y.; Bokhary, A.; Maleki, E.; Liao, B. A review of membrane fouling and its control in algal-related membrane processes. Bioresour. Technol. 2018, 264, 343–358. [Google Scholar] [CrossRef]
- Sanaei, A.; Tavassoli, S.; Sepehrnoori, K. Investigation of modified Water chemistry for improved oil recovery: Application of DLVO theory and surface complexation model. Colloids Surf. A Physicochem. Eng. Asp. 2019, 574, 131–145. [Google Scholar] [CrossRef]
- Duffadar, R.; Kalasin, S.; Davis, J.M.; Santore, M.M. The impact of nanoscale chemical features on micron-scale adhesion: Crossover from heterogeneity-dominated to mean-field behavior. J. Colloid Interface Sci. 2009, 337, 396–407. [Google Scholar] [CrossRef] [PubMed]
- Duffadar, R.D.; Davis, J.M. Interaction of micrometer-scale particles with nanotextured surfaces in shear flow. J. Colloid Interface Sci. 2007, 308, 20–29. [Google Scholar] [CrossRef] [PubMed]
- Duffadar, R.D.; Davis, J.M. Dynamic adhesion behavior of micrometer-scale particles flowing over patchy surfaces with nanoscale electrostatic heterogeneity. J. Colloid Interface Sci. 2008, 326, 18–27. [Google Scholar] [CrossRef]
- Chen, J.Y.; Ko, C.-H.; Bhattacharjee, S.; Elimelech, M. Role of spatial distribution of porous medium surface charge heterogeneity in colloid transport. Colloids Surf. A Physicochem. Eng. Asp. 2001, 191, 3–15. [Google Scholar] [CrossRef]
- Hassas, B.V.; Caliskan, H.; Guven, O.; Karakas, F.; Cinar, M.; Celik, M.S. Effect of roughness and shape factor on flotation characteristics of glass beads. Colloids Surf. A Physicochem. Eng. Asp. 2016, 492, 88–99. [Google Scholar] [CrossRef]
- Bendersky, M.; Davis, J. DLVO interaction of colloidal particles with topographically and chemically heterogeneous surfaces. J. Colloid Interface Sci. 2011, 353, 87–97. [Google Scholar] [CrossRef] [PubMed]
- Ozkan, A.; Berberoglu, H. Physico-chemical surface properties of microalgae. Colloids Surf. B Biointerfaces 2013, 112, 287–293. [Google Scholar] [CrossRef]
- Bhattacharjee, S.; Ko, C.-H.; Elimelech, M. DLVO interaction between rough surfaces. Langmuir 1998, 14, 3365–3375. [Google Scholar] [CrossRef]
- Hua, L.; Cao, H.; Ma, Q.; Shi, X.; Zhang, X.; Zhang, W. Microalgae filtration using an electrochemically reactive ceramic membrane: Filtration performances, fouling kinetics, and foulant layer characteristics. Environ. Sci. Technol. 2020, 54, 2012–2021. [Google Scholar] [CrossRef]
- Eliseus, A.; Putra, Z.; Bilad, M.; Nordin, N.; Wirzal, M.; Jaafar, J.; Khan, A.L. Energy minimization of a tilted panel filtration system for microalgae filtration: Performance modeling and optimization. Algal Res. 2018, 34, 104–115. [Google Scholar] [CrossRef]
- Salou, M.; Siffert, B.; Jada, A. Study of the stability of bitumen emulsions by application of DLVO theory. Colloids Surf. A Physicochem. Eng. Asp. 1998, 142, 9–16. [Google Scholar] [CrossRef]
- Matamoros, V.; Gutiérrez, R.; Ferrer, I.; García, J.; Bayona, J.M. Capability of microalgae-based wastewater treatment systems to remove emerging organic contaminants: A pilot-scale study. J. Hazard. Mater. 2015, 288, 34–42. [Google Scholar] [CrossRef]
- Bilad, M.; Discart, V.; Vandamme, D.; Foubert, I.; Muylaert, K.; Vankelecom, I.F. Coupled cultivation and pre-harvesting of microalgae in a membrane photobioreactor (MPBR). Bioresour. Technol. 2014, 155, 410–417. [Google Scholar] [CrossRef] [PubMed]
- Melin, T.; Jefferson, B.; Bixio, D.; Thoeye, C.; De Wilde, W.; De Koning, J.; van der Graaf, J.; Wintgens, T. Membrane bioreactor technology for wastewater treatment and reuse. Desalination 2006, 187, 271–282. [Google Scholar] [CrossRef]
- Marbelia, L.; Bilad, M.R.; Passaris, I.; Discart, V.; Vandamme, D.; Beuckels, A.; Muylaert, K.; Vankelecom, I.F. Membrane photobioreactors for integrated microalgae cultivation and nutrient remediation of membrane bioreactors effluent. Bioresour. Technol. 2014, 163, 228–235. [Google Scholar] [CrossRef]
- Lin, H.; Zhang, M.; Mei, R.; Chen, J.; Hong, H. A novel approach for quantitative evaluation of the physicochemical interactions between rough membrane surface and sludge foulants in a submerged membrane bioreactor. Bioresour. Technol. 2014, 171, 247–252. [Google Scholar] [CrossRef]
- Heredia-Arroyo, T.; Wei, W.; Ruan, R.; Hu, B. Mixotrophic cultivation of Chlorella vulgaris and its potential application for the oil accumulation from non-sugar materials. Biomass Bioenergy 2011, 35, 2245–2253. [Google Scholar] [CrossRef]
- Zhang, M.; Leung, K.-T.; Lin, H.; Liao, B. The biological performance of a novel microalgal-bacterial membrane photobioreactor: Effects of HRT and N/P ratio. Chemosphere 2020, 261, 128199. [Google Scholar] [CrossRef] [PubMed]
- Jiang, S.; Xiao, S.; Chu, H.; Zhao, F.; Yu, Z.; Zhou, X.; Zhang, Y. Intelligent mitigation of fouling by means of membrane vibration for algae separation: Dynamics model, comprehensive evaluation, and critical vibration frequency. Water Res. 2020, 182, 115972. [Google Scholar] [CrossRef]
- Ozkan, A.; Berberoglu, H. Adhesion of algal cells to surfaces. Biofouling 2013, 29, 469–482. [Google Scholar] [CrossRef]
- Busscher, H.J.; Weerkamp, A.H.; van der Mei, H.C.; Van Pelt, A.; de Jong, H.P.; Arends, J. Measurement of the surface free energy of bacterial cell surfaces and its relevance for adhesion. Appl. Environ. Microbiol. 1984, 48, 980–983. [Google Scholar] [CrossRef]
- Siddiqui, M.A.Q.; Ali, S.; Fei, H.; Roshan, H. Current understanding of shale wettability: A review on contact angle measurements. Earth-Sci. Rev. 2018, 181, 1–11. [Google Scholar] [CrossRef]
- Ozkan, A.; Berberoglu, H. Cell to substratum and cell to cell interactions of microalgae. Colloids Surf. B Biointerfaces 2013, 112, 302–309. [Google Scholar] [CrossRef] [PubMed]
- Gao, W.; Huang, R. Effect of surface roughness on adhesion of graphene membranes. J. Phys. D Appl. Phys. 2011, 44, 452001. [Google Scholar] [CrossRef]
- Zhang, M.; Zhou, X.; Shen, L.; Cai, X.; Wang, F.; Chen, J.; Lin, H.; Li, R.; Wu, X.; Liao, B.-Q. Quantitative evaluation of the interfacial interactions between a randomly rough sludge floc and membrane surface in a membrane bioreactor based on fractal geometry. Bioresour. Technol. 2017, 234, 198–207. [Google Scholar] [CrossRef]
- Yuan, H.; Zhang, X.; Jiang, Z.; Wang, X.; Chen, X.; Cao, L.; Zhang, X. Analyzing the effect of pH on microalgae adhesion by identifying the dominant interaction between cell and surface. Colloids Surf. B Biointerfaces 2019, 177, 479–486. [Google Scholar] [CrossRef]
- Bhattacharjee, S.; Chen, J.Y.; Elimelech, M. DLVO interaction energy between spheroidal particles and a flat surface. Colloids Surf. A Physicochem. Eng. Asp. 2000, 165, 143–156. [Google Scholar] [CrossRef]
- Bhave, R.; Kuritz, T.; Powell, L.; Adcock, D. Membrane-based energy efficient dewatering of microalgae in biofuels production and recovery of value added co-products. Environ. Sci. Technol. 2012, 46, 5599–5606. [Google Scholar] [CrossRef] [PubMed]
- Cai, X.; Shen, L.; Zhang, M.; Chen, J.; Hong, H.; Lin, H. Membrane fouling in a submerged membrane bioreactor: An unified approach to construct topography and to evaluate interaction energy between two randomly rough surfaces. Bioresour. Technol. 2017, 243, 1121–1132. [Google Scholar] [CrossRef]
- Cai, X.; Yang, L.; Wang, Z.; Zhang, M.; Shen, L.; Hong, H.; Lin, H.; Yu, G. Influences of fractal dimension of membrane surface on interfacial interactions related to membrane fouling in a membrane bioreactor. J. Colloid Interface Sci. 2017, 500, 79–87. [Google Scholar] [CrossRef] [PubMed]
- Yu, G.; Cai, X.; Shen, L.; Chen, J.; Hong, H.; Lin, H.; Li, R. A novel integrated method for quantification of interfacial interactions between two rough bioparticles. J. Colloid Interface Sci. 2018, 516, 295–303. [Google Scholar] [CrossRef] [PubMed]
- Zhao, L.; Zhang, M.; He, Y.; Chen, J.; Hong, H.; Liao, B.-Q.; Lin, H. A new method for modeling rough membrane surface and calculation of interfacial interactions. Bioresour. Technol. 2016, 200, 451–457. [Google Scholar] [CrossRef]
- Zhao, L.; Yang, L.; Lin, H.; Zhang, M.; Yu, H.; Liao, B.-Q.; Wang, F.; Zhou, X.; Li, R. Modeling three-dimensional surface morphology of biocake layer in a membrane bioreactor based on fractal geometry. Bioresour. Technol. 2016, 222, 478–484. [Google Scholar] [CrossRef] [PubMed]
- Lu, D.; Fatehi, P. Interfacial interactions of rough spherical surfaces with random topographies. Colloids Surf. A Physicochem. Eng. Asp. 2022, 642, 128570. [Google Scholar] [CrossRef]
- Xia, L.; Li, H.; Song, S. Cell surface characterization of some oleaginous green algae. J. Appl. Phycol. 2016, 28, 2323–2332. [Google Scholar] [CrossRef]
- Huang, K.; Huang, X.; Jia, Y.; Wang, S.; Cao, Z.; Zhong, H. A novel surfactant styryl phosphonate mono-iso-octyl ester with improved adsorption capacity and hydrophobicity for cassiterite flotation. Miner. Eng. 2019, 142, 105895. [Google Scholar] [CrossRef]
- Tay, J.-H.; Xu, H.-L.; Teo, K.-C. Molecular mechanism of granulation. I: H+ translocation-dehydration theory. J. Environ. Eng. 2000, 126, 403–410. [Google Scholar] [CrossRef]
- Tamada, Y.; Ikada, Y. Effect of preadsorbed proteins on cell adhesion to polymer surfaces. J. Colloid Interface Sci. 1993, 155, 334–339. [Google Scholar] [CrossRef]
- Yin, W.-z.; Wang, J.-z. Effects of particle size and particle interactions on scheelite flotation. Trans. Nonferrous Met. Soc. China 2014, 24, 3682–3687. [Google Scholar] [CrossRef]
- Mikelonis, A.M.; Youn, S.; Lawler, D.F. DLVO approximation methods for predicting the attachment of silver nanoparticles to ceramic membranes. Langmuir 2016, 32, 1723–1731. [Google Scholar] [CrossRef]
- Chen, L.; Tian, Y.; Cao, C.-q.; Zhang, J.; Li, Z.-n. Interaction energy evaluation of soluble microbial products (SMP) on different membrane surfaces: Role of the reconstructed membrane topology. Water Res. 2012, 46, 2693–2704. [Google Scholar] [CrossRef]
- Sanjari, S.; Sarhadi, H.; Shahdadi, F. Investigating the effect of Spirulina platensis microalgae on textural and sensory properties of baguette bread. J. Nutr. Food Secur. 2018, 3, 218–225. [Google Scholar] [CrossRef]
- Walz, J.Y. The effect of surface heterogeneities on colloidal forces. Adv. Colloid Interface Sci. 1998, 74, 119–168. [Google Scholar] [CrossRef]
- Shen, C.; Li, B.; Wang, C.; Huang, Y.; Jin, Y. Surface roughness effect on deposition of nano-and micro-sized colloids in saturated columns at different solution ionic strengths. Vadose Zone J. 2011, 10, 1071–1081. [Google Scholar] [CrossRef]
- Feng, S.; Yu, G.; Cai, X.; Eulade, M.; Lin, H.; Chen, J.; Liu, Y.; Liao, B.-Q. Effects of fractal roughness of membrane surfaces on interfacial interactions associated with membrane fouling in a membrane bioreactor. Bioresour. Technol. 2017, 244, 560–568. [Google Scholar] [CrossRef] [PubMed]
- Hong, H.; Cai, X.; Shen, L.; Li, R.; Lin, H.J.B.t. Membrane fouling in a submerged membrane bioreactor: New method and its applications in interfacial interaction quantification. Bioresour. Technol. 2017, 241, 406–414. [Google Scholar] [CrossRef]
- Drelich, J.W.; Bowen, P.K. Hydrophobic nano-asperities in control of energy barrier during particle–surface interactions. Surf. Innov. 2015, 3, 164–171. [Google Scholar] [CrossRef]
- Drelich, J.W. A simplified analysis of the effect of nano-asperities on particle-bubble interactions. Physicochem. Probl. Miner. Process. 2018, 54, 10–18. [Google Scholar]
- Promdaen, S.; Wattuya, P.; Sanevas, N. Automated microalgae image classification. Procedia Comput. Sci. 2014, 29, 1981–1992. [Google Scholar] [CrossRef]
- Suresh, L.; Walz, J.Y. Effect of surface roughness on the interaction energy between a colloidal sphere and a flat plate. J. Colloid Interface Sci. 1996, 183, 199–213. [Google Scholar] [CrossRef]
- Erramilli, S.; Genzer, J. Influence of surface topography attributes on settlement and adhesion of natural and synthetic species. Soft Matter 2019, 15, 4045–4067. [Google Scholar] [CrossRef]
- Hoek, E.M.; Agarwal, G.K. Extended DLVO interactions between spherical particles and rough surfaces. J. Colloid Interface Sci. 2006, 298, 50–58. [Google Scholar] [CrossRef]
- Eom, N.; Parsons, D.F.; Craig, V.S. Roughness in surface force measurements: Extension of DLVO theory to describe the forces between hafnia surfaces. J. Phys. Chem. B 2017, 121, 6442–6453. [Google Scholar] [CrossRef]
- Hong, H.; Zhang, M.; He, Y.; Chen, J.; Lin, H. Fouling mechanisms of gel layer in a submerged membrane bioreactor. Bioresour. Technol. 2014, 166, 295–302. [Google Scholar] [CrossRef] [PubMed]
- Van Oss, C.J. Hydrophobicity and hydrophilicity of biosurfaces. Curr. Opin. Colloid Interface Sci. 1997, 2, 503–512. [Google Scholar] [CrossRef]
- Shen, C.; Wang, L.-P.; Li, B.; Huang, Y.; Jin, Y. Role of surface roughness in chemical detachment of colloids deposited at primary energy minima. Vadose Zone J. 2012, 11, vzj2011.0057. [Google Scholar] [CrossRef]
- Won, Y.-J.; Lee, J.; Choi, D.-C.; Chae, H.R.; Kim, I.; Lee, C.-H.; Kim, I.-C. Preparation and application of patterned membranes for wastewater treatment. Environ. Sci. Technol. 2012, 46, 11021–11027. [Google Scholar] [CrossRef]
- Li, F.; Meng, J.; Ye, J.; Yang, B.; Tian, Q.; Deng, C. Surface modification of PES ultrafiltration membrane by polydopamine coating and poly (ethylene glycol) grafting: Morphology, stability, and anti-fouling. Desalination 2014, 344, 422–430. [Google Scholar] [CrossRef]






| Solid | γmLW (mJ m−2) | γm− (mJ m−2) | γm+ (mJ m−2) | |||
|---|---|---|---|---|---|---|
| Algae coated surface | 81.5 | 37.0 | 33.6 | 42.641 | 0.230 | 2.965 |
| PDMS | 89.6 | 64.4 | 47.0 | 35.880 | 1.974 | 0.113 |
| PU | 55.7 | 39.5 | 17.1 | 48.581 | 21.511 | 0.026 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khosravizadeh, N.; Lu, D.; Liao, Y.; Liao, B.; Fatehi, P. Simulation and Experimental Analysis of Microalgae and Membrane Surface Interaction. Colloids Interfaces 2023, 7, 24. https://doi.org/10.3390/colloids7010024
Khosravizadeh N, Lu D, Liao Y, Liao B, Fatehi P. Simulation and Experimental Analysis of Microalgae and Membrane Surface Interaction. Colloids and Interfaces. 2023; 7(1):24. https://doi.org/10.3390/colloids7010024
Chicago/Turabian StyleKhosravizadeh, Negar, Duowei Lu, Yichen Liao, Baoqiang Liao, and Pedram Fatehi. 2023. "Simulation and Experimental Analysis of Microalgae and Membrane Surface Interaction" Colloids and Interfaces 7, no. 1: 24. https://doi.org/10.3390/colloids7010024
APA StyleKhosravizadeh, N., Lu, D., Liao, Y., Liao, B., & Fatehi, P. (2023). Simulation and Experimental Analysis of Microalgae and Membrane Surface Interaction. Colloids and Interfaces, 7(1), 24. https://doi.org/10.3390/colloids7010024








