From Repeatability to Self-Organization of Guided Streamers Propagating in a Jet of Cold Plasma
Abstract
:1. Introduction
1.1. Atmospheric Pressure Plasma Jets
1.2. Streamers
- Non-reactive species that can be excited to upper energetic levels (e.g., nitrogen molecules in the second positive system, Helium metastable species, …)
- Chemically reactive species, whether long-lived (e.g., O3) or very short-lived (e.g., radicals with one or more unpaired electrons on their outer layer such as OH, NO, O, O+).
- In nano- or micro-pulsed plasma discharges, streamers can be repeated periodically in space and time: they follow each other at regular time intervals and are formed at the same spatial coordinates. Generally, these so-called guided streamers are produced by high-voltage pulses with widths of a few µs and periods of a few tens of ms [15,16].
- In dielectric barrier devices supplied by AC power supply or in point-plate configurations supplied by DC voltage, streamers are generated with random spatial and temporal distributions. Typically, these streamers follow each other at irregular time intervals and without a predictable spatial arrangement. In the latter case, a distinction can be made between threadlike streamers (which remain single streamers while gradually decreasing in diameter and velocity without branching) and branched streamers (which split into thinner streamers to form a tree-like structure). These streamers generally branch into two new channels that move away from each other since neighboring streamers generally have the same polarity (repulsion forces) [17].
1.3. Self-Organization
2. Materials and Methods
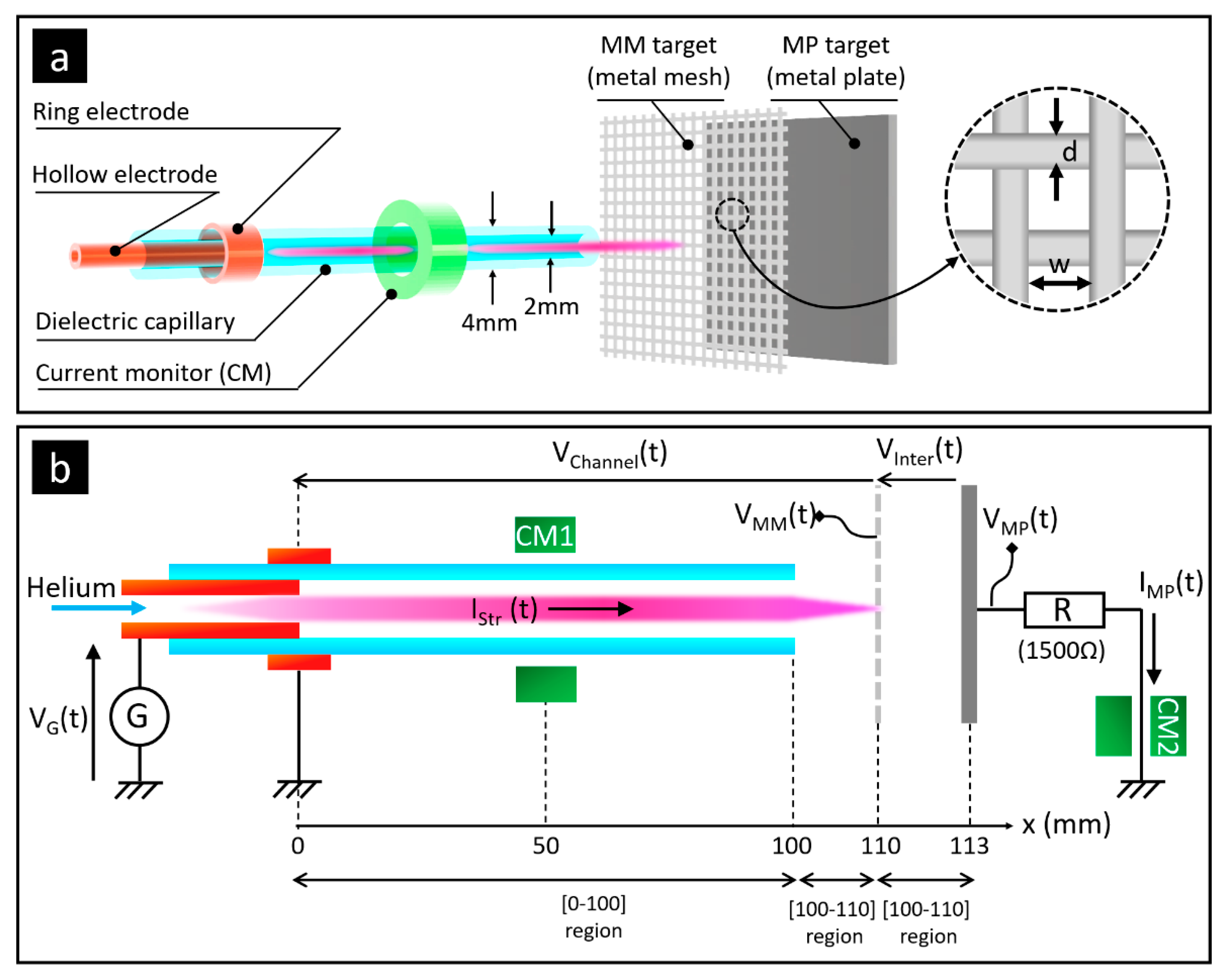
2.1. CHEREL Device and Targets
- A metal mesh target (MM) at floating potential placed 10 mm from the dielectric capillary. Surface area of the MM target is 8 cm × 8 cm and its mesh has a nominal aperture (w) of 300 µm, a wire diameter (d) of 220 µm and an estimated void percentage of .
- A metal plate target (MP) connected to a 1.5 kΩ resistor that is grounded. Surface area of the metal plate is 8 cm × 8 cm. It is placed 3 mm from the mesh, as shown in Figure 1b.
2.2. Diagnostics
2.2.1. Electrical Characterizations
- A current monitor (Model 2877, from Pearson Electronics, Beccles, UK) is coaxially centered around the dielectric capillary at x = 50 mm to measure the current of the guided streamers (IStr).
- A high-voltage probe (Model P6015A 1000:1, from Tektronix, Beaverton, OR, USA) is connected to the MM target to measure its voltage (VMM).
- A current monitor (Pearson, 2877) and a high-voltage probe (Model PPE 20 kV 1000:1, from Teledyne LeCroy) are connected to the MP target to measure its current (IMP) and voltage (VMP), respectively.
2.2.2. Fast ICCD Imaging and Optical Emission Spectroscopy
3. Results and Discussion
3.1. Experimental Conditions Driving to Self-Organization of Guided Streamers within a Plasma Jet
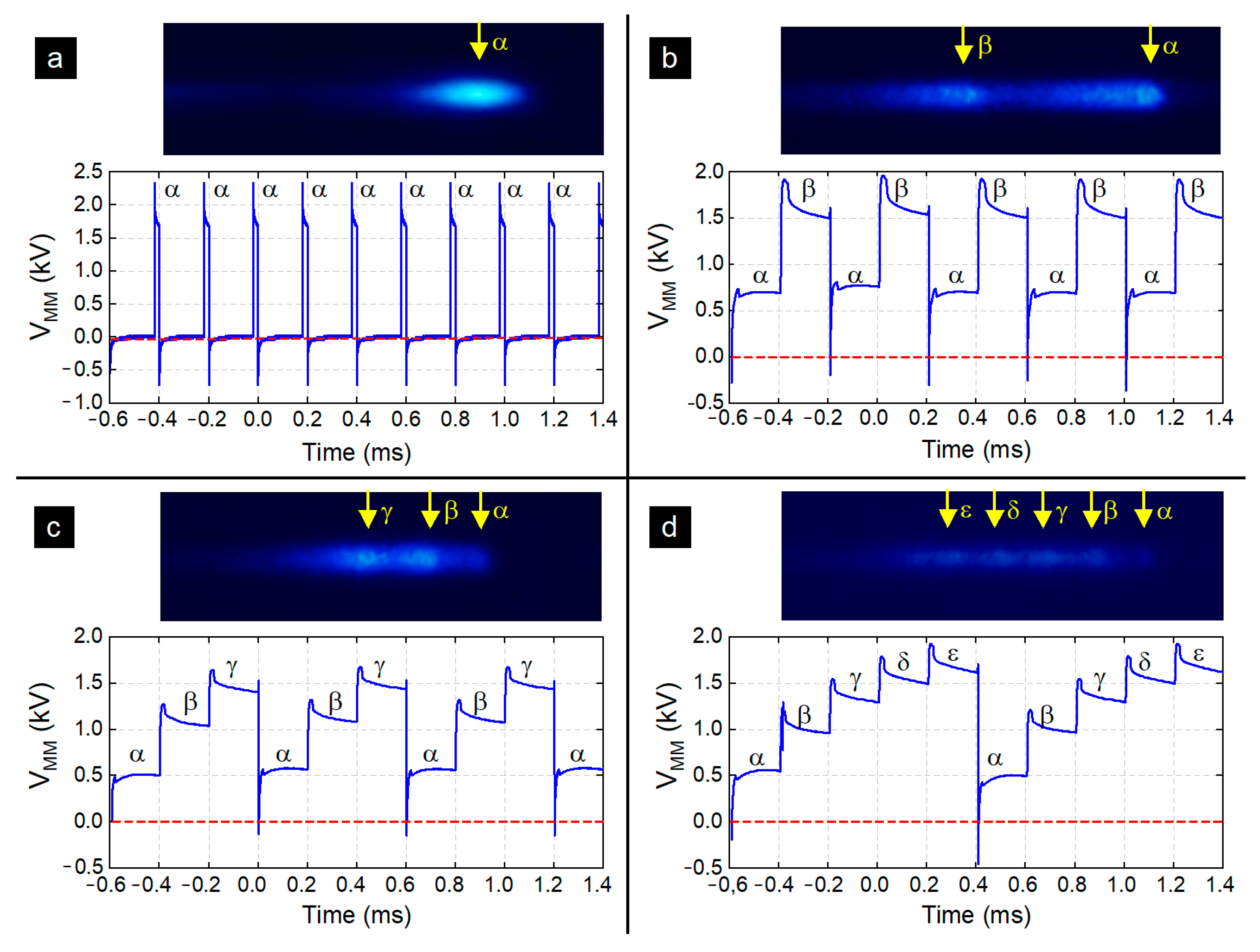
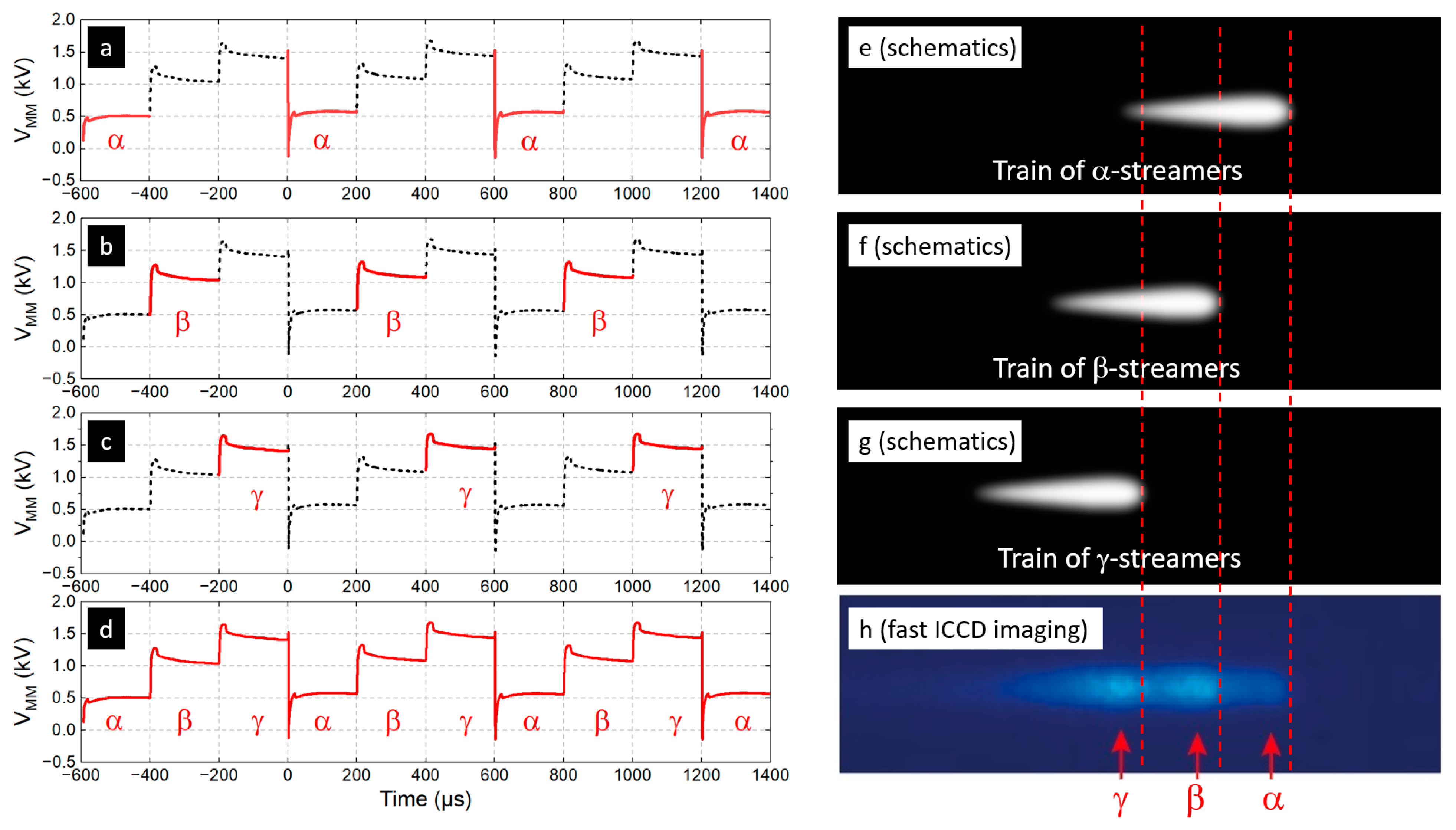
3.1.1. Emergence of Self-Organized Guided Streamers
3.1.2. Complexity Order
3.2. Optical Characterization (Ω = 3)
3.2.1. Positive Guided Streamers
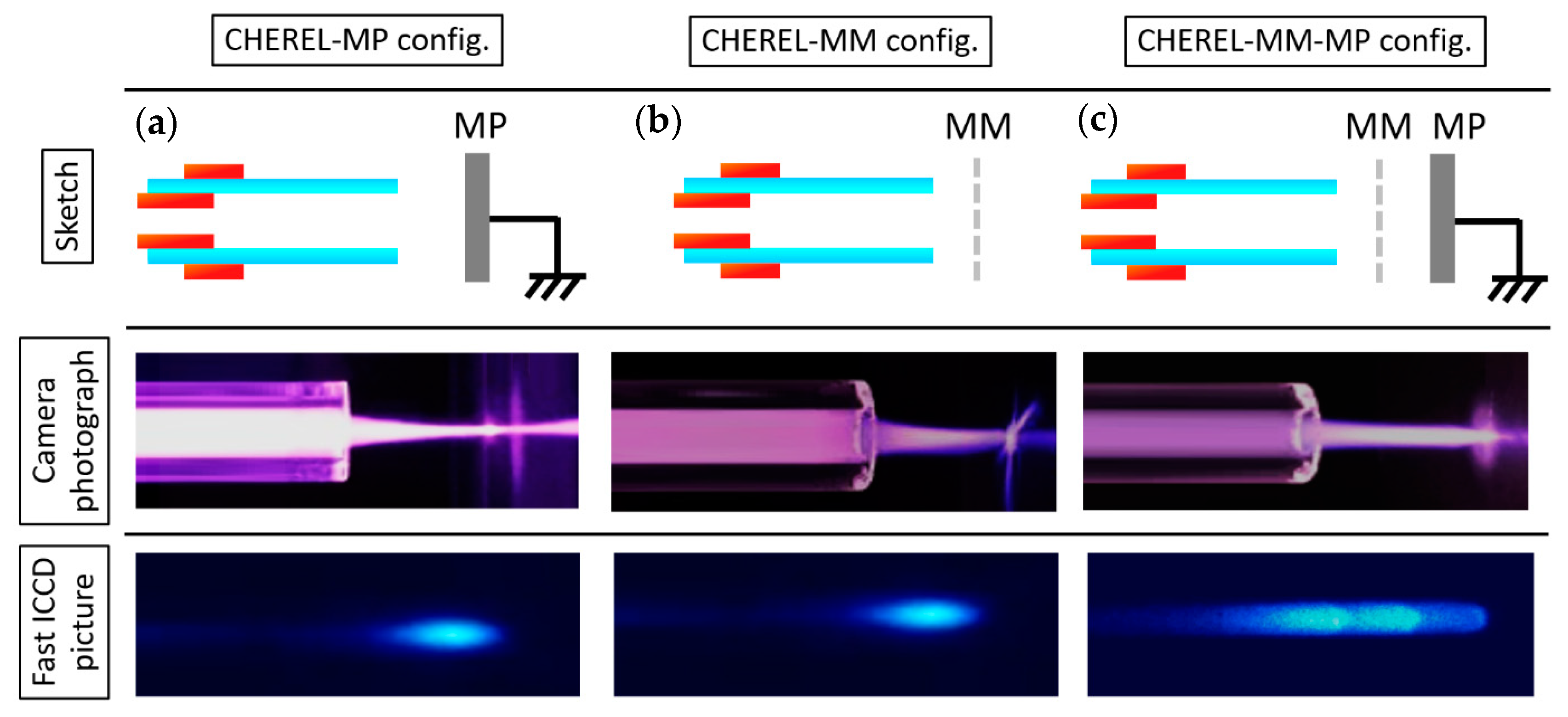
Spontaneous Emergence of Self-Organization
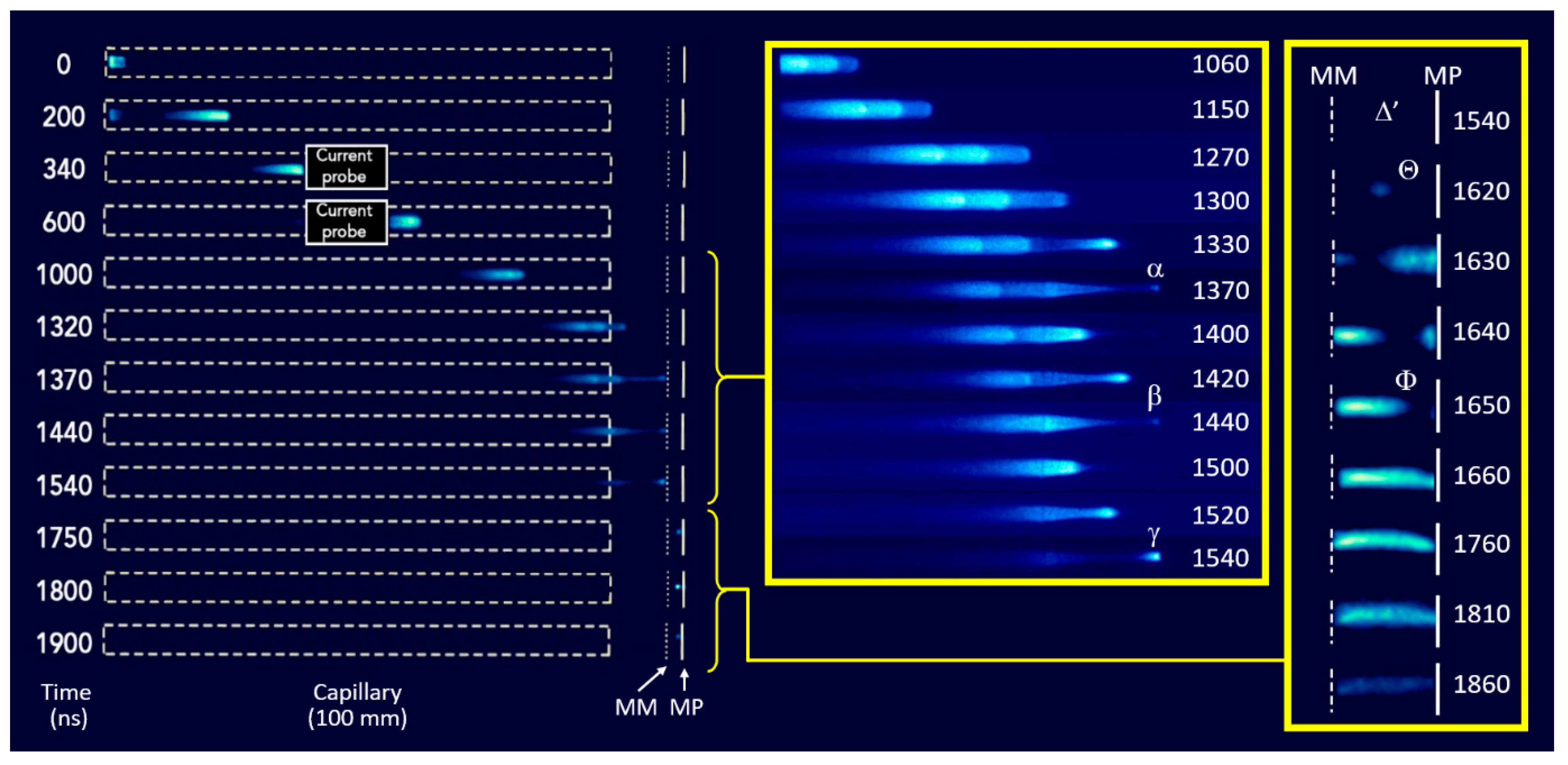
Propagation Velocities
- In the capillary, the α streamers are the fastest (75.7 km·s–1), followed by the β (66.5 km·s–1) and γ (58.2 km·s–1) streamers.
- At the capillary’s outlet, the three self-organized guided streamers gain in velocity while keeping the same inequality ratios (vα > vβ > vγ).
- A more detailed explanation on the difference of velocities between α, β and γ is proposed in Section 3.3.2.
Duration between two Consecutive Streamers
3.2.2. Transient Emissive Phenomena Induced by the Self-Organized Positive Guided Streamers
Dark Domains
Residual Surface Discharge (Outlet Capillary)
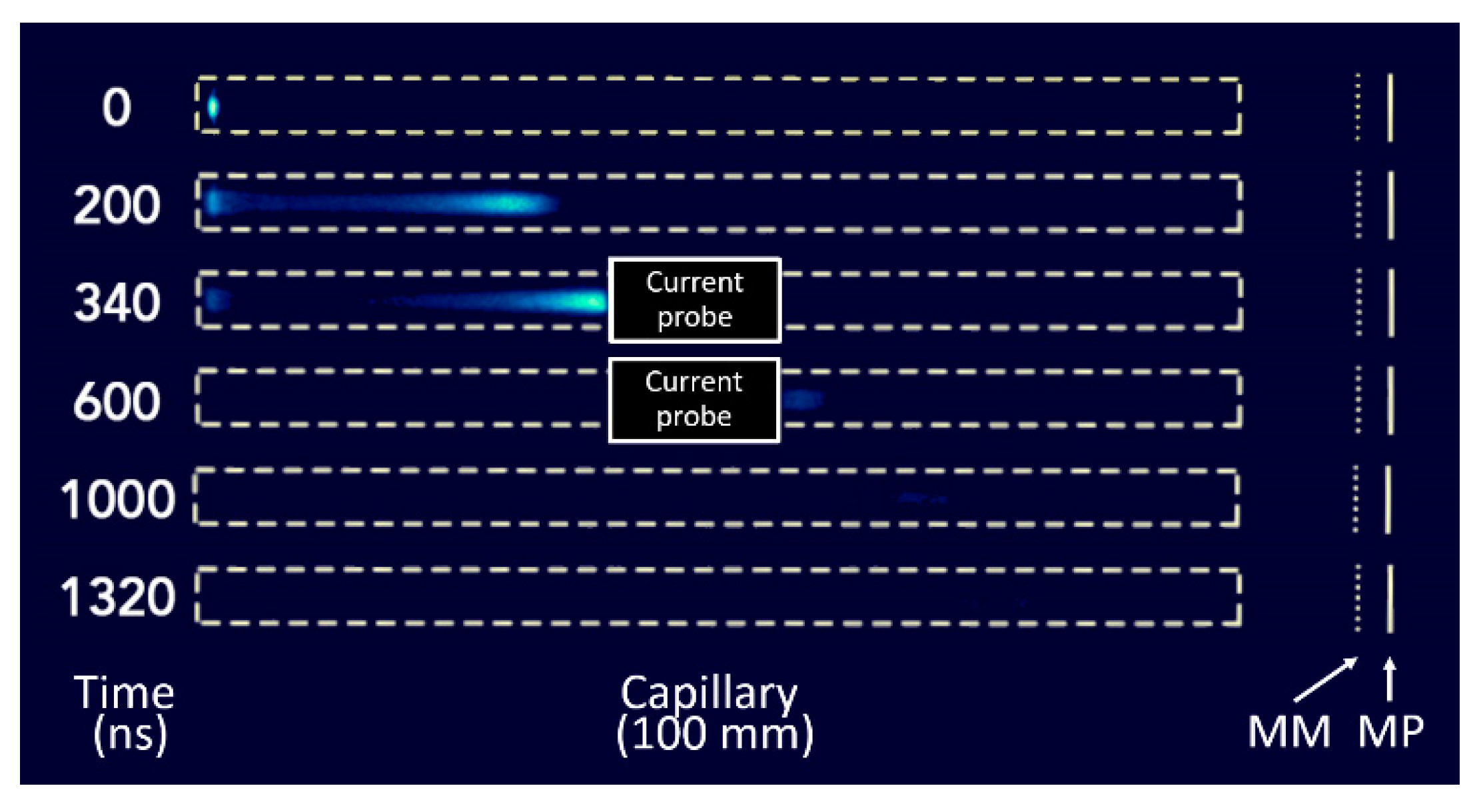
Primary Ionizing Event and Filamentary Discharge in the Inter-Target Region
- Φ could be a streamer, according to the definition recalled in Section 1.1. Nevertheless, the hypothesis of a guided streamer is unlikely because of the absence of a strongly emissive head and because of its slow and stationary disappearance (between 1760 and 1900 ns). Alternatively, the hypothesis of an unguided streamer seems also unlikely because of its linear and stable trajectory. Recall that the ICCD camera makes it possible to observe not a single streamer but a train of 25,000 streamers overlaid on each other in the same photograph. If Φ corresponded to a train of unguided streamers, then it could not have such a filamentary aspect as obtained in Figure 6. Finally, the inter-target region is characterized by a p.d product of value 250 Torr.cm, which induces an electric field too weak to generate a streamer. As an alternative, the filamentary discharge may be assimilated to an evanescent glow discharge.
- Φ could be a return stroke [36]. If so, this implies the simultaneous formation of an upward positive leader and a downward negative leader. The upward positive leader would result from an accumulation of positive charges at a very localized location of the MM target (e.g., on the few metal meshes impacted by the α, β and γ streamers). This leader would hardly propagate in the inter-target region but would remain localized in the interstitial spaces of the MM target. Simultaneously, the downward negative leader would result from the negative charges provided by the MP target which is grounded via the 1.5 kΩ resistor. Indeed, the ground has an electrical potential always maintained at 0 V and behaves as a reservoir containing an infinite number of free electrons. These electrons can therefore provide negative charges to form a downward negative leader propagating from MP to the MM target, until it reaches the upward positive leader. Then, the junction point between these two reversed polarized channels would lead to the formation of a return stroke, characterized by a uniform emission in the course of time, either during its propagation from MM to MP or during its progressive disappearance between the two targets.
Optical Emission Spectrum in the Plasma Jet
3.2.3. Negative Guided Streamers
3.3. Electrical Characterization (Ω = 3)
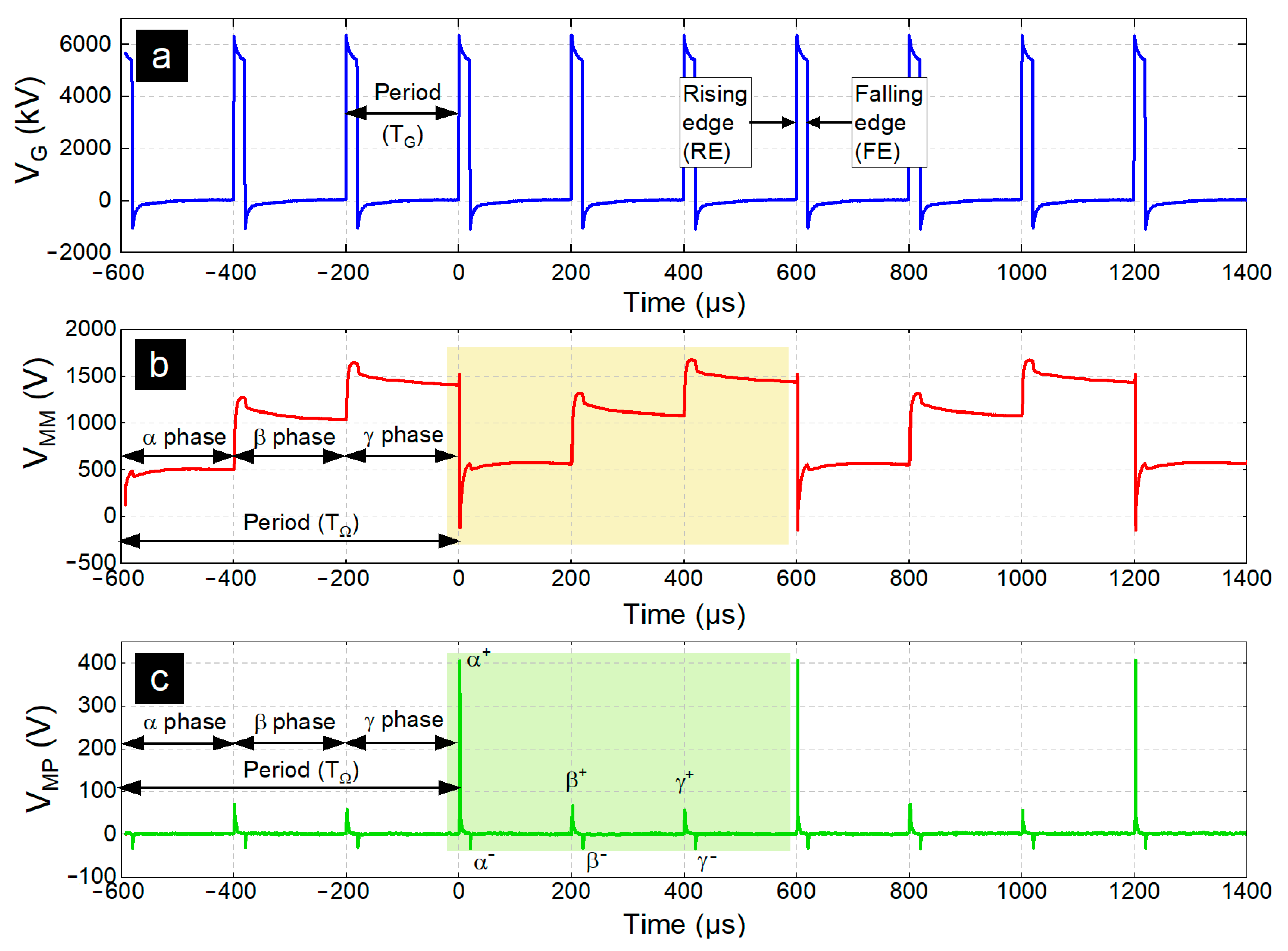
3.3.1. Overview
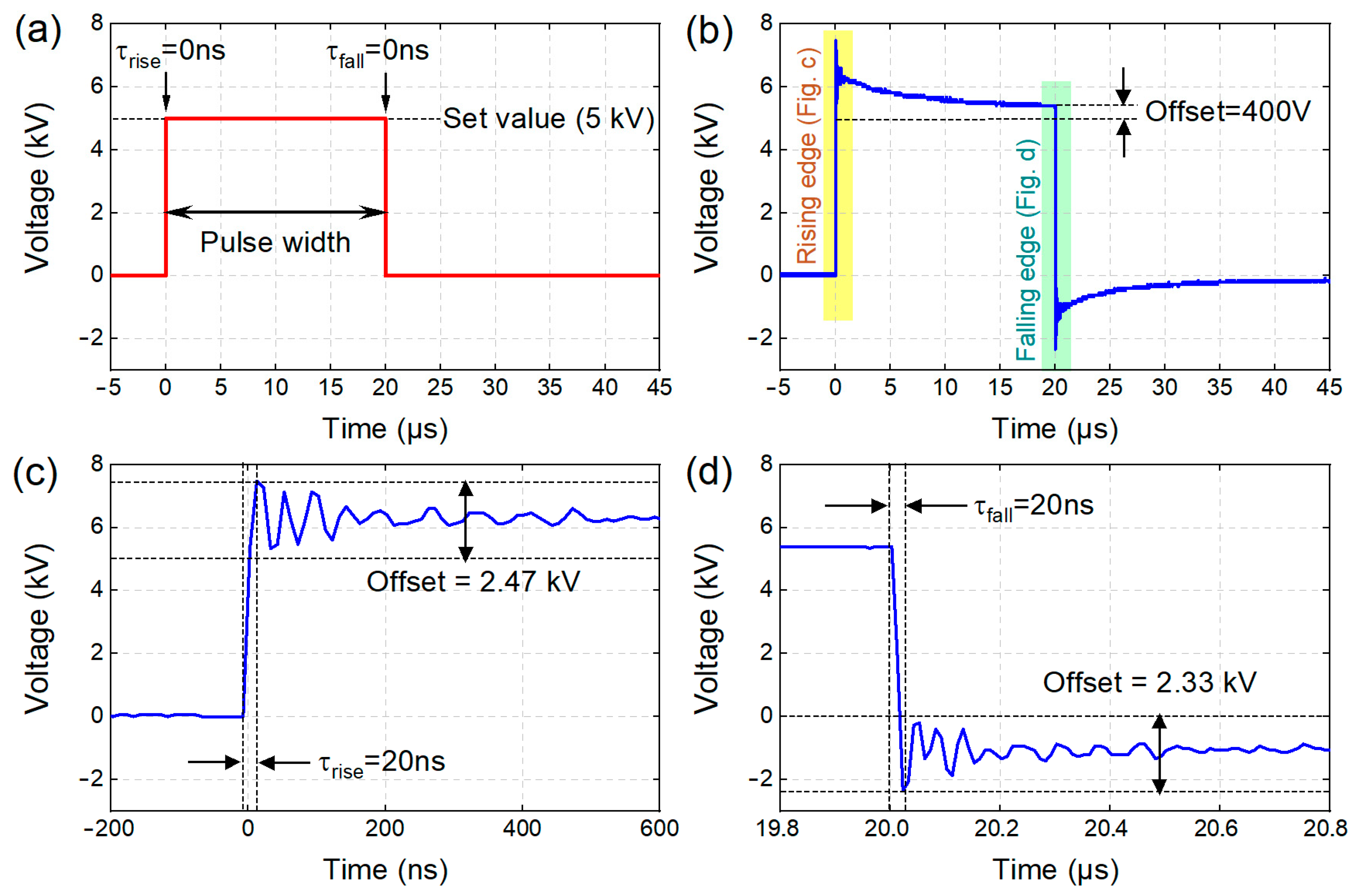
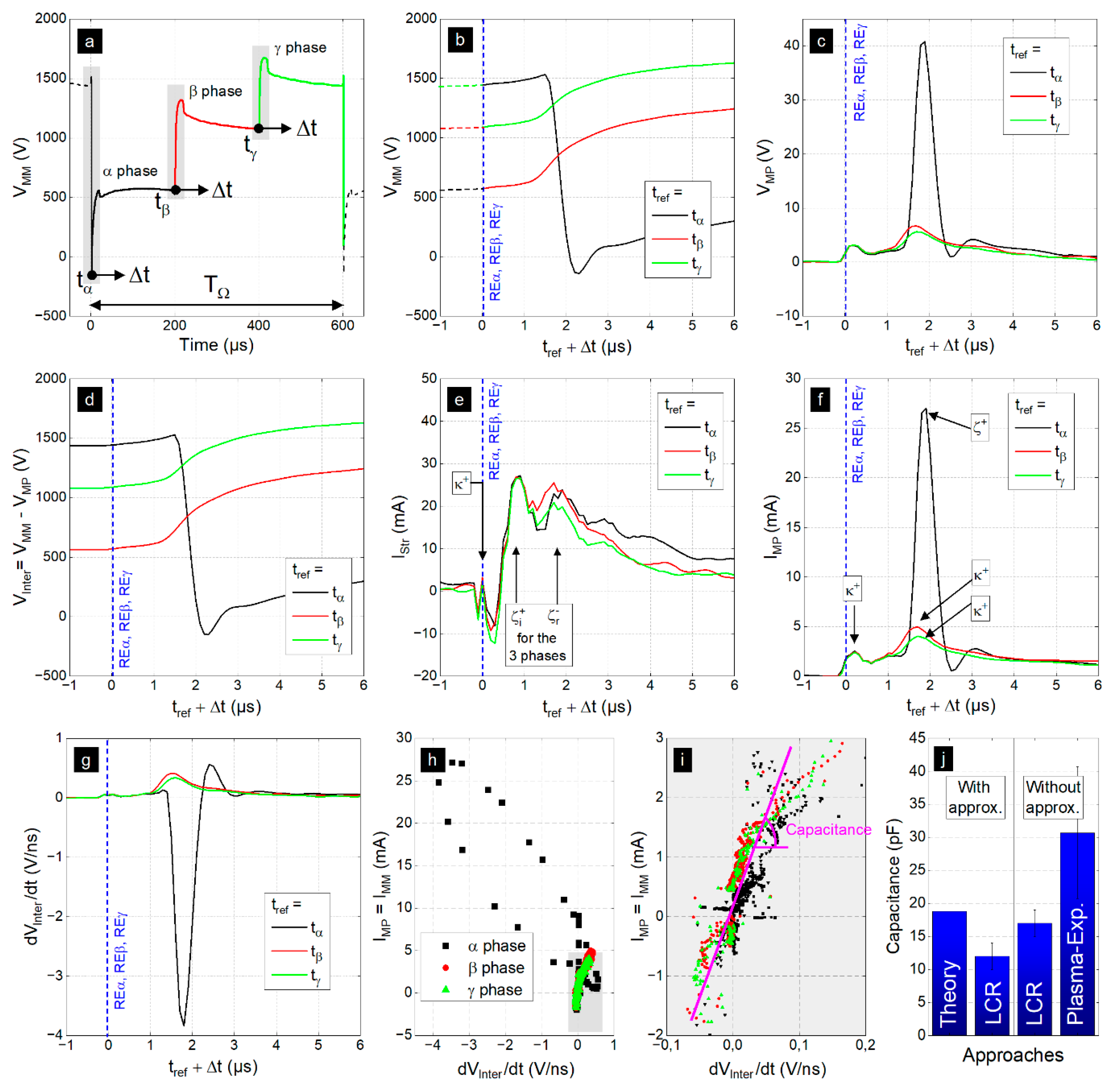
3.3.2. Analysis of the Rising Edges
Voltages
Currents
- A capacitive current peak, noted κ, appearing at the rising edge and proportional to the derivative of the supplied pulse voltage. The exponent “ + ” means that the peak is positive.
- A first conduction current peak, noted . This peak corresponds to the incident positive guided streamer.
- A second conduction current peak, noted . This peak corresponds to the negative guided streamer, reflected by the MM target and which therefore arrives with a delay of 1 µs compared to the first. The mechanisms governing this counter-propagation phenomenon are detailed in our previous article [15].
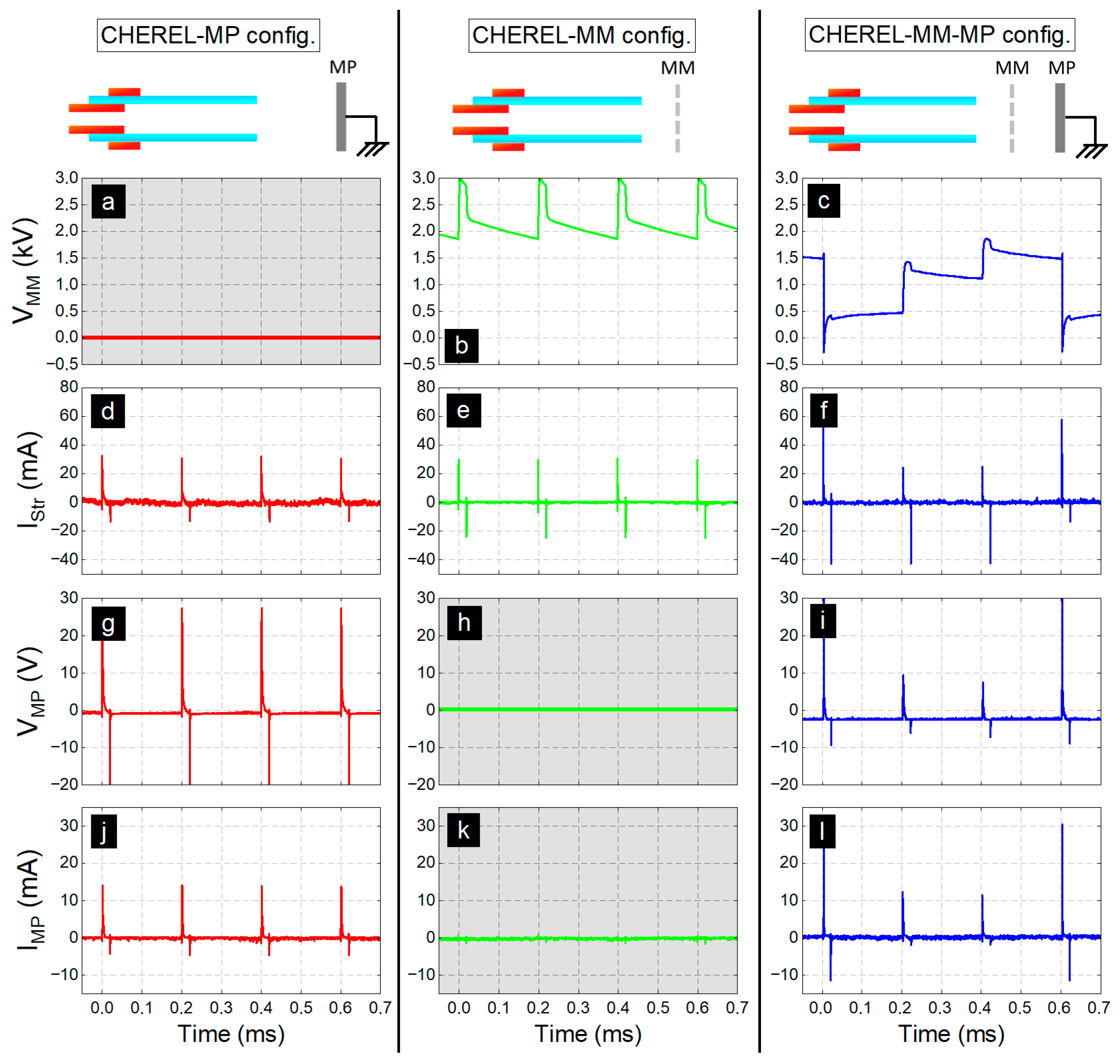
- The conservation of the electric charge is respected at all points of the experimental setup, from the CHEREL to MM and from MM to MP. In the CHEREL, the profile of the current is always the same from one phase to another, so that the conservation hypothesis is verified taking TG (200 µs) as the reference period. However, in the inter-target region, the current shows a different temporal profile so that the relations (2) and (3) are respected. Therefore, the conservation of the electric charge is validated only upon TΩ (600 µs) considered here as the reference period.
- It is important to rule on the capacitive or conductive nature of the current peaks obtained in Figure 12f because only a conductive current peak can be associated with a streamer. Conversely, a capacitive current peak has the characteristic of being proportional to the derivative of the voltage, the proportionality coefficient corresponding to the capacitance of the dielectric medium as indicated in relation (4). By plotting the derivative of VInter(t) on the α, β and γ phases, Figure 12g discriminates on the one hand the capacitive current peaks, which are positive and which correspond to those of the β and γ phases, and on the other hand the conduction current peak, which is oriented in the opposite direction and whose amplitude is in absolute value 10 times greater. Conduction and capacitive current peaks can also be differentiated by plotting IMP(t) vs. VInter/dt, as proposed in Figure 12h. According to (4), this figure represents a capacitive current as a straight line whose slope provides the capacitance while a conductive current is represented by a closed contour whose area provides its energy. Here, the closed contour (black curve) indicates the existence of a conduction current peak upon the α phase while the linear profiles of the β and γ phases indicate capacitive current peaks. For the sake of clarity, a magnification view of Figure 12h is proposed in Figure 12i for dVInter/dt between −0.1 and 0.2 V/ns. A graphical reading provides a capacitance of 31 (±9) pF.
- In Figure 12f, at tref + 0.3 µs, each phase shows a first peak of 2–3 mA, which corresponds to a capacitive peak, denoted . At tref + 1.7 µs, each phase is characterized by a second current peak: (i) the peaks of the β and γ phases are relatively small (4–5 mA) and correspond to capacitive peaks, also referenced ; (ii) The peak of the α phase has a magnitude as high as 27 mA and corresponds to a flow of free charges that propagates into the inter-target region, forming the filamentary discharge already detected by fast ICCD imaging (see Figure 6). The α peak is therefore a conduction peak noted . Finally, a third peak seems to be detected at tref + 2.9 µs, although there is no evidence to suggest whether this is a simple artifact or a capacitive peak. No third peak is detected for the β and γ phases.
Electric Fields
3.3.3. Analysis of the Falling Edges
3.3.4. Understanding How Streamer Self-Organization Triggers Electrical Phenomena in the Inter-Target Region
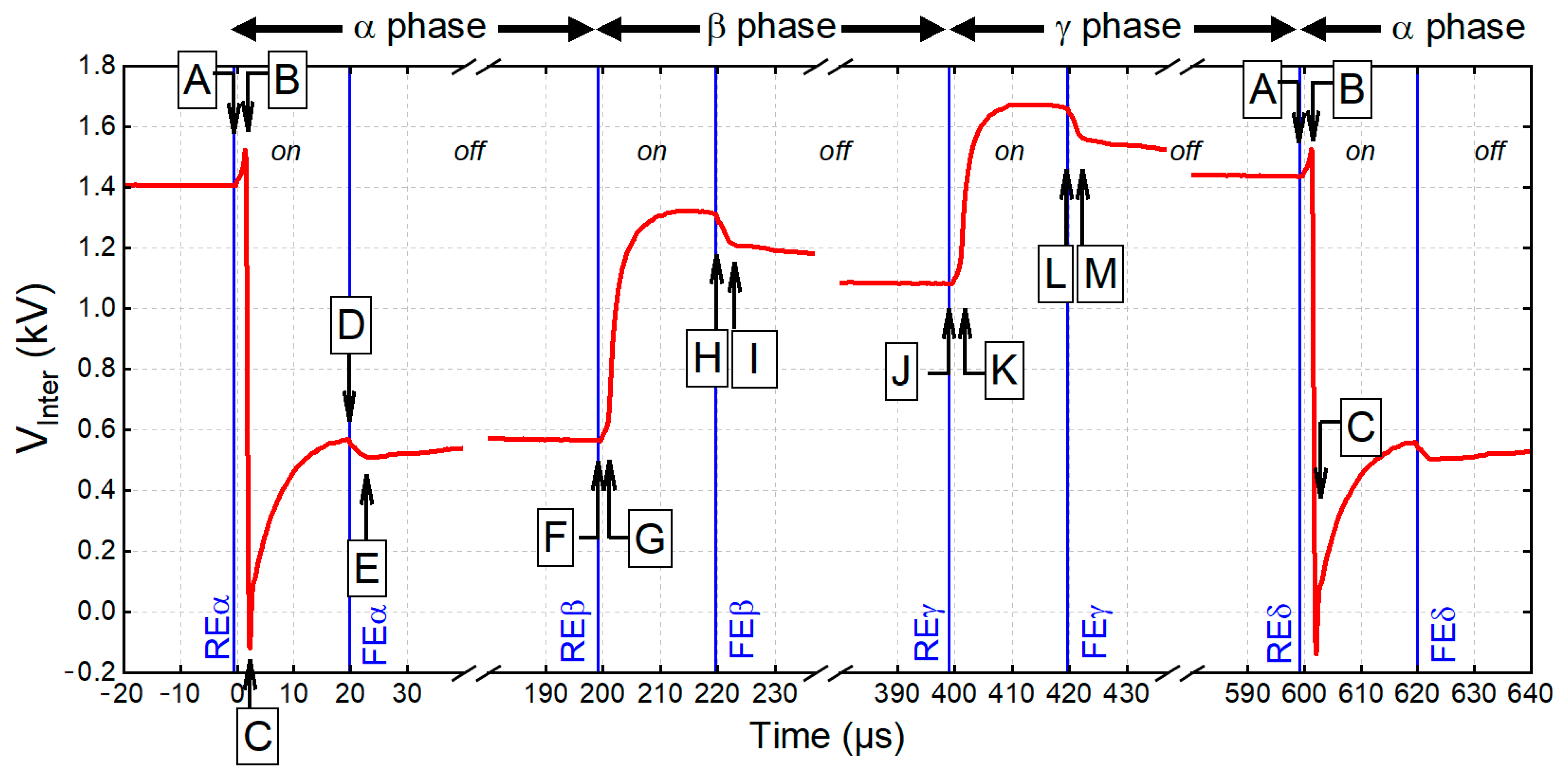
Electrical Charges Carried by the Streamers and Deposited on the MM Target
Characteristics of the Inter-Target Region
- The constant τ, given by the relation (9),
- The electrical conductivity σInter of the air, given by the relation (10),
- The maximum electric charge of the inter-target region, given by Equation (11)
- The voltage VInter whose temporal profile is shown in Figure 15.
Maximum Capacitive Load Condition—Gas Breakdown Condition
Analysis of VInter(t)
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A

References
- Lu, Z.P.; Stachowicz, L.; Kong, P.; Heberlein, J.; Pfender, E. Diamond synthesis by DC thermal plasma CVD at 1 atm. Plasma Chem. Plasma Process. 1991, 11, 387–394. [Google Scholar] [CrossRef]
- Chou, C.H.; Phillips, J. Platinum metal etching in a microwave oxygen plasma. J. Appl. Phys. 1990, 68, 2415–2423. [Google Scholar] [CrossRef]
- Arata, Y.; Kobayashi, A.; Habara, Y. Ceramic coatings produced by means of a gas tunnel-type plasma jet. J. Appl. Phys. 1987, 62, 4884–4889. [Google Scholar] [CrossRef]
- Winter, J.; Brandenburg, R.; Weltmann, K.-D. Atmospheric pressure plasma jets: An overview of devices and new directions. Plasma Sources Sci. Technol. 2015, 24, 064001. [Google Scholar] [CrossRef]
- Freeman, M.P. A quantitative examination of the LTE condition in the effluent of an atmospheric pressure argon plasma jet. J. Quant. Spectrosc. Radiat. Transf. 1986, 8, 435–450. [Google Scholar] [CrossRef]
- Schutze, A.; Jeong, J.Y.; Babayan, S.E.; Park, J.; Selwyn, G.S.; Hicks, R.F. The atmospheric-pressure plasma jet: A review and comparison to other plasma sources. IEEE Trans. Plasma Sci. 1998, 26, 1685–1694. [Google Scholar] [CrossRef]
- Laroussi, M.; Akan, T. Arc-Free Atmospheric Pressure Cold Plasma Jets: A Review. Plasma Process. Polym. 2007, 4, 777–788. [Google Scholar] [CrossRef]
- Lu, X.; Cao, Y.; Yang, P.; Xiong, Q.; Xiong, Z.; Xian, Y.; Pan, Y. An RC Plasma Device for Sterilization of Root Canal of Teeth. IEEE Trans. Plasma Sci. 2009, 37, 668–673. [Google Scholar] [CrossRef]
- Metelmann, H.-R.; Seebauer, C.; Miller, V.; Fridman, A.; Bauer, G.; Graves, D.B.; Pouvesle, J.-M.; Rutkowski, R.; Schuster, M.; Bekeschus, S.; et al. Clinical experience with cold plasma in the treatment of locally advanced head and neck cancer. Clin. Plasma Med. 2018, 9, 6–13. [Google Scholar] [CrossRef]
- Dubuc, A.; Monsarrat, P.; Virard, F.; Merbahi, N.; Sarrette, J.-P.; Laurencin-Dalicieux, S.; Cousty, S. Use of cold-atmospheric plasma in oncology: A concise systematic review. Ther. Adv. Med. Oncol. 2018, 10, 1758835918786475. [Google Scholar] [CrossRef]
- Puač, N.; Škoro, N.; Spasić, K.; Živković, S.; Milutinović, M.; Malović, G.; Petrović, Z.L. Activity of catalase enzyme in Paulownia tomentosa seeds during the process of germination after treatments with low pressure plasma and plasma activated water. Plasma Process. Polym. 2018, 15, 1700082. [Google Scholar] [CrossRef]
- Ranieri, P.; Sponsel, N.; Kizer, J.; Rojas-Pierce, M.; Hernández, R.; Gatiboni, L.; Grunden, A.; Stapelmann, K. Plasma agriculture: Review from the perspective of the plant and its ecosystem. Plasma Process. Polym. 2021, 18, 2000162. [Google Scholar] [CrossRef]
- Lu, X.; Naidis, G.V.; Laroussi, M.; Ostrikov, K. Guided ionization waves: Theory and experiments. Phys. Rep. 2014, 540, 123–166. [Google Scholar] [CrossRef]
- Nijdam, S.; Teunissen, J.; Ebert, U. The physics of streamer discharge phenomena. Plasma Sources Sci. Technol. 2020, 29, 50. [Google Scholar] [CrossRef]
- Decauchy, H.; Dufour, T. Transmission and multiple reflection mechanisms of guided streamers propagating through grounded annular electrode and interacting with grounded surface electrode. Plasma Sources Sci. Technol. 2022, 31, 27. [Google Scholar] [CrossRef]
- Schmidt-Bleker, A.; Norberg, S.A.; Winter, J.; Johnsen, E.; Reuter, S.; Weltmann, K.D.; Kushner, M.J. Propagation mechanisms of guided streamers in plasma jets: The influence of electronegativity of the surrounding gas. Plasma Sources Sci. Technol. 2015, 24, 035022. [Google Scholar] [CrossRef]
- van Veldhuizen, E.M.; Kemps, P.C.M.; Rutgers, W.R. Streamer branching in a short gap: The influence of the power supply. IEEE Trans. Plasma Sci. 2002, 30, 162–163. [Google Scholar] [CrossRef]
- Heylighen, F. The Science of Self-organization and Adaptivity, Knowledge Management, Organizational Intelligence and Learning and Complexity. In Encyclopedia of Life Support Systems; Kiel, L.D., Ed.; Eolss Publishers: Oxford, UK, 2002; pp. 1–26. Available online: http://www.eolss.net (accessed on 14 January 2023).
- de Wolf, T.; Holvoet, T. Emergence versus self-organisation: Different concepts but promising When combined Engineering Self-Organising Systems ESOA 2004. In Lecture Notes in Computer Science; Brueckner, S.A., Di Marzo Serugendo, G., Karageorgos, A., Nagpal, R., Eds.; Springer: Berlin/Heidelberg, Germany, 2005; Volume 3464. [Google Scholar] [CrossRef]
- von Foerster, H. On self-organizing systems and their environments. In Understanding; Springer: New York, NY, USA, 2003; pp. 31–50. ISBN 978-0-387-21722-2. [Google Scholar] [CrossRef]
- Atlan, H. Sources of Information in Biological Systems. IFAC Proc. Vol. 1977, 10, 177–184. [Google Scholar] [CrossRef]
- Priogigne, I. Time, Structure and Fluctuations. Science 1978, 201, 777–785. [Google Scholar] [CrossRef]
- Prigogine, I.; Stengers, I. Order Out of Chaos: Man’s New Dialogue with Nature; Bantam Books: New York, NY, USA, 1984; p. 349. ISBN 10-0553340824. [Google Scholar]
- Cattivelli, F. Self-organization in bird flight formations using diffusion adaptation. In IEEE International Workshop on Computational Advances in Multi-Sensor Adaptive Processing (CAMSAP), 3rd ed.; IEEE: Piscataway, NJ, USA, 2009. [Google Scholar] [CrossRef]
- Deneubourg, J.-L.; Aron, S.; Goss, S.; Pasteels, J.M. The self-organizing exploratory pattern of the Argentine ant. J. Insect Behav. 1990, 3, 159–168. [Google Scholar] [CrossRef]
- Garber, A.; Hallerberg, S.; Kantz, H. Predicting extreme avalanches in self-organized critical sandpiles. Phys. Rev. E. 2009, 80, 026124. [Google Scholar] [CrossRef] [PubMed]
- Devillers, T.; Jamet, M.; Barski, A.; Poydenot, V.; Dujardin, R.; Guillemaud, P.B.; Rothman, J.; Amalric, E.B.; Cibert, J.; Mattana, R.; et al. Ferromagnetism of self-organized Ge1–xMnx nano-pillars. Phys. Status Solidi. C 2007, 3, 4123–4126. [Google Scholar] [CrossRef]
- Kästner, M.; Herrmann, C.; Däweritz, L.; Ploog, K.H. Atomic scale morphology of self-organized periodic elastic domains in epitaxial ferromagnetic MnAs films. J. Appl. Phys. 2002, 92, 5711–5713. [Google Scholar] [CrossRef]
- Vukics, A.; Maschler, C.; Ritsch, H. Microscopic physics of quantum self-organization of optical lattices in cavities. New J. Phys. 2007, 9, 16. [Google Scholar] [CrossRef]
- Chubynsky, M.V.; Brière, M.-A.; Mousseau, N. Self-organization with equilibration: A model for the intermediate phase in rigidity percolation. Phys. Rev. E 2006, 74, 016116. [Google Scholar] [CrossRef]
- Schoenbach, K.H.; Moselhy, M.; Shi, W. Self-organization in cathode boundary layer microdischarges. Plasma Sources Sci. Technol. 2004, 13, 177–185. [Google Scholar] [CrossRef]
- Zhang, S.; Dufour, T. Self-organized patterns by a DC pin liquid anode discharge in ambient air: Effect of liquid types on formation. Phys. Plasmas 2018, 25, 073502. [Google Scholar] [CrossRef]
- Anders, A. Self-organization and self-limitation in high power impulse magnetron sputtering. Appl. Phys. Lett. 2012, 100, 224104. [Google Scholar] [CrossRef]
- Brandenburg, R.; Bogaczyk, M.; Höft, H.; Nemschokmichal, S.; Tschiersch, R.; Kettlitz, M.; Stollenwerk, L.; Hoder, T.; Wild, R.; Weltmann, K.-D. Novel insights into the development of barrier discharges by advanced volume and surface diagnostics. J. Phys. D Appl. Phys. 2013, 46, 12. [Google Scholar] [CrossRef]
- Darny, T.; Pouvesle, J.-M.; Puech, V.; Douat, C.; Dozias, S.; Robert, E. Analysis of conductive target influence in plasma jet experiments through helium metastable and electric field measurements. Plasma Sources Sci. Technol. 2017, 26, 045008. [Google Scholar] [CrossRef]
- Wang, D.; Takagi, N.; Gamerota, W.R.; Uman, M.A.; Hill, J.D.; Jordan, D.M. Initiation processes of return strokes in rocket-triggered lightning. J. Geophys. Res. Atmos. 2013, 118, 9880–9888. [Google Scholar] [CrossRef]
- Decauchy, H.; Pavy, A.; Camus, M.; Fouassier, L.; Dufour, T. Cold plasma endoscopy applied to biliary ducts: Feasibility risk assessment on human-like and porcine models for the treatment of cholangiocarcinoma. J. Phys. D Appl. Phys. 2022, 55, 455401. [Google Scholar] [CrossRef]
- Chen, Z.; Zhang, S.; Levchenko, I.; Beilis, I.I.; Keidar, M. In vitro demonstration of cancer inhibiting properties from stratified self-organized plasma-liquid interface. Sci. Rep. 2017, 7, 12163. [Google Scholar] [CrossRef] [PubMed]




| Ω | Amplitude (kV) | Repetition Frequency (kHz) | Duty Cycle (%) |
|---|---|---|---|
| 1 | 6.0 | 5.0 | 15 |
| 2 | 5.5 | ||
| 3 | 5.0 | 10 | |
| 4 | 4.7 | ||
| 5 | 4.5 | ||
| 6 | 4.3 |
| Streamers | In the Capillary (75 < x < 100 mm) | Region between Capillary and MM Target |
|---|---|---|
| α | 75.7 km·s–1 | 159.6 km·s–1 |
| β | 66.5 km·s–1 | 140.3 km·s–1 |
| Γ | 58.2 km·s–1 | 124.2 km·s–1 |
| Consecutive Streamers | Repeated Guided Streamers | Self-Organized Guided Streamers | ||
|---|---|---|---|---|
| Absolute Times | Relative Times | Absolute Times | Relative Times | |
| α-β | 200,000 ns | 0 ns | 200,089 ns | 89 ns |
| β-γ | 200,000 ns | 0 ns | 200,103 ns | 103 ns |
| VG(t) | VMM(t) | |
|---|---|---|
| Number of non-zero states | 1 | 3 states labeled α, β and γ at 0.5 kV, 1.1 kV and 1.4 kV, respectively |
| Period | TG = 200 µs | TΩ = 3. TG = 600 µs (self-organization period) |
| Profile | Square-like signal | Succession of linear and non-linear variations |
| Portions of the Curve in Figure 15 | τRC (µs) | CInter (pF) | RInter (kΩ) | σInter (Ω–1·m–1) |
|---|---|---|---|---|
| CD (ou DE) | 4.7 µs | 30 | 156 | 3.0 × 10–6 |
| GH (ou HI) | 1.9 µs | 30 | 65 | 7.2 × 10–6 |
| KL (ou LM) | 1.7 µs | 30 | 56 | 8.4 × 10–6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Decauchy, H.; Dufour, T. From Repeatability to Self-Organization of Guided Streamers Propagating in a Jet of Cold Plasma. Plasma 2023, 6, 250-276. https://doi.org/10.3390/plasma6020019
Decauchy H, Dufour T. From Repeatability to Self-Organization of Guided Streamers Propagating in a Jet of Cold Plasma. Plasma. 2023; 6(2):250-276. https://doi.org/10.3390/plasma6020019
Chicago/Turabian StyleDecauchy, Henri, and Thierry Dufour. 2023. "From Repeatability to Self-Organization of Guided Streamers Propagating in a Jet of Cold Plasma" Plasma 6, no. 2: 250-276. https://doi.org/10.3390/plasma6020019
APA StyleDecauchy, H., & Dufour, T. (2023). From Repeatability to Self-Organization of Guided Streamers Propagating in a Jet of Cold Plasma. Plasma, 6(2), 250-276. https://doi.org/10.3390/plasma6020019







