Exploring the Effects of Additional Vibration on the Perceived Quality of an Electric Cello

Abstract

Haptic feedback holds the potential to enhance the engagement and expressivity of future digital and electric musical instruments. This study investigates the impact of artificial vibration on the perceived quality of a silent electric cello. We developed a haptic cello prototype capable of rendering vibration signals of varying degree of congruence with the produced sound. Experienced cellists participated in an experiment comparing setups with and without vibrotactile feedback, rating them on preference, perceived power, liveliness, and feel. Results show nuanced effects, with added vibrations moderately enhancing feel and liveliness, and significantly increasing perceived power when using vibrations obtained from the pickup at the cello’s bridge. High uncertainty in our statistical model parameters underscores substantial individual differences in the participants responses, as commonly found in qualitative assessments, and highlights the importance of consistent feedback in the vibrotactile and auditory channels. Our findings contribute valuable insights to the intersection of haptics and music technology, paving the way for creating richer and more engaging experiences with future musical instruments.

1. Introduction

The physical exchange between musicians and their acoustic musical instruments, with the complex perception–action mechanisms involved, has always been key to the development of musicianship, in terms of expressivity, performance, and experience [1]. In recent years, the development of electric and subsequently electronic musical instruments has initiated a process where players have progressively detached from the sound source [2]. In the course of technological evolution, electric and bodyless (or silent) string instruments have been developed, offering unique features in terms of portability, volume control, and signal processing. However, these instruments generally lack resonant bodies, and consequently they do not provide the natural vibratory response that musicians often associate with the quality and expressiveness of their traditional acoustic counterparts [3]. This absence raises a critical question: Can the introduction of artificial vibration to a silent string instrument enhance its qualitative features and, by extension, the performance experience for the musician?

Despite the intrinsic physical connection between sound and vibration, a systematic investigation on the role of the latter in musical instruments only started in the mid-1980s [4,5,6]. More recently, the growing interest in this subject—in parallel with the increased availability of accurate sensors, as well as affordable and compact vibrotactile actuators—resulted in the emergence of ‘musical haptics’ as a recognized topic [7], i.e., the study and application of haptic (tactile and force-feedback) sensations in the context of musical experiences, performances, and interactions. The existing studies on the role of haptic feedback on traditional musical instruments have mainly focused on the acoustic piano and the violin, generally finding that haptic cues are indeed relevant to the instruments’ perceived quality, and may be even more important than auditory feedback for their identification [8,9,10].

The present authors previously conducted several assessments of the relevance of vibration to the perceived quality of musical instruments, to the performer’s experience, and performance. In doing so, we addressed various digital musical interfaces and instruments augmented with vibrotactile feedback—either self-developed [11], or existing advanced ones [12]—as well as acoustic and digital pianos [13]. Generally, it was found that vibration consistent with the produced sound can indeed enhance the player’s engagement, their connection with the instrument, and its overall perceived quality.

Building on our previous assessments of vibrotactile feedback in musical instruments, the current study extends our investigation to the e-cello. The rationale for choosing a cello, rather than other more popular string instruments like the violin, is that its usable pitch range (63–880 Hz) significantly overlaps with the conventional range of human vibrotactile perception (20–500 Hz) [6,14]. We hypothesized that the addition of artificial vibration can affect the instrument’s perceived quality and playability. To test this hypothesis, we conducted an experiment where participants—all experienced cellists—were asked to freely play and evaluate an e-cello while switching between two setups: one with additional vibration feedback (test) and another without (reference). Vibration was provided by a compact transducer attached to the instrument, which could be fed with two different signals: one derived from the cello’s pickup at the bridge, and the other obtained from filtered noise. The findings of this study contribute to a better understanding of the audio-tactile properties of the e-cello, holding promise for influencing the design and performance experiences across a range of electric and electronic instruments.

2. Method

2.1. Apparatus

The preparation of our experiment required the implementation of an e-cello augmented with artificial vibration, which could be switched on/off via a pedalboard, and of software to manage auditory and vibrotactile feedback, as well as the experimental procedure.

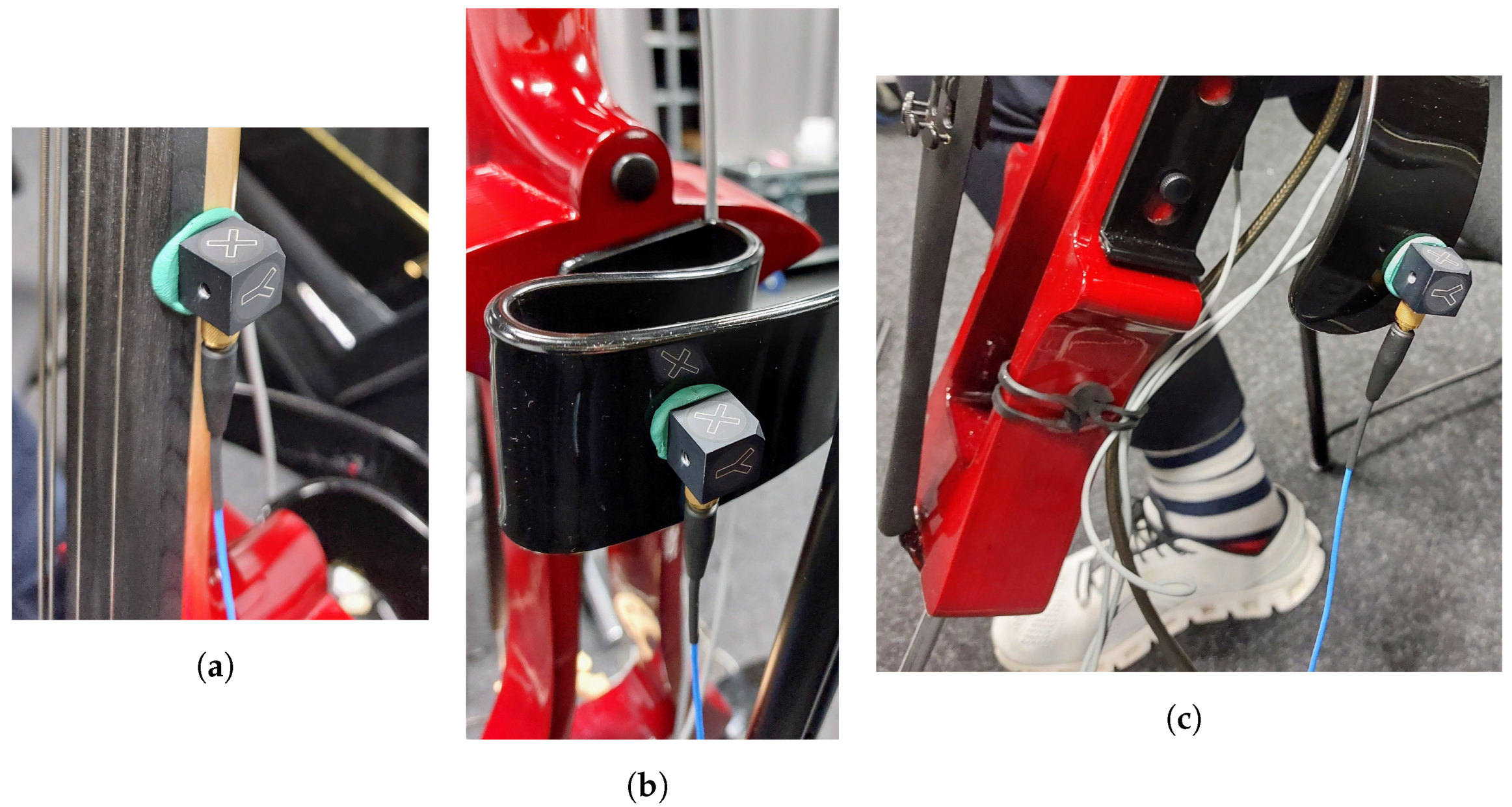
2.1.1. Haptic E-Cello Prototype

A Harley Benton HBCE 990 bodyless e-cello (https://www.thomannmusic.ch/harley_benton_hbce_990rd_electric_cello.htm, accessed on 25 March 2024) was used as a basis for the planned augmentation. The original strings were replaced with a higher quality set (D’Addario Prelude J1010, solid steel core, medium tension) for enhanced tuning stability and durability. During its development, the instrument was equipped with up to two Tactile Labs Haptuator BM3C vibrotactile transducers (see Figure 1), chosen for their compact design (dimensions $45.5\times 20\times 20$ mm) and powerful actuation capabilities in the frequency range of our interest (resonance frequency = 40 Hz, rated bandwidth = 30–1000 Hz, maximum input current = 1 A, maximum input voltage = 5 V, acceleration for 3 V input at resonance frequency = 10 G). Being a ‘silent’ electric instrument, the device offers audio output reproducing the signal captured by a piezoelectric transducer at the bridge. Such signal was sent to a computer via a professional audio interface (RME UCX II), and used to provide auditory feedback to the player, as well as to feed the vibrotactile transducers.

An expert cellist was hired to support the development of the prototype. During various stages of development, the expert would play either his own acoustic instrument or the prototype being developed, offering a subjective comparison between the two instruments, providing qualitative feedback, and suggestions for improvement. Moreover, we could acquire, compare and match vibration produced by the two instruments, as explained further below.

The vibrotactile actuators were fed alternatively with: (i) the raw audio output from the e-cello (bridge signal); (ii) the same signal convolved with the impulse response (IR) of an acoustic cello body. Various IRs were tested, obtained from different acoustic instruments; (https://www.3sigmaaudio.com/items/category/cellos/, accessed on 25 March 2024) (iii) a white noise signal; (iv) the noise signal convolved with the same IRs used for the bridge signal. All vibration signals were band-passed in the 30–1000 Hz range, in order to best suit the actuator’s frequency response while minimizing sound spillage.

Vibrations produced by the expert’s acoustic cello when playing open strings were compared to those generated by the e-cello—the goal was to produce a realistic vibratory response from the e-cello in terms of intensity. Vibrations were recorded at locations that are typically in sustained contact with the cellist, namely the neck of the instrument (left-hand grip, for right-handed players), its top back (chest contact), the lower side (inner thigh contact), as shown in Figure 2 and Figure 3.

A triaxial accelerometer (PCB 356A17), connected to a DAQ (Dewesoft Sirius mini), was employed to record vibrations. The accelerometer was fixed to the instruments using a specialized putty commonly used for attaching contact microphones to string instruments.

Eventually, based on both the expert’s subjective evaluation and objective vibration measurements, it was decided to use only one actuator, attached at the scroll of the e-cello (see Figure 1): From our tests, that location resulted in effective and sufficiently uniform vibration propagating across the instrument. To ensure optimal mechanical coupling and stability, a 3D-printed holder was designed that fitted into the opening of the scroll and accommodated the actuator while allowing to secure it with a zip tie.

2.1.2. Experimental Setup

The final setup used for the experiment is described in what follows. Two vibration signals were selected, based on the expert’s choices:

(1)

A noise signal convolved with the IR preferred by the expert, with further processing to make it more coherent with the sound of the cello (details are given below).

(2)

The bridge signal convolved with the IR preferred by the expert.

Two additional processing stages were applied to the noise signal:

• A pitch detection algorithm tracked the pitch of the bridge signal in real-time, and accordingly set the cutoff frequency of a high-pass filter applied to the noise signal. This allowed the noise signal to be consistent with the bridge output in terms of frequency content.
• An envelope follower algorithm analysing the bridge signal scaled the amplitude of the noise signal accordingly. This made the noise signal consistent with the bridge output in terms of intensity.

Finally, the RMS levels of the vibrations associated to playing open strings with mezzo-forte dynamics were matched to the respective ones recorded on the acoustic cello; this allowed to produce absolute levels of vibration that were realistic.

Table 1. RMS vibration amplitudes (dB re ${10}^{-6}$ m/s²) for the acoustic cello in mezzo-forte dynamics. Measurements taken at the neck, and band-pass filtered (40–400 Hz).

Acoustic Cello	RMS Amplitude [dB]
Strings	X	Y	Z
C	132	140	126
G	134	132	123
D	142	136	132
A	124	117	108

and

Table 2. RMS vibration amplitudes for the e-cello with and without augmentation, taken under the same conditions as in Table 1. Differences from the acoustic cello are provided. The bottom rows report the cumulative difference across strings, showing closer matching with augmentation.

E-Cello	RMS Amplitude [dB]
Strings	X	Diff. X Cello	Y	Diff. Y Cello	Z	Diff. Z Cello
C	115	$-17$	113	$-27$	111	$-15$
G	136	$+2$	135	$+3$	120	$-3$
D	129	$-13$	127	$-9$	119	$-13$
A	120	$-4$	120	$+3$		$+9$
Tot. diff. across strings		$-32$		$-30$		$-22$
E-Cello w/ vibration	RMS amplitude [dB]
Strings	X	Diff. X cello	Y	Diff. Y cello	Z	Diff. Z cello
C	132	0	131	$-9$	119	$-7$
G	139	$+5$	135	$+3$	122	$-1$
D	131	$-11$	128	$-8$	121	$-11$
A	136	$+12$	131	$+14$	126	$+18$
Tot. diff. across strings		$+6$		0		$-1$

, respectively, show RMS vibration amplitude levels for the acoustic cello, and the e-cello with and without augmentation. Measurements were taken at the neck and then band-pass filtered in the 40–400 Hz range to focus on vibrotactile perception [14]. As expected, the acoustic cello exhibits natural variation in vibration amplitude across strings due to its resonant properties. The e-cello setups are compared to these acoustic cello values, with differences shown in separate columns. The bottom rows summarize the cumulative difference across strings. Without augmentation, the e-cello generally shows lower vibration amplitudes than the acoustic cello. The added vibrations increase the e-cello’s amplitudes across most strings; this demonstrates that augmentation reduces the mismatch in vibration levels for each direction, bringing them closer to the acoustic cello’s response.

Auditory feedback was made available via in-ear phones (Shure SE425), and reproduced the bridge signal convolved with the IR of an acoustic cello’s body. Additionally, a subtle reverb was applied to simulate the acoustic space of a room, and improve sound realism. The loudness was set based on the expert’s suggestions, so as to resemble that of an acoustic cello. On top of the in-ear phones, participants wore ear muffs to prevent them from hearing any sounds coming from the instrument or the actuator.

All auditory and vibrotactile feedback was generated in real-time on the computer by means of a custom-made signal processing application (the signal processing software was implemented using Cycling ’74 Max, a graphical programming environment for interactive media), and sent to the participants and e-cello via the audio interface. The feedback latency in our augmented cello system was carefully considered. Empirical measurements confirmed a signal round-trip latency (RTL) of 7.7 ms, resulting in an action–sound delay not affecting the instrument’s perceived quality [15]. As for the vibrotactile channel, the additional delay introduced by the chosen actuator (estimated in the range 4.4–5.2 ms) results in a total delay under 13 ms, that is well within the temporal integration window for audio-tactile perception [16,17], mitigating potential perceptual discrepancies.

A computer screen showed a graphical user interface (GUI) with the qualitative features to be assessed. Responses were given on a visual analog scale (VAS) [18] using a continuous slider operated hands-free via an expression pedal on a pedalboard. The pedalboard also offered various footswitches: two were labeled A and B and allowed participants to alternate between two setups (vibrating/non-vibrating), while another one was used to record ratings and proceed to the next trial. The pedalboard sent MIDI (Musical Instrument Digital Interface) messages to the computer.

The experimental procedure and GUI were managed by a software program realized ad hoc (the experiment management software was programmed in Pure Data, an open-source counterpart to Cycling ’74 Max).

Figure 4 shows the experimental setting, and an example of GUI made available to participants to assign ratings.

2.2. Test Design, Procedure, and Participants

The experimental task was to freely play the e-cello, freely switch between two setups (A/B), and evaluate them by assigning ratings on four quality attributes.

Before starting the actual session, participants were briefed about their task. The following definitions of the qualitative attributes to be assessed were provided:

• Preference: which setup is better?
• Power: which setup is more powerful?
• Liveliness: which setup feels more alive as an instrument?
• Feel: which setup has a better feel?

In this phase, participants could play the haptic e-cello freely for a couple of minutes, while getting acquainted with the pedalboard to switch between setups A/B and to operate rating sliders on the GUI. Vibrotactile feedback was bypassed to avoid any potential disclosing of the underlying mechanism: During briefing participants did not wear earmuffs in order to listen to the experimenter’s instructions, and they were not aware of the specific changes in the two setups, nor of the experiment’s focus on vibrotactile feedback.

Each trial consisted in the assessment of a single attribute. The test stimulus was produced by a setup with added vibrations (either noise or bridge signal), compared to a reference setup without artificial vibrotactile feedback. In each trial, the two setups were randomly designated to the footswitches labeled A and B, and participants had the freedom to alternate between them as many times as desired while playing. After the suggested time of 1 min (a timer was always visible on the GUI), they would stop playing, submit their response and move on to the next trial. Each combination of four attributes ×2 vibration types was presented three times in random order, resulting in 24 trials per participant.

Eleven cellists (4 male, 7 female) participated, either professional or in advanced training (mean age = 25 years, sd = 4.6). They had extensive experience with the cello (mean training = 16 years, sd = 6.1). Only three had played an e-cello previously. Most participants used the provided bow, while two used their own. Their primary points of physical contact with the e-cello were its neck and chest support. While some players also used the optional knee support, it was not required due to potential discomfort.

3. Results

3.1. Descriptive Analysis

Responses were coded on a numerical scale within the range [0, 1]. A rating of 1 denoted maximum preference for the setup with added vibrotactile feedback (test), while a rating of 0 indicated maximum preference for the non-vibrating setup (reference).

Figure 5 illustrates raw response data through smoothed density plots and sample means for each combination of attribute and vibration type. Vertical lines at x = 0.5 mark the point of perceived equality between the test and reference setup. With bridge vibrations, sample means slightly surpass the 0.5 point for liveliness and feel, and more notably for power. Conversely, with noise vibrations, sample means for preference and power slightly fall below 0.5, indicating no discernible benefits of added vibrotactile feedback in this condition.

3.2. Statistical Analysis

Statistical analysis was conducted using Bayesian regression [19]. In Figure 5, it is evident that the ratings exhibit a substantial presence of zeros and ones. To account for this, we employed a zero-one inflated beta (ZOIB) distribution, suitable for modeling responses in the restricted range of [0, 1], encompassing binary 0,1 outcomes [20,21]. The ZOIB distribution is characterized by four parameters: mean ($\mu$) and precision ($\varphi$) of the beta distribution, along with two inflation parameters—probability of a binary $\{0,1\}$ outcome (zoi) and conditional probability of outcome $\left\{1\right\}$ (coi). The model parameters were estimated by Bayesian inference, using the brms package [22] in the R programming environment [23].

Treating the four attributes as distinct response variables, we fitted a multivariate ZOIB model. Based on the observations from the raw data, we specified a model where the estimated means ($\mu$) of the ZOIB distribution depend on vibration type, while the remaining parameters are individually fitted to each attribute without predictors. Following the brms notation, akin to typical notation for fitting generalized linear models, our model is described as follows:

$$\begin{array}{cc}\hfill \mathrm{mvbind}(\mathrm{preference},\mathrm{power},\mathrm{liveliness},\mathrm{feel})& \sim 1+\mathrm{vibration}+\left(1\right|\mathrm{p}\left|\mathrm{participant}\right),\hfill \\ \hfill \varphi & \sim 1,\hfill \\ \hfill \mathrm{zoi}& \sim 1,\hfill \\ \hfill \mathrm{coi}& \sim 1,\hfill \end{array}$$

(1)

where mvbind() specifies the four attributes as separate response variables in a multivariate model. The term $\left(1\right|\mathrm{p}\left|\mathrm{participant}\right)$ fits varying intercepts over participants, modeled as correlated between response variables, as denoted by the letter p in the expression.

Conditional effects from the output of model 1 are shown in Figure 6 and

Table 3. Estimated mean ratings for the combinations of attribute and vibration type.

Attribute	Vibration	Estimate	l-95% CI	u-95% CI
preference	bridge	0.48	0.38	0.59
preference	noise	0.45	0.35	0.56
feel	bridge	0.54	0.44	0.63
feel	noise	0.52	0.43	0.62
power	bridge	0.56	0.46	0.66
power	noise	0.49	0.39	0.58
liveliness	bridge	0.55	0.47	0.64
liveliness	noise	0.55	0.47	0.64

, presenting estimated means and their 95% CI (CI = credible intervals, Bayesian equivalent to frequentist confidence intervals) for all combinations of attribute and vibration type. Notably, added vibrations slightly increased the estimates for feel and liveliness regardless of vibration type. Noise vibrations consistently yielded lower scores compared to bridge vibrations across attributes, with a particularly noticeable difference for perceived power, amounting to 7% of the rating scale. As seen in Table 3, however, these effects are not credibly non-zero due to high uncertainty in the estimates, as indicated by wide 95% CI encompassing the point of perceived equality (0.5). The wide estimates are by no means surprising: the statistical model reflects the inherent variability in the raw data. The causes and implications of such low prediction performance are discussed in Section 4.

As the raw data (Figure 5) suggested that the coi parameter might vary with vibrations, we fitted a second model where this parameter depends on vibration type. However, this effect was not credibly non-zero, either. Additionally, a comparison of predictive performance using the loo (leave-one-out cross validation) criterion [24] revealed that our model (1), nevertheless, describes the data somewhat better.

In terms of estimated correlations within participants (group-level terms), the model’s output shows a small positive correlation between preference and liveliness, a similar negative correlation between preference and feel, and a weaker negative correlation between preference and power (see

Table 4. (a) Group-level correlations between preference and other response variables from model (1) and (b) Spearman correlations between all response variables in raw data.

(a) Model:
Effect	Estimate	Est. Error	Q2.5	Q97.5
preference_Intercept	1.00	0.00	1.00	1.00
power_Intercept	−0.16	0.44	−0.89	0.73
liveliness_Intercept	0.27	0.42	−0.65	0.90
feel_Intercept	−0.27	0.40	−0.89	0.61
(b) Raw data:
	preference	power	liveliness	feel
preference	1.00	−0.03	0.35	−0.01
power	−0.03	1.00	0.10	0.13
liveliness	0.35	0.10	1.00	−0.22
feel	−0.01	0.13	−0.22	1.00

a). Again, these estimates exhibit high uncertainty and are, therefore, inconclusive. Spearman correlations calculated directly from the raw data mainly indicate a medium positive correlation between preference and liveliness (see Table 4b). Given these modest correlations, a univariate model with attribute as a predictor could also be considered to describe the data.

4. Discussion

The present study, involving 11 professional cellists and one type of e-cello, showed a nuanced impact of added vibrations. Assessment of feel and liveliness were moderately enhanced, with the most notable effect being increased perceived power when using vibrations derived from the bridge-signal. Conversely, noise vibrations did not yield a similar enhancement. These outcomes are intriguing when considered alongside our prior study on passive auditory and vibrotactile perception of cello performance [25], where added vibrotactile feedback significantly increased perceived arousal, both in terms of a constant boost and perceived variation, especially when the vibrations matched the auditory feedback. Drawing a connection between perceived power and arousal through loudness [26,27], our current results suggest a potential dynamic increase in arousal through the vibrotactile channel during active cello playing.

The estimated parameters of the statistical model had high uncertainty, though, and the effect of vibration type was, therefore, not shown to be credibly non-zero. Causes for this uncertainty are evident from the distributions of raw data in Figure 5, exhibiting a wide spread in the responses. In some conditions, the response distributions show a bimodality which the ZOIB model cannot always account for. In these cases, the estimated means are more separated from the peak of the corresponding density plot, notably so for power and feel with signal vibrations. The cause of this bimodality does not seem to be systematic differences of opinion between participants such as was observed in an earlier study on digital pianos [13]. Rather, both inter- and intraindividual variability were high. Multivariate intraindividual variability (IIV)—a measure of variability within participants across the four attributes—was calculated using the Frobenius norm (https://quantdev.ssri.psu.edu/sites/qdev/files/APA-ATI_ILD_Session_E_-_IntraVarMetricsComputation.html, accessed on 25 March 2024). Mean participant-specific IIV was 1.70 (sd = 0.17). Two participants’ IIV were more than one standard deviation above the mean IIV (1.97 and 1.94). Here, some more habituation with the test and response setup might have been useful. Although many participants said that the task was not difficult, they may have sometimes confused the test and reference setups as they were randomly named A and B in each trial. Furthermore, the high proportion of binary {0,1} responses may indicate that participants had difficulties giving refined responses using the foot pedal.

As is often the case in studies requiring high-skilled participants to invest their time without proper payment, our sample size was rather low (N = 11). In such cases, an alternative would be a qualitative case study. In the early development phase, we cooperated with a professional cellist who provided extensive insight and qualitative data. However, it was impossible not to disclose too many details of the experiment during the process. To prevent such sources of bias, we chose to carry out a controlled quantitative measurement with as many participants as would be available. In spite of the weaker generalization power, a detailed data analysis allowed us to better interpret the data and recognize possible sources of statistical uncertainty.

The present results show generally smaller effects than previous studies concerning a digital piano [13] and a haptic surface for musical expression [12]. A key difference is that those studies compared a haptic feedback condition with a setup lacking vibration entirely. In contrast, a silent cello inherently produces some vibrotactile feedback through its vibrating strings. Therefore, our comparison was not against a non-vibrating setup, but one with reduced vibration amplitude (see Table 2). In this respect, it is not surprising that additional vibrotactile feedback was not preferred when the vibration signal was noise. The present results, therefore, strengthen the evidence for the importance of congruent vibrotactile and auditory channels in a highly applied environment and active musical task. The observed positive effect of added bridge-signal vibrations on perceived power offers a compelling hypothesis for further research. These insights inform musical instrument technology, contributing to the understanding of the complexities of haptic feedback in digital instrument design.

5. Conclusions

Our study investigated the impact of adding vibrotactile feedback to an electric cello (e-cello) on the playing experience using four attributes: preference, power, liveliness, and feel. Vibrations driven by the piezo transducer at the bridge yielded slightly higher ratings for feel and liveliness compared to the non-vibrating setup, while noise vibrations did not show any clear benefit. However, due to wide confidence intervals in the statistical analysis, these effects were not statistically significant, suggesting high variability in individual responses.

Several factors likely contributed to this variability. First, the bimodality observed in some response distributions indicates that participants may have interpreted the attributes differently. Second, the relatively small sample size could limit the generalizability of our findings. Additionally, potential habituation to the test setup and confusion due to the naming of setups during trials might have affected responses.

By investigating the impact of vibrations on the e-cello playing experience, we hope this study contributes both knowledge and methodology towards the understanding of the complex interplay between auditory and haptic perception in a real-world musical context. Furthermore, we aim to advance the development of effective haptic feedback that enhances the expressivity and engagement of both electric and digital musical instruments. This contribution is particularly relevant for the ongoing digital transformation of traditional instruments, where haptic feedback holds the potential to bridge the gap between acoustic and digital experiences.