H-Terminated Diamond Surface Band Bending Characterization by Angle-Resolved XPS
Abstract
:1. Introduction
2. Materials and Methods
3. Results
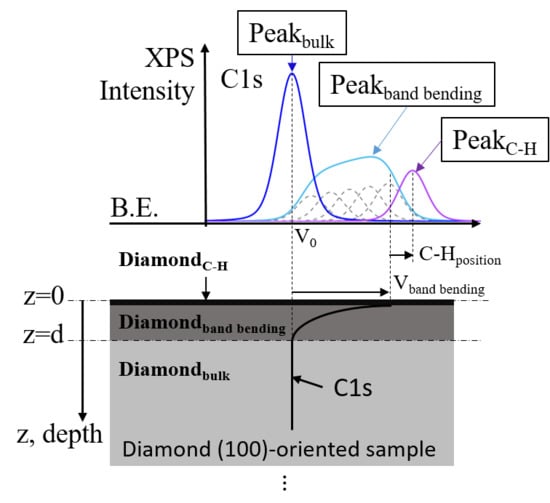
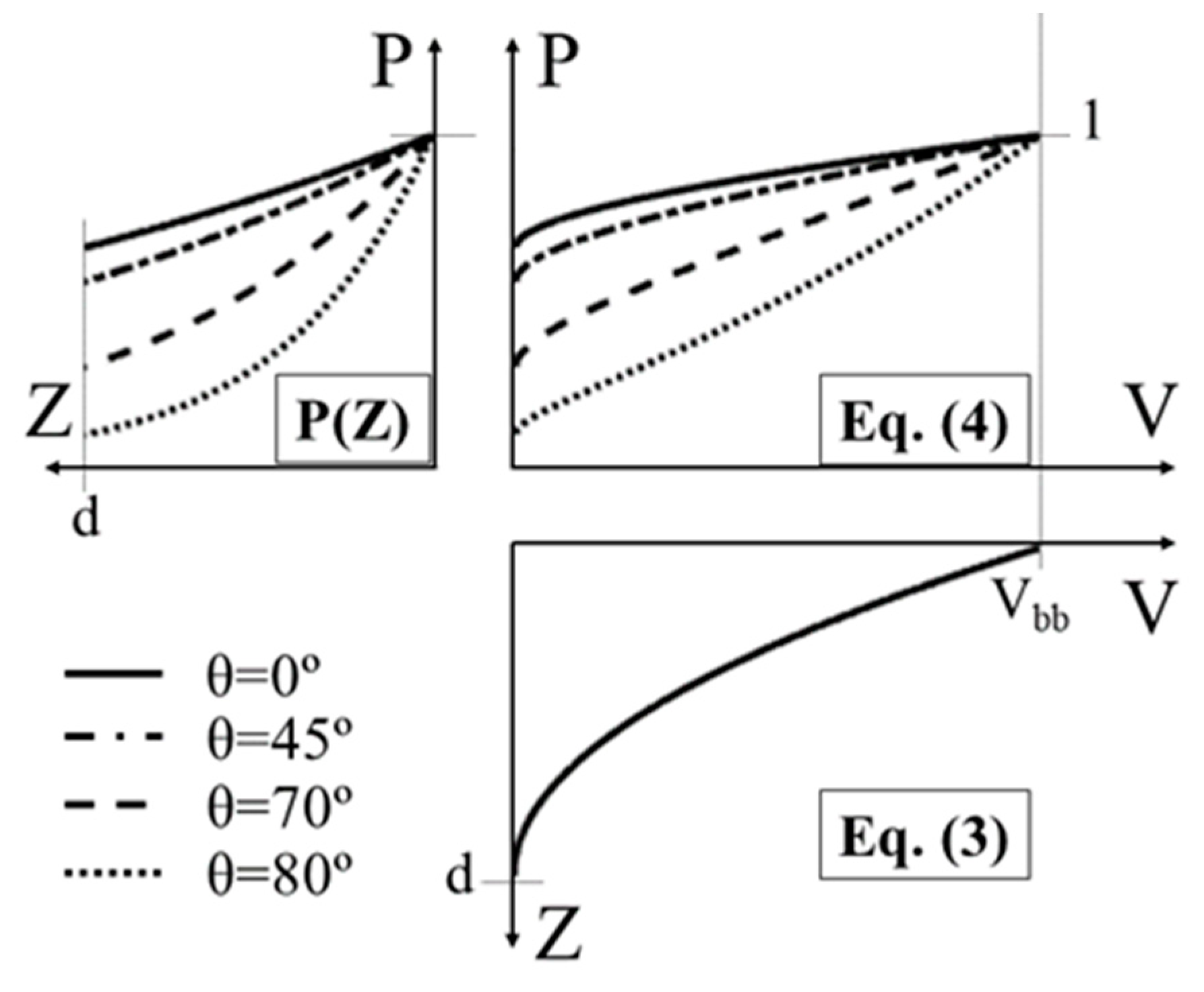
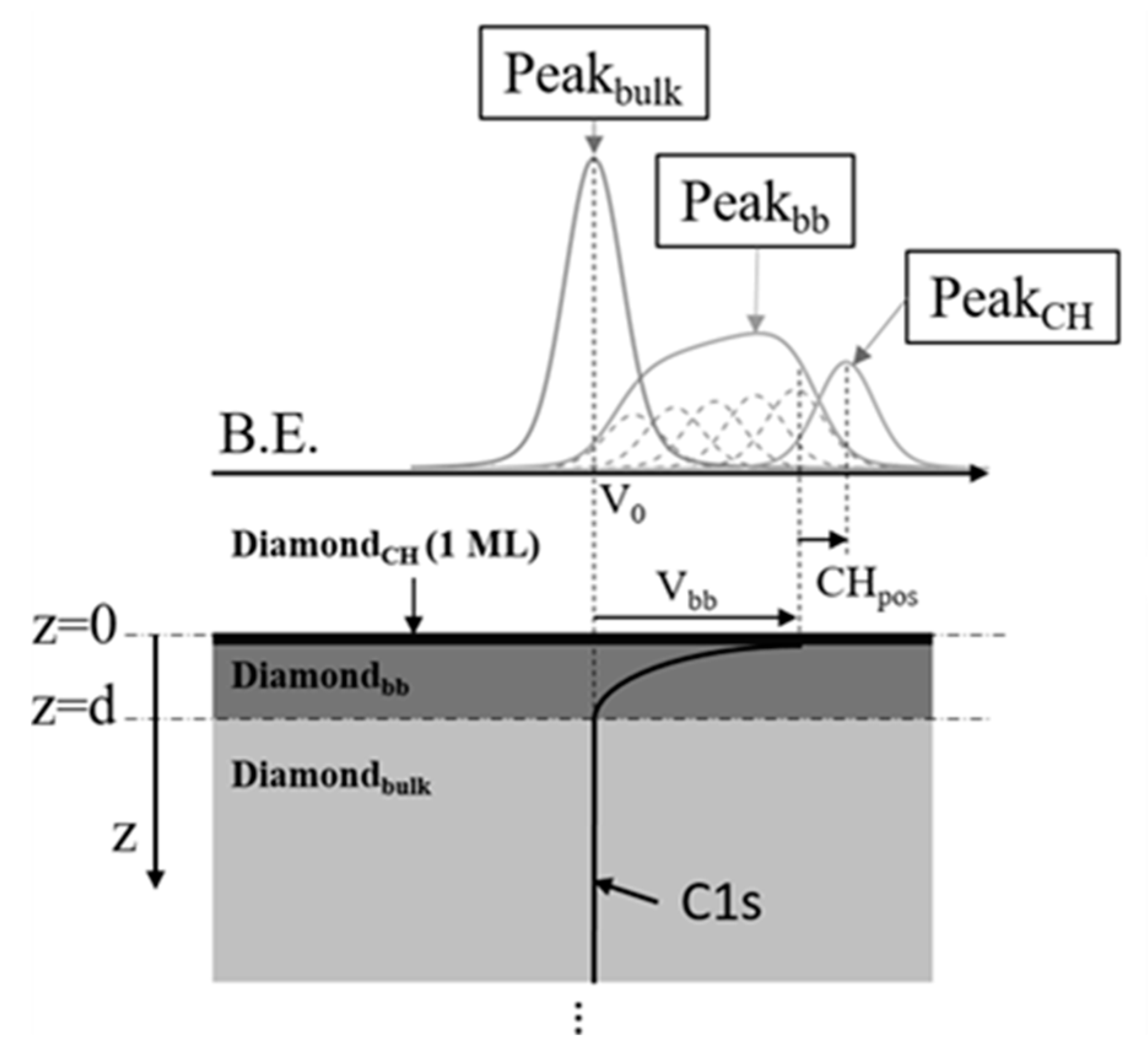
3.1. Shape Generation of a Band Bending XPS Contribution
3.2. Surface Band Bending Estimation
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Balmer, R.S.; Friel, I.; Woollard, S.M.; Wort, C.J.H.; Scarsbrook, G.A.; Coe, S.E.; El-Hajj, H.; Kaiser, A.; Denisenko, A.; Kohn, E.; et al. Unlocking diamond’s potential as an electronic material. Philos. Trans. A Math. Phys. Eng. Sci. 2008, 366, 251–265. [Google Scholar] [CrossRef]
- Denisenko, A.T.; Kohn, E. Diamond power devices. Concepts and limits. Diam. Relat. Mater. 2005, 14, 491–498. [Google Scholar] [CrossRef]
- Muret, P.; Pruvost, F.; Saby, C.; Lucazeau, E.; Tan, T.A.N.; Gheeraert, E.; Deneuville, A. Carbide contacts on homoepitaxial diamond films. Diam. Relat. Mater. 1999, 8, 961–965. [Google Scholar] [CrossRef]
- Pernot, J.; Chicot, G.; Fiori, A.; Traore, A.; Thi, T.N.T.; Volpe, P.-N.; Eon, D.; Omnès, F.; Bustarret, E.; Gheeraert, E.; et al. Recent progress of diamond device toward power application. In Proceedings of the EXMATEC 2012: 11th Expert Evaluation and Control of Compound Semiconductor Materials and Technologies conference, Porquerolles Islands, France, 30 May–1 June 2012. [Google Scholar]
- Kohn, E.; Denisenko, A. Concepts for diamond electronics. Thin Solid Films 2007, 515, 4333–4339. [Google Scholar] [CrossRef]
- Piñero, J.C.; Araujo, D.; Fiori, A.; Traore, A.; Villar, M.P.; Eon, D.; Muret, P.; Pernot, J.; Teraji, T. Atomic composition of WC/ and Zr/O-terminated diamond Schottky interfaces close to ideality. Appl. Surf. Sci. 2017, 395, 200–207. [Google Scholar] [CrossRef]
- Traoré, A.; Muret, P.; Fiori, A.; Eon, D.; Gheeraert, E.; Pernot, J.; Muret, P.; Fiori, A.; Eon, D.; Gheeraert, E.; et al. Zr/oxidized diamond interface for high power Schottky diodes. Appl. Phys. Lett. 2014, 104, 052105. [Google Scholar] [CrossRef]
- Maréchal, A.; Kato, Y.; Liao, M.; Koizumi, S. Interfacial energy barrier height of Al2O3/H-terminated (111) diamond heterointerface investigated by X-ray photoelectron spectroscopy. Appl. Phys. Lett. 2017, 111, 141605. [Google Scholar] [CrossRef] [Green Version]
- Umezawa, H.; Saito, T.; Tokuda, N.; Ogura, M.; Ri, S.G.; Yoshikawa, H.; Shikata, S.I. Leakage current analysis of diamond Schottky barrier diode. Appl. Phys. Lett. 2007, 90, 28–31. [Google Scholar] [CrossRef] [Green Version]
- Umezawa, H. Recent advances in diamond power semiconductor devices. Mater. Sci. Semicond. Process. 2018, 78, 147–156. [Google Scholar] [CrossRef]
- Thoms, B.D.; Butler, J.E. Hreels and Leed of H/C(100): The 2 × 1 monohydride dimer row reconstruction. Surf. Sci. 1995, 328, 291–301. [Google Scholar] [CrossRef]
- Sato, H.; Kasu, M. Maximum hole concentration for Hydrogen-terminated diamond surfaces with various surface orientations obtained by exposure to highly concentrated NO2. Diam. Relat. Mater. 2013, 31, 47–49. [Google Scholar] [CrossRef]
- Wade, T.; Geis, M.W.; Fedynyshyn, T.H.; Vitale, S.A.; Varghese, J.O.; Lennon, D.M.; Grotjohn, T.A.; Nemanich, R.J.; Hollis, M.A. Effect of surface roughness and H–termination chemistry on diamond’s semiconducting surface conductance. Diam. Relat. Mater. 2017, 76, 79–85. [Google Scholar] [CrossRef]
- Chen, W.; Qi, D.; Gao, X.; Wee, A.T.S. Surface transfer doping of semiconductors. Prog. Surf. Sci. 2009, 84, 279–321. [Google Scholar] [CrossRef]
- Ristein, J. Surface science of diamond: Familiar and amazing. Surf. Sci. 2006, 600, 3677–3689. [Google Scholar] [CrossRef]
- Maier, F.; Riedel, M.; Mantel, B.; Ristein, J.; Ley, L. Origin of Surface Conductivity in Diamond. Phys. Rev. Lett. 2000, 85, 14–17. [Google Scholar] [CrossRef]
- Kono, S.; Saito, T.; Kang, S.H.; Jung, W.Y.; Kim, B.Y.; Kawata, H.; Goto, T.; Kakefuda, Y.; Yeom, H.W. Band diagram for chemical vapor deposition diamond surface conductive layer: Presence of downward band bending due to shallow acceptors. Surf. Sci. 2010, 604, 1148–1165. [Google Scholar] [CrossRef]
- Kono, S.; Sawabe, A.; Kodama, H.; Hayashi, Y.; Kageura, T.; Ogura, M.; Inaba, M.; Kawarada, H.; Teraji, T.; Ri, S.-G.; et al. Carbon 1s X-ray photoelectron spectra of realistic samples of hydrogen-terminated and oxygen-terminated CVD diamond (111) and (001). Diam. Relat. Mater. 2019, 93, 105–130. [Google Scholar] [CrossRef]
- Hamza, A.V.; Kubiak, G.D.; Stulen, R.H. Hydrogen chemisorption and the structure of the diamond C (100) − (2 × 1) surface. Surf. Sci. 1990, 237, 35–52. [Google Scholar] [CrossRef]
- Kanai, C.; Shichibu, Y.; Watanabe, K.; Takakuwa, Y. Ab initio study on surface segregation of hydrogen from diamond C(100) surfaces. Phys. Rev. B 2002, 65, 153312. [Google Scholar] [CrossRef]
- Kanai, C.; Watanabe, K.; Takakuwa, Y. Ab initio study on the electronic states of hydrogen defects in diamond subsurfaces. Jpn. J. Appl. Phys. 2003, 42, 3510. [Google Scholar] [CrossRef]
- Kono, S.; Takano, T.; Shimomura, M.; Goto, T.; Sato, K.; Abukawa, T.; Tachiki, M.; Kawarada, H. Electron-spectroscopy and -diffraction study of the conductivity of CVD diamond (2 × 1) surface. Surf. Sci. 2003, 529, 180–188. [Google Scholar] [CrossRef]
- David, D.G.F.; Pinault-Thaury, M.A.; Ballutaud, D.; Godet, C. Sensitivity of photoelectron energy loss spectroscopy to surface reconstruction of microcrystalline diamond films. Appl. Surf. Sci. 2013, 273, 607–612. [Google Scholar] [CrossRef] [Green Version]
- Navas, J.; Araujo, D.; Piñero, J.C.; Sánchez-Coronilla, A.; Blanco, E.; Villar, P.; Alcántara, R.; Montserrat, J.; Florentin, M.; Eon, D.; et al. Oxygen termination of homoepitaxial diamond surface by ozone and chemical methods: An experimental and theoretical perspective. Appl. Surf. Sci. 2018, 433, 408–418. [Google Scholar] [CrossRef]
- Kozak, H.; Kromka, A.; Ukraintsev, E.; Houdkova, J.; Ledinsky, M.; Vaněček, M.; Rezek, B. Detecting sp2 phase on diamond surfaces by atomic force microscopy phase imaging and its effects on surface conductivity. Diam. Relat. Mater. 2009, 18, 722–725. [Google Scholar] [CrossRef]
- Speranza, G.; Torrengo, S.; Miotello, A.; Minati, L.; Bernagozzi, I.; Ferrari, M.; Dipalo, M.; Kohn, E. XPS and UPS in situ study of oxygen thermal desorption from nanocrystalline diamond surface oxidized by different process. Diam. Relat. Mater. 2011, 20, 560–563. [Google Scholar] [CrossRef]
- Maier, F.; Graupner, R.; Hollering, M.; Hammer, L.; Ristein, J.; Ley, L. Hydrogenated and bare diamond (110) surface: A combined LEED-, XPS-, and ARPES study. Surf. Sci. 1999, 443, 177–185. [Google Scholar] [CrossRef]
- Kono, S.; Teraji, T.; Kodama, H.; Ichikawa, K.; Ohnishi, S.; Sawabe, A. Direct determination of the barrier height of Ti-based ohmic contact on p-type diamond (001). Diam. Relat. Mater. 2015, 60, 117–122. [Google Scholar] [CrossRef]
- Nečas, D.; Klapetek, P. Gwyddion: An open-source software for SPM data analysis. Cent. Eur. J. Phys. 2012, 10, 181–188. [Google Scholar] [CrossRef]
- Tougaard, S.; Simund, P. Elastic and inelastic scattering of electrons reflected from solids: Effects on energy spectra. Phys. Rev. B 1982, 25, 4452–4466. [Google Scholar] [CrossRef]
- Graupner, R.; Maier, F.; Ristein, J.; Ley, L.; Jung, C. High-resolution surface-sensitive C core-level spectra of clean and hydrogen-terminated diamond (100) and (111) surfaces. Phys. Rev. B 1998, 57, 12397–12409. [Google Scholar] [CrossRef]
- Chen, C.T.; Sette, F. High Resolution Soft X-ray Spectroscopies with the Dragon Beamline. Phys. Scr. 1990, 1990, 119–126. [Google Scholar] [CrossRef]
- Fadley, C.S. Angle-resolved X-ray photoelectron spectroscopy. Prog. Surf. Sci. 1984, 16, 275–388. [Google Scholar] [CrossRef]
- Kono, S.; Saitou, T.; Kawata, H.; Goto, T.; Kakefuda, Y.; Komeda, T. Characteristic energy band values and electron attenuation length of a chemical-vapor-deposition diamond (0 0 1) 2 × 1 surface. Surf. Sci. 2009, 603, 860–866. [Google Scholar] [CrossRef]
- Kasu, M.; Ueda, K.; Yamauchi, Y.; Makimoto, T. Gate capacitance-voltage characteristics of submicron-long-gate diamond field-effect transistors with hydrogen surface termination. Appl. Phys. Lett. 2007, 90, 5–8. [Google Scholar] [CrossRef]
- Kimura, K.; Nakajima, K.; Yamanaka, S.; Hasegawa, M.; Okushi, H. Hydrogen depth-profiling in chemical-vapor-deposited diamond films by high-resolution elastic recoil detection. Appl. Phys. Lett. 2001, 78, 1679–1681. [Google Scholar] [CrossRef] [Green Version]



© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alba, G.; Eon, D.; Villar, M.P.; Alcántara, R.; Chicot, G.; Cañas, J.; Letellier, J.; Pernot, J.; Araujo, D. H-Terminated Diamond Surface Band Bending Characterization by Angle-Resolved XPS. Surfaces 2020, 3, 61-71. https://doi.org/10.3390/surfaces3010007
Alba G, Eon D, Villar MP, Alcántara R, Chicot G, Cañas J, Letellier J, Pernot J, Araujo D. H-Terminated Diamond Surface Band Bending Characterization by Angle-Resolved XPS. Surfaces. 2020; 3(1):61-71. https://doi.org/10.3390/surfaces3010007
Chicago/Turabian StyleAlba, Gonzalo, David Eon, M. Pilar Villar, Rodrigo Alcántara, Gauthier Chicot, Jesús Cañas, Juliette Letellier, Julien Pernot, and Daniel Araujo. 2020. "H-Terminated Diamond Surface Band Bending Characterization by Angle-Resolved XPS" Surfaces 3, no. 1: 61-71. https://doi.org/10.3390/surfaces3010007
APA StyleAlba, G., Eon, D., Villar, M. P., Alcántara, R., Chicot, G., Cañas, J., Letellier, J., Pernot, J., & Araujo, D. (2020). H-Terminated Diamond Surface Band Bending Characterization by Angle-Resolved XPS. Surfaces, 3(1), 61-71. https://doi.org/10.3390/surfaces3010007