Analytical Modeling of the Temporal Evolution of Epidemics Outbreaks Accounting for Vaccinations
Abstract
:1. Introduction
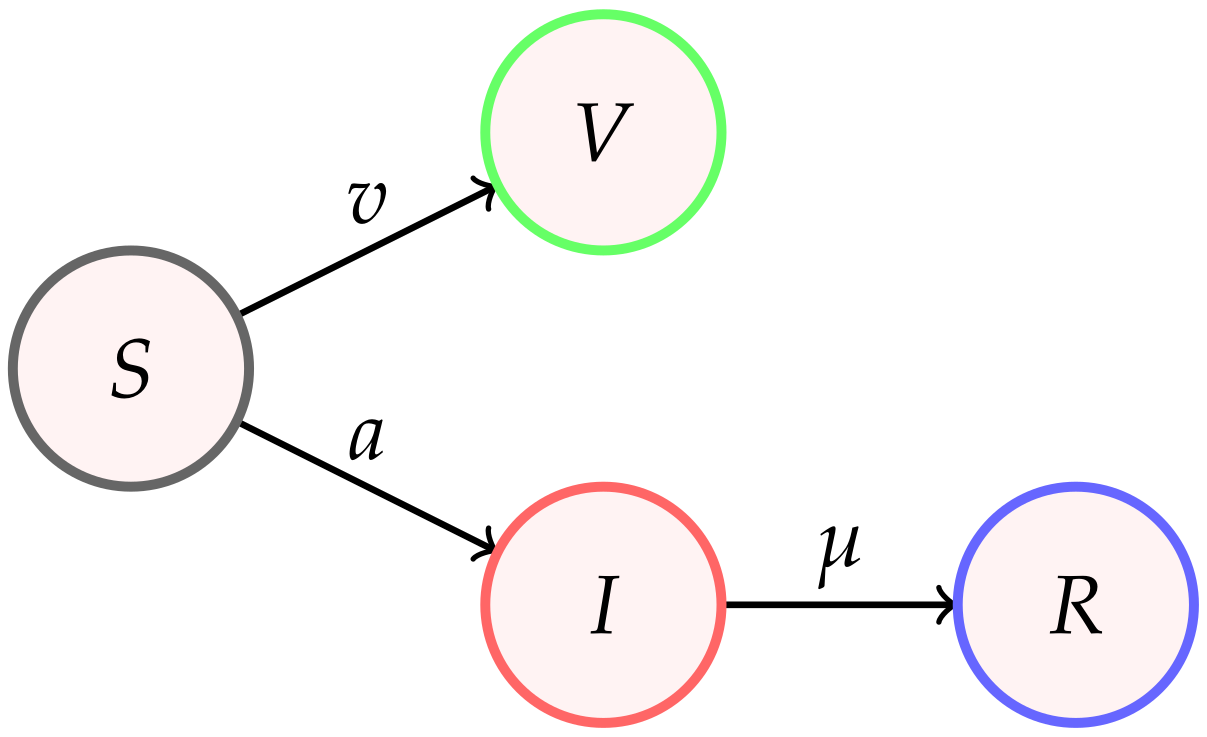
2. General SIRV Equations
2.1. Condition for Pandemic Outburst
- (i)
- If initially more than 50 percent () are infectious, no new pandemic outburst will occur. However, such high values of are unlikely and unrealistic.
- (ii)
- For small given values of and the ratio of recovered to infection rate k, new emerging outbreaks can be fully prevented for values of the ratio of vaccination to infection rate . The more pathogenic a virus mutation is, the smaller and closer to zero is the value of the ratio k so that the lower limit for b has to be close to unity to prevent a new outburst.
- (iii)
- For any finite value of for modeling epidemic outbreaks the relevant range of the two parameters k and b is .
- (iv)
- As an aside comment, let us note that Equation (12) demonstrates that in the SIR model with (no vaccination), a pandemic does not occur if the parameter . The SIR model correctly indicates that epidemic waves end in the case . Therefore, the recent criticism [120] about the SIR model is inappropriate and misguided.
2.2. Reduced Time
3. Dynamics of the Epidemics
3.1. Summary of Results
3.2. Two Useful Functions
3.3. Mathematical Analysis
3.4. Inverse Solution for the General Case
3.5. Determination of the Minimum Value for
4. Approximated Reduction of the Exact Solution
4.1. Approximate Inverse Solution
4.2. Approximate Direct Solution
4.3. Time-Dependency of All Remaining SIRV Quantities
4.4. Critical Reduced Vaccination Rate
4.5. Peak Times and Peak Amplitudes
4.6. Total Fraction of Infected Persons
4.7. Differential Rate
4.8. Time Scales
5. Comparison of Approximate with Exact Solutions
6. Application to Real Data
7. Summary and Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Range of Application for the Two Lambert Functions

Appendix B. The Critical Vaccination Rate b c
| k | |||
|---|---|---|---|
Appendix C. Proof of Equation (72)
Appendix D. Proofs of Equations (102) and (103)
Appendix E. Cumulative Fraction of Infected Persons J(τ) for Arbitrary η
Appendix F. Peak Time and Amplitude for b ≥ bc and Arbitrary η
Special Case of bc ≤ b ≪ k
Appendix G. Exact Solutions for Special Cases
Appendix G.1. The Equal Value Case b = k Corresponding to α = 0
Appendix G.2. SIR-Case b = 0, k > 0
Alternative Inverse Solution
Appendix G.3. SIV-Case b > 0, k = 0
Appendix G.3.1. Symmetry Argument
Appendix G.3.2. Alternative Inverse Solution
References
- Wang, Z.; Bausch, C.T.; Bhattacharyya, S.; d’Onofrio, A.; Manfredi, P.; Perc, M.; Perra, N.; Salathe, M.; Zhao, D.W. Statistical physics of vaccination. Phys. Rep. 2016, 664, 1–113. [Google Scholar] [CrossRef]
- Cadoni, M.; Gaeta, G. Size and timescale of epidemics in the SIR framework. Phys. D 2020, 411, 132626. [Google Scholar] [CrossRef] [PubMed]
- Chekroun, A.; Kuniya, T. Global threshold dynamics of aninfection age-structured SIR epidemic model with diffusion under the Dirichlet boundary condition. J. Differ. Equ. 2020, 269, 117–148. [Google Scholar] [CrossRef]
- Imron, C.; Hariyanto; Yunus, M.; Surjanto, S.D.; Dewi, N.A.C. Stability and persistence analysis on the epidemic model multi-region multi-patches. J. Phys. Conf. Ser. 2019, 1218, 012035. [Google Scholar] [CrossRef]
- Karaji, P.T.; Nyamoradi, N. Analysis of a fractional SIR model with general incidence function. Appl. Math. Lett. 2020, 108, 106499. [Google Scholar] [CrossRef]
- Mohamadou, Y.; Halidou, A.; Kapen, P.T. A review of mathematical modeling, artificial intelligence and datasets used in the study, prediction and management of COVID-19. Appl. Intell. 2020. [Google Scholar] [CrossRef]
- Samanta, S.; Sahoo, B.; Das, B. Dynamics of an epidemic system with prey herd behavior and alternative resource to predator. J. Phys. A 2019, 52, 425601. [Google Scholar] [CrossRef]
- Sene, N. SIR epidemic model with Mittag-Leffler fractional derivative. Chaos Solitons Fractals 2020, 137, 109833. [Google Scholar] [CrossRef]
- Simon, M. SIR epidemics with stochastic infectious periods. Stoch. Proc. Appl. 2020, 130, 4252–4274. [Google Scholar] [CrossRef]
- Tian, C.R.; Zhang, Q.Y.; Zhang, L. Global stability in a networked SIR epidemic model. Appl. Math. Lett. 2020, 107, 106444. [Google Scholar] [CrossRef]
- El Koufi, A.; Adnani, J.; Bennar, A.; Yousfi, N. Analysis of a stochastic SIR model with vaccination and nonlinear incidence rate. Int. J. Diff. Equ. 2019, 2019, 9275051. [Google Scholar] [CrossRef] [Green Version]
- Houy, N. Are better vaccines really better? The case of a simple stochastic epidemic SIR model. Econ. Bull. 2013, 33, 207–216. [Google Scholar]
- Jornet-Sanz, M.; Corberan-Vallet, A.; Santonja, F.J.; Villanueva, R.J. A bayesian stochastic SIRS model with a vaccination strategy for the analysis of respiratory syncytial virus. Stat. Oper. Res. Trans. 2017, 41, 159–175. [Google Scholar]
- Li, X.N.; Zhang, Q.M. Time to extinction and stationary distribution of a stochastic susceptible-infected-recovered-susceptible model with vaccination under markov switching. Math. Popul. Stud. 2020, 27, 259–274. [Google Scholar] [CrossRef]
- Liu, Q.; Jiang, D.Q. The threshold of a stochastic delayed SIR epidemic model with vaccination. Phys. A 2016, 461, 140–147. [Google Scholar] [CrossRef]
- Liu, Q.; Jiang, D.Q.; Shi, N.Z.; Hayat, T. Dynamics of a stochastic delayed SIR epidemic model with vaccination and double diseases driven by levy jumps. Phys. A 2018, 492, 2010–2018. [Google Scholar] [CrossRef]
- Miao, A.Q.; Zhang, J.; Zhang, T.Q.; Pradeep, B. Threshold dynamics of a stochastic SIR model with vertical transmission and vaccination. Comput. Math. Meth. Med. 2017, 2017, 4820183. [Google Scholar] [CrossRef] [Green Version]
- Nguyen, C.; Carlson, J.M. Optimizing real-time vaccine allocation in a stochastic SIR model. PLoS ONE 2016, 11, 0152950. [Google Scholar] [CrossRef]
- Wang, F.Y.; Wang, X.Y.; Zhang, S.W.; Ding, C.M. On pulse vaccine strategy in a periodic stochastic SIR epidemic model. Chaos Solitons Fractals 2014, 66, 127–135. [Google Scholar] [CrossRef]
- Wang, L.; Teng, Z.D.; Tang, T.T.; Li, Z.M. Threshold dynamics in stochastic SIRS epidemic models with nonlinear incidence and vaccination. Comput. Math. Meth. Med. 2017, 2017, 7294761. [Google Scholar] [CrossRef] [Green Version]
- Witbooi, P.J. Stability of a stochastic model of an SIR epidemic with vaccination. Acta Biotheor. 2017, 65, 151–165. [Google Scholar] [CrossRef] [PubMed]
- Xu, C.Y.; Li, X.Y. The threshold of a stochastic delayed SIRS epidemic model with temporary immunity and vaccination. Chaos Solitons Fractals 2018, 111, 227–234. [Google Scholar] [CrossRef]
- Zhang, Y.; Li, Y.; Zhang, Q.L.; Li, A.H. Behavior of a stochastic SIR epidemic model with saturated incidence and vaccination rules. Phys. A 2018, 501, 178–187. [Google Scholar] [CrossRef]
- Zhao, X.; He, X.; Feng, T.; Qiu, Z.P. A stochastic switched SIRS epidemic model with nonlinear incidence and vaccination: Stationary distribution and extinction. Int. J. Biomath. 2020, 13, 2050020. [Google Scholar] [CrossRef]
- Colombo, R.M.; Garavello, M. Optimizing vaccination strategies in an age structured SIR model. Math. Biosci. Eng. 2020, 17, 1074–1089. [Google Scholar] [CrossRef]
- Cui, Q.Q.; Xu, J.B.; Zhang, Q.; Wang, K. An nsfd scheme for sir epidemic models of childhood diseases with constant vaccination strategy. Adv. Differ. Equ. 2014, 2014, 172. [Google Scholar] [CrossRef] [Green Version]
- D’Onofrio, A. Pulse vaccination strategy in the SIR epidemic model: Global asymptotic stable eradication in presence of vaccine failures. Math. Comput. Model. 2002, 36, 473–489. [Google Scholar] [CrossRef]
- d’Onofrio, A. On pulse vaccination strategy in the SIR epidemic model with vertical transmission. Appl. Math. Lett. 2005, 18, 729–732. [Google Scholar] [CrossRef] [Green Version]
- Gao, S.J.; Ouyang, H.S.; Nieto, J.J. Mixed vaccination strategy in SIRS epidemic model with seasonal variability on infection. Int. J. Biomath. 2011, 4, 473–491. [Google Scholar] [CrossRef]
- Gao, S.J.; Xie, D.H.; Chen, L.S. Pulse vaccination strategy in a delayed SIR epidemic model with vertical transmission. Discr. Contin. Dyn. Syst. B 2007, 7, 77–86. [Google Scholar] [CrossRef]
- Kabir, K.M.A.; Tanimoto, J. Vaccination strategies in a two-layer sir/v-ua epidemic model with costly information and buzz effect. Commun. Nonlin. Sci. Numer. Simul. 2019, 76, 92–108. [Google Scholar] [CrossRef]
- Li, J.Q.; Yang, Y.L. SIR-SVS epidemic models with continuous and impulsive vaccination strategies. J. Theor. Biol. 2011, 280, 108–116. [Google Scholar] [CrossRef]
- Liu, H.L.; Yu, J.Y.; Zhu, G.T. Global stability of an age-structured SIR epidemic model with pulse vaccination strategy. J. Syst. Sci. Complex. 2012, 25, 417–429. [Google Scholar] [CrossRef]
- Liu, L.; Luo, X.F.; Chang, L.L. Vaccination strategies of an SIR pair approximation model with demographics on complex networks. Chaos Solitons Fractals 2017, 104, 282–290. [Google Scholar] [CrossRef]
- Makinde, O.D. Adomian decomposition approach to a SIR epidemic model with constant vaccination strategy. Appl. Math. Comput. 2007, 184, 842–848. [Google Scholar] [CrossRef]
- Meng, X.Z.; Chen, L.S. The dynamics of a new SIR epidemic model concerning pulse vaccination strategy. Appl. Math. Comput. 2008, 197, 582–597. [Google Scholar] [CrossRef]
- Moneim, I.A.; Greenhalgh, D. Threshold and stability results for an SIRS epidemic model with a general periodic vaccination strategy. J. Biol. Syst. 2005, 13, 131–150. [Google Scholar] [CrossRef]
- Mu, X.J.; Zhang, Q.M.; Rong, L.B. Optimal vaccination strategy for an SIRS model with imprecise parameters and levy noise. J. Franklin Inst. Eng. Appl. Math. 2019, 356, 11385–11413. [Google Scholar] [CrossRef]
- Mungkasi, S. Variational iteration and successive approximation methods for a SIR epidemic model with constant vaccination strategy. Appl. Math. Model. 2021, 90, 1–10. [Google Scholar] [CrossRef]
- Pei, Y.Z.; Liu, S.Y.; Chen, L.S.; Wang, C.H. Two different vaccination strategies in an SIR epidemic model with saturated infectious force. Int. J. Biomath. 2008, 1, 147–160. [Google Scholar] [CrossRef]
- Rashidinia, J.; Sajjadian, M.; Duarte, J.; Januario, C.; Martins, N. On the dynamical complexity of a seasonally forced discrete SIR epidemic model with a constant vaccination strategy. Complexity 2018, 2018, 7191487. [Google Scholar] [CrossRef]
- Terry, A.J. PULSE vaccination strategies in a metapopulation SIR model. Math. Biosci. Eng. 2010, 7, 455–477. [Google Scholar] [PubMed]
- Zhou, L.H.; Wang, Y.; Xiao, Y.Y.; Li, M.Y. Global dynamics of a discrete age-structured SIR epidemic model with applications to measles vaccination strategies. Math. Biosci. 2019, 308, 27–37. [Google Scholar] [CrossRef] [PubMed]
- Karatayev, V.A.; Anand, M.; Bauch, C.T. Local lockdowns outperform global lockdown on the far side of the COVID-19 epidemic curve. Proc. Natl. Acad. Sci. USA 2020, 117, 24575–24580. [Google Scholar] [CrossRef]
- Wang, X.; Jia, D.; Gao, S.; Xia, C.; Li, X.; Wang, Z. Vaccination behavior by coupling the epidemic spreading with the human decision under the game theory. Appl. Math. Comput. 2020, 380, 125232. [Google Scholar] [CrossRef]
- Priesemann, V.; Balling, R.; Brinkmann, M.M.; Ciesek, S.; Czypionka, T.; Eckerle, I.; Giordano, G.; Hanson, C.; Hel, Z.; Hotulainen, P.; et al. An action plan for pan-European defence against new SARS-CoV-2 variants. Lancet 2021, 397, 469–470. [Google Scholar] [CrossRef]
- Priesemann, V.; Brinkmann, M.M.; Ciesek, S.; Cuschieri, S.; Czypionka, T.; Giordano, G.; Gurdasani, D.; Hanson, C.; Hens, N.; Iftekhar, E.; et al. Calling for pan-European commitment for rapid and sustained reduction in SARS-CoV-2 infections. Lancet 2021, 397, 92–93. [Google Scholar] [CrossRef]
- Zhou, Y.G.; Yang, K.; Zhou, K.; Liang, Y.T. Optimal vaccination policies for an SIR model with limited resources. Acta Biotheor. 2014, 62, 171–181. [Google Scholar] [CrossRef]
- Abouelkheir, I.; El Kihal, F.; Rachik, M.; Elmouki, I. Optimal impulse vaccination approach for an SIR control model with short-term immunity. Mathematics 2019, 7, 420. [Google Scholar] [CrossRef] [Green Version]
- Church, K.E.M.; Liu, X.Z. Analysis of a SIR model with pulse vaccination and temporary immunity: Stability, bifurcation and a cylindrical attractor. Nonlinear Anal. Real World Appl. 2019, 50, 240–266. [Google Scholar] [CrossRef]
- Gao, S.J.; Teng, Z.D.; Xie, D.H. Analysis of a delayed SIR epidemic model with pulse vaccination. Chaos Solitons Fractals 2009, 40, 1004–1011. [Google Scholar] [CrossRef]
- Gao, S.J.; Zhidong, Z.D.; Nieto, J.J.; Torres, A. Analysis of an SIR epidemic model with pulse vaccination and distributed time delay. J. Biomed. Biotechn. 2007, 2007, 64870. [Google Scholar] [CrossRef]
- He, Z.L.; Nie, L.F. The effect of pulse vaccination and treatment on SIR epidemic model with media impact. Discr. Dyn. Nat. Soc. 2015, 2015, 532494. [Google Scholar] [CrossRef] [Green Version]
- Jiang, G.R.; Yang, Q.G. Bifurcation analysis in an SIR epidemic model with birth pulse and pulse vaccination. Appl. Math. Comput. 2009, 215, 1035–1046. [Google Scholar] [CrossRef]
- Liu, X.S.; Dai, B.X. Qualitative and bifurcation analysis of an SIR epidemic model with saturated treatment function and nonlinear pulse vaccination. Discr. Dyn. Nat. Soc. 2016, 2016, 9146481. [Google Scholar] [CrossRef]
- Liu, X.S.; Dai, B.X. Flip bifurcations of an SIR epidemic model with birth pulse and pulse vaccination. Appl. Math. Model. 2017, 43, 579–591. [Google Scholar] [CrossRef]
- Lu, Z.H.; Chi, X.B.; Chen, L.S. The effect of constant and pulse vaccination on SIR epidemic model with horizontal and vertical transmission. Math. Comput. Model. 2002, 36, 1039–1057. [Google Scholar] [CrossRef]
- Meng, X.Z.; Chen, L.S. Global dynamical behaviors for an SIR epidemic model with time delay and pulse vaccination. Taiwan. J. Math. 2008, 12, 1107–1122. [Google Scholar] [CrossRef]
- Meng, X.Z.; Chen, L.S.; Wu, B. A delay SIR epidemic model with pulse vaccination and incubation times. Nonlin. Anal. Real World Appl. 2010, 11, 88–98. [Google Scholar] [CrossRef]
- Nie, L.F.; Teng, Z.D.; Torres, A. Dynamic analysis of an SIR epidemic model with state dependent pulse vaccination. Nonlin. Anal. Real World Appl. 2012, 13, 1621–1629. [Google Scholar] [CrossRef]
- Pang, G.P.; Chen, L.S. A delayed SIRS epidemic model with pulse vaccination. Chaos Solitons Fractals 2007, 34, 1629–1635. [Google Scholar] [CrossRef]
- Qin, W.J.; Tang, S.Y.; Cheke, R.A. Nonlinear pulse vaccination in an SIR epidemic model with resource limitation. Abstr. Appl. Anal. 2013, 2013, 670263. [Google Scholar] [CrossRef]
- Sekiguchi, M.; Ishiwata, E. Dynamics of a discretized SIR epidemic model with pulse vaccination and time delay. J. Comput. Appl. Math. 2011, 236, 997–1008. [Google Scholar] [CrossRef] [Green Version]
- Stone, L.; Shulgin, B.; Agur, Z. Theoretical examination of the pulse vaccination policy in the SIR epidemic model. Math. Comput. Model. 2000, 31, 207–215. [Google Scholar] [CrossRef]
- Wang, L. Existence of periodic solutions of seasonally forced SIR models with impulse vaccination. Taiwan. J. Math. 2015, 19, 1713–1729. [Google Scholar] [CrossRef]
- Zhang, X.B.; Huo, H.F.; Sun, X.K.; Fu, Q. The differential susceptibility SIR epidemic model with time delay and pulse vaccination. J. Appl. Math. Comput. 2010, 34, 287–298. [Google Scholar] [CrossRef]
- Zhang, X.B.; Huo, H.F.; Sun, X.K.; Fu, Q.A. The differential susceptibility SIR epidemic model with stage structure and pulse vaccination. Nonlin. Anal. Real World Appl. 2010, 11, 2634–2646. [Google Scholar] [CrossRef]
- Zhang, X.B.; Huo, H.F.; Xiang, H.; Meng, X.Y. An SIRS epidemic model with pulse vaccination and non-monotonic incidence rate. Nonlin. Anal. Hybrid Syst. 2013, 8, 13–21. [Google Scholar] [CrossRef]
- Zhao, W.C.; Li, J.; Meng, X.Z. Dynamical analysis of SIR epidemic model with nonlinear pulse vaccination and lifelong immunity. Discret. Dyn. Nat. Soc. 2015, 2015, 848623. [Google Scholar] [CrossRef]
- Zhao, Z.; Pang, L.Y.; Chen, Y. Nonsynchronous bifurcation of SIRS epidemic model with birth pulse and pulse vaccination. Nonlin. Dyn. 2015, 79, 2371–2383. [Google Scholar] [CrossRef]
- Zhou, A.R.; Sattayatham, P.; Jiao, J.J. Dynamics of an SIR epidemic model with stage structure and pulse vaccination. Adv. Diff. Equ. 2016, 2016, 140. [Google Scholar] [CrossRef] [Green Version]
- d’Onofrio, A.; Manfredi, P.; Salinelli, E. Bifurcation thresholds in an SIR model with information-dependent vaccination. Math. Model. Nat. Phenom. 2007, 2, 26–43. [Google Scholar] [CrossRef]
- Gumus, O.A.K.; Selvam, A.G.M.; Vianny, D.A. Bifucaction and stability analysis of a discrete time SIR epidemic model with vaccination. Int. J. Anal. Appl. 2019, 17, 809–820. [Google Scholar]
- Rostamy, D.; Mottaghi, E. Forward and backward bifurcation in a fractional-order SIR epidemic model with vaccination. Iran. J. Sci. Technol. Trans. A 2018, 42, 663–671. [Google Scholar] [CrossRef]
- Zhang, Q.Q.; Tang, B.; Tang, S.Y. Vaccination threshold size and backward bifurcation of SIR model with state-dependent pulse control. J. Theor. Biol. 2018, 455, 75–85. [Google Scholar] [CrossRef]
- Elazzouzi, A.; Alaoui, A.L.; Tilioua, M.; Tridane, A. Global stability analysis for a generalized delayed SIR model with vaccination and treatment. Adv. Diff. Equ. 2019, 2019, 532. [Google Scholar] [CrossRef]
- Laarabi, H.; Abta, A.; Hattaf, K. Optimal control of a delayed SIRS epidemic model with vaccination and treatment. Acta Biotheor. 2015, 63, 87–97. [Google Scholar] [CrossRef]
- Liu, Q.; Jiang, D.Q.; Hayat, T.; Ahmad, B. Analysis of a delayed vaccinated SIR epidemic model with temporary immunity and levy jumps. Nonlin. Anal. Hybrid Syst. 2018, 27, 29–43. [Google Scholar] [CrossRef]
- Tian, X.H. Stability analysis of a delayed SIRS epidemic model with vaccination and nonlinear incidence. Int. J. Biomath. 2012, 5, 1250050. [Google Scholar] [CrossRef]
- Bakare, E.A. On the optimal control of vaccination and treatments for an SIR-epidemic model with infected immigrants. Int. J. Ecol. Econ. Statist. 2016, 37, 82–104. [Google Scholar]
- Chapman, J.D.; Evans, N.D. The structural identifiability of susceptible-infective-recovered type epidemic models with incomplete immunity and birth targeted vaccination. Biomed. Signal Proc. Control 2009, 4, 278–284. [Google Scholar] [CrossRef]
- Guo, H.J.; Chen, L.S.; Song, X.Y. Dynamical properties of a kind of SIR model with constant vaccination rate and impulsive state feedback control. Int. J. Biomath. 2017, 10, 17500930. [Google Scholar] [CrossRef]
- Kar, T.K.; Batabyal, A. Stability analysis and optimal control of an SIR epidemic model with vaccination. Biosystems 2011, 104, 127–135. [Google Scholar] [CrossRef]
- Ledzewicz, U.; Schattler, H. ON optimal singular controls for a general sir-model with vaccination and treatment. Discr. Contin. Dyn. Syst. 2011, 31, 981–990. [Google Scholar]
- Rao, F.; Mandal, P.S.; Kang, Y. Complicated endemics of an SIRS model with a generalized incidence under preventive vaccination and treatment controls. Appl. Math. Model. 2019, 67, 38–61. [Google Scholar] [CrossRef]
- Rao, X.B.; Zhao, X.P.; Chu, Y.D.; Zhang, J.G.; Gao, J.S. The analysis of mode-locking topology in an SIR epidemic dynamics model with impulsive vaccination control: Infinite cascade of stern-brocot sum trees. Chaos Solitons Fractals 2020, 139, 110031. [Google Scholar] [CrossRef]
- Zeng, G.Z.; Chen, L.S.; Sun, L.H. Complexity of an SIR epidemic dynamics model with impulsive vaccination control. Chaos Solitons Fractals 2005, 26, 495–505. [Google Scholar] [CrossRef]
- Elbasha, E.H.; Podder, C.N.; Gumel, A.B. Analyzing the dynamics of an SIRS vaccination model with waning natural and vaccine-induced immunity. Nonlin. Anal. Real World Appl. 2011, 12, 2692–2705. [Google Scholar] [CrossRef]
- Hui, J.; Chen, L.S. Impulsive vaccination of SIR epidemic models with nonlinear incidence rates. Discr. Contin. Dyn. Syst. B 2004, 4, 595–605. [Google Scholar] [CrossRef]
- Khader, M.M.; Adel, M. Numerical treatment of the fractional modeling on susceptible-infected-recovered equations with a constant vaccination rate by using gem. Int. J. Nonlin. Sci. Numer. Simul. 2019, 20, 69–75. [Google Scholar] [CrossRef]
- Li, B.L.; Qin, C.Y.; Wang, X. Analysis of an SIRS epidemic model with nonlinear incidence and vaccination. Commun. Math. Biol. Neurosci. 2020, 2020, 4262. [Google Scholar]
- Sun, C.J.; Yang, W. Global results for an SIRS model with vaccination and isolation. Nonlin. Anal. Real World Appl. 2010, 11, 4223–4237. [Google Scholar] [CrossRef]
- Lahrouz, A.; Omari, L.; Kiouach, D.; Belmaati, A. Complete global stability for an SIRS epidemic model with generalized non-linear incidence and vaccination. Appl. Math. Comput. 2012, 218, 6519–6525. [Google Scholar] [CrossRef]
- Wang, X.; Gao, S.; Zhu, P.; Wang, J. Roles of different update strategies in the vaccination behavior on two-layered networks. Phys. Lett. A 2020, 384, 126224. [Google Scholar] [CrossRef]
- Assadouq, A.; El Mahjour, H.; Settati, A. Qualitative behavior of a SIRS epidemic model with vaccination on heterogeneous networks. Ital. J. Pure Appl. Math. 2020, 43, 958–974. [Google Scholar]
- Le Chang, S.; Piraveenan, M.; Prokopenko, M. The effects of imitation dynamics on vaccination behaviours in sir-network model. Int. J. Environ. Res. Public Health 2019, 16, 16142477. [Google Scholar] [CrossRef] [Green Version]
- Auchincloss, A.H.; Roux, A.V.D. A new tool for epidemiology: The usefulness of dynamic-agent models in understanding place effects on health. Am. J. Epidemiol. 2008, 168, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Ajelli, M.; Goncalves, B.; Balcan, D.; Colizza, V.; Hu, H.; Ramasco, J.J.; Merler, S.; Vespignani, A. Comparing large-scale computational approaches to epidemic modeling: Agent-based versus structured metapopulation models. BMC Infect. Dis. 2010, 10, 190. [Google Scholar] [CrossRef] [Green Version]
- Schüttler, J.; Schlickeiser, R.; Schlickeiser, F.; Kröger, M. Covid-19 predictions using a Gauss model, based on data from April 2. Physics 2020, 2, 197–212. [Google Scholar] [CrossRef]
- Yildirim, A.; Cherruault, Y. Analytical approximate solution of a SIR epidemic model with constant vaccination strategy by homotopy perturbation method. Kybernetes 2009, 38, 1566–1575. [Google Scholar] [CrossRef]
- Heng, K.; Althaus, C.L. The approximately universal shapes of epidemic curves in the susceptible-exposed-infectious-recovered (SEIR) model. Sci. Rep. 2020, 10, 19365. [Google Scholar] [CrossRef]
- Barlow, N.S.; Weinstein, S.J. Accurate closed-form solution of the SIR epidemic model. Phys. D 2020, 408, 132540. [Google Scholar] [CrossRef]
- Bidari, S.; Chen, X.Y.; Peters, D.; Pittman, D.; Simon, P.L. Solvability of implicit final size equations for SIR epidemic models. Math. Biosci. 2016, 282, 181–190. [Google Scholar] [CrossRef] [Green Version]
- Carvalho, A.M.; Goncalves, S. An analytical solution for the Kermack-McKendrick model. Phys. A 2021, 566, 125659. [Google Scholar] [CrossRef]
- Guerrero, F.; Santonja, F.J.; Villanueva, R.J. Solving a model for the evolution of smoking habit in Spain with homotopy analysis method. Nonlinear Anal. Real World Appl. 2013, 14, 549–558. [Google Scholar] [CrossRef]
- Khan, H.; Mohapatra, R.N.; Vajravelu, K.; Liao, S.J. The explicit series solution of SIR and SIS epidemic models. Appl. Math. Comput. 2009, 215, 653–669. [Google Scholar] [CrossRef]
- Liu, J.L.; Peng, B.Y.; Zhang, T.L. Effect of discretization on dynamical behavior of SEIR and SIR models with nonlinear incidence. Appl. Math. Lett. 2015, 39, 60–66. [Google Scholar] [CrossRef]
- Van Mieghem, P. Approximate formula and bounds for the time-varying susceptible-infected-susceptible prevalence in networks. Phys. Rev. E 2016, 93, 052312. [Google Scholar] [CrossRef]
- Kröger, M.; Schlickeiser, R. Analytical solution of the SIR-model for the temporal evolution of epidemics. Part A: Time-independent reproduction factor. J. Phys. A Math. Theor. 2020, 53, 505601. [Google Scholar] [CrossRef]
- Schlickeiser, R.; Kröger, M. Analytical solution of the SIR-model for the temporal evolution of epidemics. Part B: Semi-time case. J. Phys. A Math. Theor. 2021, 54, 175601. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M. Explicit formulae for the peak time of an epidemic from the SIR model. Phys. D 2021, 422, 132902. [Google Scholar] [CrossRef] [PubMed]
- Estrada, E. Covid-19 and Sars-Cov-2. Modeling the present, looking at the future. Phys. Rep. 2020, 869, 1. [Google Scholar] [CrossRef] [PubMed]
- Kröger, M.; Schlickeiser, R. Forecast for the second Covid-19 wave based on the improved SIR-model with a constant ratio of recovery to infection rate. Preprints 2021, 2021010449. [Google Scholar] [CrossRef]
- Morton, R.; Wickwire, K.H. On the optimal control of a deterministic epidemic. Adv. Appl. Probab. 1974, 6, 622–635. [Google Scholar] [CrossRef]
- Anderson, R.M.; May, R.M. Infectious Diseases of Humans: Dynamics and Control; Oxford Science Publications: Oxford, UK, 1991. [Google Scholar]
- Behncke, H. Optimal control of deterministic epidemics. Optim. Control Appl. Methods 2000, 21, 269–285. [Google Scholar] [CrossRef]
- Hansen, E.; Day, T. Optimal control of epidemics with limited resources. J. Math. Biol. 2011, 62, 423–451. [Google Scholar] [CrossRef] [Green Version]
- Grauer, J.; Löwen, H.; Liebchen, B. Strategic spatiotemporal vaccine distribution increases the survival rate in an infectious disease like Covid-19. Sci. Rep. 2021, 10, 21594. [Google Scholar] [CrossRef]
- Grundel, S.; Heyder, S.; Hotz, T.; Ritschel, T.K.S.; Sauerteig, P.; Worthmann, K. How to coordinate vaccination and social distancing to mitigate SARS-CoV-2 outbreaks. medRxiv 2020, 2020, 20248707. [Google Scholar] [CrossRef]
- Duclos, T.; Reichert, T. The missing Link: A closed form solution to the Kermack and McKendrick epidemic model equations. medRxiv 2021, 2021, 21252781. [Google Scholar] [CrossRef]
- Abramowitz, M.; Stegun, I.A. Handbook of Mathematical Functions; National Bureau of Standards: Washington, DC, USA, 1972.
- Kröger, M.; Schlickeiser, R. Gaussian doubling times and reproduction factors of the COVID-19 pandemic disease. Front. Phys. 2020, 8, 276. [Google Scholar] [CrossRef]
- Data Repository. 2021. Available online: https://github.com/owid/covid-19-data/blob/master/public/data/vaccinations/vaccinations.csv (accessed on 20 May 2021).
- Data Repository. 2021. Available online: https://www.complexfluids.ethz.ch/cgi-bin/covid19-waveII (accessed on 20 May 2021).









| Country | k | a | b | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Code | [d] | [d] | ||||||||||||||
| ARG | 0.912 | 0.125 | 0.114 | 0.0022 | 0.039 | 0.910 | 0.07 | 20-08-17 | 20-12-28 | 0.069 | 0.228 | 0.282 | 0.277 | 0.274 | 129 | 21-10-17 |
| AUT | 0.905 | 0.520 | 0.471 | 0.0013 | 0.841 | 0.904 | 0.09 | 20-07-29 | 20-12-26 | 0.008 | 0.128 | 0.191 | 0.182 | 0.177 | 219 | 21-07-29 |
| BEL | 0.896 | 0.551 | 0.494 | 0.0011 | 0.271 | 0.895 | 0.10 | 20-09-06 | 20-12-27 | 0.162 | 0.313 | 0.332 | 0.330 | 0.329 | 244 | 21-05-26 |
| BRA | 0.790 | 0.046 | 0.037 | 0.0099 | 0.202 | 0.780 | 0.19 | 20-07-05 | 21-01-14 | 0.145 | 0.247 | 0.489 | 0.432 | 0.398 | 86 | 22-09-29 |
| CAN | 0.962 | 1.018 | 0.980 | 0.0004 | 0.692 | 0.962 | 0.04 | 20-09-26 | 20-12-13 | 0.049 | 0.069 | 0.121 | 0.106 | 0.099 | 68 | 21-06-05 |
| CHE | 0.894 | 0.458 | 0.409 | 0.0023 | 1.237 | 0.891 | 0.10 | 20-07-22 | 21-01-22 | 0.044 | 0.216 | 0.240 | 0.236 | 0.234 | 231 | 21-09-05 |
| DEU | 0.915 | 0.559 | 0.511 | 0.0012 | 1.077 | 0.913 | 0.08 | 20-08-16 | 20-12-26 | 0.019 | 0.069 | 0.182 | 0.158 | 0.143 | 180 | 21-08-06 |
| ESP | 0.876 | 0.175 | 0.153 | 0.0046 | 1.120 | 0.871 | 0.12 | 20-04-29 | 21-01-02 | 0.092 | 0.207 | 0.309 | 0.283 | 0.270 | 115 | 22-01-28 |
| FIN | 0.997 | 3.858 | 3.848 | 0.0002 | 2.181 | 0.997 | 0.00 | 20-12-21 | 20-12-30 | 0.007 | 0.008 | 0.019 | 0.012 | 0.011 | 17 | 21-02-21 |
| FRA | 0.886 | 0.228 | 0.202 | 0.0027 | 0.973 | 0.883 | 0.11 | 20-05-11 | 20-12-26 | 0.082 | 0.183 | 0.284 | 0.263 | 0.252 | 127 | 21-12-25 |
| GBR | 0.867 | 0.389 | 0.337 | 0.0053 | 1.741 | 0.862 | 0.13 | 20-09-09 | 20-12-12 | 0.120 | 0.151 | 0.343 | 0.257 | 0.223 | 200 | 21-06-16 |
| ISR | 0.855 | 0.050 | 0.042 | 0.1283 | 2.518 | 0.727 | 0.00 | 20-08-20 | 20-12-18 | 0.035 | 0.100 | 0.330 | 0.142 | 0.127 | 62 | 21-09-07 |
| ITA | 0.873 | 0.289 | 0.252 | 0.0022 | 0.982 | 0.871 | 0.12 | 20-05-27 | 20-12-26 | 0.114 | 0.233 | 0.329 | 0.315 | 0.306 | 189 | 21-11-04 |
| MEX | 0.712 | 0.038 | 0.027 | 0.0044 | 0.052 | 0.707 | 0.27 | 20-07-13 | 20-12-23 | 0.123 | 0.234 | 0.586 | 0.559 | 0.535 | 124 | 22-11-23 |
| NLD | 0.929 | 0.397 | 0.369 | 0.0022 | 2.039 | 0.927 | 0.07 | 20-06-11 | 21-01-15 | 0.072 | 0.149 | 0.201 | 0.186 | 0.179 | 89 | 21-11-18 |
| RUS | 0.933 | 0.337 | 0.314 | 0.0008 | 0.423 | 0.933 | 0.07 | 20-07-22 | 20-12-14 | 0.003 | 0.049 | 0.135 | 0.118 | 0.107 | 74 | 21-10-30 |
| SWE | 0.922 | 0.652 | 0.601 | 0.0010 | 0.540 | 0.921 | 0.08 | 20-10-11 | 20-12-26 | 0.126 | 0.167 | 0.260 | 0.240 | 0.229 | 162 | 21-06-04 |
| USA | 0.868 | 0.218 | 0.189 | 0.0081 | 0.948 | 0.860 | 0.12 | 20-09-03 | 20-12-19 | 0.094 | 0.167 | 0.326 | 0.263 | 0.238 | 156 | 21-07-28 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schlickeiser, R.; Kröger, M. Analytical Modeling of the Temporal Evolution of Epidemics Outbreaks Accounting for Vaccinations. Physics 2021, 3, 386-426. https://doi.org/10.3390/physics3020028
Schlickeiser R, Kröger M. Analytical Modeling of the Temporal Evolution of Epidemics Outbreaks Accounting for Vaccinations. Physics. 2021; 3(2):386-426. https://doi.org/10.3390/physics3020028
Chicago/Turabian StyleSchlickeiser, Reinhard, and Martin Kröger. 2021. "Analytical Modeling of the Temporal Evolution of Epidemics Outbreaks Accounting for Vaccinations" Physics 3, no. 2: 386-426. https://doi.org/10.3390/physics3020028
APA StyleSchlickeiser, R., & Kröger, M. (2021). Analytical Modeling of the Temporal Evolution of Epidemics Outbreaks Accounting for Vaccinations. Physics, 3(2), 386-426. https://doi.org/10.3390/physics3020028






