Magnetic Force-Free Theory: Nonlinear Case
Abstract
:1. Introduction
2. Nonlinear Grad–Shafranov Equation
2.1. Nonlinear
2.2. First-Order Perturbation in
2.3. Solution of Zero-Order Equation and Boundary Conditions
2.4. Solution of the First-Order Equation
3. Taylor States without the Magnet
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Taylor, J.B. Relaxation of toroidal plasma abd generation of reverse magnetic field. Phys. Rev. Lett. 1974, 33, 1139–1141. [Google Scholar] [CrossRef]
- Taylor, J.B. Relaxation and magnetic reconnection in plasmas. Rev. Mod. Phys. 1986, 58, 741–763. [Google Scholar] [CrossRef]
- Jensen, T.H.; Chu, M.S. Current drive and helicity injection. Phys. Fluids 1984, 27, 2881–2885. [Google Scholar] [CrossRef]
- Jarboe, T.R. Review of spheromak research. Plasma Phys. Control Fusion 1994, 36, 945. [Google Scholar] [CrossRef]
- Clarke, T.E.; Kronberg, P.P.; Böhringer, H. A new radio-X-ray probe of galaxy cluster magnetic fields. Astrophys. J. 2001, 547, L111. [Google Scholar] [CrossRef]
- Kronberg, P.P.; Colgate, S.A.; Li, H.; Dufton, Q.W. Giant radio galaxies and cosmic-ray acceleration. Astrophys. J. 2001, 560, 178. [Google Scholar] [CrossRef] [Green Version]
- Low, B.C.; Lou, Y.Q. Modeling solar force-free magnetic fields. Astrophys J. 1990, 382, 343–352. [Google Scholar] [CrossRef]
- Wiegelmann, T.; Sakurai, T. Solar force-free magnetic fields. Living Rev. Sol. Phys. 2021, 18, 1. [Google Scholar] [CrossRef]
- Knox, S.O.; Barnes, C.W.; Marklin, G.J.; Jarboe, T.R.; Henins, I.; Hoida, H.W.; Wright, B.L. Observations of spheromak equilibria which differ from the minimum-energy state and have internal kink distortions. Phys. Rev. Lett. 1986, 56, 842–845. [Google Scholar] [CrossRef] [PubMed]
- Rosenbluth, M.N.; Bussac, M.N. MHD stability of spheromacs. Nucl. Fusion 1979, 19, 489–498. [Google Scholar] [CrossRef]
- Tang, X.Z.; Boozer, A.H. Force-free magnetic reconnection in driven plasmas. Phys. Rev. Lett. 2005, 94, 225004. [Google Scholar] [CrossRef] [PubMed]
- Lampugnani, L.G.; Garcia-Martinez, P.L.; Farengo, R. Spherical tokamaks with a high current carrying plasma center column. Phys. Plasmas 2018, 25, 122513. [Google Scholar] [CrossRef]
- Bellan, P.M. Spheromaks. A Practical Application of Magnetohydrodynamic Dynamos and Plasma Self-Organization; Imperial College Press: London, UK, 2000; Chapter 11. [Google Scholar]
- Tang, X.Z.; Boozer, A.H. Spherical tokamak with a plasma center column. Phys. Plasmas 2006, 13, 042514. [Google Scholar] [CrossRef]
- Alladio, F.; Costa, P.; Micozzi, A.M.P.; Papastergiou, S.; Rogier, F. Design of the PROTO-SPHERA experiment and of its first step, (MULTI-PINCH). Nucl. Fusion 2006, 46, S613. [Google Scholar] [CrossRef]
- Tirozzi, B.; Buratti, P.; Alladio, F.; Micozzi, P. Bidimensional analysis of the PROTO-SPHERA flow. In Proceedings of the International Conference “Days on Diffraction 2019”, St. Petersburg, Russia, 3–7 June 2019; IEEE: Danvers, MA, USA, 2019; pp. 204–209. [Google Scholar] [CrossRef]
- Grad, H.; Rubin, H. Hydromagnetic equilibria and force-free fields. In Proceedigns of the Second United International Conference on the Peaceful Uses of Atomic Energy, Geneva, Switzerland, 1–3 September 1958. Volume 31: Theoretical and Experimental Aspects of Controlled Nuclear Fusion; United Nations: Geneva, Switzerland, 1958; pp. 190–197. Available online: http://www-naweb.iaea.org/napc/physics/2ndgenconf/data/Proceedings%201958/papers%20Vol31/Paper25_Vol31.pdf (accessed on 1 November 2021).
- Shafranov, V.D. Plasma equilibrium in a magnetic field. In Reviews of Plasma Physics Leontovich; Leontovich, M.A., Ed.; Consultants Bureau: New York, NY, USA, 1966; Volume 2, p. 103. [Google Scholar]
- Solov’ev, A.A.; Kirichek, E.A. Force-free magnetic flux ropes: Inner structure and basic properties. Mon. Not. R. Astron. Soc. 2021, 3, 4406–4416. [Google Scholar] [CrossRef]



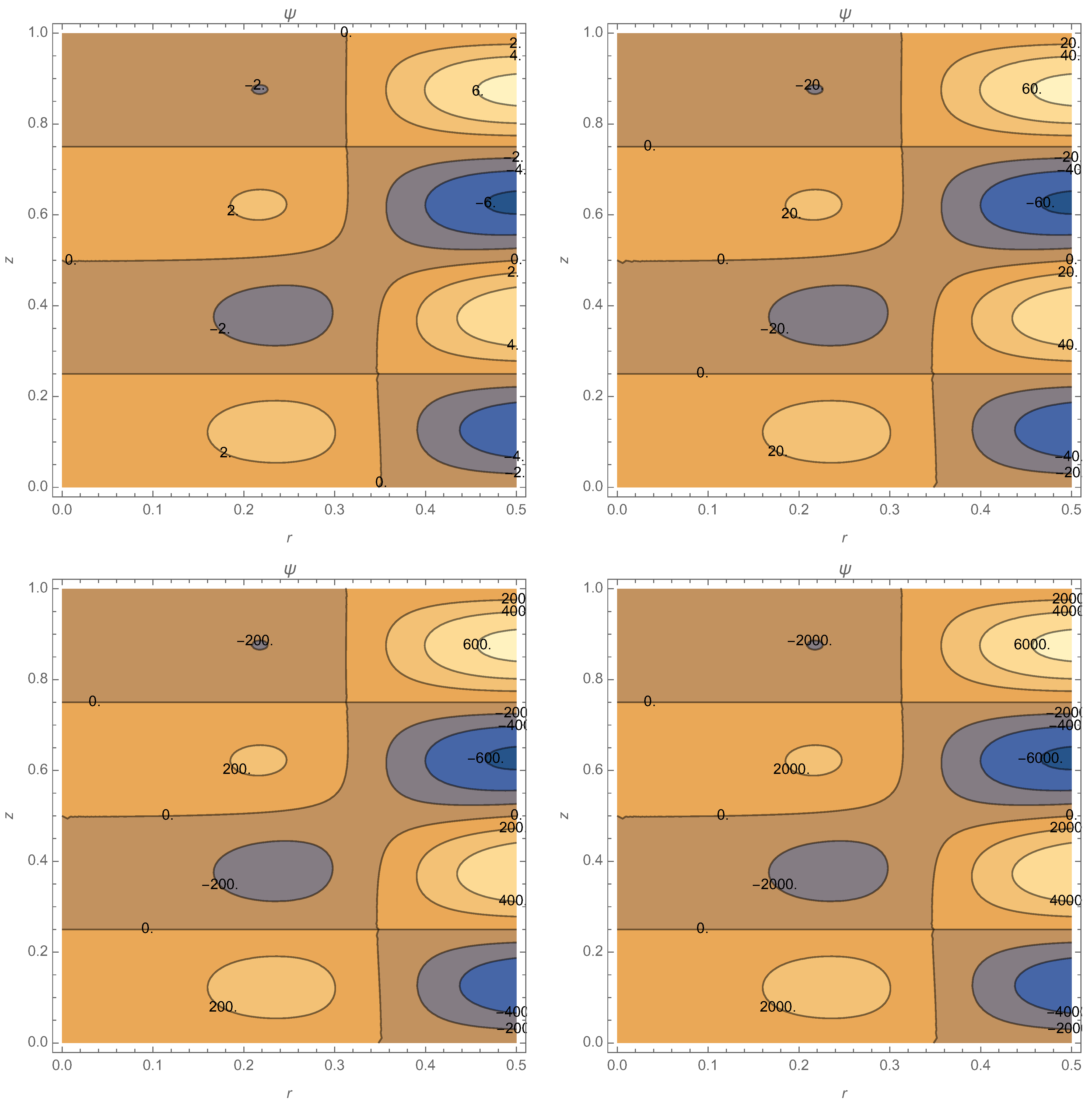


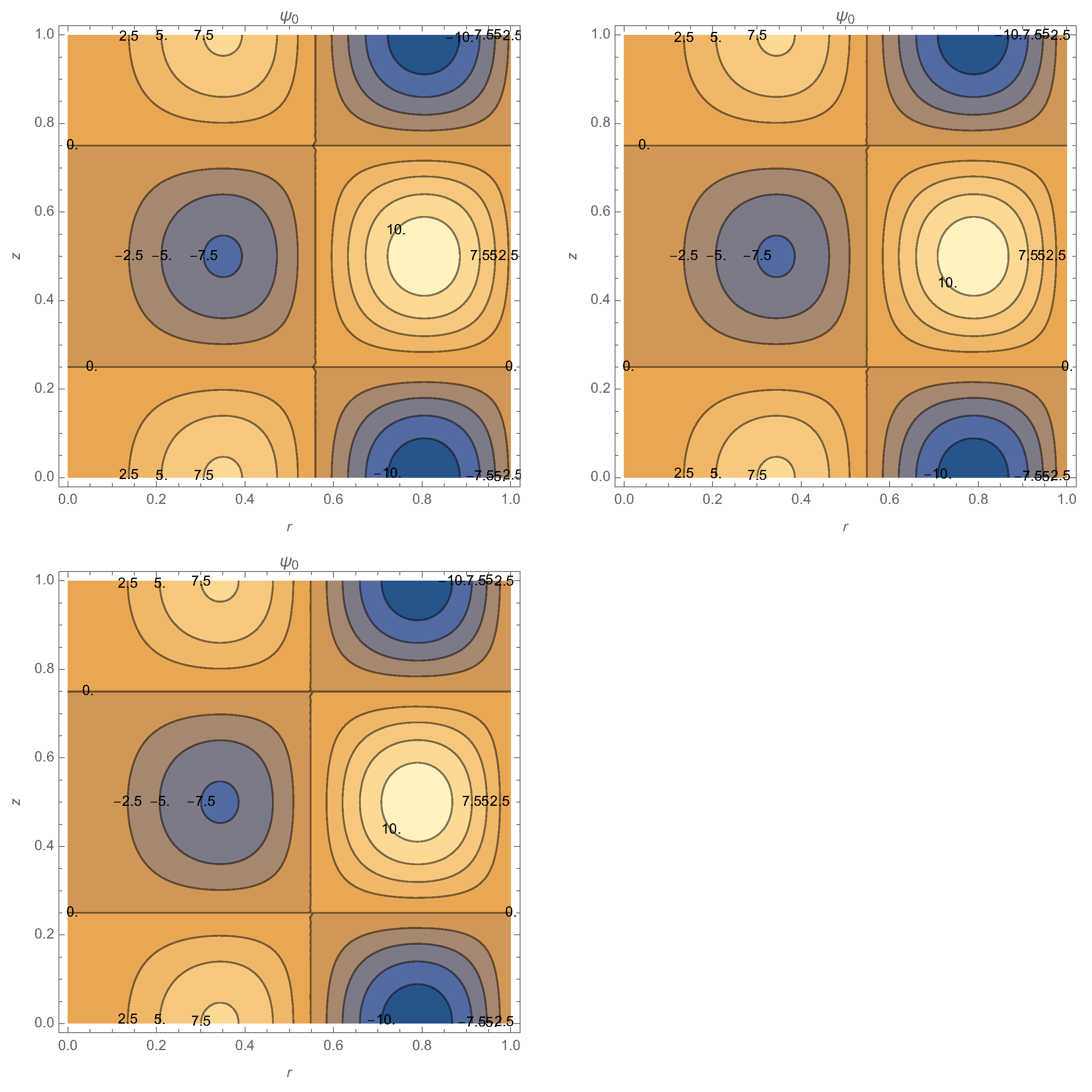
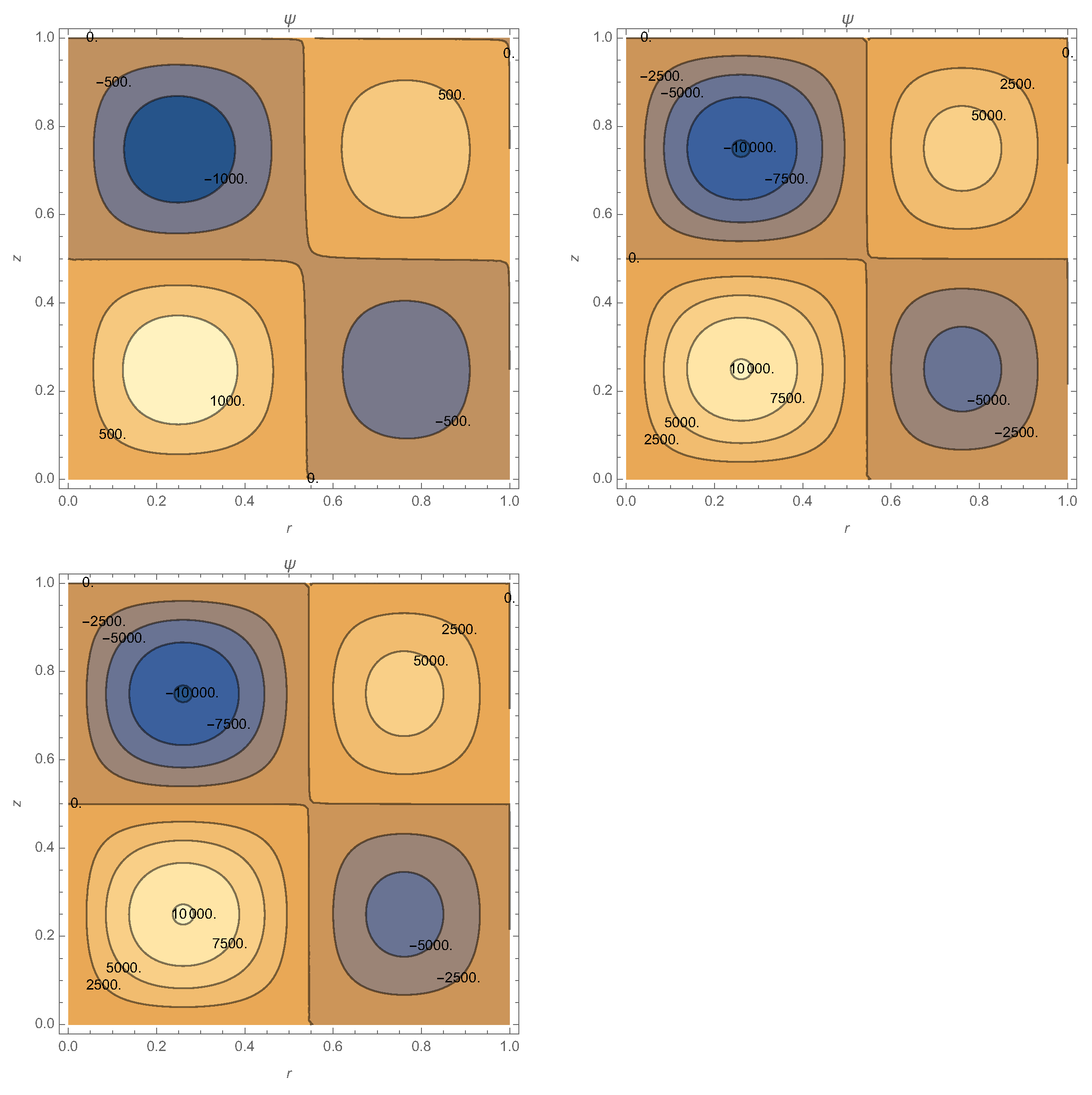
| n | |||
|---|---|---|---|
| 1 | 4,214 | 4.214 | 3.51131 |
| 2 | 4.13661 | 4.13661 | 4.71224 |
| 3 | −3.32075 | 5.66356 | |
| 4 | 7.77266 | −2.9967 | 6.47654 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tirozzi, B.; Buratti, P. Magnetic Force-Free Theory: Nonlinear Case. Physics 2022, 4, 21-36. https://doi.org/10.3390/physics4010003
Tirozzi B, Buratti P. Magnetic Force-Free Theory: Nonlinear Case. Physics. 2022; 4(1):21-36. https://doi.org/10.3390/physics4010003
Chicago/Turabian StyleTirozzi, Brunello, and Paolo Buratti. 2022. "Magnetic Force-Free Theory: Nonlinear Case" Physics 4, no. 1: 21-36. https://doi.org/10.3390/physics4010003





