Polarization and Consensus in a Voter Model under Time-Fluctuating Influences
Abstract
:1. Introduction
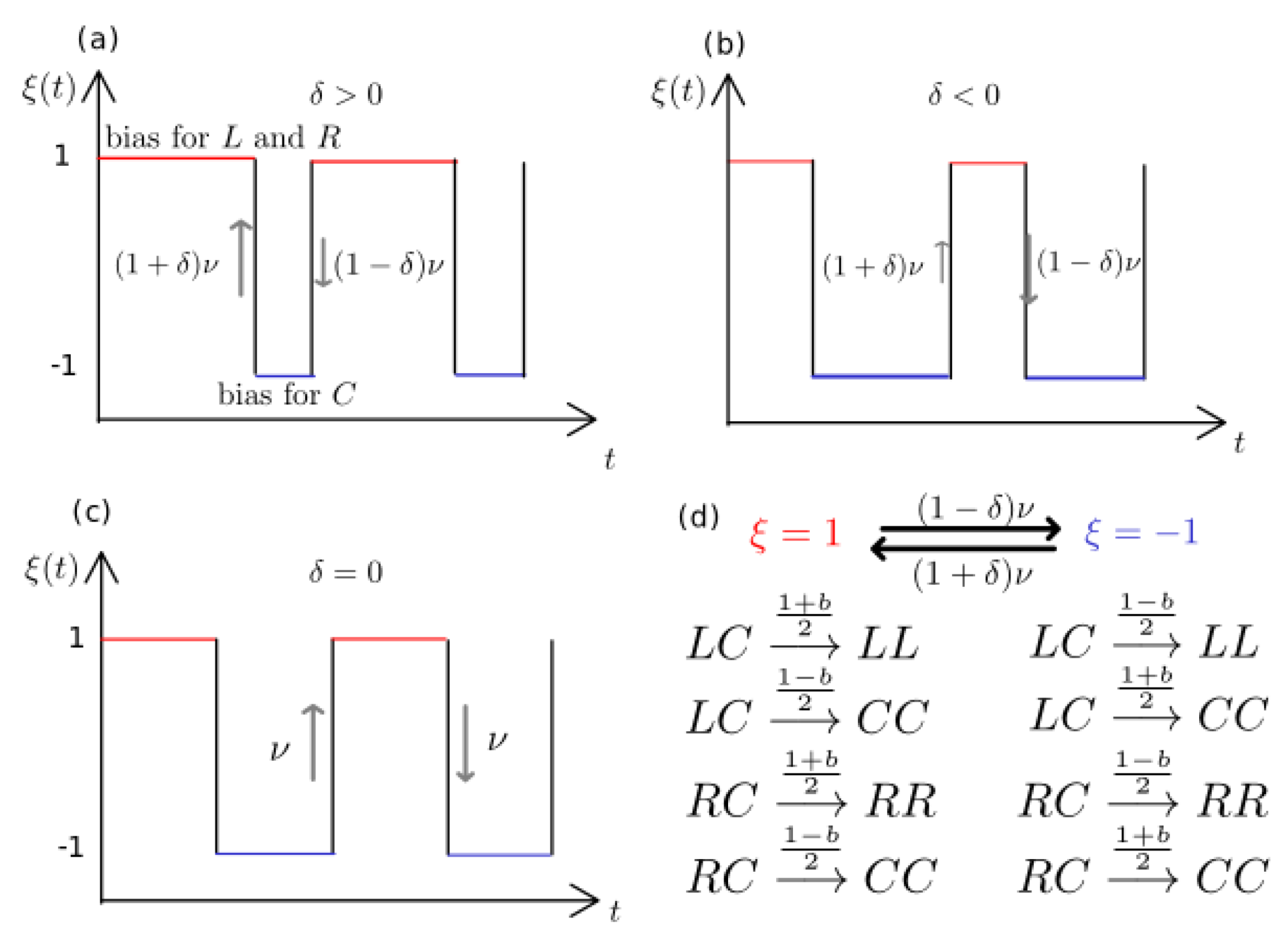
2. Three-State Constrained Voter Model under Binary Time-Fluctuating Influences

3. Final State: Polarization and Consensus Probabilities
3.1. Final State in the Regimes and
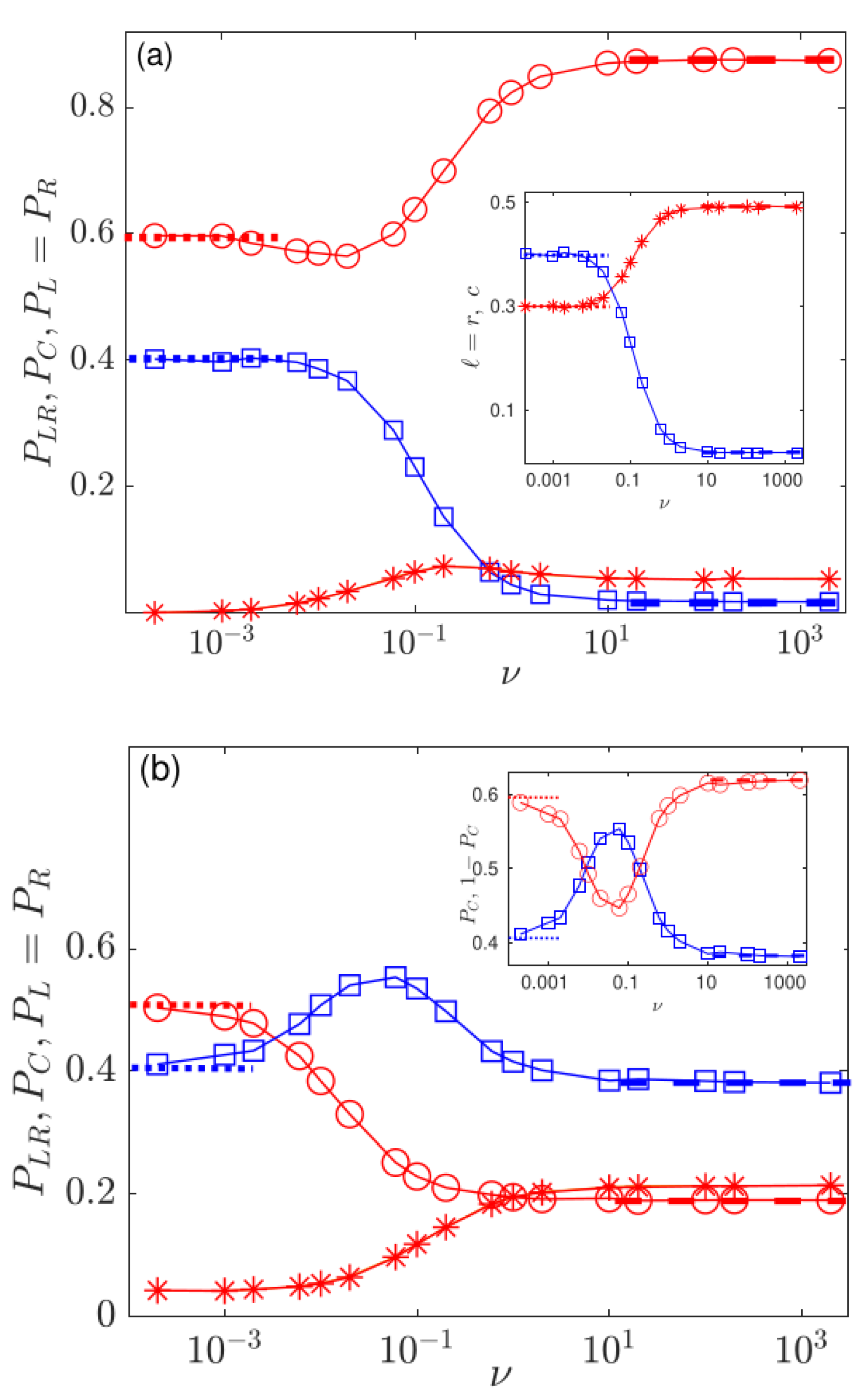
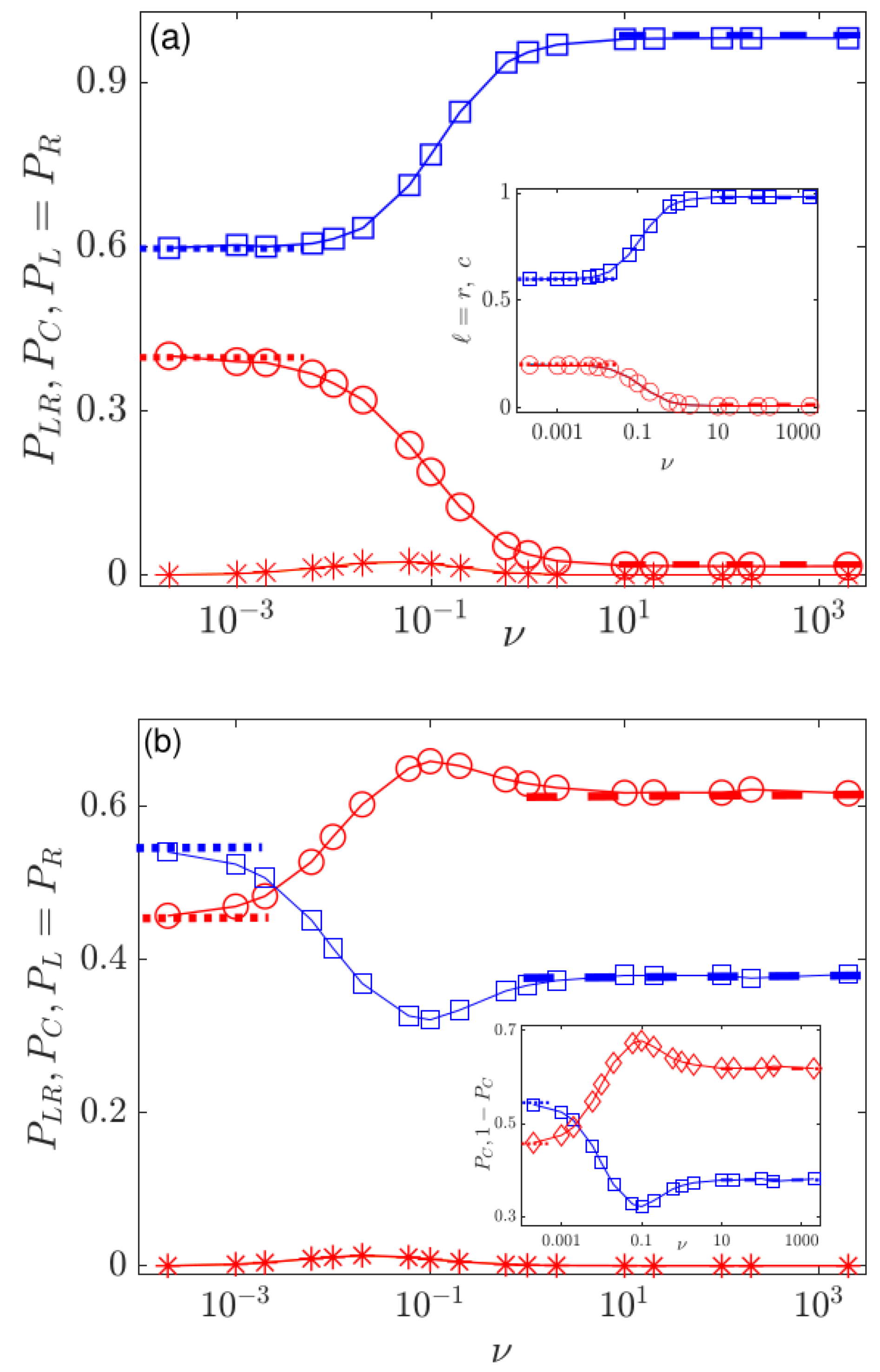
3.1.1. Polarization and Consensus Probabilities in the Regime
3.1.2. Polarization and Consensus Probabilities in the Regime
3.2. Polarization and Consensus Probabilities When
3.3. Polarization and Consensus Probabilities When
3.4. Polarization and Consensus Probabilities under Symmetric Switching ()
4. Mean Exit Time
4.1. Mean Exit Time in the Regimes and
4.1.1. MET in the Regime
4.1.2. MET in the Regime
4.2. Mean Exit Time as Function of the Switching Rate
- (i) when , the system is in the regime of “low switching rate” and the final state can possibly be reached without experiencing any switches;
- (ii) when , the population is in a regime of “intermediate switching rate”, where there are typically switches before reaching the final state;
- (iii) when , the system is in the regime of “high switching rate”, where the external noise self-averages, and the number of switches before reaching the final state is and hence grows linearly with , where ; see inset in Figure 6b.
5. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Master Equation and Mean-Field Limit When N→∞
Appendix A.1. Master Equation
Appendix A.2. Mean-Field Limit When N→∞
Appendix B. Polarization, Consensus Probabilities, and Mean Exit Time in the Absence of Time-Varying Influences
Appendix B.1. Polarization and Consensus Probabilities in the Absence of Time-Varying Influences
Appendix B.2. Mean Exit Time in the Absence of Time-Varying Influences
Appendix C. Possible Generalizations and Applications of the Model
References
- Granovetter, M. Threshold models of collective behavior. Am. J. Sociol. 1978, 83, 1420–1443. Available online: https://www.jstor.org/stable/2778111 (accessed on 9 April 2023).
- Schelling, T.C. Micromotives and Macrobehaviour; W. W. Norton & Company, Inc.: New York, NY, USA, 1978. [Google Scholar]
- Galam, S.; Gefen, Y.; Shapir, Y. Sociophysics: A new approach of sociological collective behaviour: I. Mean-behaviour description of a strike. Math. J. Sociol. 1982, 9, 1–13. [Google Scholar] [CrossRef]
- Galam, S. Majority rule, hierarchical structures, and democratic totalitarianism: A statistical approach. J. Math. Psychol. 1986, 30, 426–434. [Google Scholar] [CrossRef]
- Galam, S. Social paradoxes of majority rule voting and renormalization group. J. Stat. Phys. 1990, 61, 943–951. [Google Scholar] [CrossRef]
- Galam, S.; Moscovici, S. Towards a theory of collective phenomena: Consensus and attitude changes in groups. Eur. J. Soc. Psychol. 1991, 21, 49–74. [Google Scholar] [CrossRef]
- Castellano, C.; Fortunato, S.; Loreto, V. Statistical physics of social dynamics. Rev. Mod. Phys. 2009, 81, 591–646. [Google Scholar] [CrossRef]
- Galam, S. Sociophysics. A Physicist’s Modeling of Psycho-Political Phenomena; Springer Science+Business Media, LLC: New York, NY, USA, 2012. [Google Scholar] [CrossRef]
- Sen, P.; Chakrabarti, B. K. Sociophysics: An Introduction; Oxford University Press: New York, NY, USA, 2014. [Google Scholar]
- Perc, M.; Jordan, J.J.; Rand, D.G.; Wang, Z.; Boccaletti, S.; Szolnoki, A. Statistical physics of human cooperation. Phys. Rep. 2017, 687, 1–51. [Google Scholar] [CrossRef]
- Schweitzer, F. Sociophysics. Phys. Today 2018, 71, 40–46. [Google Scholar] [CrossRef]
- Jedrzejewski, A.; Sznajd-Weron, K. Statistical physics of opinion formation: Is it a SPOOF? C. R. Phys. 2019, 20, 244–261. [Google Scholar] [CrossRef]
- Redner, S. Reality-inspired voter models: A mini-review. C. R. Phys. 2019, 20, 275–292. [Google Scholar] [CrossRef]
- Li, Z.; Chen, X.; Yang, H.-X.; Szolnoki, A. Game-theoretical approach for opinion dynamics on social networks. Chaos 2022, 32, 73117. [Google Scholar] [CrossRef]
- Liggett, T.M. Interacting Particle Systems; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar] [CrossRef]
- Glauber, R.J. Time-dependent statistics of the Ising model. J. Math. Phys. 1963, 4, 294–307. [Google Scholar] [CrossRef]
- Asch, S.E. Opinions and social pressure. Sci. Am. 1955, 193, 31–35. Available online: https://www.jstor.org/stable/24943779 (accessed on 9 April 2023).
- Milgram, S.; Bickman, L.; Berkowitz, L. Note on the drawing power of crowds of different size. J. Personal. Soc. Psychol. 1969, 13, 79–82. [Google Scholar] [CrossRef]
- Lattané, B. The psychology of social impact. Am. Psychol. 1981, 36, 343–356. [Google Scholar] [CrossRef]
- Axelrod, R. The dissemination of culture: A model with local convergence and global olarization. J. Confl. Resolut. 1997, 41, 203–226. [Google Scholar] [CrossRef]
- Axelrod, R. The Complexity of Cooperation: Agent-Based Models of Competition and Collaboration; Princeton University Press: Princeton, NJ, USA, 1997. [Google Scholar] [CrossRef]
- Castellano, C.; Marsili, M.; Vespignani, A. Nonequilibrium phase transition in a model for social influence. Phys. Rev. Lett. 2000, 85, 3536–3539. [Google Scholar] [CrossRef]
- Klemm, K.; Eguiluz, V.M.; Toral, R.; San Miguel, M. Global culture: A noise-induced transition in finite systems. Phys. Rev. E 2003, 67, 045101. [Google Scholar] [CrossRef]
- McPherson, J.M.; Smith-Lovin, L. Homophily in voluntary organizations: Status distance and the composition of face-to-face groups. Am. Sociol. Rev. 1987, 52, 370–379. [Google Scholar] [CrossRef]
- Mobilia, M. Does a single zealot affect an infinite group of voters? Phys. Rev. Lett. 2003, 91, 028701. [Google Scholar] [CrossRef]
- Mobilia, M.; Georgiev, I.T. Voting and catalytic processes with inhomogeneities. Phys. Rev. E 2005, 71, 046102. [Google Scholar] [CrossRef] [PubMed]
- Mobilia, M.; Petersen, A.; Redner, S. On the role of zealotry in the voter model. J. Stat. Mech. 2007, 2007, P08029. [Google Scholar] [CrossRef]
- Mobilia, M. Commitment versus persuasion in the three-party constrained voter model. J. Stat. Phys. 2013, 151, 69–91. [Google Scholar] [CrossRef]
- Mobilia, M. Nonlinear q-voter model with inflexible zealots. Phys. Rev. E 2015, 92, 012803. [Google Scholar] [CrossRef] [PubMed]
- Galam, S.; Jacobs, F. The role of inflexible minorities in the breaking of democratic opinion dynamics. Phys. A 2007, 381, 366–376. [Google Scholar] [CrossRef]
- Sznajd-Weron, K.; Tabiszewski, M.; Timpanaro, A.M. Phase transition in the Sznajd model with independence. EPL (Europhys. Lett.) 2011, 96, 48002. [Google Scholar] [CrossRef]
- Acemoglu, D.; Como, G.; Fagnani, F.; Ozdaglar, A. Opinion fluctuations and disagreement in social networks. Math. Op. Res. 2013, 38, 1–27. [Google Scholar] [CrossRef]
- Nyczka, P.; Sznajd-Weron, K. Anticonformity or independence?—Insights from statistical physics. J. Stat Phys. 2013, 151, 174–202. [Google Scholar] [CrossRef]
- Masuda, N. Opinion control in complex networks. New J. Phys. 2015, 17, 033031. [Google Scholar] [CrossRef]
- Li, X.; Mobilia, M.; Rucklidge, A.M.; Zia, R.K.P. How does homophily shape the topology of a dynamic network? Phys. Rev. E 2021, 104, 044311. [Google Scholar] [CrossRef]
- Li, X.; Mobilia, M.; Rucklidge, A.M.; Zia, R.K.P. Effects of homophily and heterophily on preferred-degree networks: Mean-field analysis and overwhelming transition. J. Stat. Mech. 2022, 013402. [Google Scholar] [CrossRef]
- Castellano, C.; Muñoz, M.A.; Pastor-Satorras, R. Nonlinear q-voter model. Phys. Rev. E 2009, 80, 041129. [Google Scholar] [CrossRef]
- Sznajd-Weron, K.; Sznajd, J. Opinion evolution in closed community. Int. J. Mod. Phys. C 2000, 11, 1157–1165. [Google Scholar] [CrossRef]
- Slanina, F.; Lavicka, H. Analytical results for the Sznajd model of opinion formation. Eur. Phys. J. B 2003, 35, 279–288. [Google Scholar] [CrossRef]
- Nyczka, P.; Sznajd-Weron, K.; Cislo, J. Opinion dynamics as a movement in a bistable potential. Phys. A 2012, 391, 317–327. [Google Scholar] [CrossRef]
- Lambiotte, R.; Redner, S. Dynamics of non-conservative voters. EPL (Europhys. Lett.) 2008, 82, 18007. [Google Scholar] [CrossRef]
- Slanina, F.; Sznajd-Weron, K.; Przybyla, P. Some new results on one-dimensional outflow dynamics. EPL (Europhys. Lett.) 2008, 82, 18006. [Google Scholar] [CrossRef]
- Galam, S.; Martins, A.C.R. Pitfalls driven by the sole use of local updates in dynamical systems. EPL (Europhys. Lett.) 2011, 95, 48005. [Google Scholar] [CrossRef]
- Timpanaro, A.M.; Prado, C.P.C. Exit probability of the one-dimensional q-voter model: Analytical results and simulations for large networks. Phys. Rev. E 2014, 89, 052808. [Google Scholar] [CrossRef]
- Mellor, A.; Mobilia, M.; Zia, R.K.P. Characterization of the nonequilibrium steady state of a heterogeneous nonlinear q-voter model with zealotry. EPL (Europhys. Lett.) 2016, 113, 48001. [Google Scholar] [CrossRef]
- Mellor, A.; Mobilia, M.; Zia, R.K.P. Heterogeneous out-of-equilibrium nonlinear q-voter model with zealotry. Phys. Rev. E 2017, 95, 012104. [Google Scholar] [CrossRef]
- Deffuant, G.; Neau, D.; Amblard, F.; Weisbuch, G. Mixing Beliefs among Interacting Agents. Adv. Complex Syst. 2000, 3, 87–98. [Google Scholar] [CrossRef]
- Hegselmann, R.; Krause, U. Opinion dynamics and bounded confidence models, analysis, and simulation. J. Artif. Soc. Soc. Simul. 2002, 5, 2. Available online: https://www.jasss.org/5/3/2.html (accessed on 9 April 2023).
- Weisbuch, G.; Deffuant, G.; Amblard, F.; Nadal, J.P. Meet, discuss, and segregate! Complexity 2002, 7, 55–63. [Google Scholar] [CrossRef]
- Ben-Naim, E.; Krapivsky, P.L.; Redner, S. Bifurcations and patterns in compromise processes. Phys. D 2003, 183, 190. [Google Scholar] [CrossRef]
- Slanina, F. Dynamical phase transitions in Hegselmann-Krause model of opinion dynamics and consensus. Eur. Phys. J. B 2011, 79, 99–106. [Google Scholar] [CrossRef]
- Vazquez, F.; Krapivsky, P.L.; Redner, S. Freezing and slow evolution in a constrained opinion dynamics model. J. Phys. A Math. Gen. 2003, 36, L61–L68. [Google Scholar] [CrossRef]
- Vazquez, F.; Redner, S. Ultimate fate of constrained voters. J. Phys. A Math. Gen. 2004, 37, 8479–8494. [Google Scholar] [CrossRef]
- Mobilia, M. Fixation and polarization in a three-species opinion dynamics model. EPL (Europhys. Lett.) 2011, 95, 50002. [Google Scholar] [CrossRef]
- DellaVigna, S.; Kaplan, E. The Fox News effect: Media bias and voting. Quart. J. Econ. 2007, 122, 1187–1234. [Google Scholar] [CrossRef]
- Gerber, A.S.; Karlan, D.; Bergan, D. Does the media matter? A field experiment measuring the effect of newspapers on voting behavior and political opinions. Am. Econ. J. Appl. Econ. 2009, 1, 35–52. [Google Scholar] [CrossRef]
- Yang, J.; Leskovec, J. Patterns of temporal variation in online media. In Proceedings of the Fourth ACM International Conference on Web Search and Data Mining, Hong Kong, China, 9–12 February 2011; King, I., Nejdi, W., Li, H., Eds.; Association for Computing Machinery: New York, NY, USA, 2011; pp. 177–186. [Google Scholar] [CrossRef]
- Wettstein, M.; Wirth, W. Media effects: How media influence voters. Swiss Polit. Sci. Rev. 2017, 23, 262–269. [Google Scholar] [CrossRef]
- Holbrook, T.M. Altered States: Changing Populations, Changing Parties, and the Transformation of the American Political Landscape; Oxford University Press: New York, NY, USA, 2016. [Google Scholar] [CrossRef]
- Dewenter, R.; Linder, M.; Thomas, T. Can media drive the electorate? The impact of media coverage on voting intentions. Eur. J. Polit. Econ. 2019, 58, 245–261. [Google Scholar] [CrossRef]
- Kaviani, M.S.; Li, L.; Maleki, H. Media, partisan ideology, and corporate social responsibility. SSRN 2021, 3658502. [Google Scholar] [CrossRef]
- Bhat, D.; Redner, S. Nonuniversal opinion dynamics driven by opposing external influences. Phys. Rev. E 2019, 100, 050301. [Google Scholar] [CrossRef]
- Bhat, D.; Redner, S. Polarization and consensus by opposing external sources. J. Stat. Mech. 2020, 2020, 013402. [Google Scholar] [CrossRef]
- Lorenz-Spreen, P.; Mørch Mønsted, B.; Hövel, P.; Lehmann, S. Accelerating dynamics of collective attention. Nat. Commun. 2019, 10, 1759. [Google Scholar] [CrossRef]
- Helfmann, L.; Djurdjevac Conrad, N.; Lorenz-Spreen, P.; Schütte, C. Modelling opinion dynamics under the impact of influencer and media strategies. arXiv 2023, arXiv:2301.13661. [Google Scholar] [CrossRef]
- Hosseiny, A.; Bahrami, M.; Palestrini, A.; Gallegati, M. Metastable features of economic networks and responses to exogenous shocks. PLoS ONE 2016, 11, 1–22. [Google Scholar] [CrossRef]
- Hosseiny, A.; Absalan, M.; Sherafati, M.; Gallegati, M. Hysteresis of economic networks in an XY model. Phys. A 2019, 513, 644–652. [Google Scholar] [CrossRef]
- Crow, J.F.; Kimura, M. An Introduction to Population Genetics Theory; The Blackburn Press: Caldwell, NJ, USA, 1970; Available online: https://archive.org/details/an-introduction-to-population-genetics-theory-pdfdrive (accessed on 9 April 2023).
- Ewens, W.J. Mathematical Population Genetics. Volume 1: Theoretical Introduction; Springer Science+Business Media New York: New York, NY, USA, 2004. [Google Scholar] [CrossRef]
- Assaf, M.; Mobilia, M.; Roberts, E. Cooperation dilemma in finite populations under fluctuating environments. Phys. Rev. Lett. 2013, 111, 238101. [Google Scholar] [CrossRef] [PubMed]
- West, R.; Mobilia, M.; Rucklidge, A.M. Survival behavior in the cyclic Lotka-Volterra model with a randomly switching reaction rate. Phys. Rev. E. 2018, 97, 022406. [Google Scholar] [CrossRef] [PubMed]
- Horsthemke, W.; Lefever, R. Noise-Induced Transitions: Theory and Applications in Physics, Chemistry, and Biology; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar] [CrossRef]
- Bena, I. Dichotomous noise: Exact results for out-of-equilibrium systems. Int. J. Mod. Phys. B 2006, 20, 2825–2888. [Google Scholar] [CrossRef]
- Ridolfi, L.; D’Odorico, P.; Laio, F. Noise-Induced Phenomena in the Environmental Sciences; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar] [CrossRef]
- Wienand, K.; Frey, E.; Mobilia, M. Evolution of a fluctuating population in a randomly switching environment. Phys. Rev. Lett. 2017, 119, 158301. [Google Scholar] [CrossRef] [PubMed]
- Wienand, K.; Frey, E.; Mobilia. Eco-evolutionary dynamics of a population with randomly switching carrying capacity. J. R. Soc. Interface 2018, 15, 20180343. [Google Scholar] [CrossRef]
- Taitelbaum, A.; West, R.; Assaf, M.; Mobilia, M. Population dynamics in a changing environment: Random versus periodic switching. Phys. Rev. Lett. 2020, 125, 048105. [Google Scholar] [CrossRef]
- Taitelbaum, A.; West, R.; Mobilia, M.; Assaf, M. Evolutionary dynamics in a varying environment: Continuous versus discrete noise. Phys. Rev. Res. 2023, 5, L022004. [Google Scholar] [CrossRef]
- Kalyuzhny, M.; Kadmon, R.; Shnerb, N.M. A neutral theory with environmental stochasticity explains static and dynamic properties of ecological communities. Ecol. Lett. 2015, 18, 572–580. [Google Scholar] [CrossRef]
- Hufton, P.-G.; Lin, Y.-T.; Galla, T.; McKane, A.J. Intrinsic noise in systems with switching environments. Phys. Rev. E 2016, 93, 052119. [Google Scholar] [CrossRef]
- Hidalgo, J.; Suweis, S.; Maritan, A. Species coexistence in a neutral dynamics with environmental noise. J. Theor. Biol. 2017, 413, 1–10. [Google Scholar] [CrossRef]
- Gardiner, C. Stochastic Methods: A Handbook for the Natural and Social Sciences; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Chakrabarti, B.K.; Acharyya, M. Dynamic transitions and hysteresis. Rev. Mod. Phys. 1999, 71, 847–859. [Google Scholar] [CrossRef]
- Mobilia, M. SimData_Figs3to6; University of Leeds: Leeds, UK, 2023. [Google Scholar] [CrossRef]
- Gillespie, D.T. A general method for numerically simulating the stochastic time evolution of coupled chemical reactions. J. Comput. Phys. 1976, 22, 403–434. [Google Scholar] [CrossRef]
- Blythe, R.A.; McKane, A.J. Stochastic models of evolution in genetics, ecology and linguistics. J. Stat. Mech. 2007, 2007, P07018. [Google Scholar] [CrossRef]
- The Guardian. Datablog. Newspaper Support in UK General Elections [Dataset]. 2010. Available online: https://www.theguardian.com/news/datablog/2010/may/04/general-election-newspaper-support (accessed on 9 April 2023).


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mobilia, M. Polarization and Consensus in a Voter Model under Time-Fluctuating Influences. Physics 2023, 5, 517-536. https://doi.org/10.3390/physics5020037
Mobilia M. Polarization and Consensus in a Voter Model under Time-Fluctuating Influences. Physics. 2023; 5(2):517-536. https://doi.org/10.3390/physics5020037
Chicago/Turabian StyleMobilia, Mauro. 2023. "Polarization and Consensus in a Voter Model under Time-Fluctuating Influences" Physics 5, no. 2: 517-536. https://doi.org/10.3390/physics5020037
APA StyleMobilia, M. (2023). Polarization and Consensus in a Voter Model under Time-Fluctuating Influences. Physics, 5(2), 517-536. https://doi.org/10.3390/physics5020037




