Josephson Junction Dynamics as a Ride on a Roller Coaster
Abstract
:1. Introduction
2. Description of a Mechanical Roller Coaster as a Model of a Josephson Junction
3. The Equation of Motion of the Mechanical Undulated Suspended Track System
4. An Exercise: Dynamical Condition for the Roller Coaster Ride
4.1. The Statics of the Cart
4.2. The Dynamics of the Cart: Integral of Motion
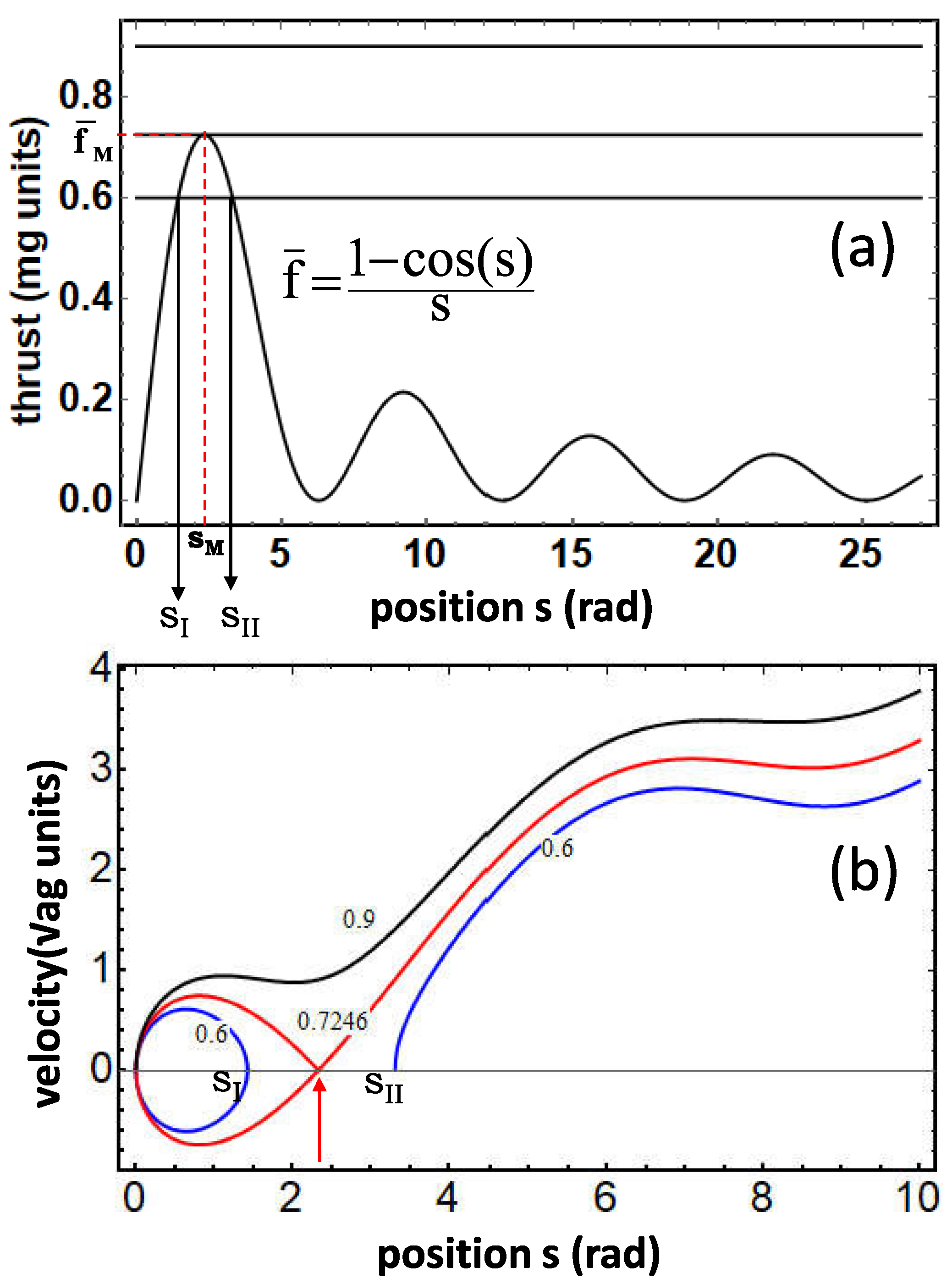
4.3. Determining the Value of the Minimum Thrust to Launch the Cart
- (a)
- , there can be no an intersection. The thrust exceeds the highest peak of the curve, that is the velocity never reaches zero along the trajectory. In this case, an open orbit is realized. The cart can well start running from the initial position (see Figure 2b, the black curve with ).
- (b)
- , the height of the first peak of the curve in Figure 1a. Now, this specific thrust is exactly the minimum thrust that is seeking, i.e., the solution to the exercise (as demonstrated in detail in Appendix C). By identifying with , the value of can be determined as follows. We solve the equationnumerically in to find , the position reached by the cart under the thrust , and calculate as (see Appendix C). One finds for the position and for the thrust threshold (see in Figure 2b, the red curve, labelled as ).In brief, is the thrust taking the cart from to the instability point , where the cart arrives with zero residual velocity. Thus, is the thrust threshold to launch the cart from . Interestingly, at the top of the track (), the cart has a velocity in units of , and the cart cannot cross this point at a lower velocity. After reaching the top of the track (or even somewhat earlier, as can be determined by energetic considerations), the engine could also be safely shut off while the cart continues to move by inertia at a constant average velocity. If the engine remains on, the velocity of the cart, in the present approximation of zero damping, continues increasing indefinitely. However, with moderate damping present, the launched cart reaches a dynamical state similar to the ‘inertial’ state where the average velocity remains constant after a transient period. In this energy balance state, the energy lost by drag is continuously provided by the engine thrust (energy balance state).
- (c)
- , two intersections occur at the points and , see Figure 2a. No running state is entered. The phase path is a closed orbit, indicating that the thrust on the cart is too weak. As a result, the cart oscillates back and forth between the origin and the point , where its velocity reverses cyclically (see Figure 2b, the closed blue curve).As far as point is concerned, this point marks a position where an open orbit can start, as Figure 2b shows. At this point, the velocity is zero, but the position is different from zero, making this solution, although possible, extraneous to the exercise. The point here is that the integral of motion, Equation (5), does not correspond uniquely to the initial conditions assumed in the exercise. The cart then describe an orbit (an open orbit in the case considered, see Figure 2b, the open blue curve) characterized by the same integral of motion, that is, also when it is set at rest in (shortly after the top) and pushed with the thrust (the integral of motion is quickly verified to be since ).
5. Further Considerations About the Launch of the Cart
5.1. Minimum Thrust for More General Initial Conditions
5.2. Quasi-Static Launch
5.3. Considerations About the Damping
6. Analogous to What? (Equation of Motion for the Phase in a Josephson Junction)
6.1. The Josephson Equations
- (a)
- The supercurrent depends on the macroscopic quantum phase difference between the two superconductors . This relationship is described by the equation (current–phase relation), where is the maximal critical current, i.e., the highest supercurrent that the junction can support.
- (b)
- When the bias current changes over time, as controlled by a current supply, a voltage difference is generated across the junction. The instantaneous value of this voltage is given by (voltage–phase relation), with ℏ the reduced Planck’s constant and e the elementary electrical charge. Note that the proportionality factor (), in the above voltage-phase relation, solely involves universal constants, which marks the macroscopic quantum nature of the effect.
6.2. CRSJ Model
6.3. Analogy Highlights
- (a)
- The bias current in the junction corresponds to the thrust on the cart. The weight of the cart corresponds to the critical current of the junction.
- (b)
- Two regimes are individuated in the dynamics of the cart: one regime when the cart remains trapped in a valley, and another regime when the cart rides on the track, according to whether the thrust f acting upon it reaches the value . In the same way, two regimes exist in the junction phase dynamics, a zero-voltage state and a finite voltage state according to whether reaches or overcomes .
- (c)
- The ‘launch attempts’ (failed attempts) experienced by the cart at the bottom of a valley of the roller coaster, under the action of an insufficient thrust, lesser than the requested weight of the cart, correspond to the zero-voltage regime of the junction. In this state, the phase difference undergoes the same kind of bound oscillations as the position of the cart experiments, and the junction is unable to develop a finite voltage state.
- (d)
- The riding of the cart on the roller coaster corresponds to the finite voltage state of the junction. In this analogy, both dynamical variables , the position of the cart (in units of a) along the track, and , the phase difference between the two superconducting electrodes of the junction, increase over time, without limits. Both the variables are characterized by a finite average rate of growth: the average velocity of the cart in the mechanical analog corresponds to the average voltage across the two electrodes in the junction.
- (e)
- Experimentally, it can be observed under measurement conditions of fast current polarization, that a JJ can switch to the finite voltage state even when reaches values lower than the critical Josephson current . Using the present didactic approach we have shown how the mechanical equivalent shows positively this peculiar effect.
6.4. Dynamical Switching and ‘Punchthrough’ Effect
7. Two-Cart Train Roller Coaster: A Josephson Interferometer
7.1. On the Statics and Dynamics of a Two-Cart Train of an Assigned Length ()
- (a)
- The equilibrium points for the center of mass , similar to the stationary points and of the single cart,
- (b)
- The limits of the static equilibrium for the two rigidly coupled cart trains. That is:Assigned H, there is a corresponding thrust, , and a corresponding position of the center of mass () that allows the train to remain stationary at . However, when the required thrust for the assigned value of H exceeds a threshold , the train can no longer maintain static equilibrium and begins to move. The threshold curve versus H is a periodic function of H and is fully defined by Equation (20).In particular, a train of length (or , or , …) does not require any force to be kept still on the track as soon as in this case, Equation (20) gives . Regardless of its position, the train remains there in a static indifferent equilibrium under the influence of gravity and tension T. In other words, an infinitesimal thrust is needed to make the train of length move against gravity along the roller coaster (surely, the friction remains to be overcome, just as a train on a well flat track). Equation (19), with and , provides the value of the tension for any position of the center of mass of the train, , as .The resulting Equation (20) is known in the Josephson effect domain. As well, Equation (20) is analogous to the form of the two-slit interference pattern of optics. The latter describes the diffraction pattern displayed by the critical current in a Josephson interferometer in response to an applied magnetic flux and is the basis of a dc SQUID magnetometer. In the analogy, the role of the flux is played by the length H of the two-cart train.
- (c)
- The critical thrust to launch the train formed by two carts (dynamical switching of a SQUID). We follow here the same consideration as that in Section 4.2. Equation (18), with the initial conditions ( and ), admits the integral of motion:Thus, the velocity as a function of the position on the track can be obtained as follows:The thrust is maximum at , where it takes the following value:
7.2. Mechanical Analog of the Self-Flux: Elastically Coupled Carts ()
8. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Schematic of a Physical Pendulum
- : angular displacement of the pendulum bowl,
- I: the moment of inertia of the composite system (bowl, rod, pulley, axis),
- r: the radius of the pulley,
- : the mass of the bowl,
- l: the length of the pendulum rod,
- F: the the external force applied to the pulley, and
- W: the weight of the pendulum bowl, .

Appendix B. Small Oscillations of the Cart
Appendix C. Demonstration that , the Value of the Minimum Thrust to Launch the Cart, Coincides with the First Peak Height of the Curve in Figure 2a
Appendix D. Time It Takes to Enter the Running State

References
- Citro, R.; Guarcello, C.; Pagano, S. Josephson junctions, superconducting circuits, and qubit for quantum technologies. In New Trends and Platforms for Quantum Technologies; Aguado, R., Citro, R., Lewenstein, M., Stern, M., Eds.; Springer: Berlin/Heidelberg, Germany, 2024; pp. 1–59. [Google Scholar] [CrossRef]
- Dejpasand, M.T.; Ghamsari, M.S. Research trends in quantum computers by focusing on qubits as their building blocks. Quant. Rep. 2023, 5, 597–608. [Google Scholar] [CrossRef]
- Kjaergaard, M.; Schwartz, M.E.; Braumüller, J.; Krantz, P.; Wang, J.I.-J.; Gustavsson, S.; Oliver, W.D. Superconducting qubits: Current state of play. Annu. Rev. Condens. Matter Phys. 2020, 11, 369–395. [Google Scholar] [CrossRef]
- Martinis, J.M. Course 13—Superconducting qubits and the physics of Josephson junctions. In Les Houches 2003: Quantum Entanglement and Information Processing; Estève, D., Raimond, J.-M., Dalibard, J., Eds.; Elsevier B.V.: Amsterdam, The Netherlands, 2004; pp. 487–489, 491–520. [Google Scholar] [CrossRef]
- Barone, A.; Paternò, G. Physics and Applications of the Josephson Effect; John Wiley & Sons, Inc.: New York, NY, USA, 1982; Chapter 6. [Google Scholar] [CrossRef]
- Mangin, P.; Kahn, R. Superconductivity. An Introduction; Springer International Publishing AG: Cham, Switzerland, 2017; Chapter 10. [Google Scholar] [CrossRef]
- Likharev, K.K. Dynamics of Josephson Junctions and Circuits; CRC Press/Taylor & Francis Group, LLC: Boca Raton, FL, USA, 1986; Available online: https://www.taylorfrancis.com/books/mono/10.1201/9781315141572/dynamics-josephson-junctions-circuits-konstantin-likharev (accessed on 15 December 2024).
- Tinkham, M. Introduction to Superconductivity; McGraw Hill, Inc.: Singapore, 1996; Available online: https://archive.org/details/introductiontosu0000mich (accessed on 15 December 2024).
- Feynman, R.P.; Leighton, R.B.; Sands, M. The Feynman Lectures on Physics; Basic Books: New York, NY, USA, 2011; Volume II, Chapter 12; Available online: https://feynmanlectures.caltech.edu/II_toc.html (accessed on 15 December 2024).
- Josephson, B.D. Possible new effects in superconductive tunnelling. Phys. Lett. 1962, 1, 251–253. [Google Scholar] [CrossRef]
- Josephson, B.D. Supercurrents through barriers. Adv. Phys. 1965, 14, 419–451. [Google Scholar] [CrossRef]
- Anderson, P.W. Special effects in superconductivity. In Lectures on the Many-Body Problem; Caianiello, E.R., Ed.; Academic Press Inc.: New York, NY, USA, 1964; Volume 2, pp. 113–135. [Google Scholar] [CrossRef]
- Sullivan, D.B.; Zimmerman, J.E. Mechanical analog of time dependent Josephson phenomena. Am. J. Phys. 1971, 39, 1504–1517. [Google Scholar] [CrossRef]
- Rochlin, G.I.; Hansma, P.K. Inexpensive mechanical model of a Josephson weak link. Am. J. Phys. 1973, 41, 878–887. [Google Scholar] [CrossRef]
- Darula, M.; Kedro, M. Dynamic reduction of the critical current in a Josephson Junction. J. Low Temp. Phys. 1990, 78, 287–296. [Google Scholar] [CrossRef]
- Fulton, T.A.; Dynes, R.C. Switching to zero voltage in Josephson tunnel Junctions. Solid State Commun. 1971, 9, 1069–1073. [Google Scholar] [CrossRef]
- Pendrill, A.-M. Acceleration in one, two and three dimensions in launched roller coasters. Phys. Educ. 2008, 43, 483–491. [Google Scholar] [CrossRef]
- De Luca, R.; Giordano, A.; D’Acunto, I. Mechanical analog of an over-damped Josephson junction. Eur. J. Phys. 2015, 36, 055042. [Google Scholar] [CrossRef]
- Josephson, B.D. Weakly coupled superconductors. In Superconductivity; Parks, R.D., Ed.; CRC Press/Taylor & Francis Group, LLC: Boca Raton, FL, USA, 1969; Chapter 9. [Google Scholar] [CrossRef]
- Tesche, C.D.; Clarke, J. dc SQUID: Noise and optimization. J. Low Temp. Phys. 1977, 29, 301–331. [Google Scholar] [CrossRef]
- Blackburn, J.A.; Cirillo, M.; Grønech-Jensen, N. A survey of classical and quantum interpretations of experiments on Josephson junctions at very low temperatures. Phys. Rep. 2016, 611, 1–59. [Google Scholar] [CrossRef]
- Scott, A.C. A nonlinear Klein–Gordon equation. Am. J. Phys. 1969, 37, 52–61. [Google Scholar] [CrossRef]
- Solymar, L. Superconductive Tunneling and Applications; Chapman and Hall Ltd; John Wiley & Sons, Inc.: London, UK, 1972; p. 202. Available online: https://archive.org/details/superconductivet0000soly/ (accessed on 15 December 2024).
- Soloviev, I.I.; Klenov, N.V.; Schegolev, A.E.; Bakurskiy, S.V.; Kupriyanov, M.Y. Analytical derivation of DC SQUID response. Supercond. Sci. Tech. 2016, 29, 094005. [Google Scholar] [CrossRef]
- Chashchina, O.; Silagadze, Z. Relativity 4-ever? Physics 2022, 4, 421–439. [Google Scholar] [CrossRef]
- Braun, O.M.; Kivshar, Y.S. The Frenkel–Kontorova Model. Concepts, Methods, and Applications; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar] [CrossRef]
- Barone, A.; Esposito, F.; Magee, C.J.; Scott, A.C. Theory and applications of the sine-Gordon equation. Riv. Nuovo Cim. 1971, 1, 227–267. [Google Scholar] [CrossRef]


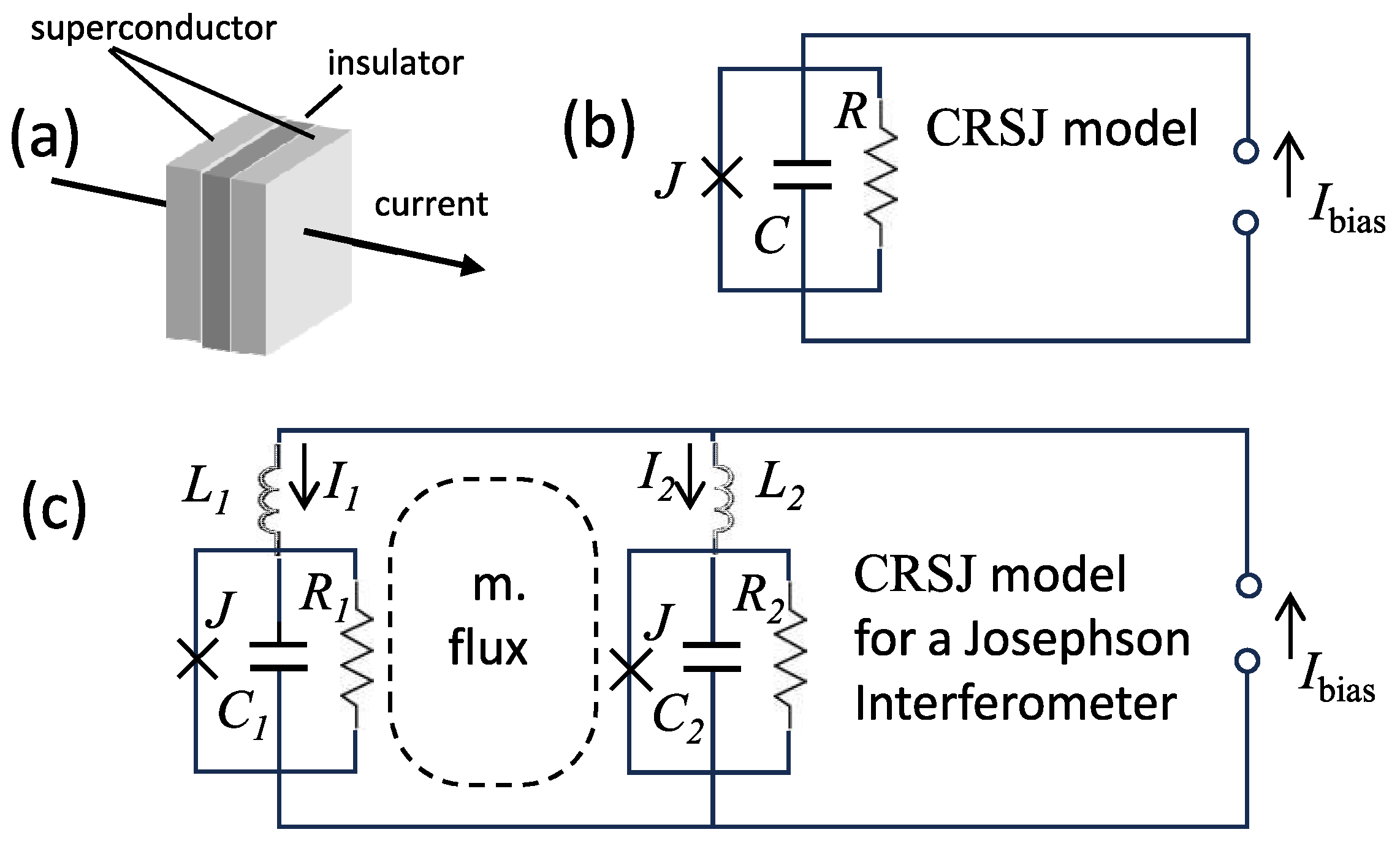


| Electric Analog (CRSJ Model) | Mechanical Analog (Cart) |
|---|---|
| junction capacitance coefficient | mass of the cart m |
| damping parameter | damping parameter |
| phase difference | dimensionless position of the cart . |
| bias current | thrust on the cart f |
| maximal Josephson critical current | weight of the cart |
| instantaneous voltage | velocity |
| Josephson plasma frequency | cart small oscillation frequency |
| Josephson current | weight parallel component |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nappi, C.; Camerlingo, C.; Cristiano, R. Josephson Junction Dynamics as a Ride on a Roller Coaster. Physics 2025, 7, 2. https://doi.org/10.3390/physics7010002
Nappi C, Camerlingo C, Cristiano R. Josephson Junction Dynamics as a Ride on a Roller Coaster. Physics. 2025; 7(1):2. https://doi.org/10.3390/physics7010002
Chicago/Turabian StyleNappi, Ciro, Carlo Camerlingo, and Roberto Cristiano. 2025. "Josephson Junction Dynamics as a Ride on a Roller Coaster" Physics 7, no. 1: 2. https://doi.org/10.3390/physics7010002
APA StyleNappi, C., Camerlingo, C., & Cristiano, R. (2025). Josephson Junction Dynamics as a Ride on a Roller Coaster. Physics, 7(1), 2. https://doi.org/10.3390/physics7010002






