Howard Flack and the Flack Parameter
Abstract
:1. Introduction
2. Impact on Service Crystallography
2.1. Background
2.2. Correction for Absorption
2.3. Determination of Absolute Structure
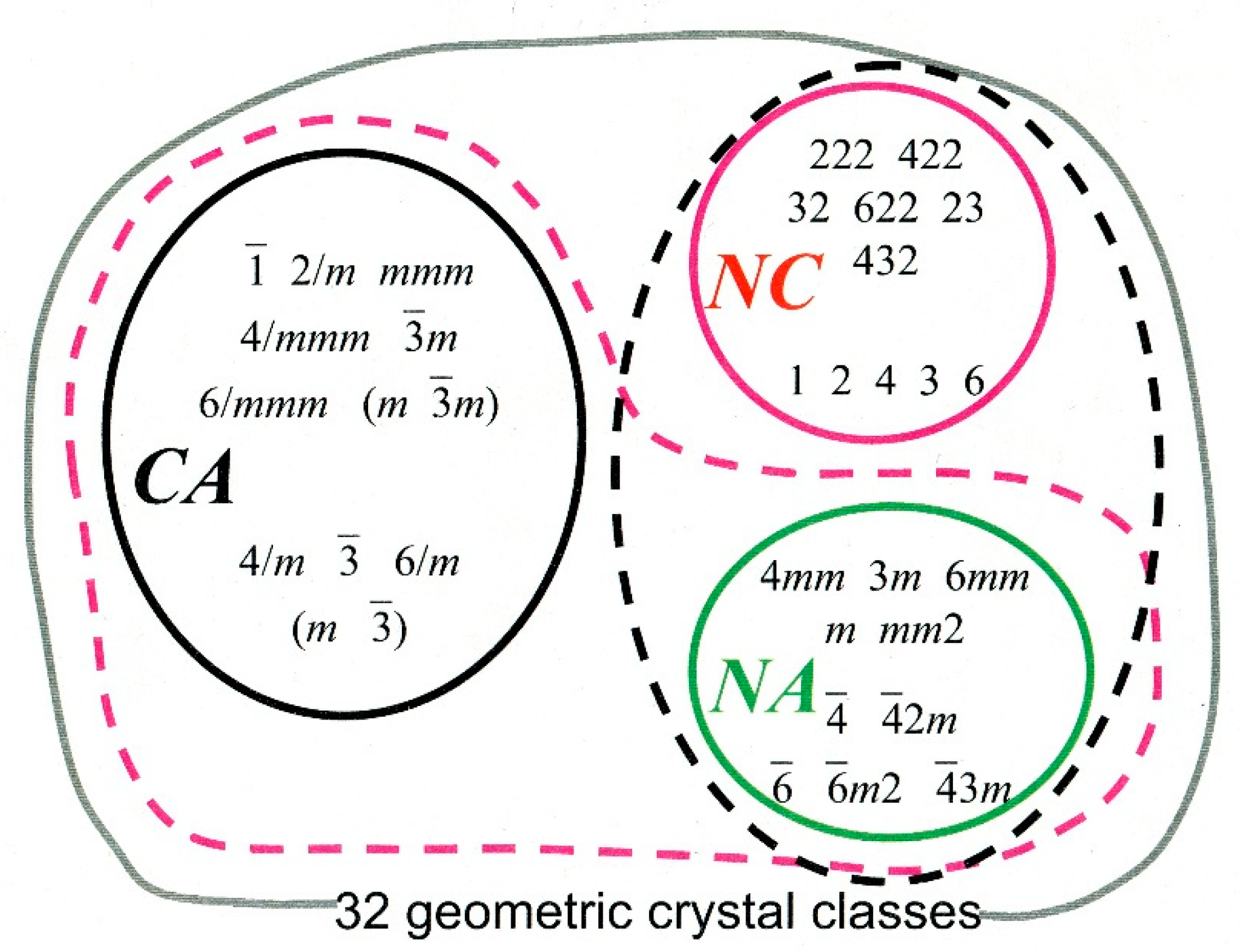
3. Twinning by Inversion
- (1)
- Spontaneous resolution, with separate crystals forming of each enantiomer.
- (2)
- Pairs of enantiomers may crystallize together form racemic crystals, with any excess concentration of one enantiomer forming enantiopure chiral crystals.
- (3)
- Formation of inversion twinned crystals, which contain contiguous domains of sufficient size to diffract coherently, some of which are related by inversion.
- (4)
- The material is achiral but forms chiral crystals.
4. Interpretation of the Flack Parameter
5. Conclusions
Practical Advice Concerning the Flack Parameter
Author Contributions
Funding
Conflicts of Interest
Appendix A. Observed and Calculated Structure Amplitudes
Appendix B. Friedel and Bijvoet Pairs
References
- Parsons, S. Determination of absolute configuration using X-ray diffraction. Tetrahedron Asymmetry 2017, 28, 1304–1313. [Google Scholar] [CrossRef] [Green Version]
- Creagh, D.C.; Hubbel, J.H. X-ray absorption (or attenuation) coefficients. In International Tables for Crystallography; Prince, E., Ed.; Springer: Heidelberg, Germany, 2006; Volume C, Section 4.2.4; pp. 220–229. [Google Scholar]
- North, A.C.T.; Phillips, D.C.; Mathews, F.S. A semi-empirical method of absorption correction. Acta Cryst. 1968, 24, 351–359. [Google Scholar] [CrossRef]
- Kopfmann, G.; Huber, R. A method of absorption correction by X-ray intensity measurements. Acta Cryst. 1968, 24, 348–351. [Google Scholar] [CrossRef]
- Flack, H.D. Automatic absorption correction using intensity measurements from azimuthal scans. Acta Cryst. 1974, 30, 569–573. [Google Scholar] [CrossRef]
- Blessing, R.H. An empirical correction for absorption anisotropy. Acta Cryst. 1995, 51, 33–38. [Google Scholar] [CrossRef] [PubMed]
- Coster, D.; Knol, K.S.; Prins, J.A. Unterschiede in der intensität der röntgenstrahlen-reflexion an den beiden 111-flächen der zinkblende. Z. Physik. 1930, 63, 345–369. [Google Scholar] [CrossRef]
- Bijvoet, J.M.; Peerdeman, A.F.; Van Bommel, A.J. Determination of the absolute configuration of optically active compounds by means of X-rays. Nature 1951, 168, 271–272. [Google Scholar] [CrossRef]
- Hamilton, W.C. Significance tests on the crystallographic R factor. Acta Cryst. 1965, 18, 502–510. [Google Scholar] [CrossRef]
- Rogers, D. On the application of hamilton’s ratio test to the assignment of absolute configuration and an alternative test. Acta Cryst. 1981, A37, 734–741. [Google Scholar] [CrossRef]
- Flack, H.D. On enantiomorph-polarity estimation. Acta Cryst. 1983, 39, 876–881. [Google Scholar] [CrossRef]
- Collet, A.; Ziminski, L.; Garcia, C.; Vigne-Maeder, F. Discrimination in crystalline enantiomer systems: Facts, interpretations, and speculations. In Supramolecular Stereochemistry; Siegel, J.S., Ed.; NATO ASI Series (Series C: Mathematical and Physical Sciences); Springer: Dordrecht, The Netherlands, 1995; Volume 473. [Google Scholar]
- Flack, H. Chiral and achiral crystal structures. Helvetica Chim. Acta 2003, 86, 905–921. [Google Scholar] [CrossRef]
- Clevers, S.; Coquerel, G. Kryptoracemic compound hunting and frequency in the cambridge structural Database. CrystEngComm 2020. [Google Scholar] [CrossRef]
- Brandel, C.; Petit, S.; Cartigny, Y.; Coquerel, G. Structural aspects of solid solutions of enantiomers. Curr. Pharm. Des. 2016, 22, 4929–4941. [Google Scholar] [CrossRef]
- Flack, H.D. The Mirror of Galadriel: Looking at Chiral and Achiral Crystal Structures; The Chemistry Research Laboratory: Oxford, UK, 2005. [Google Scholar]
- Thompson, A.L.; Watkin, D.J. CRYSTALS enhancements: Absolute structure determination. J. Appl. Cryst. 2011, 44, 1017–1022. [Google Scholar] [CrossRef]
- Tranter, G.; Le Pevelen, D.D. Chiroptical spectroscopy and the validation of crystal structure stereochemical assignments. Tetrahedron Asymmetry 2017, 28, 1192–1198. [Google Scholar] [CrossRef]
- Thompson, A.L.; Watkin, D.J. X-ray crystallography and chirality: Understanding the limitations. Tetrahedron Asymmetry 2009, 20, 712–717. [Google Scholar] [CrossRef]
- Flack, H.D. Absolute-structure reports. Acta Cryst. 2013, C69, 803–807. [Google Scholar] [CrossRef] [PubMed]
- The Quartz Page. Available online: http://www.quartzpage.de/crs_twins.html (accessed on 25 May 2020).
- Sheldrick, G.M. SHELX-76 Program for Crystal Structure Determination; University of Cambridge: Cambridge, UK, 1976. [Google Scholar]
- Hooft, R.W.W.; Straver, L.H.; Spek, A.L. Determination of absolute structure using Bayesian statistics on Bijvoet differences. J. Appl. Crystallogr. 2008, 41, 96–103. [Google Scholar] [CrossRef] [Green Version]
- Parsons, S.; Flack, H. Precise absolute-structure determination in light-atom crystals. Acta Cryst. 2004, 60, s61. [Google Scholar] [CrossRef] [Green Version]
- Parsons, S.; Wagner, T.; Presly, O.; Wood, P.A.; Cooper, R.I. Applications of leverage analysis in structure refinement. J. Appl. Crystallogr. 2012, 45, 417–429. [Google Scholar] [CrossRef] [Green Version]
- Parsons, S.; Flack, H.D.; Wagner, T. Use of intensity quotients and differences inabsolute structure refinement. Acta Cryst. 2013, B69, 249–259. [Google Scholar]
- Watkin, D.J.; Cooper, R.I. Why direct and post-refinement determinations of absolute structure may give different results. Acta Cryst. 2016, B72, 661–683. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Flack, H.; Bernardinelli, G. Reporting and evaluating absolute-structure and absolute-configuration determinations. J. Appl. Cryst. 2000, 33, 1143–1148. [Google Scholar] [CrossRef] [Green Version]
- Vijayan, M.; Ramaseshan, S. Isomorphous replacement and anomolous scattering. In International Tables for Crystallography Volume B: Reciprocal Space; Shmueli, U., Ed.; International Union of Crystallography: Chester, UK, 2010; pp. 282–296. [Google Scholar]

© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Watkin, D.J.; Cooper, R.I. Howard Flack and the Flack Parameter. Chemistry 2020, 2, 796-804. https://doi.org/10.3390/chemistry2040052
Watkin DJ, Cooper RI. Howard Flack and the Flack Parameter. Chemistry. 2020; 2(4):796-804. https://doi.org/10.3390/chemistry2040052
Chicago/Turabian StyleWatkin, David John, and Richard Ian Cooper. 2020. "Howard Flack and the Flack Parameter" Chemistry 2, no. 4: 796-804. https://doi.org/10.3390/chemistry2040052
APA StyleWatkin, D. J., & Cooper, R. I. (2020). Howard Flack and the Flack Parameter. Chemistry, 2(4), 796-804. https://doi.org/10.3390/chemistry2040052




