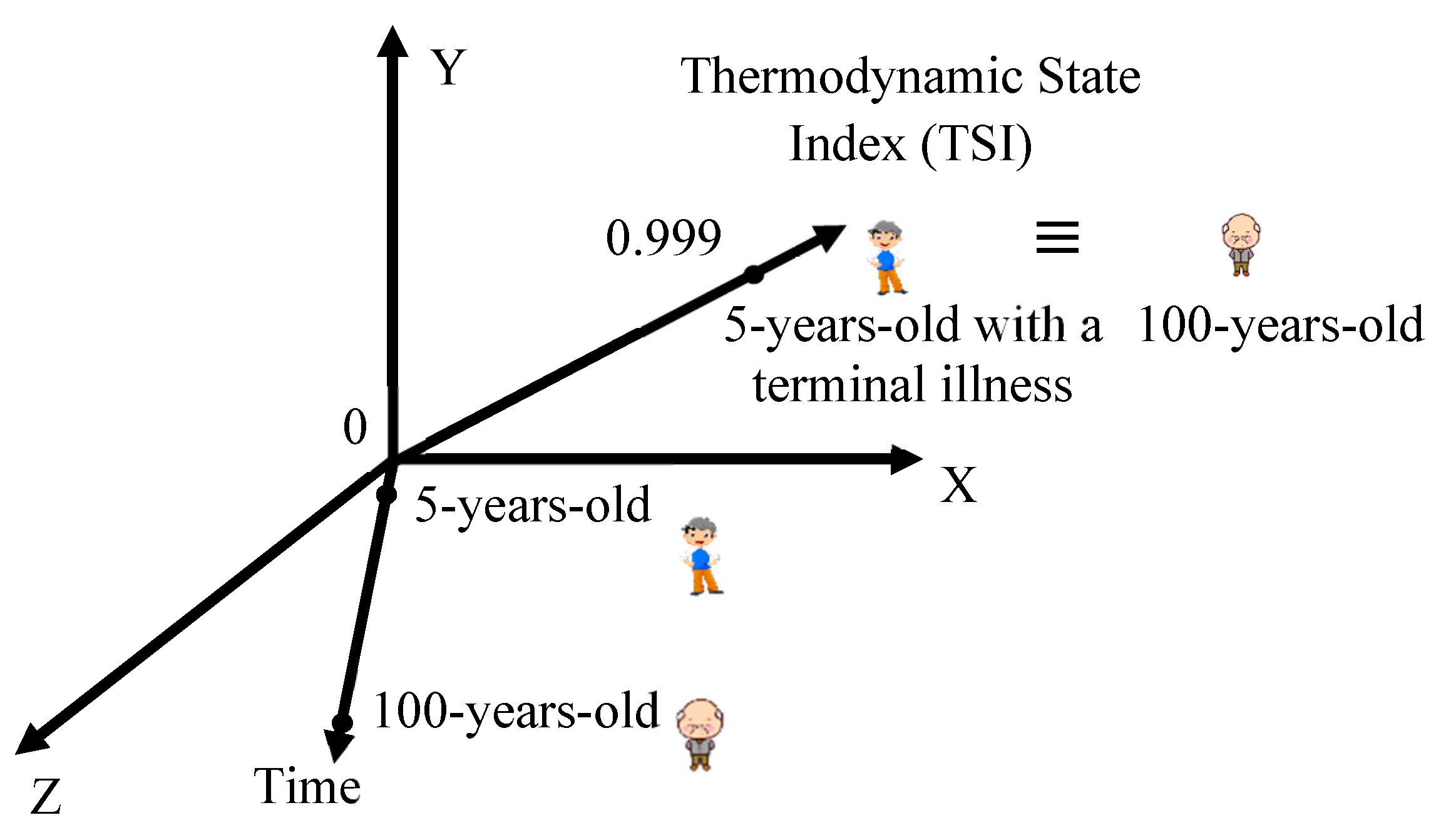
Dynamic Equilibrium Equations in Unified Mechanics Theory
Abstract
:1. Introduction
2. Derivation of the Dynamic Equilibrium Equation
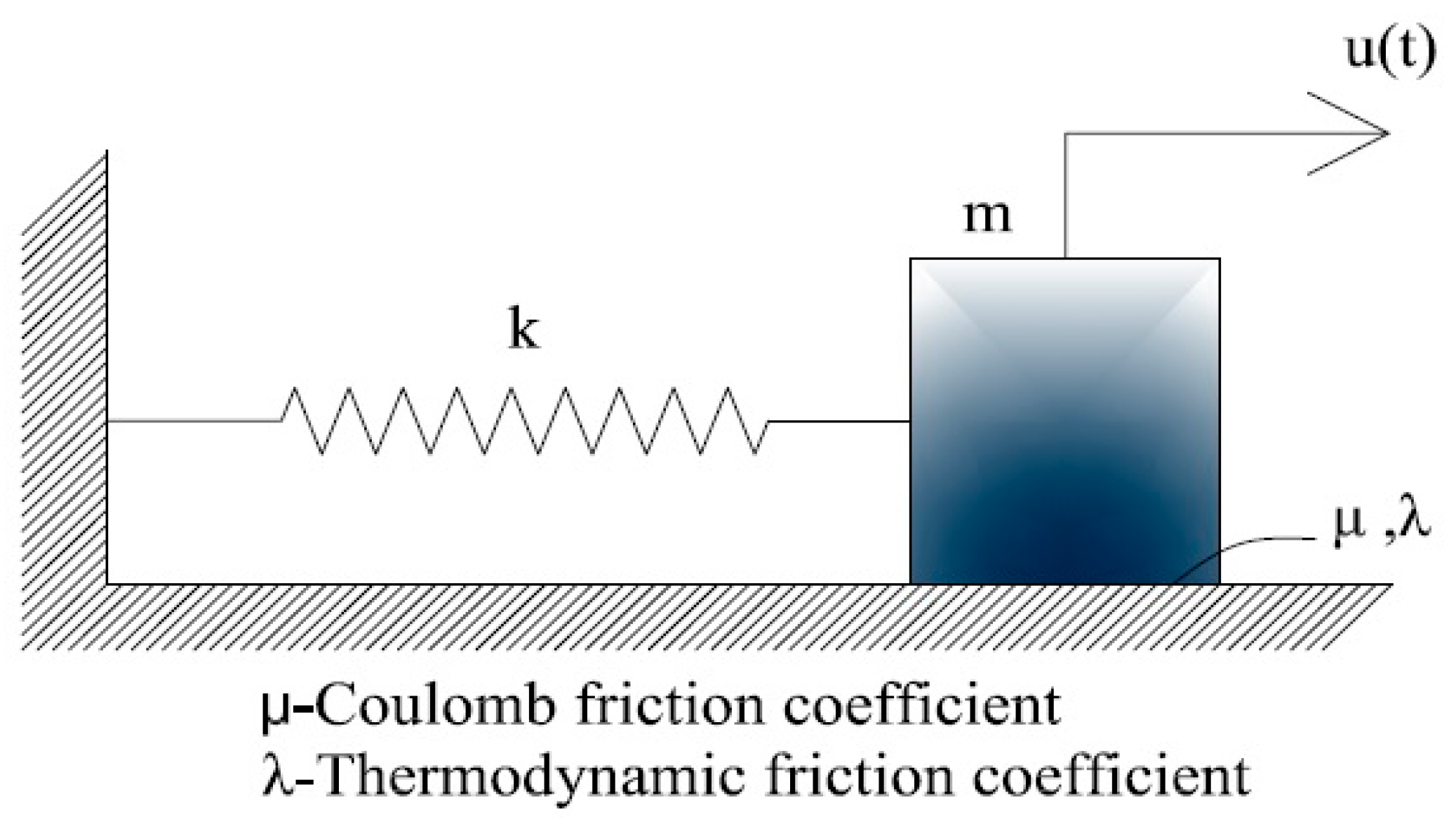
3. Application of the Equation on the Vibration of a Mass-spring System Subjected to Friction
4. Entropy Generation in Sliding Friction Contact: Thermodynamic Fundamental Equation
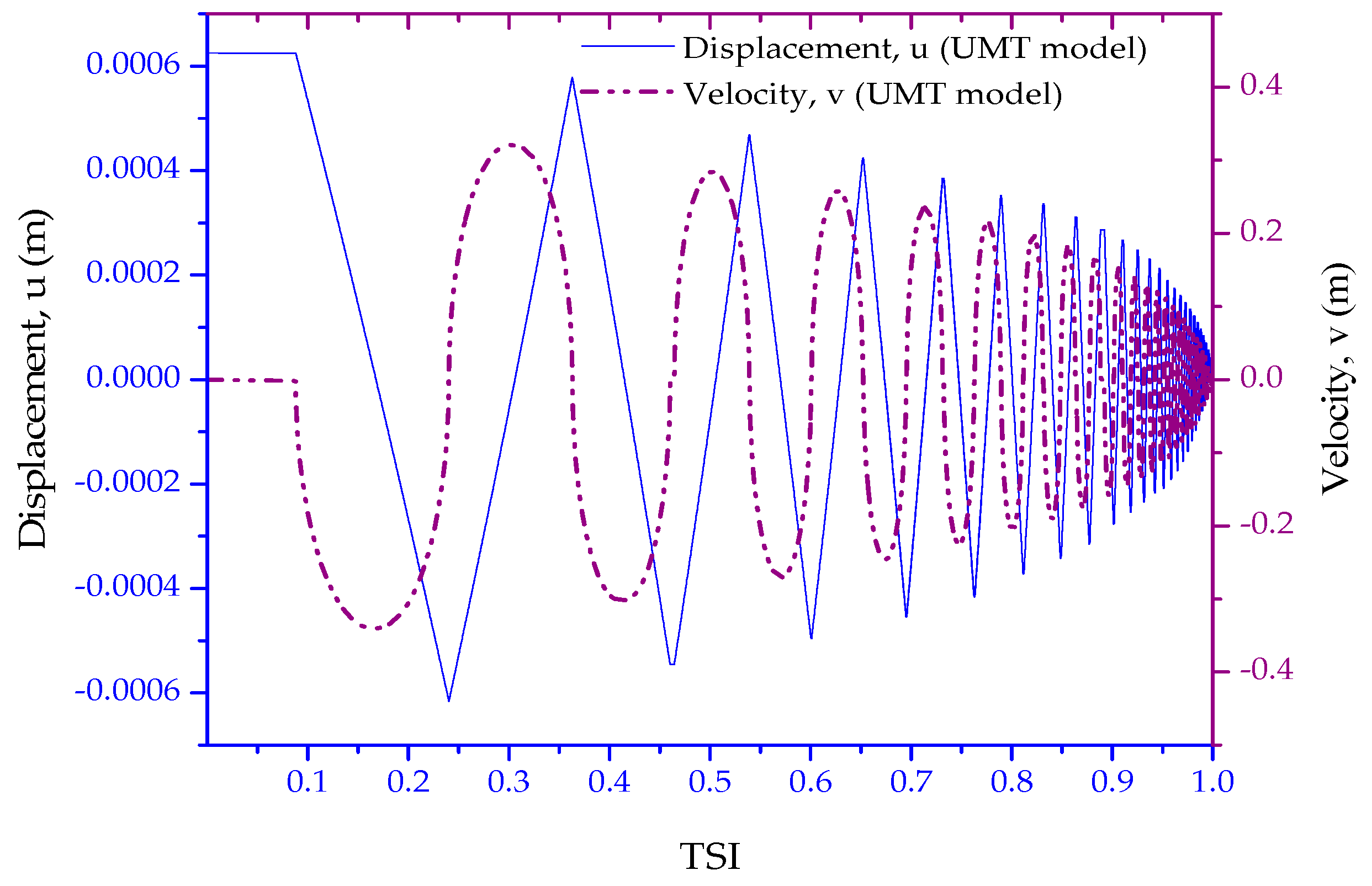
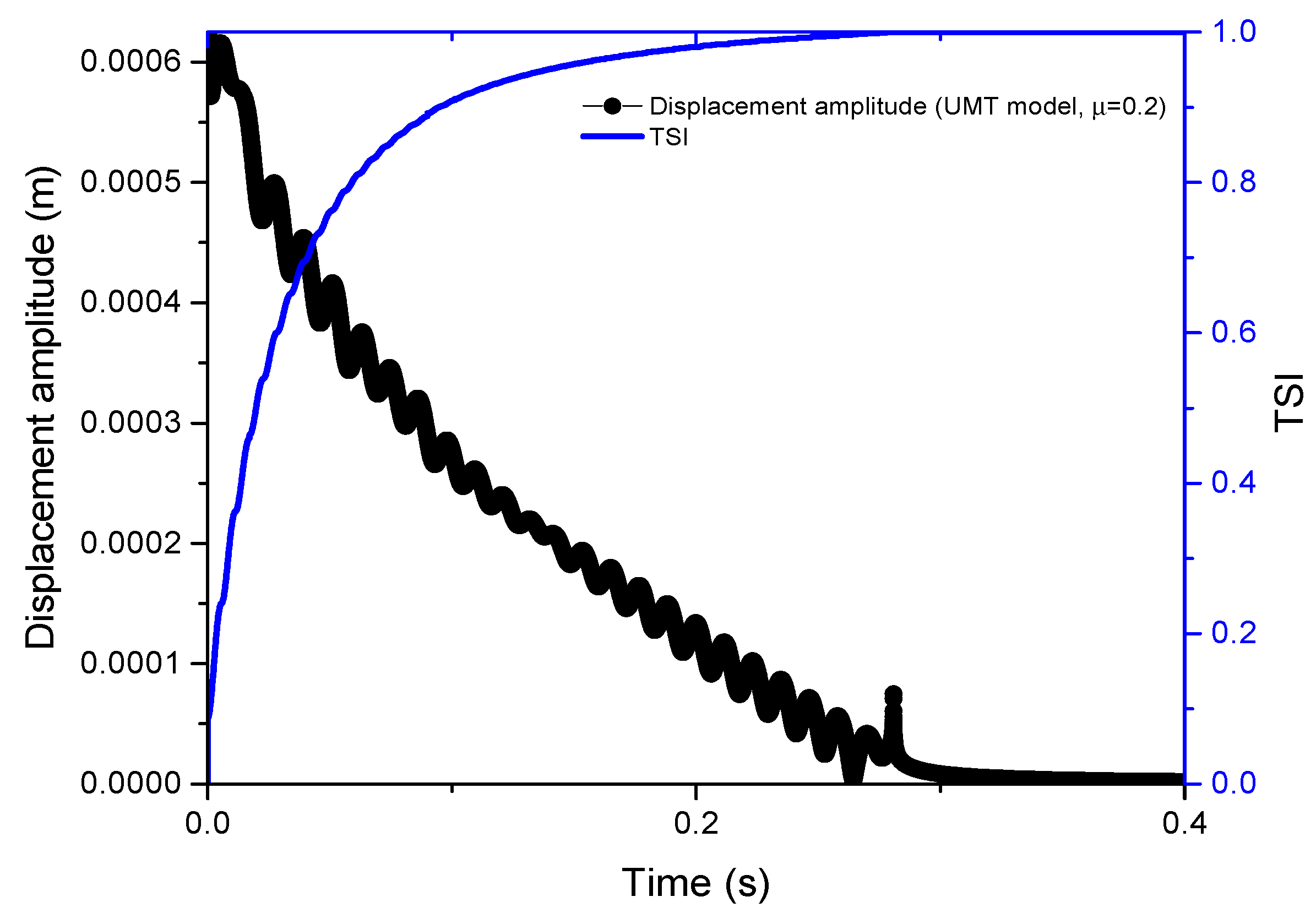
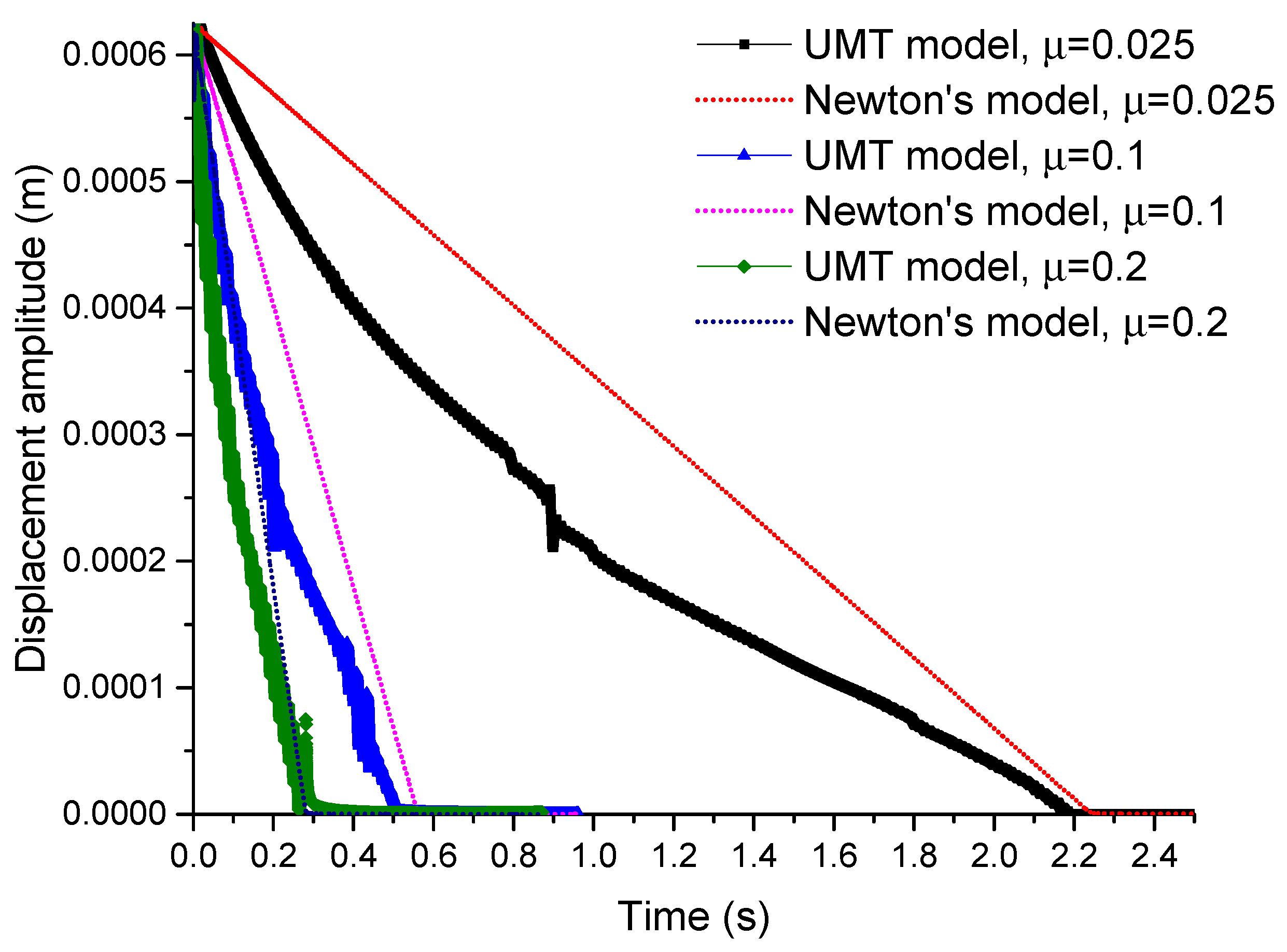
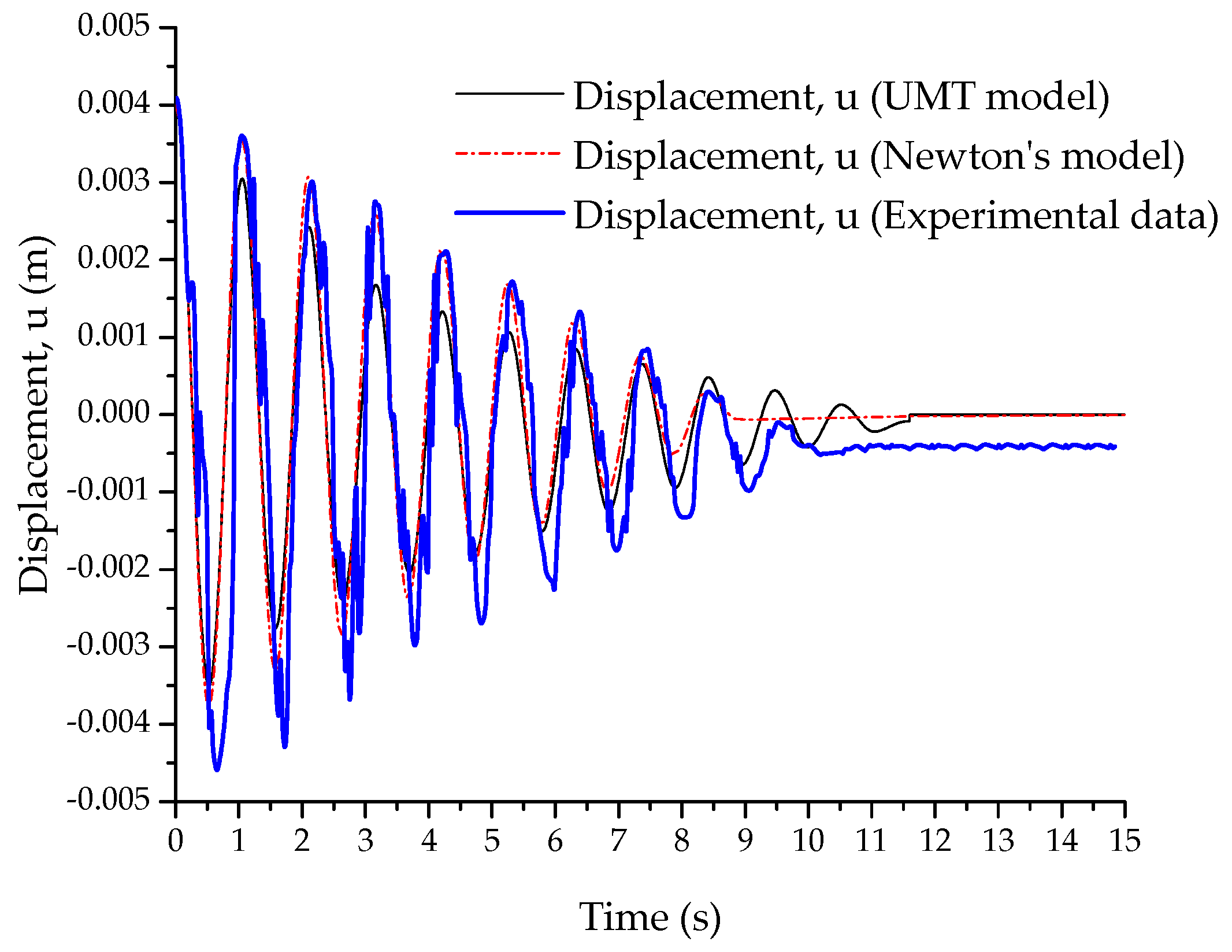
5. Results and Discussion
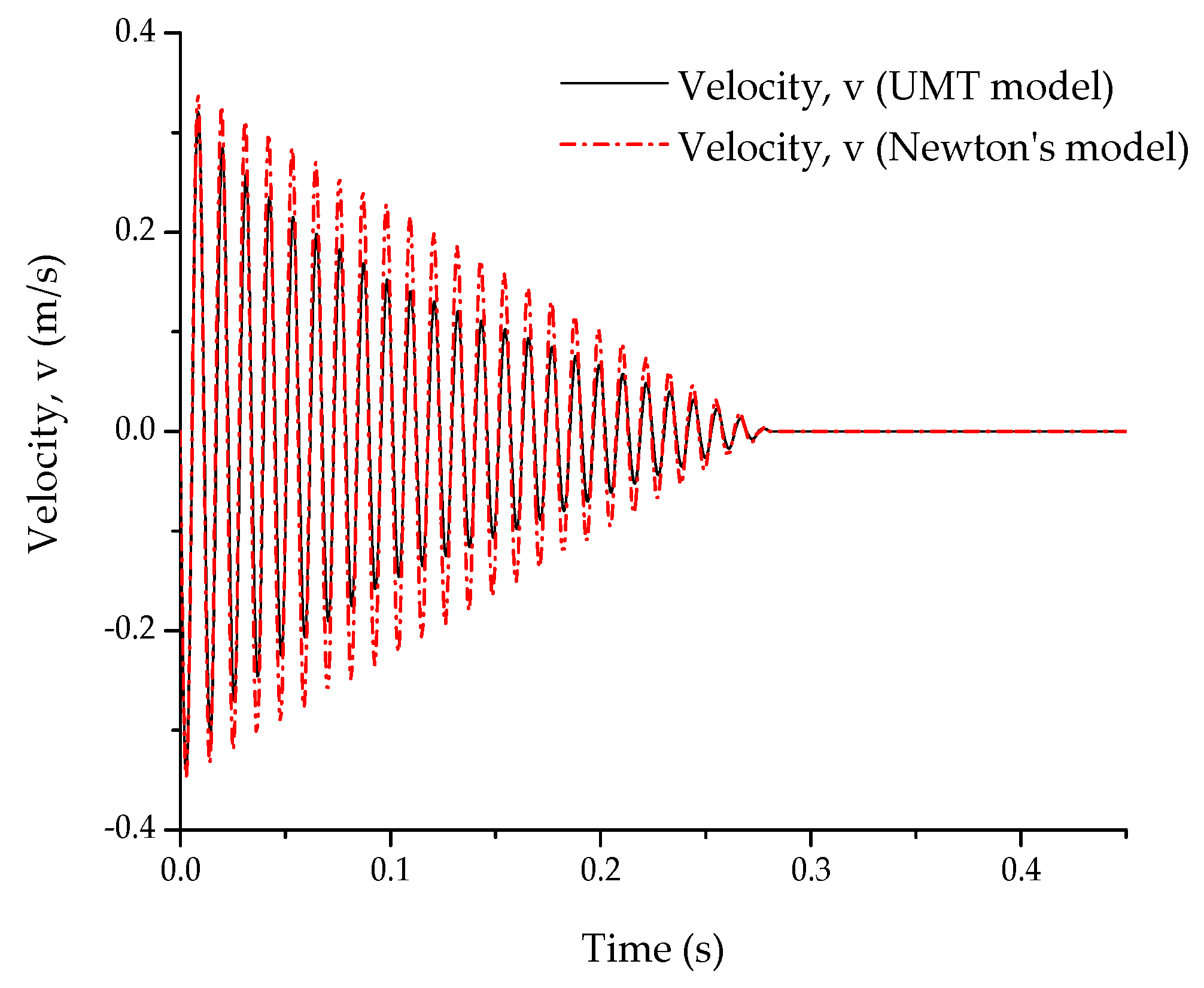
5.1. Comparison with the Newton’s Model
5.2. Comparison with Experimental Data
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- David Halliday; Resnick, R.; Walker, J. Fundamentals of Physics, 9th ed.; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Penchina, C.M. Pseudowork-energy principle. Am. J. Phys. 1978, 46, 295–296. [Google Scholar] [CrossRef]
- Silverberg, J.; Widom, A. Classical analytical mechanics and entropy production. Am. J. Phys. 2007, 75, 993–996. [Google Scholar] [CrossRef] [Green Version]
- Güémez, J.; Fiolhais, M. Dissipation effects in mechanics and thermodynamics. Eur. J. Phys. 2016, 37, 045101. [Google Scholar] [CrossRef] [Green Version]
- Basaran, C. Entropy Based Fatigue, Fracture, Failure Prediction and Structural Health Monitoring. Entropy 2020, 22, 1178. [Google Scholar] [CrossRef] [PubMed]
- Broda, E. Ludwig Boltzmann? Man, physicist, philosopher, biologist. Rheol. Acta 1982, 21, 357–359. [Google Scholar] [CrossRef]
- Boltzmann, L. On the Relation of a General Mechanical Theorem to the Second Law of Thermodynamics. In Kinetic Theory; Elsevier: Amsterdam, The Netherlands, 1966. [Google Scholar]
- Basaran, C.; Yan, C.-Y. A Thermodynamic Framework for Damage Mechanics of Solder Joints. J. Electron. Packag. 1998, 120, 379–384. [Google Scholar] [CrossRef] [Green Version]
- Basaran, C. Introduction to Unified Mechanics Theory with Applications; Springer International Publishing: Berlin/Heidelberg, Germany, 2021. [Google Scholar]
- Bin Jamal M., N.; Kumar, A.; Chebolu, L.R.; Basaran, C. Low Cycle Fatigue Life Prediction Using Unified Mechanics Theory in Ti-6Al-4V Alloys. Entropy 2020, 22, 24. [Google Scholar] [CrossRef] [Green Version]
- Basaran, C.; Nie, S. An Irreversible Thermodynamics Theory for Damage Mechanics of Solids. Int. J. Damage Mech. 2004, 13, 205–223. [Google Scholar] [CrossRef]
- Basaran, C.; Chandaroy, R. Using finite element analysis for simulation of reliability tests on solder joints in microelectronic packaging. Comput. Struct. 2000, 74, 215–231. [Google Scholar] [CrossRef]
- Basaran, C.; Lin, M. Damage mechanics of electromigration induced failure. Mech. Mater. 2008, 40, 66–79. [Google Scholar] [CrossRef]
- Basaran, C.; Lin, M. Damage mechanics of electromigration in microelectronics copper interconnects. Int. J. Mater. Struct. Integr. 2007, 1, 16. [Google Scholar] [CrossRef]
- Li, S.; Abdulhamid, M.F.; Basaran, C. Simulating Damage Mechanics of Electromigration and Thermomigration. Simulation 2008, 84, 391–401. [Google Scholar] [CrossRef]
- Gomez, J.; Basaran, C. Damage mechanics constitutive model for Pb/Sn solder joints incorporating nonlinear kinematic hardening and rate dependent effects using a return mapping integration algorithm. Mech. Mater. 2006, 38, 585–598. [Google Scholar] [CrossRef]
- Tang, H.; Basaran, C. A Damage Mechanics-Based Fatigue Life Prediction Model for Solder Joints. J. Electron. Packag. 2003, 125, 120–125. [Google Scholar] [CrossRef]
- Basaran, C.; Zhao, Y.; Tang, H.; Gomez, J. A Damage-Mechanics-Based Constitutive Model for Solder Joints. J. Electron. Packag. 2004, 127, 208–214. [Google Scholar] [CrossRef]
- M, N.B.J.; Rao, C.L.; Basaran, C. A unified mechanics theory-based model for temperature and strain rate dependent proportionality limit stress of mild steel. Mech. Mater. 2021, 155, 103762. [Google Scholar] [CrossRef]
- Basaran, C.; Nie, S. A thermodynamics based damage mechanics model for particulate composites. Int. J. Solids Struct. 2007, 44, 1099–1114. [Google Scholar] [CrossRef] [Green Version]
- Gomez, J.; Lin, M.; Basaran, C. Damage Mechanics Modeling of Concurrent Thermal and Vibration Loading on Electronics Packaging. Multidiscip. Model. Mater. Struct. 2006, 2, 309–326. [Google Scholar] [CrossRef]
- Gomez, J.; Basaran, C. Nanoindentation of Pb/Sn solder alloys; experimental and finite element simulation results. Int. J. Solids Struct. 2006, 43, 1505–1527. [Google Scholar] [CrossRef] [Green Version]
- Mostaghel, N.; Davis, T. Representations of Coulomb Friction for Dynamic Analysis. Earthq. Eng. Struct. Dyn. 1997, 26, 541–548. [Google Scholar] [CrossRef]
- Stewart, D.E. Rigid-Body Dynamics with Friction and Impact. SIAM Rev. 2000, 42, 3–39. [Google Scholar] [CrossRef] [Green Version]
- Quinn, D.D. A New Regularization of Coulomb Friction. J. Vib. Acoust. 2004, 126, 391–397. [Google Scholar] [CrossRef]
- Andreaus, U.; Casini, P. Dynamics of Friction Oscillators Excited by a Moving base and/or Driving Force. J. Sound Vib. 2001, 245, 685–699. [Google Scholar] [CrossRef]
- Shiryaev, V.; Orlik, J. A one-dimensional computational model for hyperelastic string structures with Coulomb friction. Math. Methods Appl. Sci. 2016, 40, 741–756. [Google Scholar] [CrossRef]
- Shiryaev, V.; Neusius, D.; Orlik, J. Extension of One-Dimensional Models for Hyperelastic String Structures under Coulomb Friction with Adhesion. Lubrication 2018, 6, 33. [Google Scholar] [CrossRef] [Green Version]
- Champneys, A.R.; Várkonyi, P.L. The Painlevé paradox in contact mechanics. IMA J. Appl. Math. 2016, 81, 538–588. [Google Scholar] [CrossRef] [Green Version]
- Nosonovsky, M.; Breki, A.D. Ternary Logic of Motion to Resolve Kinematic Frictional Paradoxes. Entropy 2019, 21, 620. [Google Scholar] [CrossRef] [Green Version]
- Preclik, T.; Rüde, U. Numerical Experiments with the Painlevé Paradox: Rigid Body vs. Compliant Contact. In Proceedings of the Joint International Conference on Multibody System Dynamics, Stuttgart, Germany, 29 May–1 June 2012. [Google Scholar]
- Nosonovsky, M. Entropy in Tribology: In the Search for Applications. Entropy 2010, 12, 1345–1390. [Google Scholar] [CrossRef]
- Amiri, M.; Khonsari, M.M. On the Thermodynamics of Friction and Wear―A Review. Entropy 2010, 12, 1021–1049. [Google Scholar] [CrossRef] [Green Version]
- Marion, J.B. Hamilton’s Principle—Lagrangian and Hamiltonian Dynamics. In Classical Dynamics of Particles and Systems; Elsevier: Amsterdam, The Netherlands, 1965; pp. 214–266. [Google Scholar]
- E Musielak, Z. Standard and non-standard Lagrangians for dissipative dynamical systems with variable coefficients. J. Phys. A Math. Theor. 2008, 41, 41. [Google Scholar] [CrossRef] [Green Version]
- Lazo, M.J.; Krumreich, C.E. The action principle for dissipative systems. J. Math. Phys. 2014, 55, 122902. [Google Scholar] [CrossRef] [Green Version]
- Martínez-Pérez, N.E.; Ramirez, C. On the Lagrangian description of dissipative systems. J. Math. Phys. 2018, 59, 032904. [Google Scholar] [CrossRef] [Green Version]
- Szegleti, A.; Márkus, F. Dissipation in Lagrangian Formalism. Entropy 2020, 22, 930. [Google Scholar] [CrossRef]
- Torzo, G.; Peranzoni, P. The Real Pendulum: Theory, Simulation, Experiment. Latin-American J. Phys. Educ. 2009, 3, 221–228. [Google Scholar]
- Marino, L.; Cicirello, A. Experimental investigation of a single-degree-of-freedom system with Coulomb friction. Nonlinear Dyn. 2020, 99, 1781–1799. [Google Scholar] [CrossRef] [Green Version]
- Onorato, P.; Mascoli, D.; DeAmbrosis, A. Damped oscillations and equilibrium in a mass-spring system subject to sliding friction forces: Integrating experimental and theoretical analyses. Am. J. Phys. 2010, 78, 1120–1127. [Google Scholar] [CrossRef]
- Knopoff, L.; Macdonald, G.J.F. Models for acoustic loss in solids. J. Geophys. Res. Space Phys. 1960, 65, 2191–2197. [Google Scholar] [CrossRef]
- Uday, M.B.; Fauzi, M.N.A.; Zuhailawati, H.; Ismail, A.B. Advances in friction welding process: A review. Sci. Technol. Weld. Join. 2010, 15, 534–558. [Google Scholar] [CrossRef]
- Newmark, N.M. A Method of Computation for Structural Dynamics. J. Eng. Mech. Div. 1959, 85, 67–94. [Google Scholar] [CrossRef]
- Lindfield, G.; Penny, J. Solution of Differential Equations. In Numerical Methods, 4th ed.; Academic Press: Cambridge, MA, USA, 2019; ISBN 9780128122563. [Google Scholar]
- Feeny, B.; Liang, J. A decrement method for the simultaneous estimation of coulomb and viscous friction. J. Sound Vib. 1996, 195, 149–154. [Google Scholar] [CrossRef]
- Liang, J.-W. Identifying Coulomb and viscous damping from free-vibration acceleration decrements. J. Sound Vib. 2005, 282, 1208–1220. [Google Scholar] [CrossRef]
- Leven, R.; Koch, B. Chaotic behaviour of a parametrically excited damped pendulum. Phys. Lett. A 1981, 86, 71–74. [Google Scholar] [CrossRef]
- Nedelcu, D.; Iancu, V.; Gillich, G.-R.; Bogdan, S.L. Study on the effect of the friction coefficient on the response of structures isolated with friction pendulums. Vibroeng. Procedia 2018, 19, 6–11. [Google Scholar] [CrossRef]
- Nedelcu, D.; Malin, C.-T.; Gillich, G.-R.; Petrica, A.; Padurean, I. Comparison of the performance of friction pendulums with uniform and variable radii. Vibroengineering Procedia 2019, 23, 81–86. [Google Scholar] [CrossRef]
- Hong, X.; Guo, W.; Wang, Z. Seismic Analysis of Coupled High-Speed Train-Bridge with the Isolation of Friction Pendulum Bearing. Adv. Civ. Eng. 2020, 2020, 1–15. [Google Scholar] [CrossRef]
- Min, K.-W.; Seong, J.-Y.; Kim, J. Simple design procedure of a friction damper for reducing seismic responses of a single-story structure. Eng. Struct. 2010, 32, 3539–3547. [Google Scholar] [CrossRef]
- Ribakov, Y. Semi-active predictive control of non-linear structures with controlled stiffness devices and friction dampers. Struct. Des. Tall Spéc. Build. 2004, 13, 165–178. [Google Scholar] [CrossRef]
- Seong, J.-Y.; Min, K.-W.; Kim, J.-C. Analytical investigation of an SDOF building structure equipped with a friction damper. Nonlinear Dyn. 2012, 70, 217–229. [Google Scholar] [CrossRef]
- Nammari, A.; Caskey, L.; Negrete, J.; Bardaweel, H. Fabrication and characterization of non-resonant magneto-mechanical low-frequency vibration energy harvester. Mech. Syst. Signal Process. 2018, 102, 298–311. [Google Scholar] [CrossRef]
- Witelski, T.; Virgin, L.; George, C. A driven system of impacting pendulums: Experiments and simulations. J. Sound Vib. 2014, 333, 1734–1753. [Google Scholar] [CrossRef]
- Huang, Z.; Tan, J.; Liu, C.; Lu, X. Dynamic Characteristics of a Segmented Supercritical Driveline with Flexible Couplings and Dry Friction Dampers. Symmetry 2021, 13, 281. [Google Scholar] [CrossRef]


| Parameter | Value | Unit |
|---|---|---|
| Spring stiffness, | N/m | |
| Critical TSI, | 1 | - |
| 0.003 | - | |
| Mass, | 2 | kg |
| Sliding friction coefficient, | 0.2 | - |
| Molar mass for iron, | Kg/mol | |
| Displacement at t = 0, | m |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bin Jamal M, N.; Lee, H.W.; Lakshmana Rao, C.; Basaran, C. Dynamic Equilibrium Equations in Unified Mechanics Theory. Appl. Mech. 2021, 2, 63-80. https://doi.org/10.3390/applmech2010005
Bin Jamal M N, Lee HW, Lakshmana Rao C, Basaran C. Dynamic Equilibrium Equations in Unified Mechanics Theory. Applied Mechanics. 2021; 2(1):63-80. https://doi.org/10.3390/applmech2010005
Chicago/Turabian StyleBin Jamal M, Noushad, Hsiao Wei Lee, Chebolu Lakshmana Rao, and Cemal Basaran. 2021. "Dynamic Equilibrium Equations in Unified Mechanics Theory" Applied Mechanics 2, no. 1: 63-80. https://doi.org/10.3390/applmech2010005
APA StyleBin Jamal M, N., Lee, H. W., Lakshmana Rao, C., & Basaran, C. (2021). Dynamic Equilibrium Equations in Unified Mechanics Theory. Applied Mechanics, 2(1), 63-80. https://doi.org/10.3390/applmech2010005