Applying the Action Principle of Classical Mechanics to the Thermodynamics of the Troposphere
Abstract
1. Introduction
2. Basic Theory and Methods
2.1. Complementary Fields for Virtual Quanta and Dynamics of Material Particles
- We use data from our previous action revision of the Carnot cycle [2] to examine the thermodynamics of the working fluid in expressing power from heat engines in terms of variable quantum fields. Using Carnot’s cycle, the study showed how the difference between the quantity of calorique obtained from a hot source and that absorbed by a colder sink gives the maximum power output possible from any heat engine while returning the engine to its initial thermodynamic state. The total energy (U) of the working fluid is not the source of the external work performed, but merely a scaffold for the variation in Gibbs quantum fields between the 2 distinct isothermal states that allow maximum work. A method to define the mean wavelength and frequency of the field quanta is given.
- By applying action mechanics to sustain the thermal and gravitational structure of the troposphere [7], we show how the virial theorem establishes the temperature gradient with altitude rather than adiabatic expansion. The quantum field characteristics of increasing altitude are calculated in this article, partitioned between translational and rotational energies.
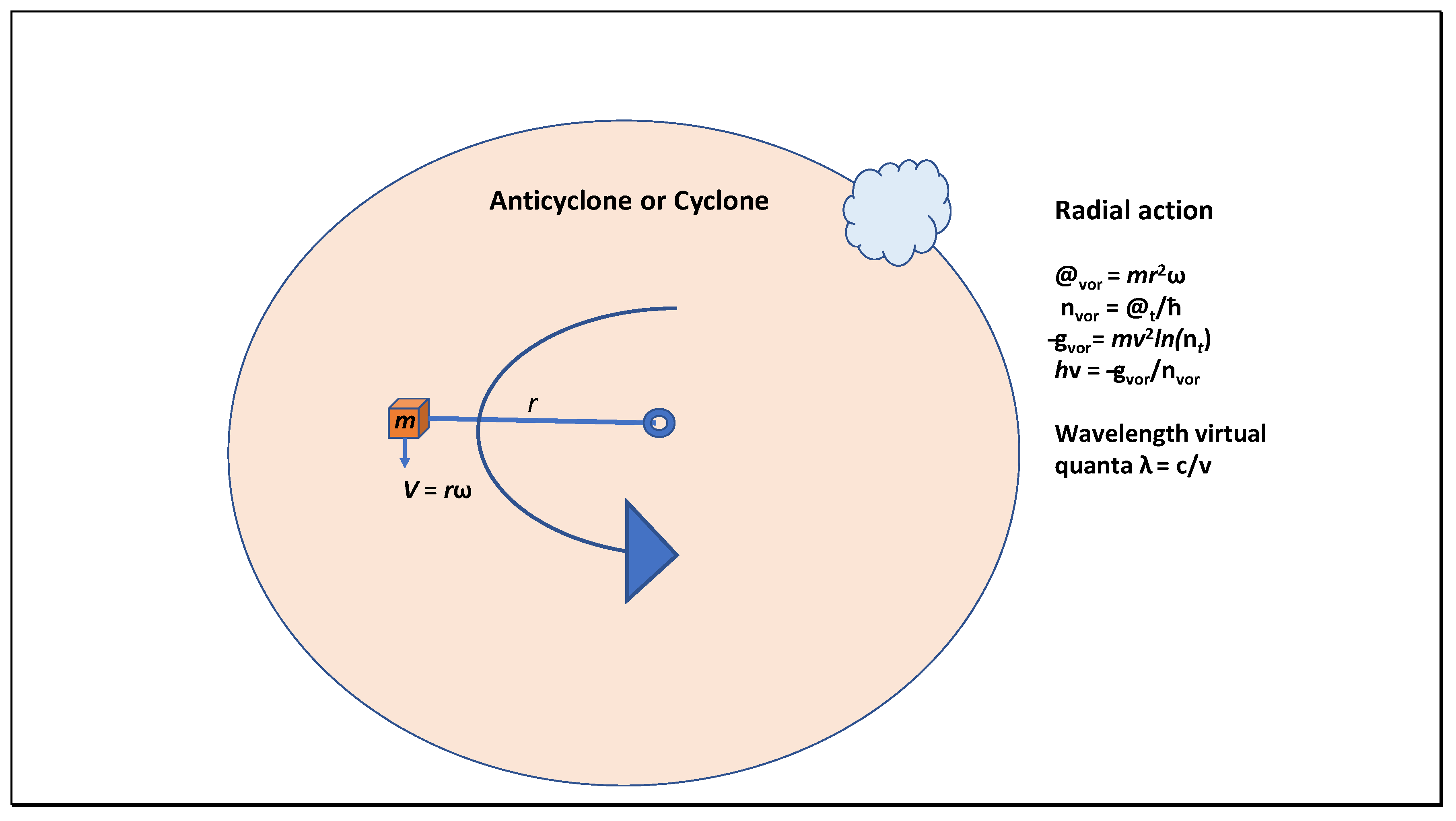
- We illustrate the role of the vortical action (ΣmR2ω) of concerted molecular flow in anticyclones and cyclones as a higher degree of freedom storing thermal energy as vortical work, capable of warming the surface by turbulent friction.
- We estimate the maximum potential of vortical wind power in the Earth’s atmosphere, already foreshadowed in an article now in review explaining a new method to calculate the maximum power of wind turbines [8].
- We explain the destructive power of tropical cyclones derived from solar heat consumed in the evaporation of seawater, which also uses vortical energy dependent on quantum impulses at the molecular level.
2.2. The Principle of Least Action Exemplified in Gibbs Field Mechanics
2.3. Mathematical Basis and Procedure for Estimating Dynamic Action and Quantum Fields
- The mean translational (or rotational) action (@t = mrvt) of molecules is estimated for the molecular field based on macroscopic concentration and temperature, including any effect of symmetry that multiplies the probability of field energy interacting with particular groups, reducing free paths. The more symmetry exhibited in a mechanical system, the lower the action and the field energy needed to sustain the system [1]. To estimate translational action (@t = mrvt), the mean velocity is required rather than the root mean square velocity, which is approximately 1.09 times less. The simple methods used in action mechanics to calculate entropy and absolute Gibbs energy based on molecular properties were applied in a paper [6] examining thermodynamics of H2 and its lysis to hydrogen atoms at the temperature of the sun’s surface. Its difficult formation by thermal decomposition of water above 4500 K and by a much easier reversible formation from ammonia in the Haber process near 400 K were shown.
- The mean number (nt) for translational quantum microstates per molecule for current mechanics is extracted by the ratio of the mean molecular action to Planck’s reduced quantum of action (n = @/ћ). For all cases examined in this article, this ratio exceeds unity by a significant margin, indicating a high entropy for this degree of freedom. For this reason, these translational processes all behave classically given the low rate of occupancy of quantum microstates.
- The mean value of virtual quanta in the field is then calculated (hv = −gt/nt), enabling the virtual frequency and wavelength in the field to be estimated. Peak values for translational quanta will reflect the vis viva, twice the kinetic energy for the Carnot cycle (mv2 = 3kT). For other processes, such as the dynamics of air molecules in wind, the vis viva involved is very low and according to wind speed, indicating a very low temperature using the 1-D relationship mv2 = kT.
3. Results and Discussion
3.1. Revising the Carnot Cycle as a Basis for a Gibbs Action Field
- At all four stages of the cycle, the relative action (@) of the working fluid calculated indicates its entropy state according to Equation (7). Gibbs energy (Gt or gt) is always zero or negative, decreasing from minimum action near absolute zero K. Uniquely, action mechanics quantifies the Gibbs field here as mean numbers of virtual quanta needed per molecule to sustain their temperature and pressure.
- Atmospheric pressure is not relevant to the enclosed Carnot cycle, so from Equation (7) all effects of changes in pressure in the cycle can be calculated as changes in Gibbs energy calculated from macroscopic temperature and pressure, given these are equivalent [2,6]. Shown in Table 1, the field of virtual quanta (Σhv) contains almost 10 times as much field energy (largely provided in melting and vaporization) as the kinetic energy of the material particles, sustaining molecular torques (mv2) and material pressures.
- Each turn of the Carnot cycle shown in Table 1 is assumed to absorb kT of heat from the hot source of quanta appropriate for the temperature and pressure and the same quantity kT removed at the colder sink as different quanta of lower frequency.
- The pressure values shown in the table also produce the ratio of torque intensity per unit volume (mv2/3a3 or kT/a3) to the negative Gibbs energy density (−gt/a3) or mean density of virtual quanta held within the mean volume a3 occupied by each molecule. For argon, this energy ratio is constant for transitions in adiabatic or isentropic states with no change in heat content. Where isothermal processes at constant temperature (or torque) occur, there is a change in this ratio as heat is added or removed.
- For nitrogen, the interaction between quantum cells for translation and rotation requires that the product of the quantum densities, shown as (nt3 × jr2), respectively, in the table, must remain constant for adiabatic processes that are isentropic, exhibiting constant action.
- The ratio for wavelength of virtual quanta and the material radial motion (λ/2πr; r = a/2) of approximately 105 for the gases is indicative of the ratio between the speed of light (λν = rω) and speed of the Brownian spiral of gas molecules. This can be visualized as the frequency of the conjugate quanta being of a similar order to that of the orbital frequency of the molecules but with the photon’s impulse cycling on a much longer radius, proportional to the ratio of speeds (c/v).
- Table 1 also illustrates the correspondence for both argon (mass 40) and nitrogen (mass 28) of the ratio of the cumulative quantum impulse (nh/λ) and the dynamic impulse (Σmv = Σmrω) per molecule. This is a factor near 1 × 10−5, the inverse of the ratio of the speed of light to that of the molecules. In calculating translational action of molecules [2] it is necessary to make two corrections. One, a factor of 1/1.09 corrects root mean square velocity from the Maxwell distribution (3kT = mv2) to mean velocity. The second corrects action for symmetry to avoid double counting of molecules (1/2). For cubic translation, this is an overall factor of 1/10.2297. This correction then allows the entropy calculated to match that for third law experiments in the literature. This correction factor (zt) was initially established empirically [1], then interpreted rationally [2]. Overall, it allows the density of quanta needed to sustain the system to fall by a factor of 2.3205.
- Another possible source for lack of correspondence in matching action impulses between quanta and molecules is that phase space for position and momentum can never exactly match true action space. The ideal coordinate system may not be Cartesian phase space since this separates variables (mv and r) that must be combined when quantized. A radial or polar system (r, ϕ, θ) is needed [7], but one that recognizes that changes in position in 3-D is absolutely quantized as jumps in the space of objects from one locus to another. There is no such thing as a smooth curve in nature for translation of rotation except by perception within the space of views, as explained a century ago by Jean Nicod [10].
3.2. Thermodynamic Stabilization with Altitude for Atmospheric Gases by Quantum Fields of Molecules in Air
3.3. Vortical Action as High-Level Atmospheric Thermodynamics in Anticyclones
3.4. Estimation of Power Produced by Wind Turbines from Vortical Energy in Anticyclones
3.5. Power in Tropical Cyclones Estimated by Heat from Volatilization on the Ocean Surface and Convective Condensation of Water at the Eyewalls
4. General Discussion
5. Conclusions
- The propositions offered here for atmospheric science are testable, both theoretically and experimentally. Certainly, the detection of the very long wavelengths of translational quanta proposed in anticyclonic winds or tropical cyclones is challenging, and new technology is needed. However, those fields proposed in the Carnot cycle are in the microwave region and for the N2 column in the atmosphere are only an order of magnitude longer. There is an acknowledged dearth of efficient detectors for wavelengths greater than far infrared or microwaves.
- A new method to estimate vortical energy is presented based on the action or quantum state of molecules. This is important as it provides a better understanding of how the Earth’s surface is heated as measured by local temperature, how wind power for wind turbines is sustained independent of kinetic energy, and how the destructive power of tropical cyclones is generated from the heat of vaporization of tropical sea water. Far more energy is stored as vortical energy than currently assumed based on laboratory measurements of heat capacity supporting internal energy. This means that heat released from turbulence caused by collisions of air masses or released by wind farms must be considered.
- Practical consequences from the separate analyses conducted in this article include (i) a new means to estimate the lapse rate in the atmosphere that can inform climate models; (ii) a method to model heat absorption in the troposphere by greenhouse gases for recycling (Figure 1) by driving vortical friction at the surface boundary layer; (iii) a new means to predict turbulent heat production downwind of wind turbines, suitable for future field studies; and (iv) a better understanding of the heat-work cycle involved in tropical cyclones, with heat radiated by condensation of water at the eyewall powering the vortical action of the cyclone. All of these findings offer opportunities for new means of gathering information by specific testing technologies.
- The quantum field hypothesis challenges the common opinion that heat is no more than the inertial motion of molecules. Instead, that molecular inertia and motion are sustained by field energy is confirmed, consistent with the density of activated quantum states as in Table 1. The smaller magnitude of translational quanta presents a difficulty for confirmation as there are few spectrometers capable of measuring the intensity of radiation at these long wavelengths. This gap in current technology is likely to be overcome in the future.
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Glossary
| @ | action or Jdϕ or Iωdϕ where I is the moment of inertia (J.s) |
| ϕ | indicates an angle, the ratio of circumference divided by radius expressed as radians or degrees (1 radian ≡ 57.296 degrees or 2π ≡ 360 degrees |
| dϕ | an infinitesimal variation in angular motion |
| ω | ≡dϕ/dt or Ω, differentiating the rate of change in angular motion with time |
| mr | inertia, expressed as mass modified by radius (r) (kg.m) |
| mr2 | moment of inertia = I |
| mr2ω | angular momentum or intensity of action (J.s) |
| mr2ω2 | energy or vis viva as torque (mv2 ≡ T) or twice the kinetic energy (J) |
| mr2ω3 | power as energy per unit time ≡ TΩ (J/s or W) |
| 1/r | curvature requiring infinite radius to achieve straight line motion or zero |
| J | energy as Joules, the work undertaken when 1 Newton acts over 1 m at constant radius from a center of force |
| J | ≡mr2ω or angular momentum (J.s) |
| S | action as ∫(T − V)dt, the time integral of the Lagrangian L, the difference between kinetic and potential energy with time (J.s) |
| S | entropy or energy per unit of temperature (J/T) |
References
- Kennedy, I.; Geering, H.; Rose, M.; Crossan, A. A Simple Method to Estimate Entropy and Free Energy of Atmospheric Gases from Their Action. Entropy 2019, 21, 454. [Google Scholar] [CrossRef] [PubMed]
- Kennedy, I.R.; Hodzic, M. Action and Entropy in Heat Engines: An Action Revision of the Carnot Cycle. Entropy 2021, 23, 860. [Google Scholar] [CrossRef] [PubMed]
- Planck, M. The Theory of Heat Radiation; Dover Publications: New York, NY, USA, 1913. [Google Scholar]
- Carnot, S. Réflexions sur la Puissance Motrice du feu et sur les Machines Propres a Developer Cette Puissance; Annales Scientifique de L’ecole Normale Superiere 2e Serie; Carnot, M.H., Ed.; Chez Bachelier: Paris, France, 1872. [Google Scholar]
- Einstein, A. On the movement of small particles suspended in a stationary liquid demanded by the molecular-kinetic theory of heat. Ann. Phys. 1905, 17, 549. [Google Scholar] [CrossRef]
- Kennedy, I.R.; Hodzic, M. Partitioning Entropy with Action Mechanics: Predicting Chemical Reaction Rates and Gaseous Equilibria of Reactions of Hydrogen from Molecular Properties. Entropy 2021, 23, 1056. [Google Scholar] [CrossRef] [PubMed]
- Kennedy, I.R. Computation of planetary atmospheres by action mechanics using temperature gradients consistent with the virial theorem. Int. J. Energy Environ. 2015, 9, 129–146. [Google Scholar]
- Kennedy, I.R.; Hodzic, M.; Crossan, A.N.; Acharige, N.; Runcie, J. A new method for estimating maximum power from wind turbines: A fundamental Newtonian approach. arXiv 2021, arXiv:2110.15117. [Google Scholar]
- Feynman, R.P. The principle of least action. In The Feynman Lectures on Physics; California Institute of Technology: Pasadena, CA, USA, 2010; Chapter 29; Volume II. [Google Scholar]
- Kennedy, I.R. Action in Ecosystems: Biothermodyamics for Sustainability; Research Studies Press: Baldock, UK; John Wiley: Baldock, UK, 2001. [Google Scholar]
- Kiehl, J.T.; Trenberth, K.E. Earth’s annual global mean energy budget. Bull. Amer. Meteor. Soc. 1997, 78, 197–208. [Google Scholar] [CrossRef]
- Tatartchenko, V.; Liu, Y.; Chen, W.; Smirnov, P. Infrared characteristic radiation of water condensation and freezing in connection with atmospheric phenomena; Part 3: Experimental data. Earth-Sci. Rev. 2012, 114, 218–223. [Google Scholar] [CrossRef]
- Montgomery, M.; Smith, R. Paradigms for tropical cyclone intensification. J. South. Hemisphere Earth Syst. Sci. 2014, 64, 37–66. [Google Scholar] [CrossRef]
- Emanuel, K.A. Some Aspects of Hurricane Inner-Core Dynamics and Energetics. J. Atmos. Sci. 1997, 54, 1014–1026. [Google Scholar] [CrossRef]
- Emanuel, K.A. Tropical cyclones. Anu. Rev. Earth Planet Sci. 2003, 31, 75–104. [Google Scholar] [CrossRef]
- Popper, K.R. Conjectures and Refutations: The Growth of Scientific Knowledge; Routledge & Kegan Paul: London, UK, 1963. [Google Scholar]
- Field, M.J.; Bash, P.A.; Karplus, M. A combined quantum mechanical and molecular mechanical potential for molecular dynamics simulations. J. Comput. Chem. 1990, 11, 700–733. [Google Scholar] [CrossRef]
- Santos, L.D.A.; Prandi, I.G.; Ramalho, T.C. Could Quantum Mechanical Properties Be Reflected on Classical Molecular Dynamics? The Case of Halogenated Organic Compounds of Biological Interest. Front. Chem. 2019, 7, 848. [Google Scholar] [CrossRef] [PubMed]
- Koehl, P.; Levitt, M. A brighter future for protein structure prediction. Nat. Struct. Biol. 1999, 6, 108–111. [Google Scholar] [CrossRef] [PubMed]
- Bartels, C. Analyzing biased Monte Carlo and molecular dynamics simulations. Chem. Phys. Lett. 2000, 331, 446–454. [Google Scholar] [CrossRef]
- Feynman, R. QED: The Strange Theory of Light and Matter. Leonardo 1991, 24, 493. [Google Scholar] [CrossRef]
- Berkowitz, R. Macroscopic systems can be controllably entangled and limitlessly measured. Phys. Today 2021, 74, 16–18. [Google Scholar] [CrossRef]
- Ockeloen-Korppi, C.F.; Damskägg, E.; Pirkkalainen, J.-M.; Asjad, M.; Clerk, A.A.; Massel, F.; Woolley, M.J.; Sillanpää, M.A. Stabilized entanglement of massive mechanical oscillators. Nature 2018, 556, 478–482. [Google Scholar] [CrossRef] [PubMed]



| Property | Stage 1 | Stage 2 | Stage 3 | Stage 4 |
|---|---|---|---|---|
| Kelvin temperature | 640–640 | 640–288 | 288–288 | 288–640 |
| Argon (Ar) | Isothermal | Isentropic | Isothermal | Isentropic |
| Radius (a/2 = r, m) | 6.410895 × 10−10 | 8.947125 × 10−10 | 13.337586 × 10−10 | 9.556798 × 10−10 |
| Pressure (kT/a3, J/m3) | 4.191891 × 106 | 1.542111 × 106 | 0.2094820 × 106 | 0.5694312 × 106 |
| Translational action (@t, J.s) | 12.43697 × 10−33 | 17.35719 × 10−33 | 17.35719 × 10−33 | 12.43697 × 10−33 |
| Mean quantum number (nt = @t,/) | 117.932 | 164.587 | 164.587 | 117.932 |
| Negative Gibbs energy (−gt, J) | 12.6446 × 10−20 (a) | 13.5282 × 10−20 (b’) | 6.0877 × 10−20 (a’) | 5.6901 × 10−20 (b) |
| Mean quantum (hv, J) | 1.07220 × 10−21 | 0.82195 × 10−21 | 0.36988 × 10−21 | 0.48249 × 10−21 |
| Energy density (gt/a3, J/m3) | 5.998728 × 107 | 2.361020 × 107 | 0.320724 × 107 | 0.814874 × 107 |
| Quantum frequency (v, Hz) | 1.61812 × 1012 | 1.24045 × 1012 | 0.55820 × 1012 | 0.72815 × 1012 |
| Wavelength (m) | 1.85272 × 10−4 | 2.41680 × 10−4 | 5.37066 × 10−4 | 4.11716 × 10−4 |
| λ/2πr (quanta/molecular) | 4.59951 × 104 | 4.29909 × 104 | 6.40870 × 104 | 6.85654 × 104 |
| Molecular frequency (ω) | 9.81843 × 1011 | 7.03521 × 1011 | 3.16585 × 1011 | 4.41829 × 1011 |
| Ratio (ν/ω) | 1.64804 | 1.76321 | 1.76321 | 1.64804 |
| Pressure ratio (gt/kT) | 14.3103 | 15.3103 | 15.3103 | 14.3103 |
| nh/λmv × 10−5 | 1.0024 c/v = 4.77 × 105 | 1.0732 c/v = 4.77 × 105 | 1.0732 c/v = 7.1 × 105 | 1.0024 c/v = 7.1 × 105 |
| Nitrogen (N2) translational | ||||
| Radius (a/2= r, m) | 6.410895 × 10−10 | 8.947125 × 10−10 | 17.40496 × 10−10 | 12.47120 × 10−10 |
| Pressure (kT/a3, J/m3) | 4.191891 × 106 | 1.542111 × 106 | 0.942669 × 105 | 2.56244 × 105 |
| Translational action (@t, J.s) | 10.40552 × 10−33 | 14.52207 × 10−33 | 18.95066 × 10−33 | 13.5787 × 10−33 |
| Mean quantum number (nt) | 98.669 | 137.703 | 179.697 | 128.758 |
| Negative Gibbs energy (−gt, J) | 12.1719 × 10−20 | 13.0555 × 10−20 | 6.1925 × 10−20 | 5.79484 × 10−20 |
| Mean quantum (hv, J) | 1.23361 × 10−21 (a) | 0.94809 × 10−21 (b’) | 0.34461 × 10−21 (a’) | 0.45006 × 10−21 (b) |
| Energy density (gt/a3, J/m3) | 5.774446 × 107 | 2.278515 × 107 | 0.146810 × 107 | 0.37345 × 107 |
| Quantum frequency (v, Hz) | 1.86172 × 1012 | 1.43082 × 1012 | 0.52007 × 1012 | 0.67921 × 1012 |
| Wavelength (m) | 1.61030 × 10−4 | 2.09525 × 10−4 | 5.76450 × 10−4 | 4.41385 × 10−4 |
| λ/2πr (quanta/molecular) | 3.99768 × 104 | 3.72712 × 104 | 5.27119 × 104 | 5.04682 × 104 |
| Molecular frequency (ω) | 11.73527 × 1011 | 8.40869 × 1011 | 2.89965 × 1011 | 4.04678 × 1011 |
| Ratio (ν/ω) | 1.58664 | 1.70159 | 1.79355 | 1.67839 |
| Pressure ratio (gt/kT) | 13.77530 | 14.77530 | 15.57381 | 14.57381 |
| nh/λmv × 10−5 | 1.1541 c/v= 3.99 × 105 | 1.2379 c/v= 3.99 × 105 | 0.8753 c/v = 1.14 × 105 | 0.8191 c/v = 1.1 × 105 |
| Nitrogen rotational | ||||
| Negative rotational Gibbs energy (−gr, J) | 4.1575 × 10−20 (a) | 4.1575 × 10−20 (b’) | 1.5534 × 10−20 (a’) | 1.5534 × 10−20 (b) |
| Mean quantum number (jr) | 10.513 | 10.513 | 7.052 | 7.052 |
| Mean quantum (hv, J) | 3.9547 × 10−21 | 3.9547 × 10−21 | 2.20268 × 10−21 | 2.20268 × 10−21 |
| Energy density (gt/a3, J/m3) | 1.97236 × 107 | 7.28400 × 106 | 0.36828 × 106 | 1.00107 × 106 |
| Frequency (v, Hz) | 5.96831 × 1012 | 5.96831 × 1012 | 3.32421 × 1012 | 3.32421 × 1012 |
| Wavelength (m) | 5.02308 × 10−5 | 5.02308 × 10−5 | 9.01846−5 | 9.01846 × 10−5 |
| λ/2πr | 1.24702 × 104 | 8.93525 × 103 | 8.24669 × 103 | 1.15092 × 104 |
| nt3 × jr2 | 1.0616259 × 108 | 2.885798 × 108 | 2.885798 × 108 | 1.0616259 × 107 |
| Alt km | Temperature K | Pressure Atm | St J/mol/K | Mean Level nt | ST Trans. kJ/mol | Mean hν(×10−22 J) Quanta | Sr J/mol/K | Mean Level nr | Mean (×10−21 J) Quanta hν | Total (St + Sr)T kJ/mol | Gibbs G kJ/mol |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 288.2 | 1.000 | 151.76 | 190.69 | 43.74 | 3.809 | 40.92 | 10.05 | 1.94900 | 55.556 | −61.225 |
| 1 | 281.7 | 0.886 | 152.28 | 194.71 | 42.90 | 3.659 | 40.73 | 9.94 | 1.91743 | 54.370 | −60.284 |
| 2 | 275.2 | 0.785 | 152.81 | 198.93 | 42.05 | 3.511 | 40.54 | 9.82 | 1.88723 | 53.210 | −59.316 |
| 3 | 268.7 | 0.692 | 153.36 | 203.34 | 41.20 | 3.446 | 40.34 | 9.70 | 1.85625 | 52.047 | −58.340 |
| 4 | 262.2 | 0.609 | 153.91 | 207.92 | 40.36 | 3.223 | 40.14 | 9.59 | 1.82303 | 50.880 | −57.365 |
| 5 | 255.7 | 0.534 | 154.48 | 212.73 | 39.50 | 3.083 | 39.93 | 9.47 | 1.79095 | 49.771 | −56.385 |
| 6 | 249.2 | 0.466 | 155.08 | 217.89 | 38.65 | 2.946 | 39.71 | 9.35 | 1.75808 | 48.544 | −55.405 |
| 7 | 242.7 | 0.401 | 155.78 | 224.09 | 37.81 | 2.802 | 39.49 | 9.22 | 1.72675 | 47.394 | −54.444 |
| 8 | 236.2 | 0.352 | 156.36 | 228.80 | 36.93 | 2.680 | 39.27 | 9.10 | 1.69318 | 46.194 | −53.475 |
| 9 | 229.7 | 0.302 | 157.00 | 235.26 | 36.06 | 2.545 | 39.04 | 8.97 | 1.66066 | 45.028 | −52.458 |
| 10 | 223.3 | 0.262 | 157.59 | 240.92 | 35.19 | 2.426 | 38.80 | 8.85 | 1.62623 | 43.854 | −51.471 |
| 11 | 216.8 | 0.224 | 158.28 | 247.67 | 34.59 | 2.319 | 38.56 | 8.72 | 1.59251 | 42.672 | −50.479 |
| 12 | 216.7 | 0.192 | 159.55 | 260.63 | 34.57 | 2.203 | 38.55 | 8.71 | 1.58327 | 42.928 | −50.737 |
| Wind Speed V (m/s) | Kinetic Energy/s 83 m Diam J | Kinetic Energy /Blade-Area/s J | Vortical Pressure, J/m3 | Vortical Power for Blade Area Watts | Power Estimated by Radial Action Model Watts |
|---|---|---|---|---|---|
| At λ = 9, pitch θ = 55° | |||||
| 5.0 | 0.21670 × 106 | 8.4066 × 103 | 0.36107 × 103 | 0.33038 × 106 | 0.031168 × 106 |
| 10.0 | 1.7336 × 106 | 6.7253 × 104 | 1.47258 × 103 | 2.69482 × 106 | 0.40541 × 106 |
| 15.0 | 5.8509 × 106 | 2.2698 × 105 | 3.35055 × 103 | 9.19727 × 106 | 1.54381 × 106 |
| 20.0 | 1.3869 × 107 | 5.3802 × 105 | 6.00353 × 103 | 21.9729 × 106 | 3.86798 × 106 |
| Wind Speed (m s−1) | Vortical Action (mrv/2 =@v)/Molecule J.s, ×1019 | Quantum Number nvor ×10−15 | 1-D Torque mv2/Mole-Cule ×10−24 J | Vortical Energy /Molecule [(mv2)ln(nvor), ×10−23 J | Vortical Energy J/m3 | Vortical Wavelength ×10−12 m | Kinetic Energy J/m3 | Ratio Vortical/ Kinetic Energy |
|---|---|---|---|---|---|---|---|---|
| 5.0 | 1.2108 | 1.14812 | 1.2108 | 4.2812 | 1062.024 | 5.3272 | 15.313 | 69.355 |
| 10.0 | 2.4215 | 2.29615 | 4.8430 | 17.1297 | 4332.826 | 2.6627 | 61.250 | 70.737 |
| 15.0 | 3.6823 | 3.49168 | 10.8968 | 38.998 | 9864.433 | 1.7786 | 137.813 | 71.758 |
| 20.0 | 4.8430 | 4.09488 | 19.372 | 69.862 | 17670.951 | 1.1643 | 245.000 | 72.127 |
| Radius km | R | 50 | 100 | 150 | 200 | 250 | 300 | 350 | 400 | 450 | 500 |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Speed m/sec | rω | 100 | 50 | 33.33 | 25 | 20 | 16.67 | 14.29 | 12.5 | 11.11 | 10 |
| @v J-sec × 1015 | nv = mr2ω/2ħ | 1.481 | 1.481 | 1.481 | 1.481 | 1.481 | 1.481 | 1.481 | 1.481 | 1.481 | 1.481 |
| Torque/molecule Joules × 10−24 | mv2 = mr2ω2 | 484.3 | 121.1 | 53.81 | 30.27 | 19.372 | 13.453 | 9.884 | 7.567 | 5.979 | 4.843 |
| −Gibbs/molecule Joules × 10−22 | mv2ln[nv] | 167.940 | 41.985 | 18.660 | 10.496 | 6.717 | 4.665 | 3.427 | 2.624 | 2.073 | 1.679 |
| Kinetic/molecule J/m3 × 10−3 | p = 1.5kT/a3 | 6.125 | 1.531 | 0.681 | 0.383 | 0.245 | 0.170 | 0.125 | 0.096 | 0.076 | 0.061 |
| −Gibbs J/m3 × 105 | p = J/m3 | 4.247 | 1.062 | 0.472 | 0.265 | 0.170 | 0.118 | 0.087 | 0.065 | 0.052 | 0.046 |
| Ratio pressures | Gibbs/kinetic | 69.3 | 69.4 | 69.3 | 69.2 | 69.4 | 69.4 | 69.6 | 68.7 | 68.4 | 75.4 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kennedy, I.R.; Hodzic, M. Applying the Action Principle of Classical Mechanics to the Thermodynamics of the Troposphere. Appl. Mech. 2023, 4, 729-751. https://doi.org/10.3390/applmech4020037
Kennedy IR, Hodzic M. Applying the Action Principle of Classical Mechanics to the Thermodynamics of the Troposphere. Applied Mechanics. 2023; 4(2):729-751. https://doi.org/10.3390/applmech4020037
Chicago/Turabian StyleKennedy, Ivan R., and Migdat Hodzic. 2023. "Applying the Action Principle of Classical Mechanics to the Thermodynamics of the Troposphere" Applied Mechanics 4, no. 2: 729-751. https://doi.org/10.3390/applmech4020037
APA StyleKennedy, I. R., & Hodzic, M. (2023). Applying the Action Principle of Classical Mechanics to the Thermodynamics of the Troposphere. Applied Mechanics, 4(2), 729-751. https://doi.org/10.3390/applmech4020037





