Determining the Deformation Characteristics of Railway Ballast by Mathematical Modeling of Elastic Wave Propagation
Abstract
:1. Introduction
2. Materials and Methods
2.1. Features of the Mathematical Model
- The model of elastic wave propagation scales well, allowing for the consideration of a sufficient length and depth of track deflection in the calculations. The spatial configuration involved in the interaction is a result of the modeling rather than being predetermined;
- It is based on solving mathematical equations for elastic waves rather than considering individual discrete elements, which is less complex in terms of input data, tuning, and computational power;
- The modeling of elastic wave propagation has a more natural physical interpretation, enabling the understanding of the influence of various factors on the behavior of the ballast and its interaction with moving trains. Such approaches are employed in mathematical models and experimental studies of the ballast layer’s condition [28].
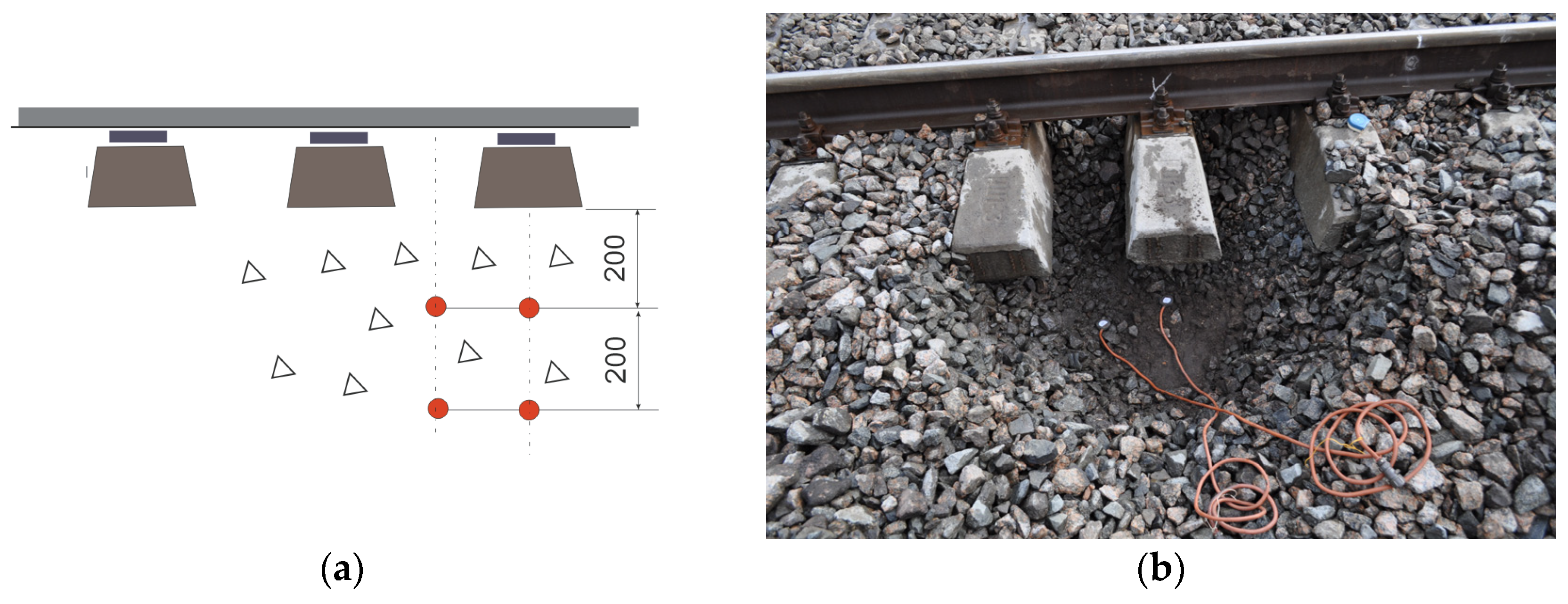
2.2. Validation of the Mathematical Model
- The difference between the modeling results and the average experimental values is small and less than expected (see the last column of Table 1);
- The deviations between the modeling results and the average experimental values are significantly smaller than the range of observations;
- The modeling results replicate the overall trend of changes in the average experimental values concerning the speed variant and ballast depth.
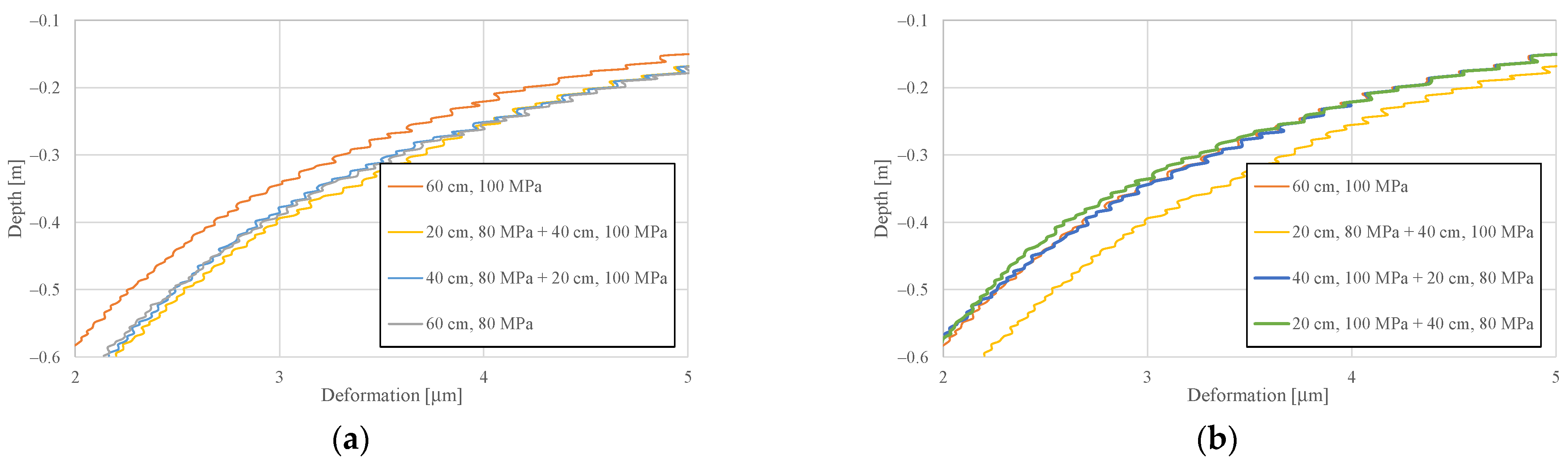
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bassey, D.; Ngene, B.; Akinwumi, I.; Akpan, V.; Bamigboye, G. Ballast Contamination Mechanisms: A Criterial Review of Characterisation and Performance Indicators. Infrastructures 2020, 5, 94. [Google Scholar] [CrossRef]
- Bassey, D.; Olukanni, D.O.; Ngene, B.U.; Bamigboye, G. Experimental characterization of track ballast under triple-fouling condition: Evidence from selected Nigerian Railways. Cogent Eng. 2021, 8, 1932240. [Google Scholar] [CrossRef]
- Indraratna, B.; Armaghani, D.J.; Correia, A.G.; Hunt, H.; Ngo, T. Prediction of resilient modulus of ballast under cyclic loading using machine learning techniques. Transp. Geotech. 2022, 38, 100895. [Google Scholar] [CrossRef]
- Wang, L.; Meguid, M.; Mitri, H. Impact of Ballast Fouling on the Mechanical Properties of Railway Ballast: Insights from Discrete Element Analysis. Processes 2021, 9, 1331. [Google Scholar] [CrossRef]
- Xiao, J.; Zhang, X.; Zhang, D.; Xue, L.; Sun, S.; Stránský, J.; Wang, Y. Morphological reconstruction method of irregular shaped ballast particles and application in numerical simulation of ballasted track. Transp. Geotech. 2020, 24, 100374. [Google Scholar] [CrossRef]
- Juhász, E.; Fischer, S. Investigation of railroad ballast particle breakage. Pollack Period. 2019, 14, 3–14. [Google Scholar] [CrossRef]
- Mohammed, A.; Amartey, Y.D.; Murana, A.A.; Lawan, A. Comparative Evaluation of Railway Ballast Degradation. FUOYE J. Eng. Technol. 2022, 7, 90–95. [Google Scholar] [CrossRef]
- Eller, B.; Fischer, S. Tutorial on the emergence of local substructure failures in the railway track structure and their renewal with existing and new methodologies. Acta Tech. Jaurinensis 2021, 14, 80–103. [Google Scholar] [CrossRef]
- Pittman, R.; Jandová, M.; Król, M.; Nekrasenko, L.; Paleta, T. The effectiveness of EC policies to move freight from road to rail: Evidence from CEE grain markets Research in Transportation. Bus. Manag. 2020, 37, 100482. [Google Scholar] [CrossRef]
- Pshinko, O.; Patlasov, O.; Andrieiev, V.; Arbuzov, M.; Hubar, O.; Hromova, O.; Markul, R. Research of railway crashed stone use of 40–70 mm fraction. In Proceedings of the 22nd International Scientific on Conference Transport Means 2018, Trakai, Lithuania, 3 October 2018; pp. 170–178. [Google Scholar]
- Shvets, A.O. Dynamic interaction of a freight car body and a three-piece bogie during axle load increase. Veh. Syst. Dyn. 2022, 60, 3291–3313. [Google Scholar] [CrossRef]
- Sysyn, M.; Przybylowicz, M.; Nabochenko, O.; Liu, J. Mechanism of Sleeper–Ballast Dynamic Impact and Residual Settlements Accumulation in Zones with Unsupported Sleepers. Sustainability 2021, 13, 7740. [Google Scholar] [CrossRef]
- Havryliuk, V. Fault Detecting of Turnouts Using DWT and ANN. In Proceedings of the 2021 IEEE International Conference on Modern Electrical and Energy Systems, Kremenchuk, Ukraine, 21–24 September 2021. [Google Scholar] [CrossRef]
- Hu, Q.; Lam, H.F.; Alabi, S.A. Use of Measured Vibration of In-Situ Sleeper for Detecting Underlying Railway Ballast Damage. Int. J. Struct. Stab. Dyn. 2015, 15, 1540026. [Google Scholar] [CrossRef]
- Fischer, S.; Eller, B.; Zoltán, K.; Németh, A. Railway Construction; Universitas Győr Nonprofit Kft.: Győr, Hungary, 2015. [Google Scholar]
- Sattari, S.; Saadat, M.; Mirtalaie, S.H.; Salehi, M.; Soleimani, A. Evaluation of Sperling’s index in passenger and freight trains under different speeds and track irregularities. Adv. Des. Manuf. Technol. 2022, 15, 87–96. [Google Scholar] [CrossRef]
- Sharma, S.K.; Sharma, R.C.; Lee, J. Effect of Rail Vehicle-Track Coupled Dynamics on Fatigue Failure of Coil Spring in a Suspension System. Appl. Sci. 2021, 11, 2650. [Google Scholar] [CrossRef]
- Dižo, J.; Blatnický, M. Influence of stiffness characteristics of a railway track on output parameters in a multibody model. In Proceedings of the 7th International Conference of Materials and Manufacturing Engineering (ICMMEN), Thessaloniki, Greece, 2–3 July 2020; EDP Sciences: Les Ulis, France, 2020; Volume 318, p. 01003. [Google Scholar] [CrossRef]
- Sattari, S.; Saadat, M.; Mirtalaie, S.H.; Salehi, M.; Soleimani, A. Modeling of a rail suspension system to investigate vertical vibration and effective parameters on it. Int. J. Railw. Res. 2022, 9, 1–20. [Google Scholar]
- Wehbi, M.; Musgrave, P. Optimisation of Track Stiffness on the UK Railways. Perm. Way Inst. 2017, 135, 24–31. [Google Scholar]
- Balcı, E.; Bezgin, N.Ö. Analytical Estimation of Dynamic Impact Forces on Railway Tracks Considering Railway Track and Rolling Stock Stiffness. In Proceedings of the 8th International Geotechnical Symposium, Istanbul, Turkey, 13–15 November 2019. [Google Scholar]
- Lamprea Pineda, A.C.; Connolly, D.P.; Hussein, M.F.M. Beams on elastic foundations—A review of railway applications and solutions. Transp. Geotech. 2021, 33, 100696. [Google Scholar] [CrossRef]
- Balamonica, K.; Bergamini, A.; Damme, B.V. Estimation of the dynamic stiffness of railway ballast over a wide frequency range using the discrete element method. J. Sound Vib. 2023, 547, 117533. [Google Scholar] [CrossRef]
- Suhr, B.; Six, K. Efficient DEM simulations of railway ballast using simple particle shapes. Granular Matter 2022, 24, 114. [Google Scholar] [CrossRef] [PubMed]
- Tolomeo, M.; McDowell, G.R. DEM study of an “avatar” railway ballast with real particle shape, fabric and contact mechanics. Granular Matter 2023, 25, 32. [Google Scholar] [CrossRef]
- Kurhan, D.; Kurhan, M. Modeling the Dynamic Response of Railway Track. In IOP Conference Series: Materials Science and Engineering; IOP Science: Bristol, UK, 2019; Volume 708, p. 012013. [Google Scholar] [CrossRef]
- Kurhan, D.; Fischer, S. Modeling of the Dynamic Rail Deflection using Elastic Wave Propagation. J. Appl. Comput. Mech. 2022, 8, 379–387. [Google Scholar] [CrossRef]
- Sysyn, M.; Gerber, U.; Liu, J.; Fischer, S. Studying the Relation of the Residual Stresses in the Ballast Layer to the Elastic Wave Propagation. Transp. Infrastruct. Geotechnol. 2022. [Google Scholar] [CrossRef]
- Sysyn, M.; Gerber, U.; Kovalchuk, V.; Nabochenko, O. The complex phenomenological model for prediction of inhomogeneous deformations of railway ballast layer after tamping works. Arch. Transp. 2018, 47, 91–107. [Google Scholar] [CrossRef] [Green Version]
- Kurhan, D. Determination of Load for Quasi-static Calculations of Railway Track Stress-strain State. Acta Tech. Jaurinensis 2016, 9, 83–96. [Google Scholar] [CrossRef] [Green Version]
- Cescon, J.T.A.M.; de Albuquerque e Silva, B.-H.; Marque, M.E.S.; Santos, R.P. Evaluation of the viability of recycling railroad ballast for reusing in railroads. Res. Soc. Dev. 2021, 10, e277101321231. [Google Scholar] [CrossRef]
- Jideani, T.; Gräbe, H. Identifying Suitable Ballast Settlement Models and Types of Ballast Breakage from Field Representative Ballast Box Tests. In Proceedings of the Fifth International Conference on Railway Technology, Edinburgh, UK, 22–25 August 2022. [Google Scholar]
- Wang, H.; Xing, C.; Deng, X. Effect of Random Lateral Ballast Resistance on Force-Deformation Characteristics of CWR with a Small-Radius Curve. Materials 2023, 16, 2876. [Google Scholar] [CrossRef]





| Depth in Ballast [cm] | Speed, [km/h] | Measured Stresses [kPa] | Number of Passes | Calculated Stresses, [kPa] | Model Adequacy Criterion, | |||
|---|---|---|---|---|---|---|---|---|
| Mean, | Standard Deviation, | Minimum | Maximum | |||||
| 20 | 60 | 60.1 | 9.73 | 46.3 | 80.2 | 8 | 65.7 | 0.578 |
| 80 | 59.0 | 9.76 | 40.4 | 84.1 | 20 | 66.4 | 0.765 | |
| 120 | 59.6 | 10.18 | 35.6 | 81.5 | 12 | 67.8 | 0.811 | |
| 140 | 63.5 | 9.87 | 41.5 | 82.7 | 15 | 68.5 | 0.505 | |
| 176 | 64.8 | 6.91 | 55.3 | 80.5 | 10 | 69.8 | 0.718 | |
| 40 | 60 | 41.6 | 5.61 | 33.7 | 54.9 | 8 | 37.8 | 0.675 |
| 80 | 41.8 | 5.76 | 30.8 | 55.5 | 20 | 38.2 | 0.622 | |
| 120 | 41.8 | 6.32 | 28.9 | 55.6 | 12 | 39.0 | 0.444 | |
| 140 | 39.6 | 6.33 | 27.7 | 52.4 | 15 | 39.4 | 0.042 | |
| 176 | 39.4 | 5.27 | 31.4 | 52.3 | 10 | 40.1 | 0.127 | |
| Initial Characteristics | Simulation Results | ||||
|---|---|---|---|---|---|
| Deformation Modulus of the Ballast Layer [MPa] | Deformation Modulus of the Soil [MPa] | Deformation Modulus of the “Ballast” Object [MPa] | Deformation Modulus of the “Sub-Rail” Object [MPa] | ||
| 0–20 cm | 20–40 cm | 40–60 cm | |||
| 100 | 20 | 100 | 26.0 | ||
| 100 | 35 | 100 | 41.2 | ||
| 80 | 100 | 87 | 38.7 | ||
| 80 | 100 | 80 | 37.1 | ||
| 80 | 80 | 37.1 | |||
| 60 | 60 | 31.9 | |||
| 90 | 90 | 39.2 | |||
| 120 | 120 | 44.4 | |||
| 150 | 150 | 48.5 | |||
| 120 | 150 | 118 | 44.1 | ||
| 200 | 200 | 53.7 | |||
| 160 | 200 | 161 | 49.6 | ||
| 80 | 25 | 80 | 31.4 | ||
| 100 | 100 | 34.4 | |||
| 80 | 100 | 105 | 31.1 | ||
| 150 | 150 | 39.8 | |||
| 120 | 150 | 148 | 36.6 | ||
| 200 | 200 | 43.4 | |||
| 160 | 200 | 195 | 40.6 | ||
| 80 | 50 | 80 | 43.4 | ||
| 100 | 100 | 48.9 | |||
| 80 | 100 | 81 | 43.4 | ||
| 120 | 120 | 53.3 | |||
| 150 | 150 | 59.1 | |||
| 120 | 150 | 117 | 52.9 | ||
| 200 | 200 | 66.5 | |||
| 160 | 200 | 159 | 60.6 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kurhan, D.; Kurhan, M.; Horváth, B.; Fischer, S. Determining the Deformation Characteristics of Railway Ballast by Mathematical Modeling of Elastic Wave Propagation. Appl. Mech. 2023, 4, 803-815. https://doi.org/10.3390/applmech4020041
Kurhan D, Kurhan M, Horváth B, Fischer S. Determining the Deformation Characteristics of Railway Ballast by Mathematical Modeling of Elastic Wave Propagation. Applied Mechanics. 2023; 4(2):803-815. https://doi.org/10.3390/applmech4020041
Chicago/Turabian StyleKurhan, Dmytro, Mykola Kurhan, Balázs Horváth, and Szabolcs Fischer. 2023. "Determining the Deformation Characteristics of Railway Ballast by Mathematical Modeling of Elastic Wave Propagation" Applied Mechanics 4, no. 2: 803-815. https://doi.org/10.3390/applmech4020041
APA StyleKurhan, D., Kurhan, M., Horváth, B., & Fischer, S. (2023). Determining the Deformation Characteristics of Railway Ballast by Mathematical Modeling of Elastic Wave Propagation. Applied Mechanics, 4(2), 803-815. https://doi.org/10.3390/applmech4020041








