Site-Specific Seismic Analysis of Buildings Supported by Lightly Reinforced Precast Concrete Walls
Abstract
1. Introduction
2. Guidance for Site-Specific Seismic Analysis of Precast RC Walls
2.1. Simplified Push-Over Analysis
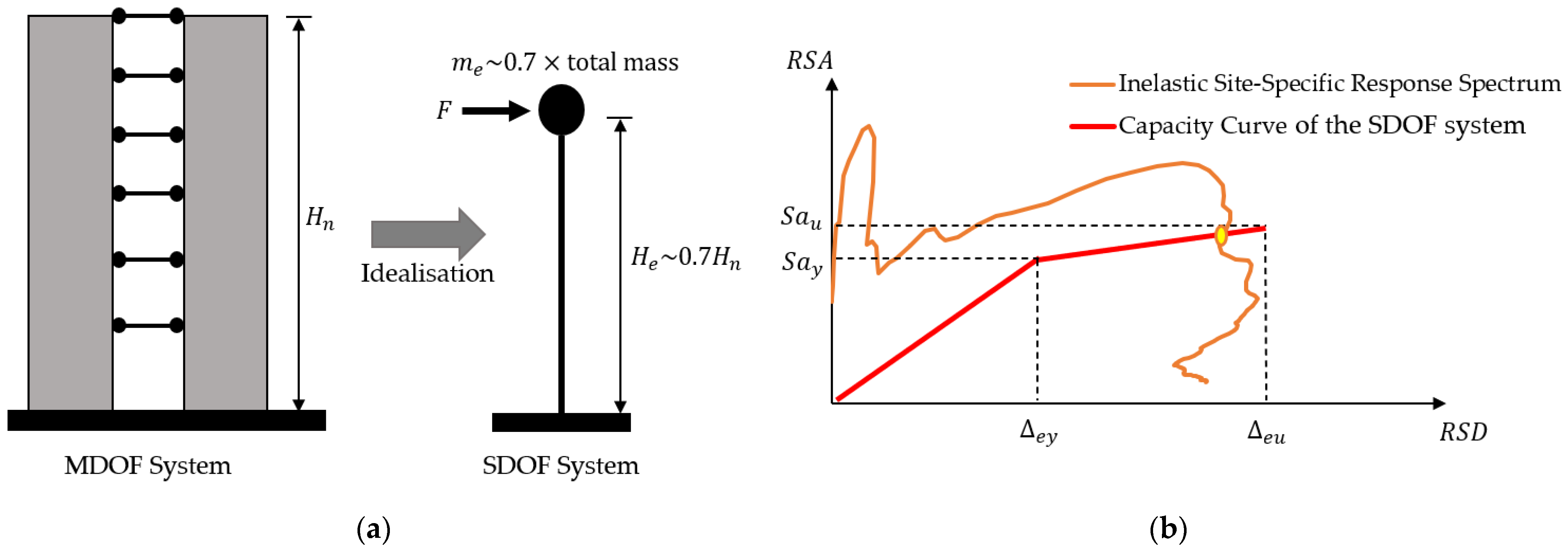
- Step 1: Idealisation of a multi-degree-of-freedom (MDOF) wall building to an equivalent single-degree-of-freedom (SDOF) system. The effective mass () and height () of the equivalent SDOF system can be estimated using Equations (1) and (2), assuming that the deflection shape of the building is triangular:
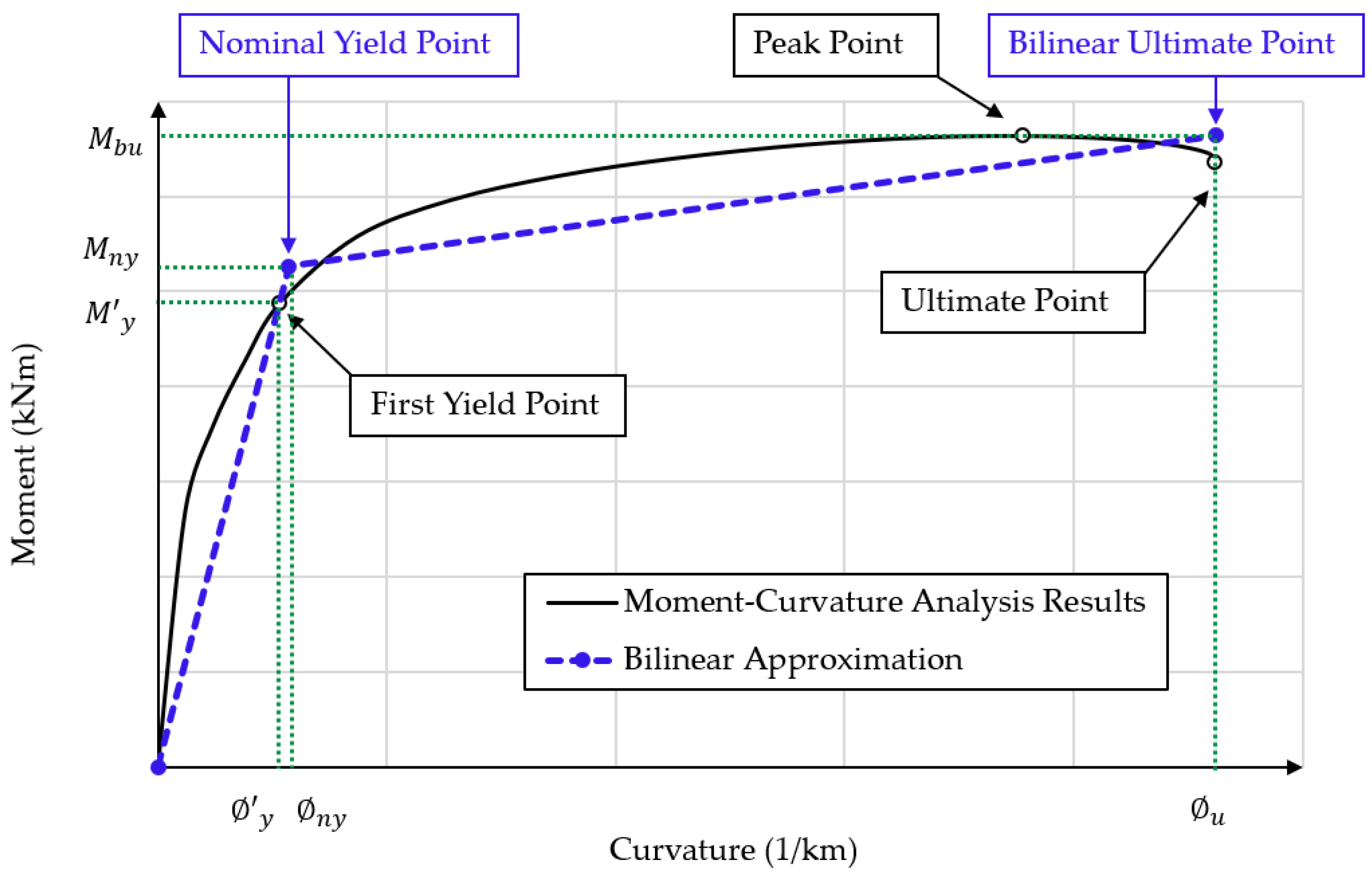
- Step 2: Derivation of the capacity curve of the equivalent SDOF system, which represents the global seismic performance of a complex MDOF building structure. The capacity curve is typically presented in a bi-linear format with two controlling points: the nominal yield point and the ultimate point. For regular low-to-medium-rise buildings supported by lightly reinforced precast walls, sectional-based moment–curvature analysis (or fibre-element analysis) and plastic hinge analysis can be used to determine the bi-linear force–displacement capacity curve. Section 2.2 of this paper summarises the procedures for conducting moment–curvature analysis for the critical section of lightly reinforced precast walls and then calculating the force–displacement response using plastic hinge analysis, as provided in Section 2.3.
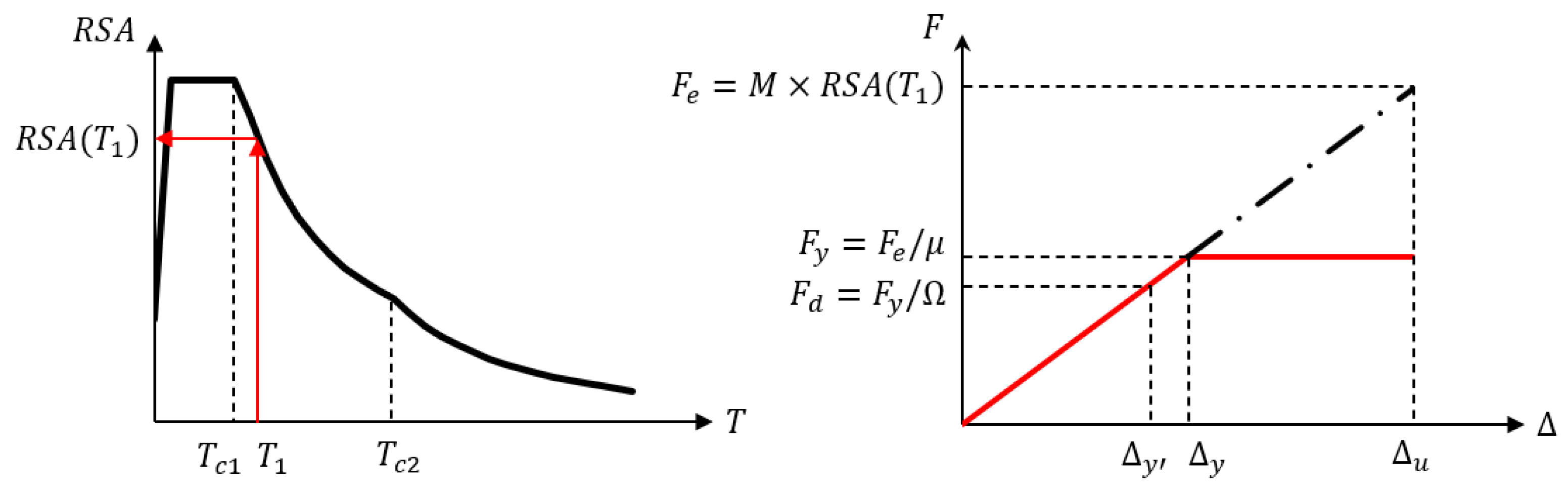
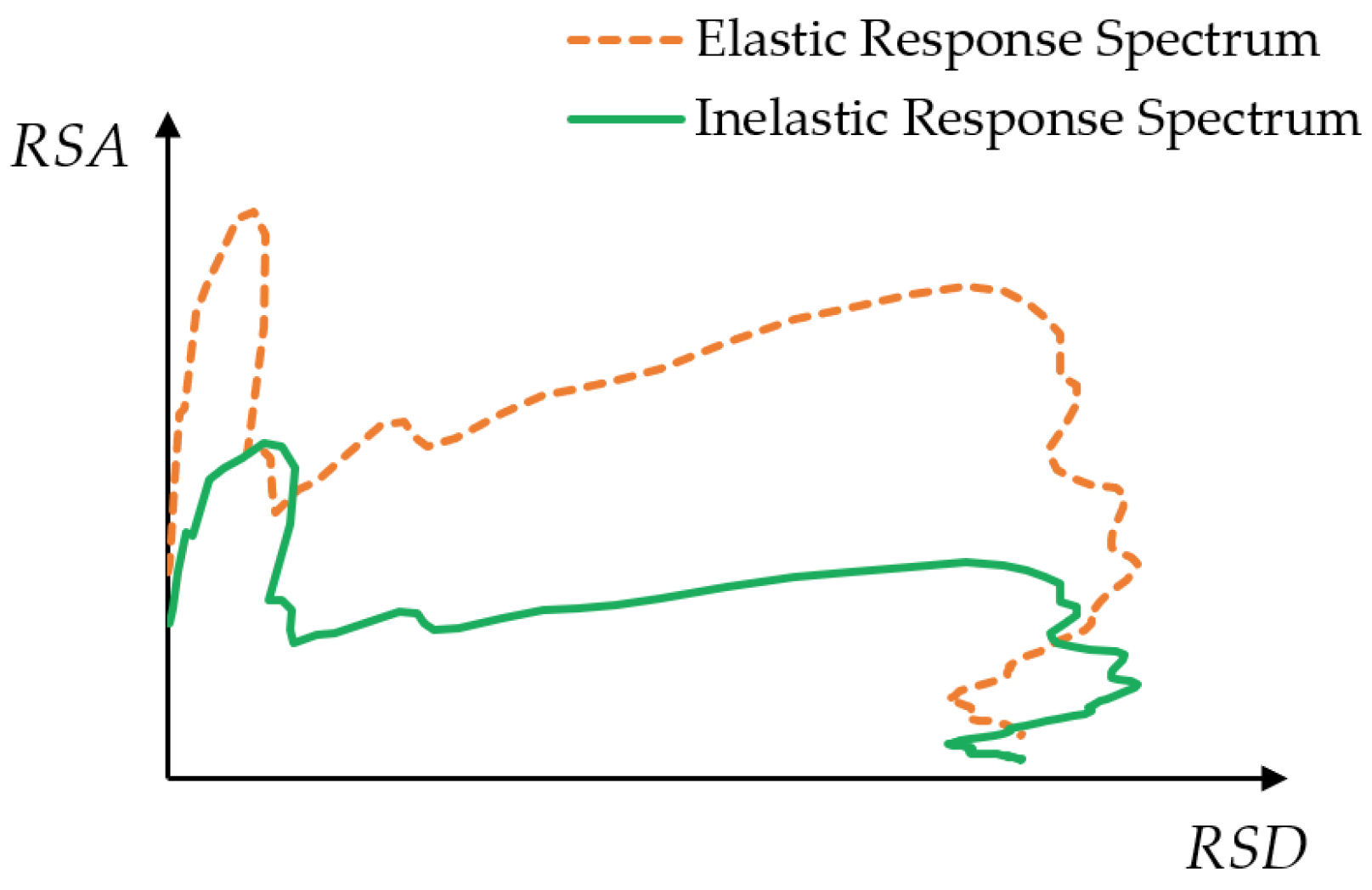
- Step 3: Derivation of the site-specific response spectra (i.e., demand curves). The discussion on the derivation of the site-specific response spectra is out of the scope of this paper. Readers can refer to Hu et al. [3] and Khatiwada et al. [6] for more details. As a demonstration, Section 3 of this paper applies the site-specific response spectra developed by Khatiwada et al. [6] to the seismic analysis of a case study building supported by lightly reinforced precast walls. It is noted that since the capacity curve represents the inelastic behaviour of structures, elastic demand curves should also be converted to inelastic curves using Equations (3)–(5) from Fajfar [36], as illustrated in Figure 4. The value of the overstrength factor () can be determined as per a seismic loading code (e.g., [1]) by assuming that the lightly reinforced precast walls are non-ductile. The ductility factor () is the ratio of the ultimate displacement () and the yield displacement (), as shown in Figure 3b, obtained from the capacity curve. The corner period () is the period at the intersection of the acceleration-controlled region and the velocity-controlled region in an elastic code response spectrum (i.e., the constraining period of the acceleration-controlled region) [36].
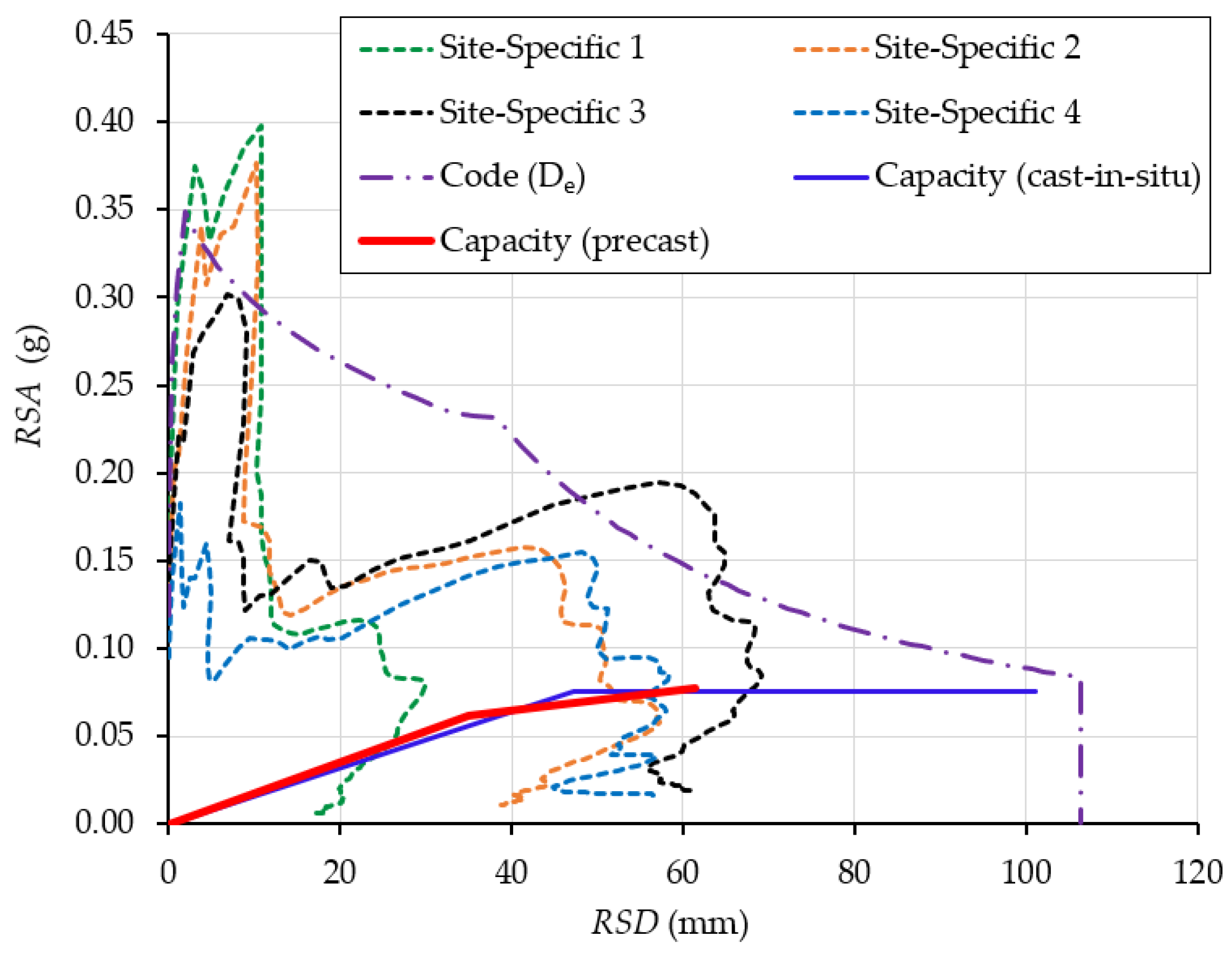
- Step 4: Superimposition of the capacity curve and demand curves: if the ultimate point of the capacity curve exceeds the envelope of the demand curves, the seismic performance of the examined precast wall is satisfactory. Otherwise, the structural elements are vulnerable to seismic ground motions. Additionally, intersections of the capacity and demand curves are often called ‘performance points’, which can be used to further optimise the design solution.
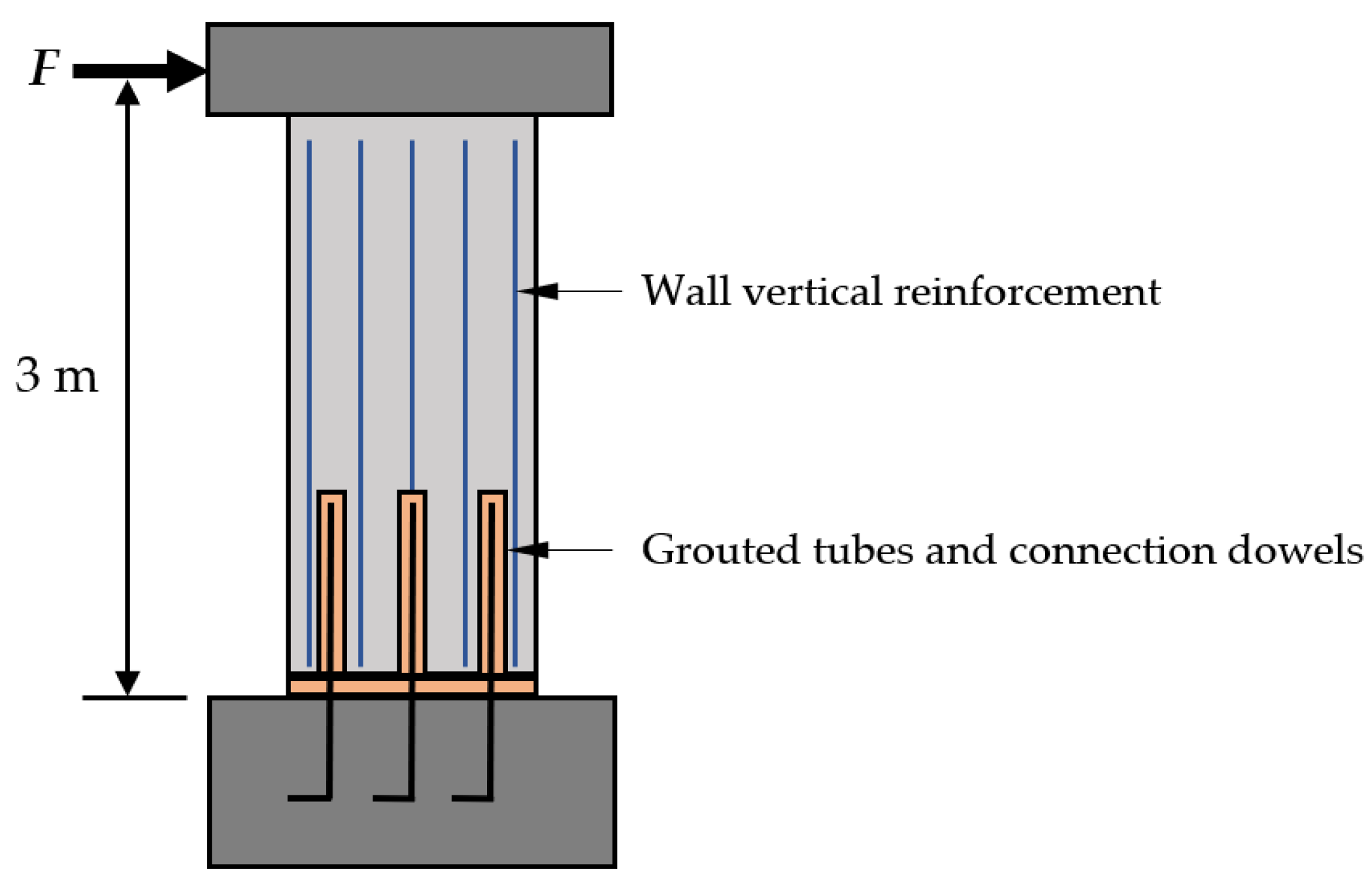
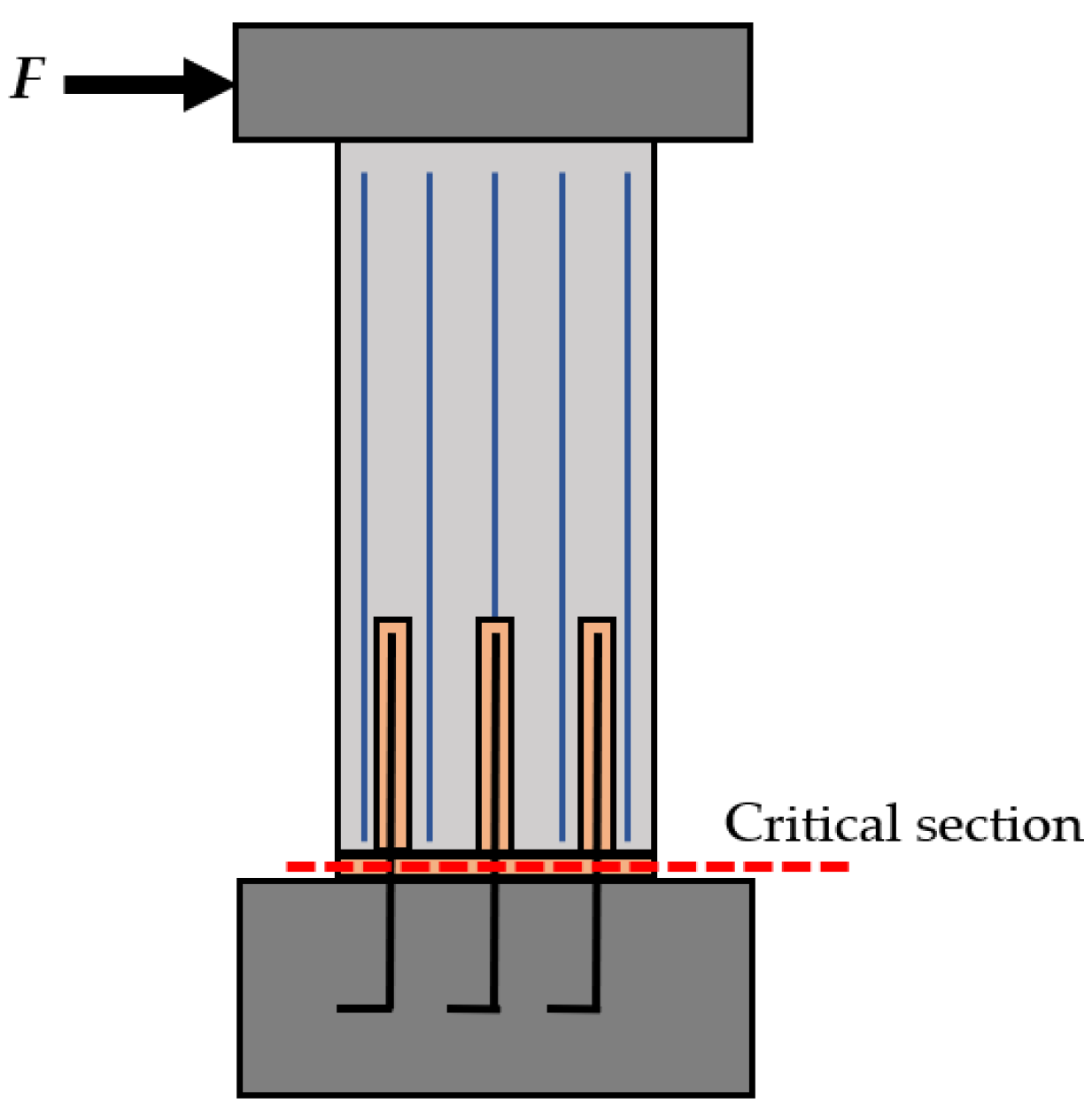
2.2. Moment–Curvature Analysis of Lightly Reinforced Precast Walls
- Subdividing the cross-section into several rectangular concrete splices with equal depth.
- Defining a tentative (i.e., unbalanced) global reference strain (i.e., concrete strain) at the extreme concrete fibre and assuming a neutral axis.
- Computing the strains of other concrete splices and converting the global average concrete strain into the local steel strain using the defined tension stiffening model.
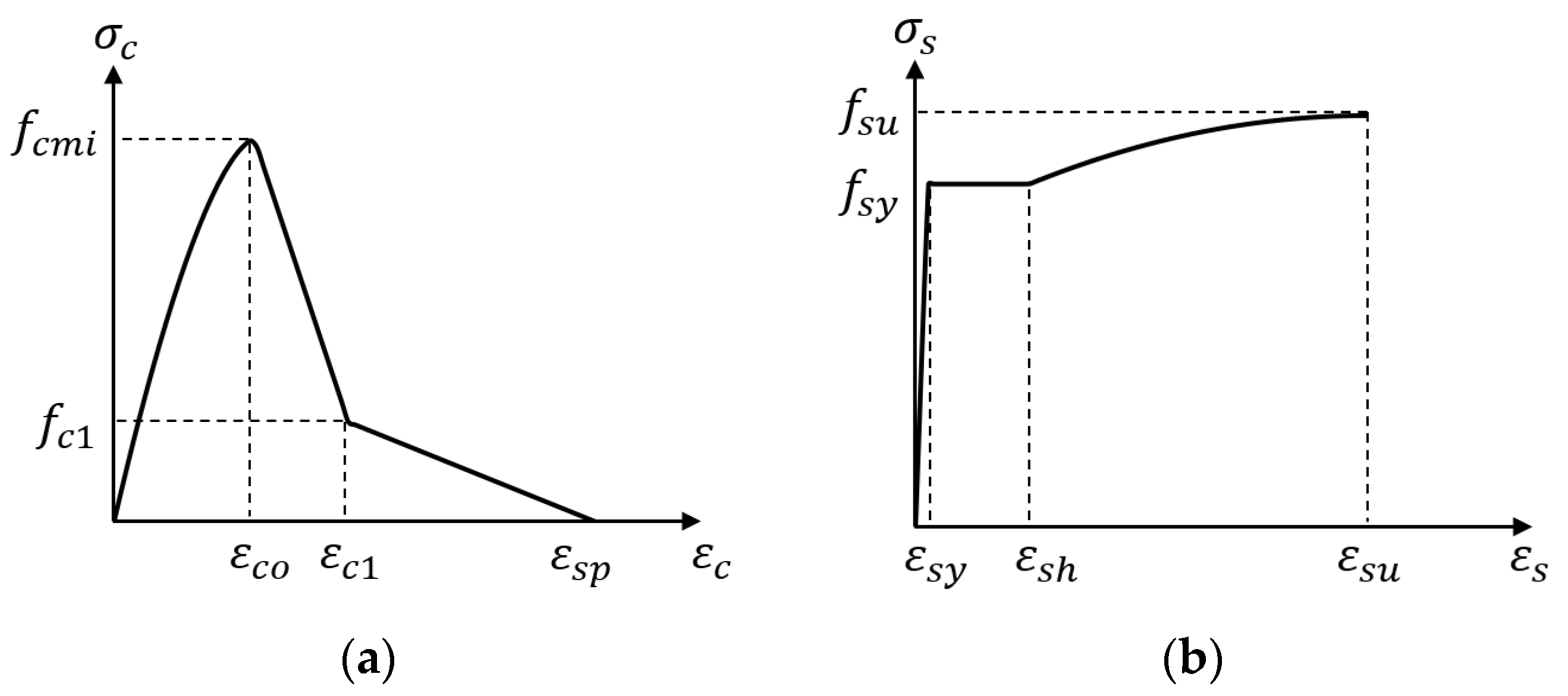
- Computing the stresses and forces of each concrete splice and reinforcing steel bar based on the selected material models. The tensile stresses (i.e., tensile strength) of concrete are typically not considered in sectional analysis.
- By iterative computation, finding out the accurate depth of the neutral axis and the balanced reference strain via force equilibrium of the internal forces (i.e., forces of each concrete splice and rebar) and the external axial load.
- Computing the moment and curvature results of the balanced cross-section. The moment can be calculated from the balanced forces, and the curvature can be estimated as the ratio of the balanced reference strain and the depth of the neutral axis.
- By repeating the above processes for gradually increasing reference strains and reaching each governing point (i.e., the yield and ultimate points discussed above), the moment and curvature relationship of a precast RC wall section can be plotted. The curved moment–curvature relationship can be converted to a bi-linear approximation based on the approach shown in Figure 10.
- If the cross-section is not rectangular, the most critical moment–curvature scenario should be identified by considering different concrete compression faces.
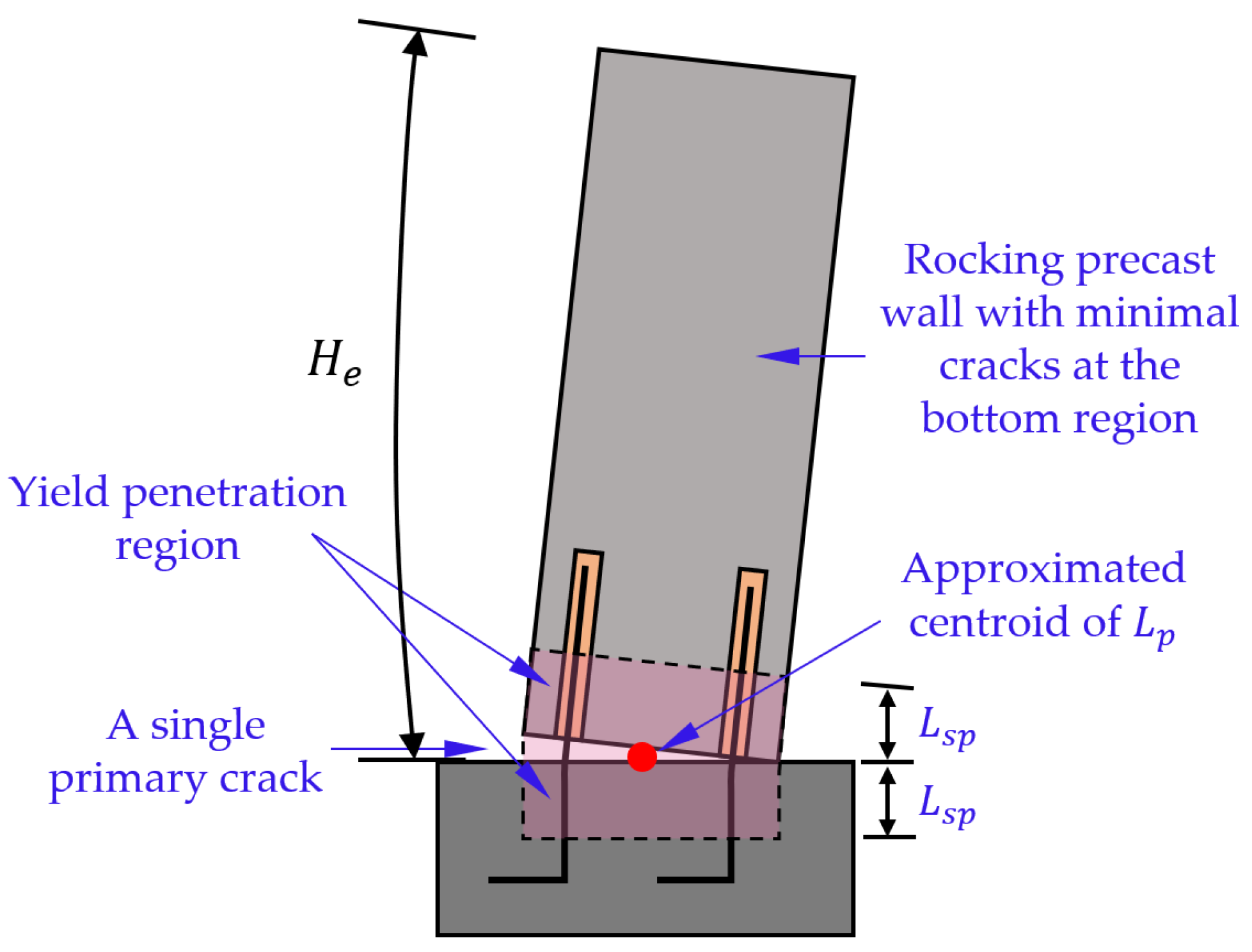
2.3. Plastic Hinge Analysis of Rectangular Lightly Reinforced Precast Walls
3. Simplified Push-Over Analysis of a Case Study Building Supported by Precast Walls
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Prediction of the Force–Displacement Capacity of a Limited-Ductile Reinforced Concrete Wall
References
- AS 1170.4-2007 (R2018); Structural Design Actions—Part 4: Earthquake Actions in Australia. Standards Australia: Sydney, NSW, Australia, 2018.
- Hoult, R.D.; Lumantarna, E.; Goldsworthy, H.M. Soil amplification in low-to-moderate seismic regions. Bull. Earthq. Eng. 2017, 15, 1945–1963. [Google Scholar] [CrossRef]
- Hu, Y.; Lam, N.; Khatiwada, P.; Menegon, S.J.; Looi, D.T.W. Site-specific response spectra: Guidelines for engineering practice. CivilEng 2021, 2, 712–735. [Google Scholar] [CrossRef]
- Khatiwada, P.; Hu, Y.; Lam, N.; Lumantarna, E. Program on generation of site-specific response spectra in Australia. In Proceedings of the Australian Earthquake Engineering Society 2021 Virtual Conference, Online, 25–26 November 2021. [Google Scholar]
- Hu, Y.; Lam, N.; Menegon, S.J.; Wilson, J. The Selection and Scaling of Ground Motion Accelerograms for Use in Stable Continental Regions. J. Earthq. Eng. 2022, 26, 6284–6303. [Google Scholar] [CrossRef]
- Khatiwada, P.; Hu, Y.; Lumantarna, E.; Menegon, S.J. Dynamic Modal Analyses of Building Structures Employing Site-Specific Response Spectra Versus Code Response Spectrum Models. CivilEng 2023, 4, 134–150. [Google Scholar] [CrossRef]
- Baker, J.W. Conditional Mean Spectrum: Tool for ground-motion selection. J. Struct. Eng. 2011, 137, 322–331. [Google Scholar] [CrossRef]
- Govindaraju, L.; Bhattacharya, S. Site-specific earthquake response study for hazard assessment in Kolkata city, India. Nat. Hazards 2012, 61, 943–965. [Google Scholar] [CrossRef]
- Tsang, H.H.; Wilson, J.L.; Lam, N.T.K.; Su, R.K.L. A design spectrum model for flexible soil sites in regions of low-to-moderate seismicity. Soil Dyn. Earthq. Eng. 2017, 92, 36–45. [Google Scholar] [CrossRef]
- Weng, X.; Lumantarna, E.; Hoult, R.D.; Lam, N.T.K. A survey focusing on precast reinforced concrete walls in Australia with some preliminary analyses. In Proceedings of the Australian Earthquake Engineering Society 2022 National Conference, Melbourne, Australia, 24–25 November 2022. [Google Scholar]
- Seifi, P.; Henry, R.S.; Ingham, J.M. Panel connection details in existing New Zealand precast concrete buildings. Bull. N. Z. Soc. Earthq. Eng. 2016, 49, 190–199. [Google Scholar] [CrossRef]
- Menegon, S.J.; Wilson, J.L.; Lam, N.T.K.; Gad, E.F. RC walls in Australia: Reconnaissance survey of industry and literature review of experimental testing. Aust. J. Struct. Eng. 2017, 18, 24–40. [Google Scholar] [CrossRef]
- Menegon, S.J.; Wilson, J.L.; Lam, N.T.; Gad, E.F. Experimental assessment of the ultimate performance and lateral drift behaviour of precast concrete building cores. Adv. Struct. Eng. 2020, 23, 2597–2613. [Google Scholar] [CrossRef]
- Seifi, P.; Henry, R.S.; Ingham, J.M. In-plane cyclic testing of precast concrete wall panels with grouted metal duct base connections. Eng. Struct. 2019, 184, 85–98. [Google Scholar] [CrossRef]
- Menegon, S.J.; Wilson, J.L.; Lam, N.T.K.; McBean, P. RC walls in Australia: Seismic design and detailing to AS 1170.4 and AS 3600. Aust. J. Struct. Eng. 2018, 19, 67–84. [Google Scholar] [CrossRef]
- AS 3600:2009; Incorporating Amendment Nos 1 and 2; Concrete Structures. Standards Australia: Sydney, NSW, Australia, 2013.
- Menegon, S.J.; Tsang, H.H.; Lumantarna, E.; Lam, N.T.K.; Wilson, J.L.; Gad, E.F. Framework for seismic vulnerability assessment of reinforced concrete buildings in Australia. Aust. J. Struct. Eng. 2019, 20, 143–158. [Google Scholar] [CrossRef]
- Blandon, C.A.; Arteta, C.A.; Bonett, R.L.; Carrillo, J.; Beyer, K.; Almeida, J.P. Response of thin lightly-reinforced concrete walls under cyclic loading. Eng. Struct. 2018, 176, 175–187. [Google Scholar] [CrossRef]
- Ile, N.; Reynouard, J.M. Behaviour of U-shaped walls subjected to uniaxial and biaxial cyclic lateral loading. J. Earthq. Eng. 2005, 9, 67–94. [Google Scholar] [CrossRef]
- AS 3600:2018; Incorporating Amendment Nos 1 and 2; Concrete Structures. Standards Australia: Sydney, NSW, Australia, 2021.
- Lu, Y.; Richard, S.H. Comparison of Minimum Vertical Reinforcement Requirements for Reinforced Concrete Walls. ACI Struct. J. 2018, 115, 673–687. [Google Scholar] [CrossRef]
- Sritharan, S.; Beyer, K.; Henry, R.S.; Chai, Y.H.; Kowalsky, M.; Bull, D. Understanding poor seismic performance of concrete walls and design implications. Earthq. Spectra 2014, 30, 307–334. [Google Scholar] [CrossRef]
- Wilson, J.L.; Robinson, A.J.; Balendra, T. Performance of precast concrete load-bearing panel structures in regions of low to moderate seismicity. Eng. Struct. 2008, 30, 1831–1841. [Google Scholar] [CrossRef]
- Priestley, M.J.N.; Calvi, G.M.; Kowalsky, M.J. Displacement-Based Seismic Design of Structures; IUSS PRESS: Pavia, Italy, 2007. [Google Scholar]
- Sullivan, T.J. Motives for and impediments facing direct displacement-based seismic design. In Proceedings of the Tenth Pacific Conference on Earthquake Engineering Building an Earthquake-Resilient Pacific, Sydney, Australia, 6–8 November 2015. [Google Scholar]
- Menegon, S.J.; Tsang, H.-H.; Wilson, J.L.; Lam, N.T.K. RC walls in Australia: Displacement-based seismic design in accordance with AS 1170.4 and AS 3600. Aust. J. Struct. Eng. 2021, 22, 205–221. [Google Scholar] [CrossRef]
- Zameeruddin, M.; Sangle, K.K. Review on recent developments in the performance-based seismic design of reinforced concrete structures. Structures 2016, 6, 119–133. [Google Scholar] [CrossRef]
- Wibowo, A.; Wilson, J.L.; Lam, N.T.K.; Gad, E.F. Collapse modelling analysis of a precast soft storey building in Australia. Eng. Struct. 2010, 32, 1925–1936. [Google Scholar] [CrossRef]
- Wibowo, A.; Wilson, J.L.; Lam, N.T.K.; Gad, E.F. Seismic performance of lightly reinforced structural walls for design purposes. Mag. Concr. Res. 2013, 65, 809–828. [Google Scholar] [CrossRef]
- Hoult, R.; Goldsworthy, H.; Lumantarna, E. Fragility functions for RC shear wall buildings in Australia. Earthq. Spectra 2019, 35, 333–360. [Google Scholar] [CrossRef]
- Wilson, J.; Lam, N. Earthquake Design of buildings in Australia using velocity and displacement principles. Aust. J. Struct. Eng. 2006, 6, 103–118. [Google Scholar] [CrossRef]
- Wilson, J.L.; Wibowo, A.; Lam, N.T.K.; Gad, E.F. Drift behaviour of lightly reinforced concrete columns and structural walls for seismic design applications. Aust. J. Struct. Eng. 2015, 16, 62–74. [Google Scholar] [CrossRef]
- Raza, S.; Tsang, H.-H.; Menegon, S.J.; Wilson, J.L. Seismic performance assessment of reinforced concrete columns in regions of low to moderate seismicity. In Resilient Structures and Infrastructure; Springer: Singapore, 2019; pp. 269–286. [Google Scholar] [CrossRef]
- Fajfar, P. Capacity spectrum method based on inelastic demand spectra. Earthq. Eng. Struct. Dyn. 1999, 28, 979–993. [Google Scholar] [CrossRef]
- Lam, N.; Wilson, J.; Lumantarna, E. Force-deformation behaviour modelling of cracked reinforced concrete by EXCEL spreadsheets. Comput. Concr. 2011, 8, 43–57. [Google Scholar] [CrossRef]
- Fajfar, P. A Nonlinear analysis method for performance-based seismic design. Earthq. Spectra 2000, 16, 573–592. [Google Scholar] [CrossRef]
- Hines, E.M.; Restrepo, J.I.; Seible, F. Force-displacement characterization of well-confined bridge piers. ACI Struct. J. 2004, 101, 537–548. [Google Scholar] [CrossRef]
- Henry, R.S. Assessment of minimum vertical reinforcement limits for RC walls. Bull. N. Z. Soc. Earthq. Eng. 2013, 46, 88–96. [Google Scholar] [CrossRef]
- Menegon, S.J. Displacement Behaviour of Reinforced Concrete Wall in Regions of Lower Seismicity. Doctoral Dissertation, Swinburne University of Technology, Melbourne, VIC, Australia, 2018. [Google Scholar]
- Menegon, S.J.; Wilson, J.L.; Lam, N.T.K.; Gad, E.F. Development of a user-friendly and transparent non-linear analysis program for RC walls. Comput. Concr. 2020, 25, 327–341. [Google Scholar] [CrossRef]
- Menegon, S.J. WHAM: A User-Friendly and Transparent Non-Linear Analysis Program for RC Walls and Building Cores. Available online: https://downloads.menegon.com.au/1/20190901/ (accessed on 29 December 2022).
- Spacone, E.; Filippou, F.C.; Taucer, F.F. Fibre beam–column model for non-linear analysis of R/C frames: Part I. formulation. Earthq. Eng. Struct. Dyn. 1996, 25, 711–725. [Google Scholar] [CrossRef]
- Monti, G.; Spacone, E. Reinforced concrete fiber beam element with bond-slip. J. Struct. Eng. 2000, 126, 654–661. [Google Scholar] [CrossRef]
- Menegon, S.J.; Wilson, J.L.; Lam, N.T.K.; Gad, E.F. Experimental testing of innovative panel-to-panel connections for precast concrete building cores. Eng. Struct. 2020, 207, 110239. [Google Scholar] [CrossRef]
- Raynor, D.J.; Lehman, D.E.; Stanton, J.F. Bond-slip response of reinforcing bars grouted in ducts. ACI Struct. J. 2002, 99, 568–576. [Google Scholar] [CrossRef]
- Steuck, K.P.; Eberhard, M.O.; Stanton, J.F. Anchorage of large-diameter reinforcing bars in ducts. ACI Struct. J. 2009, 106, 506. [Google Scholar] [CrossRef]
- Elsayed, M.; Nehdi, M.L. Experimental and analytical study on grouted duct connections in precast concrete construction. Mater. Struct. 2017, 50, 198. [Google Scholar] [CrossRef]
- Elsayed, M.; Ghrib, F.; Nehdi, M.L. Experimental and analytical study on precast concrete dowel connections under quasi-static loading. Constr. Build. Mater. 2018, 168, 692–704. [Google Scholar] [CrossRef]
- Provost-Smith, D.J.; Elsayed, M.; Nehdi, M.L. Investigation of grouted dowel connections for precast wall construction. ACI Struct. J. 2019, 116, 41–50. [Google Scholar] [CrossRef]
- AS 3600:2018 Sup1:2022; Concrete Structures—Commentary (Supplement 1 to AS 3600:2018). Standards Australia: Sydney, NSW, Australia, 2022.
- Menegon, S.; Tsang, H.; Wilson, J.; Lam, N. Overstrength and ductility of limited ductile RC walls: From the design engineers perspective. In Proceedings of the Tenth Pacific Conference on Earthquake Engineering, Sydney, Australia, 6–8 November 2015. [Google Scholar]
- Menegon, S.J.; Tsang, H.-H.; Wilson, J.L.; Lam, N.T.K. Statistical analysis of material properties and recommended values for the assessment of RC structures in Australia. Aust. J. Struct. Eng. 2021, 22, 191–204. [Google Scholar] [CrossRef]
- Karthik Madhu, M.; Mander John, B. Stress-block parameters for unconfined and confined concrete based on a unified stress-strain model. J. Struct. Eng. 2011, 137, 270–273. [Google Scholar] [CrossRef]
- Mander, J.B.; Priestley, M.J.N.; Park, R. Theoretical stress-strain model for confined concrete. J. Struct. Eng. 1988, 114, 1804–1826. [Google Scholar] [CrossRef]
- Menegon, S.J.; Wilson, J.L.; Lam, N.T.; Gad, E.F. Tension stiffening model for lightly confined reinforced concrete elements. Mag. Concr. Res. 2021, 73, 366–378. [Google Scholar] [CrossRef]
- Menegon, S.J.; Wilson, J.L.; Lam, N.T.K.; Gad, E.F. Experimental testing of nonductile reinforced concrete wall boundary elements. ACI Struct. J. 2019, 116, 213–225. [Google Scholar] [CrossRef]
- Hoult, R.; Goldsworthy, H.; Lumantarna, E. Plastic hinge length for lightly reinforced rectangular concrete walls. J. Earthq. Eng. 2018, 22, 1447–1478. [Google Scholar] [CrossRef]
- Hoult, R.D.; Goldsworthy, H.M.; Lumantarna, E. Plastic hinge length for lightly reinforced C-shaped concrete walls. J. Earthq. Eng. 2018, 24, 1083–1114. [Google Scholar] [CrossRef]
- Hoult, R. Universal plastic hinge length for reinforced concrete walls. ACI Struct. J. 2022, 119, 75–83. [Google Scholar] [CrossRef]
- Kazaz, İ. Analytical study on plastic hinge length of structural walls. J. Struct. Eng. 2013, 139, 1938–1950. [Google Scholar] [CrossRef]
- Altheeb, A.H.; Albidah, A.S.; Lam, N.T.K.; Wilson, J.L. Analytical modelling of strain penetration deformation in reinforced concrete members. In Proceedings of the Tenth Pacific Conference on Earthquake Engineering Building an Earthquake-Resilient Pacific, Sydney, Australia, 6–8 November 2015. [Google Scholar]
- Altheeb, A.H. Seismic Drift Capacity of Lightly Reinforced Concrete Shear Walls. Doctoral Dissertation, University of Melbourne, Melbourne, VIC, Australia, 2016. [Google Scholar]
- Morris, G.J.; Bull, D.K.; Bradley, B.A. In situ conditions affecting the ductility capacity of lightly reinforced concrete wall structures in the Canterbury earthquake sequence. Bull. N. Z. Soc. Earthq. Eng. 2015, 48, 190–203. [Google Scholar] [CrossRef]
- Ministry of Business, Innovation and Employment; Earthquake Commission; New Zealand Society for Earthquake Engineering; Structural Engineering Society; New Zealand Geotechnical Society. The Seismic Assessment of Existing Buildings Part C5 Concrete Buildings—Technical Proposal to Revise the Engineering Assessment Guidelines; New Zealand. 2018. Available online: http://www.eq-assess.org.nz/ (accessed on 29 December 2022).
- Paulay, T.; Priestley, M.J.N. Seismic Design of Reinforced Concrete and Masonry Buildings; John Wiley & Sons, Inc.: New York, NY, USA, 1992. [Google Scholar]
- Seifi, P.; Henry, R.S.; Ingham, J.M. Precast Concrete Wall Tests—Grouted Metal Duct Connections; The Natural Hazards Engineering Research Infrastructure (NHERI): Alexandria, VA, USA, 2021. [CrossRef]
- Xue, W.; Huang, Q.; Niu, P. Reversed cyclic tests on precast concrete shear walls with grouted corrugated metallic duct connections. Eng. Struct. 2022, 256, 113948. [Google Scholar] [CrossRef]
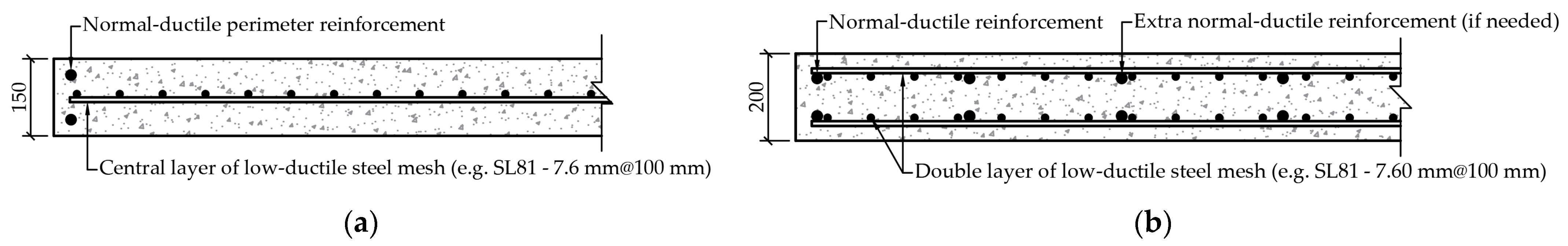


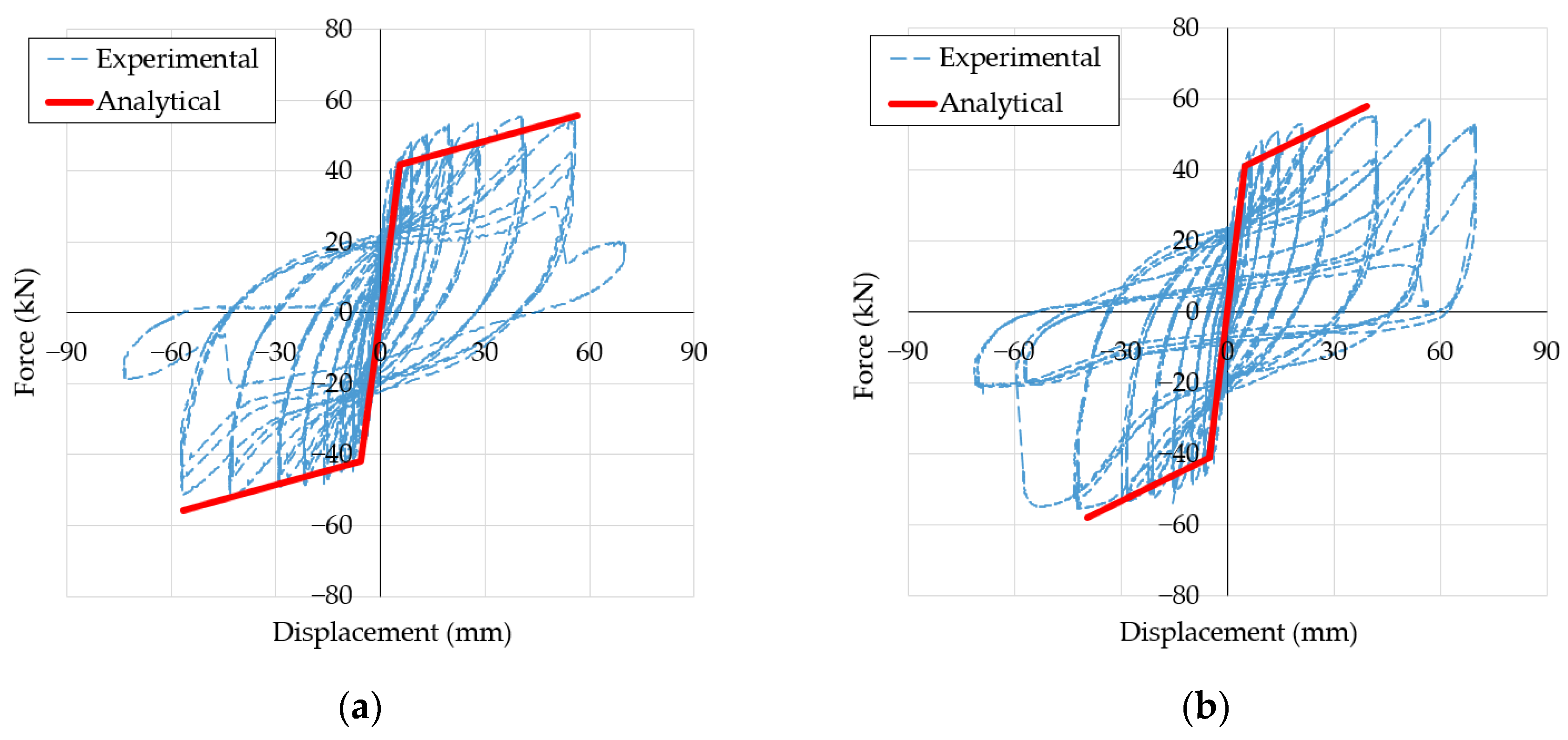

| Specimens | Dimension (Length × Height × Thickness) | Connection Dowel 1 | Wall Vertical Reinforcement 1 |
|---|---|---|---|
| SW1 | 1000 × 3000 × 150 | 16@400 () (100 mm cover to dowel centre) | Single layer 12@225 () (50 mm cover to rebar centre) |
| SW4 | 1000 × 3000 × 200 | 16@400 () (100 mm cover to dowel centre) | Double layer 12@225 () (50 mm cover to rebar centre) |
| Specimen No. | Concrete Compressive Strength | Connection Reinforcement | ||
|---|---|---|---|---|
| Yield Strength | Ultimate Strength | Ultimate Strain | ||
| SW1 | 46 MPa | 473 MPa | 632 MPa | 10% |
| SW4 | 56 MPa | 473 MPa | 632 MPa | 10% |
| First Yield | Nominal Yield | Ultimate Limit State | |
|---|---|---|---|
| Concrete Compressive Strain () | 0.003 | 0.004 | |
| Reinforcement Tensile Strain () | 0.015 | 0.6 |
| Nominal Yield | Ultimate | |||
|---|---|---|---|---|
| Curvature (1/km) | Moment (kNm) | Curvature (1/km) | Moment (kNm) | |
| SW1 | 1.9 | 125.4 | 52.7 | 171.4 |
| SW4 | 1.2 | 123.6 | 35.3 | 178.2 |
| Storey | Total Height | Height (Ground Floor) | Height (Other Floors) | Total Building Mass | Axial Load Ratio |
|---|---|---|---|---|---|
| 6 | 19.3 m | 3.8 m | 3.1 m | 3700 tons | 5% |
| First Yield | Nominal Yield | Ultimate Limit State | |
|---|---|---|---|
| Reinforcement Tensile Strain () | 0.00275 | 0.015 |
| Nominal Yield | Ultimate | |
|---|---|---|
| Curvature (1/km) | 0.58 | 4.62 |
| Moment (kNm) | 10,522 | 13,272 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Weng, X.; Hoult, R.D.; Lumantarna, E. Site-Specific Seismic Analysis of Buildings Supported by Lightly Reinforced Precast Concrete Walls. CivilEng 2023, 4, 270-291. https://doi.org/10.3390/civileng4010016
Weng X, Hoult RD, Lumantarna E. Site-Specific Seismic Analysis of Buildings Supported by Lightly Reinforced Precast Concrete Walls. CivilEng. 2023; 4(1):270-291. https://doi.org/10.3390/civileng4010016
Chicago/Turabian StyleWeng, Xiangzhe, Ryan D. Hoult, and Elisa Lumantarna. 2023. "Site-Specific Seismic Analysis of Buildings Supported by Lightly Reinforced Precast Concrete Walls" CivilEng 4, no. 1: 270-291. https://doi.org/10.3390/civileng4010016
APA StyleWeng, X., Hoult, R. D., & Lumantarna, E. (2023). Site-Specific Seismic Analysis of Buildings Supported by Lightly Reinforced Precast Concrete Walls. CivilEng, 4(1), 270-291. https://doi.org/10.3390/civileng4010016








