Arbitrary-Order Bernstein–Bézier Functions for DGFEM Transport on 3D Polygonal Grids
Abstract
:1. Introduction
2. The DGFEM Transport Equation
3. 3D Extruded Polygonal Basis Functions
3.1. Brief Overview of 2D Polygonal Functions
3.2. Extrusion to Polygonal Prisms
4. Numerical Results
4.1. Basis Function Verification
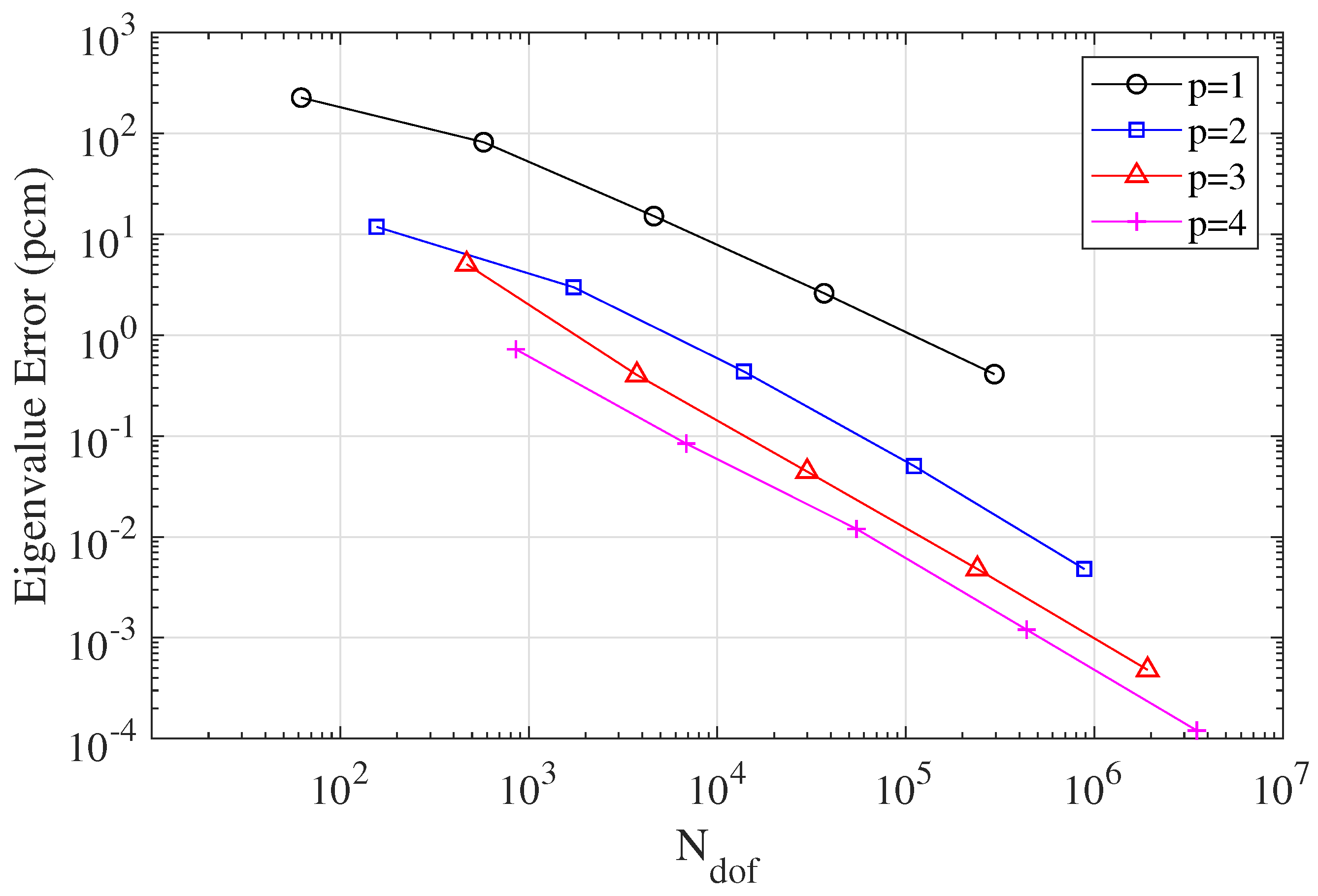
4.2. Multigroup Pincell Problem
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lewis, E.; Miller, W. Computational Methods of Neutron Transport; John Wiley and Sons, Inc.: New York, NY, USA, 1984. [Google Scholar]
- Reed, W.; Hill, T. Triangular Mesh Methods for the Neutron Transport Equation; Los Alamos Report LA-UR-73-479; Los Alamos Scientific Lab.: Los Alamos, NM, USA, 1973.
- Wang, Y.; Ragusa, J.C. On the convergence of DGFEM applied to the discrete ordinates transport equation for structured and unstructured triangular meshes. Nucl. Sci. Eng. 2009, 163, 56–72. [Google Scholar] [CrossRef]
- Hackemack, M.W. Higher-Order DGFEM Transport Calculations on Polytope Meshes for Massively-Parallel Architectures. Ph.D. Thesis, Texas A&M University, College Station, TX, USA, 2016. [Google Scholar]
- Stone, H.G.; Adams, M.L. New spatial discretization methods for transport on unstructured grids. In Proceedings of the ANS Topical Meeting Mathematics and Computation, Supercomputing, Reactor Physics and Biological Applications, Avignon, France, 12–15 September 2005. [Google Scholar]
- Bailey, T.S. The Piecewise Linear Discontinuous Finite Element Method Applied to the RZ and XYZ Transport Equations. Ph.D. Thesis, Texas A&M University, College Station, TX, USA, 2008. [Google Scholar]
- Hackemack, M.; Ragusa, J. Quadratic Serendipity Discontinuous Finite Element Discretization for SN Transport on Arbitrary Polygonal Grids. J. Comput. Phys. 2018, 374, 188–212. [Google Scholar] [CrossRef]
- Hackemack, M. Quadratic Serendipity Functions for DGFEM Transport on 2D Polygonal and 3D Extruded Polygonal Grids. In Proceedings of the PHYSOR 2018: Reactor Physics Paving the Way towards More Efficient Systems, Cancun, Mexico, 22–26 April 2018. [Google Scholar]
- Floater, M.; Lai, M.J. Polygonal spline spaces and the numerical solution of the Poisson equation. SIAM J. Numer. Anal. 2016, 54, 797–824. [Google Scholar] [CrossRef] [Green Version]
- Hackemack, M. Arbitrary-Order Discontinuous Galerkin Discretization for SN Transport Using Polygonal Bernstein-Bézier Functions. In Proceedings of the M&C 2019—International Conference on Mathematics & Computational Methods Applied to Nuclear Science & Engineering, Portland, OR, USA, 25–29 August 2019. [Google Scholar]
- Hackemack, M. Discontinuous Galerkin Solutions for Elliptic Problems on Polygonal Grids using Arbitrary-Order Bernstein-Bézier Functions. J. Comput. Phys. 2021, 437, 110293. [Google Scholar] [CrossRef]
- Adams, M.L. Discontinuous finite element transport solutions in thick diffusive problems. Nucl. Sci. Eng. 2001, 137, 298–333. [Google Scholar] [CrossRef]
- Talischi, C.; Paulino, G.H.; Pereira, A.; Menezes, I. PolyMesher: A general-purpose mesh generator for polygonal elements written in Matlab. Struct. Multidiscip. Optim. 2012, 45, 309–328. [Google Scholar] [CrossRef]
- Dahl, J. PARTISN results for the OECD/NEA 3-d extension C5G7 MOX benchmark. Prog. Nucl. Energy 2006, 48, 401–409. [Google Scholar] [CrossRef]
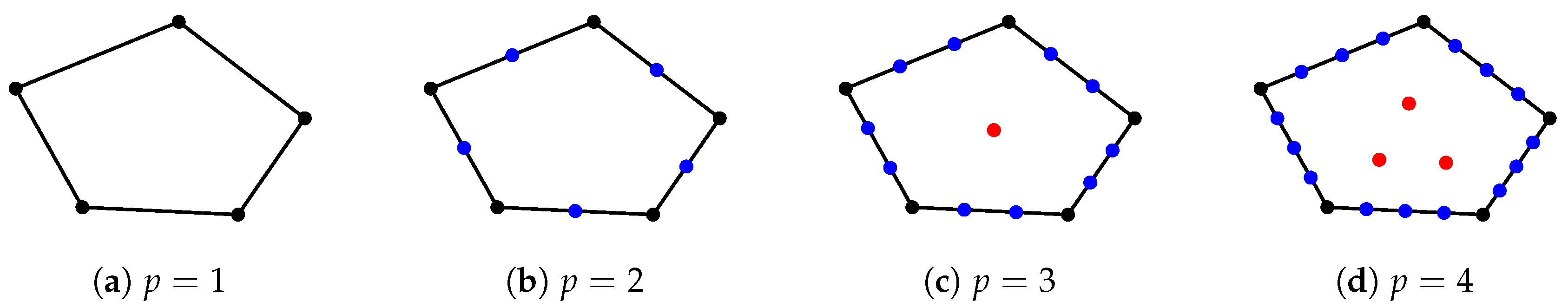
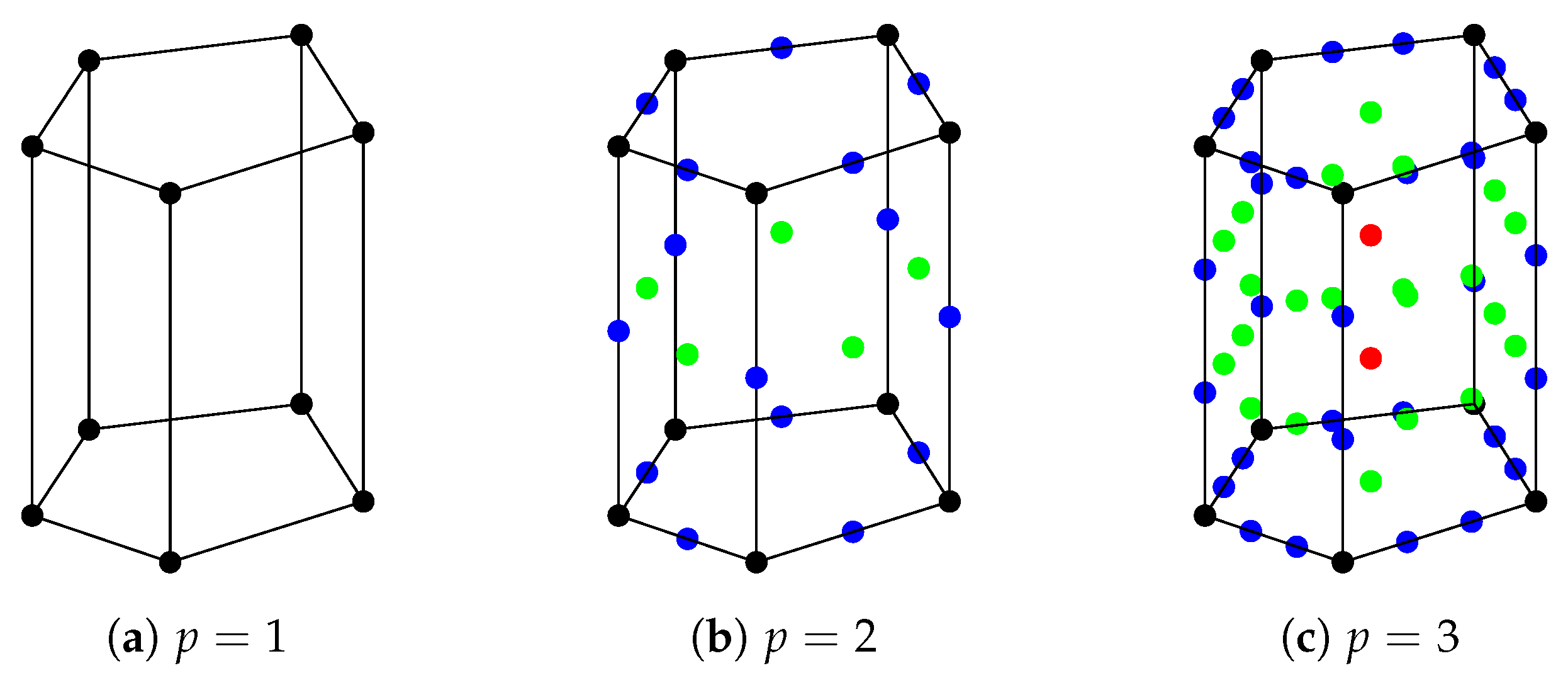
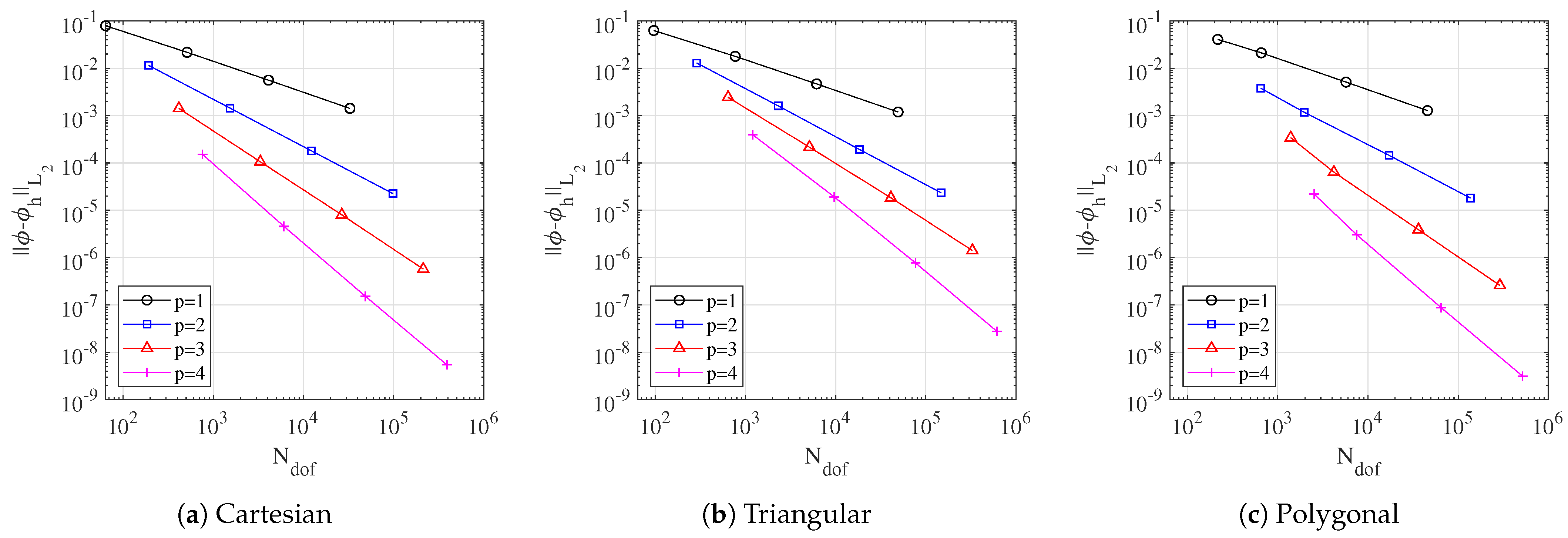
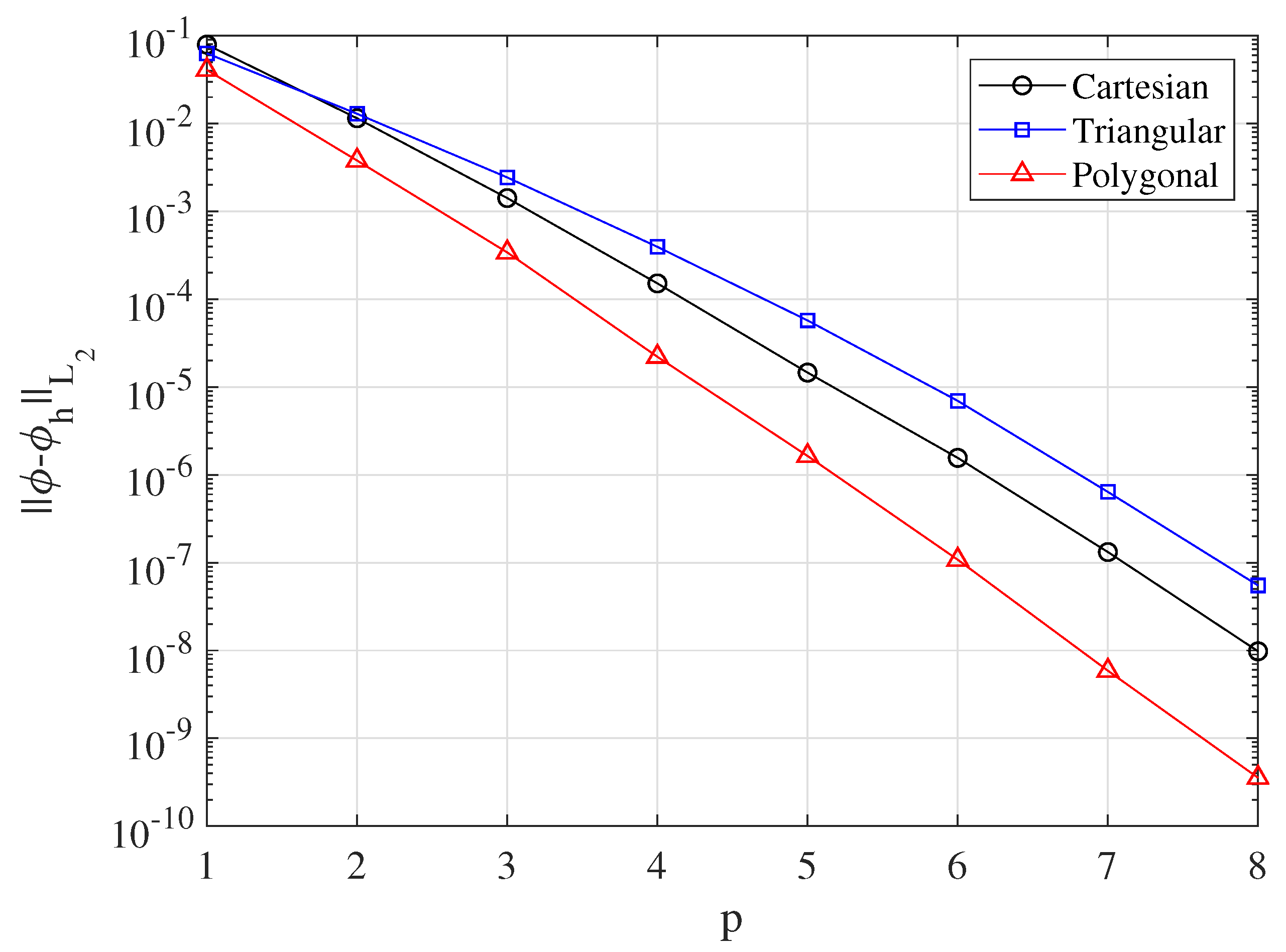
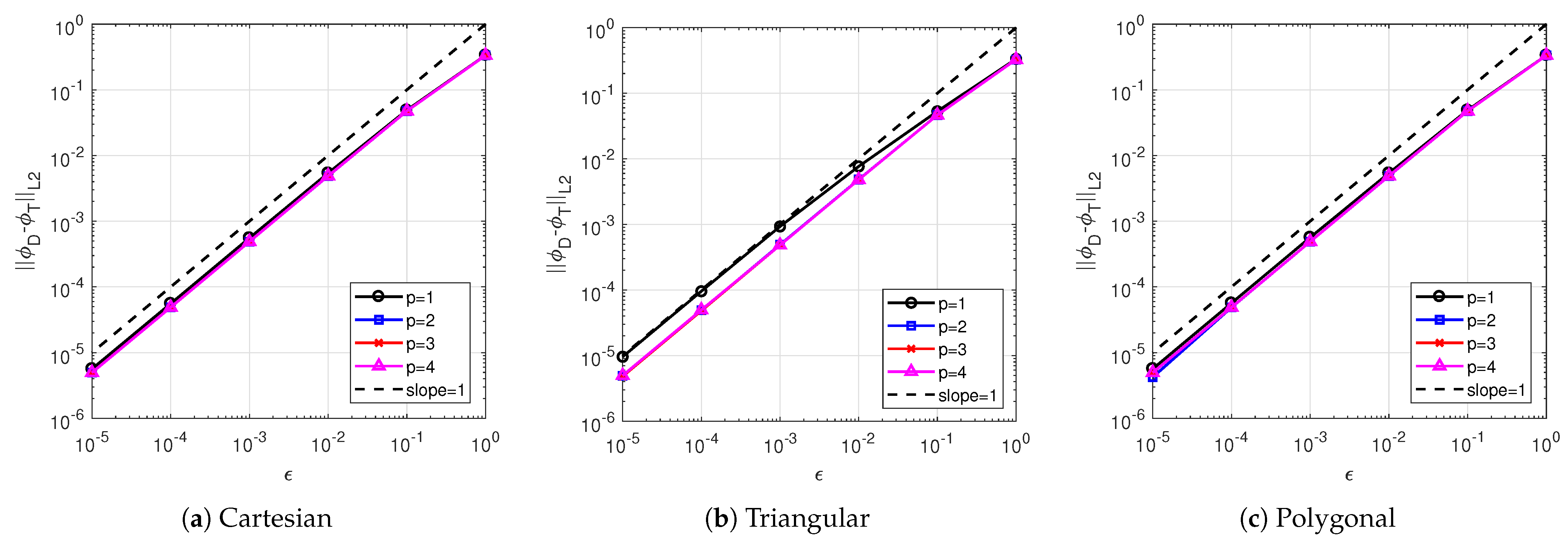
| p | Cartesian | Triangular | Polygonal |
|---|---|---|---|
| 1 | 8.97 × 10 | 8.10 × 10 | 8.81 × 10 |
| 2 | 3.86 × 10 | 2.66 × 10 | 5.36 × 10 |
| 3 | 7.52 × 10 | 5.71 × 10 | 9.24 × 10 |
| 4 | 1.33 × 10 | 9.89 × 10 | 5.50 × 10 |
| 5 | 5.74 × 10 | 4.97 × 10 | 8.88 × 10 |
| 6 | 1.04 × 10 | 8.80 × 10 | 2.19 × 10 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hackemack, M. Arbitrary-Order Bernstein–Bézier Functions for DGFEM Transport on 3D Polygonal Grids. J. Nucl. Eng. 2021, 2, 239-245. https://doi.org/10.3390/jne2030022
Hackemack M. Arbitrary-Order Bernstein–Bézier Functions for DGFEM Transport on 3D Polygonal Grids. Journal of Nuclear Engineering. 2021; 2(3):239-245. https://doi.org/10.3390/jne2030022
Chicago/Turabian StyleHackemack, Michael. 2021. "Arbitrary-Order Bernstein–Bézier Functions for DGFEM Transport on 3D Polygonal Grids" Journal of Nuclear Engineering 2, no. 3: 239-245. https://doi.org/10.3390/jne2030022
APA StyleHackemack, M. (2021). Arbitrary-Order Bernstein–Bézier Functions for DGFEM Transport on 3D Polygonal Grids. Journal of Nuclear Engineering, 2(3), 239-245. https://doi.org/10.3390/jne2030022






