Virgin Passive Colon Biomechanics and a Literature Review of Active Contraction Constitutive Models
Abstract
:1. Introduction
2. Materials and Methods
2.1. Preparation of the Equibiaxial Tensile Test Specimens
2.2. Test Protocol
2.3. Virgin Passive Anisotropic Strain Energy Function Description
2.4. Numerical Simulations
3. Results
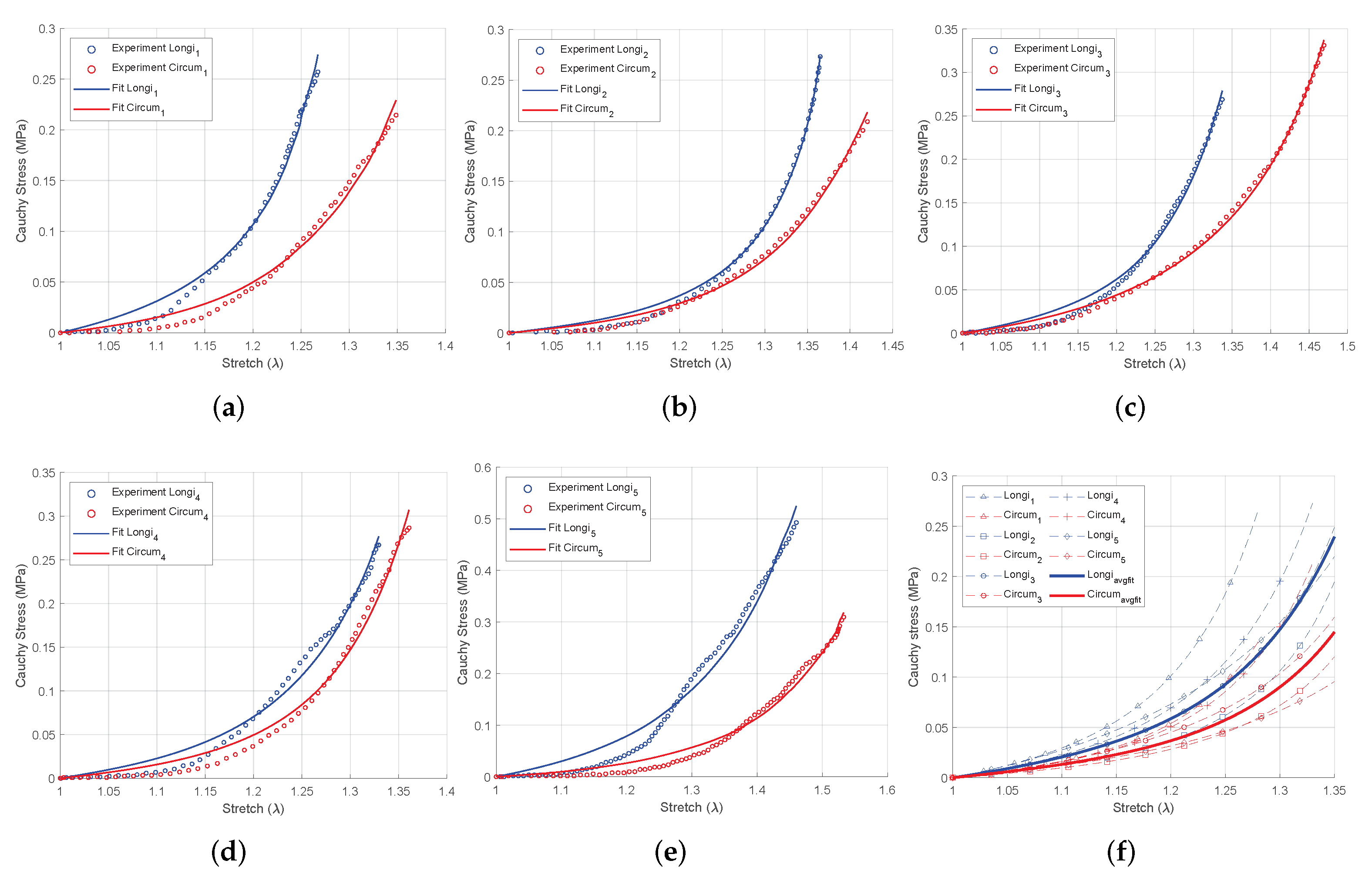
3.1. Equibiaxial Tensile Experiment
3.2. Intestinal Anisotropy
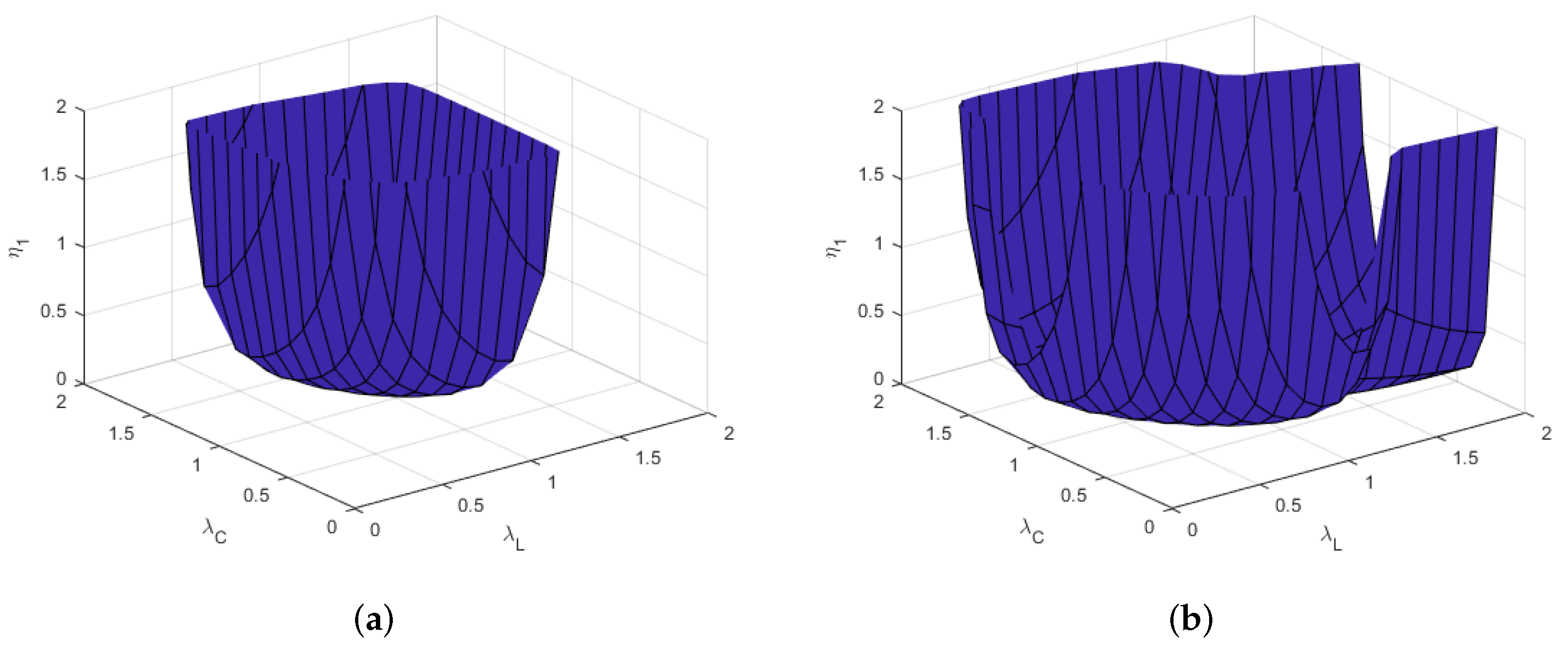
3.3. Parameter Stability
3.4. Numerical Simulations
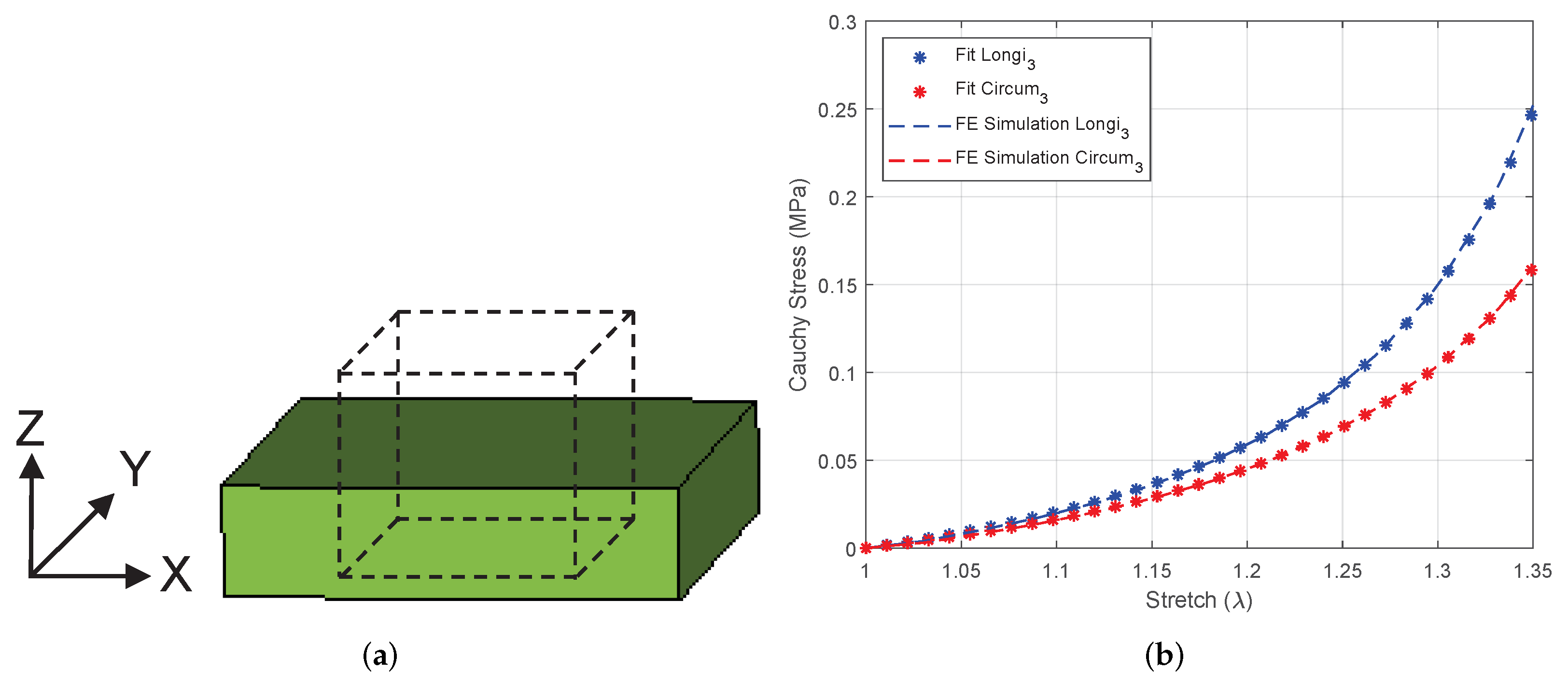
3.4.1. Equibiaxial Tension of a Linear Brick Element
3.4.2. Equibiaxial Tension of an Intestine Specimen
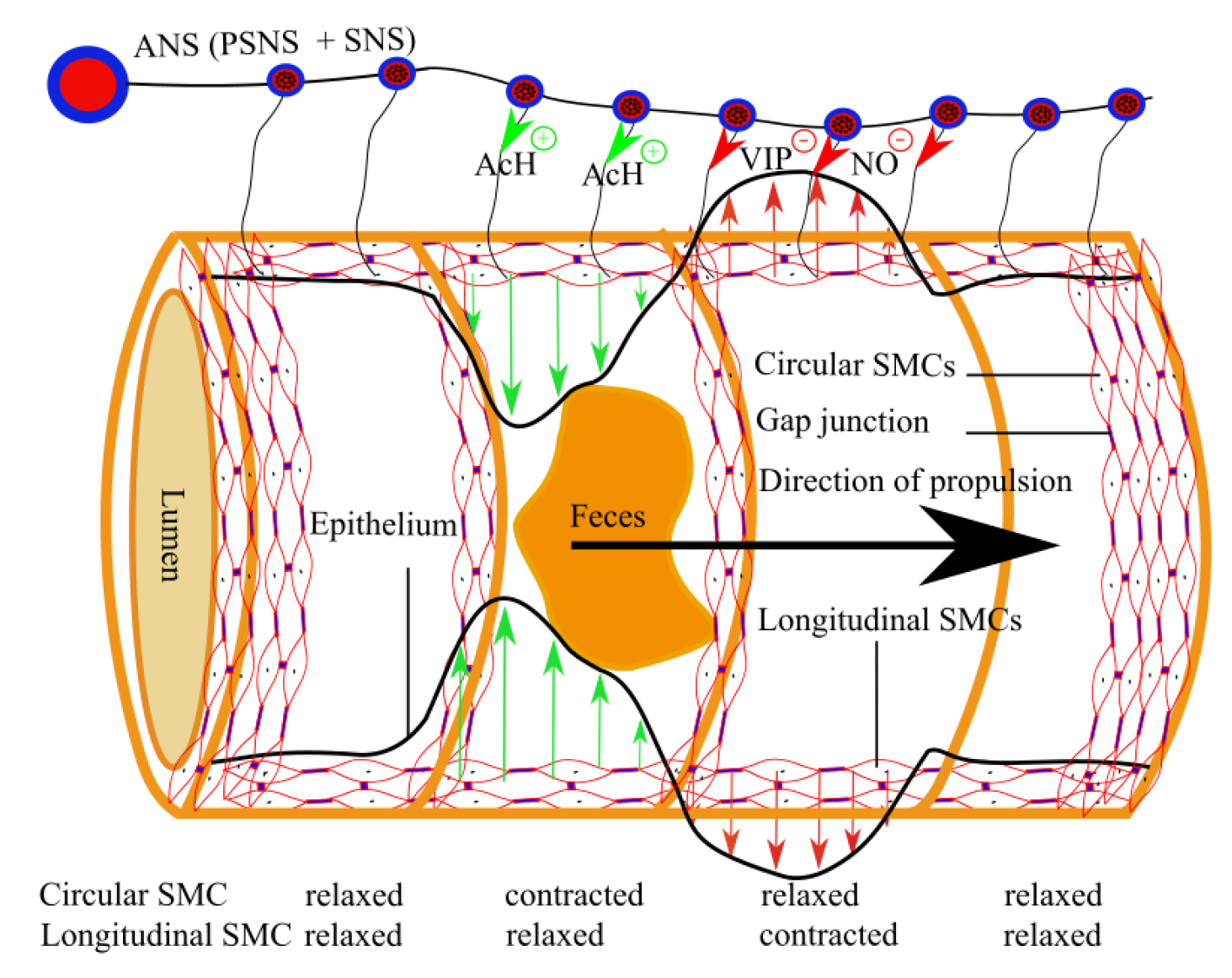
4. Physiology of Intestinal Peristalsis
5. Constitutive Modeling of Large Intestinal SMC Contraction
6. Intestinal Active Viscoelasticity
7. Discussion
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bhattarai, A.; Wojciech, K.; Tran, T.N. A literature review on large intestinal hyperelastic constitutive modeling. Clin. Biomech. 2021, 88, 105445. [Google Scholar] [CrossRef] [PubMed]
- Kühn, R.; Löhler, J.; Rennick, D.; Rajewsky, K.; Müller, W. Interleukin-10-deficient mice develop chronic enterocolitis. Cell 2009, 75, 263–274. [Google Scholar] [CrossRef]
- Kontoyiannis, D.; M Pasparakis, M.; Pizarro, T.T.; Cominelli, F.; Kollias, G. Impaired on/off regulation of TNF biosynthesis in mice lacking TNF AU-rich elements: Implications for joint and gut-associated immunopathologies. Immunity 1999, 10, 387–398. [Google Scholar] [CrossRef] [Green Version]
- Andoh, A.; Zhang, Z.; Inatomi, O.; Fujino, S.; Deguchi, Y.; Araki, Y.; Tsujikawa, T.; Kitoh, K.; Kim-Mitsuyama, S.; Takayanagi, A.; et al. Interleukin-22, a member of the IL-10 subfamily, induces inflammatory responses in colonic subepithelial myofibroblasts. Gastroenterology 2005, 129, 969–984. [Google Scholar] [CrossRef]
- Kobayashi, T.; Okamoto, S.; Hisamatsu, T.; Kamada, N.; Chinen, H.; Saito, R.; Kitazume, M.T.; Nakazawa, A.; Sugita, A.; Koganei, K.; et al. IL-23 differentially regulates the Th1/Th17 balance in ulcerative colitis and Crohn’s disease. Gut 2008, 57, 1682–1689. [Google Scholar] [CrossRef] [Green Version]
- Sugimoto, K.; Ogawa, A.; Mizoguchi, E.; Shimomura, Y.; Andoh, A.; Bhan, A.K.; Blumberg, R.S.; Xavier, R.J.; Mizoguchi, A. IL-22 ameliorates intestinal inflammation in a mouse model of ulcerative colitis. J. Clin. Investig. 2008, 118, 534–544. [Google Scholar] [CrossRef] [Green Version]
- Dinarello, C.A. Interleukin-1 in the pathogenesis and treatment of inflammatory diseases. Blood 2011, 117, 3720–3732. [Google Scholar] [CrossRef] [Green Version]
- Kamada, N.; Núñez, G. Regulation of the immune system by the resident intestinal bacteria. Gastroenterology 2014, 146, 1477–1488. [Google Scholar] [CrossRef] [Green Version]
- Seo, S.U.; Kuffa, P.; Kitamoto, S.; Nagao-Kitamoto, H.; Rousseau, J.; Kim, Y.G.; Núñez, G.; Kamada, N. Intestinal macrophages arising from CCR2(+) monocytes control pathogen infection by activating innate lymphoid cells. Nat. Commun. 2015, 6, 8010. [Google Scholar] [CrossRef]
- Watters, D.A.; Smith, A.N.; Eastwood, M.A.; Anderson, K.C.; Elton, R.A.; Mugerwa, J.W. Mechanical properties of the colon: Comparison of the features of the African and European colon in vitro. Gut 1985, 26, 384–392. [Google Scholar] [CrossRef]
- Egorov, V.I.; Schastlivtsev, I.V.; Prut, E.V.; Baranov, A.O.; Turusov, R.A. Mechanical properties of the human gastrointestinal tract. J. Biomech. 2002, 35, 1417–1425. [Google Scholar] [CrossRef]
- Qiao, Y.; Pan, E.; Chakravarthula, S.S.; Han, F.; Liang, J.; Gudlavalleti, S. Measurement of mechanical properties of rectal wall. J. Mater. Sci. Mater. Med. 2005, 16, 183–188. [Google Scholar] [CrossRef] [PubMed]
- Ciarletta, P.; Dario, P.; Tendick, F.; Micera, F. Hyperelastic model of anisotropic fiber reinforcements within intestinal walls for applications in medical robotics. Int. J. Robot. Res. 2009, 28, 1279–1288. [Google Scholar] [CrossRef]
- Bellini, C.; Glass, P.; Sitti, M.; Martino, E.S.D. Biaxial mechanical modeling of the small intestine. J. Mech. Behav. Biomed. Mater. 2011, 4, 1727–1740. [Google Scholar] [CrossRef] [PubMed]
- Howes, M.K.; Hardy, W.N. Dynamic Material Properties of the Post-Mortem Human Colon. In Proceedings of the 2013 International IRCOBI Conference on the Biomechanics of Injury, Gothenburg, Sweden, 11–13 September 2013; pp. 124–132. [Google Scholar]
- Sokolis, D.P.; Sassani, S.G. Microstructure-based constitutive modeling for the large intestine validated by histological observations. J. Mech. Behav. Biomed. Mater. 2013, 21, 149–166. [Google Scholar] [CrossRef] [PubMed]
- Carniel, E.L.; Gramigna, V.; Fontanella, C.G.; Stefanini, C.; Natali, A.N. Constitutive formulations for the mechanical investigation of colonic tissues. J. Biomed. Mater. Res. A 2014, 102, 1243–1254. [Google Scholar] [CrossRef]
- Christensen, M.B.; Oberg, K.; Wolchok, J.C. Tensile properties of the rectal and sigmoid colon: A comparative analysis of human and porcine tissue. SpringerPlus 2015, 4, 142. [Google Scholar] [CrossRef] [Green Version]
- Gong, X.; Xu, X.; Lin, S.; Cheng, Y.; Tong, J.; Li, Y. Alterations in biomechanical properties and microstructure of colon wall in early-stage experimental colitis. Exp. Ther. Med. 2017, 14, 995–1000. [Google Scholar] [CrossRef]
- Patel, B.; Chen, H.; Ahuja, A.; Krieger, J.F.; Noblet, J.; Chambers, S.; Kassab, G.S. Constitutive modeling of the passive inflation-extension behavior of the swine colon. J. Mech. Behav. Biomed. Mater. 2018, 77, 176–186. [Google Scholar] [CrossRef]
- Mossalou, D.; Masson, C.; Afquir, S.; Baqúe, P.; Arnoux, P.-J.; Bège, T. Mechanical effects of load speed on the human colon. J. Biomech. 2019, 91, 102–108. [Google Scholar] [CrossRef]
- Siri, S.; Maier, F.; Chen, L.; Santos, S.; Pierce, D.M.; Feng, B. Differential biomechanical properties of mouse distal colon and rectum innervated by the splanchnic and pelvic afferents. Am. J. Physiol.-Gastrointest. Liver Physiol. 2019, 316, G473–G481. [Google Scholar] [CrossRef] [PubMed]
- Bini, F.; Desideri, M.; Pica, A.; Novelli, S.; Marinozzi, F. 3D constitutive model of the rat large intestine: Estimation of the material parameters of the single layers. In Computer Methods, Imaging and Visualization in Biomechanics and Biomedical Engineering; Ateshian, G.A., Myers, K., Tavares, J.M.R.S., Eds.; CMBBE 2019. Lecture Notes in Computational Vision and Biomechanics; Springer Nature: Cham, Switzerland, 2020; Volume 36, pp. 608–623. [Google Scholar]
- Puértolas, S.; Peña, E.; Herrera, A.; Ibarz, E.; Garcia, L. A comparative study of hyperelastic constitutive models for colonic tissue fitted to multiaxial experimental testing. J. Mech. Behav. Biomed. Mater. 2020, 102, 103507. [Google Scholar] [CrossRef] [PubMed]
- Nováček, V.; Tran, T.N.; Klinge, U.; Tolba, R.H.; Staat, S.; Bronson, D.G.; Miesse, A.M.; Whiffen, J.; Turquier, F. Finite element modelling of stapled colorectal end-to-end anastomosis: Advantages of variable height stapler design. J. Biomech. 2012, 45, 2693–2697. [Google Scholar] [CrossRef] [PubMed]
- Rubod, C.; Brieu, M.; Cosson, M.; Rivaux, G.; Clay, J.C.; de Landsheere, L.; Gabriel, B. Biomechanical properties of human pelvic organs. Urology 2012, 79, 968.e17–968.e22. [Google Scholar] [CrossRef]
- Lecomte-Grosbras, P.; Diallo, M.N.; Witz, J.F.; Marchal, D.; Dequidt, J.; Cotin, S.; Cosson, M.; Duriez, C.; Brieu, M. Towards a better understanding of pelvic system disorders using numerical simulation. Med. Image Comput. Comput. Assist. Interv. 2013, 16 Pt 3, 307–314. [Google Scholar]
- Brandão, S.; Parente, M.; Mascarenhas, T.; Gomes da Silva, A.R.; Ramos, I.; Jorge, R.N. Biomechanical study on the bladder neck and urethral positions: Simulation of impairment of the pelvic ligaments. J. Biomech. 2015, 48, 217–223. [Google Scholar] [CrossRef]
- Chen, Z.W.; Joli, P.; Feng, Z.Q.; Rahim, M.; Pirró, N.; Bellemare, M.-E. Female patient-specific finite element modeling of pelvic organ prolapse (POP). J. Biomech. 2015, 48, 238–245. [Google Scholar] [CrossRef]
- Bhattarai, A.; Staat, M. Modelling of soft connective tissues to investigate female pelvic floor dysfunctions. Comp. Math. Methods Med. 2018, 2018, 9518076. [Google Scholar] [CrossRef] [Green Version]
- Suárez, G.; Vallejo, E.; Hoyos, L. Computational modelling of the behaviour of biomarker particles of colorectal cancer in fecal matter. In Proceedings of the XV International Conference on Computational Plasticity: Fundamentals and Applications, COMPLAS2019, Barcelona, Spain, 3–5 September 2019; pp. 334–342. [Google Scholar]
- Kondo, D.; Welemane, H.; Cormery, F. Basic concepts and models in continuum damage mechanics. Rev. Eur. Génie Civ. 2011, 11, 927–943. [Google Scholar]
- Lasn, K.; Echtermeyer, A.T.; Klauson, A.; Chati, F.; Décultot, D. An experimental study on the effects of matrix cracking to the stiffness of glass/epoxy cross plied laminates. Compos. Part B Eng. 2015, 80, 260–268. [Google Scholar] [CrossRef]
- Ricker, A.; Kröger, N.H.; Wriggers, P. Comparison of discontinuous damage models of Mullins-type. Arch. Appl. Mech. 2021, 91, 4097–4119. [Google Scholar] [CrossRef]
- Hasibeder, W. Gastrointestinal microcirculation: Still a mystery? Br. J. Anaesth. 2010, 105, 393–396. [Google Scholar] [CrossRef] [Green Version]
- Nordentoft, T.; Sørensen, M. Leakage of colon anastomoses: Development of an experimental model in pigs. Eur. Surg. Res. 2007, 39, 14–16. [Google Scholar] [CrossRef] [PubMed]
- Liao, D.; Zhao, J.; Gregersen, H. 3D mechanical properties of the partially obstructed guinea pig small intestine. J. Biomech. 2010, 43, 2079–2086. [Google Scholar] [CrossRef] [PubMed]
- Voermans, R.P.; Vergouwe, F.; Breedveld, P.; Fockens, P.; van Berge Henegouwen, M.I. Comparison of endoscopic closure modalities for standardized colonic perforations in a porcine colon model. Endoscopy 2011, 43, 217–222. [Google Scholar] [CrossRef] [PubMed]
- Holzapfel, G.A.; Gasser, T.C.; Ogden, R.W. A new constitutive framework for arterial wall mechanics and a comparative study of material models. J. Elast. Phys. Sci. Solids 2000, 61, 1–48. [Google Scholar]
- Feng, B.; Maier, F.; Siri, S.; Pierce, D.M. Quantifying the collagen-network morphology in mouse distal colon and rectum. In Proceedings of the Biomedical Engineering Society, 2019 Annual Fall Meeting, Philadelphia, PA, USA, 16–19 October 2019. [Google Scholar]
- Duong, M.T.; Nguyen, N.H.; Staat, M. Physical response of hyperelastic models for composite materials and soft tissues. Asia Pac. J. Comput. Eng. 2015, 2, 3. [Google Scholar] [CrossRef] [Green Version]
- Ogden, R.W. Nonlinear elasticity, anisotropy, material stability and residual stresses in soft tissue. In Biomechanics of Soft Tissue in Cardiovascular Systems; CISM Courses and Lectures Series; Holzapfel, G.A., Ogden, R.W., Eds.; Springer: Wien, Austria, 2003; Volume 441, pp. 65–108. [Google Scholar]
- Gasser, T.C.; Ogden, R.W.; Holzapfel, G.A. Hyperelastic modelling of arterial layers with distributed collagen fibre orientations. J. R. Soc. Interface 2005, 3, 15–35. [Google Scholar] [CrossRef]
- Furness, J.B.; Callaghan, B.P.; Rivera, L.R.; Cho, H.J. The enteric nervous system and gastrointestinal innervation: Integrated local and central control. Adv. Exp. Med. Biol. 2014, 817, 39–71. [Google Scholar]
- Patel, N.; Jiang, Y.; Mittal, R.K.; Kim, T.H.; Ledgerwood, M.; Bhargava, V. Circular and longitudinal muscles shortening indicates sliding patterns during peristalsis and transient lower esophageal sphincter relaxation. Am. J. Physiol. Gastrointest. Liver Physiol. 2015, 309, G360–G367. [Google Scholar] [CrossRef] [Green Version]
- Browning, K.N.; Travagli, R.A. Central nervous system control of gastrointestinal motility and secretion and modulation of gastrointestinal functions. Compr. Physiol. 2014, 4, 1339–1368. [Google Scholar] [PubMed] [Green Version]
- Wood, J.D. Peristalsis. In Encyclopedia of Gastroenterology; Johnson, L.R., Ed.; Elsevier: New York, NY, USA, 2004; pp. 164–165. [Google Scholar]
- Sanders, K.M.; Koh, S.D.; Ro, S.; Ward, S.M. Regulation of gastrointestinal motility-insights from smooth muscle biology. Nat. Rev. Gastroenterol. Hepatol. 2012, 9, 633–645. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ambache, N. The electrical activity of isolated mammalian intestines. J. Physiol. 1947, 106, 139–153. [Google Scholar] [CrossRef] [Green Version]
- Langton, P.; Ward, S.M.; Norell, M.A.; Sanders, K.M. Spontaneous electrical activity of interstitial cells of Cajal isolated from canine proximal colon. Proc. Natl. Acad. Sci. USA 1989, 86, 7280–7284. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lino, S.; Nojyo, Y. Immunohistochemical demonstration of c-Kit-negative fibroblast-like cells in murine gastrointestinal musculature. Arch. Histol. Cytol. 2009, 72, 107–115. [Google Scholar]
- Frearson, N.; Focant, B.W.; Perry, S.V. Phosphorylation of a light chain component of myosin from smooth muscle. FEBS Lett. 1976, 63, 27–32. [Google Scholar] [PubMed] [Green Version]
- Onishi, H.; Iljima, S.; Anzai, H.; Watanabe, S. Possible role of myosin light-chain phosphatase in the relaxation of chicken gizzard muscle. J. Biochem. 1979, 86, 1283–1290. [Google Scholar] [CrossRef]
- Aksoy, M.O.; Mras, S.; Kamm, K.E.; Murphy, R.A. Ca2+, cAMP, and changes in myosin phosphorylation during contraction of smooth muscle. Am. J. Physiol. 1983, 245, C255–C270. [Google Scholar] [CrossRef]
- Murphy, R.A. Contractile system function in mammalian smooth muscle. J. Vasc. Res. 1976, 13, 1–23. [Google Scholar] [CrossRef]
- Frotscher, R.; Koch, J.P.; Staat, M. Computational investigation of drug action on human-induced stem cell-derived cardiomyocytes. J. Biomech. Eng. 2015, 137, 071002. [Google Scholar] [CrossRef]
- Frotscher, R.; Muanghong, D.; Dursun, G.; Goßmann, M.; Temiz-Artmann, A.; Staat, M. Sample-specific adaption of an improved electro-mechanical model of in vitro cardiac tissue. J. Biomech. 2016, 49, 2428–2435. [Google Scholar] [CrossRef] [PubMed]
- Murphy, R.A. Filament organization and contractile function in vertebrate smooth muscle. Annu. Rev. Physiol. 1979, 41, 737–748. [Google Scholar] [CrossRef] [PubMed]
- Hill, A.V. The heat of shortening and dynamics constants of muscles. Proc. R. Soc. Lond. Ser. B-Biol. Sci. 1938, 126, 136–195. [Google Scholar]
- Huxley, A.F.; Niedergerke, R. Structural changes in muscle during contraction. Nature 1954, 173, 971–973. [Google Scholar] [CrossRef] [PubMed]
- Huxley, H.E.; Hanson, J. Changes in the cross-striations of muscle during contraction and stretch and their structural interpretation. Nature 1954, 173, 973–976. [Google Scholar] [CrossRef]
- Huxley, A.F. Muscle structure and theories of contraction. Prog. Biophys. Biophys. Chem. 1938, 7, 255–318. [Google Scholar] [CrossRef]
- Hodgkin, A.L.; Huxley, A.F. A quantitative description of membrane current and its application to conduction and excitation in nerve. J. Physiol. 1952, 117, 500–544. [Google Scholar] [CrossRef]
- Feng, B.; Zhu, Y.; La, J.-H.; Wills, Z.P.; Gebhart, G.F. Experimental and computational evidence for an essential role of NaV1.6 in spike initiation at stretch-sensitive colorectal afferent endings. J. Neurophysiol. 2015, 113, 2618–2634. [Google Scholar] [CrossRef] [Green Version]
- Hai, C.M.; Murphy, R.A. Cross-bridge phosphorylation and regulation of latch state in smooth muscle. Am. J. Physiol. 1988, 254 Pt 1, C99–C106. [Google Scholar] [CrossRef]
- Wingard, C.J.; Nowocin, J.M.; Murphy, R.A. Cross-bridge regulation by Ca(2+)-dependent phosphorylation in amphibian smooth muscle. Am. J. Physiol. Regul. Integr. Comp. Physiol. 2001, 281, R1769–R1777. [Google Scholar] [CrossRef] [Green Version]
- Singer, H.A.; Kamm, K.E.; Murphy, R.A. Estimates of activation in arterial smooth muscle. Am. J. Physiol. 1989, 251 Pt 1, C465–C473. [Google Scholar] [CrossRef] [PubMed]
- Kato, S.; Osa, T.; Ogasawara, T. Kinetic model for isometric contraction in smooth muscle on the basis of myosin phosphorylation hypothesis. Biophys. J. 1984, 46, 35–44. [Google Scholar] [CrossRef] [Green Version]
- Kroon, M. A constitutive model for smooth muscle including active tone and passive viscoelastic behaviour. Math. Med. Biol. 2010, 27, 129–155. [Google Scholar] [CrossRef]
- Gizzi, A.; Pandolfi, A. Visco-hyperelasticity of electro-active soft tissues. Procedia IUTAM Symp. Mech. Soft Act. Mater. 2015, 12, 162–175. [Google Scholar] [CrossRef] [Green Version]
- Sinnott, M.D.; Cleary, P.W.; Arkwright, J.W.; Dinning, P.G. Investigating the relationships between peristaltic contraction and fluid transport in the human colon using smoothed particle hydrodynamics. Comput. Biol. Med. 2012, 42, 492–503. [Google Scholar] [CrossRef] [Green Version]
- Avanzini, A.; Battini, D. Integrated experimental and numerical comparison of different approaches for planar biaxial testing of a hyperelastic material. Adv. Mater. Sci. Eng. 2016, 2016, 6014129. [Google Scholar] [CrossRef] [Green Version]
- Nguyen, N.H.; Duong, M.T.; Tran, T.N.; Pham, P.T.; Grottke, O.; Tolba, R.; Staat, M. Influence of a freeze-thaw cycle on the stress-stretch curves of tissues of porcine abdominal organs. J. Biomech. 2012, 45, 2382–2386. [Google Scholar] [CrossRef] [PubMed]
- Wang, I.; Politi, A.Z.; Tania, N.; Bai, Y.; Sanderson, M.J.; Sneyd, J. A mathematical model of airway and pulmonary arteriole smooth muscle. Biophys. J. 2008, 94, 2053–2064. [Google Scholar] [CrossRef] [Green Version]
- Yang, J.; Clark, J.W., Jr.; Bryan, R.M.; Robertson, C. The myogenic response in isolated rat cerebrovascular arteries: Smooth muscle cell model. Med. Eng. Phys. 2003, 25, 691–709. [Google Scholar] [CrossRef] [Green Version]
- Stålhand, J.; Klarbring, A.; Holzapfel, G.A. Smooth muscle contraction: Mechanochemical formulation for homogeneous finite strain. Prog. Biophys. Mol. Biol. 2008, 96, 465–481. [Google Scholar] [CrossRef]
- Tan, T.; De Vita, R. A structural constitutive model for smooth muscle contraction in biological tissues. Int. J. Non-Linear Mech. 2015, 75, 46–53. [Google Scholar] [CrossRef]
- Schmitz, A.; Böl, M. On a phenomenological model for active smooth muscle contraction. J. Biomech. 2011, 44, 2090–2095. [Google Scholar] [CrossRef] [PubMed]
- Chen, H.; Kassab, G.S. Microstructure-based constitutive model of coronary artery with active smooth muscle contraction. Sci. Rep. 2017, 7, 9339. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gestrelius, S.; Borgström, P. A dynamic model of smooth muscle contraction. Biophys. J. 1986, 50, 157–169. [Google Scholar] [CrossRef] [Green Version]
- Brandstaeter, S.; Gizzi, A.; Fuchs, S.L.; Gebauer, A.M.; Aydin, R.C.; Cyron, C.J. Computational model of gastric motility with active-strain electromechanics. ZAMM 2018, 98, 2177–2197. [Google Scholar] [CrossRef] [Green Version]






| Specimen | (MPa) | (MPa) | (MPa) | ||||
|---|---|---|---|---|---|---|---|
| 1 | 0.001 | 0.0171 | 1.5827 | 0.0233 | 2.4303 | 0.9900 | 0.9878 |
| 2 | 0.001 | 0.0153 | 0.8525 | 0.0043 | 2.5866 | 0.9968 | 0.9944 |
| 3 | 0.001 | 0.0268 | 0.5080 | 0.0072 | 2.0980 | 0.9924 | 0.9980 |
| 4 | 0.001 | 0.0218 | 1.9014 | 0.0125 | 0.9415 | 0.9873 | 0.9904 |
| 5 | 0.002 | 0.0061 | 0.7490 | 0.0300 | 0.4768 | 0.9798 | 0.9846 |
| Average curve | 0.0048 | 0.0133 | 1.2090 | 0.0136 | 1.2483 | 1.0000 | 1.0000 |
| I Electrical-chemical-mechanical constitutive parameters. Units in cm, s | ||||||||||||
| LM: | = 10 | = | = 0 | = 8 | = | = 1 | ||||||
| ICC: | = 7 | = | = | = 8 | = | = | ||||||
| LM: | = | = | ||||||||||
| ICC: | = | = | ||||||||||
| II Viscoelastic-active electromechanical model parameters | ||||||||||||
| kPa | kPa | kPa | kPa | - | kPa | - | kPa s | kPa s | - | - | - | - |
| 5.5 | 1 | 1 | 55 | 56 | 20 | 29 | 0.125 | 0.09 | 1 | 5 | 3 | 12 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bhattarai, A.; Horbach, A.J.; Staat, M.; Kowalczyk, W.; Tran, T.N. Virgin Passive Colon Biomechanics and a Literature Review of Active Contraction Constitutive Models. Biomechanics 2022, 2, 138-157. https://doi.org/10.3390/biomechanics2020013
Bhattarai A, Horbach AJ, Staat M, Kowalczyk W, Tran TN. Virgin Passive Colon Biomechanics and a Literature Review of Active Contraction Constitutive Models. Biomechanics. 2022; 2(2):138-157. https://doi.org/10.3390/biomechanics2020013
Chicago/Turabian StyleBhattarai, Aroj, Andreas Johannes Horbach, Manfred Staat, Wojciech Kowalczyk, and Thanh Ngoc Tran. 2022. "Virgin Passive Colon Biomechanics and a Literature Review of Active Contraction Constitutive Models" Biomechanics 2, no. 2: 138-157. https://doi.org/10.3390/biomechanics2020013
APA StyleBhattarai, A., Horbach, A. J., Staat, M., Kowalczyk, W., & Tran, T. N. (2022). Virgin Passive Colon Biomechanics and a Literature Review of Active Contraction Constitutive Models. Biomechanics, 2(2), 138-157. https://doi.org/10.3390/biomechanics2020013








