Static and Dynamic Cone Penetrometer Tests for Babolsar Sand Parameters via Physical Modeling
Abstract
:1. Introduction
2. Materials
3. Methods
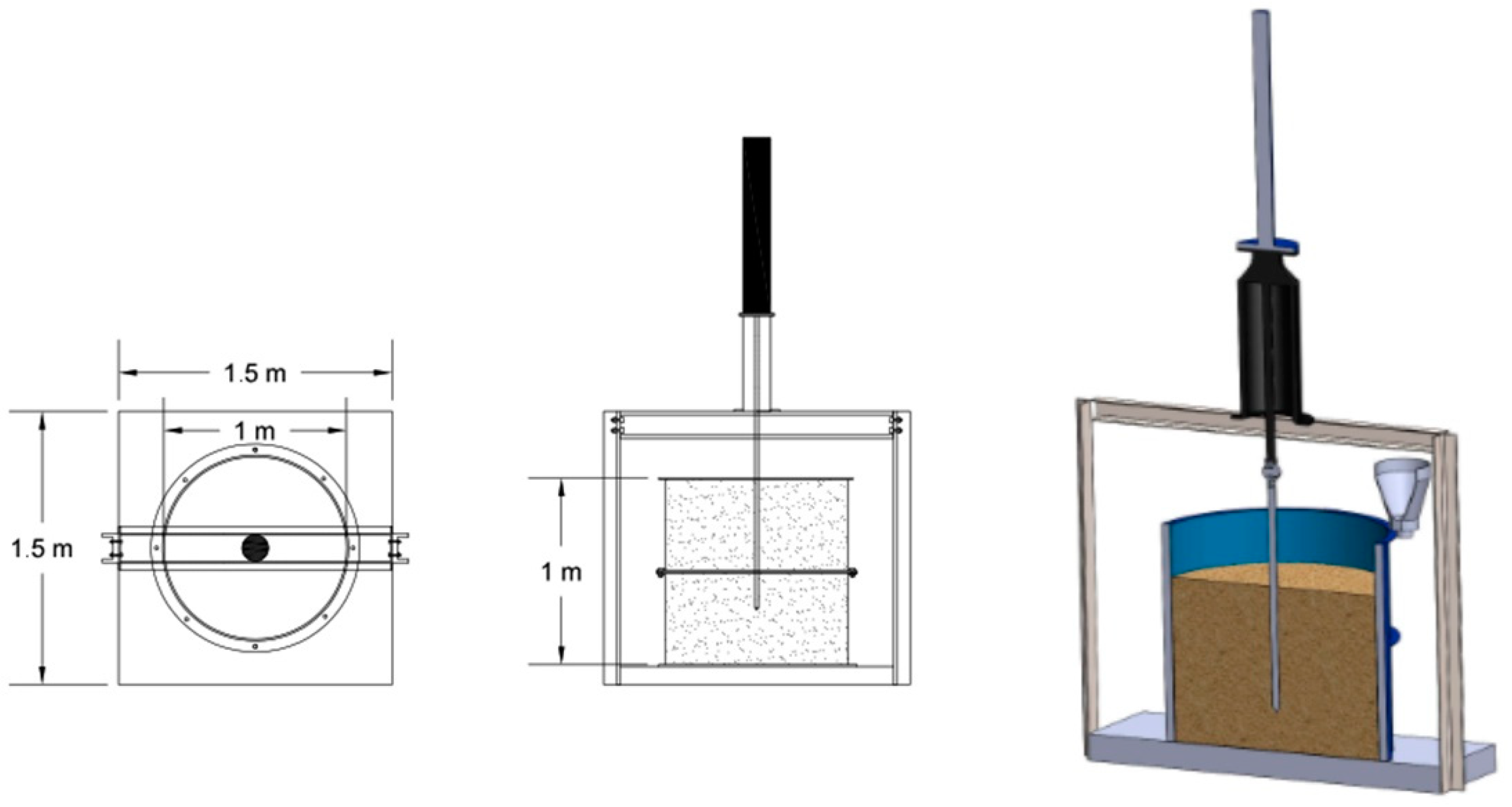
3.1. Soil Preparation and 1 g Chamber
3.2. Laboratory Investigations (CPT, DCP, and PLT)
3.2.1. Static Cone Penetration Test (CPT)
3.2.2. Dynamic Cone Penetration Test (DCP)
3.2.3. Plate Load Test
4. Results
4.1. Repeatability of Tests
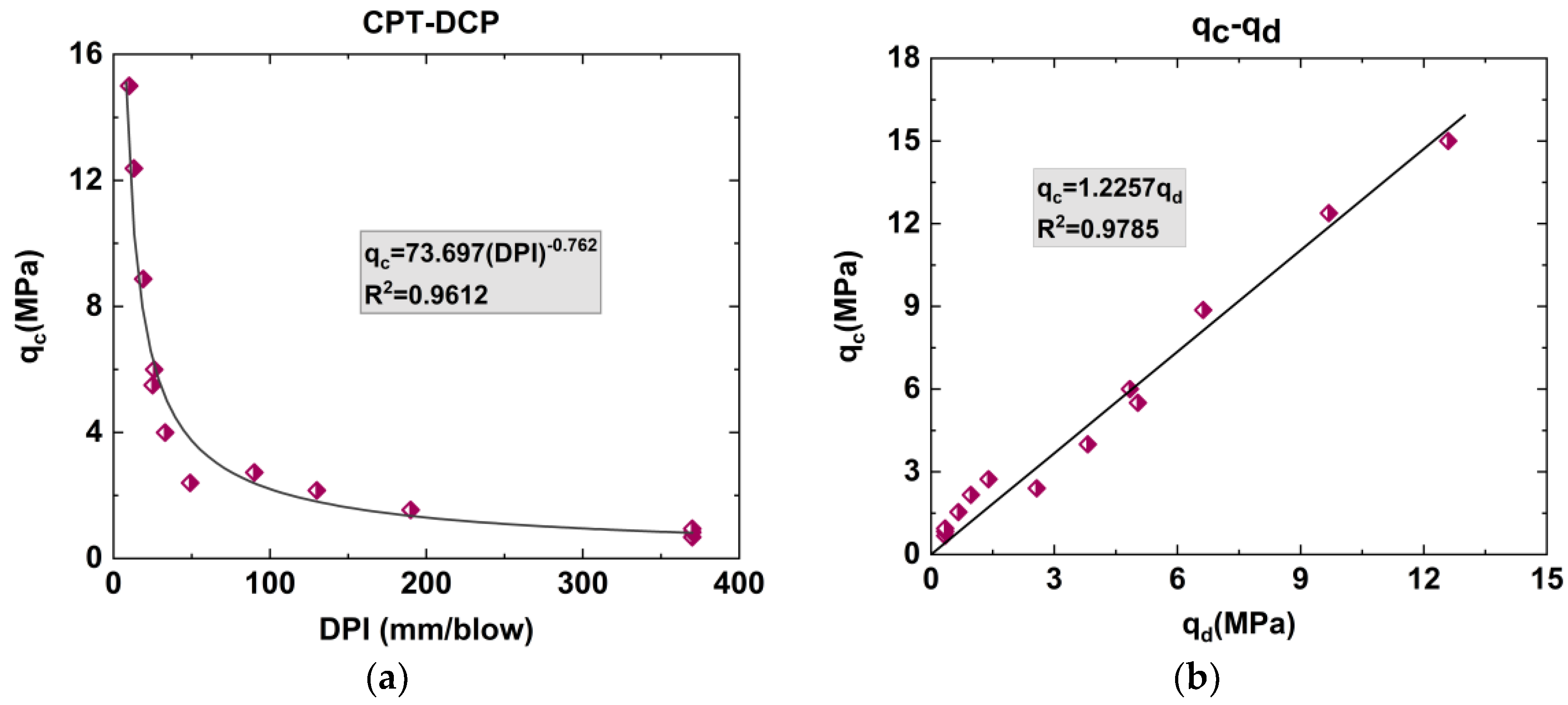
4.2. CPT Cone Resistance () vs. DCP Cone Resistance () and DCP Penetration Index (DPI)
| Reference | |
|---|---|
| Da Fonseca [48] | 1 |
| Dos Santos and Bicalho [49] | 1.3–2.5 |
| Rios et al. [50] | 0.87–1.85 |
| Kodicherla and Nandyala [51] | 1.12 |
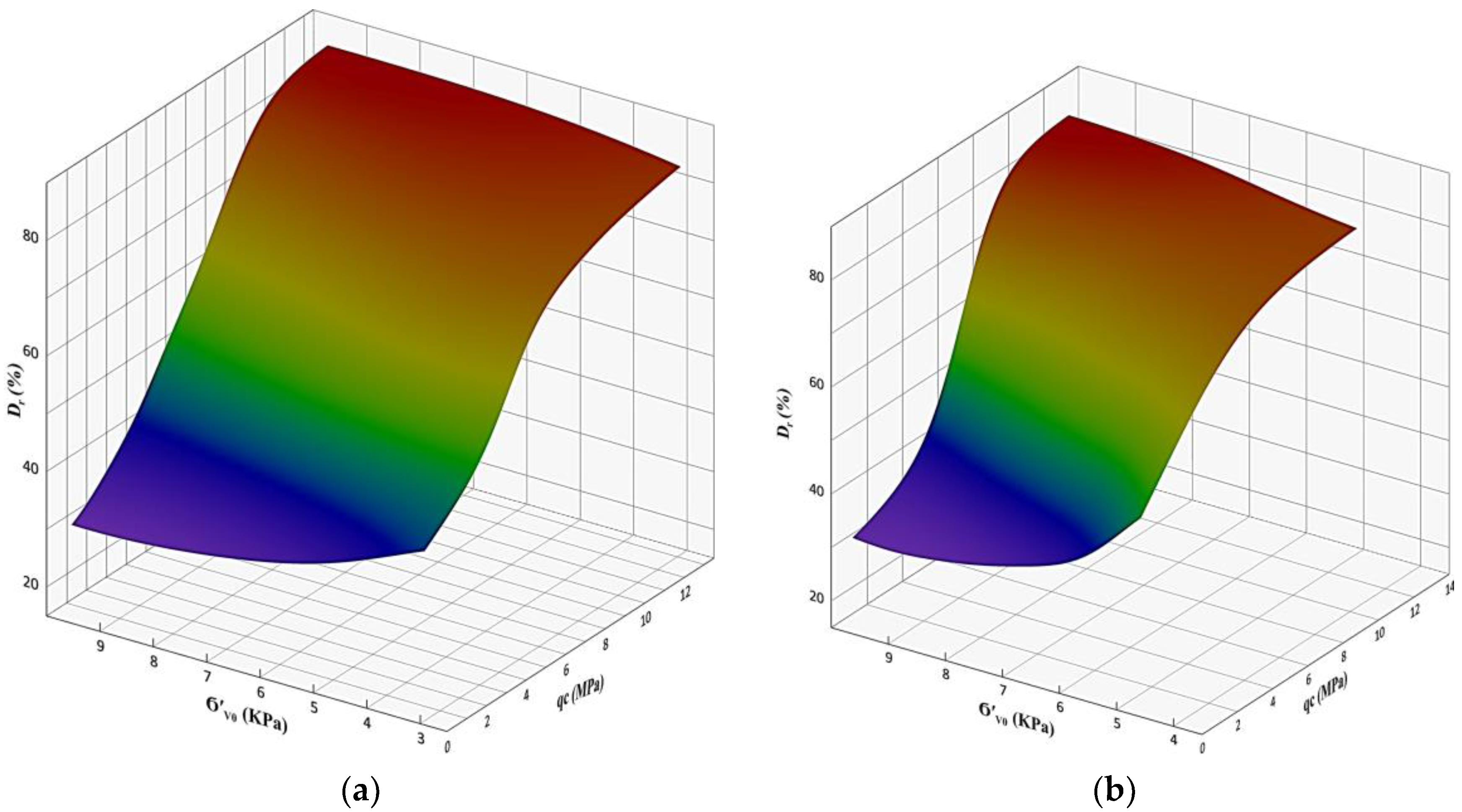
4.3. Relative Density (Dr (%)) vs. CPT Cone Resistance () and DCP Cone Resistance ()
4.4. Comparisons and Discussions
4.5. Comparisons, DCP Penetration Index (DPI) versus Relative Density (Dr %)
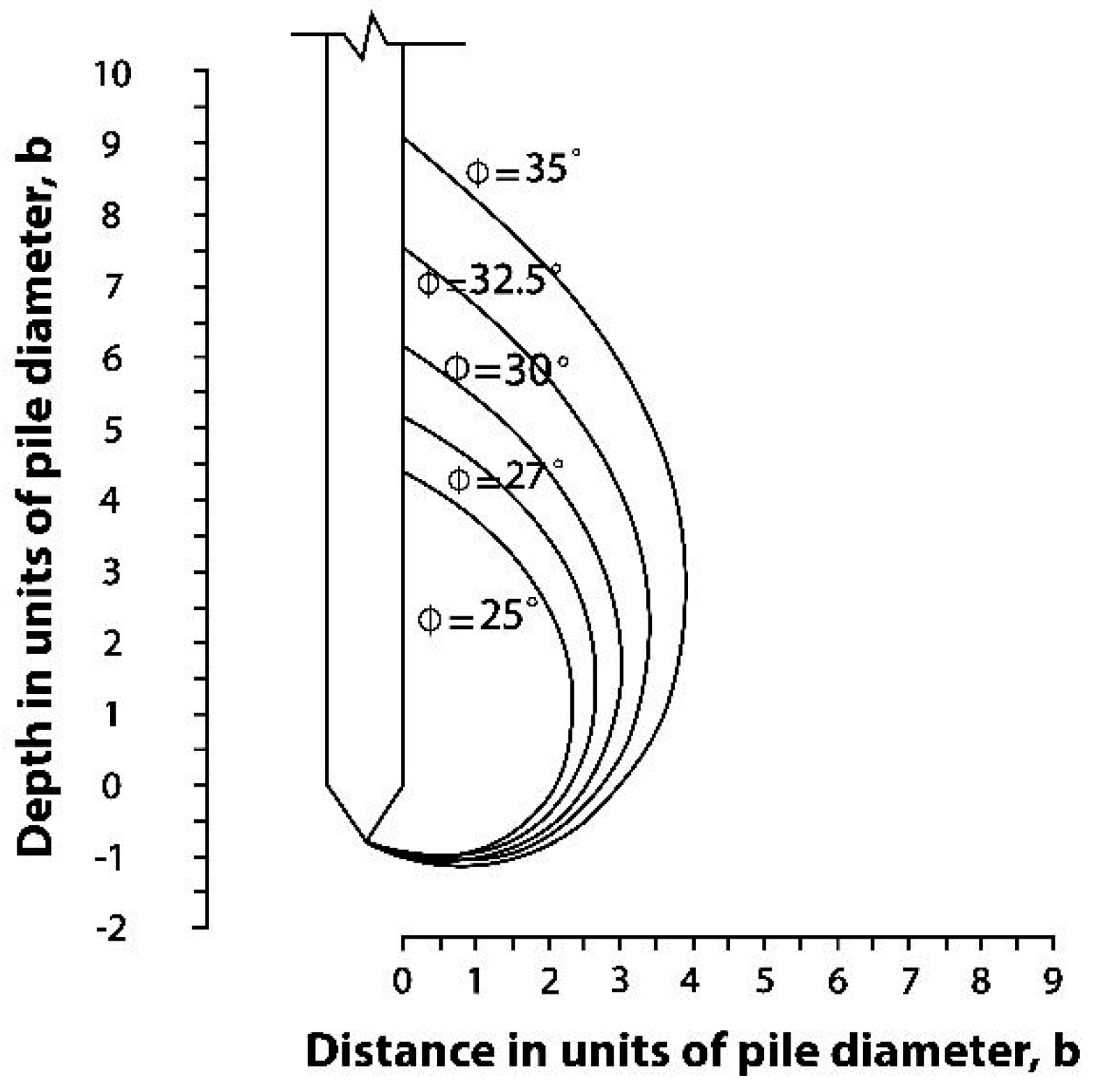
4.6. Friction Angle () versus CPT Cone Resistance () and DCP Cone Resistance ()
4.7. Friction Angle versus DCP Penetration Index (DPI)
4.8. Friction Angle versus Relative Density (Dr (%))
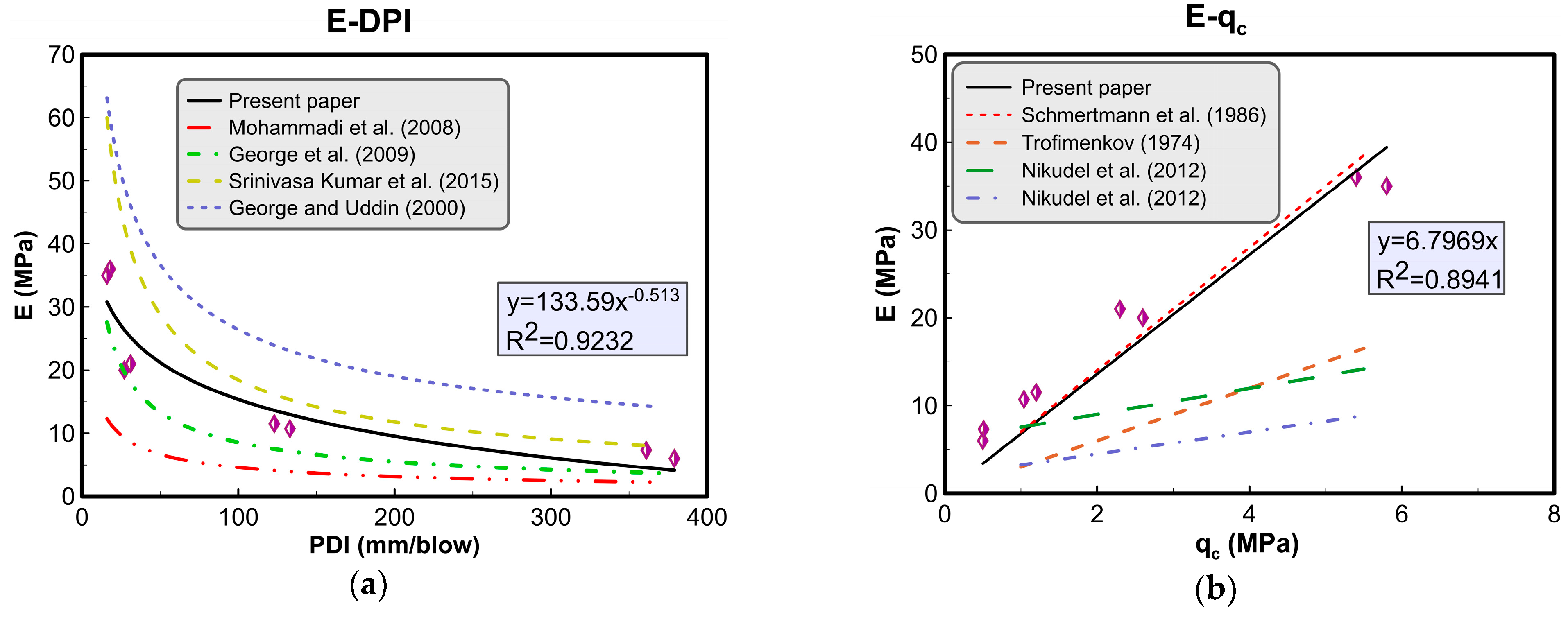
4.9. Modulus of Elasticity (E) versus Dynamic and Static Cone Penetration Test Parameters
4.10. Practical Implications
5. Conclusions
- An increase in the relative density value increases the interaction between soil particles; due to this phenomenon, the internal friction angle value is also increased. The results showed that there is a linear relationship between these two parameters;
- The cone resistance is increased in both CPT and DCP tests by an increase in the internal friction angle and relative density, and there is a logarithmic correlation between these two;
- An increase in the soil’s relative density increases the cone resistance in both CPT and DCP tests, and there is a linear correlation between these two parameters. In addition, under the same condition, the DPI factor value had an inverse correlation with cone resistance;
- The elasticity modulus is a suitable geotechnical parameter for determining soil stiffness in sandy soils. The cone resistance value in both CPT and DCP tests is increased linearly by an increase in the elasticity modulus of sands. There was also a nonlinear correlation between the DPI factor and these two parameters;
- The dynamic and static penetration tests had the same nature, and the soil shear bands formed completely during the penetration. The correlations between the parameters of both tests had a high coefficient of determination (R2) and accuracy. On the other hand, the plate load test measured stiffness and no shear band formed. Therefore, the correlation between PLT and CPT parameters had lower R2 values.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
List of Notations
| CPT | Cone penetration test |
| DCP | Dynamic cone penetration |
| Cone resistance for CPT | |
| Cone resistance for DCP | |
| Stress-normalized cone resistance | |
| Bearing capacity factor | |
| Sleeve friction for CPT | |
| DPI | DCP index |
| Friction angle | |
| P | Penetration of DCP |
| B | Blow count |
| A | Cross-sectional area of the cone |
| KE | Imparted kinetic energy |
| X | Incremental penetration |
| M | Mass of the hammer |
| P | Mass of the penetrometer |
| Dr | Relative density |
| E | Elastic modulus |
| The vertical stress | |
| Effective vertical stress | |
| Horizontal stress | |
| Effective horizontal stress | |
| Effective surcharge stress | |
| SP | Poorly graded sand |
| Diameter for 50% finer by weight | |
| Maximum void ratio | |
| Minimum void ratio | |
| Maximum dry density | |
| Minimum dry density | |
| The specific gravity | |
| Coefficient of uniformity | |
| Coefficient of curvature | |
| Optimum water content |
References
- Nobahar, M.; Khan, M.S.; Ivoke, J. Combined effect of rainfall and shear strength on the stability of highway embankments made of Yazoo clay in Mississippi. Geotech. Geol. Eng. 2020, 38, 2787–2802. [Google Scholar] [CrossRef]
- Juang, C.H.; Ching, J.; Wang, L.; Khoshnevisan, S.; Ku, C.-S. Simplified procedure for estimation of liquefaction-induced settlement and site-specific probabilistic settlement exceedance curve using cone penetration test (CPT). Can. Geotech. J. 2013, 50, 1055–1066. [Google Scholar] [CrossRef]
- Lehane, B. CPT-based design of foundations. Aust. Geomech. J. 2019, 54, 23–48. [Google Scholar]
- Esmailzade, M.; Eslami, A.; Nabizadeh, A.; Aflaki, E. Effect of cone diameter on determination of penetration resistance using a FCV. Int. J. Civ. Eng. 2022, 20, 223–236. [Google Scholar] [CrossRef]
- Eslami, A.; Ebrahimipour, A. Load-displacement appraisal and analysis for driven piles; a data-centric approach. Comput. Geotech. 2024, 171, 106377. [Google Scholar] [CrossRef]
- Ebrahimipour, A.; Eslami, A. Analytical study of piles behavior for marine challenging substructures. Ocean Eng. 2024, 292, 116514. [Google Scholar] [CrossRef]
- Lee, J.-S.; Kim, S.Y.; Hong, W.-T.; Byun, Y.-H. Assessing subgrade strength using an instrumented dynamic cone penetrometer. Soils Found. 2019, 59, 930–941. [Google Scholar] [CrossRef]
- Miller, G.A.; Tan, N.K.; Collins, R.W.; Muraleetharan, K.K. Cone penetration testing in unsaturated soils. Transp. Geotech. 2018, 17, 85–99. [Google Scholar] [CrossRef]
- Robertson, P.K.; Campanella, R. Interpretation of cone penetration tests. Part I: Sand. Can. Geotech. J. 1983, 20, 718–733. [Google Scholar] [CrossRef]
- Eslami, A.; Fellenius, B.H. Pile capacity by direct CPT and CPTu methods applied to 102 case histories. Can. Geotech. J. 1997, 34, 886–904. [Google Scholar] [CrossRef]
- Shirani, S.S.; Eslami, A.; Ebrahimipour, A.; Karakouzian, M. Dominant factors in MiniCone, CPT and pile correlations: A data-based approach. Deep Undergr. Sci. Eng. 2023, 2, 346–358. [Google Scholar] [CrossRef]
- Guo, L.; Liu, X.; Yang, Z.; Jia, C.; Shi, W.; Ling, X. CPT-based analysis of structured soil characteristics and liquefaction failure of the Yellow River Subaquatic Delta. Mar. Georesour. Geotechnol. 2022, 40, 308–320. [Google Scholar] [CrossRef]
- Bienen, B.; Qiu, G.; Pucker, T. CPT correlation developed from numerical analysis to predict jack-up foundation penetration into sand overlying clay. Ocean Eng. 2015, 108, 216–226. [Google Scholar] [CrossRef]
- Souza, J.; Danziger, B.; Danziger, F. The influence of the relative density of sands in SPT and CPT correlations. Soils Rocks 2012, 35, 99–113. [Google Scholar]
- Chang, M.-F.; Yu, G.; Na, Y.-M.; Choa, V. Evaluation of relative density profiles of sand fill at a reclaimed site. Can. Geotech. J. 2006, 43, 903–914. [Google Scholar] [CrossRef]
- Mayne, P. In-situ test calibrations for evaluating soil parameters. Charact. Eng. Prop. Nat. Soils 2007, 3, 1601–1652. [Google Scholar]
- Robertson, P.K. Cone penetration test (CPT)-based soil behaviour type (SBT) classification system—An update. Can. Geotech. J. 2016, 53, 1910–1927. [Google Scholar] [CrossRef]
- Ghafghazi, M.; Shuttle, D. Interpretation of sand state from cone penetration resistance. Géotechnique 2008, 58, 623–634. [Google Scholar] [CrossRef]
- Giretti, D.; Been, K.; Fioravante, V.; Dickenson, S. CPT calibration and analysis for a carbonate sand. Géotechnique 2018, 68, 345–357. [Google Scholar] [CrossRef]
- Lee, J.; Kyung, D.; Kim, B.; Prezzi, M. Estimation of the small-strain stiffness of clean and silty sands using stress-strain curves and CPT cone resistance. Soils Found. 2009, 49, 545–556. [Google Scholar] [CrossRef]
- Motaghedi, H.; Eslami, A. Analytical approach for determination of soil shear strength parameters from CPT and CPTu data. Arab. J. Sci. Eng. 2014, 39, 4363–4376. [Google Scholar] [CrossRef]
- Ouyang, Z.; Mayne, P.W. Effective friction angle of clays and silts from piezocone penetration tests. Can. Geotech. J. 2018, 55, 1230–1247. [Google Scholar] [CrossRef]
- Robertson, P.K. Interpretation of cone penetration tests—A unified approach. Can. Geotech. J. 2009, 46, 1337–1355. [Google Scholar] [CrossRef]
- Lim, Y.X.; Tan, S.A.; Phoon, K.-K. Friction angle and overconsolidation ratio of soft clays from cone penetration test. Eng. Geol. 2020, 274, 105730. [Google Scholar] [CrossRef]
- Arshad, M.; Tehrani, F.; Prezzi, M.; Salgado, R. Experimental study of cone penetration in silica sand using digital image correlation. Géotechnique 2014, 64, 551–569. [Google Scholar] [CrossRef]
- Robertson, P. Soil classification using the cone penetration test: Reply. Can. Geotech. J. 1991, 28, 176–178. [Google Scholar] [CrossRef]
- Fakharian, K.; Shafiei, M.; Hafezan, S. Investigation of soil setup effects on pile response in clay considering overconsolidation ratio and installation method through physical modeling. Can. Geotech. J. 2022, 60, 488–503. [Google Scholar] [CrossRef]
- Abu-Farsakh, M.Y.; Titi, H.H. Assessment of direct cone penetration test methods for predicting the ultimate capacity of friction driven piles. J. Geotech. Geoenviron. Eng. 2004, 130, 935–944. [Google Scholar] [CrossRef]
- Rahim, A.; George, K. Models to estimate subgrade resilient modulus for pavement design. Int. J. Pavement Eng. 2005, 6, 89–96. [Google Scholar] [CrossRef]
- Ampadu, S.I.K.; Ackah, P.; Nimo, F.O.; Boadu, F. A laboratory study of horizontal confinement effect on the dynamic cone penetration index of a lateritic soil. Transp. Geotech. 2017, 10, 47–61. [Google Scholar] [CrossRef]
- Hamid, A.M. The dynamic cone penetration test: A review of its correlations and applications. In Proceedings of the International Conference on Advances in Civil and Environmental Engineering, Pulau Pinang, Malaysia, 28–30 July 2015. [Google Scholar]
- Esmailzade, M.; Eslami, A.; McCartney, J.S. Comparison of frustum confining vessel (FCV) and full-scale testing for helical and expanded piles geotechnical performance. Mar. Georesour. Geotechnol. 2024, 1–21. [Google Scholar] [CrossRef]
- ASTM D2487; Standard Test Methods for Particle-Size Distribution (Gradation) of Soils Using Sieve Analysis. ASTM International: West Conshohocken, PA, USA, 2017.
- ASTM D4253; Standard Test Methods for Maximum Index Density and Unit Weight of Soils Using a Vibratory Table. ASTM International: West Conshohocken, PA, USA, 2016.
- ASTM D4254; Standard Test Methods for Minimum Index Density and Unit Weight of Soils and Calculation of Relative Density. ASTM International: West Conshohocken, PA, USA, 2016.
- ASTM D854; Standard Test Methods for Specific Gravity of Soil Solids by the Water Displacement Method. ASTM International: West Conshohocken, PA, USA, 2023.
- ASTM D698; Standard Test Methods for Laboratory Compaction Characteristics of Soil Using Standard Effort (12,400 ft-lbf/ft3 (600 kN-m/m3)). ASTM International: West Conshohocken, PA, USA, 2012.
- Mohammadi, S.; Nikoudel, M.; Rahimi, H.; Khamehchiyan, M. Application of the Dynamic Cone Penetrometer (DCP) for determination of the engineering parameters of sandy soils. Eng. Geol. 2008, 101, 195–203. [Google Scholar] [CrossRef]
- Moghadasi, H.; Eslami, A.; Akbarimehr, D.; Asgari, S. Assessment of adjacent foundations consequences and solutions for remediation via physical modeling. Soil Dyn. Earthq. Eng. 2024, 180, 108613. [Google Scholar] [CrossRef]
- Eslami, A.; Afshar, D.; Moghadasi, H.; Akbarimehr, D. Numerical and Experimental Investigations of Interference Effect of Adjacent Buildings on Sand and Fill Deposits. Int. J. Civ. Eng. 2024, 22, 723–738. [Google Scholar] [CrossRef]
- ASTM D5778; Standard Test Method for Electronic Friction Cone and Piezocone Penetration Testing of Soils. ASTM International: West Conshohocken, PA, USA, 2020.
- ASTM D6951; Standard Test Method for Use of the Dynamic Cone Penetrometer in Shallow Pavement Applications. ASTM International: West Conshohocken, PA, USA, 2018.
- Embacher, R.A. Duration of spring thaw recovery for aggregate-surfaced roads. Transp. Res. Rec. 2006, 1967, 27–35. [Google Scholar] [CrossRef]
- Gamache, R.W.; Kianirad, E.; Alshawabkeh, A.N. An automatic portable near surface soil characterization system. In Proceedings of the Recent Advancement in Soil Behavior, in Situ Test Methods, Pile Foundations, and Tunneling: Selected Papers from the 2009 GeoHunan International Conference, Changsha, China, 3–6 August 2009; pp. 89–94. [Google Scholar]
- Fonseca, A.V.d. Load tests on residual soil and settlement prediction on shallow foundation. J. Geotech. Geoenviron. Eng. 2001, 127, 869–883. [Google Scholar] [CrossRef]
- Consoli, N.C.; Schnaid, F.; Milititsky, J. Interpretation of plate load tests on residual soil site. J. Geotech. Geoenviron. Eng. 1998, 124, 857–867. [Google Scholar] [CrossRef]
- ASTM D1195; Standard Test Method for Repetitive Static Plate Load Tests of Soils and Flexible Pavement Components, for Use in Evaluation and Design of Airport and Highway Pavements. ASTM International: West Conshohocken, PA, USA, 2009.
- da Fonseca, A.J.P.V. Geomecânica dos Solos Residuais do Granito do Porto: Critérios para Dimensionamento de Fundações Directas; Universidade do Porto: Porto, Portugal, 1996. [Google Scholar]
- dos Santos, M.D.; Bicalho, K.V. Proposals of SPT-CPT and DPL-CPT correlations for sandy soils in Brazil. J. Rock Mech. Geotech. Eng. 2017, 9, 1152–1158. [Google Scholar] [CrossRef]
- Rios, S.; da Fonseca, A.V.; Pinheiro, C.; Molina-Gómez, F.; Miranda, T. Correlations between Dynamic Penetrometer Light and Cone Penetration Tests in Intermediate Soils: A Statistical Comparison. In Proceedings of the XIX Congresso Brasileiro de Mecânica dos Solos e Engenharia Geotécnica Geotecnia e Desenvolvimento Urbano, Salvador, Brazil, 28 August–1 September 2018. [Google Scholar]
- Kodicherla, S.P.K.; Nandyala, D.K. Use of CPT and DCP based correlations in characterization of subgrade of a highway in Southern Ethiopia Region. Int. J. Geo-Eng. 2016, 7, 11. [Google Scholar] [CrossRef]
- Schmertmann, J.H. Guidelines for Cone Penetration Test: Performance and Design; United States. Federal Highway Administration: Washington, DC, USA, 1978. [Google Scholar]
- Jamiolkowski, M.; Lo Presti, D.; Manassero, M. Evaluation of relative density and shear strength of sands from CPT and DMT. In Soil Behavior and Soft Ground Construction; American Society of Civil Engineers (ASCE): Reston, VA, USA, 2003; pp. 201–238. [Google Scholar]
- Sadrekarimi, A. Evaluation of CPT-based characterization methods for loose to medium-dense sands. Soils Found. 2016, 56, 460–472. [Google Scholar] [CrossRef]
- Hamid, A.M.; Al-Amoudi, O.S.B.; Aiban, S.A. Assessing the effect of density and water level on the degree of compaction of sand using dynamic cone penetration test. Arab. J. Sci. Eng. 2019, 44, 4921–4930. [Google Scholar] [CrossRef]
- Katakiya, M.; Parekh, A. Effect of gradation and particle size on correlations between DCP Index (ASTM D6951-03) and relative density for sand. In Proceedings of the International Conference on Research and Innovations in Science, Engineering and Technology, Anand, India, 19–21 February 2017; pp. 185–191. [Google Scholar]
- Kulhawy, F.H.; Mayne, P.W. Manual on Estimating Soil Properties for Foundation Design; Electric Power Research Institute: Palo Alto, CA, USA; Cornell University: Ithaca, NY, USA, 1990. [Google Scholar]
- Uzielli, M.; Mayne, P.W.; Cassidy, M.J. Probabilistic assignment of design strength for sands from in-situ testing data. In Modern Geotechnical Design Codes of Practice; IOS Press: Amsterdam, The Netherlands, 2013; pp. 214–227. [Google Scholar]
- Houlsby, G.; Hitchman, R. Calibration chamber tests of a cone penetrometer in sand. Geotechnique 1988, 38, 39–44. [Google Scholar] [CrossRef]
- Jeong, S.; Lee, J.; Lee, C.J. Slip effect at the pile–soil interface on dragload. Comput. Geotech. 2004, 31, 115–126. [Google Scholar] [CrossRef]
- Senneset, K.; Sandven, R.; Lunne, T.; Amundsen, T. Piezocone tests in silty soils. In Proceedings of the International Symposium on Penetration Testing; ISOPT-1. 1, Orlando, FL, USA, 20–24 March 1988; pp. 955–966. [Google Scholar]
- Fakharian, K.; Kaviani-Hamedani, F.; Imam, S.R. Influences of initial anisotropy and principal stress rotation on the undrained monotonic behavior of a loose silica sand. Can. Geotech. J. 2022, 59, 847–862. [Google Scholar] [CrossRef]
- Kaviani-Hamedani, F.; Esmailzade, M.; Adineh, K.; Shafiei, M.; Shirkavand, D. Quantifying three-dimensional sphericity indices of irregular fine particles from 2D images through sequential sieving tests. Granul. Matter 2024, 26, 13. [Google Scholar] [CrossRef]
- Kaviani-Hamedani, F.; Fakharian, K.; Lashkari, A. Bidirectional shear wave velocity measurements to track fabric anisotropy evolution of a crushed silica sand during shearing. J. Geotech. Geoenviron. Eng. 2021, 147, 04021104. [Google Scholar] [CrossRef]
- Kaviani-Hamedani, F.; Fakharian, K.; Shirkavand, D.; Khoshghalb, A.; Shabani, F.; Sooraki, A.; Rezaie, A. Exploring the Effects of Initial Fabric Anisotropy due to Preshearing Stress History in Different Directions on the Undrained Behavior of Loose Sands. Int. J. Geomech. 2024, 24, 04024201. [Google Scholar] [CrossRef]
- Shabani, F.; Kaviani-Hamedani, F. Cyclic response of sandy subsoil layer under traffic-induced principal stress rotations: Application of bidirectional simple shear apparatus. Soil Dyn. Earthq. Eng. 2023, 164, 107573. [Google Scholar] [CrossRef]
- Bareither, C.A.; Edil, T.B.; Benson, C.H.; Mickelson, D.M. Geological and physical factors affecting the friction angle of compacted sands. J. Geotech. Geoenviron. Eng. 2008, 134, 1476–1489. [Google Scholar] [CrossRef]
- Fakharian, K.; Kaviani-Hamedani, F.; Sooraki, A.; Amindehghan, M.; Lashkari, A. Continuous bidirectional shear moduli monitoring and micro X-ray CT to evaluate fabric evolution under different stress paths. Granul. Matter 2023, 25, 52. [Google Scholar] [CrossRef]
- Moroto, N. A new parameter to measure degree of shear deformation of granular material in triaxial compression tests. Soils Found. 1976, 16, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Simoni, A.; Houlsby, G.T. The direct shear strength and dilatancy of sand–gravel mixtures. Geotech. Geol. Eng. 2006, 24, 523–549. [Google Scholar] [CrossRef]
- Meyerhof, G. Penetration tests and bearing capacity of cohesionless soils. J. Soil Mech. Found. Div. 1956, 82, 866-1–866-19. [Google Scholar] [CrossRef]
- Al-Taie, A.J.; Al-Shakarchi, Y.J.; Mohammed, A.A. Investigation of geotechnical specifications of sand dune soil: A case study around Baiji in Iraq. IIUM Eng. J. 2013, 14. [Google Scholar] [CrossRef]
- George, K.; Uddin, W. Subgrade Characterization for Highway Pavement Design; Department of Transportation, University of Mississippi: Oxford, MS, USA, 2000. [Google Scholar]
- George, V.; Rao, N.C.; Shivashankar, R. PFWD, DCP and CBR correlations for evaluation of lateritic subgrades. Int. J. Pavement Eng. 2009, 10, 189–199. [Google Scholar] [CrossRef]
- Kumar, R.S.; Ajmi, A.S.; Valkati, B. Comparative study of sub grade soil strength estimation models developed based on CBR, DCP and FWD test results. Int. Adv. Res. J. Sci. Eng. Technol. 2015, 2, 92–102. [Google Scholar] [CrossRef]
- Schmertmann, J.; Baker, W.; Gupta, R.; Kessler, K. CPT/DMT QC of ground modification at a power plant. In Proceedings of the Use of In Situ Tests in Geotechnical Engineering, Blacksburg, VA, USA, 23–25 June 1986; pp. 985–1001. [Google Scholar]
- Nikudel, M.R.; Mousavi, S.E.; Khamehchiyan, M.; Jamshidi, A. Using Miniature Cone Penetration Test (Mini-CPT) to determine engineering properties of sandy soils. Geopersia 2012, 2, 65–76. [Google Scholar]
- Trofimenkov, J. Penetration Test in URSS-State-of the Art Report. In Proceedings of the European Symposium on Penetration Testing, Stockholm, Sweden, 5–7 June 1974; pp. 147–154. [Google Scholar]
- Nobahar, M.; Khan, M.S.; Ivoke, J.; Amini, F. Impact of rainfall variation on slope made of expansive Yazoo clay soil in Mississippi. Transp. Infrastruct. Geotechnol. 2019, 6, 318–336. [Google Scholar] [CrossRef]
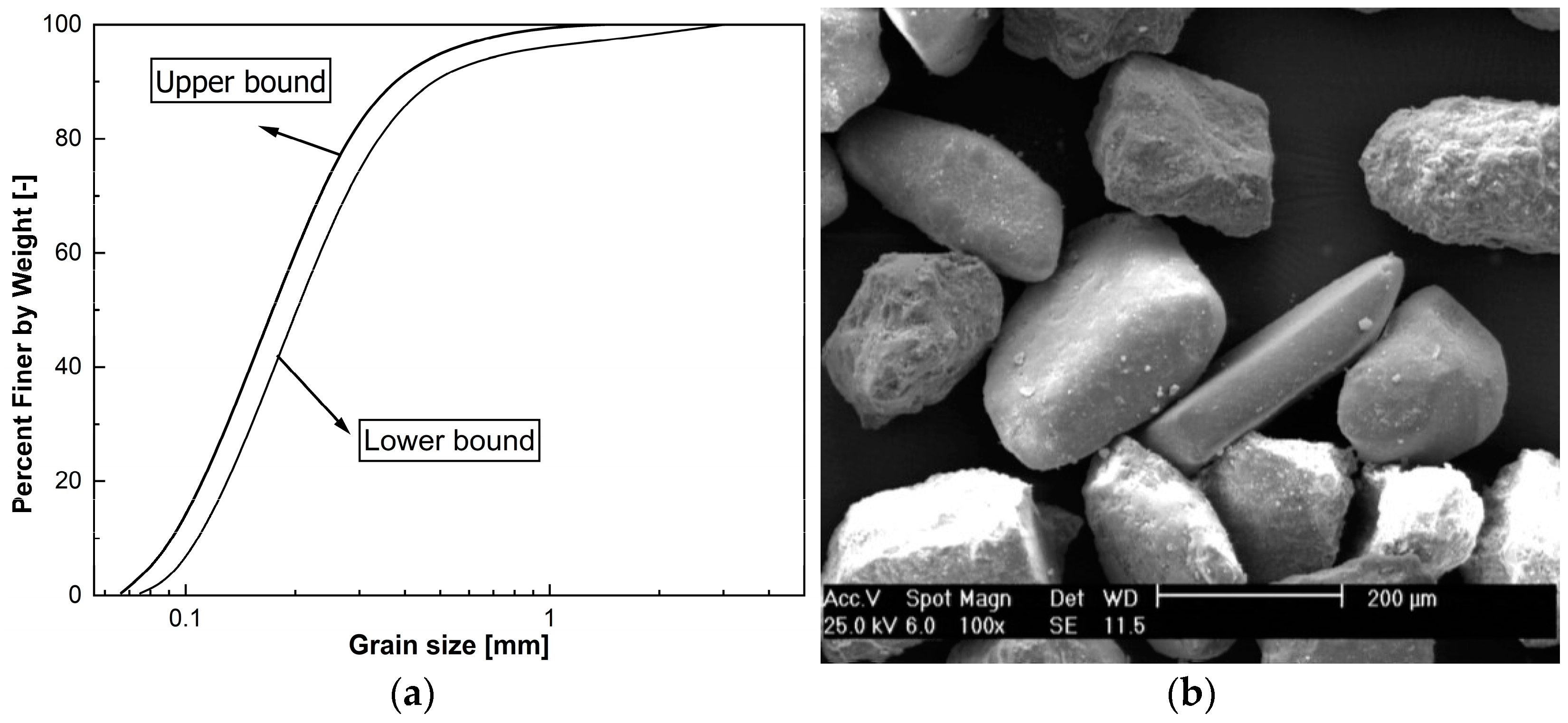



| Parameter | Value | Standard Reference |
|---|---|---|
| 1 | 0.18 | ASTM D6913 [33] |
| 2 | 0.876 | ASTM D4253 [34] |
| 3 | 0.637 | ASTM D4254 [35] |
| 4 | 17.0 | ASTM D4254 [35] |
| 5 | 14.82 | ASTM D4253 [34] |
| 6 | 2.78 | ASTM D854 [36] |
| 7 | 1.22 | ASTM D6913 [33] |
| 8 | 1.67 | ASTM D6913 [33] |
| 9 | 11 | ASTM D698 [37] |
| Sand | Reference | |||
|---|---|---|---|---|
| Several NC sands | 2.91 | 0.700 | 0.050 | [52] |
| Ticino | 2.41 | 0.550 | 0.157 | |
| Hokksund | 3.29 | 0.530 | 0.086 | |
| Ticino | 2.90 | 0.550 | 0.140 | [53] |
| Ticino, Toyoura, Hokksund | 3.10 | 0.500 | 0.175 | |
| Ottawa sand | 2.55 | 0.612 | 0.119 | [54] |
| Reference | Equation |
|---|---|
| [9] | |
| [57] | |
| [58] | |
| [59] | |
| [60] | |
| [61] | |
| [20] |
| No. | Parameters | Equations | Determination Coefficient ) |
|---|---|---|---|
| 1 | 0.96 | ||
| 2 | 0.98 | ||
| 3 | 0.96 | ||
| 4 | 0.95 | ||
| 5 | 0.96 | ||
| 6 | 0.96 | ||
| 7 | 0.95 | ||
| 8 | 0.97 | ||
| 9 | 0.97 | ||
| 10 | 0.92 | ||
| 11 | 0.89 | ||
| 12 | 0.84 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Eslami, A.; Nobahar, M.; Esmailzade, M. Static and Dynamic Cone Penetrometer Tests for Babolsar Sand Parameters via Physical Modeling. Geotechnics 2024, 4, 966-984. https://doi.org/10.3390/geotechnics4030049
Eslami A, Nobahar M, Esmailzade M. Static and Dynamic Cone Penetrometer Tests for Babolsar Sand Parameters via Physical Modeling. Geotechnics. 2024; 4(3):966-984. https://doi.org/10.3390/geotechnics4030049
Chicago/Turabian StyleEslami, Abolfazl, Masoud Nobahar, and Mohammad Esmailzade. 2024. "Static and Dynamic Cone Penetrometer Tests for Babolsar Sand Parameters via Physical Modeling" Geotechnics 4, no. 3: 966-984. https://doi.org/10.3390/geotechnics4030049
APA StyleEslami, A., Nobahar, M., & Esmailzade, M. (2024). Static and Dynamic Cone Penetrometer Tests for Babolsar Sand Parameters via Physical Modeling. Geotechnics, 4(3), 966-984. https://doi.org/10.3390/geotechnics4030049