The Relationship between Electrical Conductivity and Electromigration in Liquid Metals
Abstract
1. Introduction
1.1. The Basics of the Theory of Electromigration
1.2. Calculation of Electrical Resistance of Metal
2. Materials and Methods
2.1. Modification of the Drude–Sommerfeld Equation. Variant N. Mott
2.2. Modification of the Drude–Sommerfeld Equation. Author’s Variant
3. Results
Electromigration Equations
4. Discussing
4.1. Relationship between Effective Charge and Electrical Resistance. Drude–Sommerfeld Variant
4.2. N. Mott Variant. Integral Relations
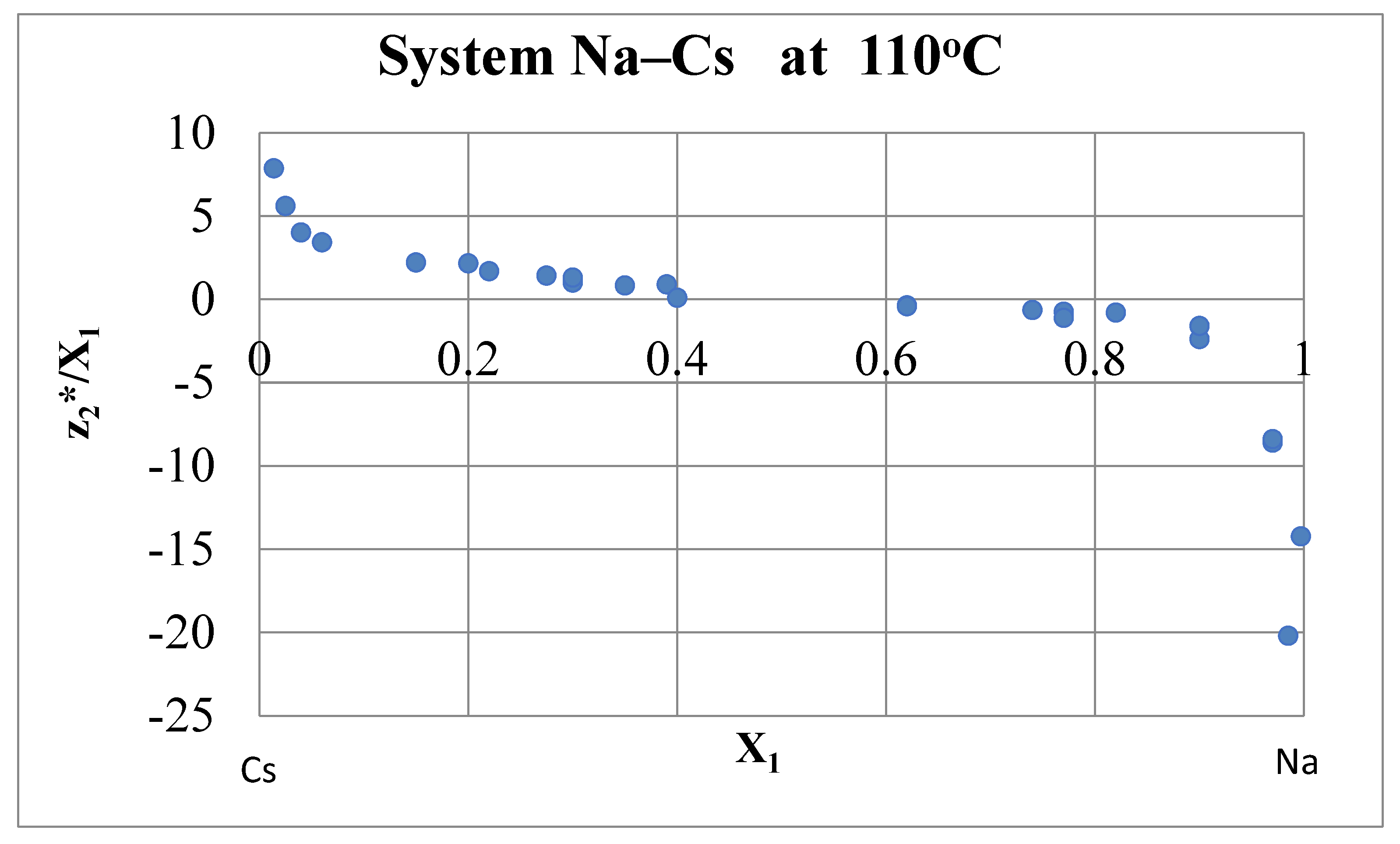
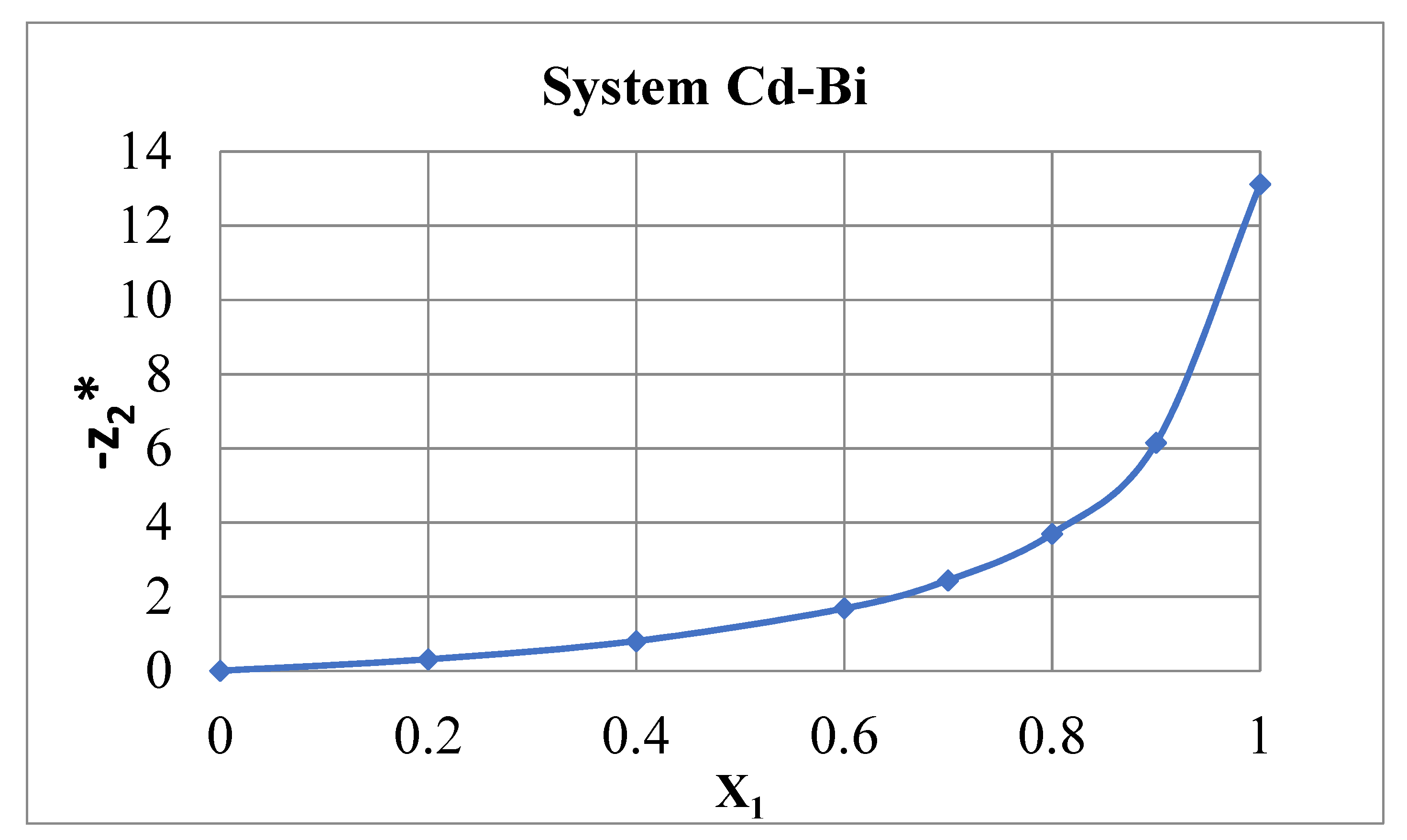
4.3. Consistency Rule
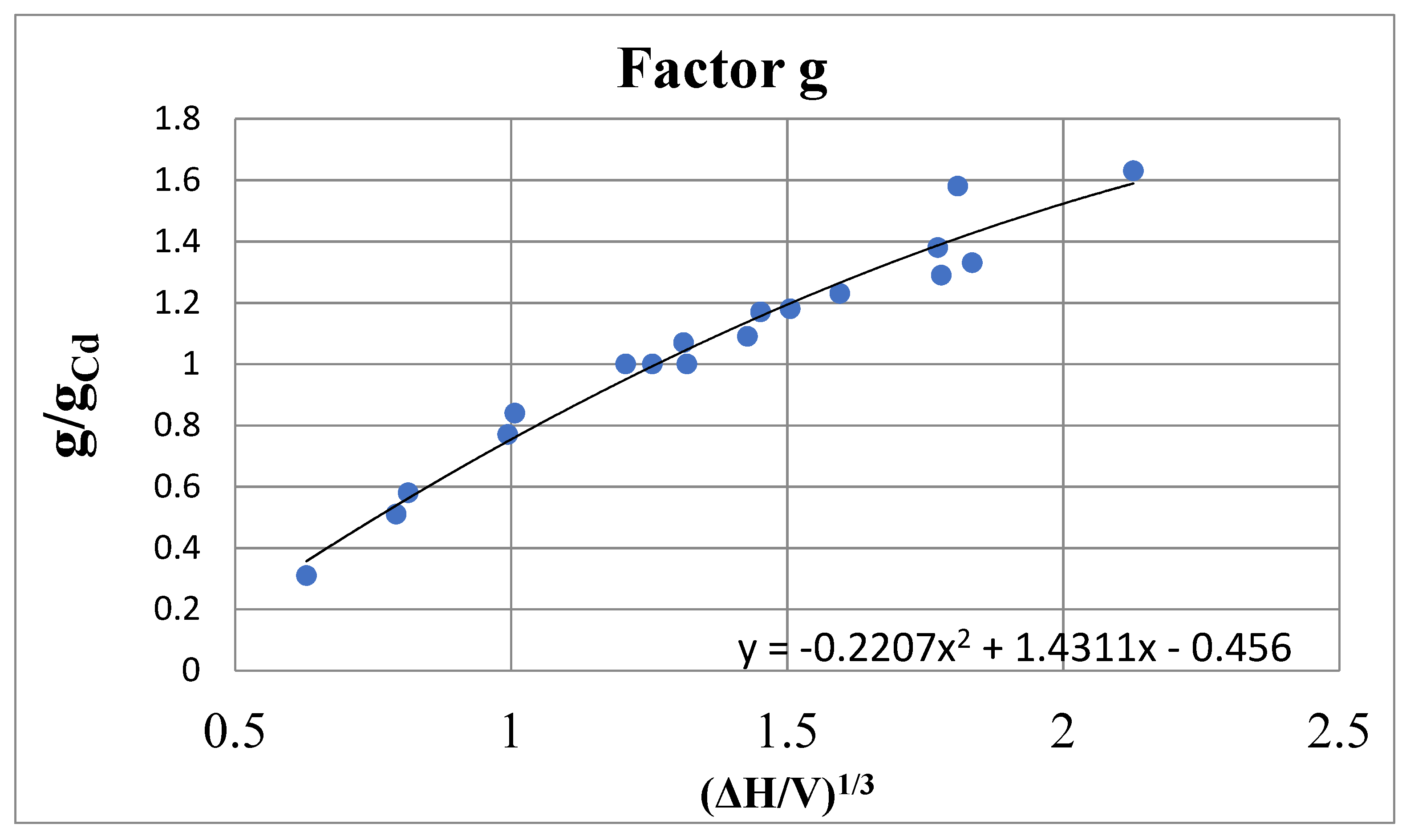
4.4. Calculation of the Factor g
4.5. Alternative Variant of the Author
5. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Schwarz, K.E. Elektrolytische Wanderung in Flüssigen und Festen Metallen; J. A. Barth: Leipzig, Germany, 1940. [Google Scholar]
- Seith, W.; Heumann, T. Diffusion in Metallen; Springer: Berlin/Heidelberg, Germany, 1955. [Google Scholar]
- Fiks, V.B. Ionic Conductivity in Metals and Semiconductors; Nauka: Moscow, Russia, 1969. (In Russian) [Google Scholar]
- Belashchenko, D.K. Transport Phenomena in Liquid Metals and Semiconductors; Atomizdat: Moscow, Russia, 1970. (In Russian) [Google Scholar]
- Kuzmenko, P.P. Electrotransfer, Thermal Transfer and Diffusion in Metals; Vishcha Shkola: Kyiv, Ukraine, 1983. (In Russian) [Google Scholar]
- Belashchenko, D.K. The Study of Liquid Metals by the Electromigration Method; Metallurgy: Moscow, Russia, 1974. (In Russian) [Google Scholar]
- Mikhailov, V.A.; Bogdanova, D.D. Electrotransfer in Liquid Metals; Nauka: Novosibirsk, Russia, 1978. (In Russian) [Google Scholar]
- Ho, P.S.; Kwok, T. Electromigration in metals. Rep. Prog. Phys. 1989, 52, 301–348. [Google Scholar] [CrossRef]
- Sorbello, R. Theory of Electromigration. J. Phys. C Solid State Phys. 1998, 51, 159–231. [Google Scholar]
- Kremann, R.; Müller, R. Elektromotorische Krafte, Elektrolyse and Polarisation, 2 Teil. In Handbuch der Allgemeine Chemie; Akademische Verlagsgesellschaft m.b.H.: Leipzig, Germany, 1931; Volume 8, p. 616. [Google Scholar]
- Huntington, H.B.; Grone, A.R. Current-Induced Marker Motion in Gold Wires. J. Phys. Chem. Solids 1961, 20, 76–87. [Google Scholar] [CrossRef]
- Verhoeven, J. Electrotransport in Metals. Metall. Rev. 1963, 8, 311–368. [Google Scholar] [CrossRef]
- Belashchenko, D.K. Electrotransfer in liquid metals. Adv. Chem. 1965, 34, 530–564. (In Russian) [Google Scholar]
- Black, J.R. Electromigration—A brief survey and some recent results. IEEE Trans. Electron Devices 1969, 16, 338–347. [Google Scholar] [CrossRef]
- Huntington, H.B. Diffusion in Solids: Recent Developments; Nowick, A.S., Burton, J.J., Eds.; Academic: New York, NY, USA, 1975; pp. 303–352. [Google Scholar]
- Tan, C.M.; Roy, A. Electromigration in ULSI interconnects. Mater. Sci. Eng. 2007, 58, 1–75. [Google Scholar] [CrossRef]
- Liu, Y.-C.; Lin, S.-K. A Critical Review on the Electromigration Effect, the Electroplastic Effect, and Perspectives on Effect of Electric Current upon Alloy Phase Stability. JOM 2019, 71, 3094–3106. [Google Scholar] [CrossRef]
- Fiks, V.B. On the mechanism of ion mobility in metals. Sov. Phys.—Solid State 1959, 1, 16–30. (In Russian) [Google Scholar]
- Fiks, V.B.; Kaganov, M.I.; Lifshits, I.M. On the scattering of an electron by an impurity center. Sov. Phys.—Solid State 1964, 6, 2723–2731. (In Russian) [Google Scholar]
- Huntington, H.B. Effect of driving forces on atom motion. Thin Solid Film. 1975, 25, 265–280. [Google Scholar] [CrossRef]
- Sorbello, R. A pseudopotential based theory of the driving forces for electromigration in metals. J. Phys. Chem. Solids 1973, 34, 937–950. [Google Scholar] [CrossRef]
- Sorbello, R.S. Electromigration in liquid metal alloys. Phys. Status Solidi (b) 1978, 86, 671–678. [Google Scholar] [CrossRef]
- Drakin, S.I. Transfer and distribution of metal alloy components in an electric field. Russ. J. Phys. Chem. 1953, 27, 1586–1593. (In Russian) [Google Scholar]
- Bresler, S.E.; Pikus, G.E. On the Theory of the Separation of Isotopes and of Components of Alloys by the Passage of Current Through Liquid Metal. Sov. Phys.—Tech. Phys. 1958, 3, 2094–2100. [Google Scholar]
- Mangelsdorf, P.C. Transport Processes in Liquid Alloys. II. The Electrical Force on an Ion. J. Chem. Phys. 1960, 33, 1151–1161. [Google Scholar] [CrossRef]
- Belashchenko, D.K. On the relationship between electrotransport and electrical conductivity of liquid metallic solutions in the Mott model. Russ. J. Phys. Chem. 1970, 44, 2907–2910. (In Russian) [Google Scholar]
- Fiks, V.B. Dynamic (effective) charge of metal ions. Sov. Phys.—Solid State 1964, 6, 2307. (In Russian) [Google Scholar]
- Ziman, J.M. A theory of the electrical properties of liquid metals. Philos. Mag. 1961, 6, 1013–1034. [Google Scholar] [CrossRef]
- Ziman, J.M. Electrons and Phonons. The Theory of Transport Phenomena in Solids; Oxford University Press: Oxford, UK, 1960. [Google Scholar]
- Mott, N.F. Electrons in Disordered Structures. Adv. Phys. 1967, 16, 49–144. [Google Scholar] [CrossRef]
- Mott, N.F. Conduction in non-crystalline materials. III Philos. Mag. 1969, 19, 835–852. [Google Scholar] [CrossRef]
- Kubo, R. A General expression for the conductivity tensor. J. Phys. 1956, 34, 1274–1277. [Google Scholar] [CrossRef]
- Greenwood, D.A. The Boltzmann Equation in the Theory of Electrical Conduction in Metals. Proc. Phys. Soc. Lond. 1958, 71, 585–596. [Google Scholar] [CrossRef]
- Faber, T.E. Optical Properties and Electronic Structure of Metals and Alloys. In Proceedings of the International Colliquium, Paris, France, 13–16 September 1965; North-Holland Publishing: Amsterdam, The Netherlands, 1966. [Google Scholar]
- Kharkov, E.I. Relation between the parameters of electrotransfer and electrical resistance of liquid binary alloys. Ukr. Phys. J. 1966, 11, 677–678. (In Russian) [Google Scholar]
- Shaw, R.E. Convection Effects during Electrotransport of Liquid Metals. Ph.D. Thesis, Iowa State University, Ames, IA, USA, 1972. (In Russian). [Google Scholar]
- Belashchenko, D.K. Electromigration in liquid binary alloys and its connection with electrical resistance. Izv. Vuzov Ferr. Metall. 1962, 120–125. (In Russian) [Google Scholar]
- Belashchenko, D.K.; Gushchina, E.I. Application of the electrotransport method for the analysis of electronic states in liquid metallic solutions based on the one-parameter Mott model. Met. Phys. Metallogr. 1970, 30, 295–302. (In Russian) [Google Scholar]
- Drakin, S.I.; Maltsev, A.K. Electrodiffusion in K-Na alloy. Russ. J. Phys. Chem. 1957, 31, 2036–2041. (In Russian) [Google Scholar]
- Kremann, R.; Bauer, F.; Vogrin, A.; Scheibel, H. Uber den Wechsel im Wanderungssinn der Alkali- und anderer Metalle bei der Elektrolyse der betreffenden Amalgame in Abhangigkeit von der Konzentration. Monatsh. Chem. 1930, 56, 35–65. [Google Scholar] [CrossRef]
- Rudenko, A.G.; Golovinsky, N.P.; Kharkov, E.I. Electrotransfer in the Cd-Zn system. Met. Phys. Metallogr. 1968, 25, 560–562. (In Russian) [Google Scholar]
- Zhmudsky, A.; Kharkov, E.I.; Rudenko, A.G. Double inversion of electrotransport in the Al-Zn system in the liquid state. Met. Phys. Metallogr. 1967, 23, 559–562. (In Russian) [Google Scholar]
- Aksenova, L.I.; Belashchenko, D.K. Electrotransport, electrical resistance and density of electronic states in melts of the Na-K system. High Temp. 1971, 9, 722–730. (In Russian) [Google Scholar]
- Larson, S.; Roxberg, C.; Lodding, A. Atomic Transport in Solids and Liquids, Proceedings of the Europhysics Conference, Marstrand, Sweden, 15–19 June 1970; Lodding, A., Lagerwall., T., Eds.; Verlag der Zeitschrift für Naturforschung: Tübingen, Germany, 1971; ISBN 3921015006/9783921015001. [Google Scholar]
- Aksenova, L.I.; Belashchenko, D.K.; Pertsin, A.I. Electrotransfer, electrical resistance and density of electronic states in Cs—K and Na—Cs melts. High Temp. 1971, 9, 1159–1167. (In Russian) [Google Scholar]





| Pairs of Metals | σ2/σ1 Using (14) | ρ1/ρ2 Using (5) | ρ1/ρ2, Experim. |
|---|---|---|---|
| Sn–Cd | 0.30 | 1.96 | 1.48 |
| Bi–Cd | 0.13 | 3.61 | 3.91 |
| Pb–Cd | 0.23 | 2.46 | 3.06 |
| Bi–Pb | 0.64 | 1.30 | 1.28 |
| Pb–Sn | 0.81 | 1.18 | 1.89 |
| Bi–Sn | 0.46 | 1.75 | 2.63 |
| Impurity | Δρ/c, μΩ·cm/at.% | Δρ/X2ρ1 | V2, cm3/mol | |
|---|---|---|---|---|
| Li | −2.0 | −1.67 | 13.48 | 0.16 |
| Na | 0.7 | 0.58 | 24.8 | −0.22 |
| К | 2.9 | 2.42 | 47.9 | −0.66 |
| Cs | - | - | 11.7 | −2.43 |
| Ag | −3.7 | −3.08 | 29.08 | 0.72 |
| Au | - | - | 9.52 | 0.73 |
| Mg | - | - | 14 | 0.5 |
| Ca | −3.51 | 2.92 | 14 | <0.05 |
| Zn | −4.4 | −3.67 | 18.1 | 0.62 |
| Cd | −3.7 | −3.08 | 19.44 | 0.62 |
| Cd | - | - | 20.9 | 0.83 [36] |
| Tl | −2.1 | −1.75 | 17.3 | 0.0 |
| Pb | −5.8 | −4.83 | 11.8 | 0.0 |
| Bi | −3.7 | −3.08 | 13.48 | −0.69 |
| Sn | −6.5 | −5.42 | 24.8 | 0.33 |
| Triangle | A |
|---|---|
| Cd–Bi–Pb | 1.057 ± 0.04 |
| Sn–Bi–Pb | 1.077 ± 0.04 |
| Cd–Sn–Bi | 0.99 ± 0.04 |
| Ag–Cu–Sn | 0.98 ± 0.03 |
| Ag–Cu–Ge | 1.03 ± 0.03 |
| K–Na–Cs | 0.97 ± 0.12 |
| everywhere | 0.995 |
| in Tl, 5 in Cd and In, linearly depends on the concentration | 1.043 |
| in Tl, 3 in Cd and In, linearly depends on the concentration | 1.053 |
| everywhere | 1.092 |
| in Tl, 1 in Cd and In, linearly depends on the concentration | 0.927 |
| everywhere | 0.930 |
| everywhere | 0.991 ± 0.03 |
| Metal | T, °C | g/gCd [6] | b/bCd | ΔHsubl, kcal/mol | Vmol, cm3/mol | ΔHsubl/V |
|---|---|---|---|---|---|---|
| Cs | 110 | 0.31 | (10.41) | 17.97 | 72.2 | 0.249 |
| K | 110 | 0.51 | (3.84) | 20.95 | 42.35 | 0.496 |
| Te | 500 | 0.58 | (2.97) | 11.9 | 22.15 | 0.538 |
| Hg | 300 | 0.77 | 1.69 | 14.54 | 14.67 | 0.981 |
| Na | 250 | 0.84 | 1.42 | 25.0 | 24.50 | 1.02 |
| Cd | 300–500 | 1.00 | 1.00 | 24.54 | 14.07 | 1.76 |
| Bi | 300–500 | 1.00 | 1.00 | 41.05 | 20.8 | 1.98 |
| Pb | 300–500 | 1.00 | 1.00 | 45.44 | 19.08 | 2.29 |
| Tl | 350 | 1.07 | 0.87 | 40.8 | 18.08 | 2.26 |
| Zn | 500 | 1.09 | 0.84 | 28.56 | 9.82 | 2.91 |
| Sb | (500) | 1.17 | 0.73 | 57.5 | 18.8 | 3.06 |
| In | 350 | 1.18 | 0.72 | 55.56 | 16.32 | 3.41 |
| Sn | 300–500 | 1.23 | 0.66 | 68.77 | 16.96 | 4.06 |
| Ga | 300 | 1.29 | 0.60 | 64.4 | 11.44 | 5.63 |
| Al | 580 | 1.33 | 0.57 | 67.8 | 10.96 | 6.18 |
| Ag | 1100 | 1.38 | 0.53 | 63.3 | 11.36 | 5.57 |
| Ge | 1100 | 1.58 | 0.40 | 78.2 | 13.2 | 5.92 |
| Cu | 1100 | 1.63 | 0.38 | 72.8 | 7.57 | 9.62 |
| Li | - | 1.20 | 0.69 | 32.2 | 13.72 | 2.35 |
| Rb | - | 0.42 | 5.67 | 18.11 | 57.9 | 0.313 |
| Au | - | 1.33 | 0.57 | 84.6 | 16.8 | 5.04 |
| Be | - | 2.21 | 0.20 | 74.1 | 5.3 | 14.0 |
| Mg | - | 0.99 | 1.02 | 31.5 | 15.33 | 2.05 |
| Ca | - | 0.88 | 1.29 | 38.6 | 27.4 | 1.41 |
| Ba | - | 0.76 | 1.73 | 35.7 | 38.7 | 0.92 |
| Si | - | 1.45 | 0.48 | 71 | 11.2 | 6.44 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Belashchenko, D.K. The Relationship between Electrical Conductivity and Electromigration in Liquid Metals. Dynamics 2023, 3, 405-424. https://doi.org/10.3390/dynamics3030022
Belashchenko DK. The Relationship between Electrical Conductivity and Electromigration in Liquid Metals. Dynamics. 2023; 3(3):405-424. https://doi.org/10.3390/dynamics3030022
Chicago/Turabian StyleBelashchenko, David K. 2023. "The Relationship between Electrical Conductivity and Electromigration in Liquid Metals" Dynamics 3, no. 3: 405-424. https://doi.org/10.3390/dynamics3030022
APA StyleBelashchenko, D. K. (2023). The Relationship between Electrical Conductivity and Electromigration in Liquid Metals. Dynamics, 3(3), 405-424. https://doi.org/10.3390/dynamics3030022