Modeling of Multiple Fatigue Cracks for the Aircraft Wing Corner Box Based on Non-ordinary State-based Peridynamics
Abstract
:1. Introduction
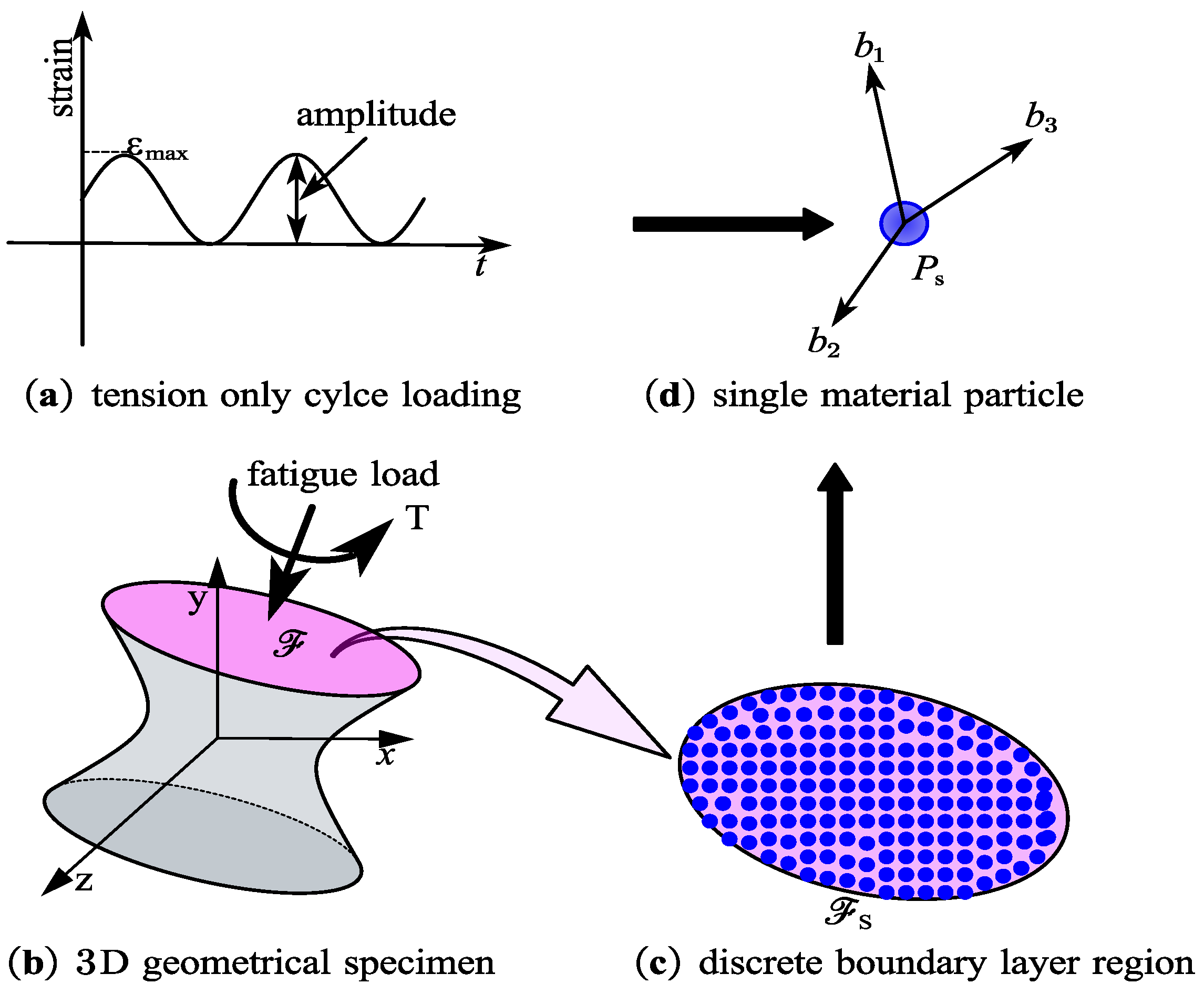
2. Progressive Fatigue Damage Evolution
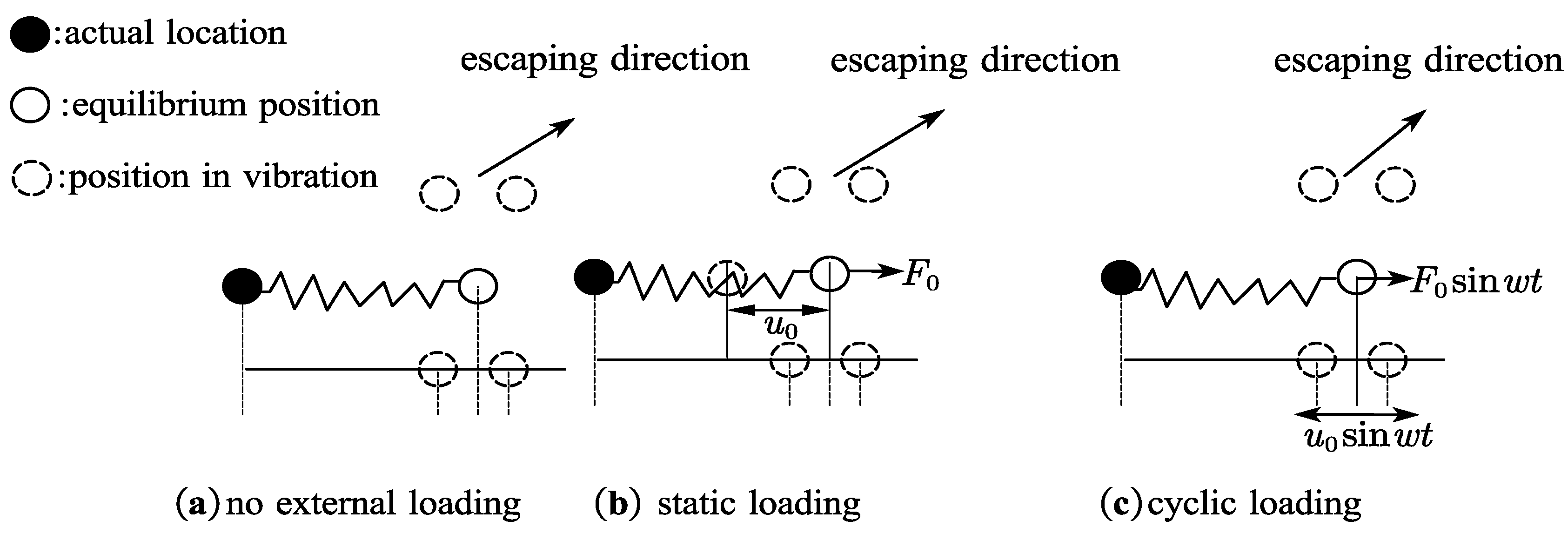
2.1. Microscopic Fatigue Crack Initiation and Propagation
2.2. Widespread Fatigue Damage
2.3. Fatigue Damage Accumulation for Multiple Cracks
3. State-Based Peridynamics for Multiple Cracks
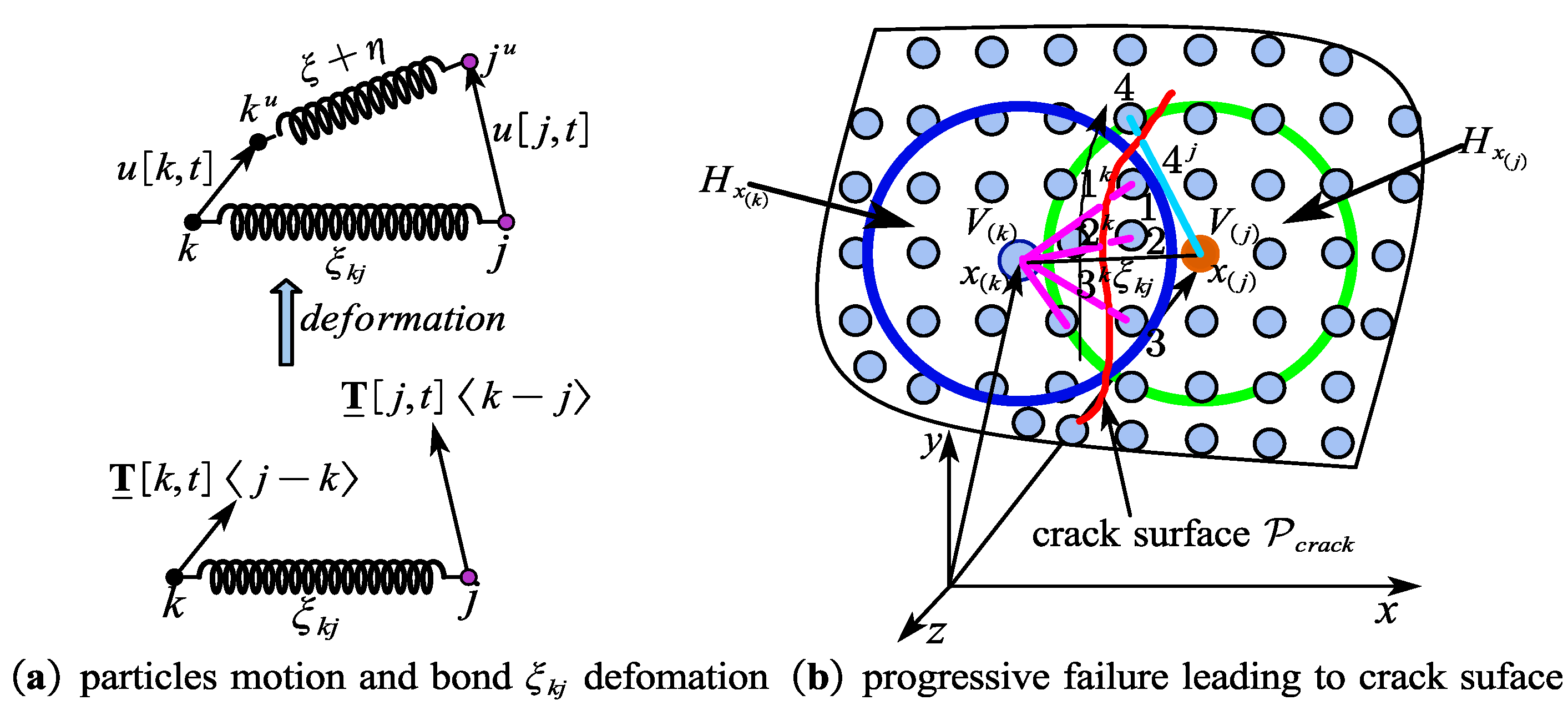
3.1. The Motion and Deformation of a Material Point
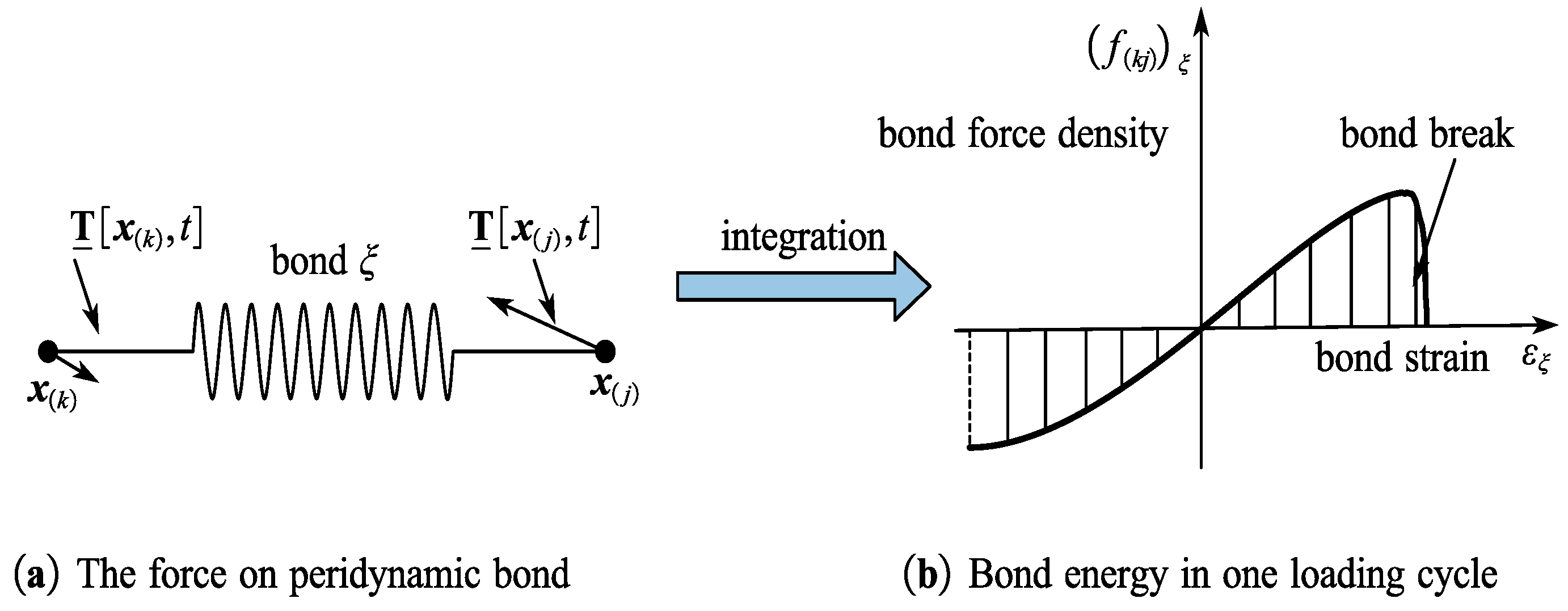
3.2. Energy-Based Failure Model
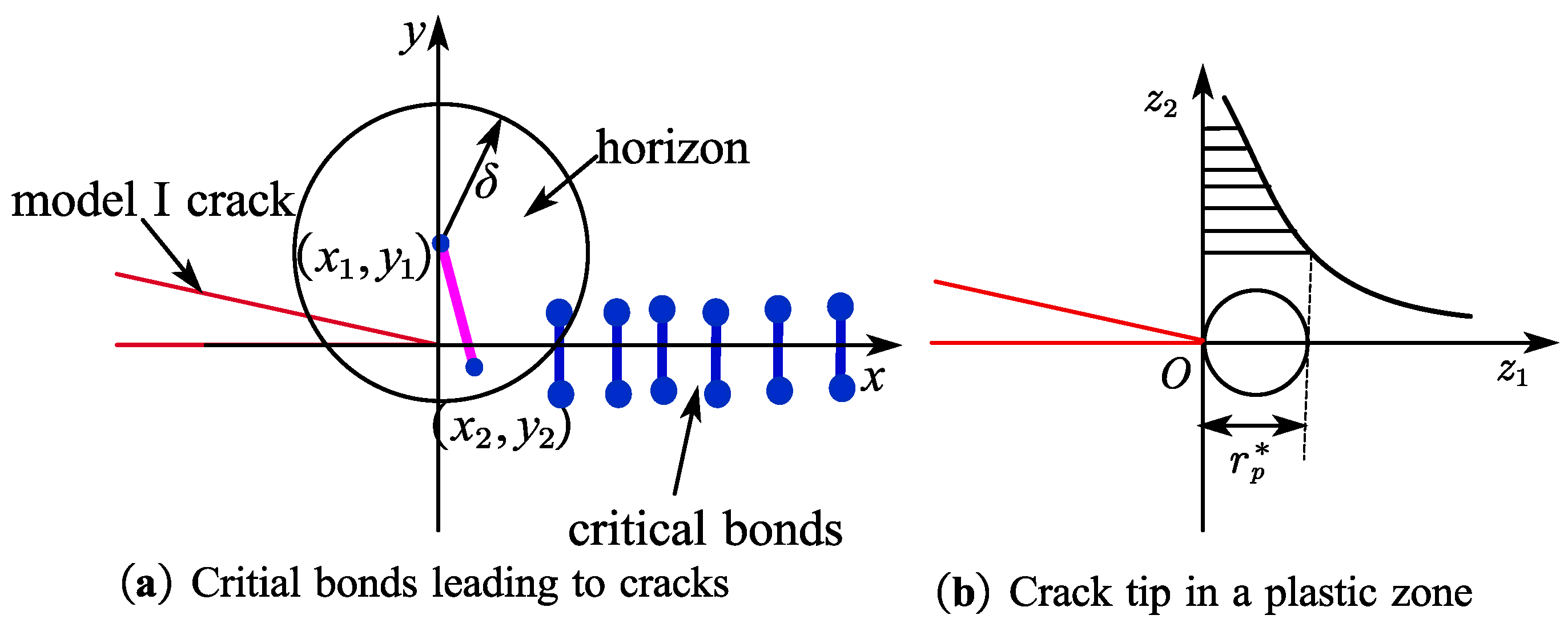
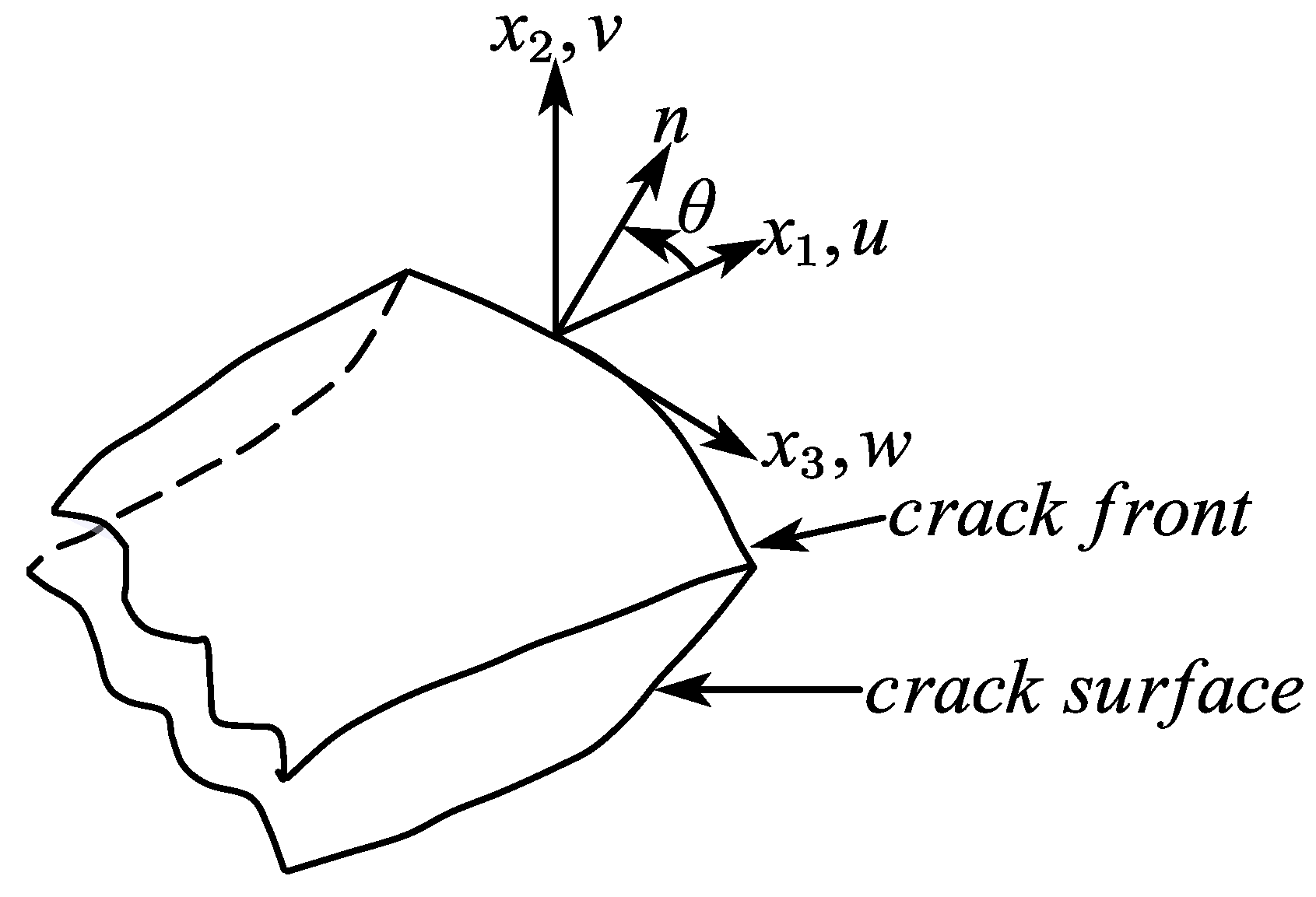
3.3. Fatigue Crack Tip Analysis in the Peridynamic Solid Body
4. Fatigue Model Based on Non-Ordinary State-Based Peridynamics
4.1. Progressive Failure in the Non-Ordinary State-Based Peridynamic Body
4.2. Multiscale Fatigue Model for Multi-Crack Process
4.3. Criterion of Fatigue Multi-Crack Initiation to Propagation
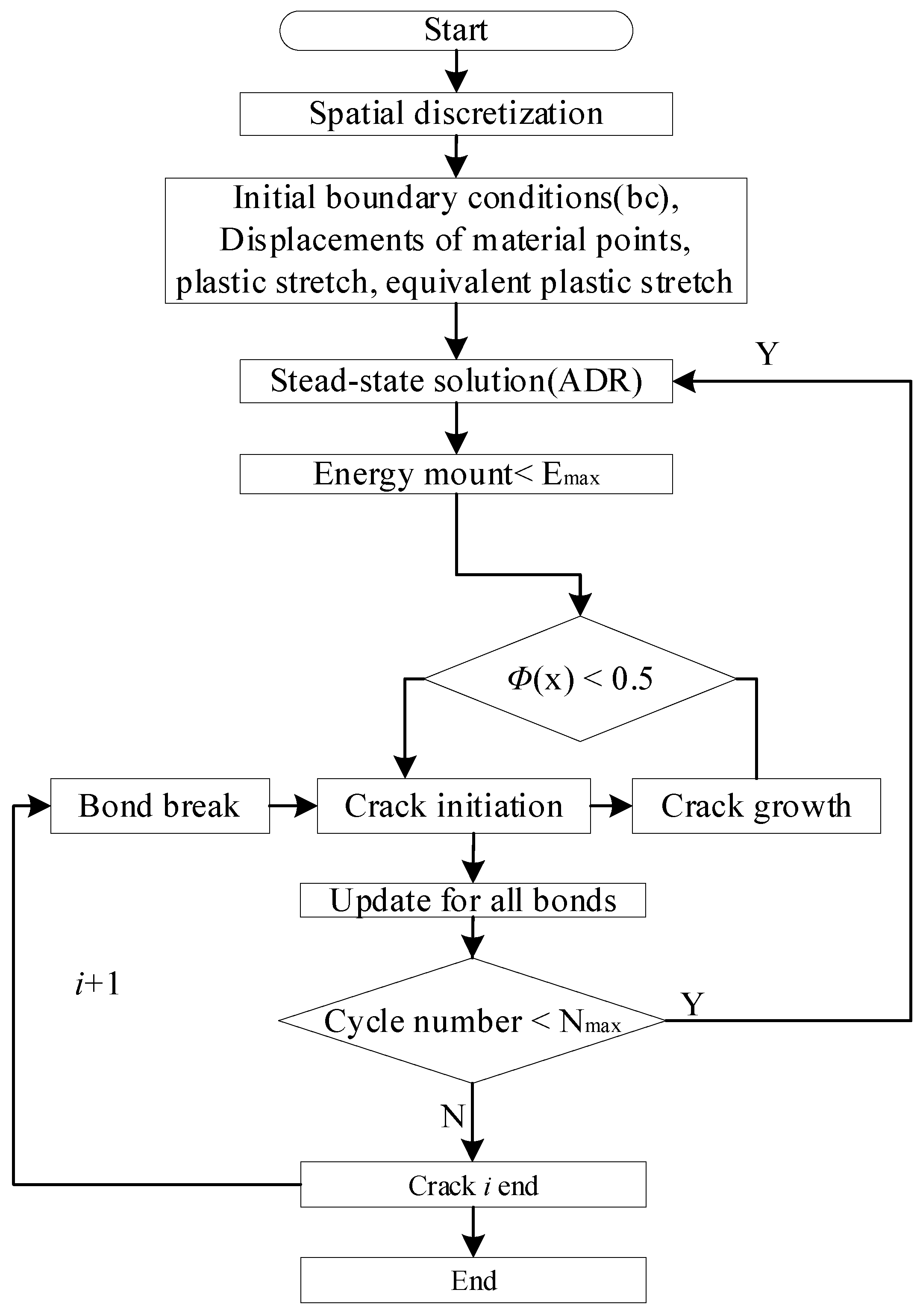
5. Validation Procedure of Numerical Solution Method
5.1. Quasi-Static Solution for Each Cyclic Loading
5.2. Equivalent Fatigue Parameters between PD and EPFM
5.3. The Whole Process of Simulating Fatigue Multi-Fatigue Crack Initiation and Propagation
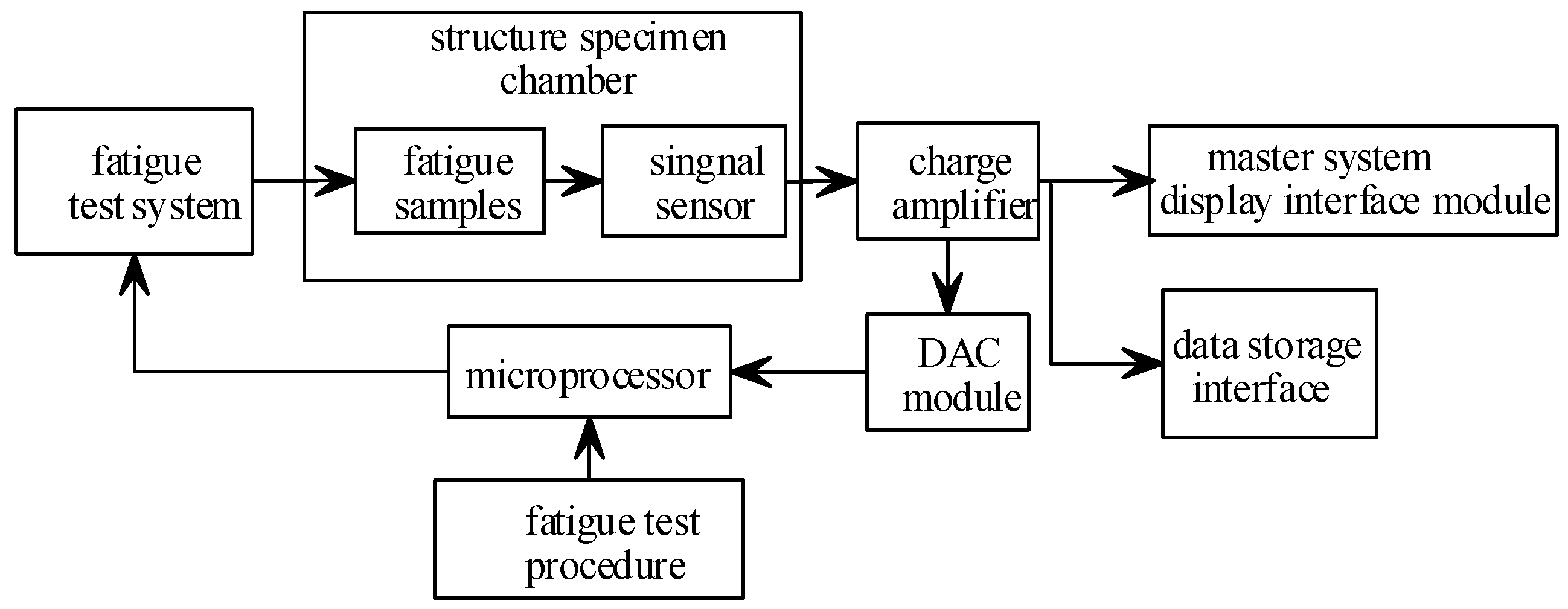
6. Fatigue Experiment
6.1. Structural Fatigue Testing Platform with Overalls Tools
6.2. Sample Dimension Description and Input Parameters
6.3. Crack Morphology and Data Recording
6.4. Data Analysis and Countermeasures
7. Conclusions
- (1)
- To evaluate fatigue crack life of the aircraft wing corner box under tension–tension fatigue loading, a novel NOSBPD fatigue model for multiple-crack nucleation and propagation was deduced. The multiple cracks nucleate and propagate autonomously with this constitutive model for fatigue.
- (2)
- The proposed non-ordinary state-based peridynamics damage model shows no scale constraints and the whole multiple crack propagation process can be applied to the model. Therefore, the NOSBPD fatigue model successfully addressed cross-scale issues during the multiple-crack lifetime.
- (3)
- Two of the cracks, crack 1 and crack 2, converged and grew into principal cracks according to the time sequence. The numerical calculation results from the proposed model agree well with the experimental results. It is more accurate and effective at reproducing the multiple-crack characteristics, such as spatial warping and multiple original positions, than classical fatigue models according to our comparison.
- (4)
- The natural nucleation and propagation of multiple cracks are obtained with no extra rules to guide the crack propagation. Quantitative analysis of the fatigue damage is obtained. The assessment of three-dimensional multi-crack nucleation for fatigue life prediction is confirmed with the NOSBPD model.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pook, L. Why Metal Fatigue Matters; Springer: Dordrecht, The Netherlands, 2007. [Google Scholar]
- Klesnil, M.; Lukác, P. Fatigue of Metallic Materials; Elsevier: Amsterdam, The Netherlands, 1992. [Google Scholar]
- Verma, A.R. Crystal Growth and Dislocations; Butterworth’s Scientific Publications: Oxford, UK, 1953. [Google Scholar]
- Álvarez, G.; Zafra, A.; Belzunce, F.J.; Rodríguez, C. Effect of Internal Hydrogen on the Fatigue Crack Growth Rate in the Coarse-Grain Heat-Affected Zone of a CrMo Steel. Metals 2022, 12, 673. [Google Scholar] [CrossRef]
- Randić, M.; Pavletić, D.; Potkonjak, Ž. The Influence of Heat Input on the Formation of Fatigue Cracks for High-Strength Steels Resistant to Low Temperatures. Metals 2022, 12, 929. [Google Scholar] [CrossRef]
- Bressot, C.; Shandilya, N.; Nogueira, E.; Cavaco-Paulo, A.; Morgeneyer, M.; Le Bihan, O.; Aguerre-Chariol, O. Exposure assessment based recommendations to improve nanosafety at nanoliposome production sites. J. Nanomater. 2015, 2015, 931405. [Google Scholar] [CrossRef] [Green Version]
- Warheit, D.B. Hazard and risk assessment strategies for nanoparticle exposures: How far have we come in the past 10 years? F1000Research 2018, 7, 376. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pineau, A.; Benzerga, A.A.; Pardoen, T. Failure of metals III: Fracture and fatigue of nanostructured metallic materials. Acta Mater. 2016, 107, 508–544. [Google Scholar] [CrossRef] [Green Version]
- Forrest, P.G. Fatigue of Metals; Elsevier: Amsterdam, The Netherlands, 2013. [Google Scholar]
- Stephens, R.I.; Fatemi, A.; Stephens, R.R.; Fuchs, H.O. Metal Fatigue in Engineering; John Wiley & Sons: New York, NY, USA, 2000. [Google Scholar]
- Man, J.; Obrtlik, K.; Blochwitz, C.H.; Polak, J. Atomic force microscopy of surface relief in individual grains of fatigued 316L austenitic stainless steel. Acta Mater. 2002, 50, 3767–3780. [Google Scholar] [CrossRef]
- Alfyorova, E.; Lychagin, D. Self-organization of plastic deformation and deformation relief in FCC single crystals. Mech. Mater. 2018, 117, 202–213. [Google Scholar] [CrossRef]
- Heczko, M.; Polák, J.; Kruml, T. Microstructure and dislocation arrangements in Sanicro 25 steel fatigued at ambient and elevated temperatures. Mater. Sci. Eng. A 2017, 680, 168–181. [Google Scholar] [CrossRef]
- Makin, M. Electron displacement damage in copper and aluminium in a high voltage electron microscope. Philos. Mag. 1968, 18, 637–653. [Google Scholar] [CrossRef]
- Kelly, A.; Knowles, K.M. Crystallography and Crystal Defects; John Wiley & Sons: New York, NY, USA, 2020. [Google Scholar]
- Nagase, T.; Umakoshi, Y. Electron irradiation induced crystallization of the amorphous phase in Zr-Cu based metallic glasses with various thermal stability. Mater. Trans. 2004, 45, 13–23. [Google Scholar] [CrossRef] [Green Version]
- Oskay, C.; Fish, J. Fatigue life prediction using 2-scale temporal asymptotic homogenization. Int. J. Numer. Methods Eng. 2004, 61, 329–359. [Google Scholar] [CrossRef]
- Fish, J. Multiscale modeling and simulation of composite materials and structures. In Multiscale Methods in Computational Mechanics; Springer: Berlin/Heidelberg, Germany, 2011; pp. 215–231. [Google Scholar]
- Ortolano González, J.M.; Hernández Ortega, J.A.; Oliver Olivella, X. A Comparative Study on Homogenization Strategies for Multi-scale Analysis of Materials; Centre Internacional de Mètodes Numèrics en Enginyeria (CIMNE): Barcelona, Spain, 2013. [Google Scholar]
- Yuan, Z.; Fish, J. Toward realization of computational homogenization in practice. Int. J. Numer. Methods Eng. 2008, 73, 361–380. [Google Scholar] [CrossRef]
- Guidault, P.A.; Allix, O.; Champaney, L.; Navarro, J. A two-scale approach with homogenization for the computation of cracked structures. Comput. Struct. 2007, 85, 1360–1371. [Google Scholar] [CrossRef]
- Schijve, J. Fatigue damage in aircraft structures, not wanted, but tolerated? Int. J. Fatigue 2009, 31, 998–1011. [Google Scholar] [CrossRef]
- Murakami, Y.; Miller, K. What is fatigue damage? A view point from the observation of low cycle fatigue process. Int. J. Fatigue 2005, 27, 991–1005. [Google Scholar] [CrossRef]
- Schijve, J. Fatigue of Structures and Materials; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Jung, J.; Seok, J. Fatigue crack growth analysis in layered heterogeneous material systems using peridynamic approach. Compos. Struct. 2016, 152, 403–407. [Google Scholar] [CrossRef]
- Jung, J.; Seok, J. Mixed-mode fatigue crack growth analysis using peridynamic approach. Int. J. Fatigue 2017, 103, 591–603. [Google Scholar] [CrossRef]
- Ondráček, J.; Materna, A. FEM evaluation of the dissipated energy in front of a crack tip under 2D mixed mode loading condition. Procedia Mater. Sci. 2014, 3, 673–678. [Google Scholar] [CrossRef] [Green Version]
- Horníková, J.; Šandera, P.; Žák, S.; Pokluda, J. Specimens for simultaneous mode II, III and II+ III fatigue crack propagation: Elasto-plastic solution of crack tip stress-strain field. Adv. Mater. Res. 2014, 891, 1585–1590. [Google Scholar]
- Shlyannikov, V.; Tumanov, A.; Boychenko, N. Surface crack growth rate under tension and bending in aluminum alloys and steel. Procedia Eng. 2016, 160, 5–12. [Google Scholar] [CrossRef] [Green Version]
- Solanki, K.; Daniewicz, S.; Newman, J., Jr. Finite element analysis of plasticity-induced fatigue crack closure: An overview. Eng. Fract. Mech. 2004, 71, 149–171. [Google Scholar] [CrossRef]
- Antunes, F.; Rodrigues, D. Numerical simulation of plasticity induced crack closure: Identification and discussion of parameters. Eng. Fract. Mech. 2008, 75, 3101–3120. [Google Scholar] [CrossRef] [Green Version]
- Jiang, Y.; Feng, M.; Ding, F. A reexamination of plasticity-induced crack closure in fatigue crack propagation. Int. J. Plast. 2005, 21, 1720–1740. [Google Scholar] [CrossRef]
- Dunne, F. Fatigue crack nucleation: Mechanistic modelling across the length scales. Curr. Opin. Solid State Mater. Sci. 2014, 18, 170–179. [Google Scholar] [CrossRef] [Green Version]
- Espinosa, H.D.; Zavattieri, P.D. A grain level model for the study of failure initiation and evolution in polycrystalline brittle materials. Part I: Theory and numerical implementation. Mech. Mater. 2003, 35, 333–364. [Google Scholar] [CrossRef]
- Raghami, E.; Schrank, C.; Kruhl, J.H. 3D modelling of the effect of thermal-elastic stress on grain-boundary opening in quartz grain aggregates. Tectonophysics 2020, 774, 228242. [Google Scholar] [CrossRef]
- Simkin, B.; Crimp, M.; Bieler, T. A factor to predict microcrack nucleation at γ–γ grain boundaries in TiAl. Scr. Mater. 2003, 49, 149–154. [Google Scholar] [CrossRef]
- Wei, B.; Liu, Z.; Nong, B.; Cao, B.; Lv, X.; Ren, Y.; Ai, Y. Microstructure, cracking behavior and mechanical properties of René 104 superalloy fabricated by selective laser melting. J. Alloys Compd. 2021, 867, 158377. [Google Scholar] [CrossRef]
- Dunne, F.; Wilkinson, A.; Allen, R. Experimental and computational studies of low cycle fatigue crack nucleation in a polycrystal. Int. J. Plast. 2007, 23, 273–295. [Google Scholar] [CrossRef]
- Mcdowell, D.; Dunne, F. Microstructure-sensitive computational modeling of fatigue crack formation. Int. J. Fatigue 2010, 32, 1521–1542. [Google Scholar] [CrossRef]
- Mcdowell, D.L. Simulation-based strategies for microstructure-sensitive fatigue modeling. Mater. Sci. Eng. A 2007, 468, 4–14. [Google Scholar] [CrossRef]
- Shenoy, M.; Tjiptowidjojo, Y.; Mcdowell, D. Microstructure-sensitive modeling of polycrystalline IN 100. Int. J. Plast. 2008, 24, 1694–1730. [Google Scholar] [CrossRef]
- Tinga, T.; Brekelmans, W.; Geers, M. Time-incremental creep–fatigue damage rule for single crystal Ni-base superalloys. Mater. Sci. Eng. A 2009, 508, 200–208. [Google Scholar] [CrossRef]
- Tschopp, M.; Tucker, G.; Mcdowell, D. Atomistic simulations of tension–compression asymmetry in dislocation nucleation for copper grain boundaries. Comput. Mater. Sci. 2008, 44, 351–362. [Google Scholar] [CrossRef]
- Madenci, E.; Oterkus, E. Peridynamic Theory and Its Applications; Springer: Berlin/Heidelberg, Germany, 2014; pp. 19–43. [Google Scholar]
- Das, S.; Hoffarth, C.; Ren, B.; Spencer, B.; Sant, G.; Rajan, S.D.; Neithalath, N. Simulating the fracture of notched mortar beams through extended finite-element method and peridynamics. J. Eng. Mech. 2019, 145, 04019049. [Google Scholar] [CrossRef] [Green Version]
- Silling, S.A.; Lehoucq, R.B. Peridynamic theory of solid mechanics. Adv. Appl. Mech. 2010, 44, 73–168. [Google Scholar]
- Silling, S.A.; Epton, M.; Weckner, O.; Xu, J.; Askari, E. Peridynamic states and constitutive modeling. J. Elast. 2007, 88, 151–184. [Google Scholar] [CrossRef] [Green Version]
- Silling, S.A.; Askari, E. A meshfree method based on the peridynamic model of solid mechanics. Comput. Struct. 2005, 83, 1526–1535. [Google Scholar] [CrossRef]
- Chen, L.Y.; Richter, G.; Sullivan, J.P.; Gianola, D.S. Lattice anharmonicity in defect-free Pd nanowhiskers. Phys. Rev. Lett. 2012, 109, 125503. [Google Scholar] [CrossRef] [Green Version]
- Wei, X.; Naraghi, M.; Espinosa, H.D. Optimal length scales emerging from shear load transfer in natural materials: Application to carbon-based nanocomposite design. ACS Nano 2012, 6, 2333–2344. [Google Scholar] [CrossRef]
- Rahman, R.; Foster, J.T.; Haque, A. A multiscale modeling scheme based on peridynamic theory. Int. J. Multiscale Comput. Eng. 2014, 12, 223–248. [Google Scholar] [CrossRef]
- Oterkus, E.; Guven, I.; Madenci, E. Fatigue failure model with peridynamic theory. In Proceedings of the 2010 12th IEEE Intersociety Conference on Thermal and Thermomechanical Phenomena in Electronic Systems, Las Vegas, NV, USA, 2–5 June 2010; pp. 1–6. [Google Scholar]
- Silling, S.A.; Askari, A. Peridynamic Model for Fatigue Cracking; SAND2014-18590; Sandia National Laboratories: Albuquerque, NM, USA, 2014. [Google Scholar]
- Zhang, G.; Le, Q.; Loghin, A.; Subramaniyan, A.; Bobaru, F. Validation of a peridynamic model for fatigue cracking. Eng. Fract. Mech. 2016, 162, 76–94. [Google Scholar] [CrossRef]
- Hu, Y.; Madenci, E. Peridynamics for fatigue life and residual strength prediction of composite laminates. Compos. Struct. 2017, 160, 169–184. [Google Scholar] [CrossRef]
- Baber, F.; Guven, I. Solder joint fatigue life prediction using peridynamic approach. Microelectron. Reliab. 2017, 79, 20–31. [Google Scholar] [CrossRef]
- Basoglu, M.F.; Zerin, Z.; Kefal, A.; Oterkus, E. A computational model of peridynamic theory for deflecting behavior of crack propagation with micro-cracks. Comput. Mater. Sci. 2019, 162, 33–46. [Google Scholar] [CrossRef] [Green Version]
- Zhu, N.; De Meo, D.; Oterkus, E. Modelling of granular fracture in polycrystalline materials using ordinary state-based peridynamics. Materials 2016, 9, 977. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Silling, S.A. Reformulation of elasticity theory for discontinuities and long-range forces. J. Mech. Phys. Solids 2000, 48, 175–209. [Google Scholar] [CrossRef] [Green Version]
- Han, J.; Chen, W. An ordinary state-based peridynamic model for fatigue cracking of ferrite and pearlite wheel material. Appl. Sci. 2020, 10, 4325. [Google Scholar] [CrossRef]











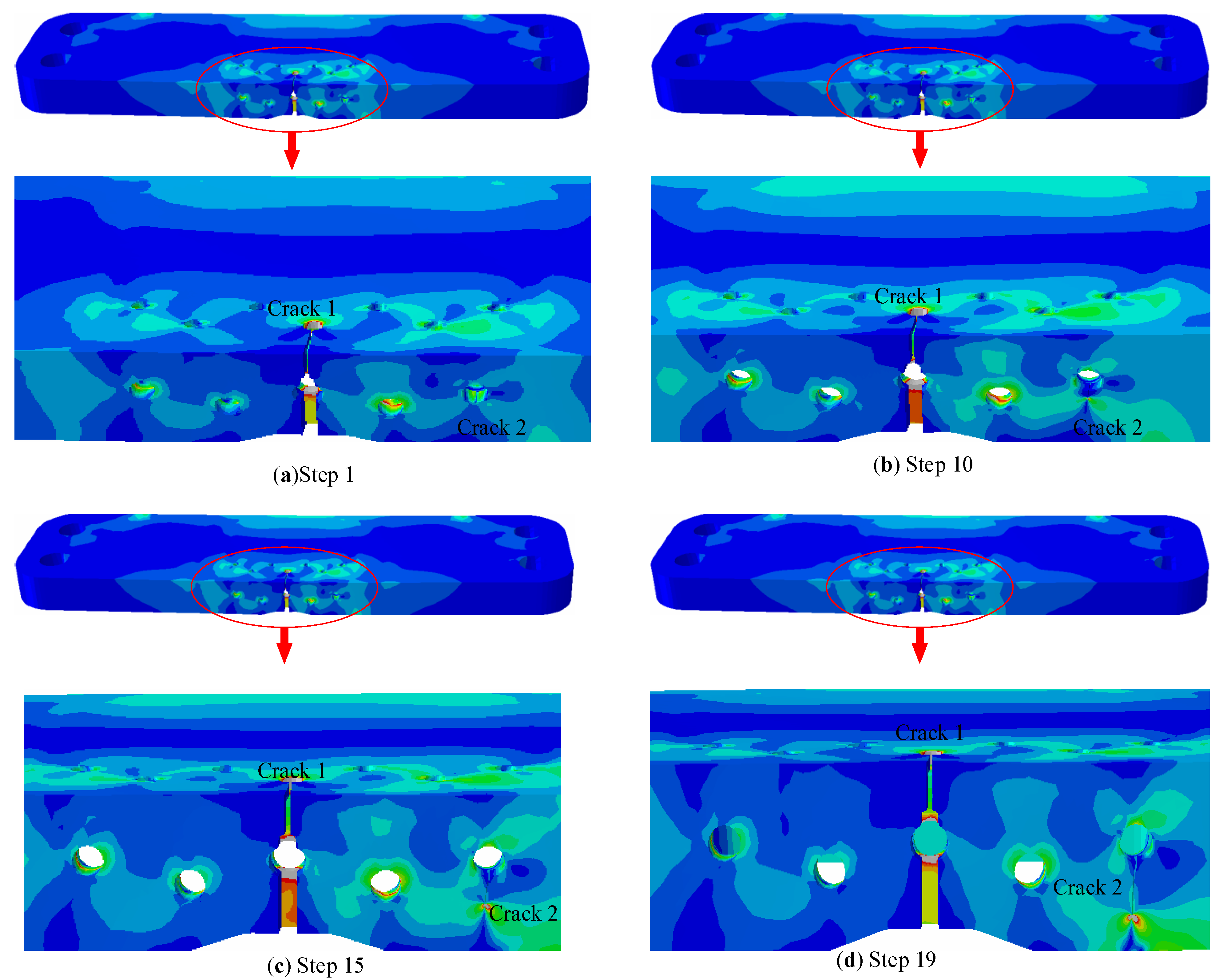
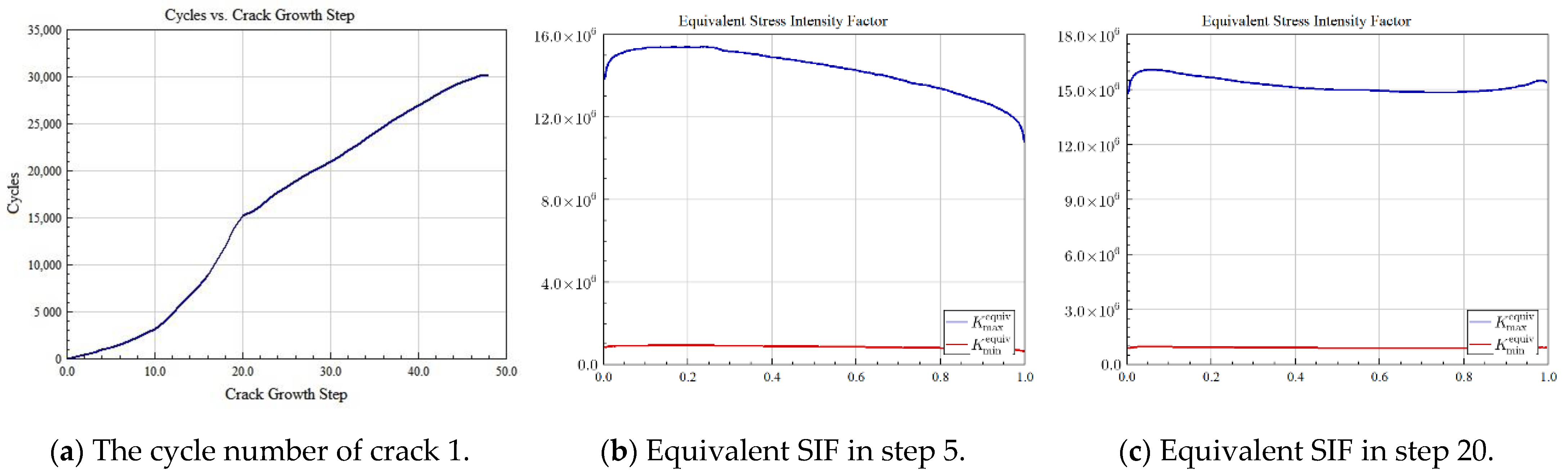
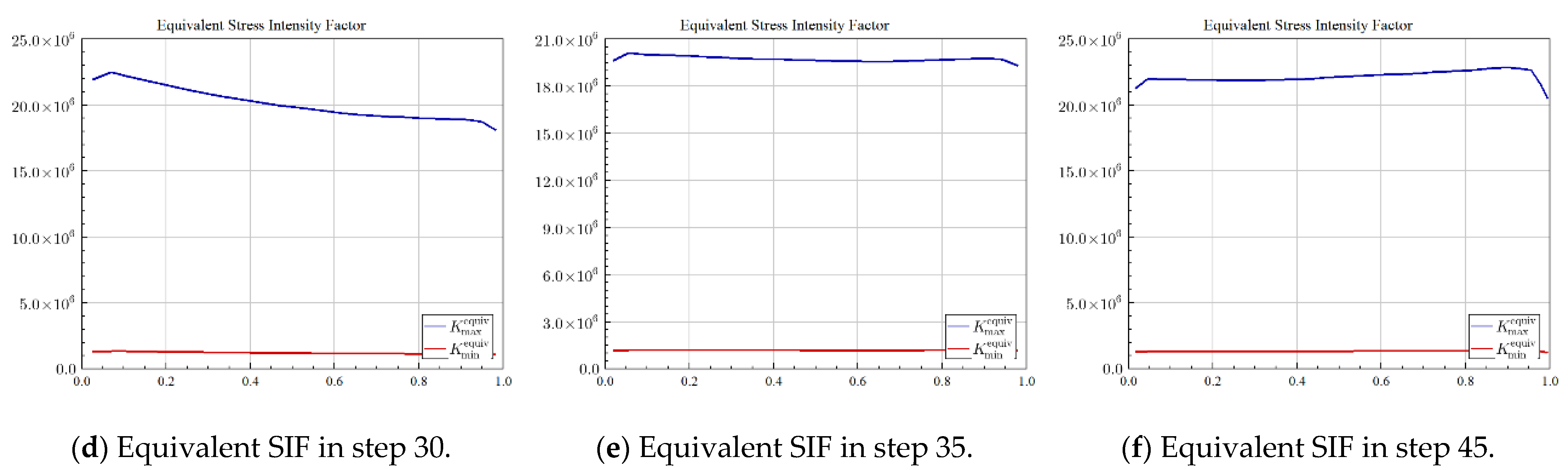

| Zn% | Cu% | Mg% | Mn% | Fe% | Si% | Cr% | Ti% | Al% |
|---|---|---|---|---|---|---|---|---|
| 5.75 | 1.57 | 2.49 | 0.29 | 0.28 | 0.27 | 0.18 | <0.1 | Others |
| Name | E/GPa | σ0.2/MPa | σb/MPa | δ% | Fatigue Strength/MPa | Density g/cm3 | Poisson’ Ratio | Brinell Hardness/HB | Shear Modulus/GPa | Shear Strength/MPa |
|---|---|---|---|---|---|---|---|---|---|---|
| 7075-T6 | 70 | 480 | 560 | 7.9 | 160 | 3.0 | 0.32 | 150 | 26 | 330 |
| σa/MPa | τa/MPa | σequ/MPa | Ra | Fmax/KN | f/HZ | Stress Ratio R |
|---|---|---|---|---|---|---|
| 340 | 196.3 | 480.83 | 9.6 | 6 | 0.1 |
| Samples | Crack 1 | Crack 2 | ||
|---|---|---|---|---|
| NO | Initiation | Propagation | Initiation | Propagation |
| 7075-T6-1 | 24,146 | 23,854 | 28,036 | 18,558 |
| 7075-T6-2 | 22,334 | 24,706 | 27,586 | 15,414 |
| 7075-T6-3 | 30,036 | 18,234 | 29,766 | 12,048 |
| 7075-T6-4 | 29,058 | 19,028 | 35,696 | 10,068 |
| 7075-T6-5 | 20,048 | 25,703 | 34,330 | 10,268 |
| 7075-T6-6 | 24,100 | 30,854 | 25,004 | 18,500 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, J.; Wang, G.; Zhao, X.; Chen, R.; Chen, W. Modeling of Multiple Fatigue Cracks for the Aircraft Wing Corner Box Based on Non-ordinary State-based Peridynamics. Metals 2022, 12, 1286. https://doi.org/10.3390/met12081286
Han J, Wang G, Zhao X, Chen R, Chen W. Modeling of Multiple Fatigue Cracks for the Aircraft Wing Corner Box Based on Non-ordinary State-based Peridynamics. Metals. 2022; 12(8):1286. https://doi.org/10.3390/met12081286
Chicago/Turabian StyleHan, Junzhao, Guozhong Wang, Xiaoyu Zhao, Rong Chen, and Wenhua Chen. 2022. "Modeling of Multiple Fatigue Cracks for the Aircraft Wing Corner Box Based on Non-ordinary State-based Peridynamics" Metals 12, no. 8: 1286. https://doi.org/10.3390/met12081286
APA StyleHan, J., Wang, G., Zhao, X., Chen, R., & Chen, W. (2022). Modeling of Multiple Fatigue Cracks for the Aircraft Wing Corner Box Based on Non-ordinary State-based Peridynamics. Metals, 12(8), 1286. https://doi.org/10.3390/met12081286






