Nonlinear q-Generalizations of Quantum Equations: Homogeneous and Nonhomogeneous Cases—An Overview
Abstract
:1. Introduction
2. Linear Schroedinger Equation
3. Generalized Nonlinear Schroedinger Equation
3.1. Free Particle and the q-Plane Wave Solution
3.2. Continuity Equation and Classical Field Theory
3.3. Other Free-Particle Solutions and Approaches
3.3.1. Solutions with the Separation of Variables
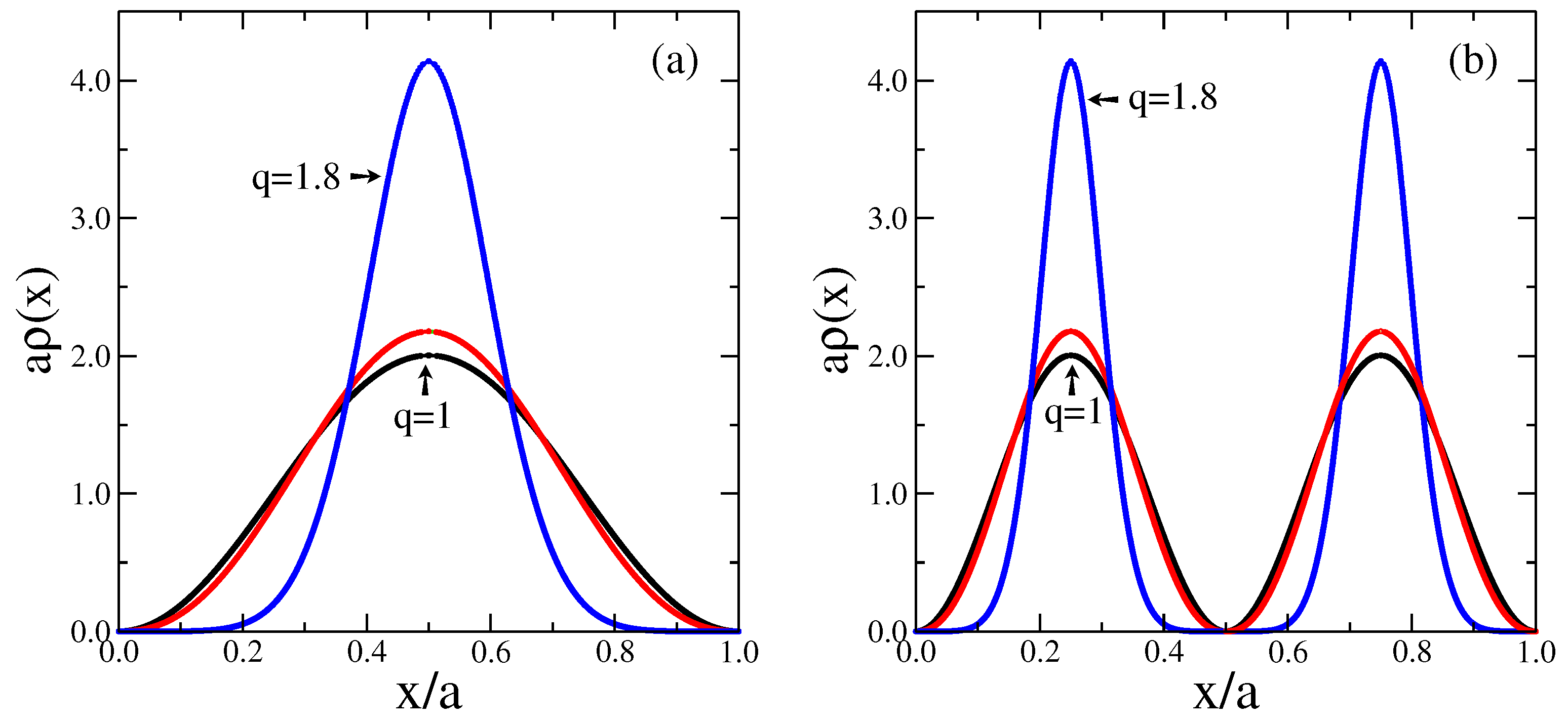
3.3.2. q-Gaussian Wave-Packet Solution
3.3.3. Further Free-Particle Approaches
3.4. Nonlinear Schroedinger Equations for a Particle in a Potential
4. Linear Nonhomogeneous Proposals of the Schroedinger Equation
The Continuity Equation
5. Generalized Nonlinear Klein–Gordon Equation
6. Conclusions and Perspectives
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Liboff, R.L. Introductory Quantum Mehanics, 4th ed.; Addison Wesley: San Francisco, CA, USA, 2003. [Google Scholar]
- Merzbacher, E. Quantum Mehanics, 3rd ed.; John Wiley and Sons: New York, NY, USA, 1998. [Google Scholar]
- Balian, R. From Microphysics to Macrophysics, vols. I and II; Springer: Berlin/Heidelberg, Germany, 1991. [Google Scholar]
- Reichl, L.E. A Modern Course in Statistical Physics, 2nd ed.; Wiley: New York, NY, USA, 1998. [Google Scholar]
- Tsallis, C. Possible generalization of Boltzmann–Gibbs statistics. J. Stat. Phys. 1988, 52, 479–487. [Google Scholar] [CrossRef]
- Tsallis, C. Introduction to Nonextensive Statistical Mechanics; Springer: New York, NY, USA, 2009. [Google Scholar]
- Curado, E.M.F.; Tsallis, C. Generalized statistical mechanics: Connection with thermodynamics. J. Phys. A 1991, 24, 3187, correction in 1992, 25, 1019. [Google Scholar] [CrossRef]
- Tsallis, C.; Mendes, R.S.; Plastino, A.R. The role of constraints within generalized nonextensive statistics. Physica A 1998, 261, 534–554. [Google Scholar] [CrossRef]
- Tsallis, C. Nonadditive entropy and nonextensive statistical mechanics—An overview after 20 years. Braz. J. Phys. 2009, 39, 337–356. [Google Scholar] [CrossRef]
- Tsallis, C. An introduction to nonadditive entropies and a thermostatistical approach to inanimate and living matter. Contemp. Phys. 2014, 55, 179–197. [Google Scholar] [CrossRef]
- Anteneodo, C.; Tsallis, C. Breakdown of exponential sensitivity to initial conditions: Role of the range of interactions. Phys. Rev. Lett. 1998, 80, 5313. [Google Scholar] [CrossRef]
- Chavanis, P.-H.; Campa, A. Inhomogeneous Tsallis distributions in the hmf model. Eur. Phys. J. B 2010, 76, 581–611. [Google Scholar] [CrossRef]
- Cirto, L.J.L.; Assis, V.R.V.; Tsallis, C. Influence of the interaction range on the thermostatistics of a classical many-body system. Physica A 2014, 393, 286–296. [Google Scholar] [CrossRef]
- Christodoulidi, H.; Tsallis, C.; Bountis, T. Fermi-Pasta-Ulam model with long-range interactions: Dynamics and thermostatistics. Europhys. Lett. 2014, 108, 40006. [Google Scholar] [CrossRef]
- Baldovin, F.; Robledo, A. Universal renormalization-group dynamics at the onset of chaos in logistic maps and nonextensive statistical mechanics. Phys. Rev. E 2002, 66, R045104. [Google Scholar] [CrossRef] [PubMed]
- Baldovin, F.; Robledo, A. Nonextensive Pesin identity. Exact renormalization group analytical results for the dynamics at the edge of chaos of the logistic map. Phys. Rev. E 2004, 69, R045202. [Google Scholar] [CrossRef] [PubMed]
- Mayoral, E.; Robledo, A. Tsallis’ q index and Mori’s q phase transitions at edge of chaos. Phys. Rev. E 2005, 72, 026209. [Google Scholar] [CrossRef] [PubMed]
- Tirnakli, U.; Tsallis, C.; Beck, C. A closer look at time averages of the logistic map at the edge of chaos. Phys. Rev. E 2009, 79, 056209. [Google Scholar] [CrossRef] [PubMed]
- Luque, B.; Lacasa, L.; Robledo, A. Feigenbaum graphs at the onset of chaos. Phys. Lett. A 2012, 376, 3625–3629. [Google Scholar] [CrossRef]
- Douglas, P.; Bergamini, S.; Renzoni, F. Tunable Tsallis distributions in dissipative optical lattices. Phys. Rev. Lett. 2006, 96, 110601. [Google Scholar] [CrossRef] [PubMed]
- Bagci, G.B.; Tirnakli, U. Self-organization in dissipative optical lattices. Chaos 2009, 19, 033113. [Google Scholar] [CrossRef] [PubMed]
- Lutz, E.; Renzoni, F. Beyond Boltzmann-Gibbs statistical mechanics in optical lattices. Nat. Phys. 2013, 9, 615–619. [Google Scholar] [CrossRef]
- Liu, B.; Goree, J. Superdiffusion and non-Gaussian statistics in a driven-dissipative 2D dusty plasma. Phys. Rev. Lett. 2008, 100, 055003. [Google Scholar] [CrossRef] [PubMed]
- Bouzit, O.; Gougam, L.A.; Tribeche, M. Screening and sheath formation in a nonequilibrium mixed Cairns-Tsallis electron distribution. Phys. Plasmas 2015, 22, 052112. [Google Scholar] [CrossRef]
- DeVoe, R.G. Power-law distributions for a trapped ion interacting with a classical buffer gas. Phys. Rev. Lett. 2009, 102, 063001. [Google Scholar] [CrossRef] [PubMed]
- Pickup, R.M.; Cywinski, R.; Pappas, C.; Farago, B.; Fouquet, P. Generalized spin glass relaxation. Phys. Rev. Lett. 2009, 102, 097202. [Google Scholar] [CrossRef] [PubMed]
- Tsallis, C.; de Souza, A.M.C.; Maynard, R. Derivation of Lévy-type anomalous superdiffusion from generalized statistical mechanics. In Lévy Flights and Related Topics in Physics; Shlesinger, M.F., Zaslavsky, G.M., Frisch, U., Eds.; Springer: Berlin/Heidelberg, Germany, 1995; p. 269. [Google Scholar]
- Tsallis, C.; Levy, S.V.F.; de Souza, A.M.C.; Maynard, R. Statistical-mechanical foundation of the ubiquity of Levy distributions in nature. Phys. Rev. Lett. 1996, 75, 3589. [Google Scholar] [CrossRef] [PubMed]
- Combe, G.; Richefeu, V.; Stasiak, M.; Atman, A.P.F. Experimental validation of nonextensive scaling law in confined granular media. Phys. Rev. Lett. 2015, 115, 238301. [Google Scholar] [CrossRef] [PubMed]
- CMS Collaboration. Transverse-momentum and pseudorapidity distributions of charged hadrons in pp collisions at = 0.9 and 2.36 TeV. J. High Energy Phys. 2010, 2, 041. [Google Scholar]
- CMS Collaboration. Transverse-momentum and pseudorapidity distributions of charged hadrons in pp collisions at = 7 TeV. Phys. Rev. Lett. 2010, 105, 022002. [Google Scholar]
- Marques, L.; Andrade-II, E.; Deppman, A. Nonextensivity of hadronic systems. Phys. Rev. D 2013, 87, 114022. [Google Scholar] [CrossRef]
- Marques, L.; Cleymans, J.; Deppman, A. Description of high-energy pp collisions using Tsallis thermodynamics: Transverse momentum and rapidity distributions. Phys. Rev. D 2015, 91, 054025. [Google Scholar] [CrossRef]
- Oliveira, H.P.; Soares, I.D. Dynamics of black hole formation: Evidence for nonextensivity. Phys. Rev. D 2005, 71, 124034. [Google Scholar] [CrossRef]
- Komatsu, N.; Kimura, S. Entropic cosmology for a generalized black-hole entropy. Phys. Rev. D 2013, 88, 083534. [Google Scholar] [CrossRef]
- Silva, V.H.C.; Aquilanti, V.; de Oliveira, H.C.B.; Mundim, K.C. Uniform description of non-Arrhenius temperature dependence of reaction rates, and a heuristic criterion for quantum tunneling vs. classical non-extensive distribution. Chem. Phys. Lett. 2013, 590, 201–207. [Google Scholar] [CrossRef]
- Borland, L. Closed form option pricing formulas based on a non-Gaussian stock price model with statistical feedback. Phys. Rev. Lett. 2002, 89, 098701. [Google Scholar] [CrossRef] [PubMed]
- Ludescher, J.; Tsallis, C.; Bunde, A. Universal behaviour of interoccurrence times between losses in financial markets: An analytical description. Europhys. Lett. 2011, 95, 68002. [Google Scholar] [CrossRef]
- Ludescher, J.; Bunde, A. Universal behavior of the interoccurrence times between losses in financial markets: Independence of the time resolution. Phys. Rev. 2014, 90, 062809. [Google Scholar] [CrossRef] [PubMed]
- Antonopoulos, C.G.; Michas, G.; Vallianatos, F.; Bountis, T. Evidence of q-exponential statistics in Greek seismicity. Physica A 2014, 409, 71–77. [Google Scholar] [CrossRef]
- Upadhyaya, A.; Rieu, J.-P.; Glazier, J.A.; Sawada, Y. Anomalous diffusion and non-Gaussian velocity distribution of Hydra cells in cellular aggregates. Physica A 2001, 293, 549–558. [Google Scholar] [CrossRef]
- Bogachev, M.I.; Kayumov, A.R.; Bunde, A. Universal internucleotide statistics in full genomes: A footprint of the DNA structure and packaging? PLoS ONE 2014, 9, e112534. [Google Scholar] [CrossRef] [PubMed]
- Burlaga, L.F.; Vinas, A.F. Triangle for the entropic index q of non-extensive statistical mechanics observed by Voyager 1 in the distant heliosphere. Physica A 2005, 356, 375–384. [Google Scholar] [CrossRef]
- Burlaga, L.F.; Ness, N.F. Magnetic field strength fluctuations and the q-triplet in the heliosheath: Voyager 2 observations from 91.0 to 94.2 AU at latitude 30 S. Astrophys. J. 2013, 765, 35. [Google Scholar] [CrossRef]
- Moyano, L.G.; Tsallis, C.; Gell-Mann, M. Numerical indications of a q-generalised central limit theorem. Europhys. Lett. 2006, 73, 813. [Google Scholar] [CrossRef]
- Thistleton, W.J.; Marsh, J.A.; Nelson, K.P.; Tsallis, C. q-Gaussian approximants mimic non-extensive statistical-mechanical expectation for many-body probabilistic model with long-range correlations. Cent. Eur. J. Phys. 2009, 7, 387–394. [Google Scholar] [CrossRef]
- Chavanis, P.-H. Nonlinear mean field Fokker-Planck equations. Application to the chemotaxis of biological population. Eur. Phys. J. B 2008, 62, 179–208. [Google Scholar] [CrossRef]
- Umarov, S.; Tsallis, C.; Steinberg, S. On a q-central limit theorem consistent with nonextensive statistical mechanics. Milan J. Math. 2008, 76, 307–328. [Google Scholar] [CrossRef]
- Queiros, S.M.D.; Tsallis, C. Nonextensive statistical mechanics and central limit theorems II—Convolution of q-independent random variables. In Complexity, Metastability and Nonextensivity; Abe, S., Herrmann, H.J., Quarati, P., Rapisarda, A., Tsallis, C., Eds.; AIP Publishing: New York, NY, USA, 2007. [Google Scholar]
- Umarov, S.; Tsallis, C.; Gell-Mann, M.; Steinberg, S. Generalization of symmetric α-stable Lévy distributions for q > 1. J. Math. Phys. 2010, 51, 033502. [Google Scholar] [CrossRef] [PubMed]
- Nelson, K.P.; Umarov, S. Nonlinear statistical coupling. Physica A 2010, 389, 2157–2163. [Google Scholar] [CrossRef]
- Hanel, R.; Thurner, S.; Tsallis, C. Limit distributions of scale-invariant probabilistic models of correlated random variables with the q-Gaussian as an explicit example. Eur. Phys. J. B 2009, 72, 263–268. [Google Scholar] [CrossRef]
- Umarov, S.; Tsallis, C. The limit distribution in the q-CLT for q ≥ 1 is unique and can not have a compact support. J. Phys. A 2016, 49, 415204. [Google Scholar] [CrossRef]
- Caruso, F.; Tsallis, C. Nonadditive entropy reconciles the area law in quantum systems with classical thermodynamics. Phys. Rev. E 2008, 78, 021102. [Google Scholar] [CrossRef] [PubMed]
- Carrasco, J.A.; Finkel, F.; Gonzalez-Lopez, A.; Rodriguez, M.A.; Tempesta, P. Generalized isotropic Lipkin-Meshkov-Glick models: Ground state entanglement and quantum entropies. J. Stat. Mech. 2016, 2016, 033114. [Google Scholar] [CrossRef]
- Weinstein, Y.S.; Lloyd, S.; Tsallis, C. Border between between regular and chaotic quantum dynamics. Phys. Rev. Lett. 2002, 89, 214101. [Google Scholar] [CrossRef] [PubMed]
- Betzler, A.S.; Borges, E.P. Nonextensive distributions of asteroid rotation periods and diameters. Astron. Astrophys. 2012, 539, A158. [Google Scholar] [CrossRef]
- Betzler, A.S.; Borges, E.P. Nonextensive statistical analysis of meteor showers and lunar flashes. Mon. Not. R. Astron. Soc. 2015, 447, 765–771. [Google Scholar] [CrossRef]
- Capurro, A.; Diambra, L.; Lorenzo, D.; Macadar, O.; Martin, M.T.; Mostaccio, C.; Plastino, A.; Rofman, E.; Torres, M.E.; Velluti, J. Tsallis entropy and cortical dynamics: The analysis of EEG signals. Physica A 1998, 257, 149–155. [Google Scholar] [CrossRef]
- Mohanalin, J.; Beenamol; Kalra, P.K.; Kumar, N. A novel automatic microcalcification detection technique using Tsallis entropy and a type II fuzzy index. Comput. Math. Appl. 2010, 60, 2426–2432. [Google Scholar] [CrossRef]
- Nivanen, L.; Le Mehaute, A.; Wang, Q.A. Generalized algebra within a nonextensive statistics. Rep. Math. Phys. 2003, 52, 437–444. [Google Scholar] [CrossRef]
- Borges, E.P. A possible deformed algebra and calculus inspired in nonextensive thermostatistics. Physica A 2004, 340, 95–101. [Google Scholar] [CrossRef]
- Tempesta, P. Group entropies, correlation laws, and zeta functions. Phys. Rev. E 2011, 84, 021121. [Google Scholar] [CrossRef] [PubMed]
- Ruiz, G.; Tsallis, C. Reply to comment on “towards a large deviation theory for strongly correlated systems”. Phys. Lett. A 2013, 377, 491–495. [Google Scholar] [CrossRef]
- Soares, D.J.B.; Tsallis, C.; Mariz, A.M.; Silva, L.R. Preferential attachment growth model and nonextensive statistical mechanics. Europhys. Lett. 2005, 70, 70. [Google Scholar] [CrossRef]
- Brito, S.G.A.; da Silva, L.R.; Tsallis, C. Role of dimensionality in complex networks. Nat. Sci. Rep. 2016, 6, 27992. [Google Scholar] [CrossRef] [PubMed]
- Nobre, F.D.; Rego-Monteiro, M.A.; Tsallis, C. Nonlinear Relativistic and Quantum Equations with a Common Type of Solution. Phys. Rev. Lett. 2011, 106, 140601. [Google Scholar] [CrossRef] [PubMed]
- Scott, A.C. (Ed.) Encyclopedia of Nonlinear Science; Taylor and Francis: New York, NY, USA, 2005.
- Scott, A.C. The Nonlinear Universe; Springer: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Polyanin, A.D.; Zaitsev, V.F. Handbook of Nonlinear Partial Differential Equations; Chapman and Hall—CRC: Boca Raton, FL, USA, 2004. [Google Scholar]
- Frank, T.D. Nonlinear Fokker-Planck Equations: Fundamentals and Applications; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Plastino, A.R.; Plastino, A. Non-extensive statistical mechanics and generalized Fokker-Planck equation. Physica A 1995, 222, 347–354. [Google Scholar] [CrossRef]
- Tsallis, C.; Bukman, D.J. Anomalous diffusion in the presence of external forces: Exact time-dependent solutions and their thermostatistical basis. Phys. Rev. E 1996, 54, R2197. [Google Scholar] [CrossRef]
- Schwämmle, V.; Nobre, F.D.; Curado, E.M.F. Consequences of the H theorem from nonlinear Fokker-Planck equations. Phys. Rev. E 2007, 76, 041123. [Google Scholar] [CrossRef] [PubMed]
- Schwämmle, V.; Curado, E.M.F.; Nobre, F.D. A general nonlinear Fokker-Planck equation and its associated entropy. Eur. Phys. J. B 2007, 58, 159–165. [Google Scholar] [CrossRef]
- Ribeiro, M.S.; Nobre, F.D.; Curado, E.M.F. Classes of N-Dimensional Nonlinear Fokker-Planck Equations Associated to Tsallis Entropy. Entropy 2011, 13, 1928–1944. [Google Scholar] [CrossRef]
- Sulem, C.; Sulem, P.-L. The Nonlinear Schroedinger Equation: Self-Focusing and Wave Collapse; Springer: New York, NY, USA, 1999. [Google Scholar]
- Greiner, W. Relativistic Quantum Mechanics, 3rd ed.; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar]
- Greiner, W.; Reinhardt, J. Field Quantization; Springer: Berlin/Heidelberg, Germany, 1996. [Google Scholar]
- Huang, K. Quantum Field Theory—From Operators to Path Integrals; WILEY-VCH: Weinheim, Germany, 2004. [Google Scholar]
- Vázquez, J.L. The Porous Medium Equation; Oxford University Press: Oxford, UK, 2007. [Google Scholar]
- Borges, E.P. On a q-generalization of circular and hyperbolic functions. J. Phys. A 1998, 31, 5281. [Google Scholar] [CrossRef]
- Nobre, F.D.; Plastino, A.R. Generalized nonlinear Proca equation and its free-particle solutions. Eur. Phys. J. C 2016, 76, 343. [Google Scholar] [CrossRef]
- Jauregui, M.; Tsallis, C. New representations of π and Dirac delta using the nonextensive-statistical- mechanics q-exponential function. J. Math. Phys. 2010, 51, 063304. [Google Scholar] [CrossRef]
- Nobre, F.D.; Rego-Monteiro, M.A.; Tsallis, C. A generalized nonlinear Schroedinger equation: Classical field-theoretic approach. Europhys. Lett. 2012, 97, 41001. [Google Scholar] [CrossRef]
- Rego-Monteiro, M.A.; Nobre, F.D. Nonlinear quantum equations: Classical field theory. J. Math. Phys. 2013, 54, 103302. [Google Scholar] [CrossRef]
- Plastino, A.R.; Souza, A.M.C.; Nobre, F.D.; Tsallis, C. Stationary and uniformly accelerated states in nonlinear quantum mechanics. Phys. Rev. A 2014, 90, 062134. [Google Scholar] [CrossRef]
- Bountis, T.; Nobre, F.D. Travelling-wave and separated variable solutions of a nonlinear Schroedinger equation. J. Math. Phys. 2016, 57, 082106. [Google Scholar] [CrossRef]
- Plastino, A.; Rocca, M.C. From the hypergeometric differential equation to a non-linear Schroedinger one. Phys. Lett. A 2015, 379, 2690–2693. [Google Scholar] [CrossRef]
- Plastino, A.; Rocca, M.C. Hypergeometric connotations of quantum equations. Physica A 2016, 450, 435–443. [Google Scholar] [CrossRef]
- Curilef, S.; Plastino, A.R.; Plastino, A. Tsallis’ maximum entropy ansatz leading to exact analytical time dependent wave packet solutions of a nonlinear Schroedinger equation. Physica A 2013, 392, 2631–2642. [Google Scholar] [CrossRef]
- Pennini, F.; Plastino, A.R.; Plastino, A. Pilot wave approach to the NRT nonlinear Schroedinger equation. Physica A 2014, 403, 195–205. [Google Scholar] [CrossRef]
- Alves, L.G.A.; Ribeiro, H.V.; Santos, M.A.F.; Mendes, R.S.; Lenzi, E.K. Solutions for a q-generalized Schroedinger equation of entangled interacting particles. Physica A 2015, 429, 35–44. [Google Scholar] [CrossRef]
- Holland, P.R. The Quantum Theory of Motion; Cambridge University Press: Cambridge, UK, 1993. [Google Scholar]
- Bell, J.S. Speakable and Unspeakable in Quantum Mechanics; Cambridge University Press: Cambridge, UK, 1987. [Google Scholar]
- Plastino, A.R.; Tsallis, C. Nonlinear Schroedinger equation in the presence of uniform acceleration. J. Math. Phys. 2013, 54, 041505. [Google Scholar] [CrossRef]
- Toranzo, I.V.; Plastino, A.R.; Dehesa, J.S.; Plastino, A. Quasi-stationary states of the NRT nonlinear Schroedinger equation. Physica A 2013, 392, 3945–3951. [Google Scholar] [CrossRef]
- Case, W.B. Wigner functions and Weyl transforms for pedestrians. Am. J. Phys. 2008, 76, 937–946. [Google Scholar] [CrossRef]
- Bastard, G. Wave Mechanics Applied to Semiconductor Heterostructures; Les Editions de Physique: Les Ulis, France, 1988. [Google Scholar]
- Weisbuch, C.; Vinter, B. Quantum Semiconductor Structures: Fundamentals and Applications; Academic Press: New York, NY, USA, 1991. [Google Scholar]
- Zhao, F.Q.; Liang, X.X.; Ban, S.L. Influence of the spatially dependent effective mass on bound polarons in finite parabolic quantum wells. Eur. Phys. J. B 2003, 33, 3–8. [Google Scholar] [CrossRef]
- Keshavarz, A.; Zamani, N. Optical properties of spherical quantum dot with position-dependent effective mass. Superlattices Microstruct. 2013, 58, 191–197. [Google Scholar] [CrossRef]
- Plastino, A.R.; Rigo, A.; Casas, M.; Garcias, F.; Plastino, A. Supersymmetric approach to quantum systems with position-dependent effective mass. Phys. Rev. A 1999, 60, 4318. [Google Scholar] [CrossRef]
- Costa Filho, R.N.; Almeida, M.P.; Farias, G.A.; Andrade, J.S., Jr. Displacement operator for quantum systems with position-dependent mass. Phys. Rev. A 2011, 84, 050102. [Google Scholar] [CrossRef]
- Rego-Monteiro, M.A.; Nobre, F.D. Classical field theory for a non-Hermitian Schroedinger equation with position-dependent masses. Phys. Rev. A 2013, 88, 032105. [Google Scholar] [CrossRef]
- Nobre, F.D.; Rego-Monteiro, M.A. Non-Hermitian PT Symmetric Hamiltonian with Position-Dependent Masses: Associated Schroedinger Equation and Finite-Norm Solutions. Braz. J. Phys. 2015, 45, 79–88. [Google Scholar] [CrossRef]
- Costa Filho, R.N.; Alencar, G.; Skagerstam, B.-S.; Andrade, J.S., Jr. Morse potential derived from first principles. Europhys. Lett. 2013, 101, 10009. [Google Scholar] [CrossRef]
- Sinha, A.; Roychoudhury, R. Scattering in a spatially varying mass-symmetric double heterojunction. Europhys. Lett. 2013, 103, 50007. [Google Scholar] [CrossRef]
- Da Costa, B.G.; Borges, E.P. Generalized space and linear momentum operators in quantum mechanics. J. Math. Phys. 2014, 55, 062105. [Google Scholar] [CrossRef]
- Rego-Monteiro, M.A.; Rodrigues, L.M.C.S.; Curado, E.M.F. Position-dependent mass quantum Hamiltonians: General approach and duality. J. Phys. A Math. Theor. 2016, 49, 125203. [Google Scholar] [CrossRef]
- Bollini, C.G.; Giambiagi, J.J. Lagrangian Procedures for Higher Order Field Equations. Rev. Bras. Fís. 1987, 17, 14. [Google Scholar]
- Barcelos-Neto, J.; Braga, N.R.F. Higher Order Canonical Formalism for the Scalar Field Theory. Acta Phys. Polonica B 1989, 20, 205–210. [Google Scholar]
- Plastino, A.R.; Tsallis, C. Dissipative effects in nonlinear Klein-Gordon dynamics. Europhys. Lett. 2016, 113, 50005. [Google Scholar] [CrossRef]

© 2017 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nobre, F.D.; Rego-Monteiro, M.A.; Tsallis, C. Nonlinear q-Generalizations of Quantum Equations: Homogeneous and Nonhomogeneous Cases—An Overview. Entropy 2017, 19, 39. https://doi.org/10.3390/e19010039
Nobre FD, Rego-Monteiro MA, Tsallis C. Nonlinear q-Generalizations of Quantum Equations: Homogeneous and Nonhomogeneous Cases—An Overview. Entropy. 2017; 19(1):39. https://doi.org/10.3390/e19010039
Chicago/Turabian StyleNobre, Fernando D., Marco Aurélio Rego-Monteiro, and Constantino Tsallis. 2017. "Nonlinear q-Generalizations of Quantum Equations: Homogeneous and Nonhomogeneous Cases—An Overview" Entropy 19, no. 1: 39. https://doi.org/10.3390/e19010039




