A Microwave Ring-Resonator Sensor for Non-Invasive Assessment of Meat Aging
Abstract
:1. Introduction
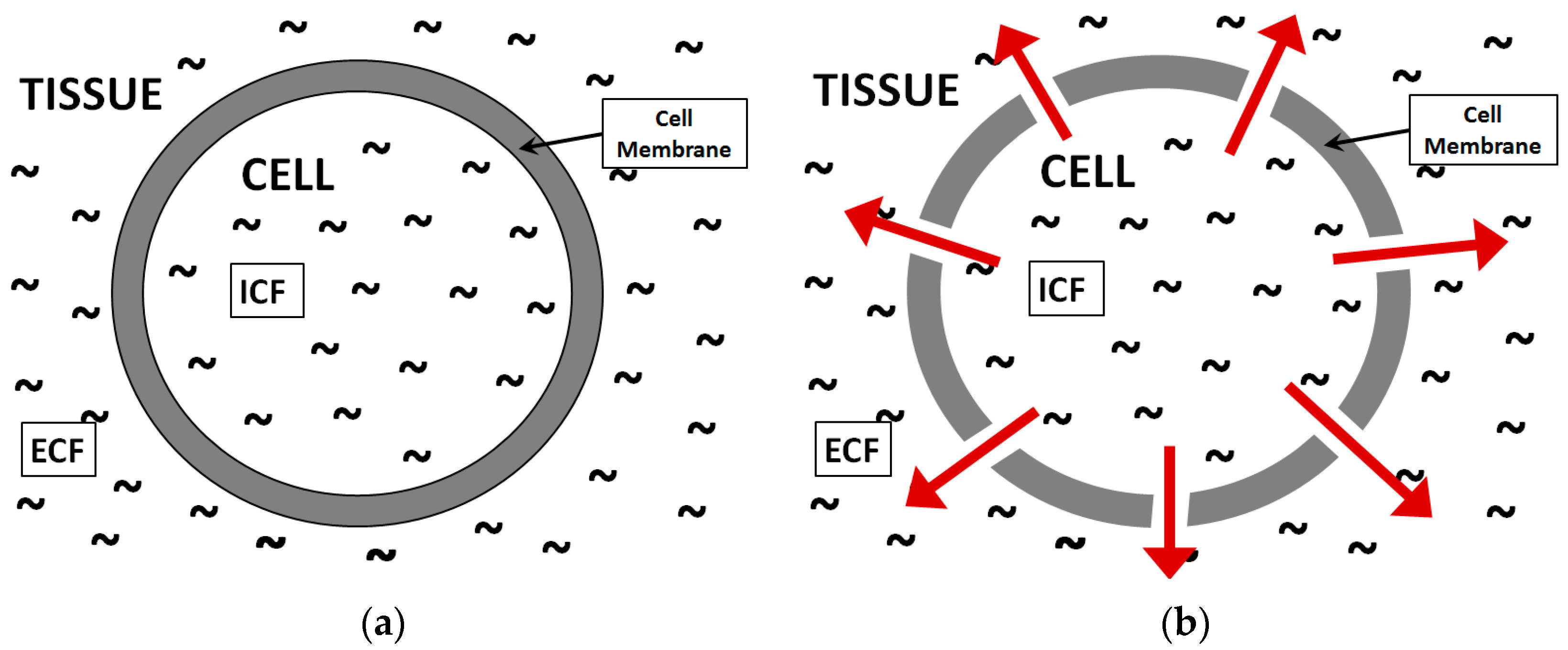
2. Microwave Ring-Resonator Sensor: Design and Theoretical Analysis
2.1. Design and Development
| Design Parameter | Value |
|---|---|
| Substrate dielectric constant (ɛr) | 2.2 |
| Dissipation factor (tan δ) | 0.0009 |
| Ring outer-radius (ra) | 34.87 mm |
| Ring inner-radius (rb) | 34.27 mm |
| Feed width (wl) | 35 mm |
| Ring width (wr) | 2.4 mm |
| Coupling gap (s) | 600 µm |
| Conductor strip (t) | 17.5 µm |
| Substrate height (h) | 787 µm |


2.2. Theoretical Analysis

3. Experimental
3.1. Sample Handling and Preparation
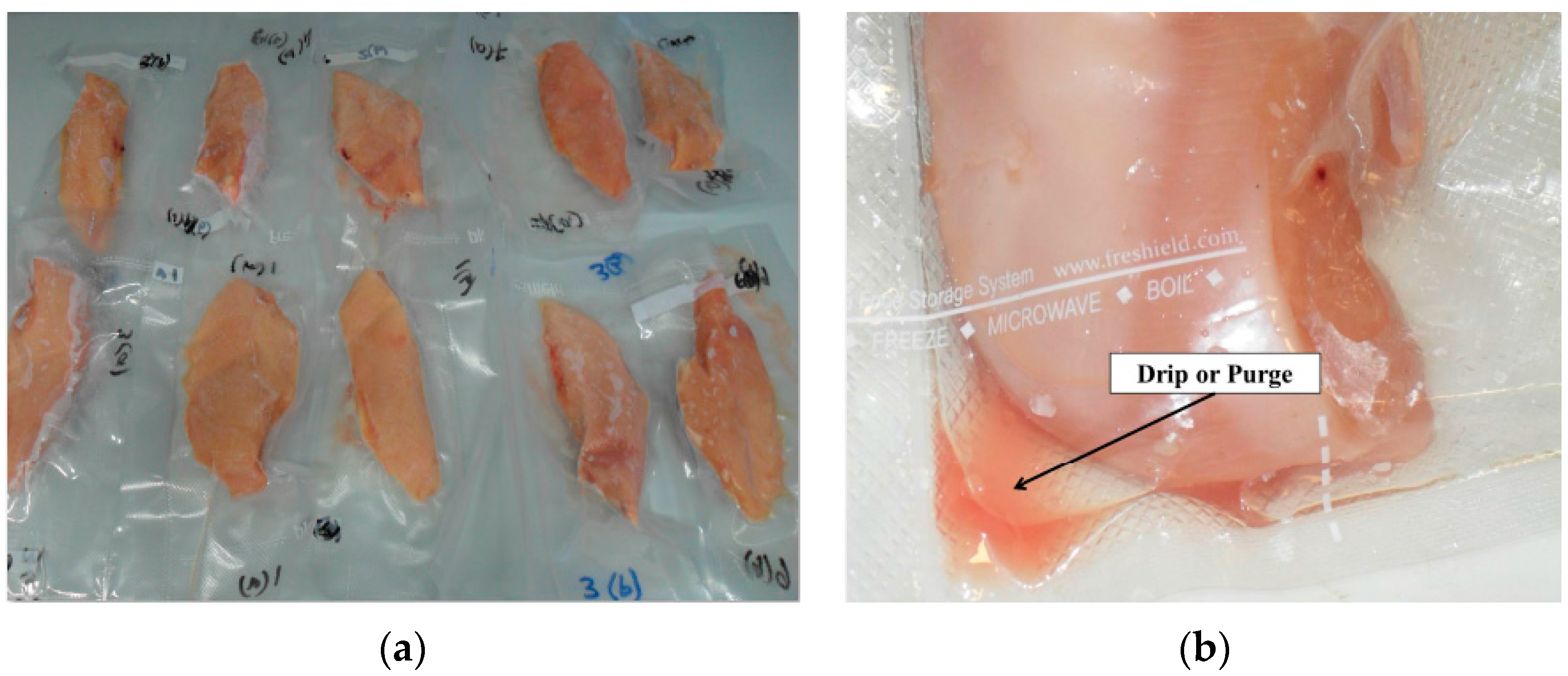
3.2. Physical Measurements—pH, Color and Temperature
3.3. Electrical Measurements

4. Results and Discussion
| Physical Properties | Measured Value | |
|---|---|---|
| Color 1 | L* | 57.61 |
| a* | 1.77 | |
| b* | 5.19 | |
| pH 2 | 8 h | 5.67 |
| 24 h (postmortem time) | 5.44 | |


Equivalent Circuit Modeling for Storage Effect



5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Trabelsi, S. Investigating Effects of Aging on Radiofrequency Dielectric Properties of Chicken Meat. In Proceedings of the IEEE International Instrumentation and Measurement Technology Conference (I2MTC), Minneapolis, MN, USA, 6–9 May 2013; pp. 1685–1688.
- Damez, J.-L.; Clerjon, S.; Abouelkaram, S.; Lepetit, J. Beef meat electrical impedance spectroscopy and anisotropy sensing for non-invasive early assessment of meat ageing. J. Food Engin. 2008, 85, 116–122. [Google Scholar] [CrossRef]
- You, K.Y.; Mun, H.K.; You, L.L.; Salleh, J.; Abbas, Z. A small and slim coaxial probe for single rice grain moisture sensing. Sensors 2013, 13, 3652–3663. [Google Scholar] [CrossRef] [PubMed]
- Guermazi, M.; Kanoun, O.; Derbel, N. Investigation of long time beef and veal meat behavior by bioimpedance spectroscopy for meat monitoring. IEEE Sens. J. 2014, 14, 3624–3630. [Google Scholar] [CrossRef]
- Damez, J.-L.; Clerjon, S. Quantifying and predicting meat and meat products quality attributes using electromagnetic waves: An overview. Meat Sci. 2013, 95, 879–896. [Google Scholar] [CrossRef] [PubMed]
- Siddiqui, O.; Kashanianfard, M.; Ramahi, O. Dielectric sensors based on electromagnetic energy tunneling. Sensors 2015, 15, 7844–7856. [Google Scholar] [CrossRef] [PubMed]
- Castro-Giráldez, M.; Aristoy, M.; Toldrá, F.; Fito, P. Microwave dielectric spectroscopy for the determination of pork meat quality. Food Res. Int. 2010, 43, 2369–2377. [Google Scholar] [CrossRef]
- Huff-Lonergan, E.; Lonergan, S.M. Mechanisms of water-holding capacity of meat: The role of postmortem biochemical and structural changes. Meat Sci. 2005, 71, 194–204. [Google Scholar] [CrossRef] [PubMed]
- Trabelsi, S.; Roelvink, J. Investigating the influence of aging on radiofrequency dielectric properties of chicken meat. J. Microw. Power Electromagn. Energy 2014, 48, 215–220. [Google Scholar]
- Castro-Giráldez, M.; Dols, L.; Toldrá, F.; Fito, P. Development of a dielectric spectroscopy technique for the determination of key biochemical markers of meat quality. Food Chem. 2011, 127, 228–233. [Google Scholar] [CrossRef]
- Damez, J.-L.; Clerjon, S.; Abouelkaram, S.; Lepetit, J. Dielectric behavior of beef meat in the 1–1500 kHz range: Simulation with the fricke/cole–cole model. Meat Sci. 2007, 77, 512–519. [Google Scholar] [CrossRef] [PubMed]
- Roelvink, J.; Trabelsi, S. Measuring the Complex Permittivity of Poultry Meat with a Planar Transmission-Line Sensor. In Proceedings of the IEEE International Instrumentation and Measurement Technology Conference (I2MTC), Minneapolis, MN, USA, 6–9 May 2013; pp. 1689–1693.
- Note, Application. In Basics of Measuring the Dielectric properties of Material; Agilent Technologies: Santa Clara, CA, USA, 2006.
- Jilani, M.T.; Wen, W.P.; Zakariya, M.A.; Cheong, L.Y.; Rehman, M.Z.U. An Improved Design of Microwave Biosensor for Measurement of Tissues Moisture. In Proceedings of the IEEE MTT-S International Microwave Workshop Series on RF and Wireless Technologies for Biomedical and Healthcare Applications (IMWS-Bio), London, UK, 8–10 December 2014; pp. 1–3.
- Bernard, P.A.; Gautray, J.M. Measurement of dielectric constant using a microstrip ring resonator. IEEE Trans. Microw. Theory Tech. 1991, 39, 592–595. [Google Scholar] [CrossRef]
- Jilani, M.T.; Wen, W.P.; Rehman, M.Z.U.; Khan, A.M.; Cheong, L.Y. Microwave sensor for non-destructive dielectric characterization of biological systems. Int. J. Appl. Electromagn. Mech. 2016, in press. [Google Scholar]
- Bahl, I.; Stuchly, S.S.; Stuchly, M. A new microstrip radiator for medical applications. EEE Trans. Microw. Theory Tech. 1980, 28, 1464–1469. [Google Scholar] [CrossRef]
- Saeed, K.; Guyette, A.C.; Hunter, I.C.; Pollard, R.D. Microstrip Resonator Technique for Measuring Dielectric Permittivity of Liquid Solvents and for Solution Sensing. In Proceedings of the IEEE/MTT-S International Microwave Symposium, Honolulu, HI, USA, 3–8 June 2007; pp. 1185–1188.
- Jilani, M.T.; Wen, W.P.; Cheong, L.Y.; Zakariya, M.A.; Rehman, M.Z.U. Equivalent circuit modeling of the dielectric loaded microwave biosensor. Radioengineering 2014, 23, 1038–1047. [Google Scholar]
- Hsieh, L.-H.; Chang, K. Equivalent lumped elements g, l, c, and unloaded q's of closed-and open-loop ring resonators. IEEE Trans. Microw. Theory Tech. 2002, 50, 453–460. [Google Scholar] [CrossRef]
- Hopkins, R.; Free, C. Equivalent circuit for the microstrip ring resonator suitable for broadband materials characterisation. Microw. Antennas Propag. IET 2008, 2, 66–73. [Google Scholar] [CrossRef]
- Garg, R.; Bahl, I. Microstrip discontinuities. Int. J. Electron. Theor. Exp. 1978, 45, 81–87. [Google Scholar] [CrossRef]
- Jilani, M.T.; Wen, W.P.; Cheong, L.Y.; Rehman, M.Z.U.; Khan, M.T. Determination of size-independent effective permittivity of an overlay material using microstrip ring resonator. Microw. Op. Technol. Lett. 2016, 58, 4–9. [Google Scholar] [CrossRef]
- Castro-Giráldez, M.; Botella, P.; Toldrá, F.; Fito, P. Low-frequency dielectric spectrum to determine pork meat quality. Innov. Food Sci. Emerg. Technol. 2010, 11, 376–386. [Google Scholar] [CrossRef]
- Clerjon, S.; Damez, J.-L. Microwave sensing for an objective evaluation of meat ageing. J. Food Engin. 2009, 94, 379–389. [Google Scholar] [CrossRef]
- Samuel, D.; Trabelsi, S. Measurement of dielectric properties of intact and ground broiler breast meat over the frequency range from 500 MHz to 50 GHz. Int. J. Poult. Sci. 2012, 11, 172–176. [Google Scholar] [CrossRef]
- Byrne, C.; Troy, D.; Buckley, D. Postmortem changes in muscle electrical properties of bovine M. longissimus dorsi and their relationship to meat quality attributes and pH fall. Meat Sci. 2000, 54, 23–34. [Google Scholar] [CrossRef]
- Lesiów, T.; Kijowski, J. Impact of PSE and DFD meat on poultry processing—A review. Pol. J. Food Nutr. Sci. 2003, 12, 3–8. [Google Scholar]
- Adzitey, F.; Nurul, H. Pale soft exudative (PSE) and dark firm dry (DFD) meats: Causes and measures to reduce these incidences—A mini review. Int. Food Res. J. 2011, 18, 11–20. [Google Scholar]
- Fennema, O. Comparative water holding properties of various muscle foods. J. Muscle Foods 1990, 1, 363–381. [Google Scholar] [CrossRef]
- Wagner, J.R.; AÑOn, M.C. Effect of frozen storage on protein denaturation in bovine muscle 1. Myofibrillar ATPase activity and differential scanning calorimetric studies. Int. J. Food Sci. Technol. 1986, 21, 9–18. [Google Scholar] [CrossRef]
- Den Hertog-Meischke, M.J.A.; van Laack, R.J.L.M.; Smulders, F.J.M. The water-holding capacity of fresh meat. Vet. Q. 1997, 19, 175–181. [Google Scholar] [CrossRef] [PubMed]
- Huff-Lonergan, E. Chemistry and Biochemistry of Meat; Wiley-Blackwell: Oxford, UK, 2010. [Google Scholar]
- Huff-Lonergan, E. Water-holding capacity of fresh meat. Fact. Sheet 2002, 4669. [Google Scholar]
- Damez, J.-L.; Clerjon, S.; Abouelkaram, S.; Lepetit, J. Electrical impedance probing of the muscle food anisotropy for meat ageing control. Food Control 2008, 19, 931–939. [Google Scholar] [CrossRef]
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jilani, M.T.; Wen, W.P.; Cheong, L.Y.; Ur Rehman, M.Z. A Microwave Ring-Resonator Sensor for Non-Invasive Assessment of Meat Aging. Sensors 2016, 16, 52. https://doi.org/10.3390/s16010052
Jilani MT, Wen WP, Cheong LY, Ur Rehman MZ. A Microwave Ring-Resonator Sensor for Non-Invasive Assessment of Meat Aging. Sensors. 2016; 16(1):52. https://doi.org/10.3390/s16010052
Chicago/Turabian StyleJilani, Muhammad Taha, Wong Peng Wen, Lee Yen Cheong, and Muhammad Zaka Ur Rehman. 2016. "A Microwave Ring-Resonator Sensor for Non-Invasive Assessment of Meat Aging" Sensors 16, no. 1: 52. https://doi.org/10.3390/s16010052
APA StyleJilani, M. T., Wen, W. P., Cheong, L. Y., & Ur Rehman, M. Z. (2016). A Microwave Ring-Resonator Sensor for Non-Invasive Assessment of Meat Aging. Sensors, 16(1), 52. https://doi.org/10.3390/s16010052





