Modeling Electronic Skin Response to Normal Distributed Force
Abstract
:1. Introduction
2. Materials and Methods
2.1. Electronic Skin Materials and Structure
2.2. Electronic Skin Model
2.3. Simplified Analysis
2.3.1. Effect of Sensor Size on Sensor Response to a Single Normal Point Force
2.3.2. Effect of Normal Force Distributed Over Circular Contact Area on Point-Like Sensor Response
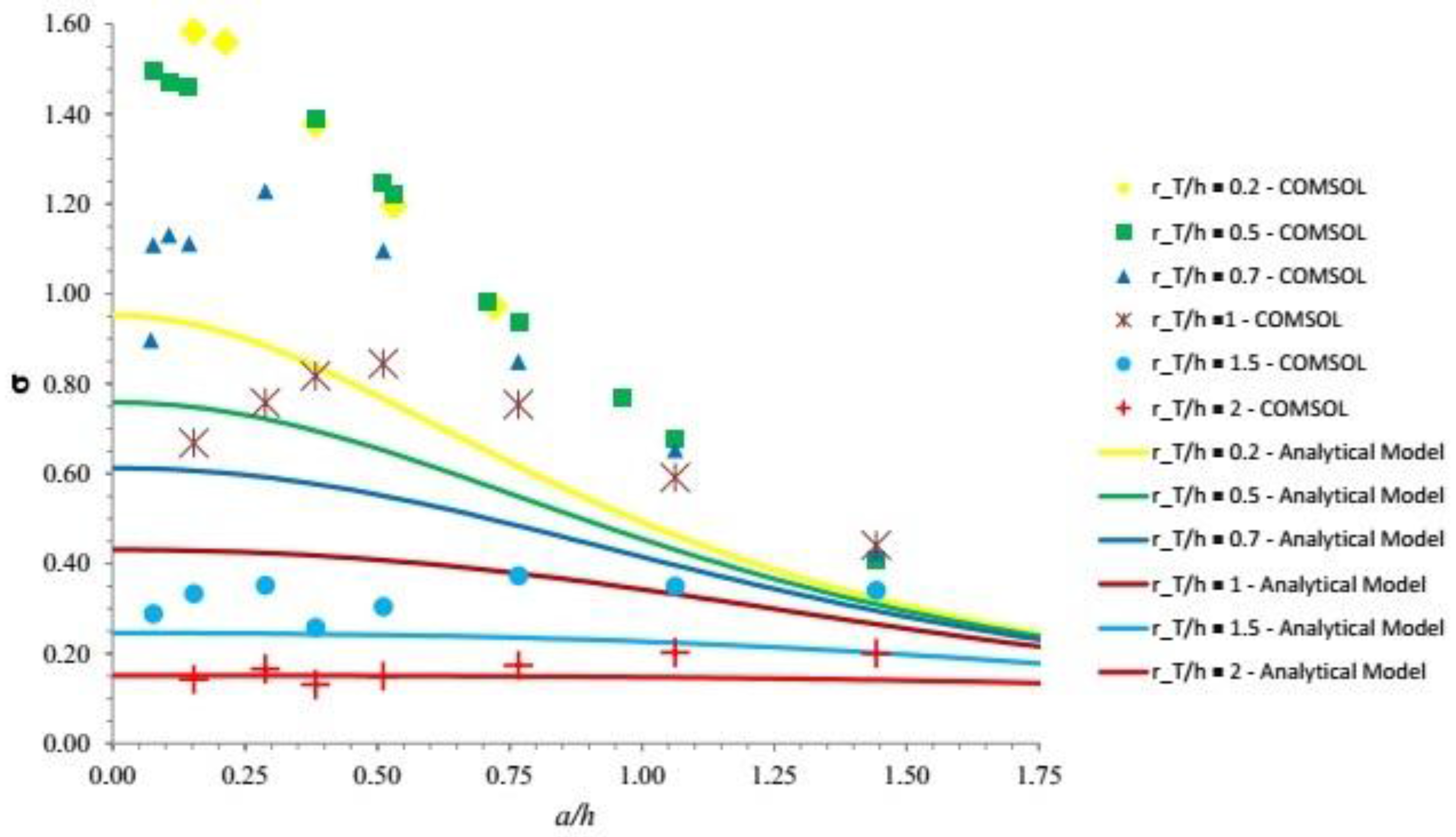
2.3.3. Combination of the Two Contributions: Effect of Distributed Normal Force on the Response of an Extended Sensor
2.4. FEM Simulations
3. Experimental Results and Discussion
3.1. Experimental Setup
3.2. Results
3.2.1. Characterization of the Elastic Properties of the Elastomer
3.2.2. Frequency Selection
3.2.3. Indenter Selection
3.2.4. Response Function of the Sensor Array
4. Conclusions
Acknowledgments
Conflicts of Interest
References
- Kim, J.; Lee, M.; Shim, H.J.; Ghaffari, R.; Cho, H.R.; Son, D.; Jung, Y.H.; Soh, M.; Choi, C.; Jung, S.; et al. Stretchable silicon nanoribbon electronics for skin prosthesis. Nat. Commun. 2014, 5, 5747. [Google Scholar] [CrossRef] [PubMed]
- Hammock, M.L.; Chortos, A.; Tee, B.C.-K.; Tok, J.B.-H.; Bao, Z. 25th Anniversary Article: The Evolution of Electronic Skin (E-Skin): A Brief History, Design Considerations, and Recent Progress. Adv. Mater. 2013, 25, 5997–6038. [Google Scholar] [CrossRef] [PubMed]
- Tiwana, M.I.; Redmond, S.J.; Lovell, N.H. A review of tactile sensing technologies with applications in biomedical engineering. Sens. Actuators A Phys. 2012, 179, 17–31. [Google Scholar] [CrossRef]
- Gerratt, A.P.; Michaud, H.O.; Lacour, S.P. Elastomeric Electronic Skin for Prosthetic Tactile Sensation. Adv. Funct. Mater. 2015, 25, 2287–2295. [Google Scholar] [CrossRef]
- Tawil, D.S.; Rye, D.; Velonaki, M. Interpretation of Social Touch on an Artificial Arm Covered with an EIT-based Sensitive Skin. J. Soc. Robot. 2014, 6, 489–505. [Google Scholar] [CrossRef]
- Kappassov, Z.; Corrales, J.-A.; Perdereau, V. Tactile sensing in dexterous robot hands—Review. Robot. Auton. Syst. 2015, 74, 195–220. [Google Scholar] [CrossRef]
- Dahiya, R.S.; Metta, G.; Valle, M.; Sandini, G. Tactile sensing—From humans to humanoids. IEEE Trans. Robot. 2010, 26, 1–20. [Google Scholar] [CrossRef]
- Yousef, H.; Boukallel, M.; Althoefer, K. Tactile sensing for dexterous in-hand manipulation in robotics—A review. Sens. Actuators A Phys. 2011, 167, 171–187. [Google Scholar] [CrossRef]
- De Rossi, D.; Canepa, G.; Magenes, G.; Germagnoli, F.; Caiti, A.; Parisini, T. Skin-like tactile sensor arrays for contact stress field extraction. Mater. Sci. Eng. C 1993, 1, 23–36. [Google Scholar] [CrossRef]
- Howe, R.D.; Cutkosky, M.R. Dynamic tactile sensing: Perception of fine surface features with stress rate sensing. IEEE Trans. Robot. Autom. 1993, 9, 140–151. [Google Scholar] [CrossRef]
- Shimojo, M. Mechanical filtering effect of elastic cover for tactile sensor. IEEE Trans. Robot. Autom. 1997, 13, 128–132. [Google Scholar] [CrossRef]
- Fearing, R.S. Tactile sensing mechanisms. Int. J. Robot. Res. 1990, 9, 3–23. [Google Scholar] [CrossRef]
- Fearing, R.S.; Hollerbach, J.M. Basic solid mechanics for tactile sensing. Int. J. Robot. Res. 1985, 4, 40–54. [Google Scholar] [CrossRef]
- Seminara, L.; Pinna, L.; Ibrahim, A.; Noli, L.; Caviglia, S.; Gastaldo, P.; Valle, M. Towards integrating intelligence in electronic skin. Mechatronics 2016, 34, 84–94. [Google Scholar] [CrossRef]
- Seminara, L.; Capurro, M.; Valle, M. Tactile data processing method for the reconstruction of contact force distributions. Mechatronics 2015, 27, 28–37. [Google Scholar] [CrossRef]
- Nalwa, H.S. Ferroelectric Polymers—Chemistry, Physics and Applications; Marcel Dekker Inc.: New York, NY, USA, 1995; pp. 203–214. [Google Scholar]
- IEEE Standard on Piezoelectricity. Available online: http://blogs.cimav.edu.mx/luis.fuentes/data/files/Curso_Cristalograf%C3%ADa/piezo_ieee.pdf (accessed on 1 February 2018).
- Pinna, L.; Valle, M. Charge amplifier design methodology for PVDF-based tactile sensors. J. Circuits Syst. Comput. 2013, 22, 1350066. [Google Scholar] [CrossRef]
- Boussinesq, J. Application des Potentielles à l’étude de l’équilibre et du Mouvement des Solides élastiques; Gauthier Villars: Paris, France, 1885. [Google Scholar]
- Johnson, K.L. Contact Mechanics; Cambridge University Press: Cambridge, UK, 1985. [Google Scholar]
- Seminara, L.; Capurro, M.; Cirillo, P.; Cannata, G.; Valle, M. Electromechanical characterization of piezoelectric PVDF polymer films for tactile sensors in robotics applications. Sens. Actuators A Phys. 2011, 169, 49–58. [Google Scholar] [CrossRef]
- Kuo Alex, C.M. Poly(dimethylsiloxane). In Polymer Data Handbook; Oxford University Press: Oxford, UK, 1999; pp. 411–435. [Google Scholar]
- Franceschi, M.; Seminara, L.; Pinna, L.; Dosen, S.; Farina, D.; Valle, M. Preliminary evaluation of the tactile feedback system based on artificial skin and electrotactile stimulation. In Proceedings of the 2015 37th Annual International Conference of the IEEE Engineering in Medicine and Biology Society (EMBC), Milan, Italy, 25–29 August 2015; pp. 4554–4557. [Google Scholar]
- Selvadurai, A.P.S. On Boussinesq’s problem. Int. J. Eng. Sci. 2001, 39, 317–322. [Google Scholar] [CrossRef]















© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Seminara, L. Modeling Electronic Skin Response to Normal Distributed Force. Sensors 2018, 18, 459. https://doi.org/10.3390/s18020459
Seminara L. Modeling Electronic Skin Response to Normal Distributed Force. Sensors. 2018; 18(2):459. https://doi.org/10.3390/s18020459
Chicago/Turabian StyleSeminara, Lucia. 2018. "Modeling Electronic Skin Response to Normal Distributed Force" Sensors 18, no. 2: 459. https://doi.org/10.3390/s18020459
APA StyleSeminara, L. (2018). Modeling Electronic Skin Response to Normal Distributed Force. Sensors, 18(2), 459. https://doi.org/10.3390/s18020459



