Discussion on Incentive Compatibility of Multi-Period Temporal Locational Marginal Pricing
Abstract
:1. Introduction
2. Temporal Locational Marginal Pricing
3. Numerical Testing on Incentive Compatibility of TLMP
3.1. Data for Numerical Testing
3.2. Incentive Compatibility of TLMP with Piecewise Linear Costs
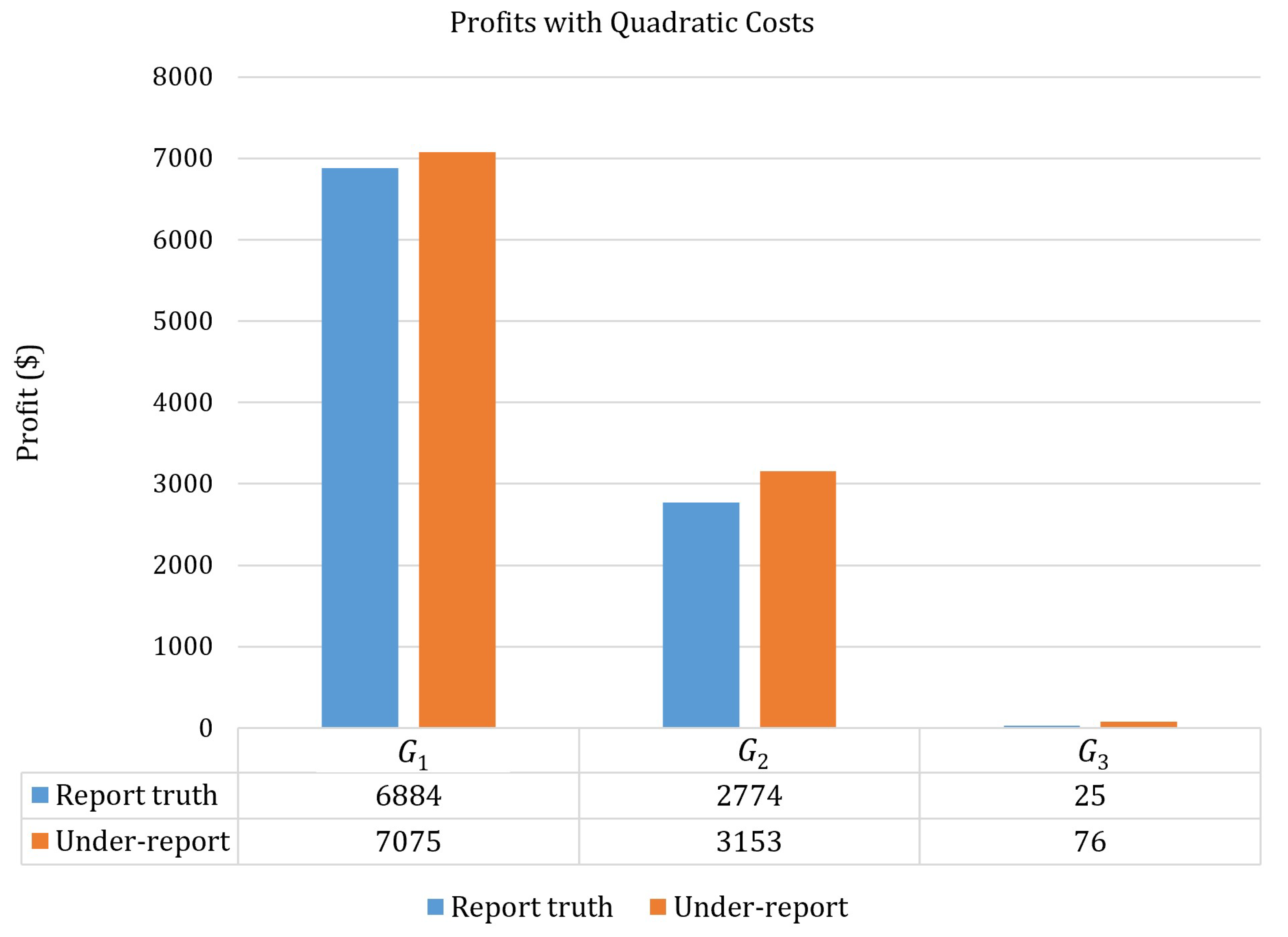
3.3. Incentive Compatibility of TLMP with Quadratic Costs
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- New York Independent System Operator, Inc. Market Administration and Control Area Services Tariff. 2022. Available online: https://nyisoviewer.etariff.biz/ViewerDocLibrary/MasterTariffs/9FullTariffNYISOMST.pdf (accessed on 21 April 2023).
- Corporation, C.I.S.O. California Independent System Operator Corporation Fifth Replacement. 2019. FERC electric tariff. Available online: http://www.caiso.com/Documents/Conformed-Tariff-asof-Sept28-2019.pdf (accessed on 21 April 2023).
- Tong, J.; Ni, H. Look-ahead multi-time frame generator control and dispatch method in PJM real time operations. In Proceedings of the 2011 IEEE Power and Energy Society General Meeting, Detroit, MI, USA, 24–29 July 2011; p. 1. [Google Scholar]
- Hogan, W.W. Electricity market design and zero-marginal cost generation. Curr. Sustain./Renew. Energy Rep. 2022, 9, 15–26. [Google Scholar] [CrossRef]
- Hogan, W.W. Electricity market design: Optimization and market equilibrium. In Proceedings of the Workshop on Optimization and Equilibrium in Energy Economics, Institute for Pure and Applied Mathematics, Los Angeles, CA, USA, 13 January 2016. [Google Scholar]
- Hua, B.; Schiro, D.A.; Zheng, T.; Baldick, R.; Litvinov, E. Pricing in multi-interval real-time markets. IEEE Trans. Power Syst. 2019, 34, 2696–2705. [Google Scholar] [CrossRef]
- Schiro, D.A. Flexibility procurement and reimbursement: A multi-period pricing approach. In Proceedings of the FERC Technical Conference, Washington, DC, USA, 26 June 2017. [Google Scholar]
- Zhao, J.; Zheng, T.; Litvinov, E. A multi-period market design for markets with intertemporal constraints. IEEE Trans. Power Syst. 2019, 35, 3015–3025. [Google Scholar] [CrossRef] [Green Version]
- Yang, Z.; Wang, Y.; Yu, J.; Yang, Y. On the minimization of uplift payments for multi-period dispatch. IEEE Trans. Power Syst. 2020, 35, 2479–2482. [Google Scholar] [CrossRef]
- Guo, Y.; Chen, C.; Tong, L. Pricing multi-interval dispatch under uncertainty part I: Dispatch-following incentives. IEEE Trans. Power Syst. 2021, 36, 3865–3877. [Google Scholar] [CrossRef]
- Chen, C.; Guo, Y.; Tong, L. Pricing multi-interval dispatch under uncertainty part II: Generalization and performance. IEEE Trans. Power Syst. 2020, 36, 3878–3886. [Google Scholar] [CrossRef]

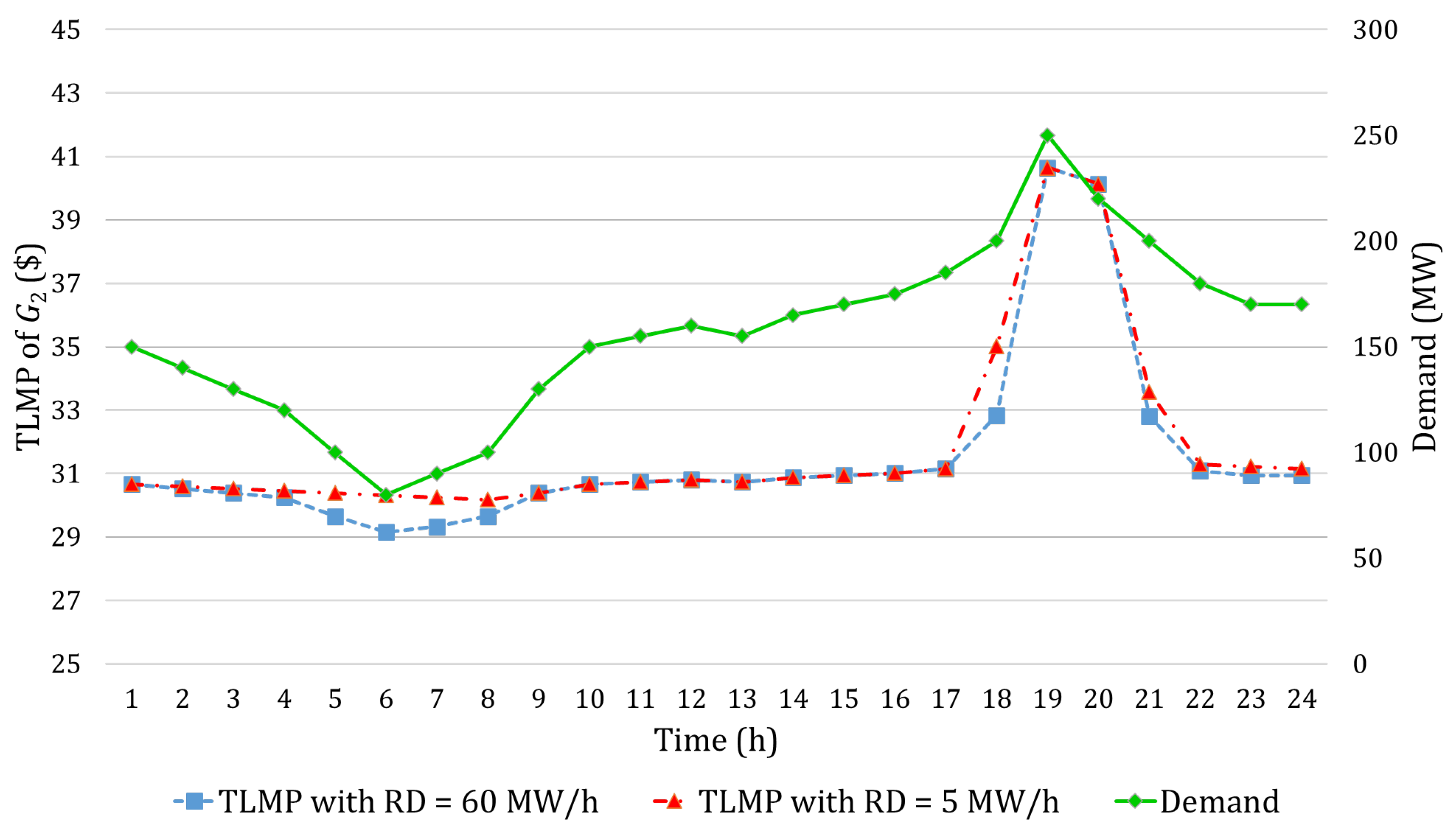
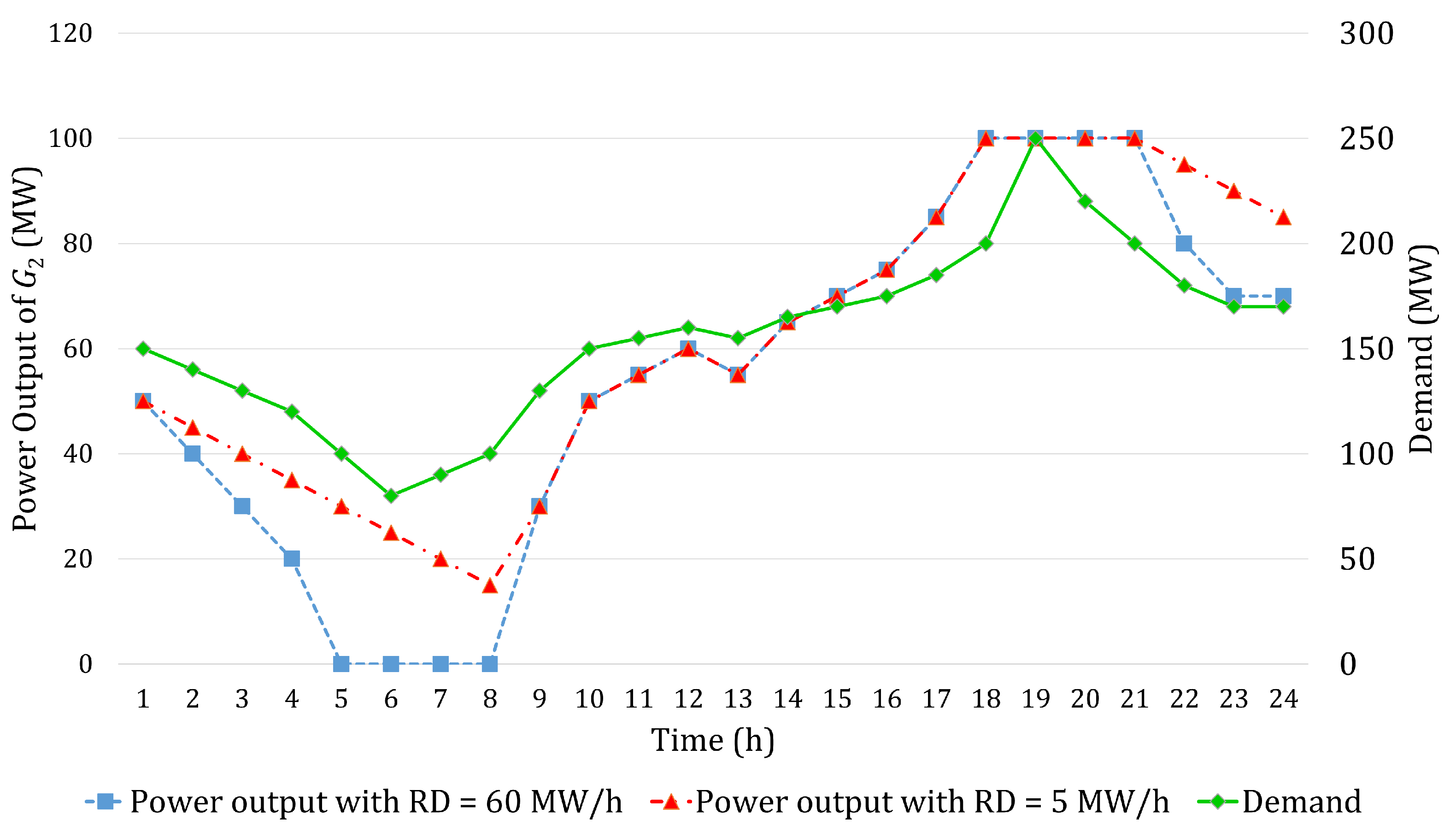
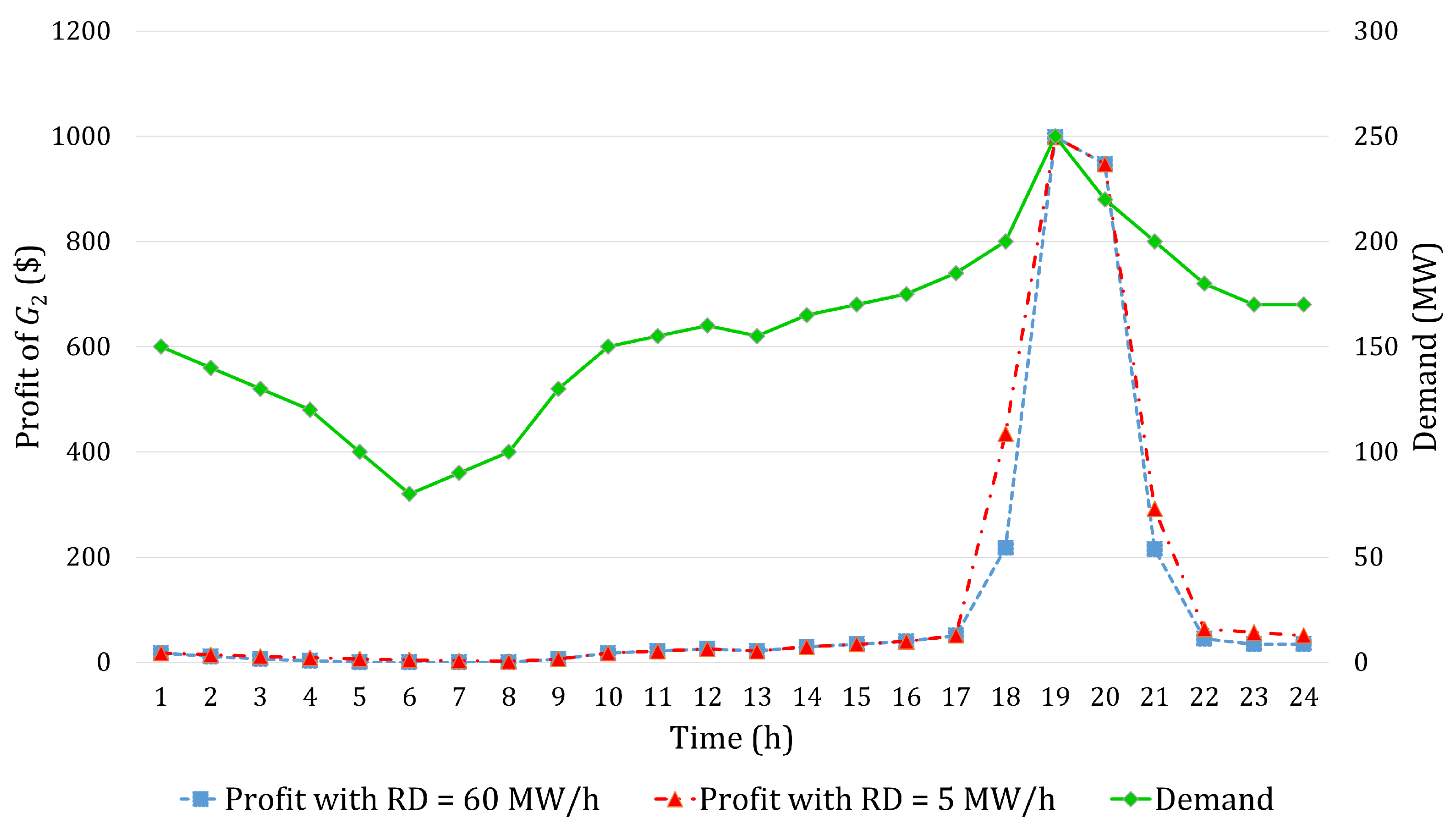
| G | Capacity (MW) | True Ramp Rate (MW/h) | Linear Costs ($/MW) | Piecewise Linear Costs ($/MW) | Quadratic Cost Functions ($) |
|---|---|---|---|---|---|
| 100 | 25 | 28 | (28,29) | 0.008568 + 28.7897g | |
| 100 | 60 | 30 | (30,31) | 0.007 + 29.9626g | |
| 100 | 60 | 40 | (40,41) | 0.008568 + 39.7897g |
| G | Report Truth | Under-Report | ||
|---|---|---|---|---|
| RD (MW/h) | Profit ($) | RD (MW/h) | Profit ($) | |
| 25 | 7460 | 5 | 8260 | |
| 60 | 3340 | 5 | 3420 | |
| 60 | 0 | 5 | 120 | |
| G | Report Truth | Under-Report | ||
|---|---|---|---|---|
| RD (MW/h) | Profit ($) | RD (MW/h) | Profit ($) | |
| 25 | 6884 | 5 | 7075 | |
| 60 | 2774 | 5 | 3153 | |
| 60 | 25 | 5 | 76 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hyder, F.; Yan, B.; Luh, P.; Bragin, M.; Zhao, J.; Zhao, F.; Schiro, D.; Zheng, T. Discussion on Incentive Compatibility of Multi-Period Temporal Locational Marginal Pricing. Energies 2023, 16, 4977. https://doi.org/10.3390/en16134977
Hyder F, Yan B, Luh P, Bragin M, Zhao J, Zhao F, Schiro D, Zheng T. Discussion on Incentive Compatibility of Multi-Period Temporal Locational Marginal Pricing. Energies. 2023; 16(13):4977. https://doi.org/10.3390/en16134977
Chicago/Turabian StyleHyder, Farhan, Bing Yan, Peter Luh, Mikhail Bragin, Jinye Zhao, Feng Zhao, Dane Schiro, and Tongxin Zheng. 2023. "Discussion on Incentive Compatibility of Multi-Period Temporal Locational Marginal Pricing" Energies 16, no. 13: 4977. https://doi.org/10.3390/en16134977







