Assessment of the Performance of Phasor-Based and Transients-Based Faulted Phase Identification Techniques in the Presence of Inverter Interfaced Resources
Abstract
1. Introduction
- Although there is a theoretical understanding of the reasons behind the phase selection problems due to IBR penetration, the extent of the issue is not well understood. A simulation-based analysis was carried out to validate the theoretical explanations and demonstrate the deficiencies of conventional faulted phase identification algorithms in the presence of IBRs.
- The phase selection performance of commercial relays under the presence of IBR is not well documented. Thus, a few samples of commercial relays were tested in a hardware-in-the-loop (HIL) simulation setup using an electromagnetic-transient (EMT) type digital real-time simulator (DRTS) to assess the performance and further validate the theoretical premise.
- Although alternative phase selection algorithms have been proposed in the recent literature to overcome the deficiencies of conventional phasor-based techniques, most of them are only verified by using offline simulations. In order to investigate the real-time applicability of two such methods, transient-based and incremental quantities-based faulted phase identification methods were implemented in hardware and tested using HIL simulations. The paper also highlighted practical considerations that require special attention while implementing the transients-based algorithm in real hardware.
- A true direct comparison of the real-time performance of the commercial relays and novel phase selection algorithms was lacking. Therefore, the performance of traditional and transient/incremental quantities-based protection algorithms was systematically compared under the presence of IBRs, leading to clear conclusions.
2. Theoretical Analysis of Contemporary Faulted Phase Selection Algorithms and the Effect of IBRs
2.1. Contemporary Faulted Phase Selection Algorithms
2.2. Effect of IBR on Faulted Phase Selection Techniques
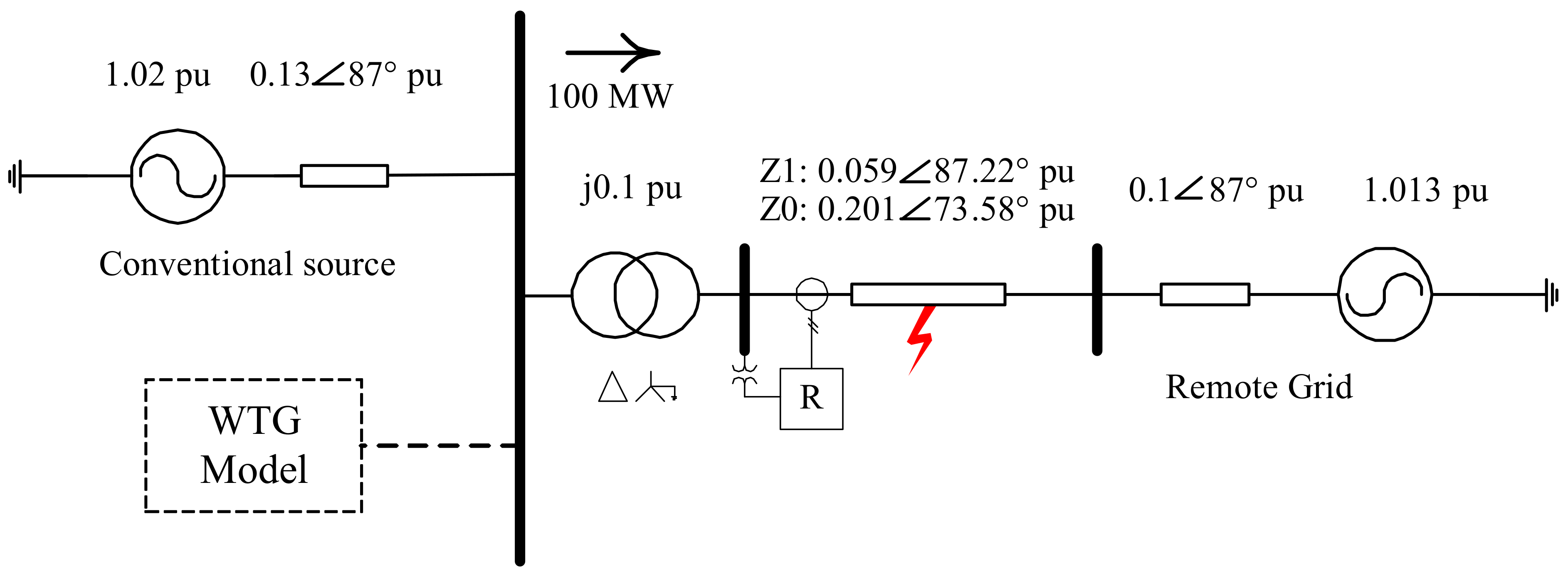
3. Testing and Analysis
3.1. Performance of Commercial Relays
3.1.1. Test Setup
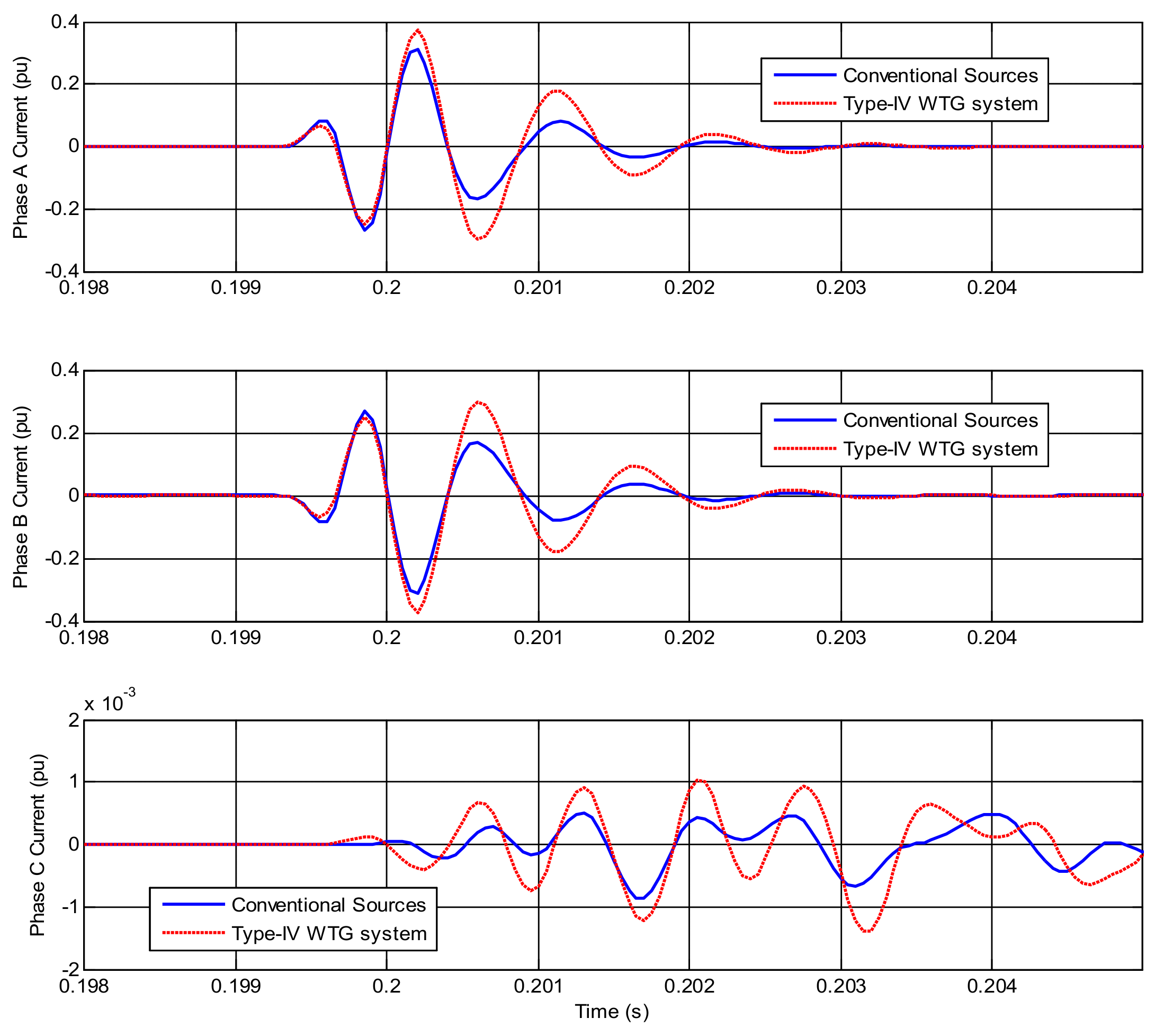
3.1.2. Test Results and Discussion
3.2. Transient-Based and Incremental Quantity-Based Fault Classification Schemes
3.2.1. Transient Based Faulted Phase Selection Algorithm
3.2.2. Incremental Quantity Based Faulted Phase Classification
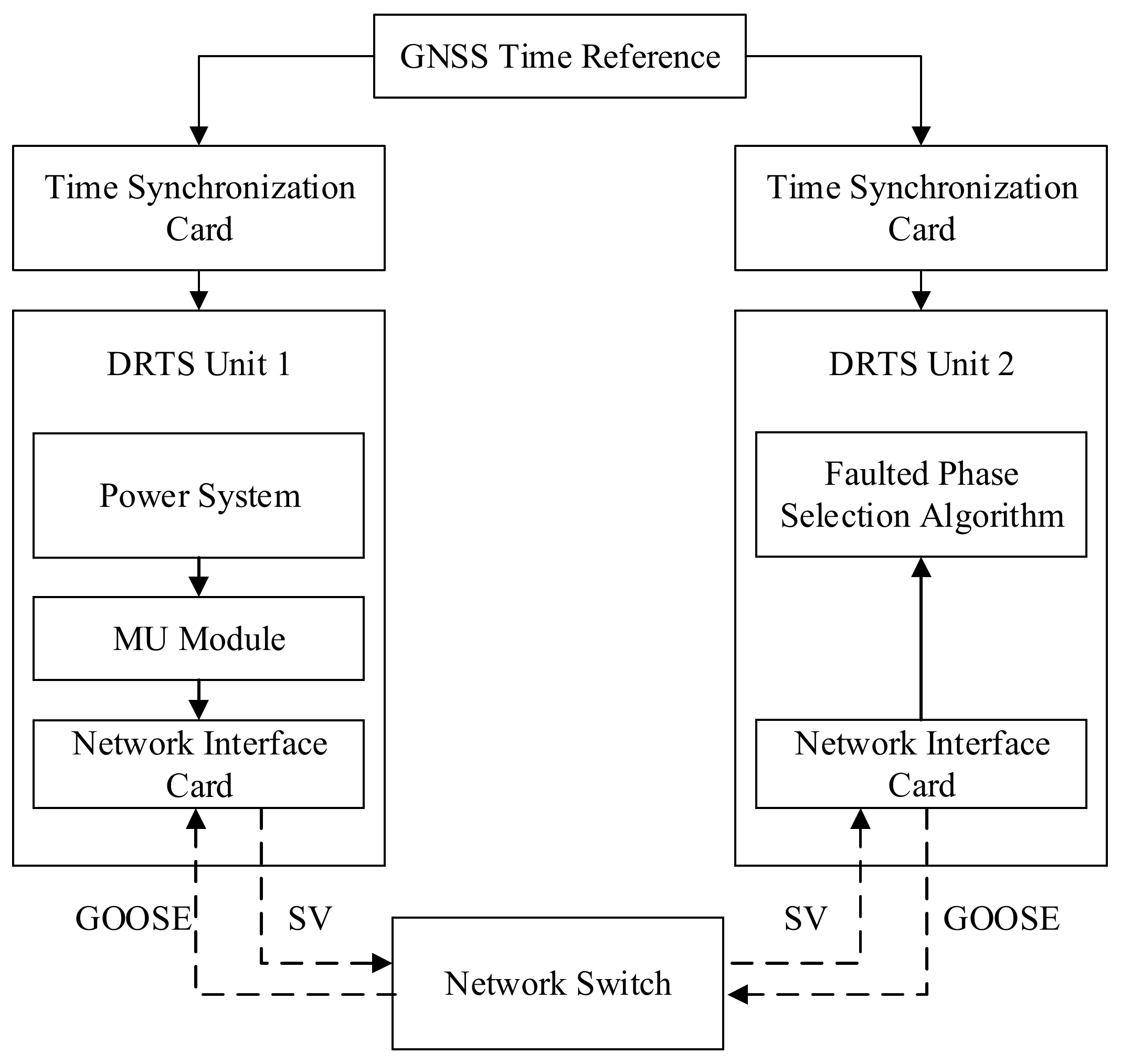
3.2.3. Test Setup and the Implementation of Algorithms
3.2.4. Test Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
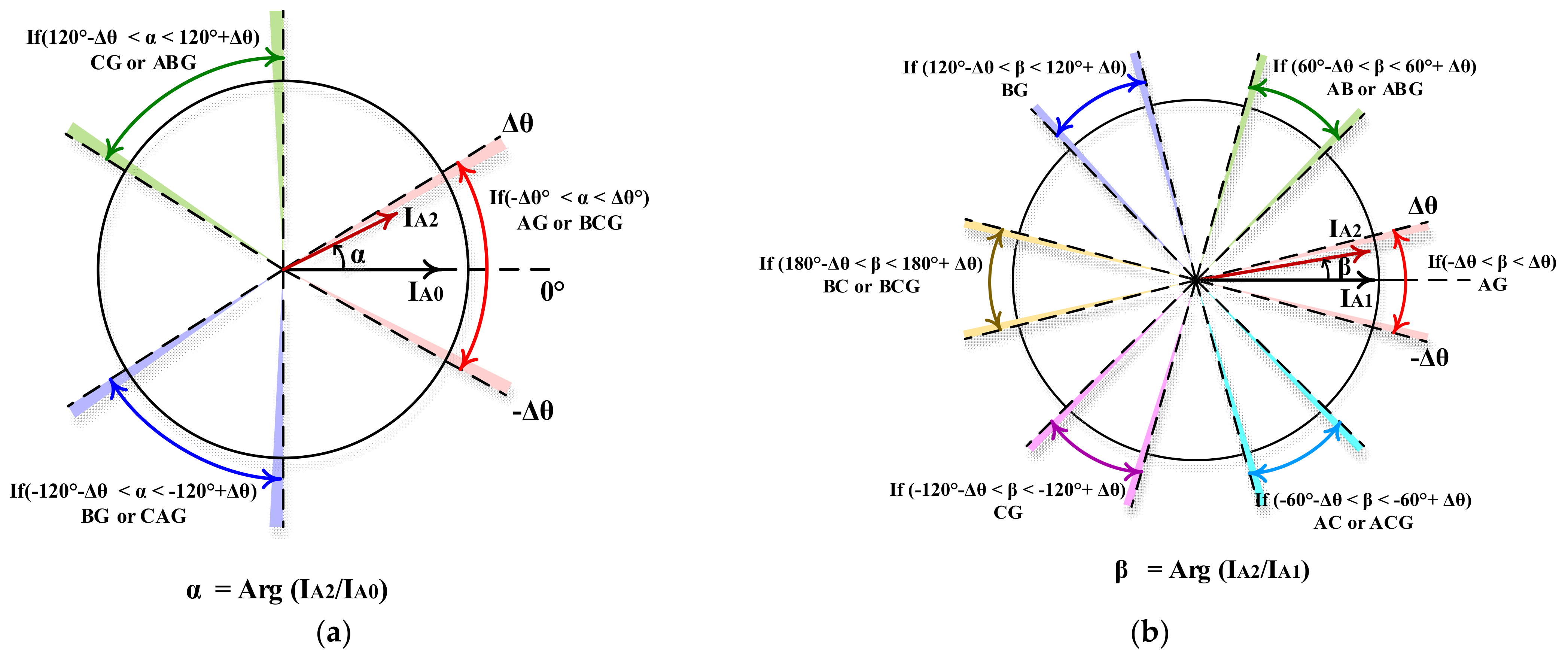
Appendix A. Use of an Overreaching Impedance Zone for Phase Identification

Appendix B. Transient Based Faulted Phase Identification Algorithm
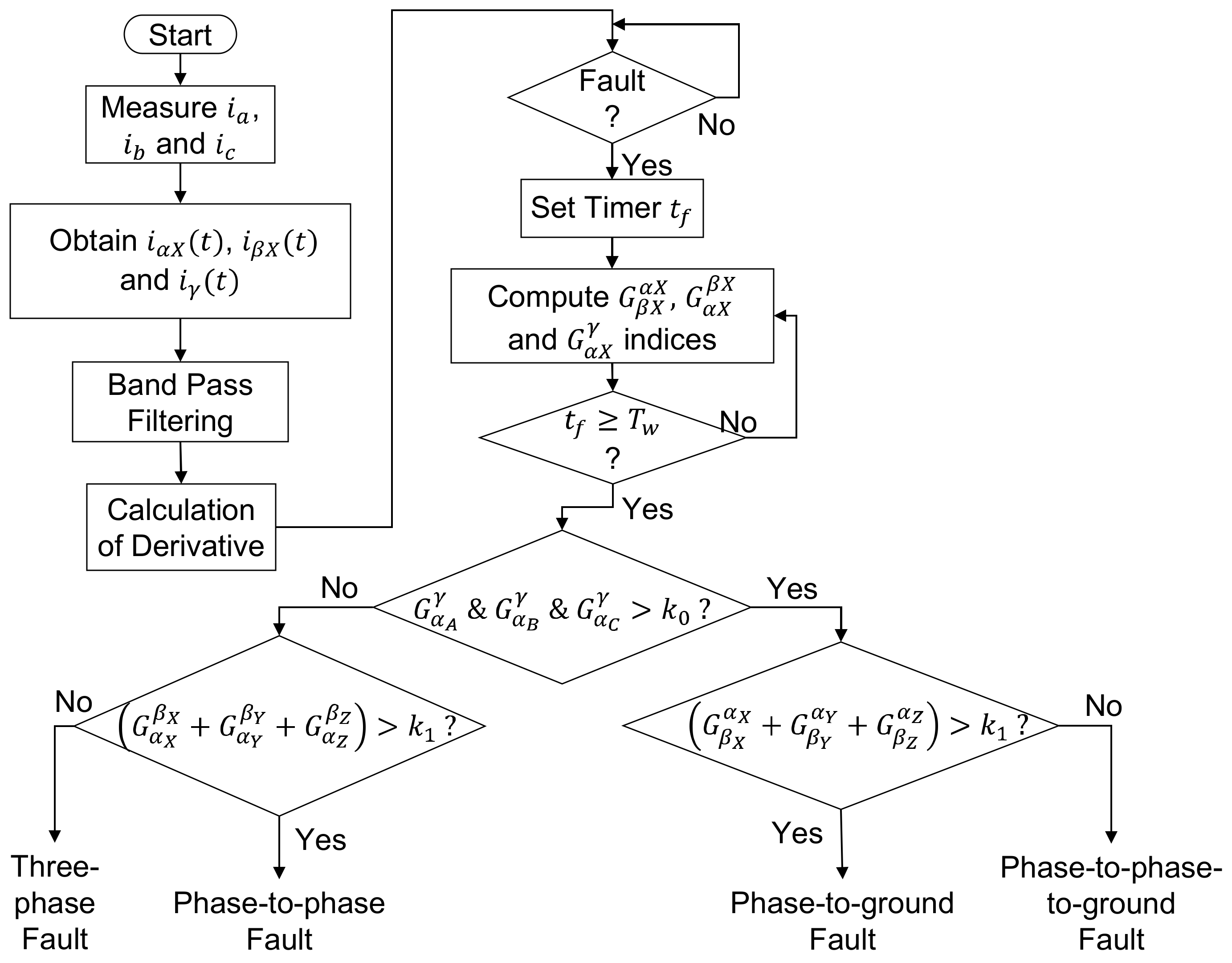
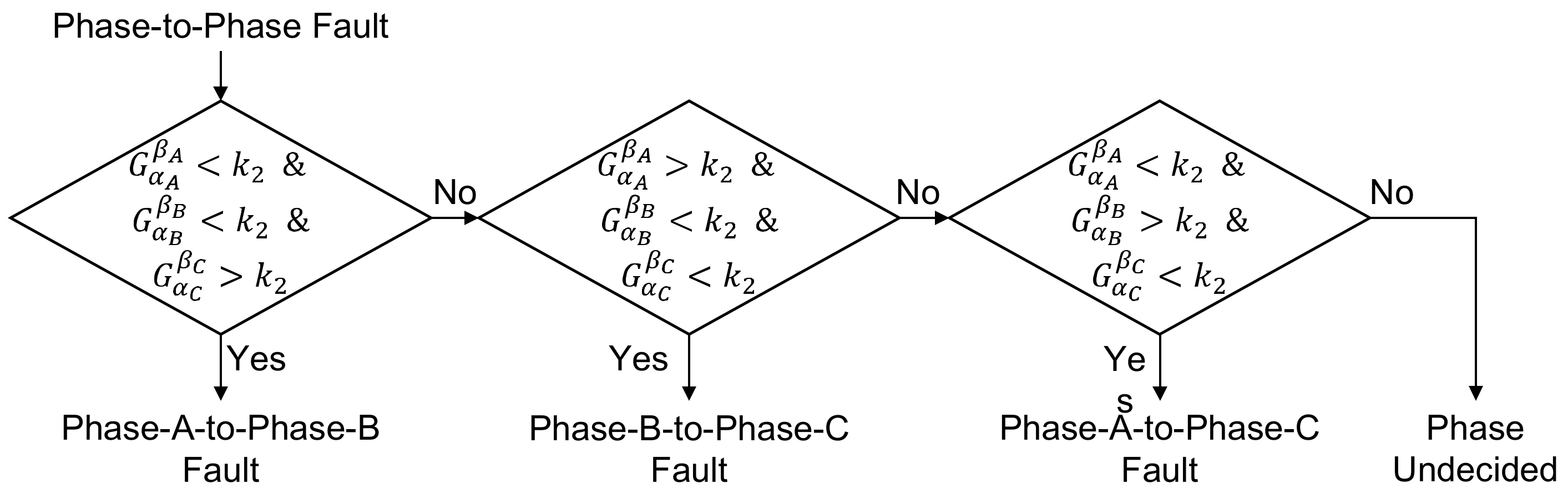
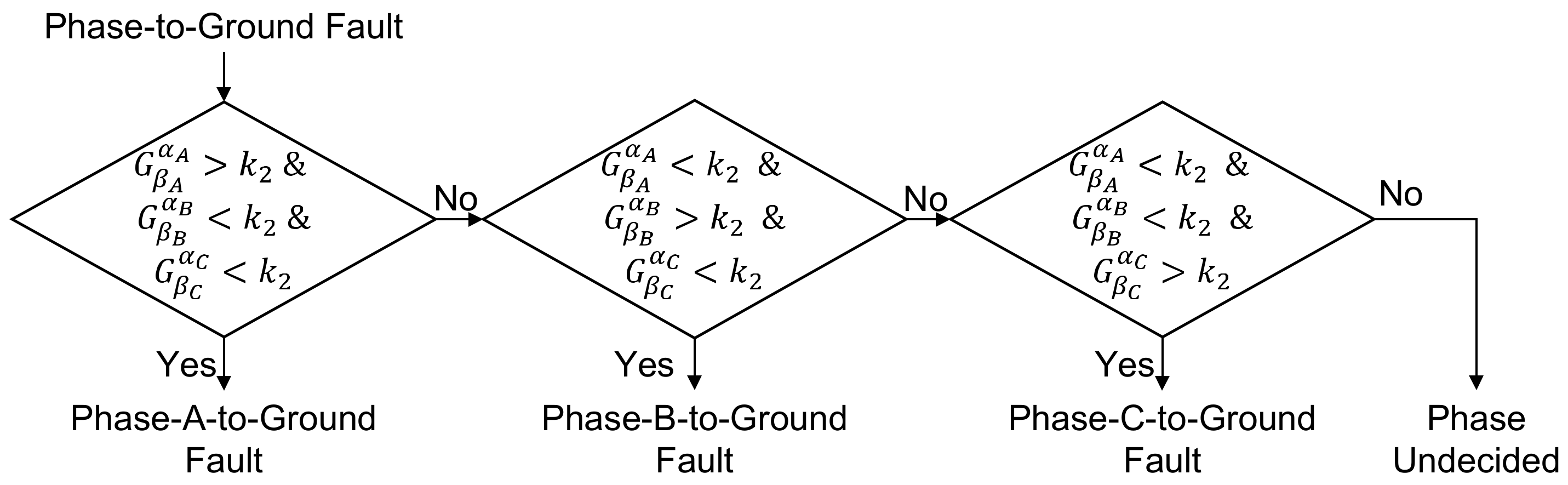

Appendix C. Incremental Quantity Based Phase Selection Algorithm
References
- Nagpal, M.; Jensen, M.; Higginson, M. IEEE Power System Relaying and Control Committee Report of Working Group C32 of the System Protection Subcommittee. Protection Challenges and Practices for Interconnecting Inverter Based Resources to Utility Transmission Systems. September 2020. Available online: https://www.pes-psrc.org/kb/published/reports/Working%20Group%20C32%20Report%20Draft%208.05%20-%20Final%20-%20PSRCC%20Format.pdf (accessed on 27 December 2022).
- Brahma, S.; Farantatos, E. IEEE Power System Relaying and Control Committee Report of Working Group C24 of the System Protection Subcommittee. Modification of Commercial Fault Calculation Programs for Wind Turbine Generators. July 2020. Available online: https://www.pes-psrc.org/kb/published/reports/C24_WG_Report_Jun_2020_Final.pdf (accessed on 27 December 2022).
- NERC. 1200 MW Fault Induced Solar Photovoltaic Resource Interruption Disturbance Report—Southern California 8/16/2016 Event; NERC: Atlanta, GA, USA, 2017. [Google Scholar]
- Telukunta, V.; Pradhan, J.; Agrawal, A.; Singh, M.; Srivani, S.G. Protection challenges under bulk penetration of renewable energy resources in power systems: A review. CSEE J. Power Energy Syst. 2017, 3, 365–379. [Google Scholar] [CrossRef]
- Haddadi, A.; Kocar, I.; Farantatos, E. Impact of Inverter-Based Resources on Protection Schemes Based on Negative Sequence Components; EPRI: Palo Alto, CA, USA, 2019. [Google Scholar]
- Teodorescu, R.; Liserre, M.; Rodriguez, P. Grid Converters for Photovoltaic and Wind Power Systems; Wiley-IEEE Press: Hoboken, NJ, USA, 2007. [Google Scholar]
- Jia, K.; Yang, Z.; Fang, Y.; Bi, T.; Sumner, M. Influence of Inverter-Interfaced Renewable Energy Generators on Directional Relay and an Improved Scheme. IEEE Trans. Power Electron. 2019, 34, 11843–11855. [Google Scholar] [CrossRef]
- Fang, Y.; Jia, K.; Yang, Z.; Li, Y.; Bi, T. Impact of Inverter-Interfaced Renewable Energy Generators on Distance Protection and an Improved Scheme. IEEE Trans. Ind. Electron. 2018, 66, 7078–7088. [Google Scholar] [CrossRef]
- Keller, J.; Kroposki, B. Understanding Fault Characteristics of Inverter-Based Distributed Energy Resources; Technical Report NREL/TP-550-46698; NREL: Golden, CO, USA, 2010. [Google Scholar]
- Haddadi, A.; Zhao, M.; Kocar, I.; Karaagac, U.; Chan, K.W.; Farantatos, E. Impact of Inverter-Based Resources on Negative Sequence Quantities-Based Protection Elements. IEEE Trans. Power Deliv. 2021, 36, 289–298. [Google Scholar] [CrossRef]
- Haddadi, A.; Farantatos, E.; Kocar, I.; Karaagac, U. Impact of inverter based resources on system protection. Energies 2021, 14, 1050. [Google Scholar] [CrossRef]
- Hooshyar, A.; Azzouz, M.; El-Saadany, E. Distance Protection of Lines Connected to Induction Generator-Based Wind Farms During Balanced Faults. IEEE Trans. Sustain. Energy 2014, 5, 1193–1203. [Google Scholar] [CrossRef]
- Chowdhury, R.; Fischer, N. Transmission Line Protection for Systems With Inverter-Based Resources—Part I: Problems. IEEE Trans. Power Deliv. 2021, 36, 2416–2425. [Google Scholar] [CrossRef]
- Chowdhury, R.; Fischer, N. Transmission Line Protection for Systems With Inverter-Based Resources—Part II: Solutions. IEEE Trans. Power Deliv. 2021, 36, 2426–2433. [Google Scholar] [CrossRef]
- Irwin, G.D.; Jindal, A.K.; Isaacs, A.L. Sub-synchronous control interactions between type 3 wind turbines and series compensated AC transmission systems. In Proceedings of the IEEE Power and Energy Society General Meeting, Detroit, MI, USA, 24–28 July 2011. [Google Scholar]
- Costello, D.; Zimmerman, K. Determining the Faulted Phase. In Proceedings of the 36th Annual Western Protective Relay Conference, Spokane, WA, USA, 20–22 October 2009. [Google Scholar]
- Carrasco, E.M.; Moreno, M.P.C.; Martínez, M.T.V.; Vicente, S.B. Improved Faulted Phase Selection Algorithm for Distance Protection under High Penetration of Renewable Energies. Energies 2020, 13, 558. [Google Scholar] [CrossRef]
- Kasztenny, B.; Campbell, B.; Mazereeuw, J. Phase Selection for Single-Pole Tripping—Weak Infeed Conditions and Cross-Country Faults. In Proceedings of the 27th Annual Western Protective Relay Conference, Spokane, WA, USA, 24–26 October 2000. [Google Scholar]
- Price, E.; Warren, B. The Performance of Faulted Phase Selectors used in Transmission Line Distance Applications. In Proceedings of the 61st Annual Conference for Protective Relay Engineers, College Station, TX, USA, 1–3 April 2008; pp. 484–490. [Google Scholar]
- L-PRO 4000 Transmission Line Protection Relay, Manual, ERLPhase Power Technologies Ltd. Available online: https://www.erlphase.com/downloads/manuals/L_PRO_4000_manual.pdf (accessed on 27 December 2022).
- Wijekoon, J.; Rajapakse, A.D.; Haleem, N.M. Fast and Reliable Method for Identifying Fault Type and Faulted Phases Using Band Limited Transient Currents. IEEE Trans. Power Deliv. 2020, 36, 2839–2850. [Google Scholar] [CrossRef]
- Dong, X.; Kong, W.; Cui, T. Fault Classification and Faulted-Phase Selection Based on the Initial Current Traveling Wave. IEEE Trans. Power Deliv. 2008, 24, 552–559. [Google Scholar] [CrossRef]
- Namdari, F.; Salehi, M. High-Speed Protection Scheme Based on Initial Current Traveling Wave for Transmission Lines Employing Mathematical Morphology. IEEE Trans. Power Deliv. 2017, 32, 246–253. [Google Scholar] [CrossRef]
- Silva, N.S.D.B.; M, K.; Souza, B.A. Fault Detection and Classification in Transmission Line Using Wavelet Transform and ANN. IEEE Trans. Power Deliv. 2006, 21, 2058–2063. [Google Scholar] [CrossRef]
- Abdullah, A. Ultrafast Transmission Line Fault Detection Using a DWT-Based ANN. IEEE Trans. Ind. Appl. 2017, 54, 1182–1193. [Google Scholar] [CrossRef]
- He, Z.; Fu, L.; Lin, S.; Bo, Z. Fault Detection and Classification in EHV Transmission Line Based on Wavelet Singular Entropy. IEEE Trans. Power Deliv. 2010, 25, 2156–2163. [Google Scholar] [CrossRef]
- Liu, Y.; Xie, D.; Xiao, W.; Wang, W.; Zheng, Y. Research of fault phase selection on UHV transmission lines based on wavelet analysis. In Proceedings of the 2016 International Conference on Smart Grid and Clean Energy Technologies, ICSGCE 2016, Chengdu, China, 19–22 October 2016; pp. 284–289. [Google Scholar]
- Paladhi, S.; Pradhan, A.K. Adaptive Fault Type Classification for Transmission Network Connecting Converter-Interfaced Renewable Plants. IEEE Syst. J. 2020, 15, 4025–4036. [Google Scholar] [CrossRef]
- IEC 61400-27-1; Wind Energy Generation—Part 27-1: Electrical Simulation Models—Generic Models. IEC: Geneva, Switzerland, 2020.
- IEC 61850-9-2; IEC Standard for Communication Networks and Systems for Power Utility Automation, Part 9-2: Specific Communication Service Mapping (SCSM)—Sampled Values over ISO/IEC 8802-3’. IEC: Geneva, Switzerland, 2011.
- IEC 61869-9; Instrument Transformers—Part 9: Digital Interface for Instrument Transformers’. IEC: Geneva, Switzerland, 2016.
- UCA International Users Group. Implementation Guideline for DIGITAL Interface to Instrument Transformers Using IEC 61850-9-2; UCA International Users Group: Raleigh, NC, USA, 2004. [Google Scholar]
- IEC 61850-8-1; IEC Standard for Communication Networks and Systems for Power Utility Automation—Part 8-1: Specific Communication Service Mapping (SCSM—Mappings to MMS (ISO 9506-1 and ISO 9506-2) and to ISO/IEC 8802-3’. IEC: Geneva, Switzerland, 2011.




| Applied Fault | Fault Type Identification | ||
|---|---|---|---|
| Type | Location | Relay 1 | Relay 2 |
| AG | 5% | Unreliable | Does not identify * |
| 50% | Unreliable | Does not identify * | |
| 75% | Unreliable | Does not identify * | |
| BC | 5% | BC | Does not identify * |
| 50% | BC | Does not identify * | |
| 75% | BC | Does not identify * | |
| ACG | 5% | ACG | Does not identify * |
| 50% | ACG | Does not identify * | |
| 75% | ACG | Does not identify * | |
| Applied Fault | Fault Type Identification | ||
|---|---|---|---|
| Type | Location | Relay 1 | Relay 2 |
| AG | 5% | Does not identify | Does not identify |
| 50% | Does not identify | Does not identify | |
| 75% | Does not identify | Does not identify | |
| BC | 5% | Does not identify | Does not identify |
| 50% | Does not identify | Does not identify | |
| 75% | Does not identify | Does not identify | |
| ACG | 5% | Does not identify | Does not identify |
| 50% | Does not identify | Does not identify | |
| 75% | Does not identify | Does not identify | |
| Applied Fault | Number of Cases | Correct Identification | Accuracy (%) | ||
|---|---|---|---|---|---|
| Relay 1 | Relay 2 | Relay 1 | Relay 2 | ||
| AG | 1086 | 261 | 0 | 24.03 | 0 |
| BG | 1086 | 373 | 0 | 34.34 | 0 |
| CG | 1086 | 275 | 0 | 25.32 | 0 |
| Total | 3258 | 909 | 0 | 27.9 | 0 |
| ABG | 1086 | 464 | 0 | 42.72 | 0 |
| ACG | 1086 | 540 | 0 | 49.72 | 0 |
| BCG | 1086 | 542 | 0 | 49.90 | 0 |
| Total | 3258 | 1546 | 0 | 47.45 | 0 |
| AB | 1086 | 543 | 0 | 50.0 | 0 |
| AC | 1086 | 543 | 0 | 50.0 | 0 |
| BC | 1086 | 543 | 0 | 50.0 | 0 |
| ABC | 1086 | 543 | 0 | 50.0 | 0 |
| Total | 4344 | 2172 | 0 | 50.0 | 0 |
| Synchronous Generator Apparent Power | Applied Fault | Fault Type Identification | |
|---|---|---|---|
| Relay 1 | Relay 2 | ||
| 10 MVA | AG | Unreliable | AG |
| BCG | BCG | ABC | |
| CA | CA | ABC | |
| 15 MVA | AG | AG | AG |
| BCG | BCG | ABC | |
| CA | CA | CA | |
| 20 MVA | AG | AG | AG |
| BCG | BCG | BCG | |
| CA | CA | CA | |
| Applied Fault | Fault Type Identification (Transient-Based) | Fault Type Identification (Incremental Quantities-Based) | |||
|---|---|---|---|---|---|
| Type | Location | Bolted Fault | High Impedance Fault | Bolted Fault | High Impedance Fault |
| AG | 5% | AG | AG | AG | AG |
| 50% | AG | AG | AG | AG | |
| 75% | AG | AG | AG | AG | |
| BC | 5% | BC | BC | BC | BC |
| 50% | BC | BC | BC | BC | |
| 75% | BC | BC | BC | BC | |
| ACG | 5% | ACG | ACG | ACG | ACG |
| 50% | ACG | ACG | ACG | ACG | |
| 75% | ACG | ACG | ACG | ACG | |
| Applied Fault | Number of Cases | Transient Based Method | Incremental Component Based Method | ||
|---|---|---|---|---|---|
| Correctly Identified Cases | Accuracy (%) | Correctly Identified Cases | Accuracy (%) | ||
| AG | 1086 | 1062 | 97.79 | 1086 | 100 |
| BG | 1086 | 1067 | 98.25 | 1086 | 100 |
| CG | 1086 | 1062 | 97.29 | 1086 | 100 |
| Total | 3258 | 3191 | 97.94 | 3258 | 100 |
| ABG | 1086 | 796 | 73.29 | 1086 | 100 |
| ACG | 1086 | 813 | 74.86 | 1086 | 100 |
| BCG | 1086 | 811 | 74.67 | 1086 | 100 |
| Total | 3258 | 2420 | 74.28 | 3258 | 100 |
| AB | 1086 | 1079 | 99.36 | 1086 | 100 |
| AC | 1086 | 1080 | 99.44 | 1086 | 100 |
| BC | 1086 | 1080 | 99.45 | 1086 | 100 |
| ABC | 1086 | 1028 | 94.66 | 1086 | 100 |
| Total | 4344 | 4267 | 98.22 | 4344 | 100 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kariyawasam, S.; Wijekoon, J.; Rajapakse, A. Assessment of the Performance of Phasor-Based and Transients-Based Faulted Phase Identification Techniques in the Presence of Inverter Interfaced Resources. Energies 2023, 16, 640. https://doi.org/10.3390/en16020640
Kariyawasam S, Wijekoon J, Rajapakse A. Assessment of the Performance of Phasor-Based and Transients-Based Faulted Phase Identification Techniques in the Presence of Inverter Interfaced Resources. Energies. 2023; 16(2):640. https://doi.org/10.3390/en16020640
Chicago/Turabian StyleKariyawasam, Sachintha, Jagannath Wijekoon, and Athula Rajapakse. 2023. "Assessment of the Performance of Phasor-Based and Transients-Based Faulted Phase Identification Techniques in the Presence of Inverter Interfaced Resources" Energies 16, no. 2: 640. https://doi.org/10.3390/en16020640
APA StyleKariyawasam, S., Wijekoon, J., & Rajapakse, A. (2023). Assessment of the Performance of Phasor-Based and Transients-Based Faulted Phase Identification Techniques in the Presence of Inverter Interfaced Resources. Energies, 16(2), 640. https://doi.org/10.3390/en16020640








