Formation Rate and Energy Efficiency of Ice Plug in Pipelines Driven by the Cascade Utilization of Cold Energy
Abstract
:1. Introduction
2. Numerical Simulations
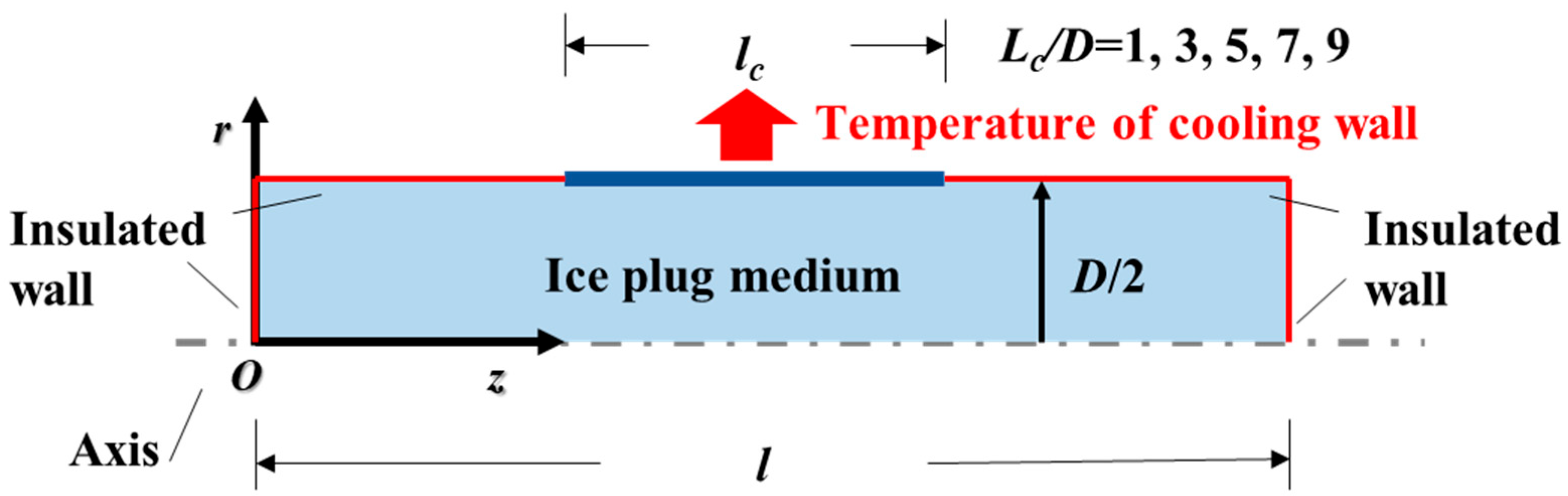
2.1. Physical Model
2.2. Mathematical Model
2.3. Numerical Method
3. Results
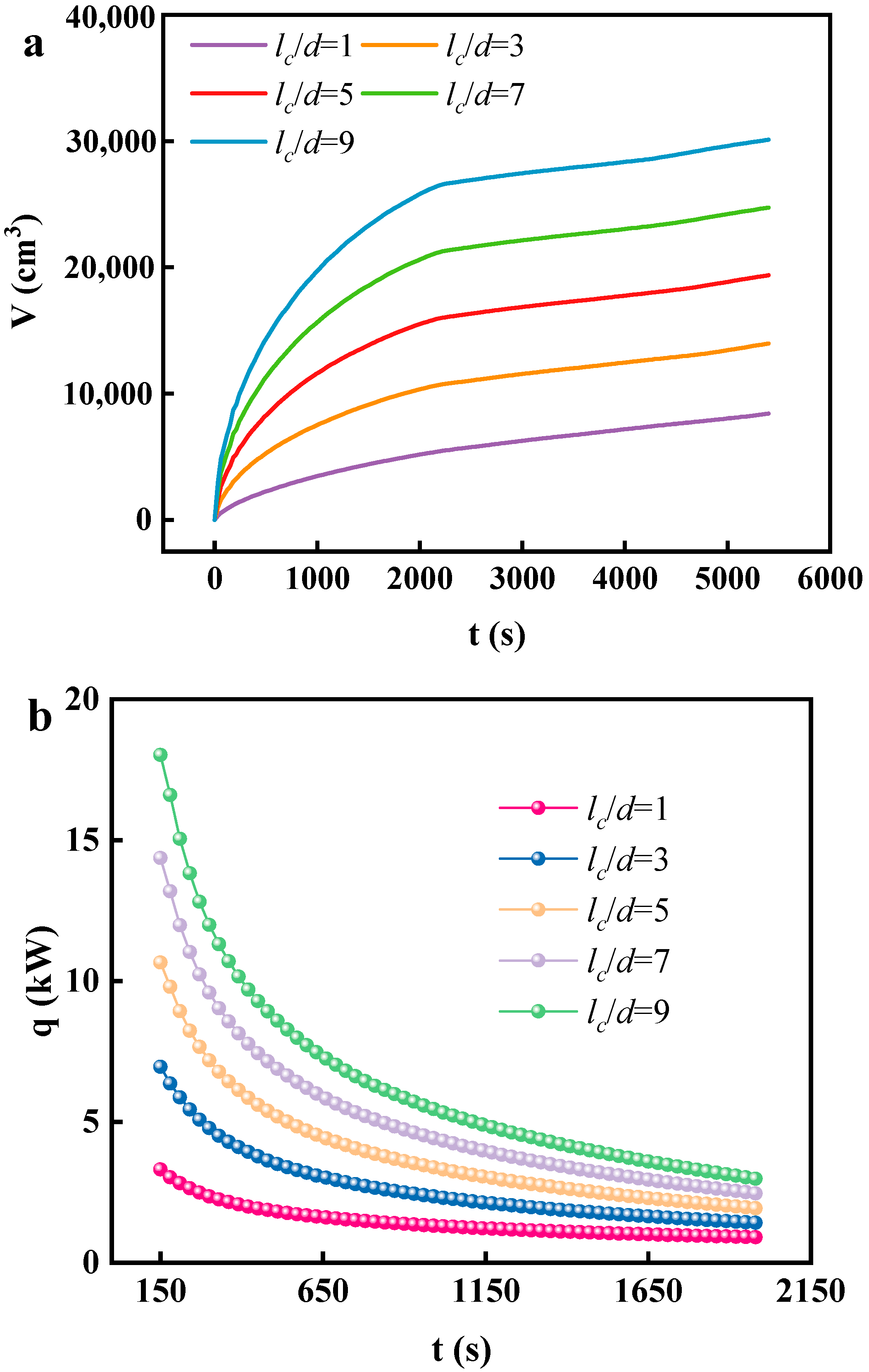
3.1. Effects of the Ratio of Length and Diameter lc/d on the Formation of Ice Plug
3.2. Effects of the Multi-Stage Temperature of Cooling Wall on Formation Rate
3.3. Effects of the Multi-Stage Temperature on Cold Energy Consumption
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Meng, N.; Li, T.; Gao, X.; Liu, Q.; Li, X.; Gao, H. Thermodynamic and techno-economic performance comparison of two-stage series organic Rankine cycle and organic Rankine flash cycle for geothermal power generation from hot dry rock. Appl. Therm. Eng. 2022, 200, 117715. [Google Scholar] [CrossRef]
- Messelier-Gouze, C.; Brézillon, C.; Tubiana, A.; Rousseau, G. Justification of the Use of Liquid Nitrogen to Make Ice-Plugs in Carbon-Manganese Steel Pipes for Maintenance Purposes in Nuclear Plants. In Proceedings of the ASME 2002 Pressure Vessels and Piping Conference, Vancouver, BC, Canada, 5–9 August 2002. [Google Scholar]
- Zhang, X.; Zhang, Y.; Wang, J. Evaluation and selection of dry and isentropic working fluids based on their pump performance in small-scale organic Rankine cycle. Appl. Therm. Eng. 2021, 191, 116919. [Google Scholar] [CrossRef]
- Khater, A.M.; Soliman, A.; Ahmed, T.S.; Ismail, I.M. Power generation in white cement plants from waste heat recovery using steam-organic combined Rankine cycle. Case Stud. Chem. Environ. Eng. 2021, 4, 100138. [Google Scholar] [CrossRef]
- Xu, H.; Bbosa, B.; Pereyra, E.; Volk, M.; Mannan, M.S. Oil transportation in pipelines with the existence of ice. J. Loss Prev. Process Ind. 2018, 56, 137–146. [Google Scholar] [CrossRef]
- Takefuj, Y.; Okubo, T. Double-ice-plug freezing using liquid nitrogen for water pipe repairs. Urban Water J. 2018, 15, 97–99. [Google Scholar] [CrossRef]
- Jain, A.; Miglani, A.; Huang, Y.; Weibel, J.A.; Garimella, S.V. Ice formation modes during flow freezing in a small cylindrical channel. Int. J. Heat Mass Transf. 2018, 128, 836–848. [Google Scholar] [CrossRef]
- Corbescu, B.; Puiu, D.; Gyongyosi, T.; Panaitescu, V. Forming an ice plug inside a high diameter pipeline in stationary water using a nitrogen vapour exhaust restriction. J. Phys. Conf. Ser. 2018, 2018, 012002. [Google Scholar] [CrossRef]
- Dong, W.; Ding, J.; Zhou, Z.X. Experimental Study on the Ice Freezing Adhesive Characteristics of Metal Surfaces. J. Aircr. 2014, 51, 719–726. [Google Scholar] [CrossRef]
- Cruz, J.; Davis, B.; Gramann, P. A Study of the Freezing Phenomena in PVC and CPVC Pipe Systems. In Proceedings of the Annual Technical Conference of the Society of Plastics Engineers 2010, Orlando, FL, USA, 16–20 May 2010; pp. 201–206. [Google Scholar]
- Bowen, R.J. An Experimental Study of Ice Formation in Pipes. Ph.D. Thesis, University of Southampton, Southampton, UK, 2000. [Google Scholar]
- Xie, L.; Wang, C.; Zhang, W.; Shen, X.; Hu, M.; Bian, C.; Xu, Y. Stress Distribution and Safety Evaluation of Pipeline in Ice Plug of Nuclear Power. Nucl. Sci. Eng. 2021, 196, 221–233. [Google Scholar] [CrossRef]
- Lyu, Y.; Bergseth, E.; Olofsson, U. Open System Tribology and Influence of Weather Condition. Sci. Rep. 2016, 6, 32455. [Google Scholar] [CrossRef] [PubMed]
- Xu, H.J.; Zhao, C.Y. Thermal efficiency analysis of the cascaded latent heat/cold storage with multi-stage heat engine model. Renew. Energy 2016, 86, 228–237. [Google Scholar] [CrossRef]
- Chiu, J.N.W.; Martin, V. Multistage latent heat cold thermal energy storage design analysis. Appl. Energy 2013, 112, 1438–1445. [Google Scholar] [CrossRef]
- Tian, Y.; Zhao, C.Y. Thermal and exergetic analysis of Metal Foam-enhanced Cascaded Thermal Energy Storage (MF-CTES). Int. J. Heat Mass Transf. 2013, 58, 86–96. [Google Scholar] [CrossRef]
- Mosaffa, A.H.; Ferreira, C.A.I.; Talati, F.; Rosen, M.A. Thermal performance of a multiple PCM thermal storage unit for free cooling. Energy Convers. Manag. 2013, 67, 1–7. [Google Scholar] [CrossRef]
- Mosaffa, A.H.; Garousi Farshi, L.; Infante Ferreira, C.A.; Rosen, M.A. Energy and exergy evaluation of a multiple-PCM thermal storage unit for free cooling applications. Renew. Energy 2014, 68, 452–458. [Google Scholar] [CrossRef]
- Al-Shehri, S.A. Cooling Computer Chips with Cascaded and Non-Cascaded Thermoelectric Devices. Arab. J. Sci. Eng. 2019, 44, 9105–9126. [Google Scholar] [CrossRef]
- Saber, H.H.; AlShehri, S.A.; Maref, W. Performance optimization of cascaded and non-cascaded thermoelectric devices for cooling computer chips. Energy Convers. Manag. 2019, 191, 174–192. [Google Scholar] [CrossRef]
- Mahian, O.; Kolsi, L.; Amani, M.; Estellé, P.; Ahmadi, G.; Kleinstreuer, C.; Marshall, J.S.; Taylor, R.A.; Abu-Nada, E.; Rashidi, S.; et al. Recent advances in modeling and simulation of nanofluid flows—Part II: Applications. Phys. Rep. 2019, 791, 1–59. [Google Scholar] [CrossRef]






| Medium | Thermal Conductivity W·m−1·K−1 | Density kg·m−3 | Specific Heat Capacity J·kg−1·K−1 |
|---|---|---|---|
| Water | 0.61 | 1000 | 4200 |
| Ice | 0.61 | 900 | 2100 |
| Numder | Grid Number | V (cm3) | V Error (%) | VE (m3/kWh) | VE Error (%) |
|---|---|---|---|---|---|
| No.1 | 30,000 | 26.6 | 16.6% | 147.59 | 39.5% |
| No.2 | 66,640 | 22.8 | Baseline | 105.82 | Baseline |
| No.3 | 75,040 | 22.0 | 3.5% | 91.37 | 13.7% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, M.; Zhang, W.; Xu, K.; Yang, Z.; Wang, L.; Feng, Y.; Chen, H. Formation Rate and Energy Efficiency of Ice Plug in Pipelines Driven by the Cascade Utilization of Cold Energy. Energies 2024, 17, 1994. https://doi.org/10.3390/en17091994
Hu M, Zhang W, Xu K, Yang Z, Wang L, Feng Y, Chen H. Formation Rate and Energy Efficiency of Ice Plug in Pipelines Driven by the Cascade Utilization of Cold Energy. Energies. 2024; 17(9):1994. https://doi.org/10.3390/en17091994
Chicago/Turabian StyleHu, Minglei, Wei Zhang, Ke Xu, Zijiang Yang, Liqun Wang, Yongqiang Feng, and Hao Chen. 2024. "Formation Rate and Energy Efficiency of Ice Plug in Pipelines Driven by the Cascade Utilization of Cold Energy" Energies 17, no. 9: 1994. https://doi.org/10.3390/en17091994
APA StyleHu, M., Zhang, W., Xu, K., Yang, Z., Wang, L., Feng, Y., & Chen, H. (2024). Formation Rate and Energy Efficiency of Ice Plug in Pipelines Driven by the Cascade Utilization of Cold Energy. Energies, 17(9), 1994. https://doi.org/10.3390/en17091994






