Effects of Viscous Dissipation on the Slip MHD Flow and Heat Transfer past a Permeable Surface with Convective Boundary Conditions
Abstract
:1. Introduction
2. Mathematical Formulation
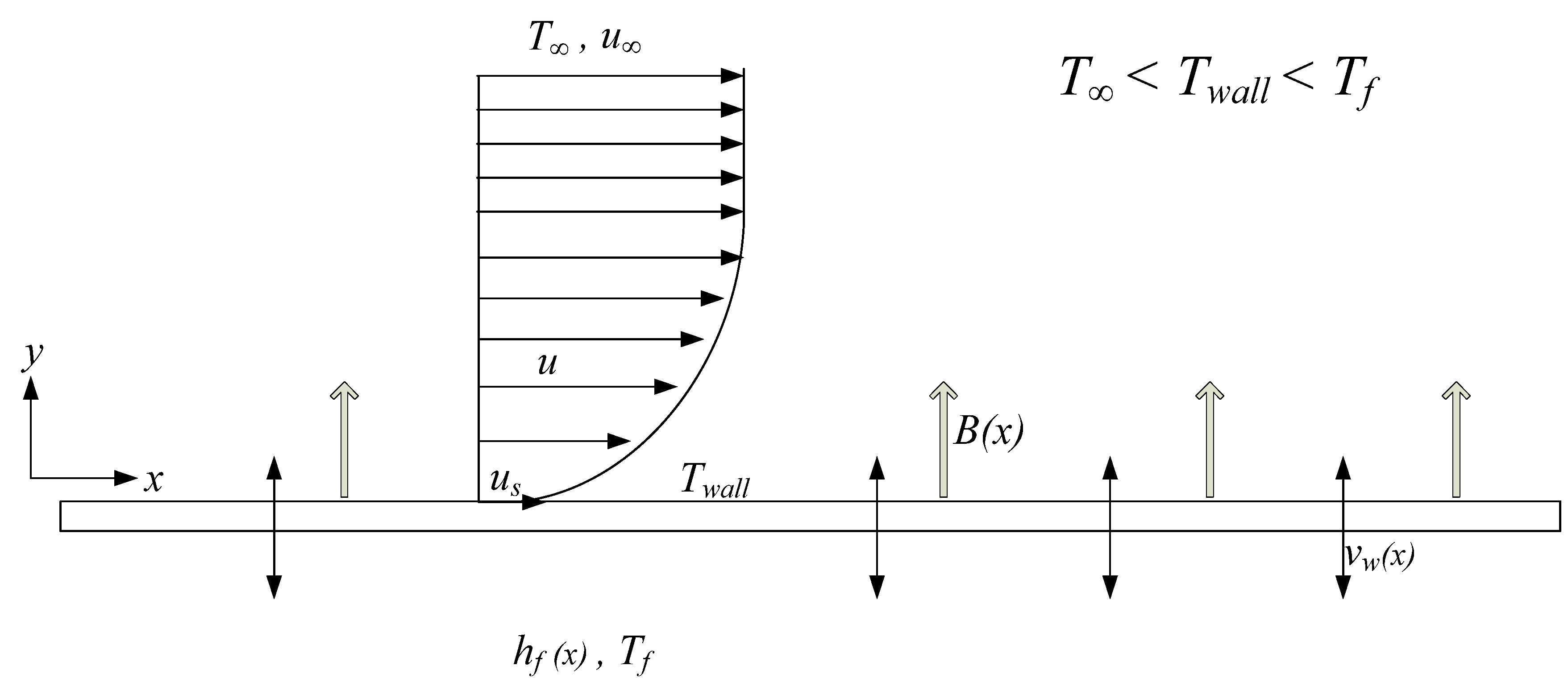
3. Results and Discussion
| M | Pr | K | a | Rahman | Aziz | Ishak | Present results | ||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| [33] | [33] | [33] | [30] | [31] | |||||||
| f''(0) | θ(0) | |θ'(0)| | |θ'(0)| | |θ'(0)| | f''(0) | θ(0) | |θ'(0)| | ||||
| 1 | 1 | 0 | 0.01 | 1.0440 | 0.0236 | 0.0098 | 1.0440 | 0.0237 | 0.0098 | ||
| 0.5 | 1.0440 | 0.5478 | 0.2261 | 1.0440 | 0.5480 | 0.2261 | |||||
| 1 | 1.0440 | 0.7078 | 0.2921 | 1.0440 | 0.7078 | 0.2920 | |||||
| 0.5 | 0.01 | 0.6987 | 0.0208 | 0.0098 | 0.6987 | 0.0209 | 0.0098 | ||||
| 0.5 | 0.6987 | 0.5163 | 0.2418 | 0.6987 | 05164 | 0.2417 | |||||
| 1 | 0.6987 | 0.6811 | 0.3189 | 0.6987 | 0.6812 | 0.3188 | |||||
| 0 | 0.72 | 0 | 0.05 | 0.0428 | 0.0428 | 0.0428 | 0.0428 | ||||
| 0.1 | 0.0747 | 0.0747 | 0.0747 | 0.0747 | |||||||
| 0.2 | 0.1193 | 0.1193 | 0.1193 | 0.1193 | |||||||
| 0.4 | 0.1701 | 0.1700 | 0.1699 | 0.1700 | |||||||
| 0.6 | 0.1981 | 0.1981 | 0.1980 | 0.1980 | |||||||
| 0.8 | 0.2160 | 0.2159 | 0.2159 | 0.2159 | |||||||
| 1 | 0.2282 | 0.2282 | 0.2282 | 0.2282 | |||||||
| 5 | 0.2793 | 0.2791 | 0.2791 | 0.2791 | |||||||
| 10 | 0.2873 | 0.2871 | 0.2871 | 0.2871 | |||||||
| 20 | 0.2915 | 0.2913 | 0.2913 | 0.2913 | |||||||
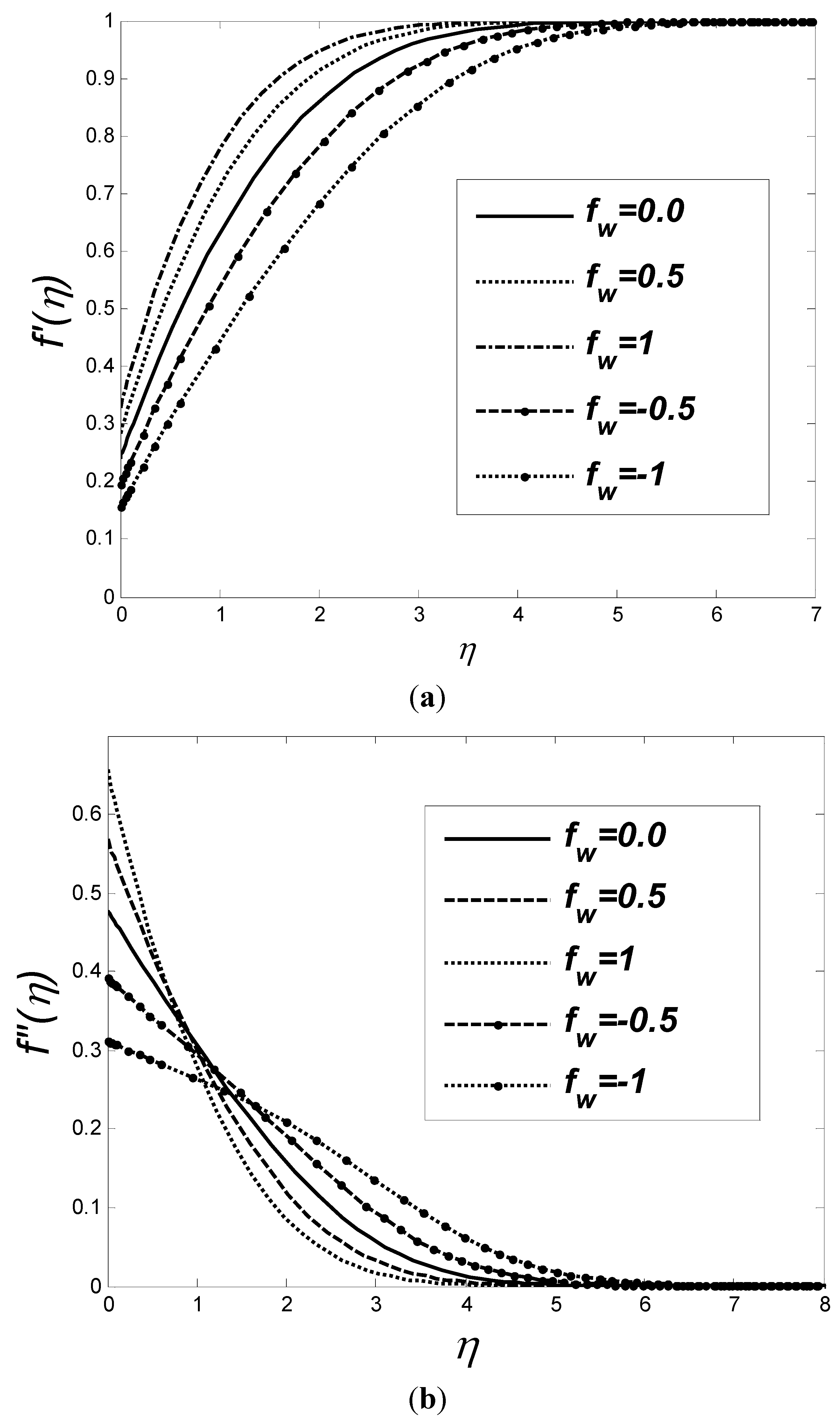
3.1. Effects on Velocity Field and Friction


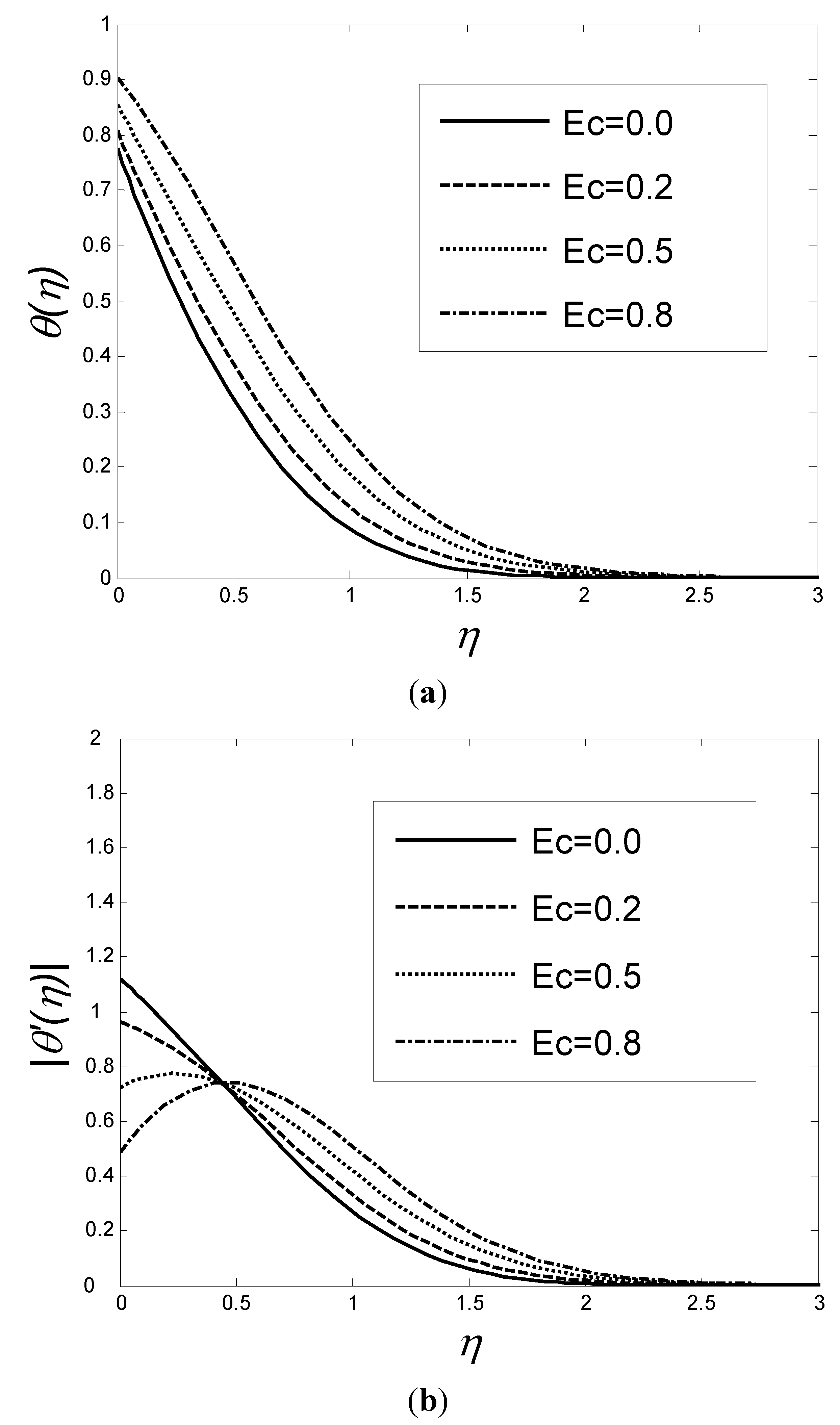
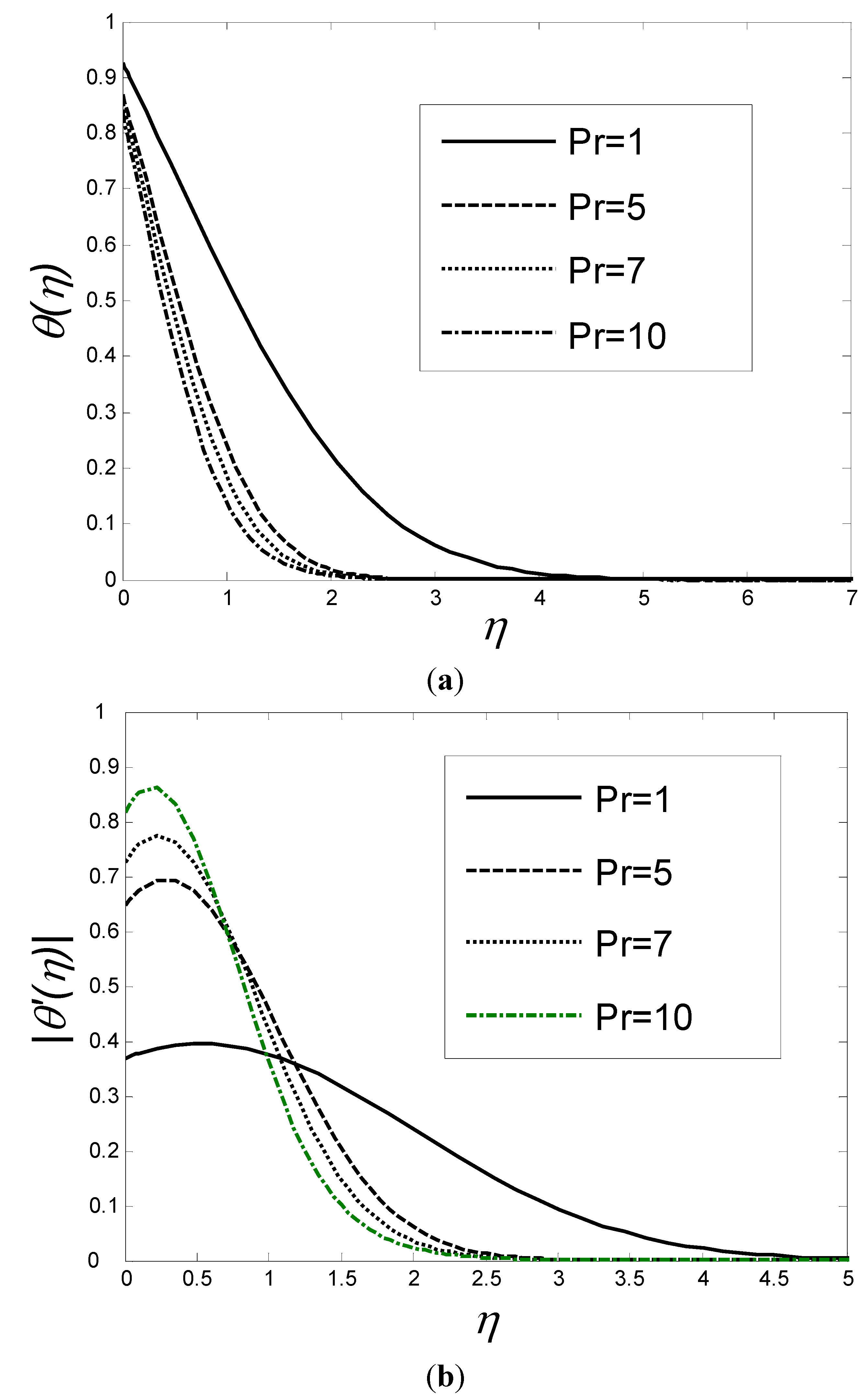
3.2. Effects on Temperature Field and Heat Transfer



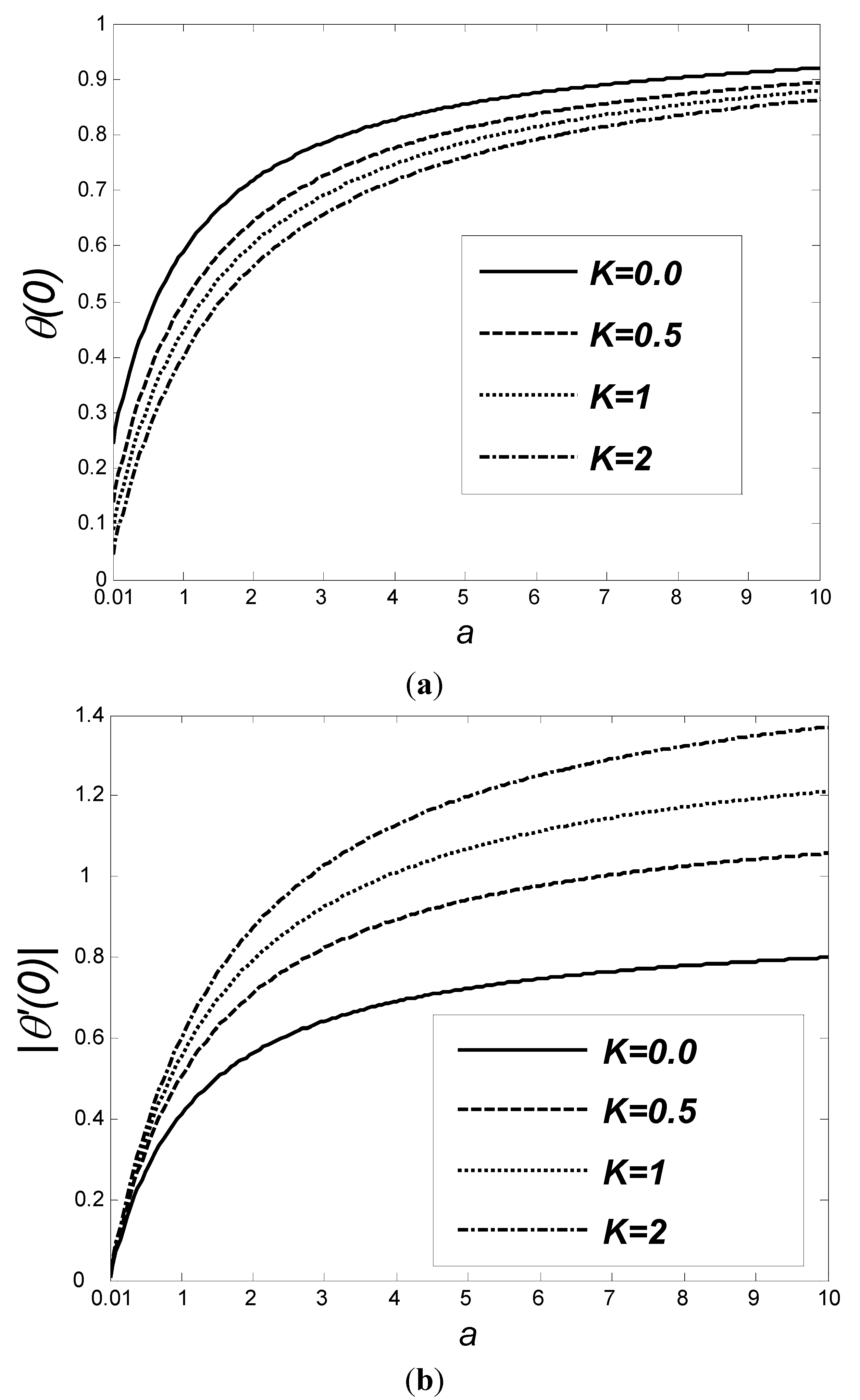
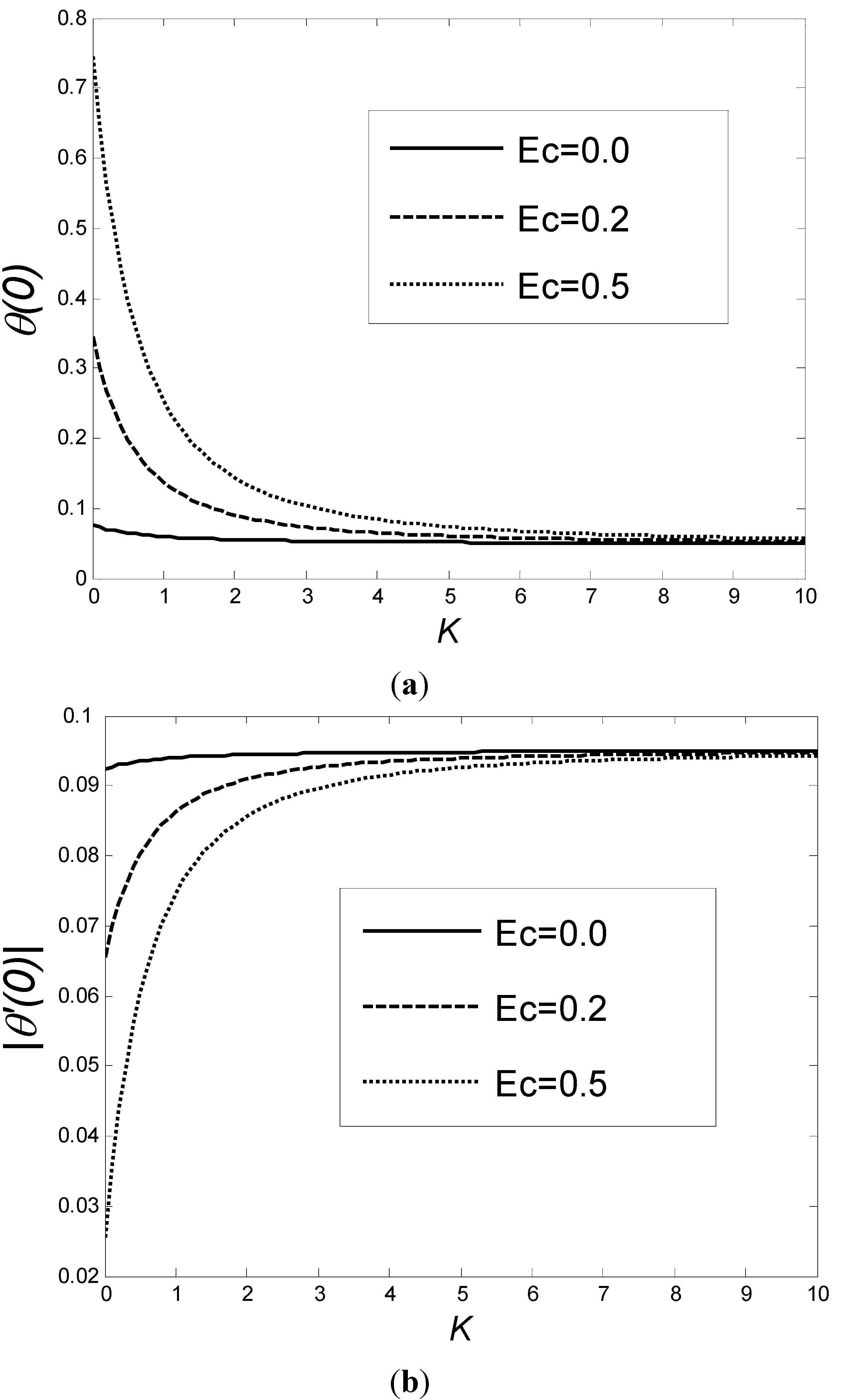
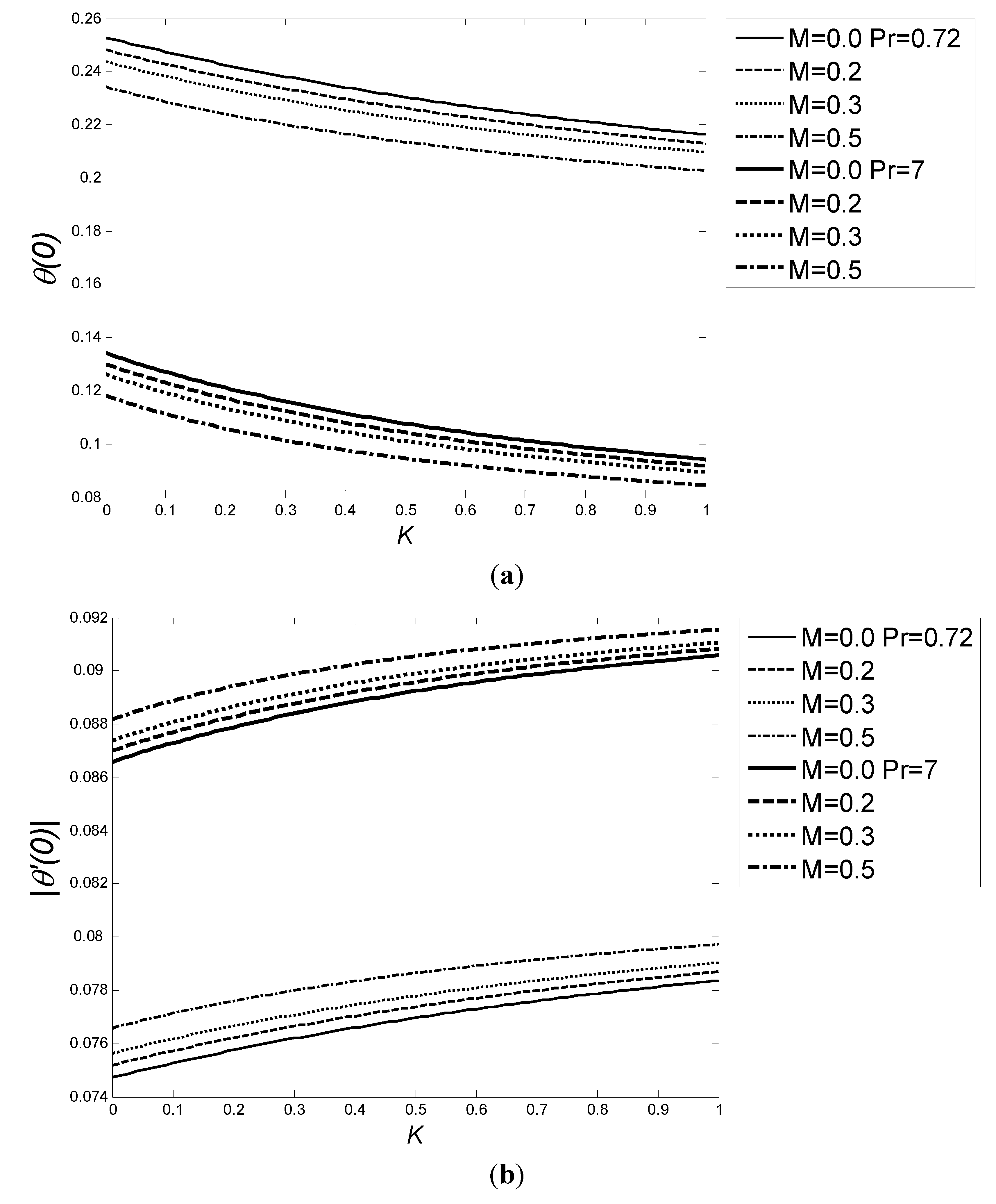
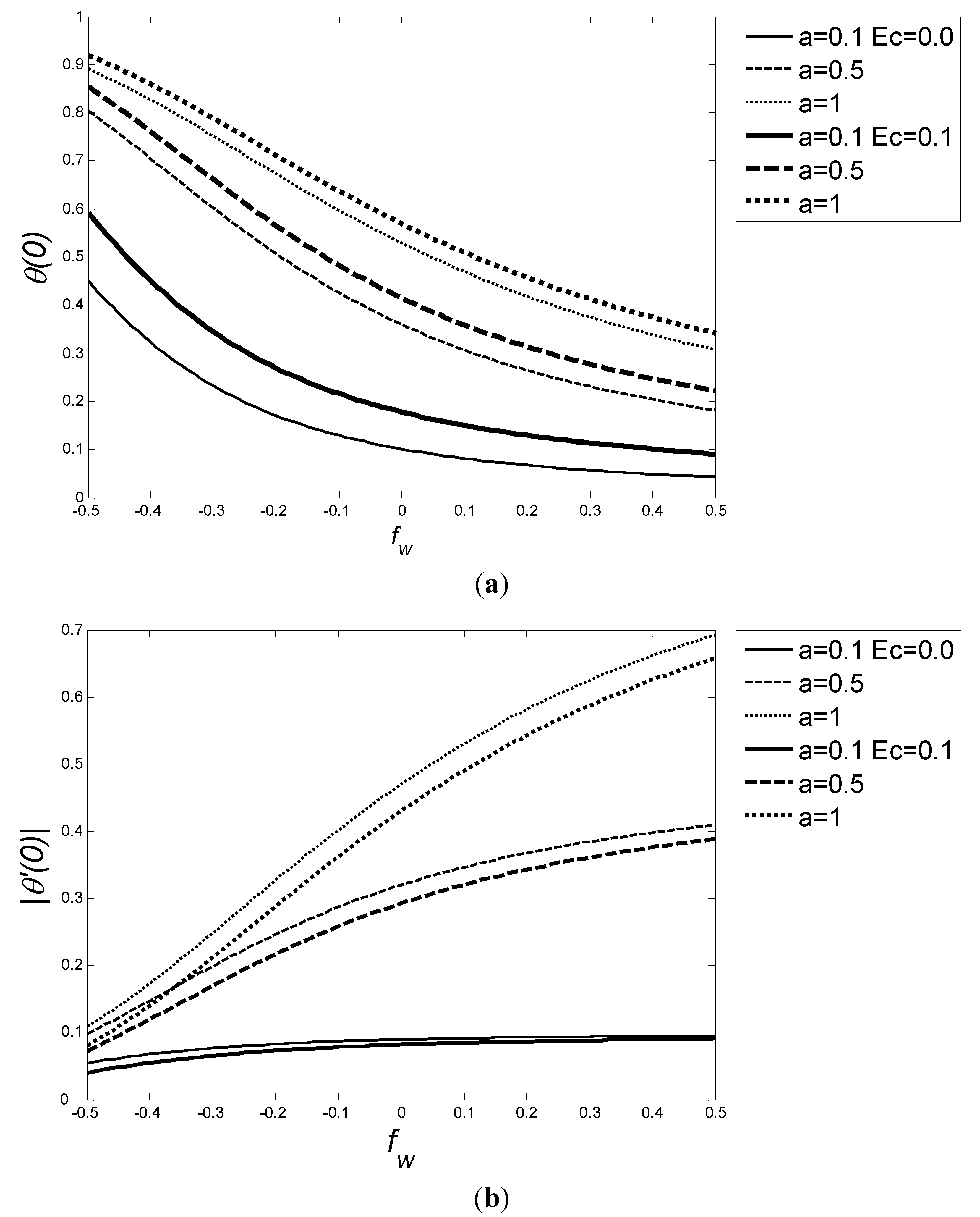
4. Conclusions
- The skin friction coefficient increases with the rise of both suction and M, while it decreases with K and injection. Moreover, the effects of both M and fw on the wall shear stress are much more significant at low K.
- Changes in M and fw in the presence of slip boundary condition can efficiently manipulate the wall fluid velocity, which increases with the increase of M, K and suction.
- The wall temperature decreases with the increase in K, M, Pr and suction, whereas it increases with the increase of Ec, a, and injection.
- The heat transfer rate increases with the increase of Pr, a, K, M and suction, while it decreases with the increase of Ec and injection.
- In the slip flow regime, under low Biot number conditions, the permeability effects on heat transfer rate tend to be negligible.
Nomenclature:
| a | Biot number |
| B | magnetic induction [Wb m−2] |
| Cf | local skin-friction coefficient |
| cp | specific heat at constant pressure [J kg−1 K−1] |
| Ec | Eckert number |
| f | dimensionless function |
| hf | convective heat transfer coefficient [W m−2 K−1] |
| K | slip coefficient |
| kT | thermal conductivity [W m−1 K−1] |
| l | slip length [m] |
| M | magnetic parameter |
| P | Pressure [Pa] |
| Pr | Prandtl number |
| Rex | local Reynolds number |
| Tf | temperature of the hot fluid at the bottom of the plate [K] |
| Tw | temperature at the surface of the plate [K] |
| T | temperature of the fluid within the boundary layer [K] |
| T∞ | temperature of the ambient fluid [K] |
| u∞ | free stream velocity [m s−1] |
| u, v | the x-, y-components of velocity [m s−1] |
| x, y | distance along and normal to the plate [m] |
Greek
| α | thermal diffusivity [m2 s–1] |
| η | similarity variable |
| θ | dimensionless temperature |
| μ | dynamic viscosity [Pa s] |
| ν∞ | kinematic viscosity [m2 s−1] |
| ρ | fluid density [kg m–3] |
| σ | electrical conductivity of the fluid [S m–1] |
Subscripts
| s | slip condition |
| w | surface condition |
| ∞ | ambient condition |
Acknowledgments
References
- Nisar, A.; Afzulpurkar, N.; Mahaisavariya, B.; Tuantranont, A. MEMS-based micropumps in drug delivery and biomedical applications. Sens. Actuators B 2008, 130, 917–942. [Google Scholar] [CrossRef]
- Chen, C.H.; Jang, L.S. Recent patents on micromixing technology and micromixers. Recent Patents Mech. Eng. 2009, 2, 240–247. [Google Scholar] [CrossRef]
- Capretto, L.; Cheng, W.; Hill, M.; Zhang, X. Micromixing within microfluidic devices. Top. Curr. Chem. 2011, 304, 27–68. [Google Scholar] [PubMed]
- Kleinstreuer, C.; Li, J.; Koo, J. Microfluidics of nano-drug delivery. Int. J. Heat Mass Transf. 2008, 51, 5590–5597. [Google Scholar] [CrossRef]
- Avsec, J. Nanofluid and Ferrofluid Slip Flow in Rectangular and Circular Microchannels. In Proceedings of 7th EUROMECH Solid Mechanics Conference, Lisbon, Portugal, 7–11 September 2009.
- Eijkel, J.C.T.; Berg, A. Nanofluidics: What is it and what can we expect from it? Microfluid. Nanofluid. 2005, 1, 249–267. [Google Scholar] [CrossRef]
- Gad-el-Hak, M. The MEMS Handbook; CRC Press: Boca Raton, FL, USA, 2002. [Google Scholar]
- Gad-el-Hak, M. Mems Applications; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]
- Gad-el-Hak, M. The fluid mechanics of microdevices-The Freeman scholar lecture. J. Fluids Eng. Trans. ASME 1999, 121, 5–33. [Google Scholar] [CrossRef]
- Bocquet, L.; Barrat, J.L. Flow boundary conditions from nano-to micro-scales. Soft Matter 2007, 3, 685–693. [Google Scholar] [CrossRef]
- Haldar, K. Effects of the shape of stenosis on the resistance to blood flow through an artery. Bull. Math. Biol. 1985, 47, 545–550. [Google Scholar] [CrossRef] [PubMed]
- Ponalagusamy, R.; Tamil Selvi, R. A study on two-layered model (casson-newtonian) for blood flow through an arterial stenosis: Axially variable slip velocity at the wall. J. Frankl. Inst. 2011, 348, 2308–2321. [Google Scholar] [CrossRef]
- Van Rij, J.; Ameel, T.; Harman, T. The effect of viscous dissipation and rarefaction on rectangular microchannel convective heat transfer. Int. J. Therm. Sci. 2009, 48, 271–281. [Google Scholar]
- Koo, J.; Kleinstreuer, C. Viscous dissipation effects in microtubes and microchannels. Int. J. Heat Mass Transf. 2004, 47, 3159–3169. [Google Scholar] [CrossRef]
- Fang, T. Further study on a moving-wall boundary-layer problem with mass transfer. Acta Mech. 2003, 163, 183–188. [Google Scholar] [CrossRef]
- Fang, T.; Lee, C.F. A moving-wall boundary layer flow of a slightly rarefied gas free stream over a moving flat plate. Appl. Math. Lett. 2005, 18, 487–495. [Google Scholar] [CrossRef]
- Martin, M.J.; Boyd, I.D. Momentum and heat transfer in a laminar boundary layer with slip flow. J. Thermophys. Heat Transf. 2006, 20, 710–719. [Google Scholar] [CrossRef]
- Yazdi, M.H.; Abdullah, S.; Hashim, I.; Zaharim, A.; Sopian, K. Friction and Heat Transfer in Slip Flow Boundary Layer at Constant Heat Flux Boundary Conditions. In Proceedings of the 10th International Conference on Mathematical Methods, Computational Techniques and Intelligent Systems, Corfu, Greece, 26–28 October 2008; pp. 207–212.
- Yazdi, M.H.; Abdullah, S.; Hashim, I.; Nopiah, Z. M.; Zaharim, A.; Sopian, K. Convective Heat Transfer of Slip Liquid Flow Past Horizontal Surface within the Porous Media at Constant Heat Flux Boundary Conditions. In Proceedings of the American Conference on Applied Mathematics: Recent Advances in Applied Mathematics, Harvard University, Cambridge, MA, USA, 27–29 January 2009; pp. 527–533.
- Yazdi, M.H.; Abdullah, S.; Hashim, I.; Sopian, K. Reducing Exergy Losses of Liquid Fluid Using Embedded Open Parallel Microchannels within the Surface. In Proceedings of the 13th WSEAS International Conference on Applied Mathematics, Puerto De La Cruz, Spain, 15–17 December 2008; pp. 345–350.
- Yazdi, M.H.; Abdullah, S.; Hashim, I.; Sopian, K.; Zaharim, A. Entropy generation analysis of liquid fluid past embedded open parallel microchannels within the surface. Eur. J. Sci. Res. 2009, 28, 462–470. [Google Scholar]
- Hayat, T.; Javed, T.; Abbas, Z. Slip flow and heat transfer of a second grade fluid past a stretching sheet through a porous space. Int. J. Heat Mass Transf. 2008, 51, 4528–4534. [Google Scholar] [CrossRef]
- Hayat, T.; Qasim, M.; Mesloub, S. MHD flow and heat transfer over permeable stretching sheet with slip conditions. Int. J. Numer. Methods Fluids 2011, 66, 963–975. [Google Scholar] [CrossRef]
- Qasim, M.; Hayat, T.; Hendi, A. Effects of slip conditions on stretching flow with ohmic dissipation and thermal radiation. Heat Transf.—Asian Res. 2011, 40, 641–654. [Google Scholar] [CrossRef]
- Fang, T.; Yao, S.; Zhang, J.; Aziz, A. Viscous flow over a shrinking sheet with a second order slip flow model. Commun. Nonlinear Sci. Numer. Simul. 2010, 15, 1831–1842. [Google Scholar] [CrossRef]
- Abbas, Z.; Wang, Y.; Hayat, T.; Oberlack, M. Slip effects and heat transfer analysis in a viscous fluid over an oscillatory stretching surface. Int. J. Numer. Methods Fluids 2009, 59, 443–458. [Google Scholar] [CrossRef]
- Fang, T.; Zhang, J.; Yao, S. Slip MHD viscous flow over a stretching sheet—An exact solution. Commun. Nonlinear Sci. Numer. Simul. 2009, 14, 3731–3737. [Google Scholar] [CrossRef]
- Yazdi, M.H.; Abdullah, S.; Hashim, I.; Sopian, K. Slip MHD liquid flow and heat transfer over non-linear permeable stretching surface with chemical reaction. Int. J. Heat Mass Transf. 2011, 54, 3214–3225. [Google Scholar] [CrossRef]
- Yazdi, M.H.; Abdullah, S.; Hashim, I.; Sopian, K. Slip MHD Flow over Permeable Stretching Surface with Chemical Reaction. In Proceedings of 17th Australasian Fluid Mechanics Conference, Auckland, New Zealand, 5–9 December 2010.
- Aziz, A. A similarity solution for laminar thermal boundary layer over a flat plate with a convective surface boundary condition. Commun. Nonlinear Sci. Numer. Simul. 2009, 14, 1064–1068. [Google Scholar] [CrossRef]
- Ishak, A. Similarity solutions for flow and heat transfer over a permeable surface with convective boundary condition. Appl. Math. Comput. 2010, 217, 837–842. [Google Scholar] [CrossRef]
- Yao, S.; Fang, T.; Zhong, Y. Heat transfer of a generalized stretching/shrinking wall problem with convective boundary conditions. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 752–760. [Google Scholar] [CrossRef]
- Rahman, M. Locally similar solutions for hydromagnetic and thermal slip flow boundary layers over a flat plate with variable fluid properties and convective surface boundary condition. Meccanica 2011, 46, 1127–1143. [Google Scholar] [CrossRef]
- Rossow, V.J.; Center, N.A.R. On Flow of Electrically Conducting Fluids over a Flat Plate in the Presence of a Transverse Magnetic Field; National Advisory Committee for Aeronautics: Washington, DC, USA, 1957; NACA TN 3971, Report no. 1358. [Google Scholar]
- Hang, X. Erratum to “An explicit analytic solution for convective heat transfer in an electrically conducting fluid at a stretching surface with uniform free stream”. Int. J. Eng. Sci. 2005, 43, 859–874. [Google Scholar] [CrossRef]
- Lund, N.J.; Hendy, S.C. Effective slip length of nanoscale mixed-slip surfaces. ANZIAM J. 2009, 50, 381–394. [Google Scholar] [CrossRef]
© 2011 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Yazdi, M.H.; Abdullah, S.; Hashim, I.; Sopian, K. Effects of Viscous Dissipation on the Slip MHD Flow and Heat Transfer past a Permeable Surface with Convective Boundary Conditions. Energies 2011, 4, 2273-2294. https://doi.org/10.3390/en4122273
Yazdi MH, Abdullah S, Hashim I, Sopian K. Effects of Viscous Dissipation on the Slip MHD Flow and Heat Transfer past a Permeable Surface with Convective Boundary Conditions. Energies. 2011; 4(12):2273-2294. https://doi.org/10.3390/en4122273
Chicago/Turabian StyleYazdi, Mohammad H., Shahrir Abdullah, Ishak Hashim, and Kamaruzzaman Sopian. 2011. "Effects of Viscous Dissipation on the Slip MHD Flow and Heat Transfer past a Permeable Surface with Convective Boundary Conditions" Energies 4, no. 12: 2273-2294. https://doi.org/10.3390/en4122273
APA StyleYazdi, M. H., Abdullah, S., Hashim, I., & Sopian, K. (2011). Effects of Viscous Dissipation on the Slip MHD Flow and Heat Transfer past a Permeable Surface with Convective Boundary Conditions. Energies, 4(12), 2273-2294. https://doi.org/10.3390/en4122273




