Modeling of Proton-Conducting Solid Oxide Fuel Cells Fueled with Syngas
Abstract
:1. Introduction
2. Model Development

- (1)
- The model is 2D, neglecting the 3D effect;
- (2)
- Heat radiation is assumed to be negligible;
- (3)
- The flow in the gas channels is laminar due to low velocity and small dimension;
- (4)
- Electrochemical reactions are assumed to take place at the electrode-electrolyte interface only.
2.1. Electrochemical Model

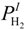 is the partial pressure of H2 at the anode-electrolyte interface.
is the partial pressure of H2 at the anode-electrolyte interface. 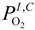 and
and  are the partial pressure of O2 and H2O at the cathode-electrolyte interface, respectively. Thus, the concentration overpotentials at the electrodes are included in the Nernst potential (Equation (5)). ηohmic is the ohmic overpotential of the electrolyte and can be determined as:
are the partial pressure of O2 and H2O at the cathode-electrolyte interface, respectively. Thus, the concentration overpotentials at the electrodes are included in the Nernst potential (Equation (5)). ηohmic is the ohmic overpotential of the electrolyte and can be determined as:
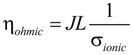
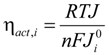
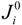 is the exchange current densities (A·m−2) which indicates the activity of the electrode. n is the number of electrons involved in the electrochemical reaction. Based on the previous studies, the exchange current densities for anode and cathode are 5300 A·m−2 and 2000 A·m−2, respectively [15].
is the exchange current densities (A·m−2) which indicates the activity of the electrode. n is the number of electrons involved in the electrochemical reaction. Based on the previous studies, the exchange current densities for anode and cathode are 5300 A·m−2 and 2000 A·m−2, respectively [15].2.2. Chemical Model
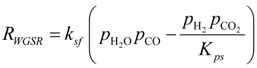
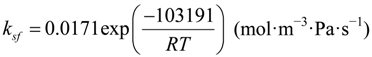

2.3. Computational Fluid Dynamic (CFD) Model
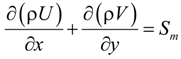
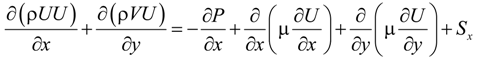
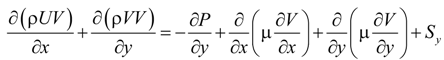


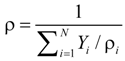
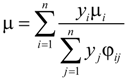
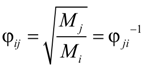
 only includes the molecular diffusion while both molecular diffusion and Knudsen diffusion are considered in the porous electrodes. The value of
only includes the molecular diffusion while both molecular diffusion and Knudsen diffusion are considered in the porous electrodes. The value of  can thus be calculated as [20]:
can thus be calculated as [20]:

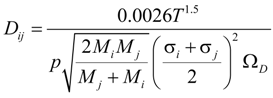
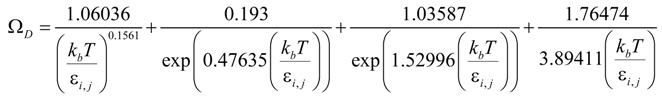

| CO | CO2 | H2 | O2 | N2 | H2O | |
|---|---|---|---|---|---|---|
| σi (A) | 3.69 | 3.941 | 2.827 | 3.467 | 3.798 | 2.641 |
| εi/k (K2·J−1) | 91.7 | 195.2 | 59.7 | 106.7 | 71.4 | 809.1 |
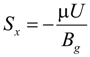
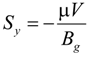


3. Numerical Methodologies
4. Results and Analysis
| Parameter | Value |
|---|---|
| Operating temperature, T (K) | 1073 |
| Operating pressure, P (bar) | 1.0 |
| Electrode porosity, ε | 0.4 |
| Electrode tortuosity, ξ | 3.0 |
| Average pore radius, rp (μm) | 0.5 |
| Anode-supported electrolyte: | - |
| Anode thickness da (μm) | 500 |
| Electrolyte thickness, L (μm) | 100 |
| Cathode thickness, dc (μm) | 100 |
| Height of gas flow channel (mm) | 1.0 |
| Length of the planar SOFC (mm) | 40 |
| Thickness of interconnector (mm) | 0.5 |
| Inlet velocity at anode: U0 (m·s−1) | 1.0 |
| Cathode inlet gas molar ratio: O2/N2/ | 0.18/0.79/0.03 |
| Anode inlet gas molar ratio: H2/CO (Syngas) | 3/1 |
| SOFC operating potential (V) | 0.7 |
| Thermal conductivity of SOFC component (W·m−1·K−1) | - |
| Anode | 11.0 |
| Electrolyte | 2.7 |
| Cathode | 6.0 |
| Interconnect | 1.1 |
4.1. Effect of Syngas Composition




4.2. Effect of Operating Temperature

4.3. Effect of Steam Addition

5. Conclusions
Acknowledgments
Author Contributions
Nomenclature
| Bg | Permeability of the porous electrode (m2) |
| cp | Heat capactity (J·kg−1·K−1) |
| da | Thickness of anode (µm) |
| dc | Thickness of cathode (µm) |
 | Effective diffusion coefficient of species i in gas mixture (cm2·s−1) |
| Di,k | Knudsen diffusion coefficient of i (cm2·s−1) |
| Di,j | Binary diffusion coefficient of i and j (cm2·s−1) |
| E | Equilibrium potential (V) |
| E0 | Reversible potential at standard condition (V) |
| F | Faraday constant (9.6485 × 104 C·mol−1) |
| HWGS | Heat generation from water gas shift reaction (J·mol−1) |
| J | Current density (A·m−2) |
| k | Thermal conductivity (W·m−1·K−1) |
| L | Thickness of the electrolyte (m) |
| Mi | Molecular weight of species i (kg·mol-1) |
| n | Number of electrons transferred |
| P | Operating pressure (bar) |
 | Partial pressure (bar) of species i at electrode-electrolyte interface |
| RWGSR | Rate of water gas shift reaction (mol·m−3·s−1) |
| rp | Mean pore radius of electrode (µm) |
| R | Universal gas constant (8.3145 J·mol−1·K−1) |
| ∆S | Entropy change of electrochitalicical reactions (kJ·kg−1·K−1) |
| Sm | Source term in continuity equation (kg·m−3·s−1) |
| Sx, Sy | Source terms in momentum equations (kg·m−2·s−2) |
| ST | Source terms in energy equations (W·m−3) |
| Ssp | Source terms in species equations (kg·m−3·s−1) |
| T | Operating titalicperature (K) |
| U | Velocity in x direction (m·s−1) |
| U0 | Gas velocity at the SOFC inlet (m·s−1) |
| V | SOFC operating potential (V); Velocity in y direction (m·s−1) |
| X | Molar fraction of species i |
| Y | Mass fraction of species i |
| ε | Electrode porosity |
| ξ | Electrode tortuosity |
| σi,j | Mean characteristic length of species i and j (Å) |
| σionic | Ionic conductivity of the electrolyte (Ω−1·m−1) |
| ΩD | Dimensionless diffusion collision integral |
| ρ | Density of the gas mixture (kg·m−3) |
| µ | Viscosity of gas mixture (kg·m−1·s−1) |
| ηact,a | Activation overpotential at anode (V) |
| ηact,c | Activation overpotential at cathode (V) |
| ηohmic | Ohmic overpotential of the electrolyte (V) |
Conflicts of Interest
References
- Wachsman, E.D.; Lee, K.T. Lowering the temperature of solid oxide fuel cells. Science 2011, 334, 935–939. [Google Scholar] [CrossRef]
- Lee, K.T.; Yoon, H.S.; Wachsman, E.D. The evolution of low temperature solid oxide fuel cells. J. Mater. Res. 2012, 27, 2063–2078. [Google Scholar] [CrossRef]
- Wang, Z.R.; Qian, J.; Cao, J.; Wang, S.R.; Wen, T.L. A study of multilayer tape casting method for anode-supported planar type solid oxide fuel cells (SOFCs). J. Alloys Compd. 2007, 437, 264–268. [Google Scholar] [CrossRef]
- Iwahara, H. High temperature protonic conductors and their applications. Solid State Ion. 1992, 575–586. [Google Scholar]
- Liu, H.; Akhtar, Z.; Li, P.W.; Wang, K. Mathematical modeling analysis and optimization of key design parameters of proton-conductive solid oxide fuel cells. Energies 2014, 7, 173–190. [Google Scholar] [CrossRef]
- Ni, M.; Leung, D.Y.C.; Leung, M.K.H. An improved electrochemical model for the NH3 fed proton conducting solid oxide fuel cells at intermediate temperatures. J. Power Sources 2008, 185, 233–240. [Google Scholar] [CrossRef]
- Ni, M.; Leung, M.K.H.; Leung, D.Y.C. Mathematical modeling of proton-conducting solid oxide fuel cells and comparison with oxygen ion conducting counterpart. Fuel Cells 2007, 7, 269–278. [Google Scholar] [CrossRef]
- Ni, M. The effect of electrolyte type on performance of solid oxide fuel cells running on hydrocarbon fuels. Int. J. Hydrog. Energy 2013, 38, 2846–2858. [Google Scholar] [CrossRef]
- Andersson, M.; Yuan, J.L.; Sunden, B. SOFC modeling considering hydrogen and carbon monoxide as electrochemical reactants. J. Power Sources 2013, 232, 42–54. [Google Scholar] [CrossRef]
- Pieratti, E.; Baratieri, M.; Ceschini, S.; Tognana, L.; Baggio, P. Syngas suitability for solid oxide fuel cells applications produced via biomass steam gasification process: Experimental and modeling analysis. J. Power Sources 2011, 196, 10038–10049. [Google Scholar] [CrossRef]
- Lorente, E.; Millan, M.; Brandon, N.P. Use of gasification syngas in SOFC: Impact of real tar on anode materials. Int. J. Hydrog. Energy 2012, 37, 7271–7281. [Google Scholar] [CrossRef]
- Ni, M. Modeling of SOFC running on partially pre-reformed gas mixture. Int. J. Hydrog. Energy 2012, 37, 1731–1745. [Google Scholar] [CrossRef]
- Matsumoto, H. Proton-conducting Perovskite—Properties and Experiences for Hydrogen Transport and Energy Applications. In Proceedings of the Prospects Protonic Ceramic Cells 2011—International Workshop on Protonic Ceramic Fuel Cell and Steam Electrolysis: Status and Prospects, Botanical Institute, Montpellier, France, 3–4 November 2011.
- Sohal, M.S.; Idaho National Laboratory, Idaho Falls, ID, USA. Personal communication at ASME 2010 8th International Fuel Cell Science, Engineering & Technology Conference, Brooklyn, NY, USA, 14–16 June 2010.
- Ni, M.; Leung, M.K.H.; Leung, D.Y.C. Parametric study of solid oxide fuel cell performance. Energy Convers. Manag. 2007, 48, 1525–1535. [Google Scholar] [CrossRef]
- Haberman, B.A.; Young, J.B. Three-dimensional simulation of chemically reacting gas flows in the porous support structure of an integrated-planar solid oxide fuel cell. Int. J. Heat Mass Transf. 2004, 47, 3617–3629. [Google Scholar] [CrossRef]
- Chase, M.W. NIST-JANAF Thermochemical Tables, 4th ed.; American Chemical Society, American Institute of Physics for the National Institute of Standards and Technology: Washington, DC, USA, 1998. [Google Scholar]
- Zheng, K.Q.; Sun, Q.; Ni, M. On the local thermal non-equilibrium in SOFCs considering internal reforming and ammonia thermal cracking reaction. Energy Technol. 2013, 1, 35–41. [Google Scholar] [CrossRef]
- Wang, C.Y. Fundamental models for fuel cell engineering. Chem. Rev. 2004, 104, 4727–4765. [Google Scholar] [CrossRef]
- Reid, R.C.; Prausnitz, J.M.; Poling, B.E. The Properties of Gases & Liquids, 4th ed.; McGraw-Hill Book Company: New York, NY, USA, 1987. [Google Scholar]
- Yuan, J.L.; Lv, X.R.; Sunden, B.; Yue, D.T. Analysis of parameter effects on transport phenomena in conjunction with chemical reactions in ducts relevant for methane reformers. Int. J. Hydrog. Energy 2007, 32, 3887–3898. [Google Scholar] [CrossRef]
- Ni, M. 2D thermal-fluid modeling and parametric analysis of a planar solid oxide fuel cell. Energy Convers. Manag. 2010, 51, 714–721. [Google Scholar] [CrossRef]
- Ni, M. Thermo-electrochemical modeling of ammonia-fueled solid oxide fuel cells considering ammonia thermal decomposition in the anode. Int. J. Hydrog. Energy 2011, 36, 3153–3166. [Google Scholar] [CrossRef]
© 2014 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Ni, M.; Shao, Z.; Chan, K.Y. Modeling of Proton-Conducting Solid Oxide Fuel Cells Fueled with Syngas. Energies 2014, 7, 4381-4396. https://doi.org/10.3390/en7074381
Ni M, Shao Z, Chan KY. Modeling of Proton-Conducting Solid Oxide Fuel Cells Fueled with Syngas. Energies. 2014; 7(7):4381-4396. https://doi.org/10.3390/en7074381
Chicago/Turabian StyleNi, Meng, Zongping Shao, and Kwong Yu Chan. 2014. "Modeling of Proton-Conducting Solid Oxide Fuel Cells Fueled with Syngas" Energies 7, no. 7: 4381-4396. https://doi.org/10.3390/en7074381
APA StyleNi, M., Shao, Z., & Chan, K. Y. (2014). Modeling of Proton-Conducting Solid Oxide Fuel Cells Fueled with Syngas. Energies, 7(7), 4381-4396. https://doi.org/10.3390/en7074381




