Energy Management of a Hybrid-Power Gas Engine-Driven Heat Pump
Abstract
:1. Introduction
2. Description of Energy Management on the Coaxial Parallel-Type HPGHP System

3. Model of the HPGHP System
3.1. Model of the Engine

3.2. Model of the Motor [12]
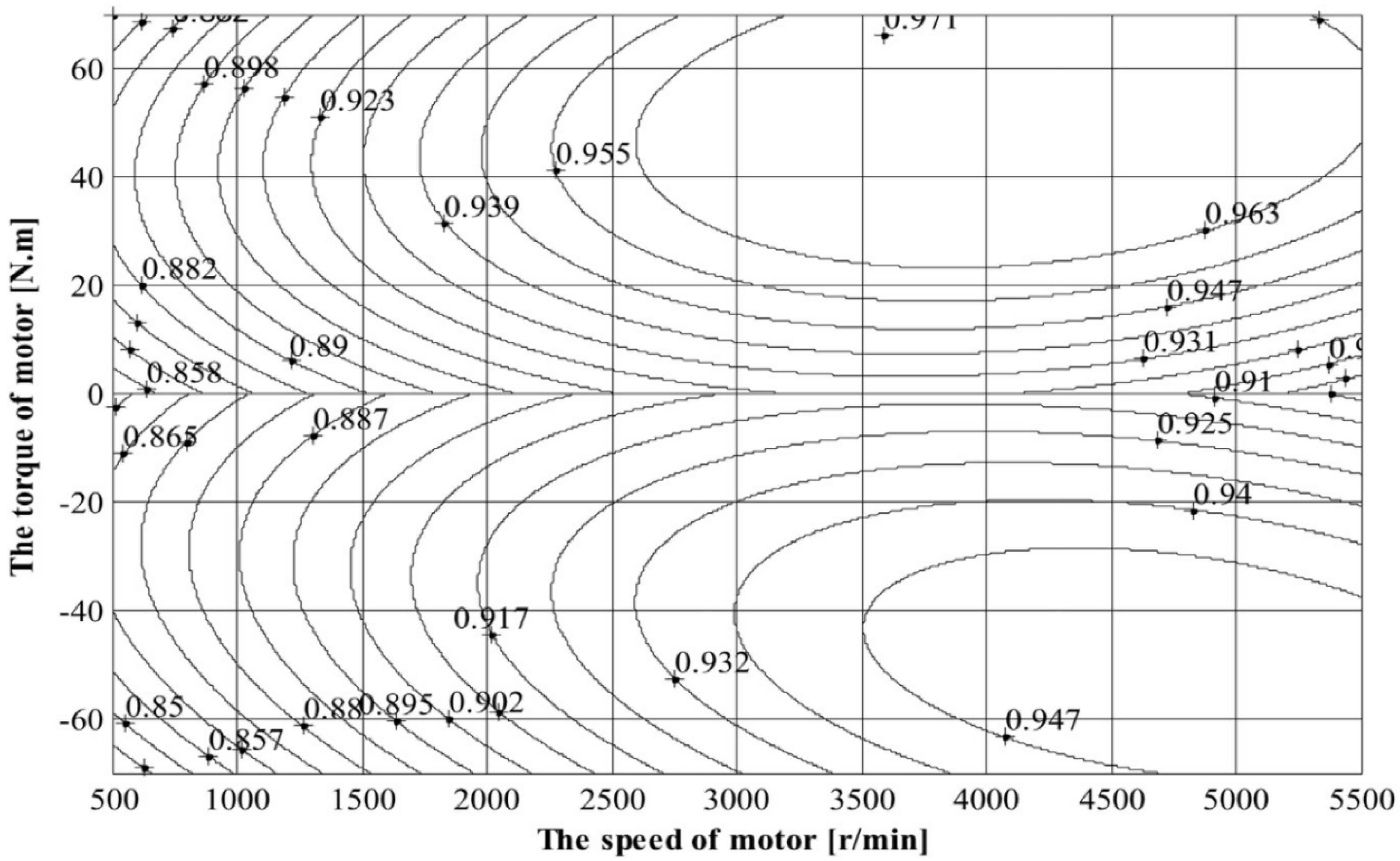
4. The Energy Management Optimization Strategy of the HPGHP System
4.1. The Comprehensive Efficiency of the HPGHP System
4.1.1. The Comprehensive Efficiency of the HPGHP System under Charging Conditions

4.1.2. The Comprehensive Efficiency of the HPGHP System under Discharging Conditions

4.2. The Energy Management Optimization Strategy Model of the HPGHP System [15]
4.2.1. The Energy Management Optimization Strategy Model of the HPGHP System under Charging Conditions
4.2.2. The Energy Management Optimization Strategy Model of the HPGHP System under Discharging Conditions
4.3. The Results of Energy Management Strategy
4.3.1. The Energy Management Optimization Strategy of the HPGHP System under Charging Conditions
| Parameter | ||||
|---|---|---|---|---|
| Value range | 0.8–0.95 | 0.9–0.98 | 0.8–0.9 | 0.75–0.92 |
| Selected value | 0.9 | 0.92 | 0.85 | 0.9 |
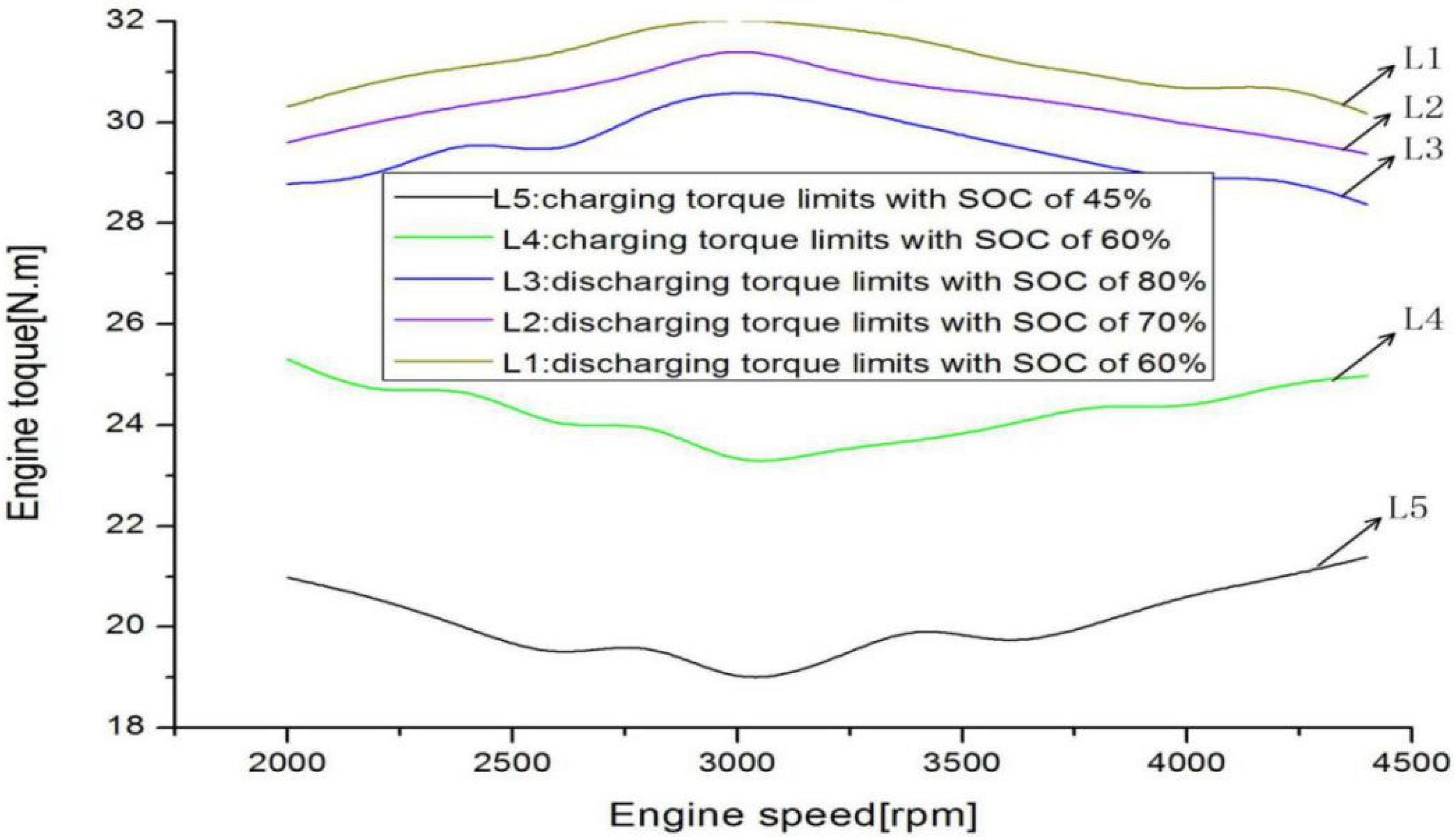

4.3.2. The Energy Management Optimization Strategy of the HPGHP System under Discharging Conditions
5. Control Strategy
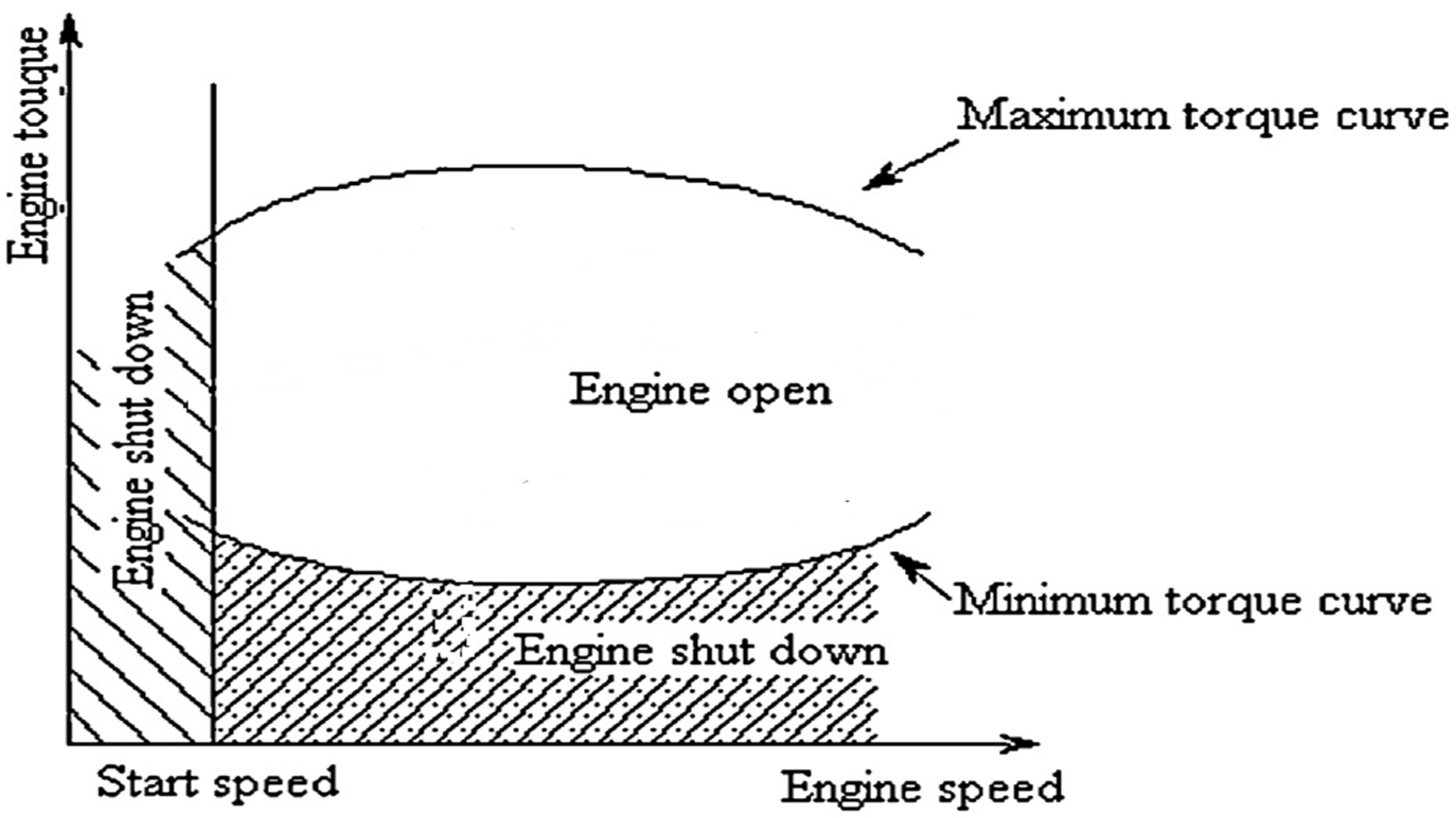
| Operation Mode | Boundary Conditions |
|---|---|
| mode D | ωlow < ω < ωhigh and Tlow < T < Thigh |
| mode C | ωlow < ω < ωhigh and T < Tlow and SOC < SOChigh |
| mode L | ωlow < ω < ωhigh and Thigh < T and SOC > SOClow |
| mode M | ω > ωhigh or ω < ωlow and SOC > SOClow |
| mode S | SOC < SOClow |

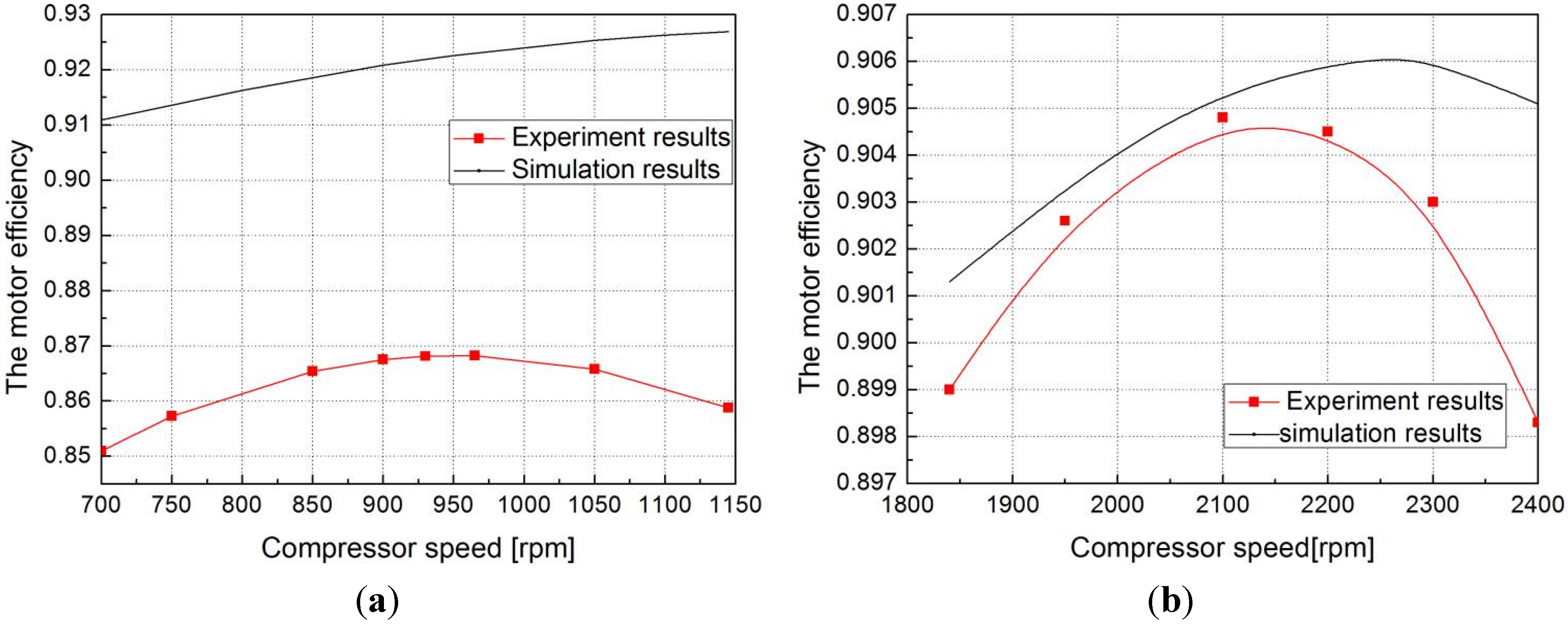
6. Simulation and Experimental Comparison








7. Conclusions
- (1)
- A comprehensive charging/discharging efficiency model and the energy management optimization strategy model is established. The results show that the comprehensive charging efficiency of the HPGHP system under charging/discharging conditions relates to the operating points, the engine and motor efficiency, respectively.
- (2)
- Different charging/discharging torque limits are obtained. The results show that the battery packs should be charged only when the required power is less than the charging power limit. In addition, the discharging torque limits become higher and the charging torque limits become lower when the SOC value becomes lower. The motor starts running only when the demand torque or power becomes larger at this moment.
- (3)
- In the HPGHP system operation process, during 3600 s of run-time, the SOC value of battery packs ranges between 0.58 and 0.705, the fuel consumption rate reaches a minimum value of approximately 291.3 g/(kW h) when the compressor speed is 1550 rpm in mode D and ranges between 291–330 g/(kW h), the engine thermal efficiency and comprehensive efficiency reach a maximum value of approximately 0.2727/0.2648 when the compressor speed is 1575 rpm/1475 rpm in mode D, respectively and ranges between 0.2400 and 0.2750, 0.2300 and 0.2700, respectively. In general, the fuel consumption rate reach a minimum value of approximately 291.3 g/(kW h) when the compressor speed is nearly 1550 rpm in mode D, the engine thermal efficiency and comprehensive efficiency reach maximum values of approximately 0.2727/0.2648 when the compressor speed is 1575 rpm/1475 rpm, respectively, in mode D. In addition, the motor efficiency can be maintained above 0.85 in either mode. Finally, because of the application of the gas engine economical zone control strategy to the HPGHP system, the fuel consumption rate is about 1.6% less than that analyzed in Wang et al. [9].
Acknowledgments
Author Contributions
Conflicts of Interest
Nomenclature
| ηf | the thermal efficiency of the gas engine |
| Tf | the torque of the gas engine (Nm) |
| ωf | the speed of the gas engine (rpm) |
| Td | the torque of the motor (Nm) |
| ωd | the speed of the motor (rpm) |
| ηd | the thermal efficiency of the motor |
| ηdc | the motor charging efficiency |
| ηdf | the motor discharging efficiency |
| ηb | transmission efficiency (%) |
| ηn | inverter efficiency (%) |
average comprehensive efficiency (%) | |
| Pq | gas output power (KW) |
| Pf | the output power of the engine (KW) |
| Pdc | the battery charging power (KW) |
| Pd | the motor input power (KW) |
the battery discharging power (KW) | |
| Pd’ | the motor output power (KW) |
| ω | the speed of the compressor (rpm) |
| Py | input power of the compressor (KW) |
| Pqd | gas bottle equivalent input power of the battery (KW) |
Subscripts
| y | the compressor |
| dc | the condition of charging |
| df | the condition of discharging |
| f | the engine |
| d | the motor |
| max | the maximum value |
| min | the minimum value |
| η | efficiency (%) |
| T | the torque (Nm) |
| ω | the speed (rpm) |
Abbreviations
| COP | coefficient of performance |
| GHP | gas engine heat pump |
| HPGHP | hybrid-power gas engine heat pump |
| SOC | the state of charge |
References
- Tyrus, J.M.; Long, R.M.; Kramskaya, M. Hybrid electric sport utility vehicles. IEEE Trans. Veh. Technol. 2004, 53, 1607–1622. [Google Scholar] [CrossRef]
- Huang, J.Y.; Gonzalez, L.F. On parallel hybrid-electric propulsion system for unmanned aerial vehicles. Prog. Aerosp. Sci. 2012, 51, 1–17. [Google Scholar] [CrossRef] [Green Version]
- Huang, K.D.; Tzeng, S.C. A new parallel-type hybrid electric-vehicle. J. Appl. Energy 2004, 79, 51–64. [Google Scholar] [CrossRef]
- Juang, Y.S.; Lin, S.S.; Kao, H.P. A knowledge management system for series-parallel availability optimization and design. J. Expert Syst. Appl. 2008, 34, 181–193. [Google Scholar] [CrossRef]
- Borba, B.S.M.C.; Szklo, A.; Schaeffer, R. Plug-in hybrid electric vehicles as a way to maximize the integration of variable renewable energy in power systems: The case of wind generation in northeastern Brazil. Energy 2012, 37, 469–481. [Google Scholar] [CrossRef]
- Elgendy, E.; Schmidt, J. Experimental study of gas engine driven air to water heat pump in cooling mode. Energy 2010, 35, 2461–2467. [Google Scholar] [CrossRef]
- Huang, X. Analysis of Economic Operation and Thermodynamic of the Gas Heat Pump System; Beijing Institute of Civil Engineering and Architecture: Beijing, China, 2011. [Google Scholar]
- Li, Y.L.; Zhang, X.S.; Cai, L. A novel parallel-type hybrid power gas engine-driven heat pump system. Int. J. Refrig. 2007, 30, 1134–1142. [Google Scholar] [CrossRef]
- Wang, Y.; Cai, L.; Yu, Y.; Zhang, X. Performance study of parallel-type hybrid-power gas engine-driven heat pump system. Energy Build. 2013, 62, 37–44. [Google Scholar] [CrossRef]
- Wang, J.Y.; Cai, L.; Wang, Y.W.; Maa, Y.; Zhang, X. Modeling and optimization matching on drive system of a coaxial parallel-type hybrid-power gas engine heat pump. Energy 2013, 55, 1196–1204. [Google Scholar] [CrossRef]
- Zhou, G. Universal characteristics curve plotting method based on MATLAB. I.C.E Power Plant 2009, 2, 34–36. [Google Scholar]
- Jiang, W.; Cai, L.; Wang, J.; Deng, W.; Zhang, X. Simulation and validation of a hybrid-power gas engine heat pump. Int. J. Refrig. 2014, 50, 114–126. [Google Scholar] [CrossRef]
- Kim, C.; Goong, E.N.; Lee, S. Fuel economy optimization for parallel hybrid vehicle with CVT. SAE Paper 1999, 1148, 337–342. [Google Scholar]
- Dorri, M.; Shamekhi, A.H. Design and optimization of a new control strategy in a parallel hybrid electric vehicle in order to improve fuel economy. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2011, 225, 747–759. [Google Scholar] [CrossRef]
- Shu, H. Study on Energy Management Strategy for Parallel Hybrid Electric Vehicles; Chongqing University: Chongqing, China, 2008. [Google Scholar]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meng, Q.; Cai, L.; Ji, W.; Yan, J.; Zhang, T.; Zhang, X. Energy Management of a Hybrid-Power Gas Engine-Driven Heat Pump. Energies 2015, 8, 11254-11275. https://doi.org/10.3390/en81011254
Meng Q, Cai L, Ji W, Yan J, Zhang T, Zhang X. Energy Management of a Hybrid-Power Gas Engine-Driven Heat Pump. Energies. 2015; 8(10):11254-11275. https://doi.org/10.3390/en81011254
Chicago/Turabian StyleMeng, Qingkun, Liang Cai, Wenxiu Ji, Jie Yan, Tao Zhang, and Xiaosong Zhang. 2015. "Energy Management of a Hybrid-Power Gas Engine-Driven Heat Pump" Energies 8, no. 10: 11254-11275. https://doi.org/10.3390/en81011254
APA StyleMeng, Q., Cai, L., Ji, W., Yan, J., Zhang, T., & Zhang, X. (2015). Energy Management of a Hybrid-Power Gas Engine-Driven Heat Pump. Energies, 8(10), 11254-11275. https://doi.org/10.3390/en81011254





