A Simple Expression for the Tortuosity of Gas Transport Paths in Solid Oxide Fuel Cells’ Porous Electrodes
Abstract
:1. Introduction
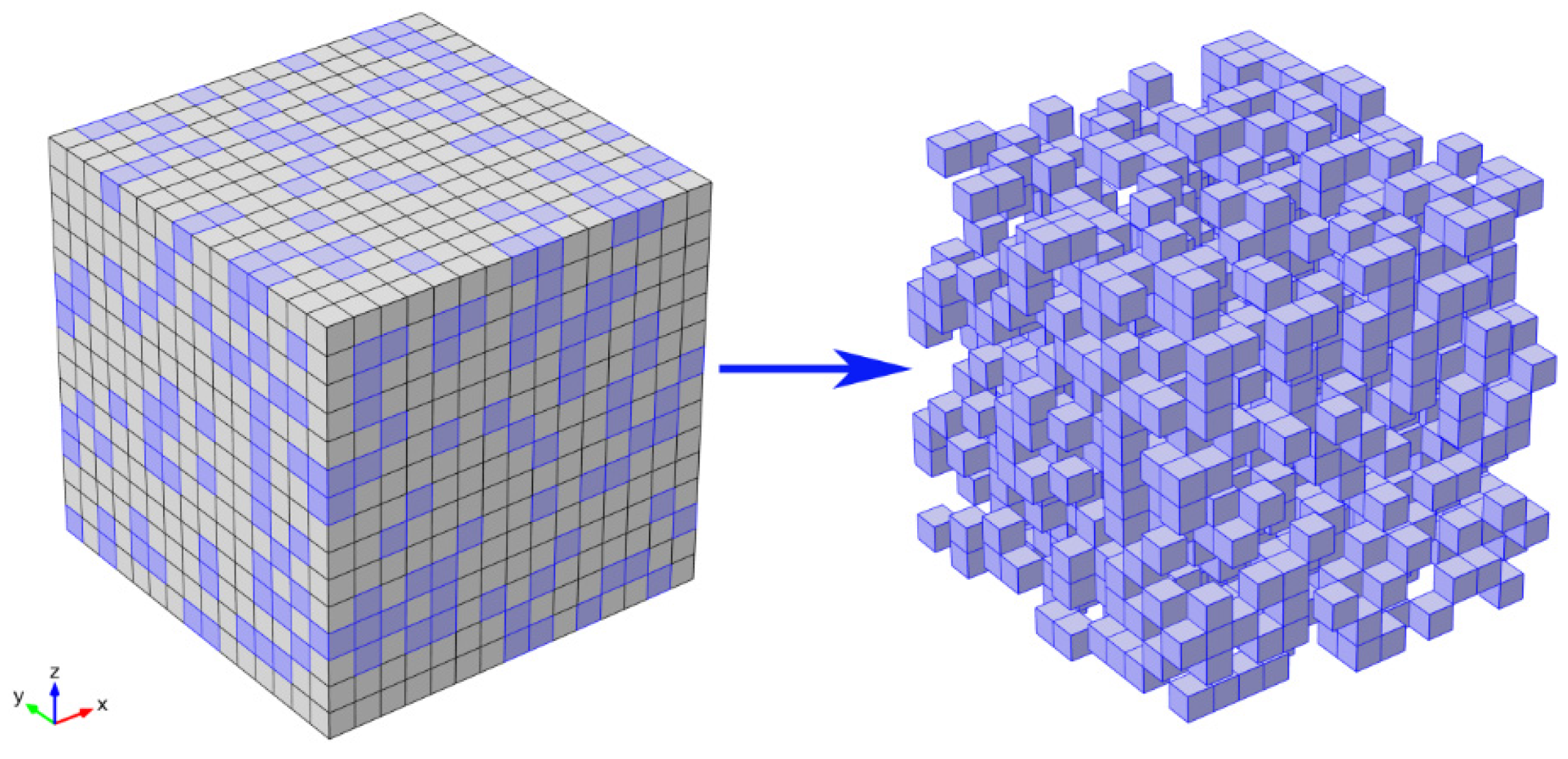
2. Theory

3. Results and Discussion


4. Summary
Acknowledgments
Author Contributions
Conflicts of Interest
Nomenclature
Bulk gas diffusion coefficient | |
Effective gas diffusion coefficient | |
Porosity of electrode | |
Tortuosity of gas transport paths | |
Straight distance | |
Average actual path length | |
| , , | Number of lattice unit in x, y and z direction |
Cross-section of representative volume element | |
Cross-section of the first typical path | |
Cross-section of the second typical path | |
Total cross section area of the tortuous paths | |
Molar flux | |
Molar concentration of gas at the top (bottom) surface of representative volume element |
References
- Mukhopadhyay, M.; Mukhopadhyay, J.; Das Sharma, A.; Basu, R.N. Effect of anode configuration on electrical properties and cell polarization in planar anode supported SOFC. Solid State Ion. 2013, 233, 20–31. [Google Scholar] [CrossRef]
- Yan, M.; Fu, P.; Chen, Q.Y.; Wang, Q.W.; Zeng, M.; Pandit, J. Electrical performance and carbon deposition differences between the bi-layer interconnector and conventional straight interconnector solid oxide fuel cell. Energies 2014, 7, 4601–4613. [Google Scholar] [CrossRef]
- Zhang, Z.; Chen, J.; Yue, D.; Yang, G.; Ye, S.; He, C.; Wang, W.; Yuan, J.; Huang, N. Three-dimensional CFD modeling of transport phenomena in a cross-flow anode-supported planar SOFC. Energies 2013, 7, 80–98. [Google Scholar] [CrossRef]
- Mukhopadhyay, M.; Mukhopadhyay, J.; Das Sharma, A.; Basu, R.N. Engineered anode structure for enhanced electrochemical performance of anode-supported planar solid oxide fuel cell. Int. J. Hydrog. Energy 2012, 37, 2524–2534. [Google Scholar] [CrossRef]
- Zheng, K.; Li, L.; Ni, M. Investigation of the electrochemical active thickness of solid oxide fuel cell anode. Int. J. Hydrog. Energy 2014, 39, 12904–12912. [Google Scholar] [CrossRef]
- Cui, D.; Yang, C.; Huang, K.; Chen, F. Effects of testing configurations and cell geometries on the performance of a SOFC: A modeling approach. Int. J. Hydrog. Energy 2010, 35, 10495–10504. [Google Scholar] [CrossRef]
- Kong, W.; Gao, X.; Liu, S.; Su, S.; Chen, D. Optimization of the interconnect ribs for a cathode-supported solid oxide fuel cell. Energies 2014, 7, 295–313. [Google Scholar] [CrossRef]
- Chen, D.; Wang, H.; Zhang, S.; Tade, M.O.; Chen, H.; Shao, Z. Multi-scale model for solid oxide fuel cell with electrode containing mixed conducting material. AIChE J. 2015, 61, 3786–3803. [Google Scholar] [CrossRef]
- Kong, W.; Li, J.; Liu, S.; Lin, Z. The influence of interconnect ribs on the performance of planar solid oxide fuel cell and formulae for optimal rib sizes. J. Power Sources 2012, 204, 106–115. [Google Scholar] [CrossRef]
- Lichtner, A.Z.; Jauffrès, D.; Roussel, D.; Charlot, F.; Martin, C.L.; Bordia, R.K. Dispersion, connectivity and tortuosity of hierarchical porosity composite SOFC cathodes prepared by freeze-casting. J. Eur. Ceram. Soc. 2015, 35, 585–595. [Google Scholar] [CrossRef]
- Kong, W.; Zhang, Q.; Gao, X.; Zhang, J.; Chen, D.; Su, S. A method for predicting the tortuosity of pore phase in solid oxide fuel cells electrode. Int. J. Electrochem. Sci. 2015, 10, 5800–5811. [Google Scholar]
- Espinoza, M.; Sundén, B.; Andersson, M.; Yuan, J. Analysis of porosity and tortuosity in a 2d selected region of solid oxide fuel cell cathode using the lattice boltzmann method. ECS Trans. 2015, 65, 59–73. [Google Scholar] [CrossRef]
- Zheng, K.; Zhang, Y.; Li, L.; Ni, M. On the tortuosity factor of solid phase in solid oxide fuel cell electrodes. Int. J. Hydrog. Energy 2015, 40, 665–669. [Google Scholar] [CrossRef]
- Wilson, J.R.; Cronin, J.S.; Duong, A.T.; Rukes, S.; Chen, H.-Y.; Thornton, K.; Mumm, D.R.; Barnett, S. Effect of composition of (La0.8Sr0.2MnO3–Y2O3-stabilized ZrO2) cathodes: Correlating three-dimensional microstructure and polarization resistance. J. Power Sources 2010, 195, 1829–1840. [Google Scholar] [CrossRef]
- Iwai, H.; Shikazono, N.; Matsui, T.; Teshima, H.; Kishimoto, M.; Kishida, R.; Hayashi, D.; Matsuzaki, K.; Kanno, D.; Saito, M.; et al. Quantification of SOFC anode microstructure based on dual beam FIB-SEM technique. J. Power Sources 2010, 195, 955–961. [Google Scholar] [CrossRef]
- Berson, A.; Choi, H.W.; Pharoah, J.G. Determination of the effective gas diffusivity of a porous composite medium from the three-dimensional reconstruction of its microstructure. Phys. Rev. E 2011, 83. [Google Scholar] [CrossRef] [PubMed]
- Bertei, A.; Nucci, B.; Nicolella, C. Microstructural modeling for prediction of transport properties and electrochemical performance in SOFC composite electrodes. Chem. Eng. Sci. 2013, 101, 175–190. [Google Scholar] [CrossRef]
- Andersson, M.; Yuan, J.; Sundén, B. Review on modeling development for multiscale chemical reactions coupled transport phenomena in solid oxide fuel cells. Appl. Energy 2010, 87, 1461–1476. [Google Scholar] [CrossRef]
- Cooper, S.J.; Kishimoto, M.; Tariq, F.; Bradley, R.S.; Marquis, A.J.; Brandon, N.P.; Kilner, J.A.; Shearing, P.R. Microstructural analysis of an LSCF cathode using in situ tomography and simulation. ECS Trans. 2013, 57, 2671–2678. [Google Scholar] [CrossRef]
- Gostovic, D.; Smith, J.; Kundinger, D.; Jones, K.; Wachsman, E. Three-dimensional reconstruction of porous LSCF cathodes. Electrochem. Solid-State Lett. 2007, 10, B214–B217. [Google Scholar] [CrossRef]
- Joos, J.; Ender, M.; Carraro, T.; Weber, A.; Ivers-Tiffée, E. Representative volume element size for accurate solid oxide fuel cell cathode reconstructions from focused ion beam tomography data. Electrochim. Acta 2012, 82, 268–276. [Google Scholar] [CrossRef]
- Kishimoto, M.; Miyawaki, K.; Iwai, H.; Saito, M.; Yoshida, H. Effect of composition ratio of Ni-YSZ anode on distribution of effective three-phase boundary and power generation performance. Fuel Cells 2013, 13, 476–486. [Google Scholar] [CrossRef]
- Gunda, N.S.K.; Choi, H.-W.; Berson, A.; Kenney, B.; Karan, K.; Pharoah, J.G.; Mitra, S.K. Focused ion beam-scanning electron microscopy on solid-oxide fuel-cell electrode: Image analysis and computing effective transport properties. J. Power Sources 2011, 196, 3592–3603. [Google Scholar] [CrossRef]
- Kishimoto, M.; Iwai, H.; Saito, M.; Yoshida, H. Quantitative evaluation of solid oxide fuel cell porous anode microstructure based on focused ion beam and scanning electron microscope technique and prediction of anode overpotentials. J. Power Sources 2011, 196, 4555–4563. [Google Scholar] [CrossRef]
- Usseglio-Viretta, F.; Laurencin, J.; Delette, G.; Villanova, J.; Cloetens, P.; Leguillon, D. Quantitative microstructure characterization of a Ni–YSZ bi-layer coupled with simulated electrode polarisation. J. Power Sources 2014, 256, 394–403. [Google Scholar] [CrossRef]
- Wargo, E.A.; Kotaka, T.; Tabuchi, Y.; Kumbur, E.C. Comparison of focused ion beam versus nano-scale X-ray computed tomography for resolving 3-D microstructures of porous fuel cell materials. J. Power Sources 2013, 241, 608–618. [Google Scholar] [CrossRef]
- Brus, G.; Miyawaki, K.; Iwai, H.; Saito, M.; Yoshida, H. Tortuosity of an SOFC anode estimated from saturation currents and a mass transport model in comparison with a real micro-structure. Solid State Ion. 2014, 265, 13–21. [Google Scholar] [CrossRef]
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kong, W.; Zhang, Q.; Xu, X.; Chen, D. A Simple Expression for the Tortuosity of Gas Transport Paths in Solid Oxide Fuel Cells’ Porous Electrodes. Energies 2015, 8, 13953-13959. https://doi.org/10.3390/en81212406
Kong W, Zhang Q, Xu X, Chen D. A Simple Expression for the Tortuosity of Gas Transport Paths in Solid Oxide Fuel Cells’ Porous Electrodes. Energies. 2015; 8(12):13953-13959. https://doi.org/10.3390/en81212406
Chicago/Turabian StyleKong, Wei, Qiang Zhang, Xiuwen Xu, and Daifen Chen. 2015. "A Simple Expression for the Tortuosity of Gas Transport Paths in Solid Oxide Fuel Cells’ Porous Electrodes" Energies 8, no. 12: 13953-13959. https://doi.org/10.3390/en81212406
APA StyleKong, W., Zhang, Q., Xu, X., & Chen, D. (2015). A Simple Expression for the Tortuosity of Gas Transport Paths in Solid Oxide Fuel Cells’ Porous Electrodes. Energies, 8(12), 13953-13959. https://doi.org/10.3390/en81212406






