Symmetric-in-Plane Compression of Polyamide Pantographic Fabrics—Modelling, Experiments and Numerical Exploration
Abstract
:1. Introduction
1.1. Generalised Continua and Their Use to Model Pantographic Structures
1.2. Model-Driven Design and Synthesis of Pantographic Structures
1.3. Out-of-Plane and In-Plane Compression of Pantographic Fabrics
1.4. Plan of the Present Work
2. Mathematical and Numerical Modelling of Pantographic Structures
2.1. Geometrically Non Linear Second Gradient Elasticity Model
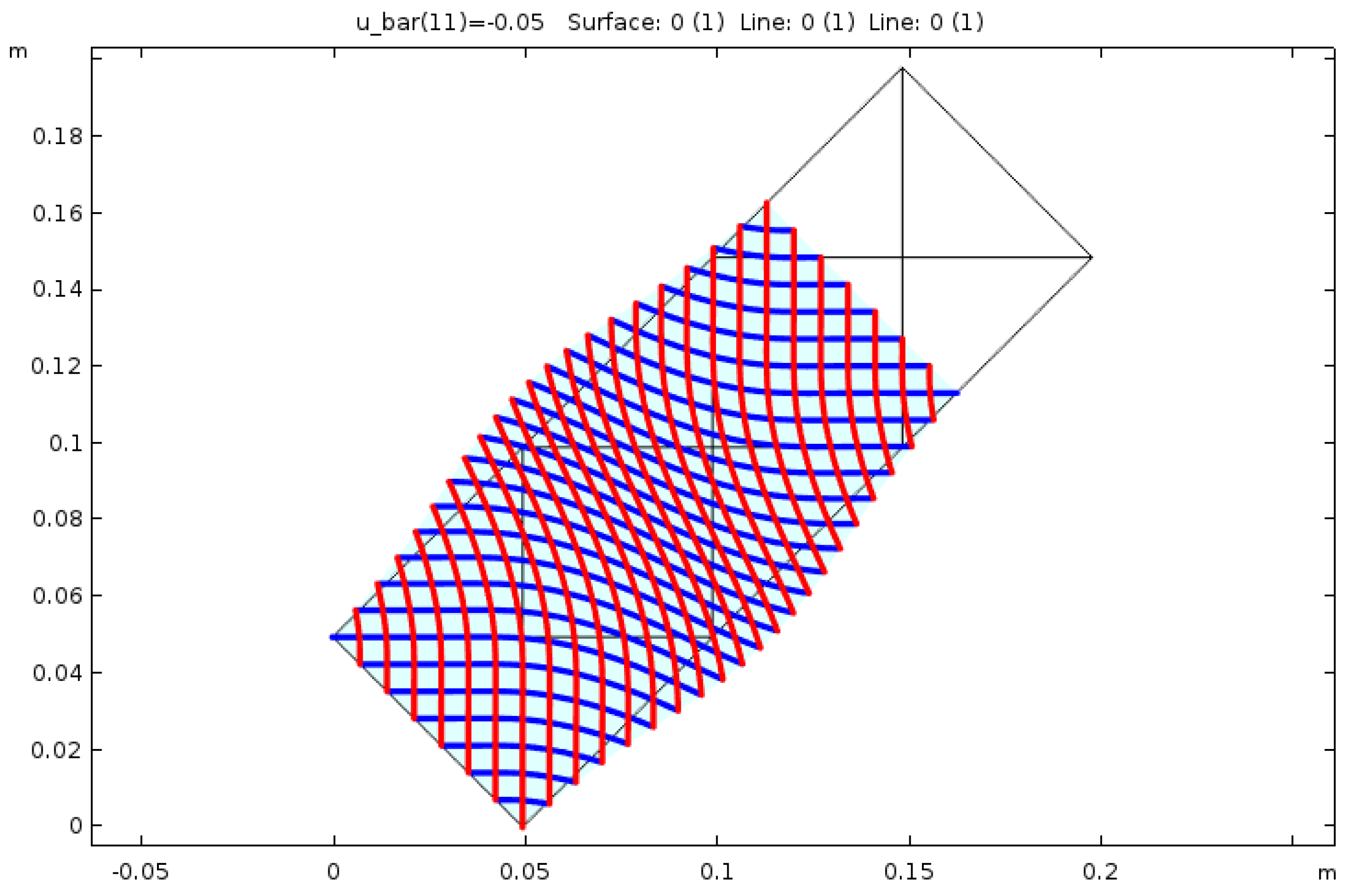
2.1.1. Kinematics
2.1.2. Potential Energy
External Work
Strain Energy—Linear Elasticity
Strain Energy—Higher Deformations
2.1.3. Variational Approach
- prescribed null displacement at the lower edge:
- no applied load on either side:
- prescribed displacement on the upper edge:
2.2. Finite Elements Numerical Model
Function Space
Modelling with Software
3. Experimental Specimen
3.1. Studied Class of Pantographic Structure
3.2. Modelled Specimen: Production and Characterisation
- Width of beam: 0.9 mm
- Height of beam: 4 mm
- Width of pivot: 0.9 mm
- Height of pivot: 1 mm
3.3. Experimental Procedure
3.4. Outputs of the Experiments
Measurement Uncertainty
4. Model Fitting and Numerical Validation
4.1. Numerical Parameters
4.1.1. Fixed Parameters
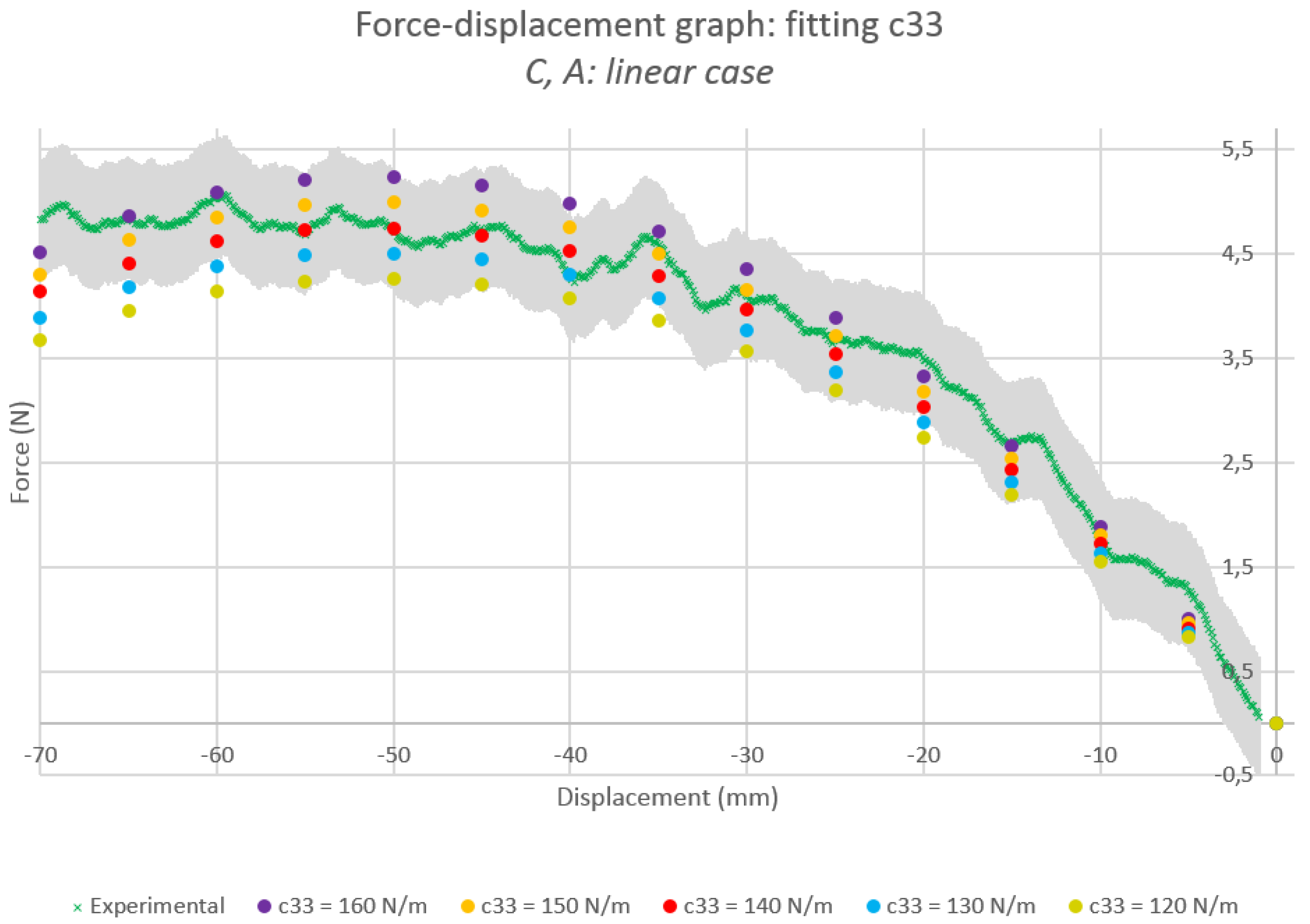
4.1.2. Parameters to Be Fitted
- begin with the linear case values: ; and
- fit to experimental results (force-displacement graph)
4.2. First Experiment—Single Swelling
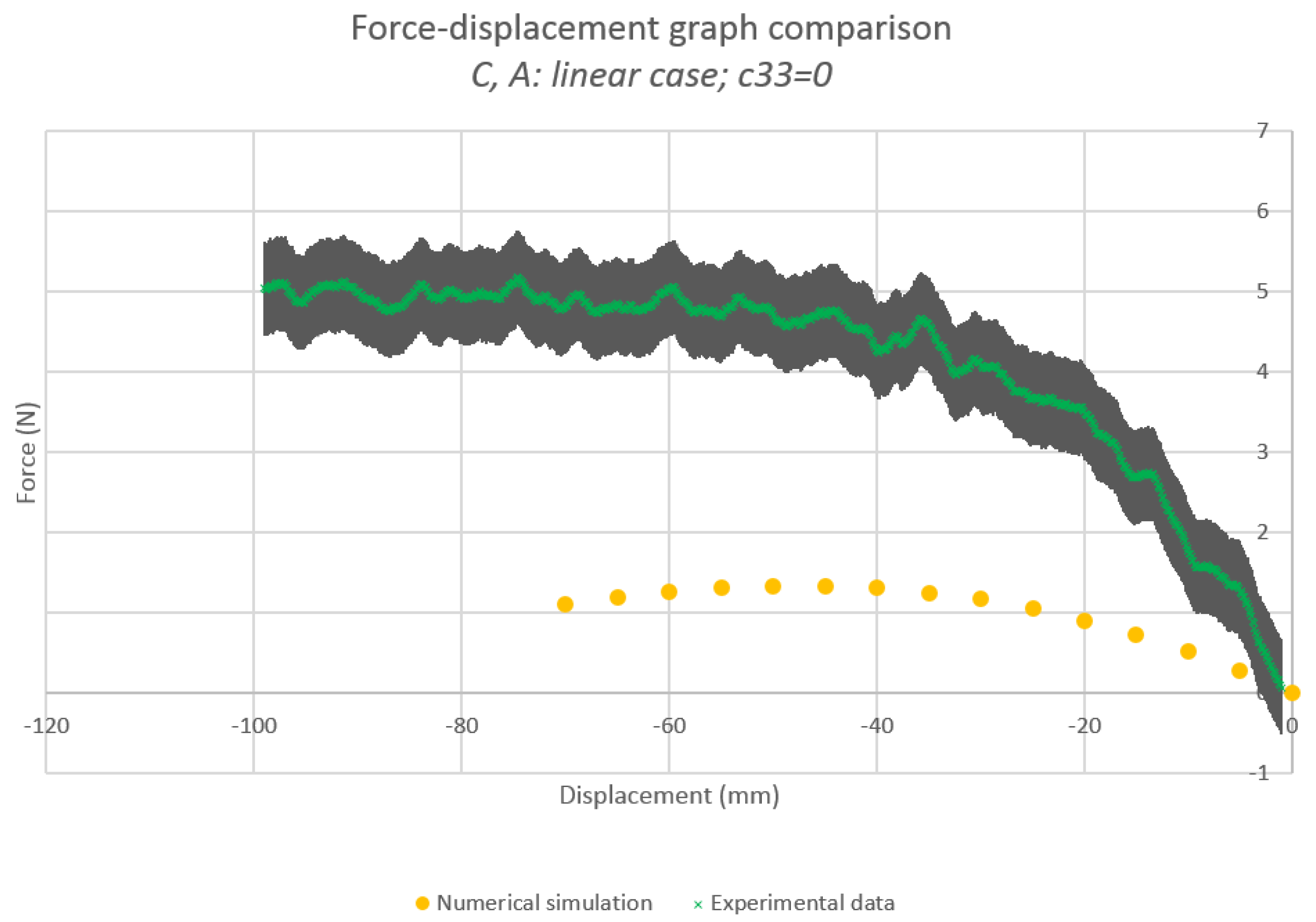
4.2.1. Without Torsional Energy
Parameter Values
Force-Displacement Graph Comparison
4.2.2. Fitting the Torsional Parameter
4.3. Second Experiment—Two Swellings
Parameter Values
Qualitative Comparison
5. Conclusion and Possible Future Work
5.1. Work Done
5.2. Results
5.3. Possible Future Work
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Maugin, G.A. Generalized continuum mechanics: What do we mean by that? In Mechanics of Generalized Continua; Springer: New York, NY, USA, 2010; pp. 3–13. [Google Scholar]
- Green, A.E. Micro-materials and multipolar continuum mechanics. Int. J. Eng. Sci. 1965, 3, 533–537. [Google Scholar] [CrossRef]
- Mindlin, R.D. Second gradient of strain and surface-tension in linear elasticity. Int. J. Solids Struct. 1965, 1, 417–438. [Google Scholar] [CrossRef]
- Toupin, R.A. Theories of Elasticity with Couple-Stress; Springer: Berlin, Germany, 1964. [Google Scholar]
- Dell’Isola, F.; Corte, A.D.; Giorgio, I. Higher-gradient continua: The legacy of Piola, Mindlin, Sedov and Toupin and some future research perspectives. Math. Mech. Solids 2017, 22, 852–872. [Google Scholar] [CrossRef]
- dell’Isola, F.; Giorgio, I.; Pawlikowski, M.; Rizzi, N.L. Large deformations of planar extensible beams and pantographic lattices: Heuristic homogenization, experimental and numerical examples of equilibrium. Proc. R. Soc. A Math. Phys. Eng. Sci. 2016, 472, 20150790. [Google Scholar] [CrossRef] [Green Version]
- Dell’Isola, F.; Lekszycki, T.; Pawlikowski, M.; Grygoruk, R.; Greco, L. Designing a light fabric metamaterial being highly macroscopically tough under directional extension: First experimental evidence. Z. Für Angew. Math. Und Phys. 2015, 66, 3473–3498. [Google Scholar] [CrossRef]
- Turco, E.; Dell’Isola, F.; Rizzi, N.L.; Grygoruk, R.; Müller, W.H.; Liebold, C. Fiber rupture in sheared planar pantographic sheets: Numerical and experimental evidence. Mech. Res. Commun. 2016, 76, 86–90. [Google Scholar] [CrossRef] [Green Version]
- Turco, E.; Golaszewski, M.; Cazzani, A.; Rizzi, N.L. Large deformations induced in planar pantographic sheets by loads applied on fibers: Experimental validation of a discrete Lagrangian model. Mech. Res. Commun. 2016, 76, 51–56. [Google Scholar] [CrossRef]
- Turco, E.; Rizzi, N.L. Pantographic structures presenting statistically distributed defects: Numerical investigations of the effects on deformation fields. Mech. Res. Commun. 2016, 77, 65–69. [Google Scholar] [CrossRef]
- Turco, E.; Giorgio, I.; Misra, A.; Dell’Isola, F. King post truss as a motif for internal structure of (meta) material with controlled elastic properties. R. Soc. Open Sci. 2017, 4, 171153. [Google Scholar] [CrossRef] [Green Version]
- Turco, E.; Misra, A.; Pawlikowski, M.; Dell’Isola, F.; Hild, F. Enhanced Piola–Hencky discrete models for pantographic sheets with pivots without deformation energy: Numerics and experiments. Int. J. Solids Struct. 2018, 147, 94–109. [Google Scholar] [CrossRef] [Green Version]
- Andreaus, U.; Spagnuolo, M.; Lekszycki, T.; Eugster, S.R. A Ritz approach for the static analysis of planar pantographic structures modeled with nonlinear Euler–Bernoulli beams. Contin. Mech. Thermodyn. 2018, 30, 1103–1123. [Google Scholar] [CrossRef]
- Carcaterra, A.; dell’Isola, F.; Esposito, R.; Pulvirenti, M. Macroscopic description of microscopically strongly inhomogenous systems: A mathematical basis for the synthesis of higher gradients metamaterials. Arch. Ration. Mech. Anal. 2015, 218, 1239–1262. [Google Scholar] [CrossRef]
- Alibert, J.J.; Seppecher, P.; Dell’Isola, F. Truss modular beams with deformation energy depending on higher displacement gradients. Math. Mech. Solids 2003, 8, 51–73. [Google Scholar] [CrossRef]
- Abdoul-Anziz, H.; Seppecher, P. Strain gradient and generalized continua obtained by homogenizing frame lattices. Math. Mech. Complex Syst. 2018, 6, 213–250. [Google Scholar] [CrossRef] [Green Version]
- Steigmann, D.J.; Faulkner, M.G. Variational theory for spatial rods. J. Elast. 1993, 33, 1–26. [Google Scholar] [CrossRef] [Green Version]
- Spagnuolo, M.; Andreaus, U. A targeted review on large deformations of planar elastic beams: Extensibility, distributed loads, buckling and post-buckling. Math. Mech. Solids 2019, 24, 258–280. [Google Scholar] [CrossRef]
- Cazzani, A.; Malagù, M.; Turco, E.; Stochino, F. Constitutive models for strongly curved beams in the frame of isogeometric analysis. Math. Mech. Solids 2016, 21, 182–209. [Google Scholar] [CrossRef]
- Cazzani, A.; Malagù, M.; Turco, E. Isogeometric analysis: A powerful numerical tool for the elastic analysis of historical masonry arches. Contin. Mech. Thermodyn. 2016, 28, 139–156. [Google Scholar] [CrossRef]
- Eremeyev, V.A.; Lebedev, L.P.; Altenbach, H. Foundations of Micropolar Mechanics; Springer Science & Business Media: Berlin, Germany, 2012. [Google Scholar]
- Altenbach, H.; Eremeyev, V.A. On the linear theory of micropolar plates. ZAMM-J. Appl. Math. Mech. Für Angew. Math. Und Mech. 2009, 89, 242–256. [Google Scholar] [CrossRef] [Green Version]
- Altenbach, H.; Bîrsan, M.; Eremeyev, V.A. Cosserat-type rods. In Generalized Continua from the Theory to Engineering Applications; Springer: Vienna, Austria, 2013; pp. 179–248. [Google Scholar]
- Eremeyev, V.A. Strongly anisotropic surface elasticity and antiplane surface waves. Philos. Trans. R. Soc. A 2020, 378, 20190100. [Google Scholar] [CrossRef] [Green Version]
- Eremeyev, V.A.; Sharma, B.L. Anti-plane surface waves in media with surface structure: Discrete vs. Contin. Model. Int. J. Eng. Sci. 2019, 143, 33–38. [Google Scholar] [CrossRef] [Green Version]
- Dell’Isola, F.; Placidi, L. Variational principles are a powerful tool also for formulating field theories. In Variational Models and Methods in Solid and Fluid Mechanics; Springer: Vienna, Austria, 2011; pp. 1–15. [Google Scholar]
- Dell’Isola, F.; Seppecher, P.; Corte, A.D. The postulations á la D’Alembert and á la Cauchy for higher gradient continuum theories are equivalent: A review of existing results. Proc. R. Soc. A Math. Phys. Eng. Sci. 2015, 471, 20150415. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dell’Isola, F.; Madeo, A.; Seppecher, P. Cauchy tetrahedron argument applied to higher contact interactions. Arch. Ration. Mech. Anal. 2016, 219, 1305–1341. [Google Scholar] [CrossRef] [Green Version]
- Eremeyev, V.A.; Dell’Isola, F.; Boutin, C.; Steigmann, D. Linear pantographic sheets: Existence and uniqueness of weak solutions. J. Elast. 2018, 132, 175–196. [Google Scholar] [CrossRef] [Green Version]
- Placidi, L.; El Dhaba, A.R. Semi-inverse method à la Saint-Venant for two-dimensional linear isotropic homogeneous second-gradient elasticity. Math. Mech. Solids 2017, 22, 919–937. [Google Scholar] [CrossRef]
- Placidi, L.; Andreaus, U.; Della Corte, A.; Lekszycki, T. Gedanken experiments for the determination of two-dimensional linear second gradient elasticity coefficients. Z. Für Angew. Math. Und Phys. 2015, 66, 3699–3725. [Google Scholar] [CrossRef]
- Placidi, L.; Andreaus, U.; Giorgio, I. Identification of two-dimensional pantographic structure via a linear D4 orthotropic second gradient elastic model. J. Eng. Math. 2017, 103, 1–21. [Google Scholar] [CrossRef]
- Khakalo, S.; Niiranen, J. Isogeometric analysis of higher-order gradient elasticity by user elements of a commercial finite element software. Comput.-Aided Des. 2017, 82, 154–169. [Google Scholar] [CrossRef]
- Niiranen, J.; Khakalo, S.; Balobanov, V.; Niemi, A.H. Variational formulation and isogeometric analysis for fourth-order boundary value problems of gradient-elastic bar and plane strain/stress problems. Comput. Methods Appl. Mech. Eng. 2016, 308, 182–211. [Google Scholar] [CrossRef]
- Balobanov, V.; Kiendl, J.; Khakalo, S.; Niiranen, J. Kirchhoff–Love shells within strain gradient elasticity: Weak and strong formulations and an H3-conforming isogeometric implementation. Comput. Methods Appl. Mech. Eng. 2019, 344, 837–857. [Google Scholar] [CrossRef]
- Abali, B.E.; Müller, W.H.; dell’Isola, F. Theory and computation of higher gradient elasticity theories based on action principles. Arch. Appl. Mech. 2017, 87, 1495–1510. [Google Scholar] [CrossRef] [Green Version]
- Abali, B.E.; Müller, W.H.; Eremeyev, V.A. Strain gradient elasticity with geometric nonlinearities and its computational evaluation. Mech. Adv. Mater. Mod. Process. 2015, 1, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Dell’Isola, F.; d’Agostino, M.V.; Madeo, A.; Boisse, P.; Steigmann, D. Minimization of shear energy in two dimensional continua with two orthogonal families of inextensible fibers: The case of standard bias extension test. J. Elast. 2016, 122, 131–155. [Google Scholar] [CrossRef]
- Dell’Isola, F.; Della Corte, A.; Greco, L.; Luongo, A. Plane bias extension test for a continuum with two inextensible families of fibers: A variational treatment with Lagrange multipliers and a perturbation solution. Int. J. Solids Struct. 2016, 81, 1–12. [Google Scholar] [CrossRef]
- Placidi, L.; Greco, L.; Bucci, S.; Turco, E.; Rizzi, N.L. A second gradient formulation for a 2D fabric sheet with inextensible fibres. Z. Für Angew. Math. Und Phys. 2016, 67, 114. [Google Scholar] [CrossRef]
- Eremeyev, V.A. Two-and three-dimensional elastic networks with rigid junctions: Modeling within the theory of micropolar shells and solids. Acta Mech. 2019, 230, 3875–3887. [Google Scholar] [CrossRef] [Green Version]
- Eremeyev, V.A. A nonlinear model of a mesh shell. Mech. Solids 2018, 53, 464–469. [Google Scholar] [CrossRef]
- Sharma, B.L.; Eremeyev, V.A. Wave transmission across surface interfaces in lattice structures. Int. J. Eng. Sci. 2019, 145, 103173. [Google Scholar] [CrossRef]
- Barchiesi, E.; Spagnuolo, M.; Placidi, L. Mechanical metamaterials: A state of the art. Math. Mech. Solids 2019, 24, 212–234. [Google Scholar] [CrossRef]
- Dell’Isola, F.; Seppecher, P.; Alibert, J.J.; Lekszycki, T.; Grygoruk, R.; Pawlikowski, M.; Steigmann, D.; Giorgio, I.; Andreaus, U.; Turco, E.; et al. Pantographic metamaterials: An example of mathematically driven design and of its technological challenges. Contin. Mech. Thermodyn. 2019, 31, 851–884. [Google Scholar] [CrossRef] [Green Version]
- Spagnuolo, M.; Peyre, P.; Dupuy, C. Phenomenological aspects of quasi-perfect pivots in metallic pantographic structures. Mech. Res. Commun. 2019, 101, 103415. [Google Scholar] [CrossRef]
- Dell’Erba, R. Rheo-mechanical and rheo-optical characterisation of ultra high molecular mass poly (methylmethacrylate) in solution. Polymer 2001, 42, 2655–2663. [Google Scholar] [CrossRef] [Green Version]
- Avella, M.; Dell’Erba, R.; Martuscelli, E. Fiber reinforced polypropylene: Influence of iPP molecular weight on morphology, crystallization, and thermal and mechanical properties. Polym. Compos. 1996, 17, 288–299. [Google Scholar] [CrossRef]
- Avella, M.; Casale, L.; Dell’Erba, R.; Martuscelli, E. Broom fibers as reinforcement for thermoplastic matrices. In Macromolecular Symposia; Hüthig & Wepf Verlag: Napoli, Italy, 1998. [Google Scholar]
- dell’Isola, F.; Seppecher, P.; Spagnuolo, M.; Barchiesi, E.; Hild, F.; Lekszycki, T.; Giorgio, I.; Placidi, L.; Andreaus, U.; Cuomo, M.; et al. Advances in pantographic structures: Design, manufacturing, models, experiments and image analyses. Contin. Mech. Thermodyn. 2019, 31, 1231–1282. [Google Scholar] [CrossRef] [Green Version]
- Golaszewski, M.; Grygoruk, R.; Giorgio, I.; Laudato, M.; Di Cosmo, F. Metamaterials with relative displacements in their microstructure: Technological challenges in 3D printing, experiments and numerical predictions. Contin. Mech. Thermodyn. 2019, 31, 1015–1034. [Google Scholar] [CrossRef]
- Dell’Isola, F.; Turco, E.; Misra, A.; Vangelatos, Z.; Grigoropoulos, C.; Melissinaki, V.; Farsari, M. Force–displacement relationship in micro-metric pantographs: Experiments and numerical simulations. Comptes Rendus Mécanique 2019, 347, 397–405. [Google Scholar] [CrossRef]
- Nejadsadeghi, N.; De Angelo, M.; Drobnicki, R.; Lekszycki, T.; dell’Isola, F.; Misra, A. Parametric experimentation on pantographic unit cells reveals local extremum configuration. Exp. Mech. 2019, 59, 927–939. [Google Scholar] [CrossRef] [Green Version]
- Yang, H.; Müller, W.H. Computation and experimental comparison of the deformation behavior of pantographic structures with different micro-geometry under shear and torsion. J. Theor. Appl. Mech. 2019, 57, 421–434. [Google Scholar] [CrossRef]
- Yang, H.; Ganzosch, G.; Giorgio, I.; Abali, B.E. Material characterization and computations of a polymeric metamaterial with a pantographic substructure. Z. Für Angew. Math. Und Phys. 2018, 69, 105. [Google Scholar] [CrossRef]
- Misra, A.; Singh, V. Micromechanical model for viscoelastic materials undergoing damage. Contin. Mech. Thermodyn. 2013, 25, 343–358. [Google Scholar] [CrossRef]
- Misra, A.; Poorsolhjouy, P. Granular micromechanics model for damage and plasticity of cementitious materials based upon thermomechanics. Math. Mech. Solids 2015. [Google Scholar] [CrossRef]
- Misra, A.; Singh, V. Thermomechanics-based nonlinear rate-dependent coupled damage-plasticity granular micromechanics model. Contin. Mech. Thermodyn. 2015, 27, 787–817. [Google Scholar] [CrossRef]
- De Angelo, M.; Spagnuolo, M.; D’annibale, F.; Pfaff, A.; Hoschke, K.; Misra, A.; Dupuy, C.; Peyre, P.; Dirrenberger, J.; Pawlikowski, M. The macroscopic behavior of pantographic sheets depends mainly on their microstructure: Experimental evidence and qualitative analysis of damage in metallic specimen. Contin. Mech. Thermodyn. 2019, 31, 1181–1203. [Google Scholar] [CrossRef]
- Misra, A.; Lekszycki, T.; Giorgio, I.; Ganzosch, G.; Müller, W.H.; Dell’Isola, F. Pantographic metamaterials show atypical Poynting effect reversal. Mech. Res. Commun. 2018, 89, 6–10. [Google Scholar] [CrossRef] [Green Version]
- Spagnuolo, M.; Barcz, K.; Pfaff, A.; Dell’Isola, F.; Franciosi, P. Qualitative pivot damage analysis in aluminum printed pantographic sheets: Numerics and experiments. Mech. Res. Commun. 2017, 83, 47–52. [Google Scholar] [CrossRef] [Green Version]
- Yildizdag, M.E.; Tran, C.A.; Barchiesi, E.; Spagnuolo, M.; dell’Isola, F.; Hild, F. A Multi-disciplinary Approach for Mechanical Metamaterial Synthesis: A Hierarchical Modular Multiscale Cellular Structure Paradigm. In State of the Art and Future Trends in Material Modeling; Springer: Cham, Switzerland, 2019; pp. 485–505. [Google Scholar]
- Dell’Isola, F.; Steigmann, D.J. (Eds.) Discrete and Continuum Models for Complex Metamaterials; Cambridge University Press: Cambridge, UK, 2020. [Google Scholar]
- Barchiesi, E.; Ganzosch, G.; Liebold, C.; Placidi, L.; Grygoruk, R.; Müller, W.H. Out-of-plane buckling of pantographic fabrics in displacement-controlled shear tests: Experimental results and model validation. Contin. Mech. Thermodyn. 2019, 31, 33–45. [Google Scholar] [CrossRef]
- Auffray, N.; Dirrenberger, J.; Rosi, G. A complete description of bi-dimensional anisotropic strain-gradient elasticity. Int. J. Solids Struct. 2015, 69, 195–206. [Google Scholar] [CrossRef]





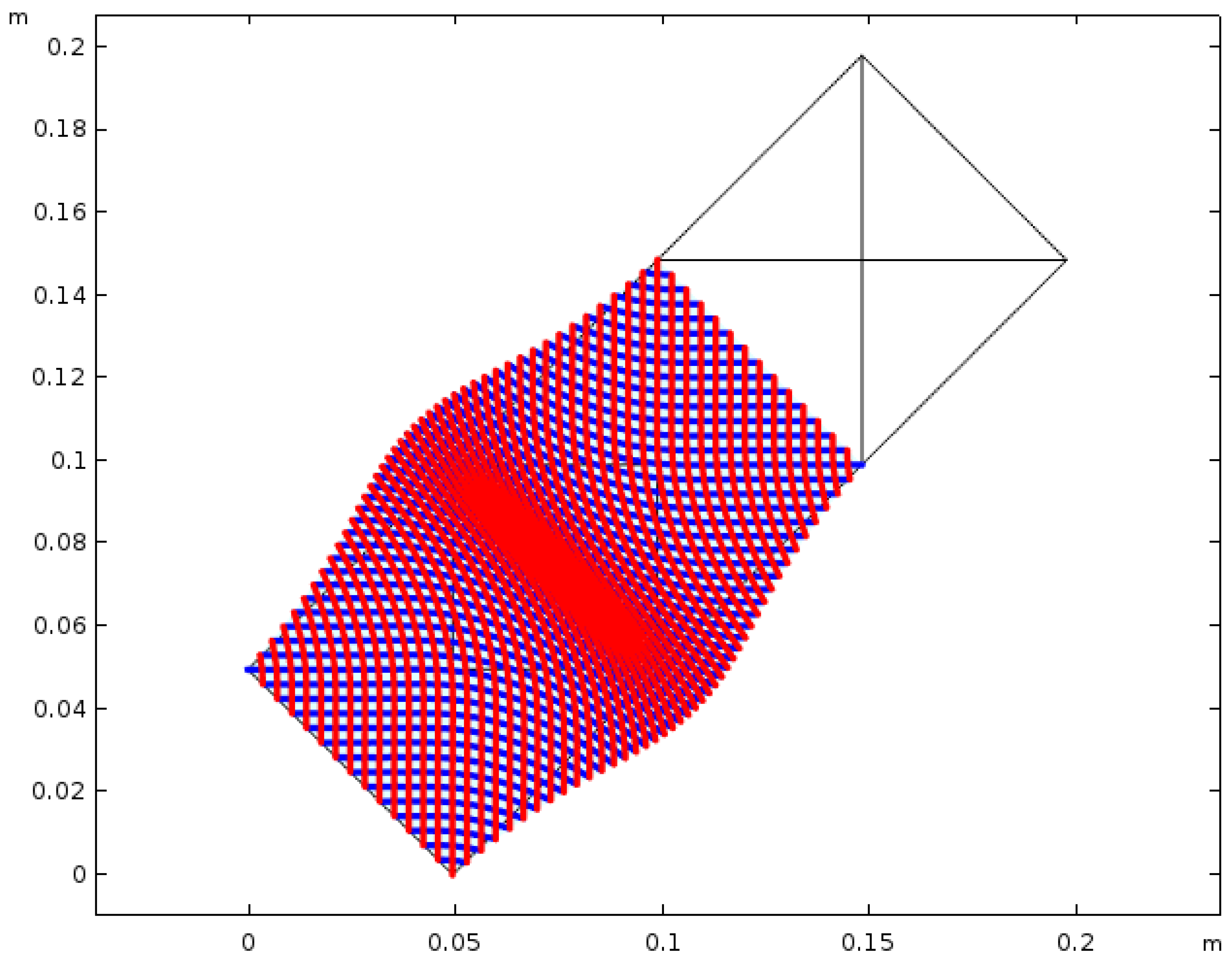
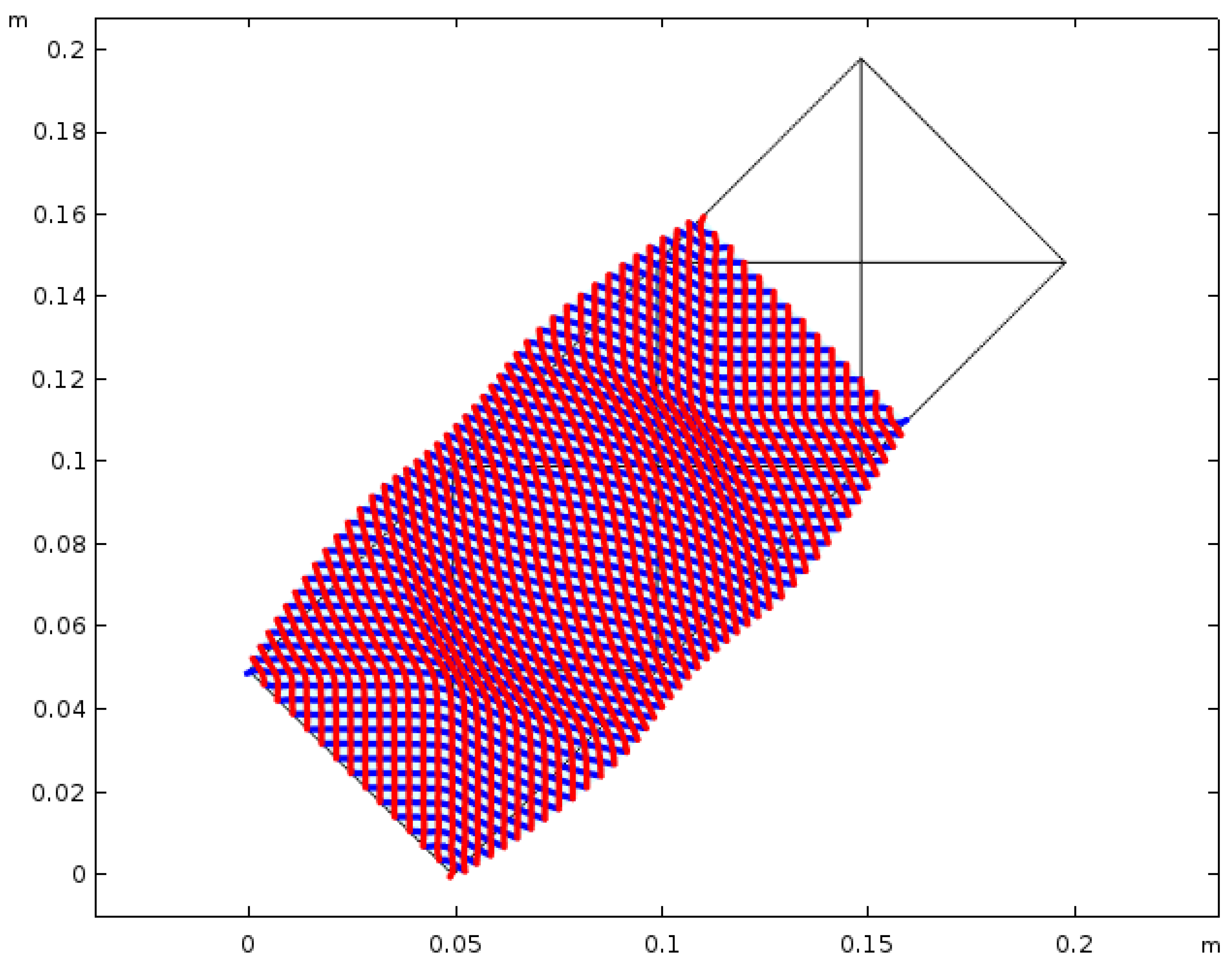
| Young Modulus | Size of Cross-Section | Cross-Section Area | Second Moment of Area |
|---|---|---|---|
| (Pa) | (m) | (m2) | (m4) |
| Specimen | 1 | 2 |
|---|---|---|
| (m) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tran, C.A.; Gołaszewski, M.; Barchiesi, E. Symmetric-in-Plane Compression of Polyamide Pantographic Fabrics—Modelling, Experiments and Numerical Exploration. Symmetry 2020, 12, 693. https://doi.org/10.3390/sym12050693
Tran CA, Gołaszewski M, Barchiesi E. Symmetric-in-Plane Compression of Polyamide Pantographic Fabrics—Modelling, Experiments and Numerical Exploration. Symmetry. 2020; 12(5):693. https://doi.org/10.3390/sym12050693
Chicago/Turabian StyleTran, Chuong Anthony, Maciej Gołaszewski, and Emilio Barchiesi. 2020. "Symmetric-in-Plane Compression of Polyamide Pantographic Fabrics—Modelling, Experiments and Numerical Exploration" Symmetry 12, no. 5: 693. https://doi.org/10.3390/sym12050693





