Analogies between Lattice QCD and the Truncated Nambu–Jona-Lasinio Model
Abstract
:1. Introduction
- (i)
- A solvable model is formulated which can support the validity of the popular Hartree–Fock approximation for massive constituent quarks together with the random phase approximation (RPA) for pions in the full Nambu–Jona-Lasinio model. It also supports the meaningfulness of the limit of large numbers of colours.
- (ii)
- Lattice models as well as few-body models with a finite Hilbert space do not provide a continuum description of the two-body decay channel. Instead, the diagonalization of the Hamiltonian yields a discrete spectrum which hides a lot of information about the relevant continuum which one is trying to extract. As an example, approximate methods for scattering at low energy, as well as via the meson resonance, are studied. It is shown how the discrete eigenvalue spectrum can provide some information on scattering using the first order Born approximation, in analogy to the Luscher formula used in Lattice QCD for the same dilemma of how to extract scattering from a discrete spectrum.
2. The Two-Level Quasispin Model
- (i)
- Periodic box of volume ;
- (ii)
- A sharp three-momentum cut-off ;
- (iii)
- An average kinetic energy for all momentum states ;
- (iv)
- Restriction to one flavour of quarks ;
- (v)
- Truncation of interaction.
- In the large N limit, the exact results of the quasispin model tend, in fact, to the Hartree–Fock and RPA values, which is a popular approximation for full NJL.
- The spectrum of the “ground state band” (Table 1) is almost equidistant and can be interpreted as multipion states. The energy deficit can be assumed to be due to an attractive average pion–pion interaction:
- This average potential is, in fact, proportional to the density of each pion, , which supports such an interpretation.
- The idea of an average pion–pion potential allows us to calculate the pion–pion scattering length a in the first order Born approximation ( equivalent to the so-called Lüscher formula which is frequently used in the literature [17,18,19]) , which is qualitatively consistent with the two-flavour experimental analysis of Lesniak, or [20].
- The parity of multipion states alternates. There are, however, intruders which do not follow the alternation. In Table 1, they are written in boldface and the lowest can be interpreted as the meson (now called a(500)). The sigma meson is not a six-pion state but an intruder at the position around six pions; it has an overlap with a decaying two-pion state. Also, the states around may be perturbed by admixtures of .
3. Some Lessons for Lattice-like Models
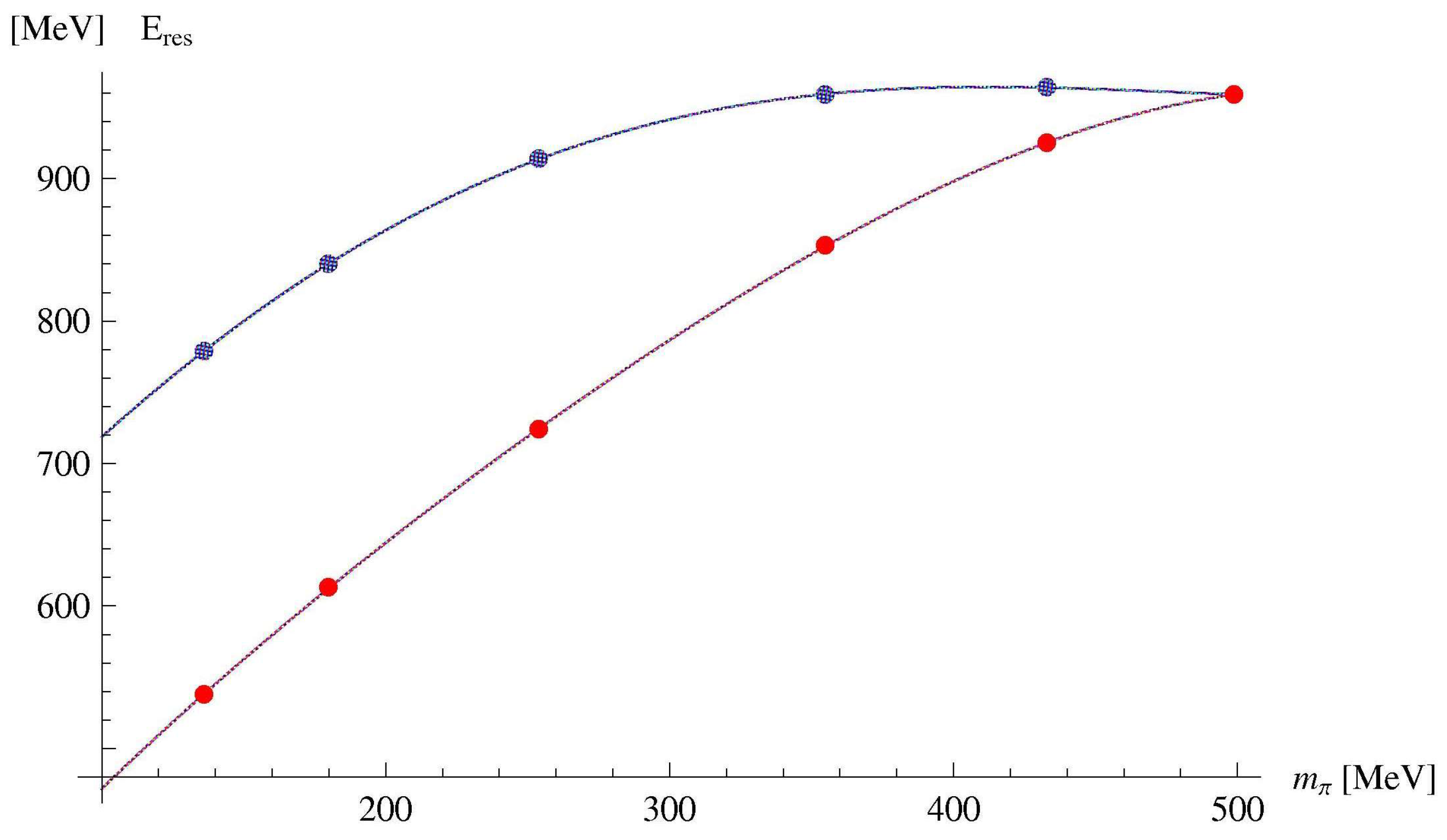
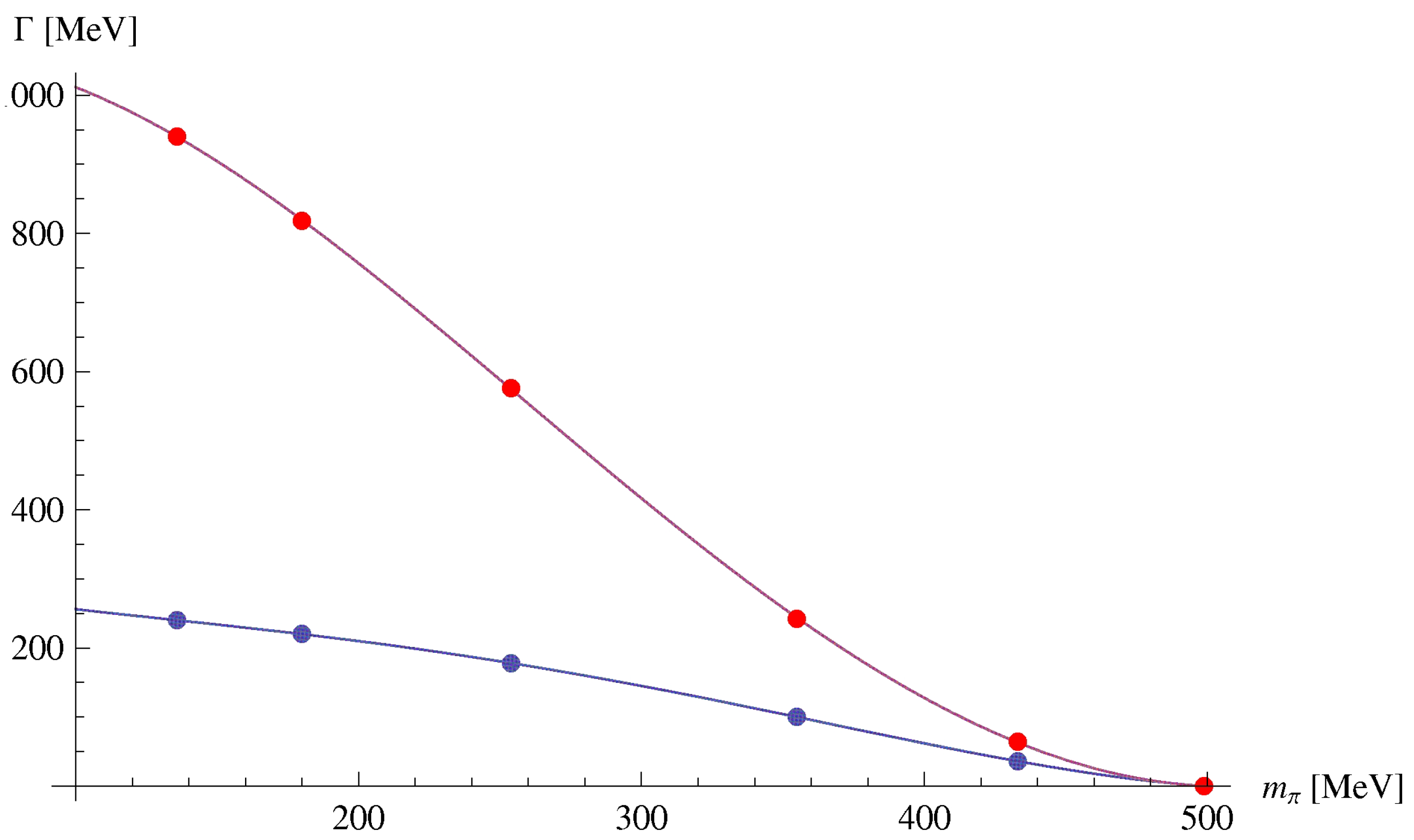
4. The Width of the Sigma Meson
5. Conclusions
Funding
Data Availability Statement
Conflicts of Interest
References
- Nambu, Y.; Jona-Lasinio, G. Dynamical Model of Elementary Particles Based on an Analogy with Superconductivity. I. Phys. Rev. 1961, 122, 345–358. [Google Scholar] [CrossRef]
- Nambu, Y.; Jona-Lasinio, G. Dynamical Model of Elementary Particles Based on an Analogy with Superconductivity. II. Phys. Rev. 1961, 124, 246–254. [Google Scholar] [CrossRef]
- da Providencia, J.; Ruivo, M.C.; de Sousa, C.A. Time-dependent Hartree-Fock formalism and the excitations of the Dirac sea in the Nambu-Jona-Lasinio model. Phys. Rev. D 1987, 36, 1882. [Google Scholar] [CrossRef] [PubMed]
- Bernard, V.; Blin, A.H.; Hiller, B.; Meissner, U.-G.; Ruivo, M.C. Strong and radiative meson decays in a generalized Nambu-Jona-Lasinio model. Phys. Lett. B 1993, 305, 163–167. [Google Scholar] [CrossRef]
- Mota, A.L.; Nemes, M.C.; Hiller, B.; Walliser, H. Meson properties in a renormalizable version of the NJL model. Nucl. Phys. 1999, A652, 73–87. [Google Scholar] [CrossRef]
- Osipov, A.A.; Hiller, B.; Blin, A.H.; da Providencia, J. Effects of eight-quark interactions on the hadronic vacuum and mass spectra of light mesons. Ann. Phys. 2007, 322, 2021–2054. [Google Scholar] [CrossRef]
- Hellstern, G.; Alkofer, R.; Reinhardt, H. Diquark confinement in an extended NJL model. Nucl. Phys. 1997, A625, 697–712. [Google Scholar] [CrossRef]
- Yuan, W.; Chao, J.; Li, A. Diquark and chiral condensates in a self-consistent NJL-type model. Phys. Rev. D 2023, 108, 043008. [Google Scholar] [CrossRef]
- Arbuzov, B.A.; Volkov, M.K.; Zaitsev, I.V. NJL model derived from QCD. Int. J. Mod. Phys. 2006, A21, 5721–5742. [Google Scholar] [CrossRef]
- Rosina, M.; Oblak, B.T. The two-level Nambu–Jona-Lasino model. Bled Work. Phys. 2006, 7, 92. [Google Scholar]
- Rosina, M.; Oblak, B.T. Sigma meson in a two-level Nambu–Jona-Lasino model. Bled Work. Phys. 2007, 8, 66. [Google Scholar]
- Rosina, M.; Oblak, B.T. Immitating continuum in lattice models. Bled Work. Phys. 2008, 9, 98. [Google Scholar]
- Rosina, M.; Oblak, B.T. Pion–Pion Interaction in a Soluble Two-Level Nambu–Jona-Lasinio Model. Few-Body Syst. 2010, 47, 117–123. [Google Scholar] [CrossRef]
- Rosina, M. Resonances in the Nambu–Jona-Lasinio model. Bled Work. Phys. 2015, 16, 91. [Google Scholar]
- Fiolhais, M.; da Providência, J.; Rosina, M.; Sousa, C.A. Soliton formation in σ models. Phys. Rev. C 1997, 56, 3311. [Google Scholar] [CrossRef]
- Buballa, M. NJL-model analysis of dense quark matter. Phys. Rep. 2005, 407, 205. [Google Scholar] [CrossRef]
- Lüscher, M. Volume dependence of the energy spectrum in massive quantum field theories. 1. stable particle states. Commun. Math. Phys. 1986, 104, 177. [Google Scholar] [CrossRef]
- Lüscher, M. Volume dependence of the energy spectrum in massive quantum field theories. 2. scattering states. Commun. Math. Phys. 1986, 105, 153. [Google Scholar] [CrossRef]
- Lüscher, M. Two particle states on a torus and their relation to the scattering matrix. Nucl. Phys. 1991, B354, 531. [Google Scholar] [CrossRef]
- Kaminski, R.; Lesniak, L.; Loiseau, B. Amplitudes Fitted to Experimental Data and to Roy’s Equations. Int. J. Mod. Phys. 2005, A20, 693. [Google Scholar] [CrossRef]
- Krasnopolsky, V.M.; Kukulin, V.I. Theory of resonance states based on analytical continuation in the coupling constant. Phys. Lett. 1978, 69A, 251. [Google Scholar] [CrossRef]
- Krasnopolsky, V.M.; Kukulin, V.I. A new method to describe the stripping to unbound states and other reactions with unstable particles. Phys. Lett. 1980, 96B, 4–6. [Google Scholar] [CrossRef]
- Tanaka, N.; Suzuki, Y.; Varga, K.; Lovas, R.G. Unbound states by analytic continuation in the coupling constant. Phys. Rev. 1999, C59, 1391. [Google Scholar] [CrossRef]

| n | Parity | E − E0 | E − E0 | ||||
|---|---|---|---|---|---|---|---|
| N= 144 | N= 144 | N= 144 | N= 192 | N= 192 | N= 192 | ||
| 8 | + | 771 | 4 | −11.3 | 861 | 59 | −8.3 |
| 7 | − | 767 | 121 | −8.8 | 802 | 93 | −7.3 |
| 6 | + | 646 | 66 | −11.4 | 709 | 98 | −7.3 |
| 6 | + | 634 | (−12.2) | 655 | (−10.9) | ||
| 5 | − | 580 | 98 | −10.0 | 611 | 108 | −7.2 |
| 4 | + | 482 | 114 | −10.5 | 503 | 115 | −7.1 |
| 3 | − | 378 | 117 | −10.1 | 388 | 122 | −7.1 |
| 2 | + | 261 | 125 | −10.3 | 266 | 129 | −7.1 |
| 1 | − | 136 | 136 | 137 | 137 | ||
| 0 | + | 0 | 0 |
| Pion Mass | 136 | 180 | 254 | 355 | 433 | 499 |
|---|---|---|---|---|---|---|
| (order 1) | 779 | 840 | 914 | 959 | 964 | 959 |
| (order 2) | 538 | 613 | 724 | 853 | 925 | 959 |
| (order 1) | 240 | 220 | 178 | 100 | 36 | 0 |
| (order 2) | 940 | 818 | 576 | 242 | 64 | 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rosina, M. Analogies between Lattice QCD and the Truncated Nambu–Jona-Lasinio Model. Symmetry 2024, 16, 607. https://doi.org/10.3390/sym16050607
Rosina M. Analogies between Lattice QCD and the Truncated Nambu–Jona-Lasinio Model. Symmetry. 2024; 16(5):607. https://doi.org/10.3390/sym16050607
Chicago/Turabian StyleRosina, Mitja. 2024. "Analogies between Lattice QCD and the Truncated Nambu–Jona-Lasinio Model" Symmetry 16, no. 5: 607. https://doi.org/10.3390/sym16050607





