1. Introduction
Let
be a finite alphabet and let
denote the set of words formed from the letters in
. Let
be a language, and let
be the equivalence relation defined as
if and only if
exactly when
. The Myhill–Nerode Theorem of computer science states that
L is accepted by a finite automaton if and only if
has finite index (cf. [
1, 1, Chapter III, §9, Proposition 9.2], [
2, §3.4, Theorem 3.9]). In [
3, Theorem 5.4] the authors generalize the Myhill–Nerode theorem to an algebraic setting in which a finiteness condition involving the action of a semigroup on a certain function plays the role of the finiteness of the index of
, while a bialgebra plays the role of the finite automaton which accepts the language. We call these bialgebras
Myhill–Nerode bialgebras.
The purpose of this paper is to investigate the quasitriangular structure of Myhill–Nerode bialgebras.
By construction, a Myhill–Nerode bialgebra B is cocommutative and finite dimensional over its base field. Thus B admits (at least) the trivial quasitriangular structure . We ask: does B (or its linear dual ) have any non-trivial quasitriangular structures?
Towards a solution to this problem, we construct a class of commutative Myhill–Nerode bialgebras and give a complete account of the quasitriangular structure of one of them. We begin with some background information regarding algebras, coalgebras, and bialgebras.
2. Algebras, Coalgebras and Bialgebras
Let
K be an arbitrary field of characteristic 0 and let
A be a vector space over
K with scalar product
for all
,
. Scalar product defines two maps
with
and
with
, for
,
. Let
denote the identity map. A
K-algebra is a triple
where
is a
K-linear map which satisfies
and
is a
K-linear map for which
for all
,
. The map
is the
multiplication map of
A and
is the
unit map of
A. Condition (1) is the
associative property and Condition (2) is the
unit property.
We write as . The element is the unique element of A for which for all . Let be algebras. An algebra homomorphism from A to B is a K-linear map such that In particular, for A to be a subalgebra of B we require .
For any two vector spaces
V,
W let
denote the
twist map defined as
, for
,
. For
K-algebras
, we have that
is a
K-algebra with multiplication
defined by
for
,
. The unit map
given as
for
.
Let
C be a
K-vector space. A
K-coalgebra is a triple
in which
is
K-linear and satisfies
and
is
K-linear with
for all
. The maps
and
are the
comultiplication and
counit maps, respectively, of the coalgebra
C. Condition (3) is the
coassociative property and Condition (4) is the
counit property.
We use the notation of M. Sweedler [
4, §1.2] to write
Note that Condition (4) implies that
Let
C be a
K-coalgebra. A nonzero element
c of
C for which
is a
grouplike element of
C. If
c is grouplike, then
and so,
. The grouplike elements of
C are linearly independent [
4, Proposition 3.2.1].
Let
be coalgebras. A
K-linear map
is a
coalgebra homomorphism if
and
for all
. The tensor product
of two coalgebras is again a coalgebra with comultiplication map
defined by
for
,
. The counit map
is defined as
for
,
.
A K-bialgebra is a K-vector space B together with maps , , , for which is a K-algebra and is a K-coalgebra and for which and are algebra homomorphisms. Let be bialgebras. A K-linear map is a bialgebra homomorphism if ϕ is both an algebra and coalgebra homomorphism.
A
K-Hopf algebra is a bialgebra
H together with an additional
K-linear map
that satisfies
for all
. The map
is the
coinverse (or
antipode) map and property Condition (6) is the
coinverse (or
antipode)
property. Though we will not consider Hopf algebras here, more details on the subject can be found in [
5,
6,
7,
8].
An important example of a
K-bialgebra is given as follows. Let
G be a semigroup with unity, 1. Let
denote the semigroup algebra. Then
is a bialgebra with comultiplication map
defined by
, for all
, and counit map
given by
, for all
. The bialgebra
is the
semigroup bialgebra on G.
Let
B be a bialgebra, and let
A be an algebra which is a left
B-module with action denoted by “·”. Suppose that
and
for all
,
. Then
A is a
left B-module algebra. A
K-linear map
is a
left B-module algebra homomorphism if
ϕ is both an algebra and a left
B-module homomorphism.
Let
C be a coalgebra and a right
B-module with action denoted by “·”. Suppose that for all
,
,
and
Then
C is a
right B-module coalgebra. A
K-linear map
is a
right B-module coalgebra homomorphism if
ϕ is both a coalgebra and a right
B-module homomorphism.
Let C be a coalgebra and let denote the linear dual of C. Then the coalgebra structure of C induces an algebra structure on .
Proposition 2.1 If C is a coalgebra, then is an algebra.
Proof. Recall that
C is a triple
where
is
K-linear and satisfies the coassociativity property, and
is
K-linear and satisfies the counit property. The dual map of
is a
K-linear map
Since
, we define the multiplication map of
, denoted as
, to be the restriction of
to
. For
,
,
The coassociatively property of
yields the associative property of
. Indeed, for
,
,
In addition, the counit map of
C dualizes to yield
defined as
. Thus we define the unit map
to be
. One can show that the counit property of
implies the unit property for
. To this end, for
,
,
,
In a similar manner, one obtains
Thus
is an algebra. Note that
, and so,
is the unique element of
for which
for all
. ⋄
Let be a K-algebra. Then one may wonder if is a K-coalgebra. The multiplication map dualizes to yield . Unfortunately, if A is infinite dimensional over K, then is a proper subset of , and hence may not induce the required comultiplication map .
There is still however a
K-coalgebra arising via duality from the algebra
A. An ideal
I of
A is
cofinite if
. The
finite dual of
A is defined as
Note that
is the largest subspace
W of
for which
.
Proposition 2.2 If A is an algebra, then is a coalgebra.
Proof. The proof is similar to the method used in Proposition 2.1. We restrict the map
to
to yield the
K-linear map
. Now by [
4, Proposition 6.0.3],
. Let
denote the restriction of
to
. We show that
satisfies the coassociative condition. For
,
, we have
For the counit map of
, we consider the dual map
. Now
restricts to a map
. We let
denote the restriction of
to
. For
,
,
and so,
. We show that
satisfies the counit property. First let
be defined by the scalar multiplication of
. For
,
,
,
In a similar manner, one obtains
where
is given by scalar multiplication. Thus
is a coalgebra. ⋄
Proposition 2.3 If B is a bialgebra, then is a bialgebra.
Proof. As a coalgebra, B is a triple . By Proposition 2.1, is an algebra with maps and . Let denote the restriction of to , and let denote the restriction of to . Then the triple is a K-algebra.
As an algebra,
B is a triple
. By Proposition 2.2,
is a coalgebra with maps
and
. It remains to show that
and
are algebra homomorphisms. First observe that for
,
one has
and
We have
and so
is an algebra map. We next show that
is an algebra map. For
,
Thus
and so,
is an algebra map. ⋄
Proposition 2.4 Suppose that B is a bialgebra that is finite dimensional over K. Then is a bialgebra.
Proof. If , then . The result then follows from Proposition 2.3. ⋄
Let be a finite semigroup with unity element , and let denote the semigroup bialgebra. By Proposition 2.4 is a bialgebra of dimension n over K. Let be the dual basis for defined as .
Proposition 2.5 The comultiplication map is given as and the counit map is defined as . Proof, See [
7, (1.3.7)]. ⋄
Let
B be a
K-bialgebra. Then
B is
cocommutative if
for all
.
Proposition 2.6 If B is cocommutative, then is a commutative algebra. If B is a commutative algebra, then is cocommutative.
Proof. See [
7, Lemma 1.2.2, Proposition 1.2.4]. ⋄
3. Quasitriangular Bialgebras
Let
B be a bialgebra and let
be the tensor product algebra. Let
denote the group of units in
and let
. The pair
is
almost cocommutative if the element
R satisfies
for all
.
If the bialgebra B is cocommutative, then the pair is almost cocommutative since satisfies Condition (7). However, if B is commutative and non-cocommutative, then cannot be almost cocommutative for any since Condition (7) in this case reduces to the condition for cocommutativity.
Write
. Let
The pair
is
quasitriangular if
is almost cocommutative and the following conditions hold
Clearly, if B is cocommutative then is quasitriangular.
Let B be a bialgebra. A quasitriangular structure is an element so that is quasitriangular. Let and be quasitriangular bialgebras. Then , are isomorphic as quasitriangular bialgebras if there exists a bialgebra isomorphism for which . Two quasitriangular structures on a bialgebra B are equivalent quasitriangular structures if as quasitriangular bialgebras.
The following proposition shows that every bialgebra isomorphism with B quasitriangular extends to an isomorphism of quasitriangular bialgebras.
Proposition 3.1 Suppose is quasitriangular and suppose that is an isomorphism of K-bialgebras. Let . Then is quasitriangular.
Proof. Note that
. Let
. Then there exists
for which
. Now
and so,
is almost cocommutative. Moreover,
In a similar manner one shows that
Thus
is quasitriangular. ⋄
Quasitriangular bialgebras are important since they give rise to solutions of the equation
which is known as the
quantum Yang–Baxter equation (QYBE). The QYBE was first introduced in statistical mechanics, see [
9]. An element
which satisfies (10) is a
solution to the QYBE.
Certainly, the QYBE admits the trivial solution
, and of course, if
B is commutative, then any
is a solution to the QYBE. For
B non-commutative, it is of great interest to find non-trivial solutions
to the QYBE. We have the following result due to V. G. Drinfeld [
10].
Proposition 3.2 (Drinfeld) Suppose is quasitriangular. Then R is a solution to the QYBE.
The following proposition provides necessary conditions on in order for to be quasitriangular.
Proposition 3.3 Suppose is quasitriangular. Then
(i) ,
(ii) .
Proof. For (i) one has
In view of Condition (8)
Thus
and consequently,
A similar argument is used to prove (ii). ⋄
4. Myhill–Nerode Bialgebras
In this section we review the main result of [
3] in which the authors give a bialgebra version of the Myhill–Nerode Therorem. Let
G be a semigroup with unity, 1 and let
be the semigroup bialgebra. There is a right
H-module structure on
defined as
for all
,
. For
,
, the element
is the
right translate of p by x.
Proposition 4.1 ([3, Proposition 5.4].) Let G be a semigroup with 1, let denote the semigroup bialgebra. Let . Then the following are equivalent. (i) The set of right translates is finite.
(ii) There exists a finite dimensional bialgebra B, a bialgebra homomorphism , and an element so that for all .
(Note: The bialgebras of (ii) are defined to be Myhill–Nerode bialgebras.)
Proof. . Let
be the finite set of right translates. For each
, we define a right operator
by the rule
Observe that the set
is finite with
. The set
is a semigroup with unity,
under composition of operators. Indeed,
Thus
, for all
. Let
B denote the semigroup bialgebra on
. Let
be the
K-linear map defined by
. Then
and
and so, Ψ is a homomorphism of bialgebras.
Let
be defined by
Then
, for all
, as required.
. Suppose there exists a finite dimensional bialgebra
B, a bialgebra homomorphism
, and an element
so that
for all
. Define a right
H-module action · on
B as
for all
,
. Then for
,
,
and
Thus
B is a right
H-module coalgebra.
Now, let
Q be the collection of grouplike elements of
B. Since
Q is a linearly independent subset of
B and
B is finite dimensional,
Q is finite. Since
B is a right
H-module coalgebra with action “·”,
for
,
. Thus · restricts to give an action (also denoted by “·”) of
G on
Q. Now for
,
Let
In view of Condition (11) there exists a function
defined as
Since
ϱ is surjective and
S is finite,
is finite. ⋄
We illustrate the connection between Proposition 4.1 and the usual Myhill–Nerode Theorem. Let denote the set of words in a finite alphabet . Let be a language. Suppose that the equivalence relation (as in the Introduction) has finite index. Then the usual Myhill–Nerode Theorem says that there exists a finite automaton which accepts L. We show how to construct this finite automaton using Proposition 4.1.
Consider
as a semigroup with unity where the semigroup operation is concatenation and the unity element is the empty word. Let
denote the semigroup bialgebra. Then the characteristic function of
L extends to an element
. Since
has finite index, the set of right translates
is finite [
3, Proposition 2.3]. Now Proposition 4.1 (i)⟹ (ii) applies to show that there exists a finite dimensional bialgebra
B, a bialgebra homomorphism
and an element
so that
, for all
.
This bialgebra determines a finite automaton
, where
Q is the finite set of states, Σ is the input alphabet,
δ is the transition function,
is the initial state, and
F is the set of final states (see [
2, Chapter 2] for details on finite automata.)
For the states of the automata, we let
Q be the (finite) set of grouplike elements of
B. For the input alphabet, we choose
. As we have seen, the right
H-module structure of
B restricts to an action “·” of
G on
Q, and so we define the transition function
by the rule
, for
,
. The initial state is
, and the set of final states
F is the subset of
Q of the form
,
for which
By construction, the finite automaton
accepts
L.
5. Quasitriangular Structure of Myhill–Nerode Bialgebras
In this section we use Proposition 4.1 to construct a collection of Myhill–Nerode bialgebras. We then compute the quasitriangular structure of one of these bialgebras.
Let
be the alphabet on a single letter
a. Let
denote the collection of all words of finite length formed from
. Here 1 denotes the empty word of length 0. For convenience, we shall write
for
.
Fix an integer
and let
. Then the language
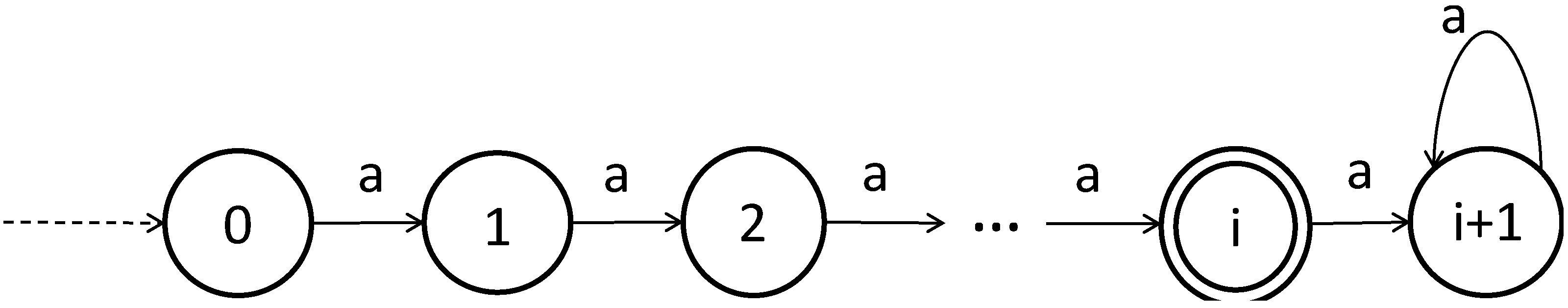
is accepted by the finite automaton given in
Figure 1.
Figure 1.
Finite automaton accepting , accepting state is i.
Figure 1.
Finite automaton accepting , accepting state is i.
By the usual Myhill–Nerode Theorem, the equivalence relation , defined as if and only if exactly when , has finite index. If is the characteristic function of , then is equivalent to the relation defined as: if and only if . Let denote the equivalence class of x under . The Myhill–Nerode theorem now says that the set is finite.
Now we consider
as a semigroup with unity 1 with concatenation as the binary operation. Let
be the semigroup bialgebra. The characteristic function
of
extends to an element of
. By [
3, Proposition 2.3], the set of right translates
is finite. Thus by Proposition 4.1, there exists a finite dimensional bialgebra
, a bialgebra homomorphism
, and an element
so that
for all
.
In what follows, we give the bialgebra structure of the collection and compute the quasitriangular structure of the bialgebra .
For
, the finite set of right translates of
is
One finds that the set of right operators on
is
. Under composition, the set of right operators is a semigroup with unity
. We have, for
,
By construction, is the semigroup bialgebra on .
5.1. Quasitriangular Structure of
In the case
,
is the semigroup bialgebra on
with algebra structure defined by
,
,
,
. Let
be the dual basis defined as
,
,
,
. Then
is the set of minimal idempotents for
. Comultiplication on
is given as
and the counit map is defined by
Proposition 5.1 Let be the K-bialgebra as above. Then there is exactly one quasitriangular structure on , namely, .
Proof. Certainly,
is a quasitriangular structure for
. We claim that
is the only quasitriangular structure. Observe that there is bialgebra isomorphism
defined as
,
. Thus if
is quasitriangular, then
,
, is quasitriangular by Proposition 3.1. So, we first compute all of the quasitriangular structures of
. To this end, suppose that
is quasitriangular for some element
. Since
for
. By Proposition 3.3(i),
and so,
. From Proposition 3.3(ii), one also has
. Thus
for
. Now,
Moreover,
Equations 12 and 13 yield the relation
. Thus either
or
. If
, then
is not a unit in
. Thus
is the only quasitriangular structure for
.
Consequently, if is quasitriangular, then . It follows that . ⋄
5.2. Questions for Future Research
Though the Myhill–Nerode bialgebra has only the trivial quasitriangular structure, it remains to compute the quasitriangular structure of for . Moreover, the linear dual is a commutative, cocommutative K-bialgebra and it would be of interest to find its quasitriangular structure. Unlike the case, we may have (for instance, ) and so this is indeed a separate problem.
Suppose that
L is a language of words built from the alphabet
. If
L is accepted by a finite automaton, then by Proposition 4.1,
L gives rise to a Myhill–Nerode bialgebra
B (see for example, [
3, §6].) By construction,
B is a cocommutative
K-bialgebra and hence
B has at least the trivial quasitriangular structure. Are there any other structures? Note that
is a commutative
K-algebra. For which
R (if any) is
quasitriangular?