Combining Faraday Tomography and Wavelet Analysis
Abstract
:1. Introduction
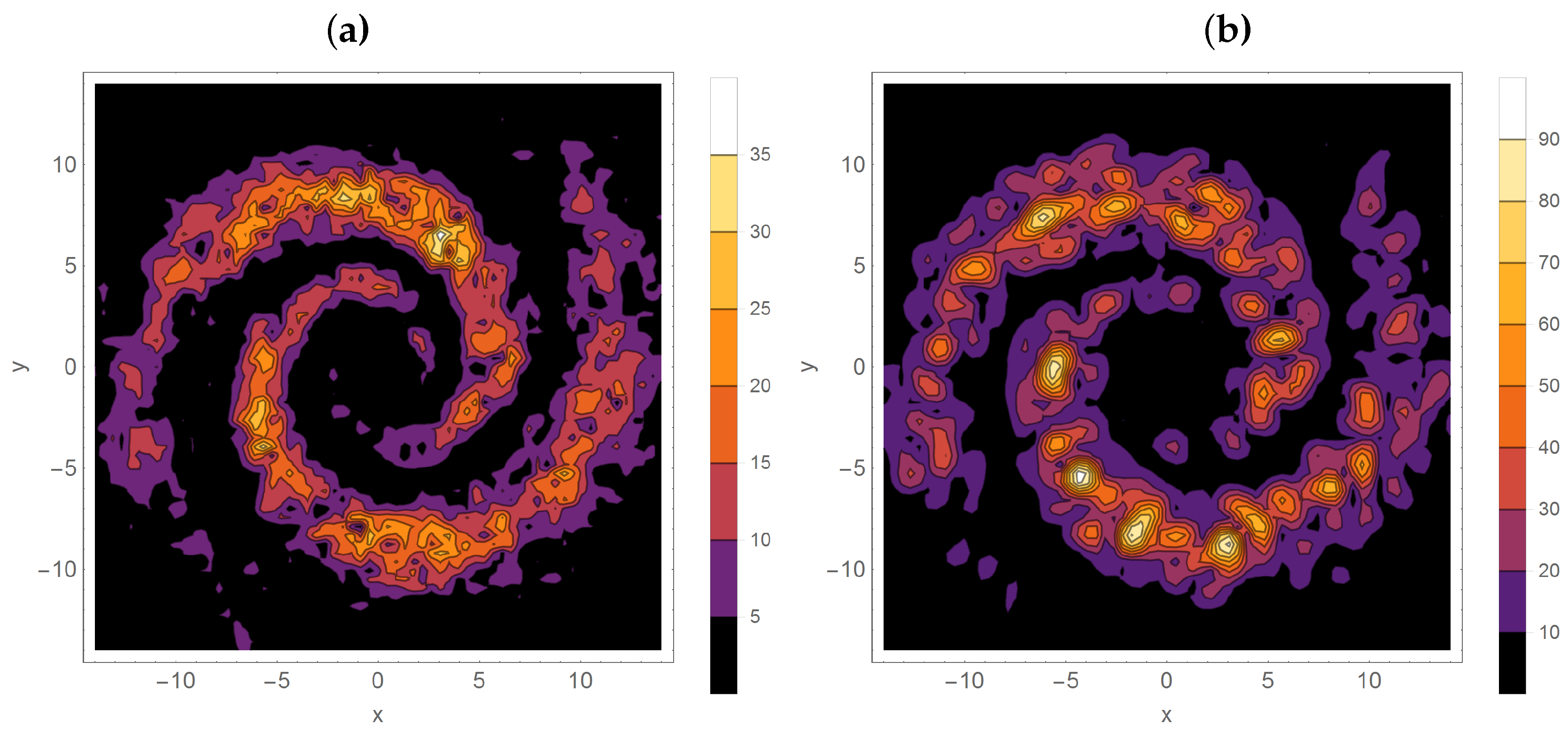
2. Idea of the Method Applied to the NGC 6946 Data
3. Synthetic Data Analysis
4. Discussion
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| NGC | New General Catalogue |
| SKA | Square Kilometre Array |
| RM | Rotation measure |
References
- Beck, R.; Frick, P.; Stepanov, R.; Sokoloff, D. Recognizing magnetic structures by present and future radio telescopes with Faraday rotation measure synthesis. Astron. Astrophys. 2012, 543, A113. [Google Scholar] [CrossRef] [Green Version]
- Weżgowiec, M.; Ehle, M.; Beck, R. Hot gas and magnetic arms of NGC 6946: Indications for reconnection heating? Astron. Astrophys. 2016, 585, A3. [Google Scholar] [CrossRef]
- Beck, R. Magnetic fields in spiral galaxies. Astron. Astrophys. Rev. 2015, 24, 4. [Google Scholar] [CrossRef] [Green Version]
- Chamandy, L.; Shukurov, A.; Subramanian, K. Magnetic spiral arms and galactic outflows. Mon. Not. R. Astron. Soc. 2015, 446, L6–L10. [Google Scholar] [CrossRef]
- Burn, B.J. On the depolarization of discrete radio sources by Faraday dispersion. Mon. Not. R. Astron. Soc. 1966, 133, 67. [Google Scholar] [CrossRef]
- Brentjens, M.A.; de Bruyn, A.G. Faraday rotation measure synthesis. Astron. Astrophys. 2005, 441, 1217–1228. [Google Scholar] [CrossRef] [Green Version]
- Sun, X.H.; Rudnick, L.; Akahori, T.; Anderson, C.S.; Bell, M.R.; Bray, J.D.; Farnes, J.S.; Ideguchi, S.; Kumazaki, K.; O’Brien, T.; et al. Comparison of Algorithms for Determination of Rotation Measure and Faraday Structure. I. 1100–1400 MHz. Astron. J. 2015, 149, 60. [Google Scholar] [CrossRef]
- Heald, G.; Braun, R.; Edmonds, R. The Westerbork SINGS survey. II Polarization, Faraday rotation, and magnetic fields. Astron. Astrophys. 2009, 503, 409–435. [Google Scholar] [CrossRef]
- Chupin, A.; Beck, R.; Frick, P.; Heald, G.; Sokoloff, D.; Stepanov, R. Magnetic arms of NGC6946 traced in the Faraday cubes at low radio frequencies. Astron. Nachr. 2018, 339, 440–446. [Google Scholar] [CrossRef]
- Schwinn, J.; Baugh, C.M.; Jauzac, M.; Bartelmann, M.; Eckert, D. Uncovering substructure with wavelets:proof of concept using Abell 2744. Mon. Not. R. Astron. Soc. 2018, 481, 4300–4310. [Google Scholar] [CrossRef]
- Frick, P.; Sokoloff, D.; Stepanov, R.; Beck, R. Wavelet-based Faraday rotation measure synthesis. Mon. Not. R. Astron. Soc. 2010, 401, L24–L28. [Google Scholar] [CrossRef] [Green Version]
- Frick, P.; Sokoloff, D.; Stepanov, R.; Beck, R. Faraday rotation measure synthesis for magnetic fields of galaxies. Mon. Not. R. Astron. Soc. 2011, 414, 2540–2549. [Google Scholar] [CrossRef] [Green Version]
- Stepanov, R.; Arshakian, T.G.; Beck, R.; Frick, P.; Krause, M. Magnetic field structures of galaxies derived from analysis of Faraday rotation measures, and perspectives for the SKA. Astron. Astrophys. 2008, 480, 45–59. [Google Scholar] [CrossRef] [Green Version]
- Stepanov, R.; Shukurov, A.; Fletcher, A.; Beck, R.; La Porta, L.; Tabatabaei, F. An observational test for correlations between cosmic rays and magnetic fields. Mon. Not. R. Astron. Soc. 2014, 437, 2201–2216. [Google Scholar] [CrossRef]
- Ferrière, K. Faraday tomography: A new, three-dimensional probe of the interstellar magnetic field. J. Phys. Conf. Ser. 2016, 767, 012006. [Google Scholar] [CrossRef]
- Lazarian, A.; Yuen, K.H. Gradients of Synchrotron Polarization: Tracing 3D Distribution of Magnetic Fields. Astrophys. J. 2018, 865, 59. [Google Scholar] [CrossRef]
- Frick, P.; Stepanov, R.; Beck, R.; Sokoloff, D.; Shukurov, A.; Ehle, M.; Lundgren, A. Magnetic and gaseous spiral arms in M83. Astron. Astrophys. 2016, 585, A21. [Google Scholar] [CrossRef]
- Ossenkopf-Okada, V.; Stepanov, R. Measuring the filamentary structure of interstellar clouds through wavelets. arXiv, 2018; arXiv:1811.02082. [Google Scholar]
- Van Eck, C.L.; Haverkorn, M.; Alves, M.I.R.; Beck, R.; de Bruyn, A.G.; Enßlin, T.; Farnes, J.S.; Ferrière, K.; Heald, G.; Horellou, C.; et al. Faraday tomography of the local interstellar medium with LOFAR: Galactic foregrounds towards IC 342. Astron. Astrophys. 2017, 597, A98. [Google Scholar] [CrossRef] [Green Version]





© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sokoloff, D.; Beck, R.; Chupin, A.; Frick, P.; Heald, G.; Stepanov, R. Combining Faraday Tomography and Wavelet Analysis. Galaxies 2018, 6, 121. https://doi.org/10.3390/galaxies6040121
Sokoloff D, Beck R, Chupin A, Frick P, Heald G, Stepanov R. Combining Faraday Tomography and Wavelet Analysis. Galaxies. 2018; 6(4):121. https://doi.org/10.3390/galaxies6040121
Chicago/Turabian StyleSokoloff, Dmitry, Rainer Beck, Anton Chupin, Peter Frick, George Heald, and Rodion Stepanov. 2018. "Combining Faraday Tomography and Wavelet Analysis" Galaxies 6, no. 4: 121. https://doi.org/10.3390/galaxies6040121
APA StyleSokoloff, D., Beck, R., Chupin, A., Frick, P., Heald, G., & Stepanov, R. (2018). Combining Faraday Tomography and Wavelet Analysis. Galaxies, 6(4), 121. https://doi.org/10.3390/galaxies6040121





