Very High Cycle Fatigue of Welds: A Review
Abstract
:1. Introduction
2. Ultrasonic Fatigue Testing and Very High Cycle Fatigue of Metals
2.1. The Ultrasonic Fatigue Testing Method
2.2. Fatigue Behaviour of Metals at Very High Cycles
3. Very High Cycle Fatigue Performance of Welds
3.1. Low-Carbon Steels
3.2. Alloy Steels
3.3. Stainless Steels
3.4. Non-Ferrous Alloys
4. Design Standards and Industrial Case Studies
4.1. Design Standards for Very High Cycle Fatigue of Welds
4.2. Industrial Case Studies
5. Future Directions
6. Conclusions
- The VHCF strength of welded joints is generally lower than that of base metals, even when the stress concentration of the weld toe is removed by machining.
- A continuous decrease in the S–N curve is observed beyond 107 loading cycles for all metallic welds studied to date (steel, aluminium, titanium, and magnesium alloys), challenging the classical assumption of a fatigue limit. This is further demonstrated by examples of in-service failures of welded components in the VHCF regime in various applications.
- A change in the design S–N curves of standards that include a fatigue limit, e.g., BS7608:2014, should be implemented to guarantee the structural integrity of welded components operating in the VHCF regime.
- The primary factor for the reduction in VHCF strength of welds is welding defects, principally inclusions and gas pores. Failures in the VHCF domain typically originate from these defects.
- Limited research on UPT has shown the process to significantly increase the VHCF strength of welded joints due to the grain refinement and compressive residual stresses caused by the process.
- Testing programmes that cover the HCF and VHCF regimes tend to show that there is a reduction in the slope of the S–N curve in the VHCF regime, an observation that has been reflected in some design standards.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Campbell, F.C. Fatigue and Fracture—Understanding the Basics; ASM International: Materials Park, OH, USA, 2012; ISBN 978-1-61503-976-0. [Google Scholar]
- Masoudi Nejad, R.; Shariati, M.; Farhangdoost, K. Prediction of Fatigue Crack Propagation and Fractography of Rail Steel. Theor. Appl. Fract. Mech. 2019, 101, 320–331. [Google Scholar] [CrossRef]
- Moore, P.; Booth, G. The Welding Engineer’s Guide to Fracture and Fatigue; Elsevier: Amsterdam, The Netherlands, 2015; ISBN 9781782423706. [Google Scholar]
- Tsutsumi, S.; Fincato, R.; Luo, P.; Sano, M.; Umeda, T.; Kinoshita, T.; Tagawa, T. Effects of Weld Geometry and HAZ Property on Low-Cycle Fatigue Behavior of Welded Joint. Int. J. Fatigue 2022, 156, 106683. [Google Scholar] [CrossRef]
- Ma, M.; Lai, R.; Qin, J.; Wang, B.; Liu, H.; Yi, D. Effect of Weld Reinforcement on Tensile and Fatigue Properties of 5083 Aluminum Metal Inert Gas (MIG) Welded Joint: Experiments and Numerical Simulations. Int. J. Fatigue 2021, 144, 106046. [Google Scholar] [CrossRef]
- Zhan, R.; Wang, D.; Ren, Z.; Deng, C.; Xu, X.; Liang, H. Evolution of Welding Residual Stresses Involving the Cutting Process and Its Effect on Fatigue Performance. Int. J. Press. Vessel. Pip. 2022, 197, 104636. [Google Scholar] [CrossRef]
- Zerbst, U.; Ainsworth, R.A.; Beier, H.T.; Pisarski, H.; Zhang, Z.L.; Nikbin, K.; Nitschke-Pagel, T.; Münstermann, S.; Kucharczyk, P.; Klingbeil, D. Review on Fracture and Crack Propagation in Weldments—A Fracture Mechanics Perspective. Eng. Fract. Mech. 2014, 132, 200–276. [Google Scholar] [CrossRef]
- Sharma, A.; Oh, M.C.; Ahn, B. Recent Advances in Very High Cycle Fatigue Behavior of Metals and Alloys—A Review. Metals 2020, 10, 1200. [Google Scholar] [CrossRef]
- Pineau, A. Low-Cycle Fatigue. In Fatigue of Materials and Structures—Fundamentals; John Wiley & Sons: Hoboken, NJ, USA, 2010; pp. 113–177. ISBN 9781118623435. [Google Scholar]
- Bathias, C.; Drouillac, L.; Le François, P. How and Why the Fatigue S-N Curve Does Not Approach a Horizontal Asymptote. Int. J. Fatigue 2001, 23, 143–151. [Google Scholar] [CrossRef]
- Bathias, C. There Is No Infinite Fatigue Life in Metallic Materials. Fatigue Fract. Eng. Mater. Struct. 1999, 22, 559–565. [Google Scholar] [CrossRef]
- He, C.; Liu, Y.; Fang, D.; Wang, Q. Very High Cycle Fatigue Behavior of Bridge Steel Welded Joint. Theor. Appl. Mech. Lett. 2012, 2, 31010. [Google Scholar] [CrossRef]
- Yeom, H.; Choi, B.; Seol, T.; Lee, M.; Jeon, Y. Very High Cycle Fatigue of Butt-Welded High-Strength Steel Plate. Metals 2017, 7, 103. [Google Scholar] [CrossRef]
- Fry, P.R. High Cycle Fatigue of Welded Structures: Design Guidelines Validated by Case Studies. Eng. Fail. Anal. 2014, 46, 179–187. [Google Scholar] [CrossRef]
- Yin, D.; Wang, D.; Jing, H.; Huo, L. The Effects of Ultrasonic Peening Treatment on the Ultra-Long Life Fatigue Behavior of Welded Joints. Mater. Des. 2010, 31, 3299–3307. [Google Scholar] [CrossRef]
- Mayer, H. Recent Developments in Ultrasonic Fatigue. Fatigue Fract. Eng. Mater. Struct. 2016, 39, 3–29. [Google Scholar] [CrossRef]
- Kuhn, H.; Medlin, D. Ultrasonic Fatigue Testing. In Mechanical Testing and Evaluation; ASM International: Materials Park, OH, USA, 2000; Volume 8, pp. 717–729. ISBN 978-1-62708-176-4. [Google Scholar]
- Trško, L.; Nový, F.; Bokůvka, O.; Jambor, M. Ultrasonic Fatigue Testing in the Tension-Compression Mode. J. Vis. Exp. 2018, 2018, 57007. [Google Scholar] [CrossRef]
- Wan, J.; Zhao, J.; Zhou, L.; Zhang, S.; Zhao, H. A Novel Methodology for Bending Ultrasonic Fatigue Testing in the VHCF Regime. Int. J. Fatigue 2023, 170, 107562. [Google Scholar] [CrossRef]
- Marines-Garcia, I.; Doucet, J.P.; Bathias, C. Development of a New Device to Perform Torsional Ultrasonic Fatigue Testing. Int. J. Fatigue 2007, 29, 2094–2101. [Google Scholar] [CrossRef]
- da Costa, P.R.; Soares, H.; Reis, L.; Freitas, M. Ultrasonic Fatigue Testing under Multiaxial Loading on a Railway Steel. Int. J. Fatigue 2020, 136, 105581. [Google Scholar] [CrossRef]
- Shimadzu Corporation. USF-2000A Hardware Instruction Manual. Shimadzu Corporation, 2017. Available online: https://www.shimadzu.com/an/sites/shimadzu.com.an/files/pim/pim_document_file/manuals/13951/349-04408.pdf (accessed on 20 September 2023).
- Feng, N.; Wang, X.; Guo, J.; Li, Q.; Yu, J.; Zhang, X. Design Theory and Experimental Research of Ultrasonic Fatigue Test. Machines 2022, 10, 635. [Google Scholar] [CrossRef]
- The Japan Welding Engineering Society. Method for Ultrasonic Fatigue Testing in Metallic Material; WES 1112: Tokyo, Japan, 2022. [Google Scholar]
- Furuya, Y.; Shimamura, Y.; Takanashi, M.; Ogawa, T. Standardization of an Ultrasonic Fatigue Testing Method in Japan. Fatigue Fract. Eng. Mater. Struct. 2022, 45, 2415–2420. [Google Scholar] [CrossRef]
- Bach, J.; Göken, M.; Höppel, H.-W. Fatigue of Low Alloyed Carbon Steels in the HCF/VHCF-Regimes. In Fatigue of Materials at Very High Numbers of Loading Cycles; Chris, H.J., Ed.; Springer Spektrum: Berlin/Heidelberg, Germany, 2018; pp. 1–23. ISBN 978-3-658-24531-3. [Google Scholar]
- Hong, Y.; Hu, Y.; Zhao, A. Effects of Loading Frequency on Fatigue Behavior of Metallic Materials—A Literature Review. Fatigue Fract. Eng. Mater. Struct. 2023, 46, 3077–3098. [Google Scholar] [CrossRef]
- Guennec, B.; Ueno, A.; Sakai, T.; Takanashi, M.; Itabashi, Y.; Ota, M. Dislocation-Based Interpretation on the Effect of the Loading Frequency on the Fatigue Properties of JIS S15C Low Carbon Steel. Int. J. Fatigue 2015, 70, 328–341. [Google Scholar] [CrossRef]
- Milne, L.; Gorash, Y.; Comlekci, T.; MacKenzie, D. Frequency Effects in Ultrasonic Fatigue Testing (UFT) of Q355B Structural Steel. Procedia Struct. Integr. 2022, 42, 623–630. [Google Scholar] [CrossRef]
- Gorash, Y.; Comlekci, T.; Styger, G.; Kelly, J.; Brownlie, F.; Milne, L. Ultrasonic Fatigue Testing of Structural Steel S275JR+AR with Insights into Corrosion, Mean Stress and Frequency Effects. Materials 2023, 16, 1799. [Google Scholar] [CrossRef]
- Guennec, B.; Kinoshita, T.; Horikawa, N.; Oguma, N.; Sakai, T. Loading Frequency Effect on the Fatigue Endurance of Structural Carbon Steels: Estimation Based on Dislocation Motion Theory and Experimental Verification of the Model. Int. J. Fatigue 2023, 172, 107634. [Google Scholar] [CrossRef]
- Zhao, A.; Xie, J.; Sun, C.; Lei, Z.; Hong, Y. Effects of Strength Level and Loading Frequency on Very-High-Cycle Fatigue Behavior for a Bearing Steel. Int. J. Fatigue 2012, 38, 46–56. [Google Scholar] [CrossRef]
- Mughrabi, H. Specific Features and Mechanisms of Fatigue in the Ultrahigh-Cycle Regime. Int. J. Fatigue 2006, 28, 1501–1508. [Google Scholar] [CrossRef]
- Pyttel, B.; Schwerdt, D.; Berger, C. Very High Cycle Fatigue—Is There a Fatigue Limit? Int. J. Fatigue 2011, 33, 49–58. [Google Scholar] [CrossRef]
- Lee, J.; Park, S.Y.; Choi, B.H. Evaluation of Fatigue Characteristics of Aluminum Alloys and Mechanical Components Using Extreme Value Statistics and C-Specimens. Metals 2021, 11, 1915. [Google Scholar] [CrossRef]
- Murakami, Y.; Nomoto, T.; Ueda, T. Factors Influencing the Mechanism of Superlong Fatigue Failure in Steels. Fatigue Fract. Eng. Mater. Struct. 1999, 22, 581–590. [Google Scholar] [CrossRef]
- Sakai, T.; Sato, Y.; Oguma, N. Characteristics S-N Properties of High-Carbon-Chromium-Bearing Steel under Axial Loading in Long-Life Fatigue. Fatigue Fract. Eng. Mater. Struct. 2002, 25, 765–773. [Google Scholar] [CrossRef]
- De Li, Y.; Zhang, L.L.; Fei, Y.H.; Liu, X.Y.; Li, M.X. On the Formation Mechanisms of Fine Granular Area (FGA) on the Fracture Surface for High Strength Steels in the VHCF Regime. Int. J. Fatigue 2016, 82, 402–410. [Google Scholar] [CrossRef]
- Sippel, J.P.; Kerscher, E. Properties of the Fine Granular Area and Postulated Models for Its Formation during Very High Cycle Fatigue—A Review. Appl. Sci. 2020, 10, 8475. [Google Scholar] [CrossRef]
- Chen, Z.; Dong, Z.; Liu, C.; Dai, Y.; He, C. Characterization on Crack Initiation and Early Propagation Region of Nickel-Based Alloys in Very High Cycle Fatigue. Materials 2022, 15, 5806. [Google Scholar] [CrossRef] [PubMed]
- Zhao, X.; Dongpo, W.; Deng, C.; Liu, Y.; Zongxian, S. The Fatigue Behaviors of Butt Welds Ground Flush in the Super-Long Life Regime. Int. J. Fatigue 2012, 36, 1–8. [Google Scholar] [CrossRef]
- Tridello, A.; Boursier Niutta, C.; Berto, F.; Tedesco, M.M.; Plano, S.; Gabellone, D.; Paolino, D.S. Design against Fatigue Failures: Lower Bound P-S-N Curves Estimation and Influence of Runout Data. Int. J. Fatigue 2022, 162, 106934. [Google Scholar] [CrossRef]
- Liu, Y.; He, C.; Huang, C.; Khan, M.K.; Wang, Q. Very Long Life Fatigue Behaviors of 16Mn Steel and Welded Joint. Struct. Eng. Mech. 2014, 52, 889–901. [Google Scholar] [CrossRef]
- He, C.; Huang, C.; Liu, Y.; Wang, Q. Fatigue Damage Evaluation of Low-Alloy Steel Welded Joints in Fusion Zone and Heat Affected Zone Based on Frequency Response Changes in Gigacycle Fatigue. Int. J. Fatigue 2014, 61, 297–303. [Google Scholar] [CrossRef]
- Shang, D.G. Measurement of Fatigue Damage Based on the Natural Frequency for Spot-Welded Joints. Mater. Des. 2009, 30, 1008–1013. [Google Scholar] [CrossRef]
- Jonsson, B.; Dobmann, G.; Hobbacher, A.F.; Kassner, M.; Marquis, G. IIW Guidelines on Weld Quality in Relationship to Fatigue Strength; Springer International Publishing: Cham, Switzerland, 2016; ISBN 978-3-319-19197-3. [Google Scholar]
- Nishikawa, H.; Furuya, Y. Gigacycle Fatigue Fracture of Low Strength Carbon Steel, Tested Using a Simulated Heat Affected Zone Microstructure. ISIJ Int. 2019, 59, 1926–1928. [Google Scholar] [CrossRef]
- Schaumann, P.; Steppeler, S. Fatigue Tests of Axially Loaded Butt Welds up to Very High Cycles. Procedia Eng. 2013, 66, 88–97. [Google Scholar] [CrossRef]
- Invernizzi, S.; Montagnoli, F.; Carpinteri, A. Experimental Evidence of Specimen-Size Effects on EN-AW6082 Aluminum Alloy in VHCF Regime. Appl. Sci. 2021, 11, 4272. [Google Scholar] [CrossRef]
- Gao, Z.; Wang, D.; Gong, B.; Deng, C.; Wu, S.; Zhang, H. VHCF Behavior of Welded Joints with HFMI Treatment under Moisture Conditions. Weld. J. 2022, 101, 27–42. [Google Scholar] [CrossRef]
- Oyyaravelu, R.; Kuppan, P.; Arivazhagan, N. Metallurgical and Mechanical Properties of Laser Welded High Strength Low Alloy Steel. J. Adv. Res. 2016, 7, 463–472. [Google Scholar] [CrossRef] [PubMed]
- Kou, S. Welding Metallurgy, 2nd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2003; ISBN 3175723993. [Google Scholar]
- Zhu, M.L.; Liu, L.L.; Xuan, F.Z. Effect of Frequency on Very High Cycle Fatigue Behavior of a Low Strength Cr-Ni-Mo-V Steel Welded Joint. Int. J. Fatigue 2015, 77, 166–173. [Google Scholar] [CrossRef]
- Murakami, Y. Metal Fatigue: Effects of Small Defects and Nonmetallic Inclusions, 2nd ed.; Elsevier: New York, NY, USA, 2019; ISBN 9780128138779. [Google Scholar]
- Zhu, M.L.; Xuan, F.Z. Failure Mechanisms and Fatigue Strength Assessment of a Low Strength Cr−Ni−Mo−V Steel Welded Joint: Coupled Frequency and Size Effects. Mech. Mater. 2016, 100, 198–208. [Google Scholar] [CrossRef]
- Hu, J.-T.; Chen, R.; Zhu, G.; Wang, C.; Zhu, M.-L.; Xuan, F.-Z. Heat Dissipation Behavior of a Low-Strength-Steel Welded Joint in Ultrasonic Fatigue. Metals 2022, 12, 1857. [Google Scholar] [CrossRef]
- He, B.; Deng, H.; Jiang, M.; Wei, K.; Li, L. Effect of Ultrasonic Impact Treatment on the Ultra High Cycle Fatigue Properties of SMA490BW Steel Welded Joints. Int. J. Adv. Manuf. Technol. 2018, 96, 1571–1577. [Google Scholar] [CrossRef]
- Wang, P.; Wang, W.; Zhang, M.; Zhou, Q.; Gao, Z. Effects of Specimen Size and Welded Joints on the Very High Cycle Fatigue Properties of Compressor Blade Steel KMN-I. Coatings 2021, 11, 1244. [Google Scholar] [CrossRef]
- Zhu, M.L.; Xuan, F.Z.; Du, Y.N.; Tu, S.T. Very High Cycle Fatigue Behavior of a Low Strength Welded Joint at Moderate Temperature. Int. J. Fatigue 2012, 40, 74–83. [Google Scholar] [CrossRef]
- Zhang, W.C.; Zhu, M.L.; Wang, K.; Xuan, F.Z. Failure Mechanisms and Design of Dissimilar Welds of 9%Cr and CrMoV Steels up to Very High Cycle Fatigue Regime. Int. J. Fatigue 2018, 113, 367–376. [Google Scholar] [CrossRef]
- Li, S.C.; Zhang, W.C.; Zhu, M.L.; Xuan, F.Z. On Specimen Design for High Cycle Fatigue Testing of Welded Joint. Int. J. Fatigue 2020, 136, 105597. [Google Scholar] [CrossRef]
- Zhang, M.; Wang, W.; Wang, P.; Liu, Y.; Li, J. Fatigue Behavior and Mechanism of FV520B-I Welding Seams in a Very High Cycle Regime. Int. J. Fatigue 2016, 87, 22–37. [Google Scholar] [CrossRef]
- Xiong, Z.; Peng, E.; Zeng, L.; Xu, Q. Giga-Cycle Fatigue Behavior of the Nuclear Structure of 316L Weldments. Front. Energy Res. 2021, 9, 696608. [Google Scholar] [CrossRef]
- Himmelbauer, F.; Winter, G.; Grün, F.; Kiesling, C. VHCF Properties and Assessment of Specimens and Thin-Walled Component-like Structures Made of High-Strength Steel X5CrNiCuNb16-4. Int. J. Fatigue 2022, 156, 106645. [Google Scholar] [CrossRef]
- Zhang, M.; Zhang, H.; Li, M.; Liu, L.; Wang, P. Fatigue Behavior and Mechanism of Dog-Bone-Shaped Specimens of FV520B-I in a Very High Cycle Regime. Fatigue Fract. Eng. Mater. Struct. 2022, 45, 3658–3676. [Google Scholar] [CrossRef]
- Xiong, Z.; Wei, D.; Wang, H.; Shi, H.J.; Ma, X. Fatigue Behavior of 316 L Stainless Steel Weldment up to Very-High-Cycle Fatigue Regime. Mater. Res. Express 2019, 6, 076514. [Google Scholar] [CrossRef]
- Cremer, M.; Zimmermann, M.; Christ, H.J. High-Frequency Cyclic Testing of Welded Aluminium Alloy Joints in the Region of Very High Cycle Fatigue (VHCF). Int. J. Fatigue 2013, 57, 120–130. [Google Scholar] [CrossRef]
- Deng, C.; Wang, H.; Gong, B.; Li, X.; Lei, Z. Effects of Microstructural Heterogeneity on Very High Cycle Fatigue Properties of 7050-T7451 Aluminum Alloy Friction Stir Butt Welds. Int. J. Fatigue 2016, 83, 100–108. [Google Scholar] [CrossRef]
- He, C.; Liu, Y.; Dong, J.; Wang, Q.; Wagner, D.; Bathias, C. Through Thickness Property Variations in Friction Stir Welded AA6061 Joint Fatigued in Very High Cycle Fatigue Regime. Int. J. Fatigue 2016, 82, 379–386. [Google Scholar] [CrossRef]
- Chen, Y.; He, C.; Yang, K.; Zhang, H.; Wang, C.; Wang, Q.; Liu, Y. Effects of Microstructural Inhomogeneities and Micro-Defects on Tensile and Very High Cycle Fatigue Behaviors of the Friction Stir Welded ZK60 Magnesium Alloy Joint. Int. J. Fatigue 2019, 122, 218–227. [Google Scholar] [CrossRef]
- Liu, F.; Zhang, H.; Liu, H.; Chen, Y.; Kashif, K.M.; Wang, Q.; Liu, Y. Influence of Welded Pores on Very Long-Life Fatigue Failure of the Electron Beam Welding Joint of TC17 Titanium Alloy. Materials 2019, 12, 1825. [Google Scholar] [CrossRef] [PubMed]
- Liu, F.; Chen, Y.; He, C.; Wang, C.; Li, L.; Liu, Y.; Wang, Q. Very Long Life Fatigue Failure Mechanism of Electron Beam Welded Joint for Titanium Alloy at Elevated Temperature. Int. J. Fatigue 2021, 152, 106446. [Google Scholar] [CrossRef]
- Liu, F.; Chen, Y.; Li, L.; Wang, C.; Wang, Q.; Liu, Y. Influence of Welded Pores on Fatigue Behavior of TC17 Titanium Alloy Welded Joints Subjected to Gigacycle Regime at Room and High Temperatures. J. Mater. Sci. Technol. 2023. [Google Scholar] [CrossRef]
- Zheng, Y.; Zhao, Z.; Zhang, Z.; Zong, W.; Dong, C. Internal Crack Initiation Characteristics and Early Growth Behaviors for Very-High-Cycle Fatigue of a Titanium Alloy Electron Beam Welded Joints. Mater. Sci. Eng. A 2017, 706, 311–318. [Google Scholar] [CrossRef]
- Matsuda, K.; Kodama, S. Observation of Fatigue Microcracks and Estimation of Fatigue Strength of a Thin Sheet Arc Welded Part Considering Micro-Ripples. Int. J. Fatigue 2021, 145, 106087. [Google Scholar] [CrossRef]
- Schork, B.; Kucharczyk, P.; Madia, M.; Zerbst, U.; Hensel, J.; Bernhard, J.; Tchuindjang, D.; Kaffenberger, M.; Oechsner, M. The Effect of the Local and Global Weld Geometry as Well as Material Defects on Crack Initiation and Fatigue Strength. Eng. Fract. Mech. 2018, 198, 103–122. [Google Scholar] [CrossRef]
- Toumpis, A.; Galloway, A.; Molter, L.; Polezhayeva, H. Systematic Investigation of the Fatigue Performance of a Friction Stir Welded Low Alloy Steel. Mater. Des. 2015, 80, 116–128. [Google Scholar] [CrossRef]
- Xing, S.; Dong, P. Fatigue of Titanium Weldments: S-N Testing and Analysis for Data Transferability among Different Joint Types. Mar. Struct. 2017, 53, 1–19. [Google Scholar] [CrossRef]
- Hobbacher, A.F. Recommendations for Fatigue Design of Welded Joints and Components; Springer International Publishing: Cham, Switzerland, 2016; ISBN 978-3-319-23756-5. [Google Scholar]
- BS 7608:2014; Guide to Fatigue Design and Assessment of Steel Products. British Standards Institution: London, UK, 2014.
- AWS D1.1/D1.1M:2020; Structural Welding Code—Steel. American Welding Society: Doral, FL, USA, 2020.
- BS EN 1993-1-9:2005; Eurocode 3: Design of Steel Structures—Fatigue. British Standards Institution: London, UK, 2005.
- DNV-RP-C203; Fatigue Design of Offshore Steel Structures. DNV: Høvik, Norway, 2019.
- Hobbacher, A.F. The New IIW Recommendations for Fatigue Assessment of Welded Joints and Components—A Comprehensive Code Recently Updated. Int. J. Fatigue 2009, 31, 50–58. [Google Scholar] [CrossRef]
- Rumiche, F.; Noriega, A.; Lean, P.; Fosca, C. Metallurgical Failure Analysis of a Welded Drive Beam of a Vibrating Screen. Eng. Fail. Anal. 2020, 118, 104936. [Google Scholar] [CrossRef]
- Wolf, T. Application of Weldment Fatigue Methods to Conveyor Pulley Design. In Proceedings of the Bulk Material Handling by Conveyor Belt II; Precision Pulley, Inc.: Orlando, FL, USA, 1998. [Google Scholar]
- Alencar, G.; de Jesus, A.; da Silva, J.G.S.; Calçada, R. Fatigue Cracking of Welded Railway Bridges: A Review. Eng. Fail. Anal. 2019, 104, 154–176. [Google Scholar] [CrossRef]
- Pakenham, B.; Ermakova, A.; Mehmanparast, A. A Review of Life Extension Strategies for Offshore Wind Farms Using Techno-Economic Assessments. Energies 2021, 14, 1936. [Google Scholar] [CrossRef]

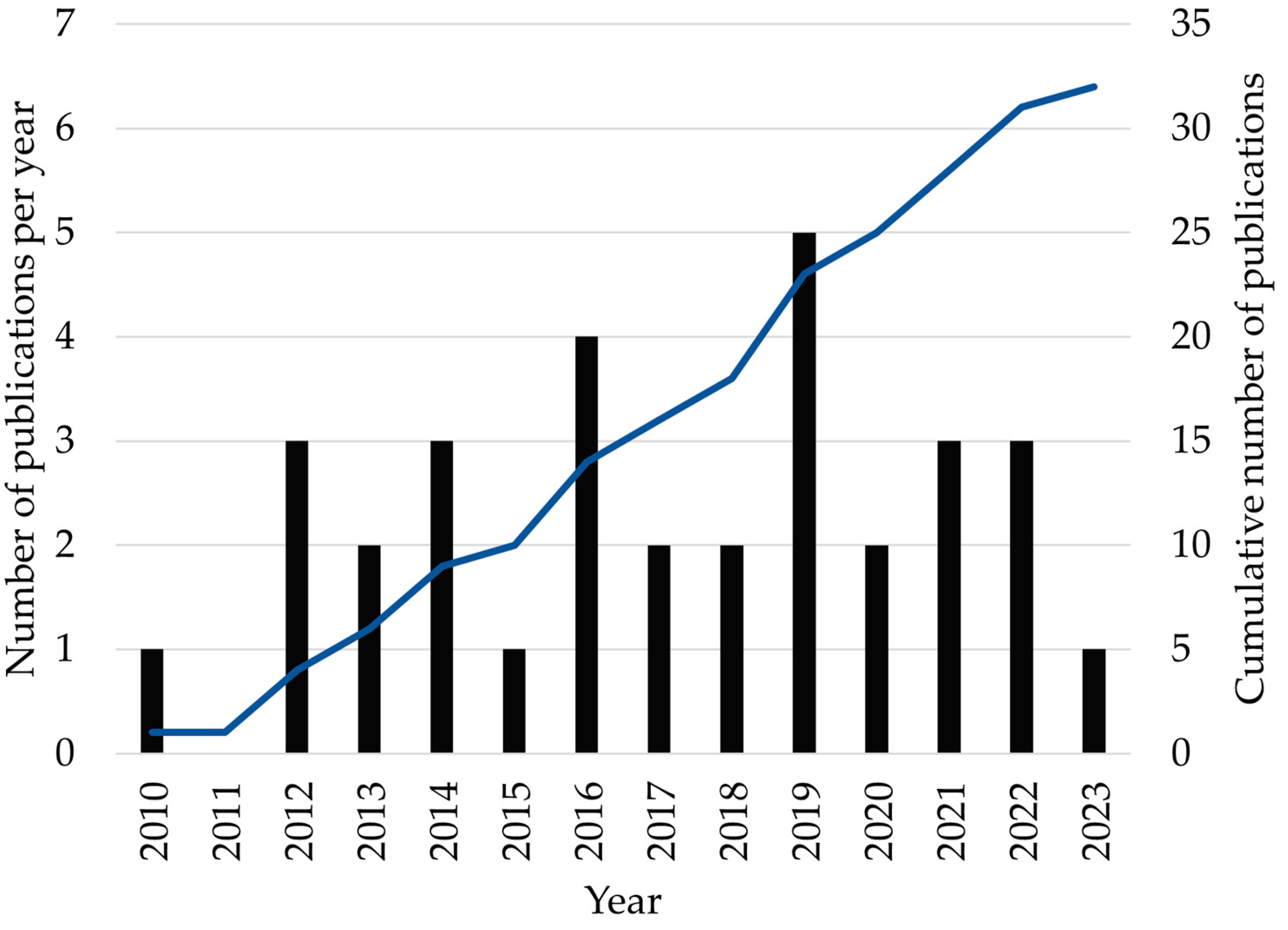
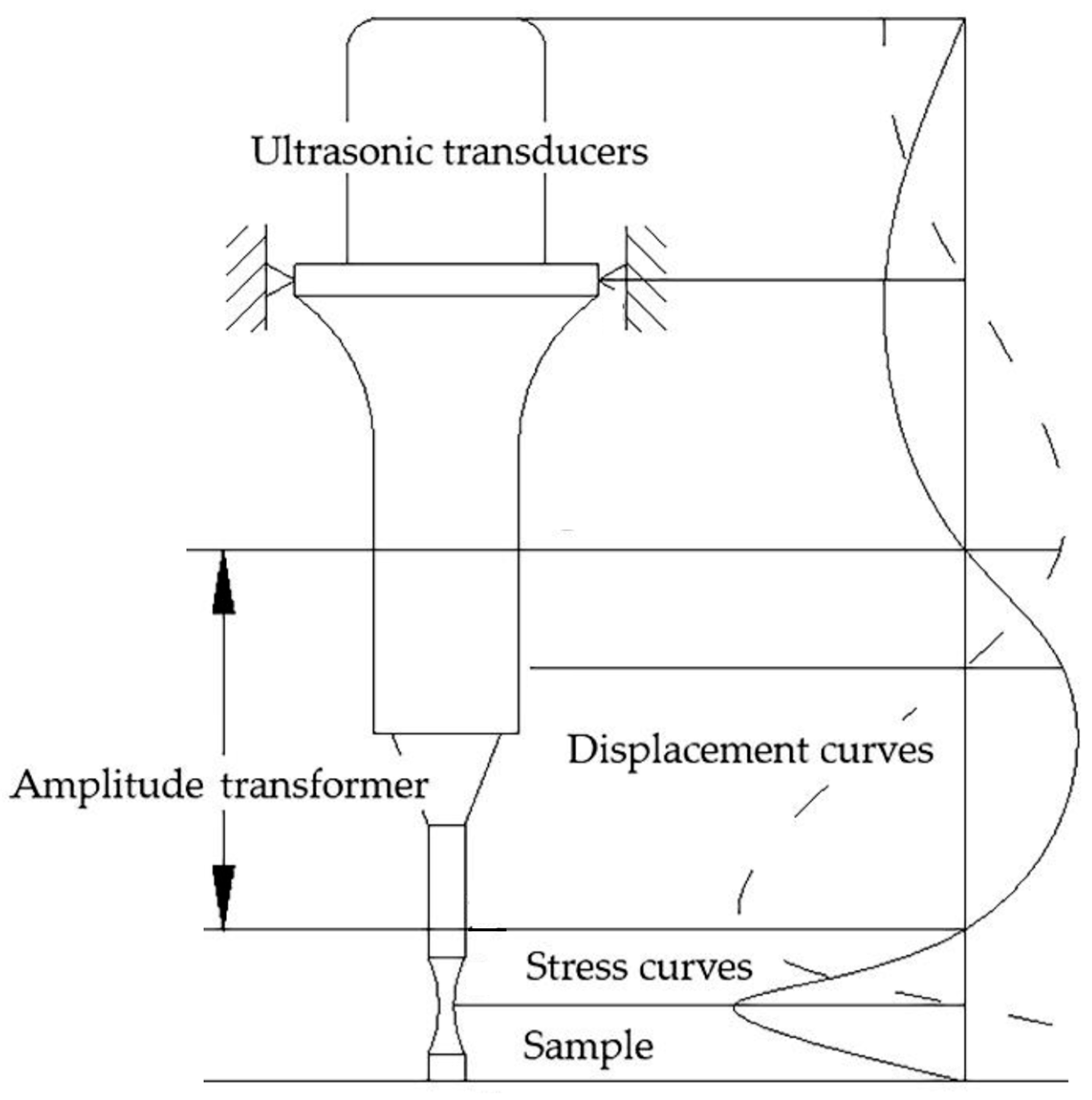
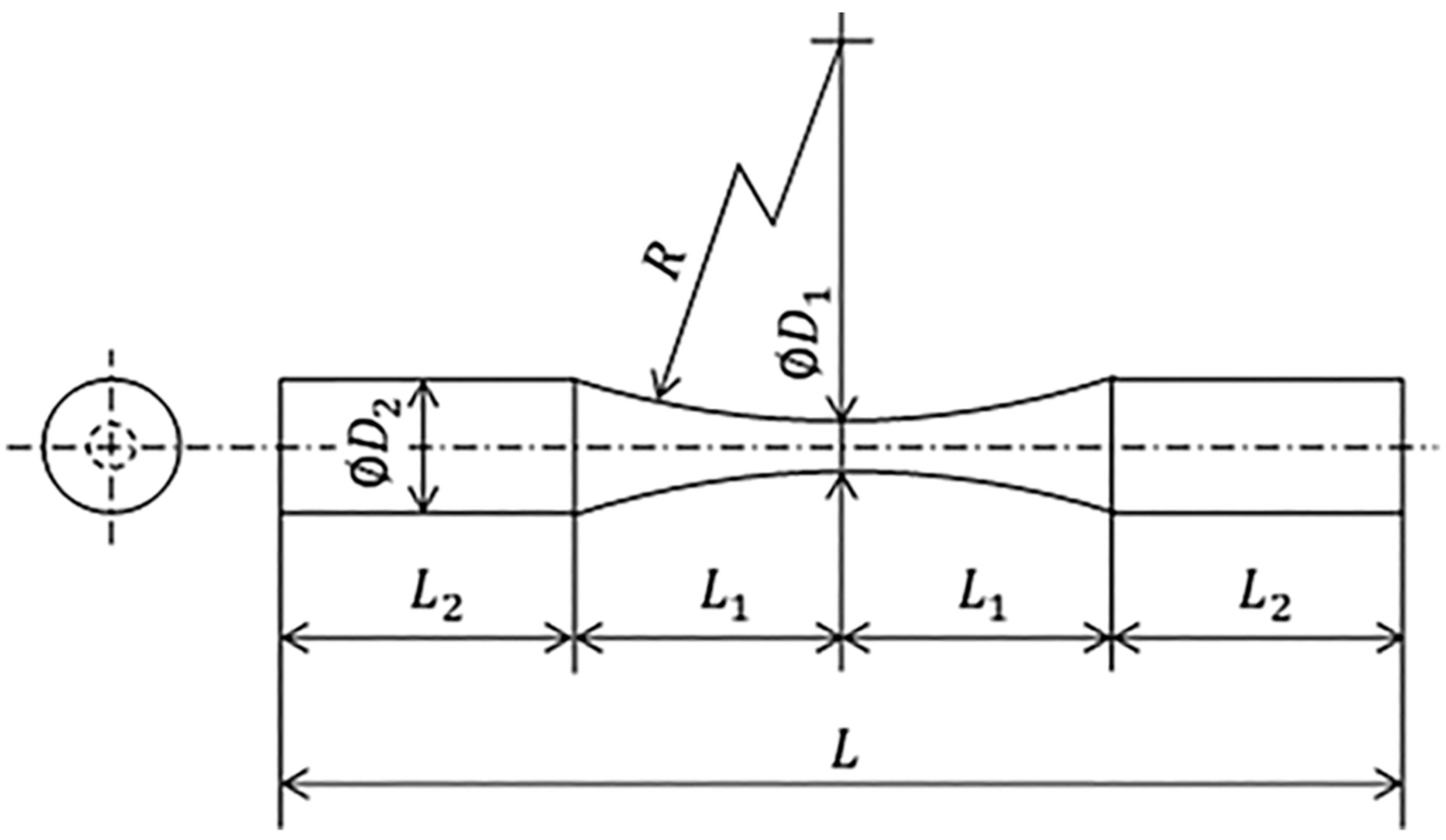
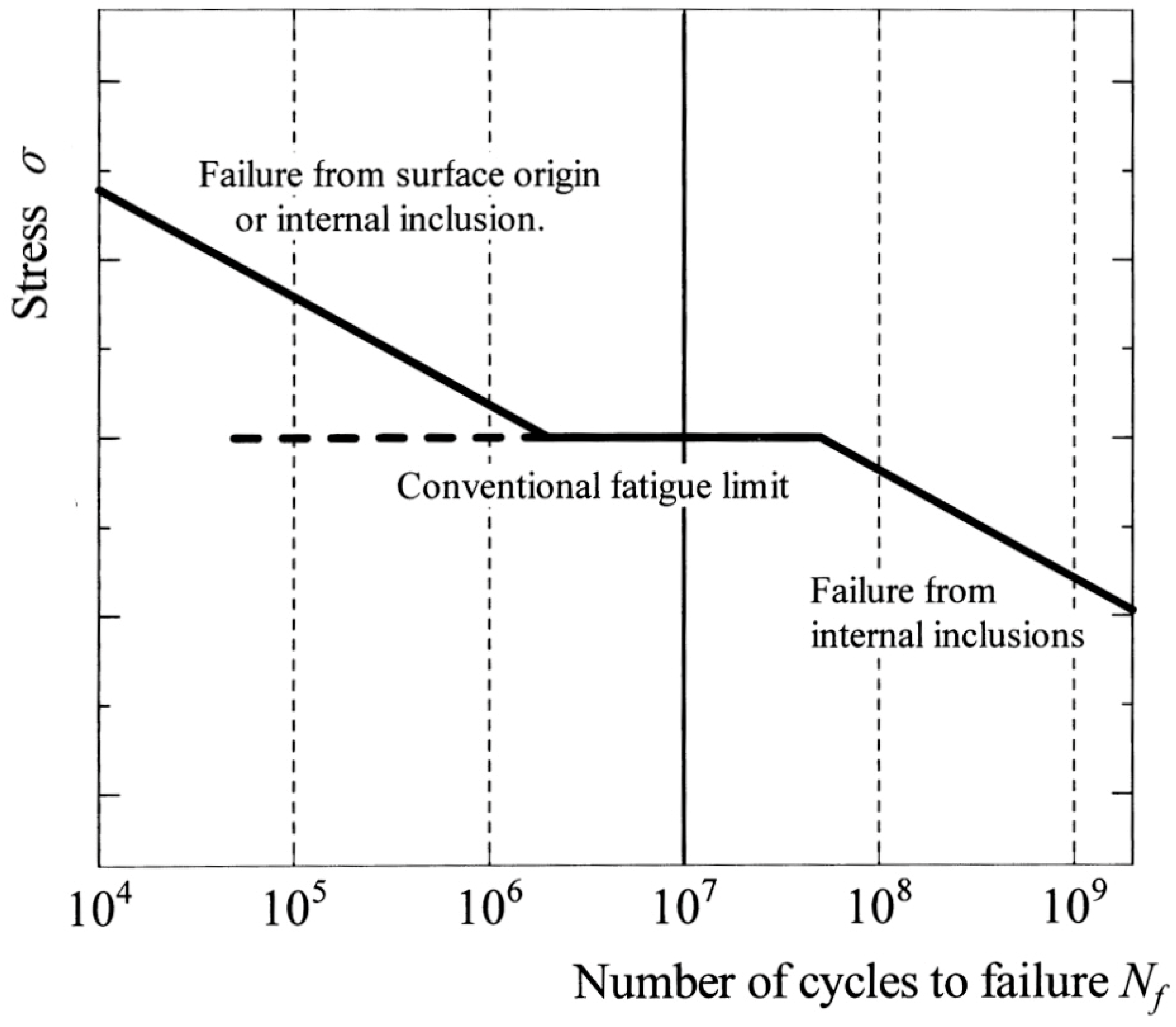
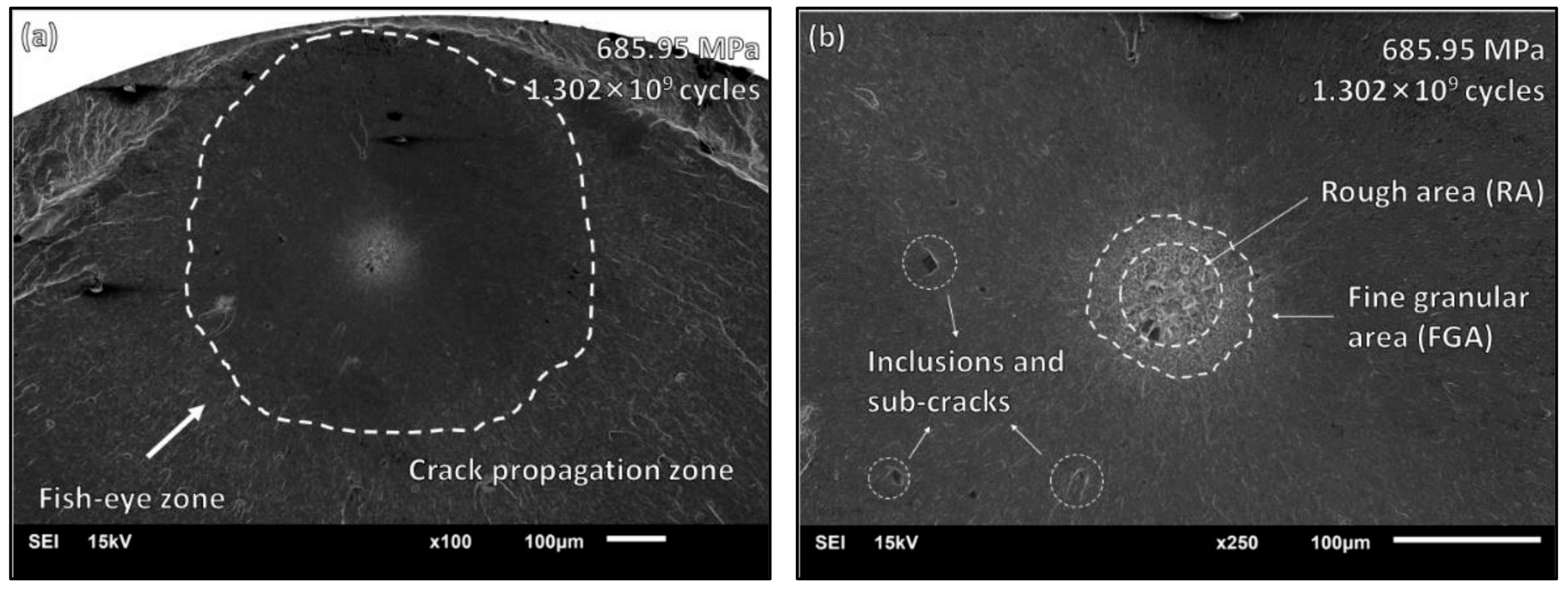
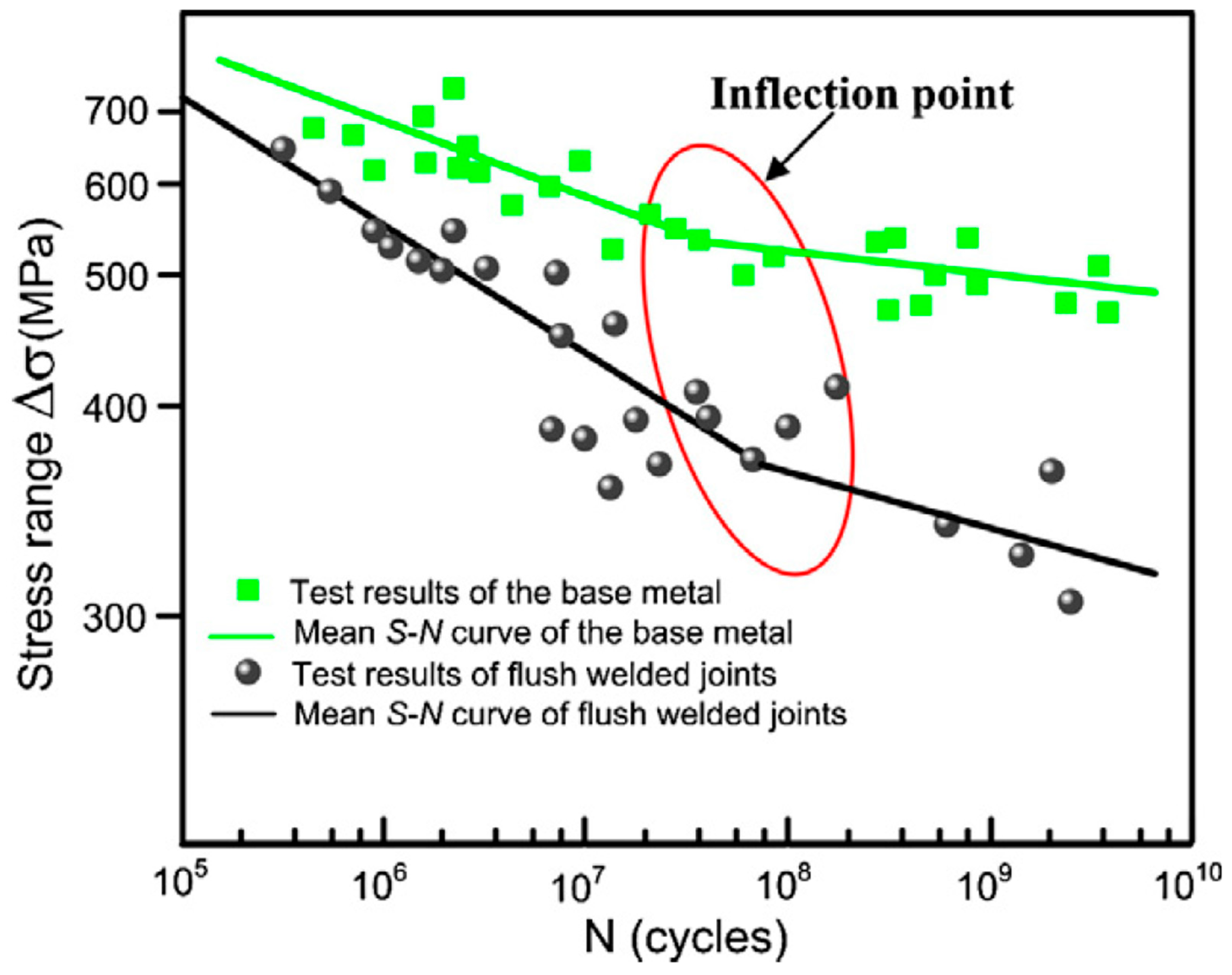


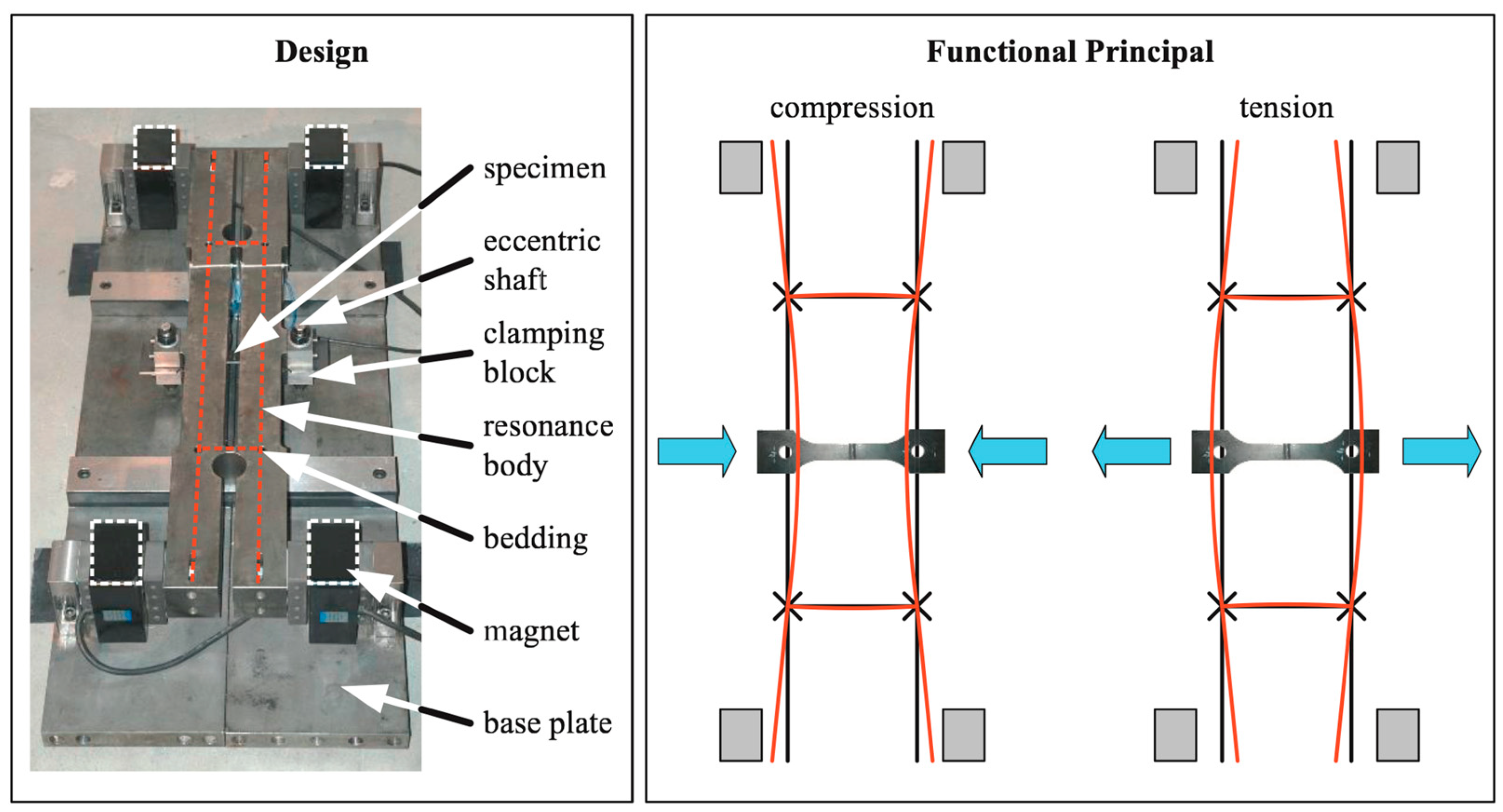
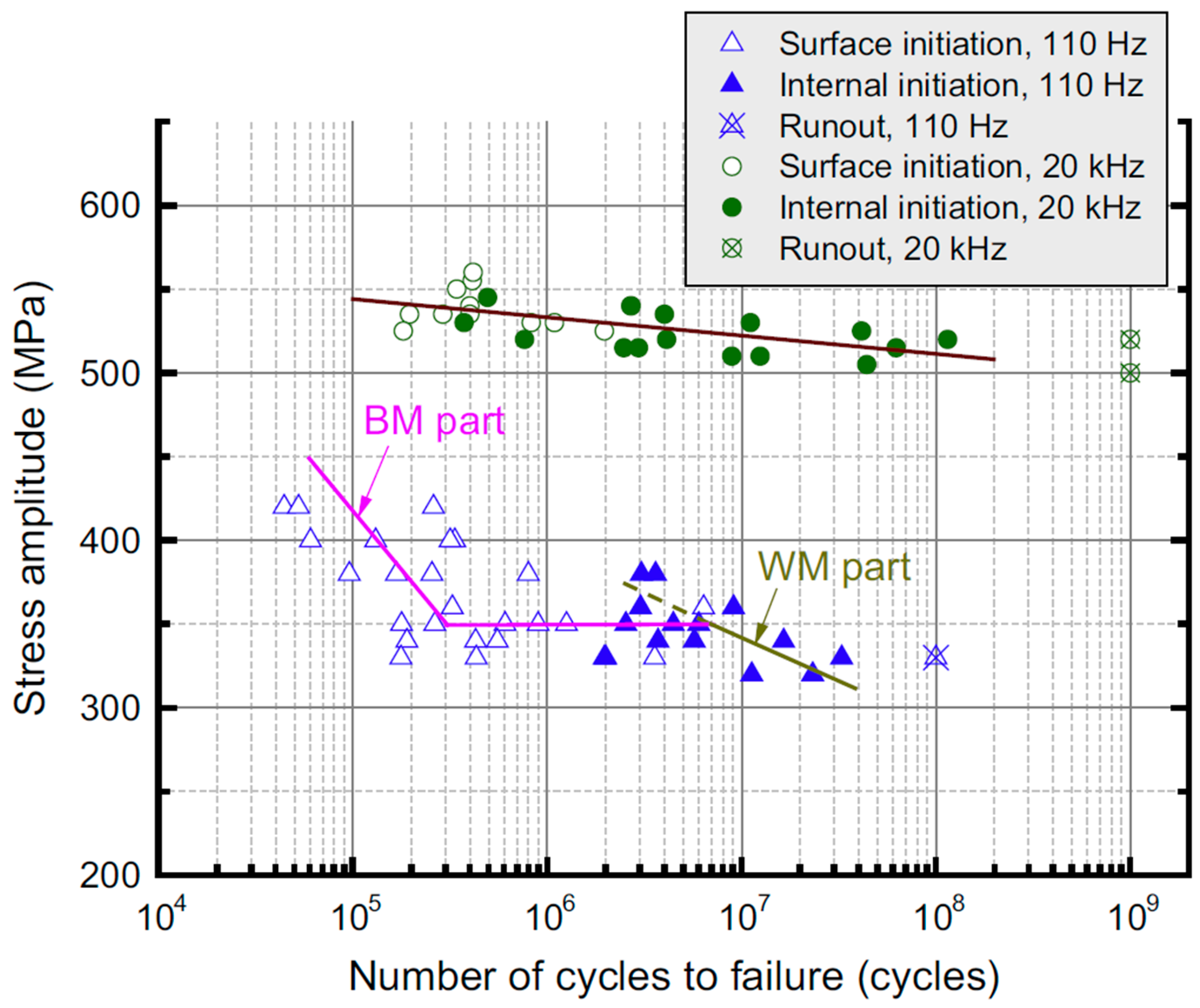



| Base Metal | Welding Method | Specimen Type | Research Focus | Reference |
|---|---|---|---|---|
| EH36 | FCAW | Cylindrical dog-bone | Comparison of fatigue strength to base metal and effect of inclusions | [41] |
| Q235 and Q345 | Arc welding (not specified) | Cruciform joint | Effect of UPT | [15] |
| Q345 | Arc welding (not specified) | Cylindrical hourglass | Comparison of fatigue strength to base metal | [12] |
| 16Mn | GTAW | As-welded cylindrical hourglass and cruciform | Specimen geometry | [43] |
| Q345 | SMAW | Notched cylindrical hourglass | Comparison of fatigue strength of different weld regions | [44] |
| 0.15% carbon steel | - | Cylindrical hourglass | Fatigue strength of HAZ simulated by heat treatment | [47] |
| S355J2+N | GMAW | Rectangular dog-bone | 390 Hz resonance fatigue testing machine | [48] |
| Q355B | FCAW | Cruciform | Corrosion fatigue testing at 110 Hz | [50] |
| Base Metal | Welding Method | Specimen Type | Research Focus | Reference |
|---|---|---|---|---|
| 25Cr2Ni2MoV | SAW | Cylindrical dog-bone | Assessment of frequency effect | [53] |
| Assessment of size effect | [55] | |||
| Heat dissipation during UFT | [56] | |||
| Elevated temperature testing at 100 Hz | [61] | |||
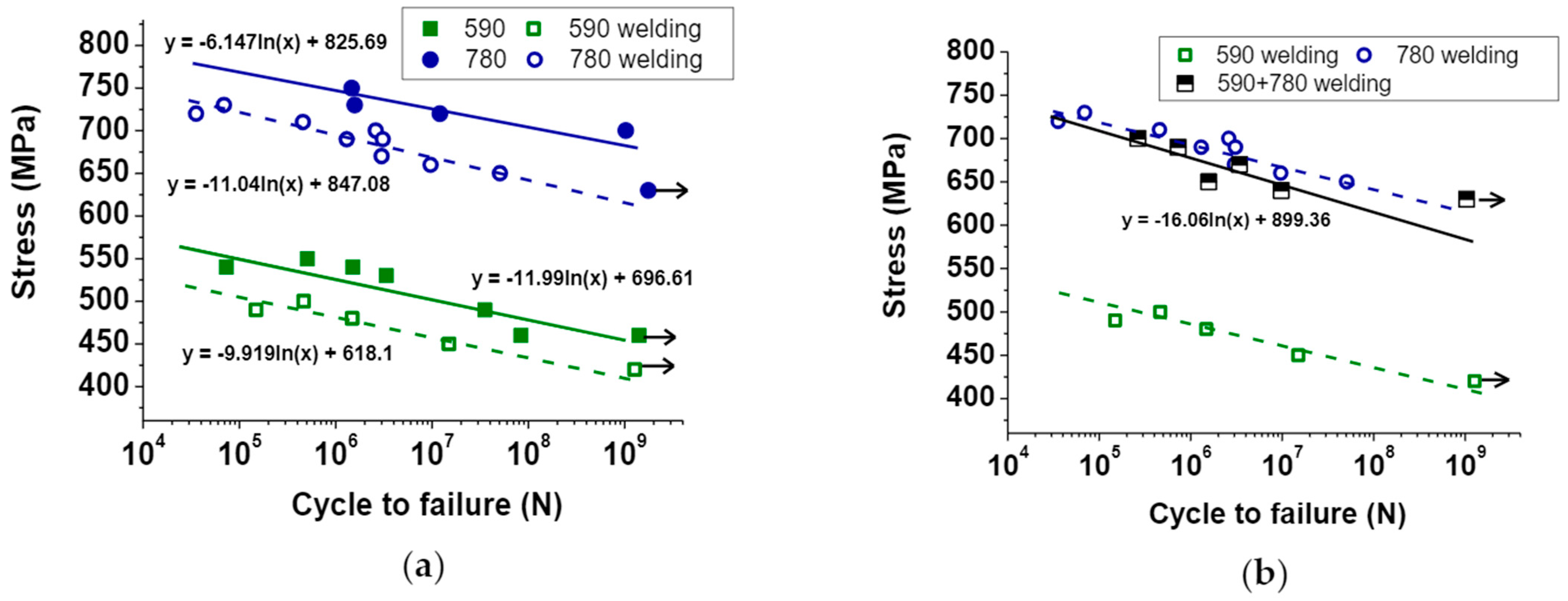
| 590 FB and 780 FB | GMAW | Rectangular dog-bone | Comparison of fatigue strength to base metal and dissimilar welds | [13] |
| SMA490BW | GMAW | As-welded rectangular dog-bone | Effect of UPT | [57] |
| KMN-I | SMAW | Cylindrical hourglass | Comparison of fatigue strength to base metal | [58] |
| CrMoV Steel | SAW | Cylindrical hourglass | Elevated temperature testing at 100 Hz | [59] |
| 9% Cr Steel and CrMoV Steel | SAW | Cylindrical dog-bone | Comparison of base metals and dissimilar weld at elevated temperature | [60] |
| Base Metal | Welding Method | Specimen Type | Research Focus | Reference |
|---|---|---|---|---|
| FV520B-I | SMAW | Cylindrical hourglass | Effect of surface roughness | [62] |
| Cylindrical dog-bone | Size effect | [65] | ||
| 316L | GTAW | Cylindrical dog-bone | Comparison to base metal | [66] |
| GTAW and LBW | Cylindrical dog-bone | Comparison of weld methods | [63] |
| Base Metal | Welding Method | Specimen Type | Research Focus | Reference |
|---|---|---|---|---|
| AA6082 | GMAW | Rectangular dog bone and notched | Comparison of fatigue strength of different weld regions | [67] |
| AA7050 | FSW | Cylindrical dog-bone | Comparison of fatigue strength to base metal | [68] |
| AA6061 | FSW | Rectangular dog-bone | Comparison of fatigue strength through-thickness | [69] |
| ZK60 | FSW | Rectangular dog-bone | Effect of welding defects | [70] |
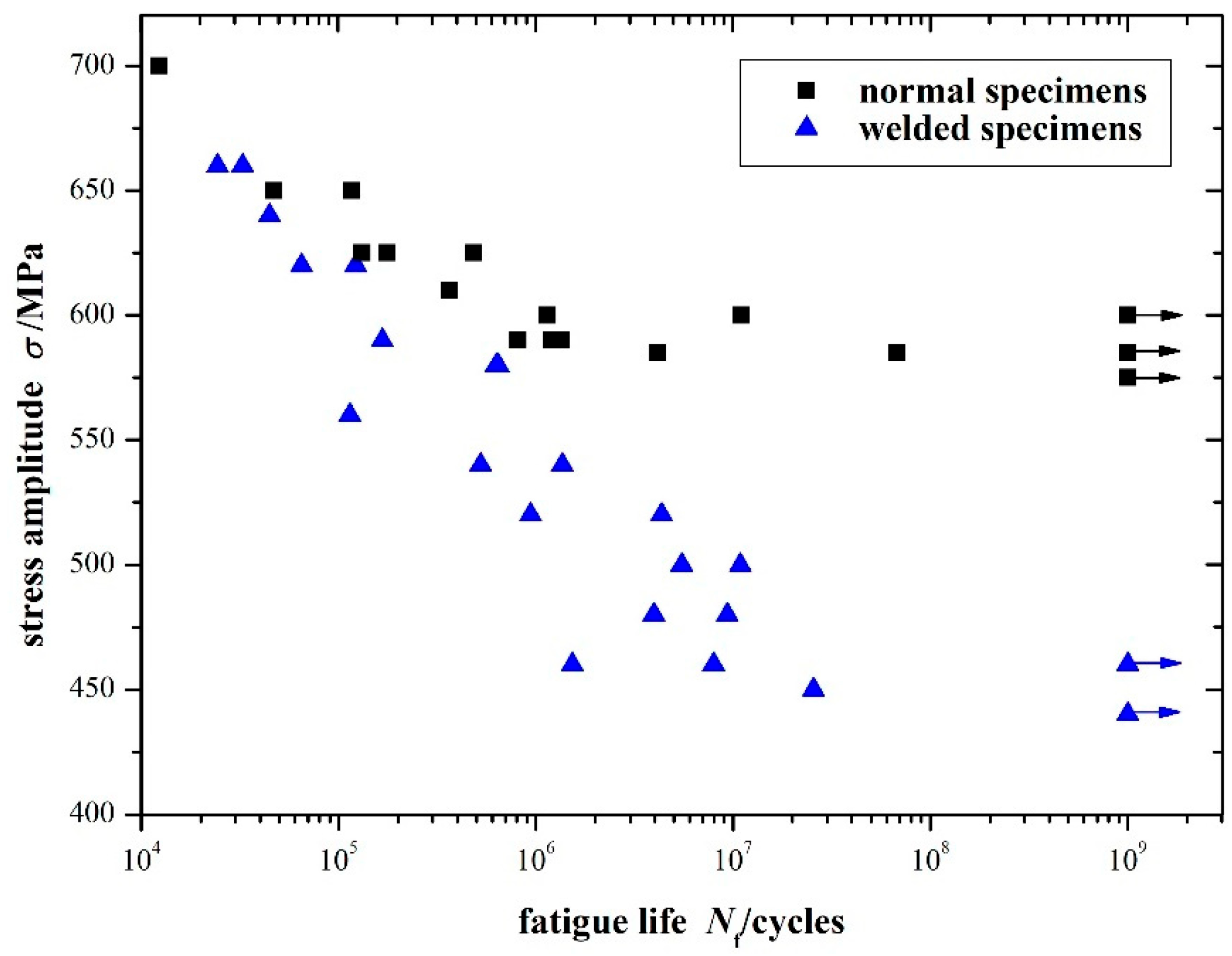
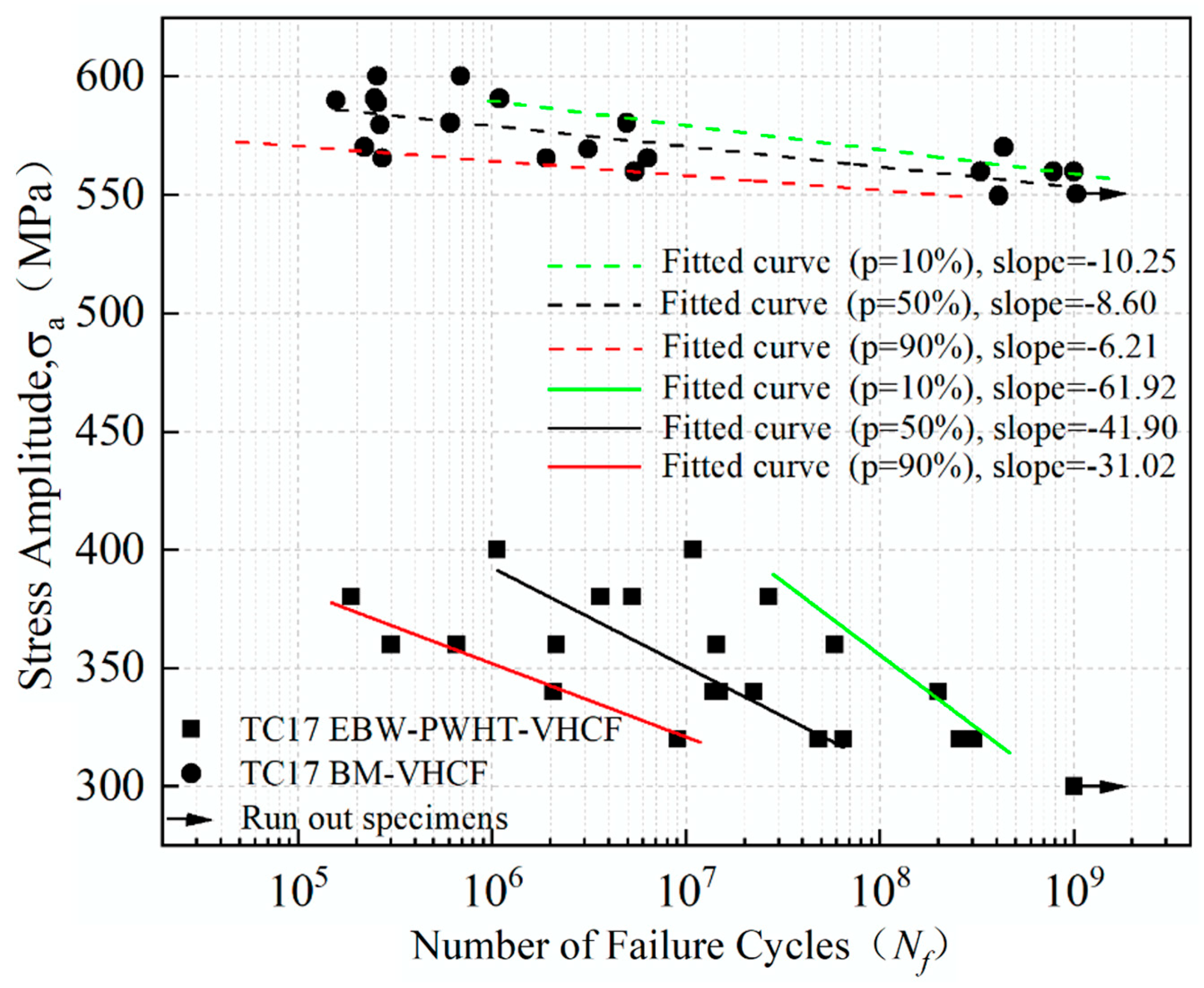
| TC17 | EBW | Cylindrical dog-bone | Effect of welding pores | [71] |
| Fatigue strength at elevated temperature | [72] | |||
| Comparison of fatigue strength to base metal at elevated temperature | [73] | |||
| TC21 | EBW | Cylindrical hourglass | Effect of welding pores | [74] |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
England, A.; Toumpis, A.; Gorash, Y. Very High Cycle Fatigue of Welds: A Review. Metals 2023, 13, 1860. https://doi.org/10.3390/met13111860
England A, Toumpis A, Gorash Y. Very High Cycle Fatigue of Welds: A Review. Metals. 2023; 13(11):1860. https://doi.org/10.3390/met13111860
Chicago/Turabian StyleEngland, Andrew, Athanasios Toumpis, and Yevgen Gorash. 2023. "Very High Cycle Fatigue of Welds: A Review" Metals 13, no. 11: 1860. https://doi.org/10.3390/met13111860
APA StyleEngland, A., Toumpis, A., & Gorash, Y. (2023). Very High Cycle Fatigue of Welds: A Review. Metals, 13(11), 1860. https://doi.org/10.3390/met13111860






