Microsegregation Influence on Austenite Formation from Ferrite and Cementite in Fe–C–Mn–Si and Fe–C–Si Steels
Abstract
1. Introduction
2. Materials and Methods
3. Results
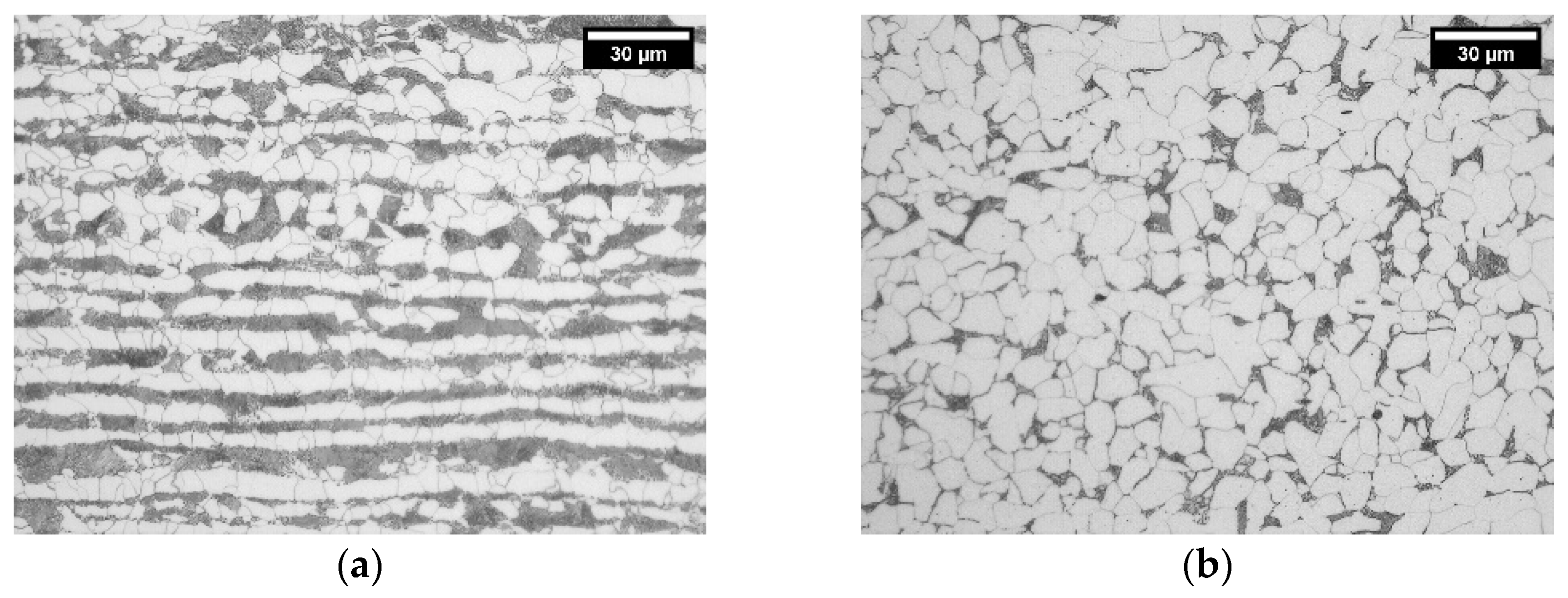
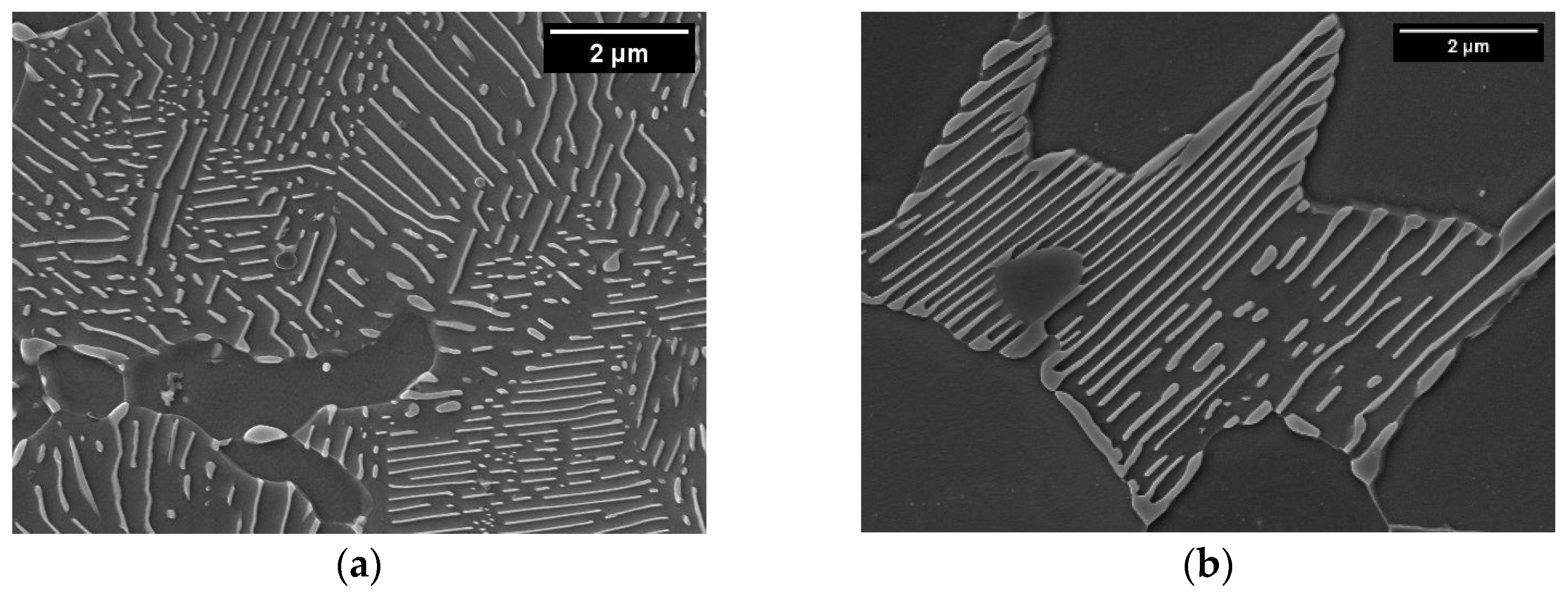
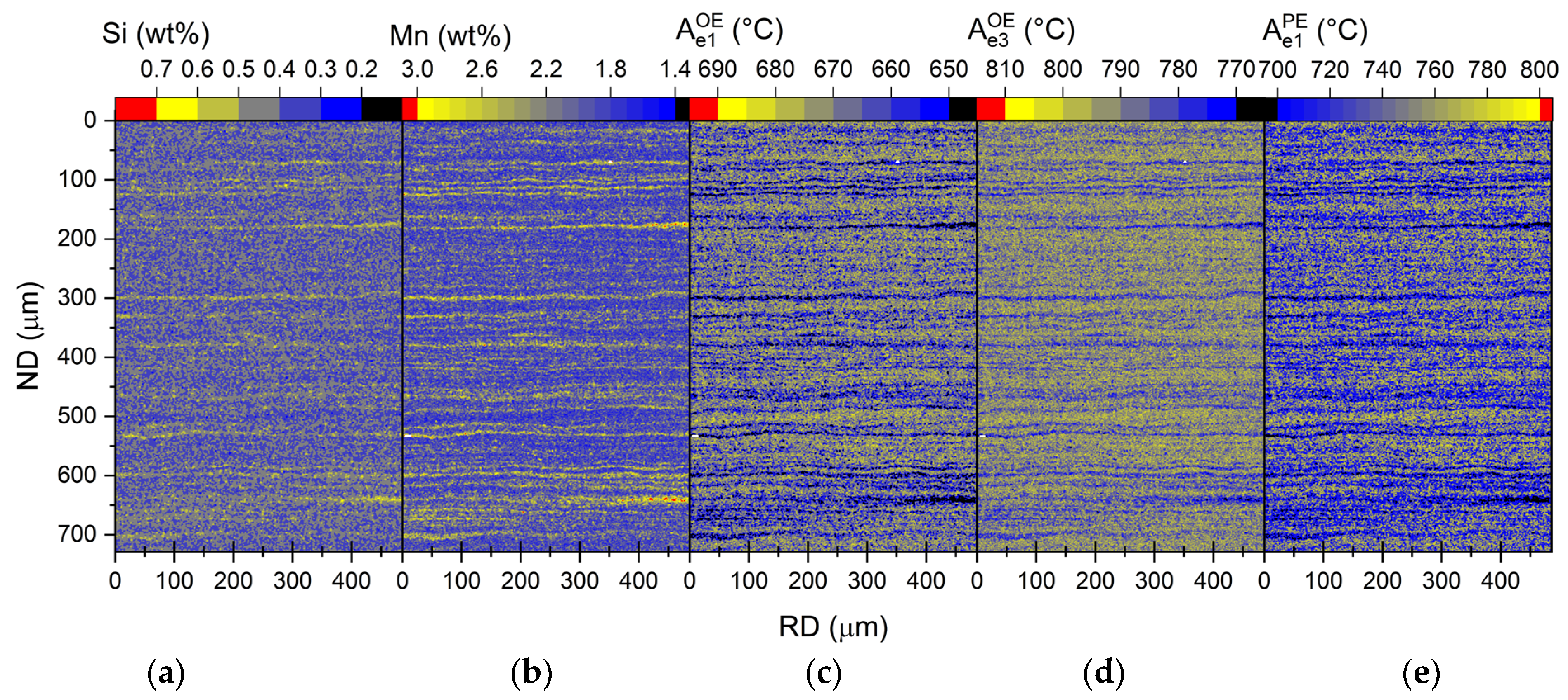
3.1. Characterization of Initial Microstructure
3.2. Ferrite/Pearlite-to-Austenite Transformation
3.3. Nucleation
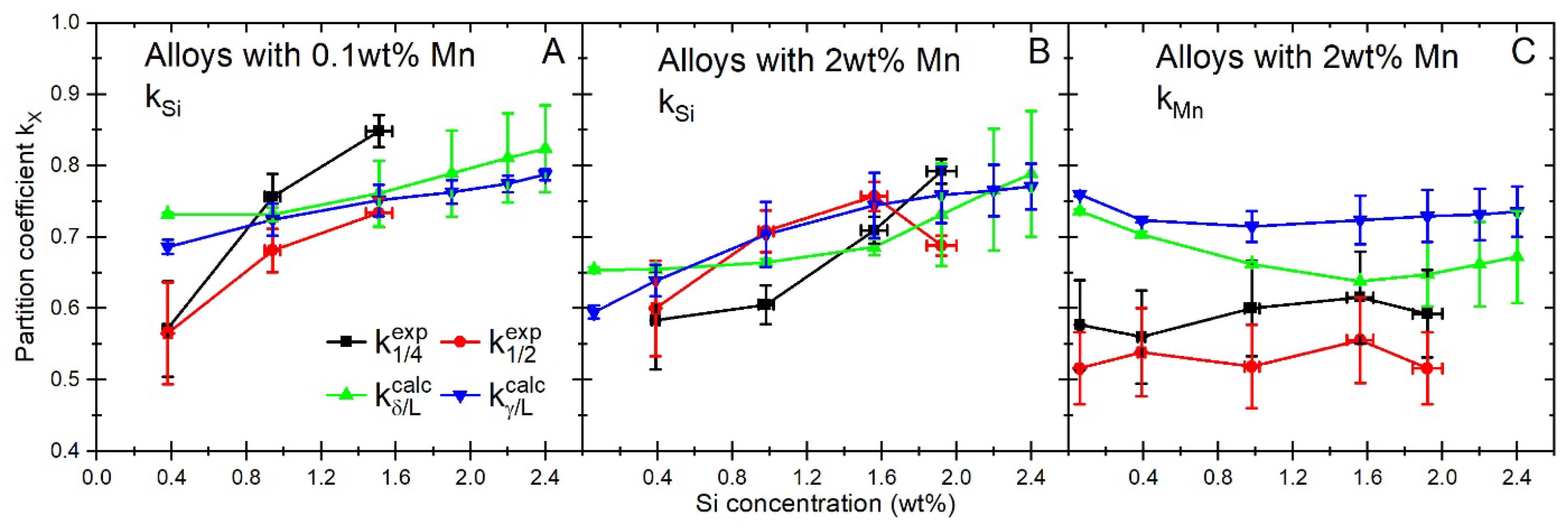
3.3.1. Nucleation Start Composition
3.3.2. Driving Force for Austenite Nucleation
3.3.3. Strain Energy of Austenite Nucleation
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Matlock, D.; Speer, J.; De Moor, E.; Gibbs, P. Recent Developments in Advanced High Strength Sheet Steels for Automotive Applications: An Overview. Jestech 2012, 15, 1–12. [Google Scholar]
- Gibbs, P.J.; De Moor, E.; Merwin, M.J.; Clausen, B.; Speer, J.G.; Matlock, D.K. Austenite Stability Effects on Tensile Behavior of Manganese-Enriched-Austenite Transformation-Induced Plasticity Steel. Metall. Mater. Trans. A Phys. Metall. Mater. Sci. 2011, 42, 3691–3702. [Google Scholar] [CrossRef]
- Allain, S.Y.P.; Pushkareva, I.; Gouné, M.; Scott, C.; Teixeira, J. Dual-Phase Steels: The First Family of Advanced High Strength Steels. Encycl. Mater. Met. Alloys 2022, 2, 37–62. [Google Scholar] [CrossRef]
- Maresca, F.; Kouznetsova, V.G.; Geers, M.G.D. Deformation Behaviour of Lath Martensite in Multi-Phase Steels. Scr. Mater. 2016, 110, 74–77. [Google Scholar] [CrossRef]
- Hasegawa, K.; Kawamura, K.; Urabe, T.; Hosoya, Y. Effects of Microstructure on Stretch-Flange-Formability of 980 MPa Grade Cold-Rolled Ultra High Strength Steel Sheets. ISIJ Int. 2004, 44, 603–609. [Google Scholar] [CrossRef]
- Hisker, F.; Thiessen, R.; Heller, T. Influence of Microstructure on Damage in Advanced High Strength Steels. Mater. Sci. Forum 2012, 706–709, 925–930. [Google Scholar] [CrossRef]
- Sun, X.; Choi, K.S.; Soulami, A.; Liu, W.N.; Khaleel, M.A. On Key Factors Influencing Ductile Fractures of Dual Phase (DP) Steels. Mater. Sci. Eng. A 2009, 526, 140–149. [Google Scholar] [CrossRef]
- Soyarslan, C.; Malekipour Gharbi, M.; Tekkaya, A.E. A Combined Experimental-Numerical Investigation of Ductile Fracture in Bending of a Class of Ferritic-Martensitic Steel. Int. J. Solids Struct. 2012, 49, 1608–1626. [Google Scholar] [CrossRef]
- Kim, J.H.; Lee, M.G.; Kim, D.; Matlock, D.K.; Wagoner, R.H. Hole-Expansion Formability of Dual-Phase Steels Using Representative Volume Element Approach with Boundary-Smoothing Technique. Mater. Sci. Eng. A 2010, 527, 7353–7363. [Google Scholar] [CrossRef]
- Battle, T.P. The Effects of Solid and Liquid-Phase Diffucion on Micro-Segregation in Castings. In Proceedings of the F. Weinberg International Symposium on Solidification Processing, Hamilton, ON, Canada, 27–29 August 1990; Lait, J.E., Samarasekera, I.V., Eds.; Pergamon: Oxford, UK, 1990; pp. 157–170. [Google Scholar]
- Emtage, P.A.; Wunnenberg, K.; Hatonen, T.; Bobadilla, M.; Llanos, J.M.; De Santis, M. Improved Control of Segregation in Contunous Casting and Hot Rolling Processes; European Commission: Brussels, Belgium, 2002.
- Bos, C.; Mecozzi, M.G.; Hanlon, D.N.; Aarnts, M.P.; Sietsma, J. Application of a Three-Dimensional Microstructure Evolution Model to Identify Key Process Settings for the Production of Dual-Phase Steels. Met. Mater. Trans. A Phys. Met. Mater. Sci. 2011, 42, 3602–3610. [Google Scholar] [CrossRef]
- Lippard, H.E.; Campbell, C.E.; Björklind, T.; Borggren, U.; Kellgren, P.; Dravid, V.P.; Olson, G.B. Microsegregation Behavior during Solidification and Homogenization of AerMet100 Steel. Metall. Mater. Trans. B Process Metall. Mater. Process. Sci. 1998, 29, 205–210. [Google Scholar] [CrossRef]
- Caballero, F.G.; García-Junceda, A.; Capdevila, C.; De Andrés, C.G. Evolution of Microstructural Banding during the Manufacturing Process of Dual Phase Steels. Mater. Trans. 2006, 47, 2269–2276. [Google Scholar] [CrossRef]
- Choudhary, S.K.; Ganguly, S.; Sengupta, A.; Sharma, V. Solidification Morphology and Segregation in Continuously Cast Steel Slab. J. Mater. Process. Technol. 2017, 243, 312–321. [Google Scholar] [CrossRef]
- Fisher, R.M.; Speich, G.R.; Cuddy, L.J.; Hu, H. Phase Transformations during Steel Production. In Physical Chemistry in Metallurgy: Proceedings of the Darken Conference; Research Lab.: Monroeville, PA, USA, 1976; pp. 463–488. [Google Scholar]
- Han, L.; Santillana, B.; Zhuang, L. Investigating the Effects of Solidus Temperature on the Analytical Microsegregation Model. J. Mater. Res. Technol. 2022, 18, 138–146. [Google Scholar] [CrossRef]
- Kučera, J.; Stránský, K. Diffusion in Iron, Iron Solid Solutions and Steels. Mater. Sci. Eng. 1982, 52, 1–38. [Google Scholar] [CrossRef]
- Al-Gahtani, M.; Dippenaar, R. Mechanical Properties of Dendritic and Inter-Dendritic Regions in as- Cast Medium-Carbon Steel. Adv. Mat. Res. 2014, 894, 104–109. [Google Scholar] [CrossRef]
- Offerman, S.E.; Van Dijk, N.H.; Rekveldt, M.T.; Sietsma, J.; Van der Zwaag, S. Ferrite/Pearlite Band Formation in Hot Rolled Medium Carbon Steel. Mater. Sci. Technol. 2002, 18, 297–303. [Google Scholar] [CrossRef]
- Ennis, B.L.; Bos, C.; Aarnts, M.P.; Lee, P.D.; Jimenez-Melero, E. Work Hardening Behaviour in Banded Dual Phase Steel Structures with Improved Formability. Mater. Sci. Eng. A 2018, 713, 278–286. [Google Scholar] [CrossRef]
- Ennis, B.L.; Jimenez-Melero, E.; Mostert, R.; Santillana, B.; Lee, P.D. The Role of Aluminium in Chemical and Phase Segregation in a TRIP-Assisted Dual Phase Steel. Acta Mater. 2016, 115, 132–142. [Google Scholar] [CrossRef]
- Wang, H.; Su, F.; Wen, Z.; Li, C. Effects of Mn and Si on the Ferrite Decarburization of Spring Steel. J. Mater. Res. Technol. 2023, 27, 363–371. [Google Scholar] [CrossRef]
- Bandi, B.; Van Krevel, J.; Nandi, S.; Husain, Z.; Srirangam, P. Effect of Manganese Bands on Austenite Formation of Low Carbon Steels in Dual Phase Steel Manufacture. Mater. Today Proc. 2021, 41, 1030–1034. [Google Scholar] [CrossRef]
- Krugla, M.; Offerman, S.E.; Sietsma, J.; Seda, P.; Hanlon, D.N. Microchemical Banding of Silicon and Manganese in Steel and Its Effect on the Microstructural Banding and Properties. Int. Symp. New Dev. Adv. High-Strength Sheet Steels 2017, 199–208. [Google Scholar]
- Andersson, J.O.H.; Hoglund, T.; Shi, L.; Sundman, B. Thermo-Calc and DICTRA. Computational Tools for Materials Science. Calphad 2002, 26, 273–312. [Google Scholar] [CrossRef]
- Gao, P.; Li, F.; An, K.; Zhao, Z.; Chu, X.; Cui, H. Microstructure and Deformation Mechanism of Si-Strengthened Intercritically Annealed Quenching and Partitioning Steels. Mater. Charact. 2022, 191, 112145. [Google Scholar] [CrossRef]
- Celada, C.; Toda-Caraballo, I.; Kim, B.; San Martín, D. Chemical Banding Revealed by Chemical Etching in a Cold-Rolled Metastable Stainless Steel. Mater. Charact. 2013, 84, 142–152. [Google Scholar] [CrossRef]
- Feng, R.; Li, S.; Zhu, X.; Ao, Q. Microstructural Characterization and Formation Mechanism of Abnormal Segregation Band of Hot Rolled Ferrite/Pearlite Steel. J. Alloys Compd. 2015, 646, 787–793. [Google Scholar] [CrossRef]
- HajyAkbary, F.; Sietsma, J.; Petrov, R.H.; Miyamoto, G.; Furuhara, T.; Santofimia, M.J. A Quantitative Investigation of the Effect of Mn Segregation on Microstructural Properties of Quenching and Partitioning Steels. Scr. Mater. 2017, 137, 27–30. [Google Scholar] [CrossRef]
- Karimi, Y.; Hossein Nedjad, S.; Miyamoto, G.; Shirazi, H.; Furuhara, T. Banding Effects on the Process of Grain Refinement by Cold Deformation and Recrystallization of Acicular C-Mn Steel. Mater. Sci. Eng. A 2017, 697, 1–7. [Google Scholar] [CrossRef]
- Verhoeven, J. Banding: Microsegregation-Induced. In Encyclopedia of Iron, Steel, and Their Alloys; Taylor and Francis: New York, NY, USA, 2016; pp. 320–330. [Google Scholar]
- Wang, J.; Guo, F.; Wang, Z.; Xie, Z.; Shang, C.; Wang, X.; Bandi, B.; Van Krevel, J.; Nandi, S.; Husain, Z.; et al. Influence of centerline segregation on the crystallographic features and mechanical properties of a high-strength low-alloy steel. Scr. Mater. 2019, 697, 89–103. [Google Scholar] [CrossRef]
- Wang, J.; Guo, F.; Wang, Z.; Xie, Z.; Shang, C.; Wang, X. Influence of Centerline Segregation on the Crystallographic Features and Mechanical Properties of a High-Strength Low-Alloy Steel. Mater. Lett. 2020, 267, 127512. [Google Scholar] [CrossRef]
- Teixeira, J.; Moreno, M.; Allain, S.Y.P.; Oberbillig, C.; Geandier, G.; Bonnet, F. Intercritical Annealing of Cold-Rolled Ferrite-Pearlite Steel: Microstructure Evolutions and Phase Transformation Kinetics. Acta Mater. 2021, 212, 116920. [Google Scholar] [CrossRef]
- Pütz, F.; Fehlemann, N.; Göksu, V.; Henrich, M.; Könemann, M.; Münstermann, S. A Data Driven Computational Microstructure Analysis on the Influence of Martensite Banding on Damage in DP-Steels. Comput. Mater. Sci. 2023, 218, 111903. [Google Scholar] [CrossRef]
- Philippot, C.; Bellavoine, M.; Dumont, M.; Hoummada, K.; Drillet, J.; Hebert, V.; Maugis, P. Influence of Heating Rate on Ferrite Recrystallization and Austenite Formation in Cold-Rolled Microalloyed Dual-Phase Steels. Met. Mater. Trans. A Phys. Met. Mater. Sci. 2018, 49, 66–77. [Google Scholar] [CrossRef]
- Chang, Y.; Haase, C.; Szeliga, D.; Madej, L.; Hangen, U.; Pietrzyk, M.; Bleck, W. Compositional Heterogeneity in Multiphase Steels: Characterization and Influence on Local Properties. Mater. Sci. Eng. A 2021, 827, 142078. [Google Scholar] [CrossRef]
- Ennis, B.L. The Effect of Chemical Segregation on Phase Transformations and Mechanical Behaviour in a TRIP-Assisted Dual Phase Steel; The University of Manchester: Manchester, UK, 2017. [Google Scholar]
- Gao, B.; Hu, R.; Pan, Z.; Chen, X.; Liu, Y.; Xiao, L.; Cao, Y.; Li, Y.; Lai, Q.; Zhou, H. Strengthening and Ductilization of Laminate Dual-Phase Steels with High Martensite Content. J. Mater. Sci. Technol. 2021, 65, 29–37. [Google Scholar] [CrossRef]
- Bellavoine, M.; Dumont, M.; Drillet, J.; Hébert, V.; Maugis, P. Combined Effect of Heating Rate and Microalloying Elements on Recrystallization during Annealing of Dual-Phase Steels. Met. Mater. Trans. A Phys. Met. Mater. Sci. 2018, 49, 2865–2875. [Google Scholar] [CrossRef]
- Basu, S.; Patra, A.; Jaya, B.N.; Ganguly, S.; Dutta, M.; Samajdar, I. Study of Microstructure-Property Correlations in Dual Phase Steels for Achieving Enhanced Strength and Reduced Strain Partitioning. Materials 2022, 25, 101522. [Google Scholar] [CrossRef]
- Van Bohemen, S.M.C. Bainite and Martensite Start Temperature Calculated with Exponential Carbon Dependence. Mater. Sci. Technol. 2012, 28, 487–495. [Google Scholar] [CrossRef]
- Boratto, F.; Barbosa, R.; Yue, S.; Jonas, J.J. Effect of Chemical Composition on the Critical Temperatures of Microalloyed Steels. In Proceedings of the International Conference on Physical Metallurgy of Thermomechanical Processing of Steels and Other Metals, Tokyo, Japan, 6–10 June 1988; pp. 383–390. [Google Scholar]
- Bhadeshia, H.K.D.H.; Honeycombe, W.K. Steels: Microstructure and Properties; Elsevier Ltd.: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Lin, S.; Borgenstam, A.; Stark, A.; Hedström, P. Effect of Si on Bainitic Transformation Kinetics in Steels Explained by Carbon Partitioning, Carbide Formation, Dislocation Densities, and Thermodynamic Conditions. Mater. Charact. 2022, 185, 111774. [Google Scholar] [CrossRef]
- Goldstein, J.; Newbury, D.; Michael, J.R.; Ritchie, N.W.M.; Scott, J.H.J.; Joy, D.C. Scanning Electron Microscopy and X-ray Microanalysis, 4th ed.; Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar]
- San Martín, D.; De Cock, T.; García-Junceda, A.; Caballero, F.G.; Capdevila, C.; García De Andrés, C. Effect of Heating Rate on Reaustenitisation of Low Carbon Niobium Microalloyed Steel. Mater. Sci. Technol. 2008, 24, 266–272. [Google Scholar] [CrossRef]
- Azizi-Alizamini, H.; Militzer, M.; Poole, W.J. Austenite Formation in Plain Low-Carbon Steels. Metall. Mater. Trans. A Phys. Metall. Mater. Sci. 2011, 42, 1544–1557. [Google Scholar] [CrossRef]
- De Cock, T.; Capdevila, C.; Caballero, F.G.; De Andrés, C.G. Interpretation of a Dilatometric Anomaly Previous to the Ferrite-to-Austenite Transformation in a Low Carbon Steel. Scr. Mater. 2006, 54, 949–954. [Google Scholar] [CrossRef]
- De Cock, T.; Capdevila, C.; Ferrer, J.P.; Caballero, F.G.; Jimènez, J.A.; García De Andrés, C. Recrystallisation and Dilatometric Behaviour of Low Carbon and Ultralow Carbon Steels. Mater. Sci. Technol. 2008, 24, 832–837. [Google Scholar] [CrossRef]
- Pawłowski, B. Critical Points of Hypoeutectoid Steel—Prediction of Pearlite Dissolution Finich Temperature Ac1f. J. Achiev. Mater. Manuf. Eng. 2011, 49, 331–337. [Google Scholar]
- Cleveland, W.S.; Devlin, S.J. Locally Weighted Regression: An Approach to Regression Analysis by Local Fitting. J. Am. Stat. Assoc. 1988, 83, 596–610. [Google Scholar] [CrossRef]
- Evans, M. An Estimation Method (LOESS) for Dealing with the Imperfections of Existing Parametric Creep Models for Time to Failure: Illustrated Using 2.25Cr–1Mo Steel. Int. J. Press. Vessel. Pip. 2023, 206, 105047. [Google Scholar] [CrossRef]
- Kop, T. A Dilatometric Study of the Austenite/Ferrite Interface Mobility; Delft University of Technology: Delft, The Netherlands, 2000. [Google Scholar]
- Van Bohemen, S.M.C. The Nonlinear Lattice Expansion of Iron Alloys in the Range 100-1600 K. Scr. Mater. 2013, 69, 315–318. [Google Scholar] [CrossRef]
- Mendonça, R.; Nogueira, I.; Lovo, J.; Canale, L. Multiple Etchings Methodology: A New Approach in Multiphase Steel Characterization. J. Microsc. 2020, 277, 93–99. [Google Scholar] [CrossRef]
- Dossett, J.L.; Totten, G.E. (Eds.) ASM Handbook; ASM International: Materials Park, OH, USA, 1986; Volume 4, ISBN 0-87170-007-7 (v. 1). [Google Scholar]
- Xu, Z.; Ding, Z.; Liang, B.; Li, H. The Analysis for Morphological Evolution and Crystallography of Degenerate Pearlite in 100Mn13 Steel. Materwiss Werksttech 2020, 51, 1251–1257. [Google Scholar] [CrossRef]
- Al Gahtani, M. Formation of Micro-Structural Banding in Hot-Rolled Medium-Carbon Steel. Ph.D. Thesis, University of Wollongong, Wollongong, Australia, 2015; p. 214. [Google Scholar]
- Li, Z.; Miyamoto, G.; Yang, Z.; Zhang, Y.; Zhang, C.; Furuhara, T. Effects of Mn and Si Additions on Pearlite-Austenite Phase Transformation in Fe-0.6C Steel. Acta Metall. Sin. 2010, 46, 1066–1074. [Google Scholar] [CrossRef]
- Shah, V.; Krugla, M.; Offerman, S.E.; Sietsma, J.; Hanlon, D.N. Effect of Silicon, Manganese and Heating Rate on the Ferrite Recrystallization Kinetics. ISIJ Int. 2020, 60, 1312–1323. [Google Scholar] [CrossRef]
- Fridberg, J.; Torndahl, L.E.; Hillert, M. Diffusion in Iron. Jernkontorets Ann. 1969, 153, 263–276. [Google Scholar]
- Guo, H.; Enomoto, M. Effects of Substitutional Solute Accumulation at α/γ Boundaries on the Growth of Ferrite in Low Carbon Steels. Met. Mater. Trans. A Phys. Met. Mater. Sci. 2007, 38, 1152–1161. [Google Scholar] [CrossRef]
- Purdy, G.R.; Brechet, Y.J.M. A Solute Drag Treatment of the Effects of Alloying Elements on the Rate of the Proeutectoid Ferrite Transformation in Steels. Acta Metall. Et Mater. 1995, 43, 3763–3774. [Google Scholar] [CrossRef]
- Landheer, H. Nucleation of Ferrite in Austenite The Role of Crystallography. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 2010. ISBN 9789088912078. [Google Scholar]
- Offerman, S.E.; Van Dijk, N.H.; Sietsma, J.; Grigull, S.; Lauridsen, E.M.; Margulies, L.; Poulsen, H.F.; Rekveldt, M.T.; Van der Zwaag, S. Grain Nucleation and Growth during Phase Transformations. Science 2002, 298, 1003–1005. [Google Scholar] [CrossRef] [PubMed]
- Sharma, H.; Sietsma, J.; Offerman, S.E. Preferential Nucleation during Polymorphic Transformations. Sci. Rep. 2016, 6, 30860. [Google Scholar] [CrossRef]
- Offerman, S.E.; Van Dijk, N.H.; Sietsma, J.; Van Der Zwaag, S.; Lauridsen, E.M.; Margulies, L.; Grigull, S.; Poulsen, H.F. Reply to the Discussion by Aaronson et al. to “Grain Nucleation and Growth during Phase Transformations” by S.E. Offerman et al., Science, 298, 1003 (November 1, 2002). Scr. Mater. 2004, 51, 937–941. [Google Scholar] [CrossRef]
- Palmer, T.A.; Elmer, J.W. Direct Observations of the Formation and Growth of Austenite from Pearlite and Allotriomorphic Ferrite in a C–Mn Steel Arc Weld. Scr. Mater. 2005, 53, 535–540. [Google Scholar] [CrossRef]
- Li, P.; Li, J.; Meng, Q.; Hu, W.; Xu, D. Effect of Heating Rate on Ferrite Recrystallization and Austenite Formation of Cold-Roll Dual Phase Steel. J. Alloys Compd. 2013, 578, 320–327. [Google Scholar] [CrossRef]
- Huang, J.; Poole, W.J.; Militzer, M. Austenite Formation during Intercritical Annealing. Met. Mater. Trans. A Phys. Met. Mater. Sci. 2004, 35 A, 3363–3375. [Google Scholar] [CrossRef]
- Enomoto, M.; NojirF, N.; Sato1, Y. Effects of Vanadium and Niobium on the Nucleation Kinetics of Proeutectoid Ferrite at Austenite Grain Boundaries in Fe-C and Fe-C-Mn Alloys. Mater. Trans. JIM 1994, 35, 859–867. [Google Scholar] [CrossRef]
- Pereloma, E.; Edmonds, D. Phase Transformations in Steels; Elsevier Ltd.: Amsterdam, The Netherlands, 2012; Volume 2, ISBN 9781845699710. [Google Scholar]
- Aaronson, H.I.; Enomoto, M.; Lee, J.K. Diffusional Nucleation in Solid–Solid Transformations. In Mechanisms of Diffusional Phase Transformations in Metals and Alloys; CRC Press: Boca Raton, FL, USA, 2010; p. 200. [Google Scholar]
- Embury, D. The Formation of Pearlite in Steels. In Phase Transformations in Steels; Pereloma, E., Edmonds, D.V., Eds.; Woodhead Publishing: Sawston, UK, 2012; pp. 276–310. ISBN 978-1-84569-970-3. [Google Scholar]
- Lâszl, F. Tessellated Stresses—Part V. J. Iron Steel Inst. 1943, 1, 173. [Google Scholar]
- Barnett, D.M.; Lee, J.K.; Aaronson, H.I.; Russell, K.C. The strain energy of a coherent ellipsoidal precipitate. Scr. Metall. 1974, 8, 1447–1450. [Google Scholar] [CrossRef]
- Ghosh, G.; Olson, G.B. The Isotropic Shear Modulus of Multicomponent Fe-Base Solid Solutions. Acta Mater. 2002, 50, 2655–2675. [Google Scholar] [CrossRef]
- Lindgren, L.E.; Gyhlesten Back, J. Elastic Properties of Ferrite and Austenite in Low Alloy Steels versus Temperature and Alloying. Materials 2019, 5, 100193. [Google Scholar] [CrossRef]
- Onink, M.; Brakman, C.M.; Tichelaar, F.D.; Mittemeijer, E.J.; van der Zwaag, S.; Root, J.H.; Konyer, N.B. The Lattice Parameters of Austenite and Ferrite in FeC Alloys as Functions of Carbon Concentration and Temperature. Scr. Metall. Mater. 1993, 29, 1011–1016. [Google Scholar] [CrossRef]
- Fruchart, D.; Chaudouet, P.; Fruchart, R.; Rouault, A.; Senateur, J.P. Etudes Structurales de Composés de Type Cémentite: Effet de l’hydrogéne Sur Fe3C Suivi Par Diffraction Neutronique. Spectrométrie Mössbauer Sur FeCo2B et Co3B Dopés Au 57Fe. J. Solid State Chem. 1984, 51, 246–252. [Google Scholar] [CrossRef]
- Umemoto, M.; Liu, Z.G.; Masuyama, K.; Tsuchiya, K. Influence of Alloy Additions on Production and Properties of Bulk Cementite. Scr. Mater. 2001, 45, 391–397. [Google Scholar] [CrossRef]
- Tanino, M. Precipitation of carbides in steel. Tetsu-to-Hagane/J. Iron Steel Inst. Jpn. 1967, 6, 23–37. [Google Scholar]
- Tanino, M. Crystal structure and precipitation characteristics of carbides in steel. Tetsu-to-Hagane/J. Iron Steel Inst. Jpn. 1972, 11, 203–220. [Google Scholar]
- Razumovskiy, V.I.; Ghosh, G. A First-Principles Study of Cementite (Fe3C) and Its Alloyed Counterparts: Structural Properties, Stability, and Electronic Structure. Comput. Mater. Sci. 2015, 110, 169–181. [Google Scholar] [CrossRef]
- Liang, X.; Hou, T.P.; Zhang, D.; Zheng, P.; Lin, H.F.; Li, Y.; Wu, K.M. Structural, Electronic, Magnetic and Mechanical Properties of Fe2SiC. Phys. B Condens Matter 2021, 618, 413136. [Google Scholar] [CrossRef]
- Garvik, N.; Carrez, P.; Cordier, P. First-Principles Study of the Ideal Strength of Fe3C Cementite. Mater. Sci. Eng. A 2013, 572, 25–29. [Google Scholar] [CrossRef]
- Jang, J.H.; Kim, I.G.; Bhadeshia, H.K.D.H. Substitutional Solution of Silicon in Cementite: A First-Principles Study. Comput. Mater. Sci. 2009, 44, 1319–1326. [Google Scholar] [CrossRef]
- Ghosh, G. A First-Principles Study of Cementite (Fe3C) and Its Alloyed Counterparts: Elastic Constants, Elastic Anisotropies, and Isotropic Elastic Moduli. AIP Adv. 2015, 5, 087102. [Google Scholar] [CrossRef]
- Kim, B.; Celada, C.; San Martín, D.; Sourmail, T.; Rivera-Díaz-Del-Castillo, P.E.J. The Effect of Silicon on the Nanoprecipitation of Cementite. Acta Mater. 2013, 61, 6983–6992. [Google Scholar] [CrossRef]
- Chang, L.; Smith, G.D.W. The Silicon Effect in the Tempering of Martensite in Steels. Le J. De Phys. Colloq. 1984, 45, C9-397–C9-401. [Google Scholar] [CrossRef]
- Ande, C.K. First-Principles Calculations on the Stabilization of Iron Carbides (Fe3C, Fe5C2 and η-Fe2C) in Steels; Technical University Delft: Delft, The Netherlands, 2013. [Google Scholar]
- Drumond, J.; Girina, O.; da Silva Filho, J.F.; Fonstein, N.; de Oliveira, C.A.S. Effect of Silicon Content on the Microstructure and Mechanical Properties of Dual-Phase Steels. Metallogr. Microstruct. Anal. 2012, 1, 217–223. [Google Scholar] [CrossRef]
- Davies, R.G. Influence of Silicon and Phosphorous on the Mechanical Properties of Both Ferrite and Dual-Phase Steels. Metall. Trans. A 1979, 10, 113–118. [Google Scholar] [CrossRef]
- Krauss, G. Solidification, Segregation, and Banding in Carbon and Alloy Steels. Metall. Mater. Trans. B Process Metall. Mater. Process. Sci. 2003, 34, 781–792. [Google Scholar] [CrossRef]
- Deming, W.E. Statistical Adjustment of Data (Dover Books on Mathematics); Dover Publications: Mineola, NY, USA, 2011; ISBN 978-0486646855. [Google Scholar]
- Kiefer, D.; Gibmeier, J.; Stark, A. Determination of Temperature-Dependent Elastic Constants of Steel AISI 4140 by Use of in Situ x-Ray Dilatometry Experiments. Materials 2020, 13, 2378. [Google Scholar] [CrossRef] [PubMed]
- Hutchinson, C.R.; Malmstrom, M.; Lonnqvist, J.; Bate, P.; Ehteshami, H.; Korzhavyi, P.A. Elasticity and Wave Velocity in FCC Iron (Austenite) at Elevated Temperatures—Experimental Verification of Ab-Initio Calculations. Ultrasonics 2018, 87, 44–47. [Google Scholar] [CrossRef] [PubMed]
- Adams, J.J.; Agosta, D.S.; Leisure, R.G.; Ledbetter, H. Elastic Constants of Monocrystal Iron from 3 to 500 K. J. Appl. Phys. 2006, 100, 113530. [Google Scholar] [CrossRef]
- Dever, D.J. Temperature Dependence of the Elastic Constants in α-Iron Single Crystals: Relationship to Spin Order and Diffusion Anomalies. J. Appl. Phys. 1972, 43, 3293–3301. [Google Scholar] [CrossRef]
- Drouin, D.; Couture, A.R.; Joly, D.; Tastet, X.; Aimez, V.; Gauvin, R. CASINO V2.42—A Fast and Easy-to-Use Modeling Tool for Scanning Electron Microscopy and Microanalysis Users. Scanning 2007, 29, 92–101. [Google Scholar] [CrossRef]
- Lin, S.; Ledbetter, H. Nitrogen Effect on Elastic Constants of f.c.c. Fe-18Cr-19Mn Alloys. Mater. Sci. Eng. A 1993, 167, 81–85. [Google Scholar] [CrossRef]
- Umemoto, M.; Kruger, S.E.; Ohtsuka, H. Ultrasonic Study on the Change in Elastic Properties of Cementite with Temperature and Mn Content Using Nearly Full Density Polycrystalline Bulk Samples. Mater. Sci. Eng. A 2019, 742, 162–168. [Google Scholar] [CrossRef]
- Hartmann, S.; Ruppersberg, H. Thermal Expansion of Cementite and Thermoelastic Stresses in White Cast Iron. Mater. Sci. Eng. A 1995, 190, 231–239. [Google Scholar] [CrossRef]
- Lee, K.M.; Lee, H.C.; Lee, J.K. Influence of Coherency Strain and Applied Stress upon Diffusional Ferrite Nucleation in Austenite: Micromechanics Approach. Philos. Mag. 2010, 90, 437–459. [Google Scholar] [CrossRef]
- Nabarro, F.R.N. The Influence of Elastic Strain on the Shape of Particles Segregating in Alloy. Proc. Phys. Soc. 1940, 175, 519–538. [Google Scholar] [CrossRef]
- Böhm, H.J.; Zickler, G.A.; Fischer, F.D.; Svoboda, J. Role of Elastic Strain Energy in Spheroidal Precipitates Revisited. Mech. Mater. 2021, 155, 103781. [Google Scholar] [CrossRef]
- Böhm, H.J.; Zickler, G.A.; Fischer, F.D.; Svoboda, J. Strain and Interface Energy of Ellipsoidal Inclusions Subjected to Volumetric Eigenstrains: Shape Factors. Arch. Appl. Mech. 2022, 92, 405–411. [Google Scholar] [CrossRef]
- Eshelby, J.D. The Determination of the Elastic Field of an Ellipsoidal Inclusion, and Related Problems. Proc. R. Soc. Lond. A Math. Phys. Sci. 1957, 241, 376–396. [Google Scholar]

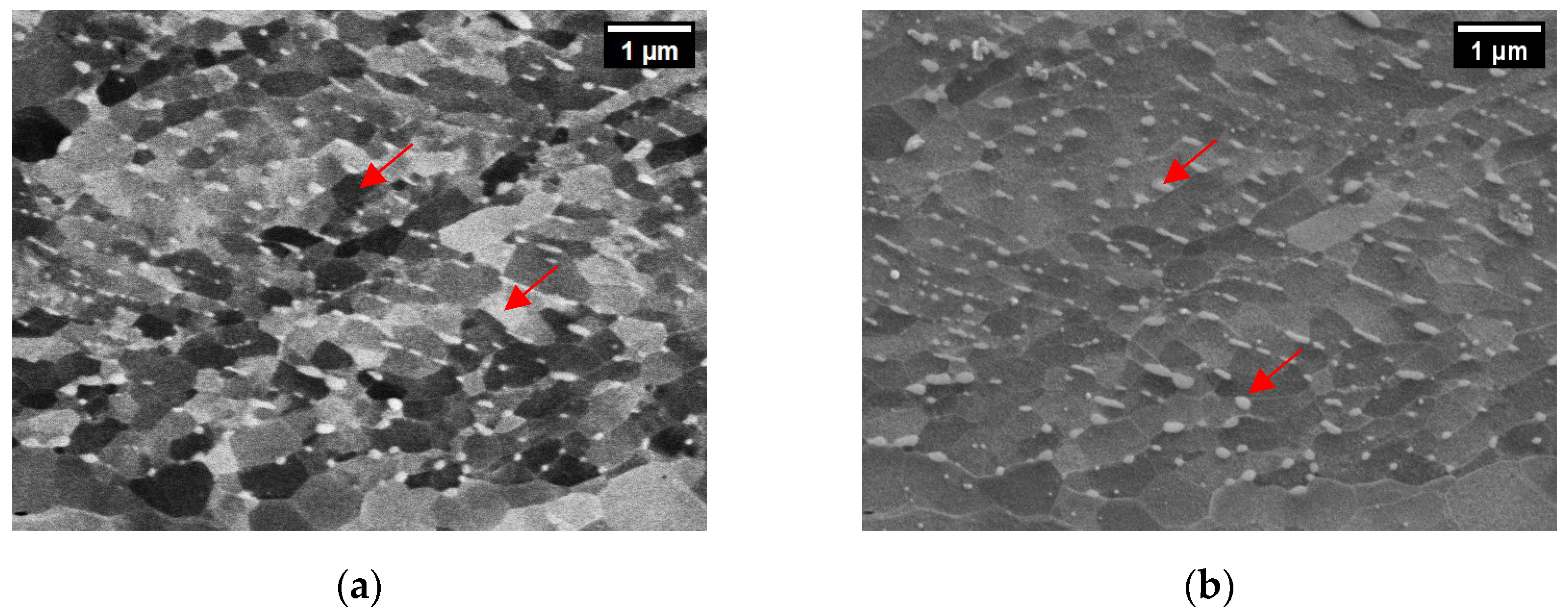


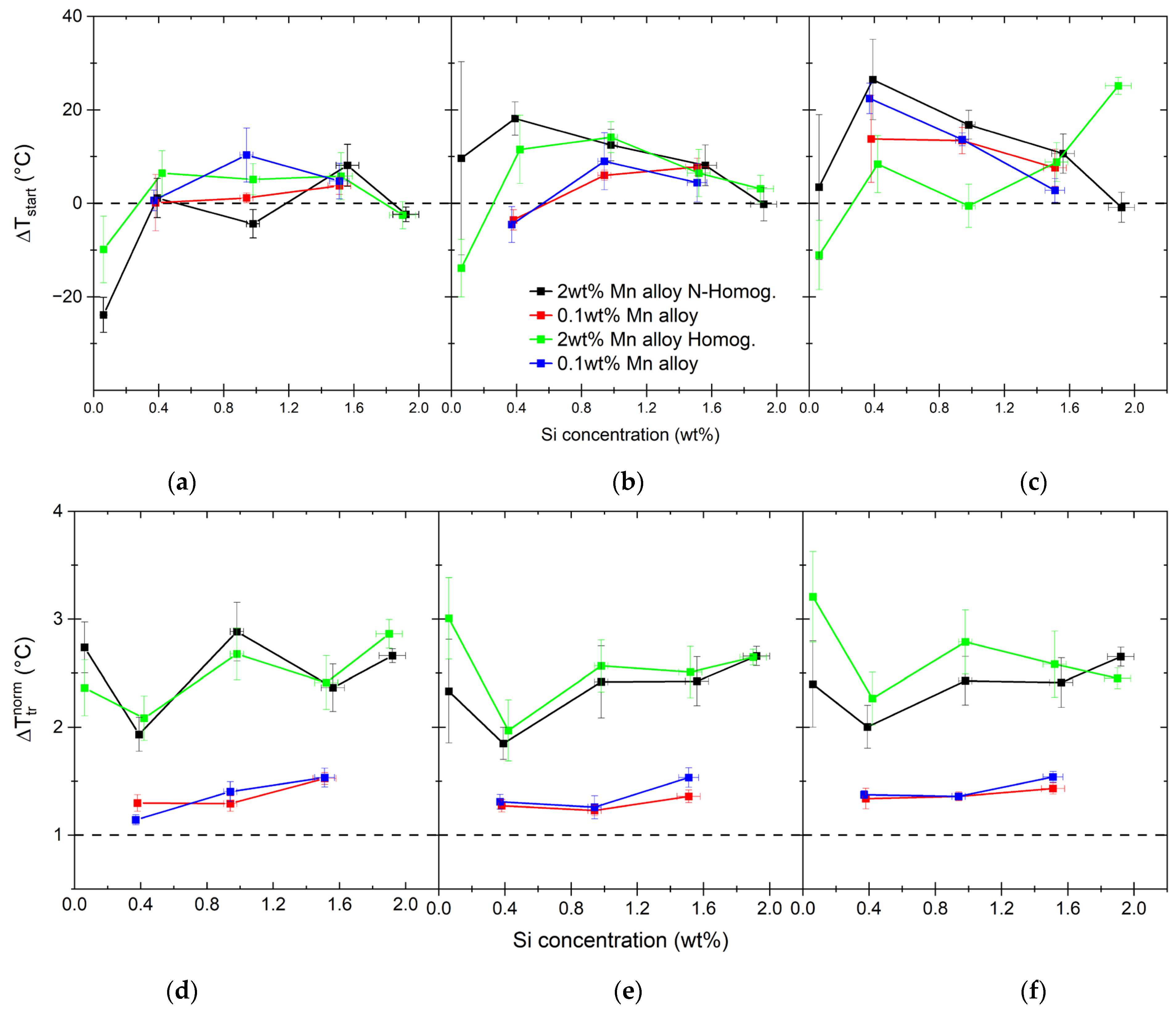
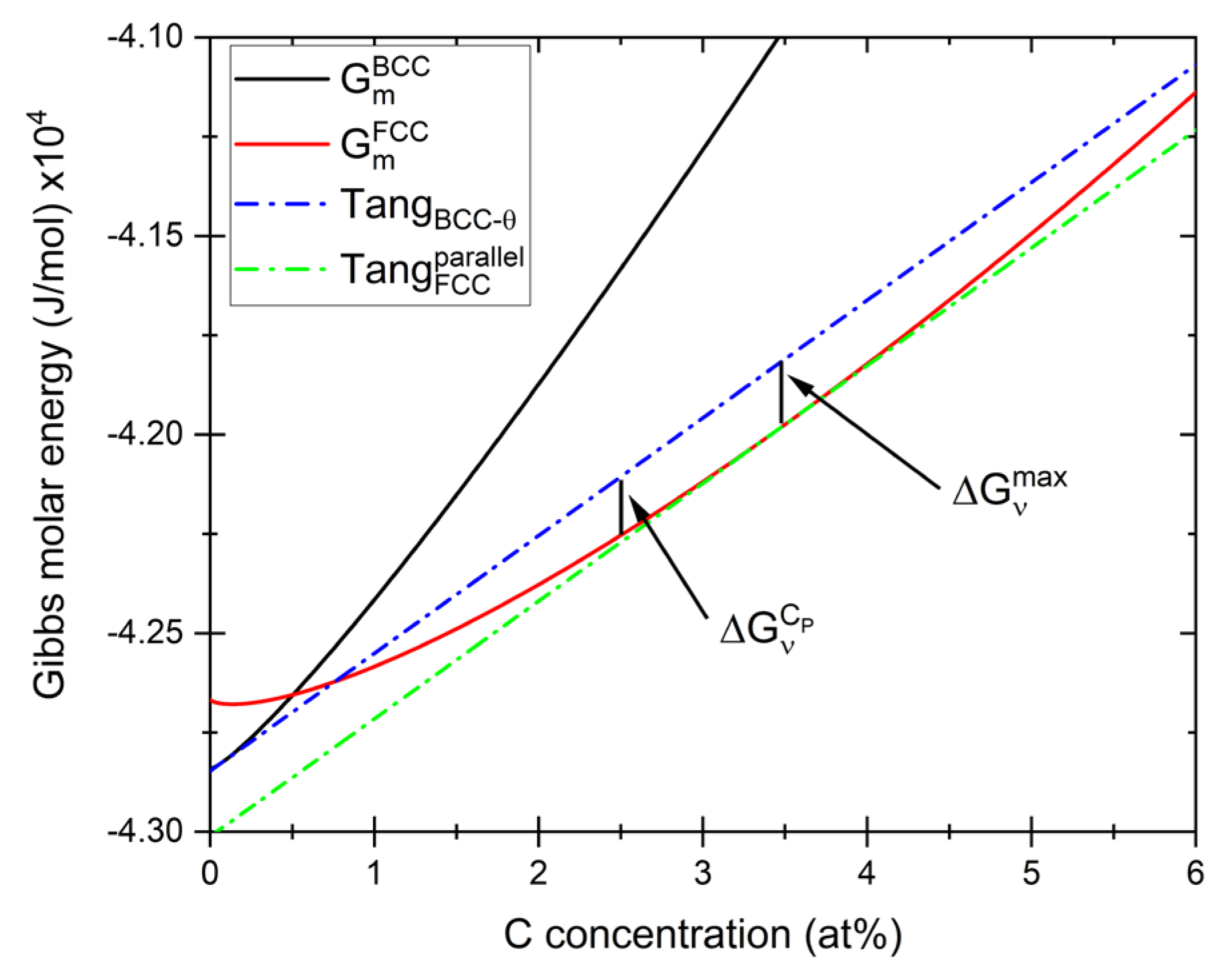

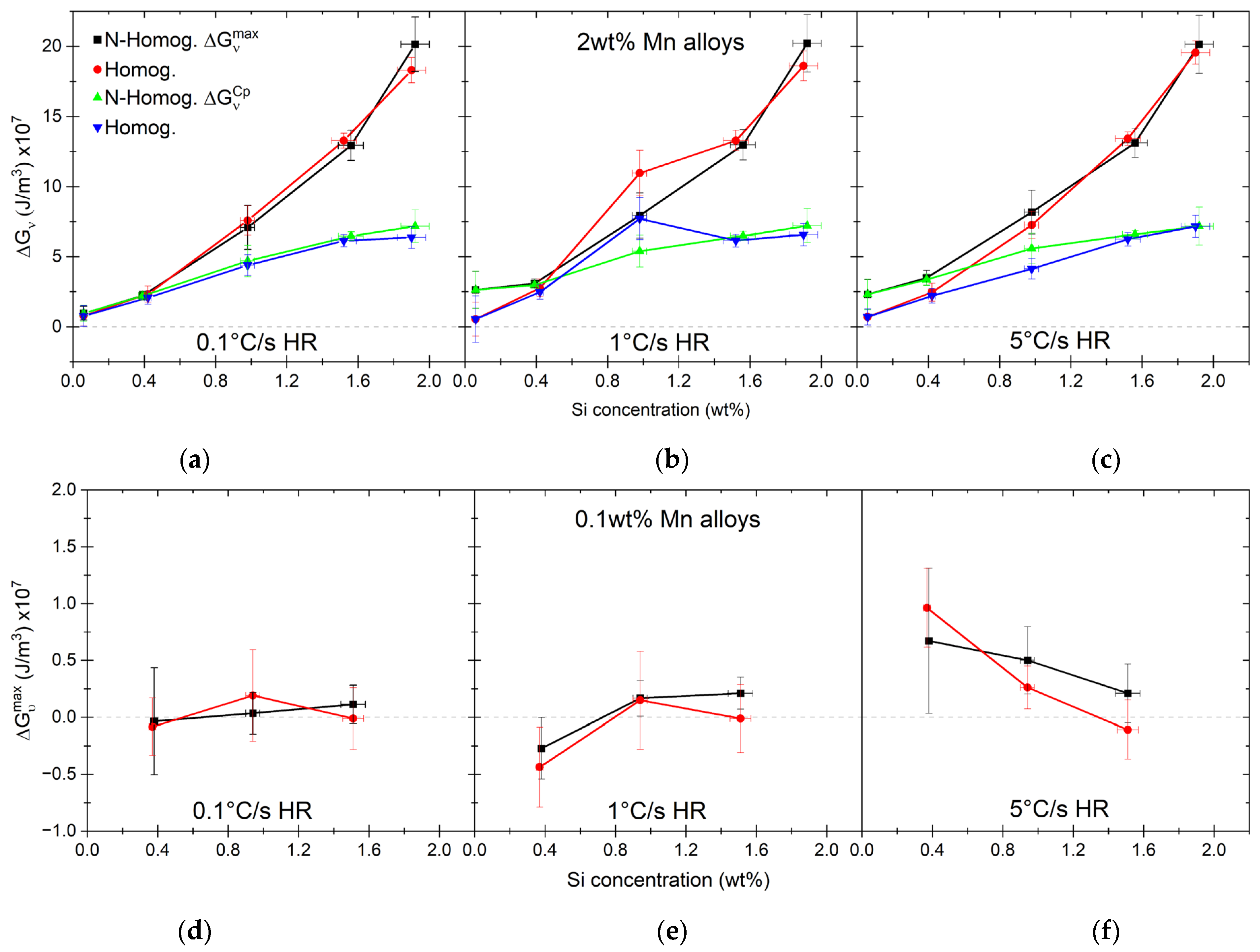

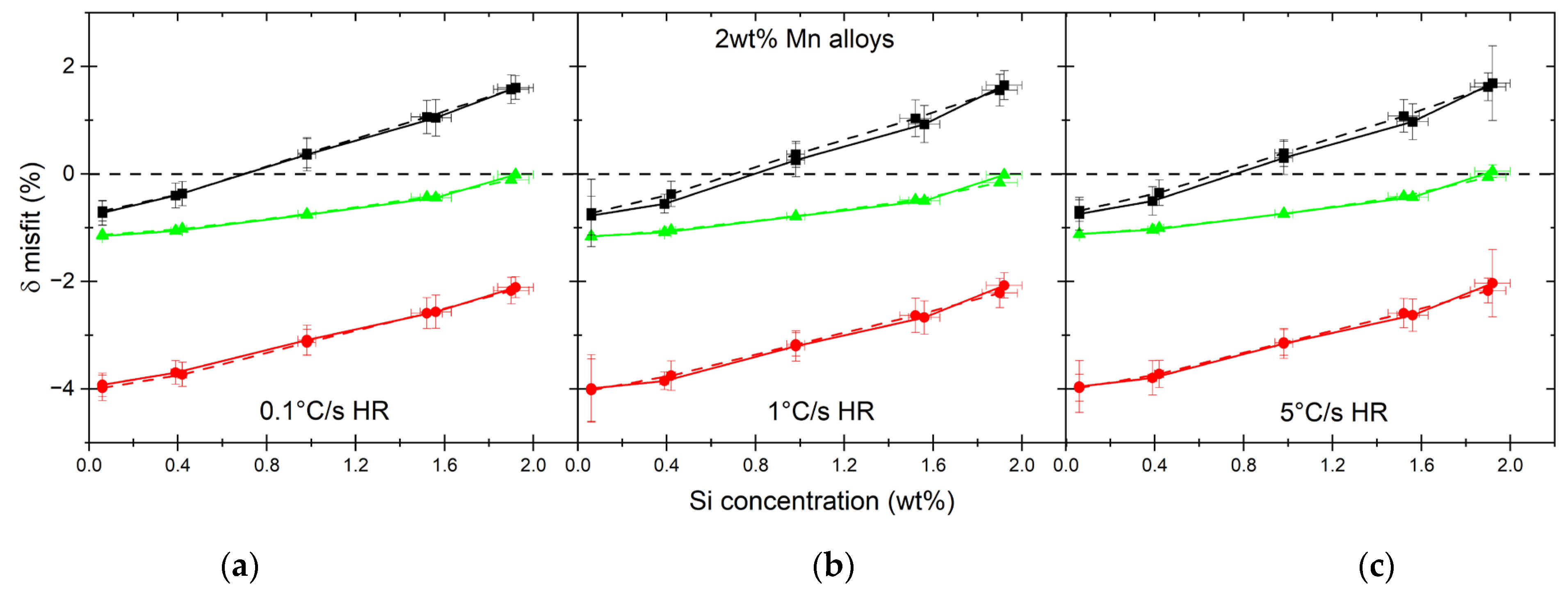

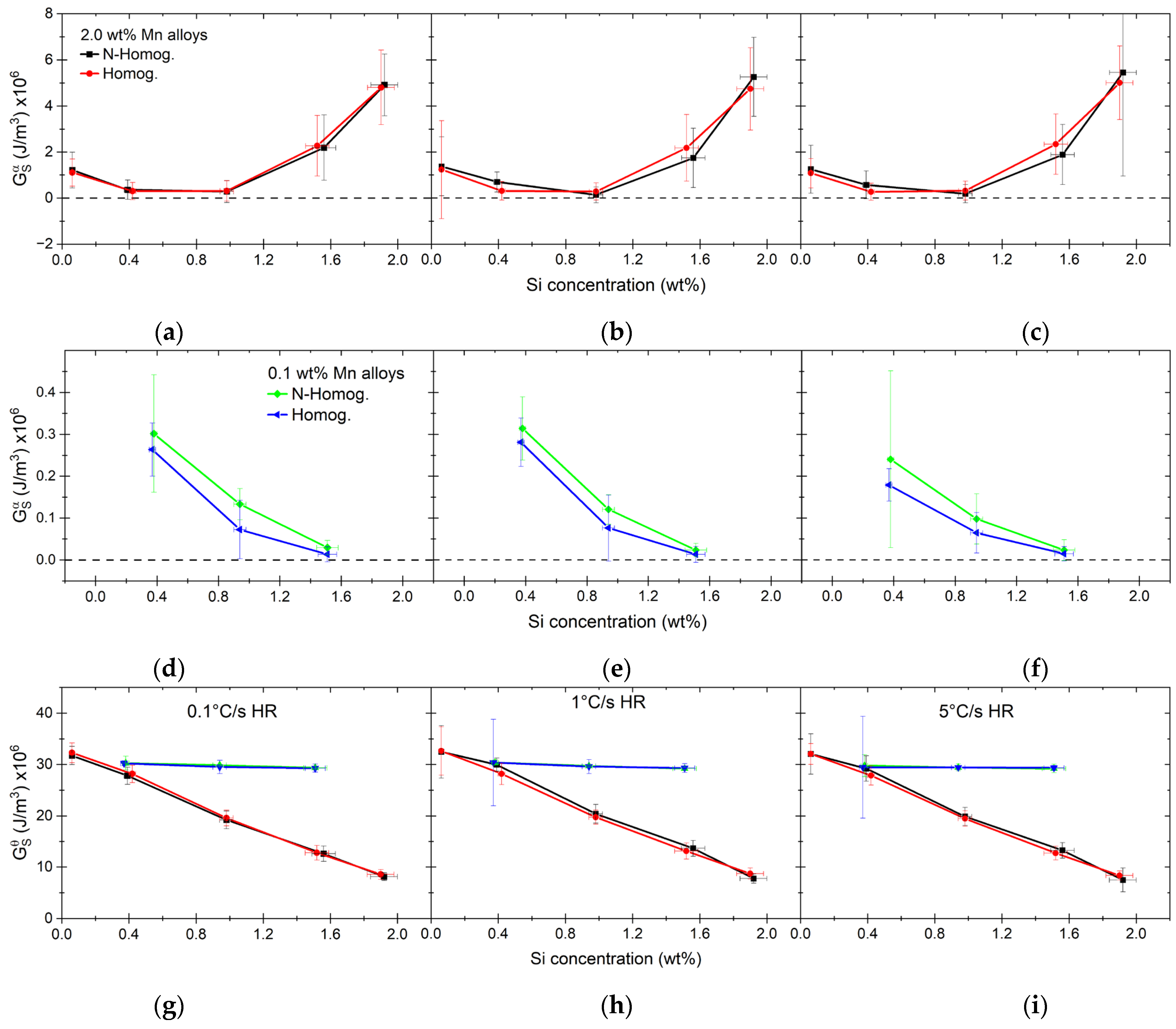


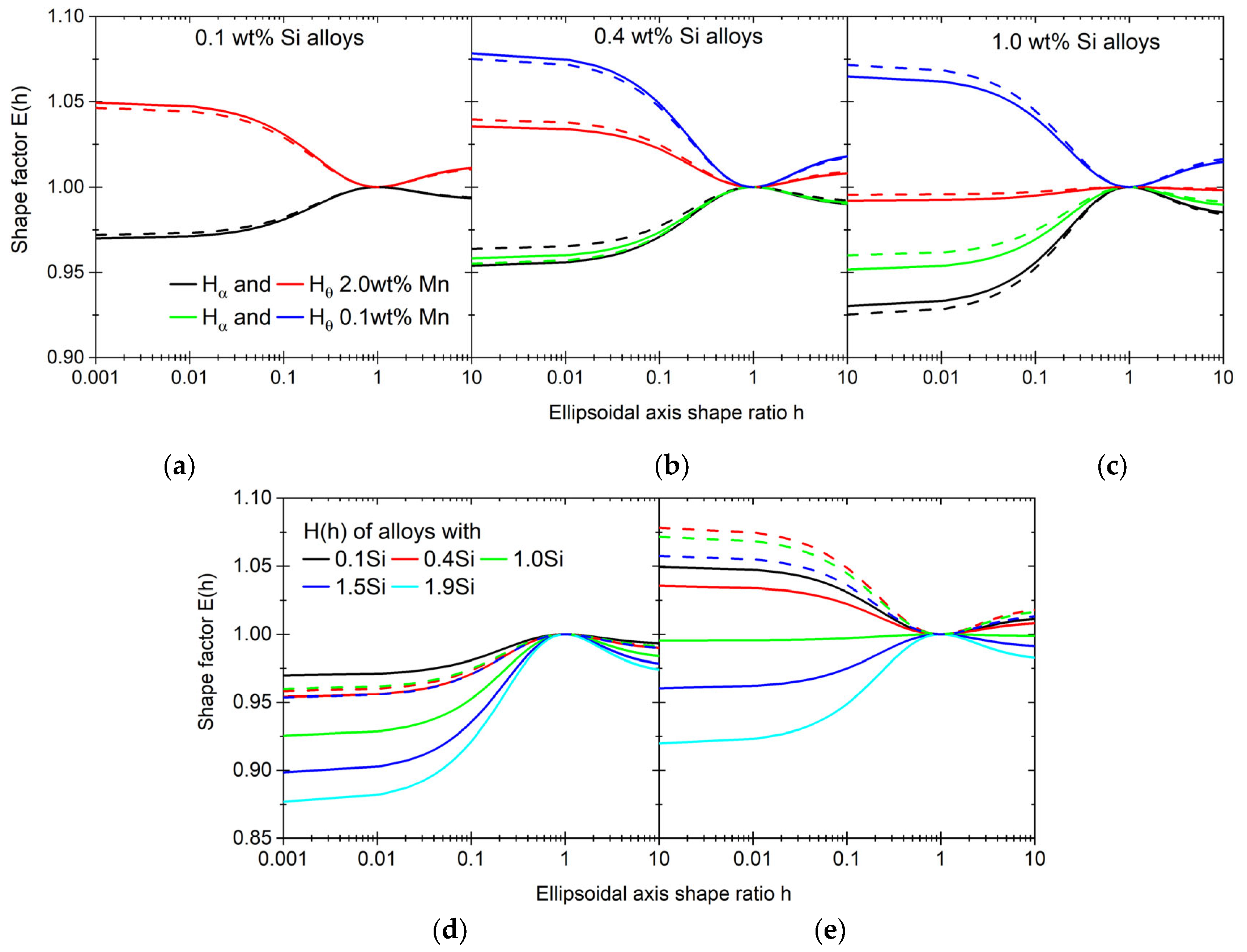
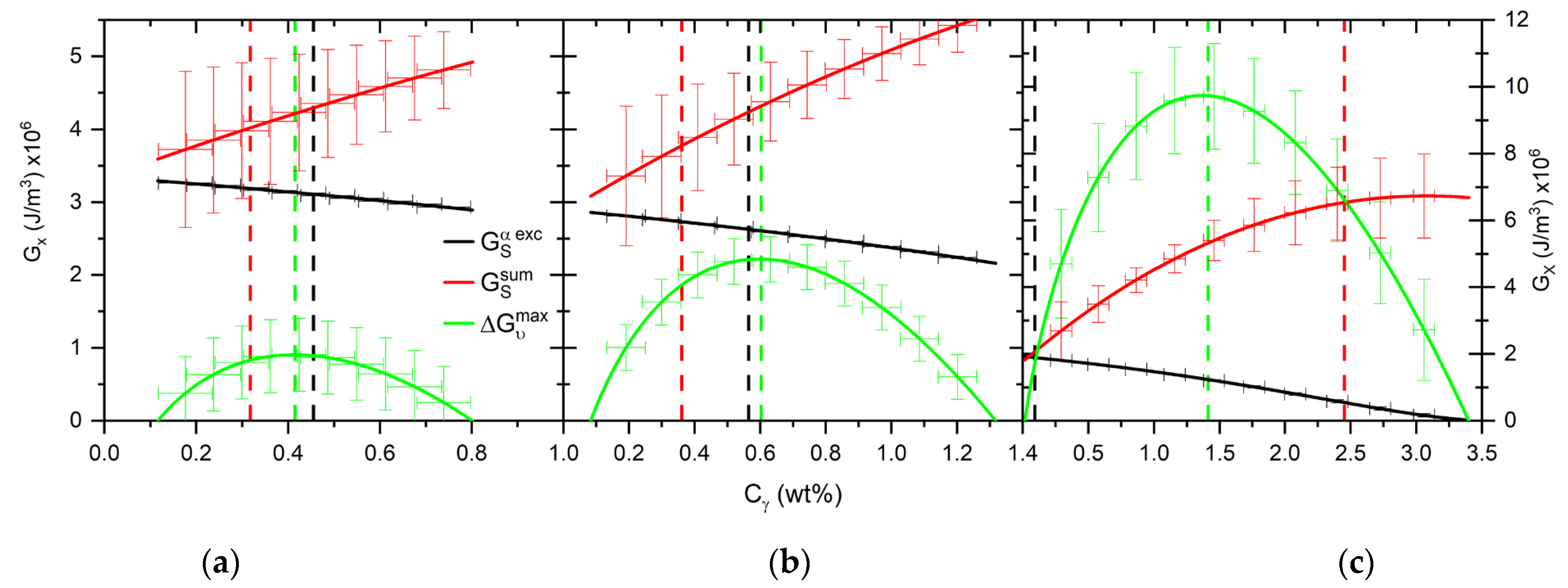
| Materials | C | Mn | Si | Ttop | |
|---|---|---|---|---|---|
| wt% | wt% | wt% | Nonhomog. | Homog. | |
| 0.1Si2Mn | 0.19 ± 0.01 | 1.95 ± 0.08 | 0.06 ± 0.01 | 900 °C | 900 °C |
| 0.4Si2Mn | 0.20 ± 0.01 | 1.96 ± 0.08 | 0.39 ± 0.02 | 900 °C | 900 °C |
| 1.0Si2Mn | 0.20 ± 0.01 | 2.03 ± 0.08 | 0.98 ± 0.04 | 950 °C | 950 °C |
| 1.5Si2Mn | 0.20 ± 0.01 | 2.01 ± 0.08 | 1.56 ± 0.07 | 950/1000 °C | 1000 °C |
| 1.9Si2Mn | 0.19 ± 0.01 | 2.00 ± 0.08 | 1.92 ± 0.08 | 1050 °C | 1000 °C |
| 0.4Si0.1Mn | 0.20 ± 0.01 | 0.08 ± 0.01 | 0.38 ± 0.02 | 950 °C | 950 °C |
| 1.0Si0.1Mn | 0.20 ± 0.01 | 0.09 ± 0.01 | 0.94 ± 0.04 | 1000 °C | 1000 °C |
| 1.5Si0.1Mn | 0.20 ± 0.01 | 0.09 ± 0.01 | 1.51 ± 0.07 | 1050 °C | 1050 °C |
| Alloy | VP | CP | VP | CP | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| vol% | SD | wt% | SD | vol% | SD | wt% | SD | |||
| 0.1Si2Mn | Nonhomogenized | 42 | 1 | 0.46 | 0.03 | Homogenized | 43 | 3 | 0.51 | 0.04 |
| 0.4Si2Mn | 34 | 6 | 0.6 | 0.1 | 40 | 2 | 0.53 | 0.04 | ||
| 1.0Si2Mn | 38 | 5 | 0.52 | 0.08 | 34 | 2 | 0.58 | 0.05 | ||
| 1.5Si2Mn | 37 | 4 | 0.54 | 0.06 | 34 | 6 | 0.6 | 0.1 | ||
| 1.9Si2Mn | 38 | 4 | 0.50 | 0.06 | 35 | 5 | 0.55 | 0.08 | ||
| 0.4Si0.1Mn | 15 | 4 | 1.4 | 0.4 | 14 | 2 | 1.5 | 0.2 | ||
| 1.0Si0.1Mn | 17 | 5 | 1.2 | 0.4 | 18 | 5 | 1.1 | 0.4 | ||
| 1.5Si0.1Mn | 17 | 5 | 1.2 | 0.3 | 17 | 3 | 1.2 | 0.3 | ||
| Alloy | Element | Avg | SD | SE | LA | HA | Δ | LA | HA | Δ |
|---|---|---|---|---|---|---|---|---|---|---|
| ¼ Thickness | ½ Thickness | |||||||||
| 0.1Si2Mn | Si | - | - | - | - | - | - | - | - | - |
| Mn | 2.1 | 0.3 | 4 × 10−4 | 1.5 | 2.6 | 1.1 | 1.6 | 3.1 | 1.5 | |
| 0.4Si2Mn | Si | 0.4 | 0.1 | 9 × 10−5 | 0.28 | 0.48 | 0.20 | 0.30 | 0.50 | 0.20 |
| Mn | 2.0 | 0.3 | 4 × 10−4 | 1.4 | 2.5 | 1.1 | 1.4 | 2.6 | 1.2 | |
| 1.0Si2Mn | Si | 1.0 | 0.1 | 1 × 10−4 | 0.72 | 1.19 | 0.47 | 0.85 | 1.20 | 0.35 |
| Mn | 2.0 | 0.4 | 7 × 10−4 | 1.5 | 2.5 | 1.0 | 1.4 | 2.7 | 1.3 | |
| 1.5Si2Mn | Si | 1.5 | 0.1 | 2 × 10−4 | 1.32 | 1.86 | 0.54 | 1.34 | 1.77 | 0.43 |
| Mn | 2.0 | 0.3 | 3 × 10−4 | 1.6 | 2.6 | 1.0 | 1.5 | 2.7 | 1.2 | |
| 1.9Si2Mn | Si | 1.8 | 0.1 | 2 × 10−4 | 1.68 | 2.12 | 0.44 | 1.68 | 2.44 | 0.76 |
| Mn | 2.1 | 0.5 | 8 × 10−4 | 1.6 | 2.7 | 1.1 | 1.6 | 3.1 | 1.5 | |
| 0.4Si0.1Mn | Si | 0.4 | 0.1 | 1 × 10−4 | 0.28 | 0.49 | 0.21 | 0.26 | 0.46 | 0.20 |
| Mn | - | - | - | - | - | - | - | - | - | |
| 1.0Si0.1Mn | Si | 0.9 | 0.1 | 2 × 10−4 | 0.84 | 1.11 | 0.27 | 0.77 | 1.13 | 0.36 |
| Mn | - | - | - | - | - | - | - | - | - | |
| 1.5Si0.1Mn | Si | 1.5 | 0.1 | 2 × 10−4 | 1.40 | 1.65 | 0.25 | 1.27 | 1.73 | 0.46 |
| Mn | - | - | - | - | - | - | - | - | - | |
| Alloy | Avg | SD | SE |
|---|---|---|---|
| μm | μm | μm | |
| 0.1Si2Mn | 4.7 | 1.7 | 0.06 |
| 0.4Si2Mn | 4.7 | 1.7 | 0.06 |
| 1.0Si2Mn | 4.8 | 1.8 | 0.05 |
| 1.5Si2Mn | 4.9 | 1.7 | 0.05 |
| 0.4Si0.1Mn | 5.2 | 2.1 | 0.06 |
| 1.0Si0.1Mn | 4.7 | 2.1 | 0.06 |
| 1.5Si0.1Mn | 5.2 | 2.0 | 0.06 |
| Alloy | θ | αP | kα/θ | α | |||||
|---|---|---|---|---|---|---|---|---|---|
| Mn | Si | Mn | Si | Mn | Si | Mn | Si | ||
| 1.9Si2Mn | Homog. | 6.55 | 1.32 | 1.87 | 1.92 | 0.29 | 1.45 | 1.93 | 1.91 |
| Nonhomog. | 6.33 | 1.45 | 2.25 | 2.10 | 0.36 | 1.45 | 2.22 | 1.94 | |
| 1.5Si0.1Mn | Homog. | 0.40 | 0.17 | - | 1.45 | - | - | - | 1.46 |
| Nonhomog. | 0.47 | 0.21 | - | 1.02 | - | - | - | 1.63 | |
| Alloy | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| T | Mn | Si | 0.1 °C/s | 1 °C/s | 5 °C/s | T | Mn | Si | 0.1 °C/s | 1 °C/s | 5 °C/s | |
| °C | wt% | wt% | °C | °C | °C | °C | wt% | wt% | °C | °C | °C | |
| Nonhomogenized | Homogenized | |||||||||||
| 0.1Si2Mn | 705 | 2.8 | 0.1 | 688 | 722 | 715 | 678 | 2.0 | 0.1 | 702 | 698 | 701 |
| 0.4Si2Mn | 697 | 2.6 | 0.3 | 702 | 719 | 727 | 657 | 2.0 | 0.4 | 707 | 712 | 709 |
| 1.0Si2Mn | 711 | 2.6 | 1.0 | 714 | 730 | 735 | 604 | 2.0 | 1.0 | 723 | 732 | 717 |
| 1.5Si2Mn | 709 | 2.5 | 1.5 | 730 | 730 | 733 | 549 | 2.0 | 1.5 | 728 | 728 | 731 |
| 1.9Si2Mn | 721 | 2.7 | 2.1 | 729 | 731 | 730 | 497 | 2.0 | 1.9 | 728 | 734 | 756 |
| 0.4Si0.1Mn | 730 | 0.1 | 0.3 | 730 | 726 | 744 | 694 | 0.1 | 0.4 | 731 | 725 | 752 |
| 1.0Si0.1Mn | 737 | 0.1 | 0.7 | 740 | 744 | 752 | 638 | 0.1 | 1.0 | 749 | 747 | 752 |
| 1.5Si0.1Mn | 749 | 0.1 | 1.3 | 752 | 756 | 756 | 580 | 0.1 | 1.5 | 753 | 753 | 751 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Krugla, M.; Offerman, S.E.; Sietsma, J.; Hanlon, D.N. Microsegregation Influence on Austenite Formation from Ferrite and Cementite in Fe–C–Mn–Si and Fe–C–Si Steels. Metals 2024, 14, 92. https://doi.org/10.3390/met14010092
Krugla M, Offerman SE, Sietsma J, Hanlon DN. Microsegregation Influence on Austenite Formation from Ferrite and Cementite in Fe–C–Mn–Si and Fe–C–Si Steels. Metals. 2024; 14(1):92. https://doi.org/10.3390/met14010092
Chicago/Turabian StyleKrugla, Monika, S. Erik Offerman, Jilt Sietsma, and Dave N. Hanlon. 2024. "Microsegregation Influence on Austenite Formation from Ferrite and Cementite in Fe–C–Mn–Si and Fe–C–Si Steels" Metals 14, no. 1: 92. https://doi.org/10.3390/met14010092
APA StyleKrugla, M., Offerman, S. E., Sietsma, J., & Hanlon, D. N. (2024). Microsegregation Influence on Austenite Formation from Ferrite and Cementite in Fe–C–Mn–Si and Fe–C–Si Steels. Metals, 14(1), 92. https://doi.org/10.3390/met14010092







