Experimental Investigation on Uniaxial Compressive Strength of Thin Building Sandstone
Abstract
:1. Introduction
2. Specimens and Methodology
2.1. Sandstone Specimens
2.2. Test Method
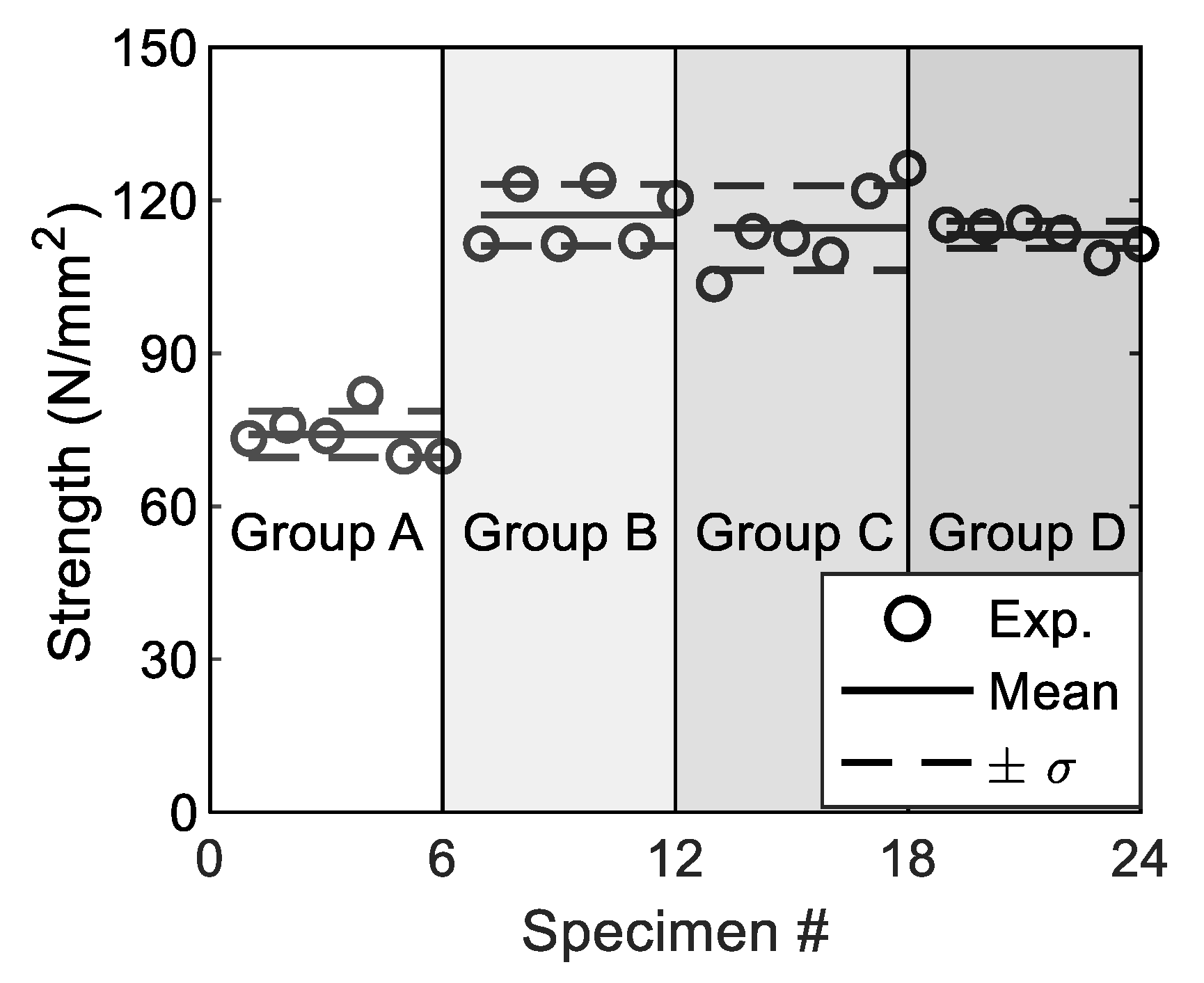
3. Results and Discussion
3.1. Failure Mode

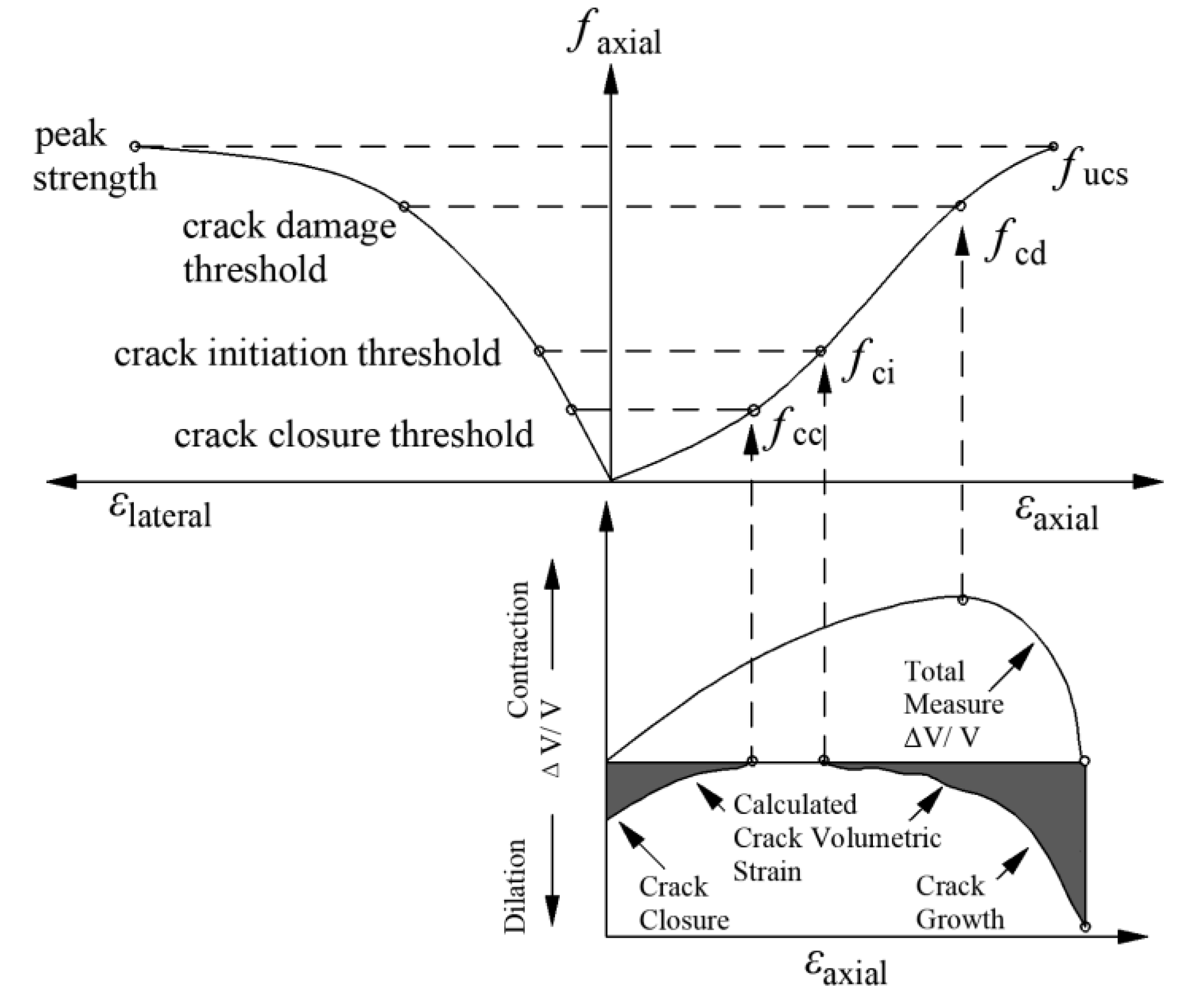
3.2. Stress–Strain Diagram
3.2.1. Experimental Diagram
3.2.2. Evolution of Diagram
3.2.3. Diagram Regression
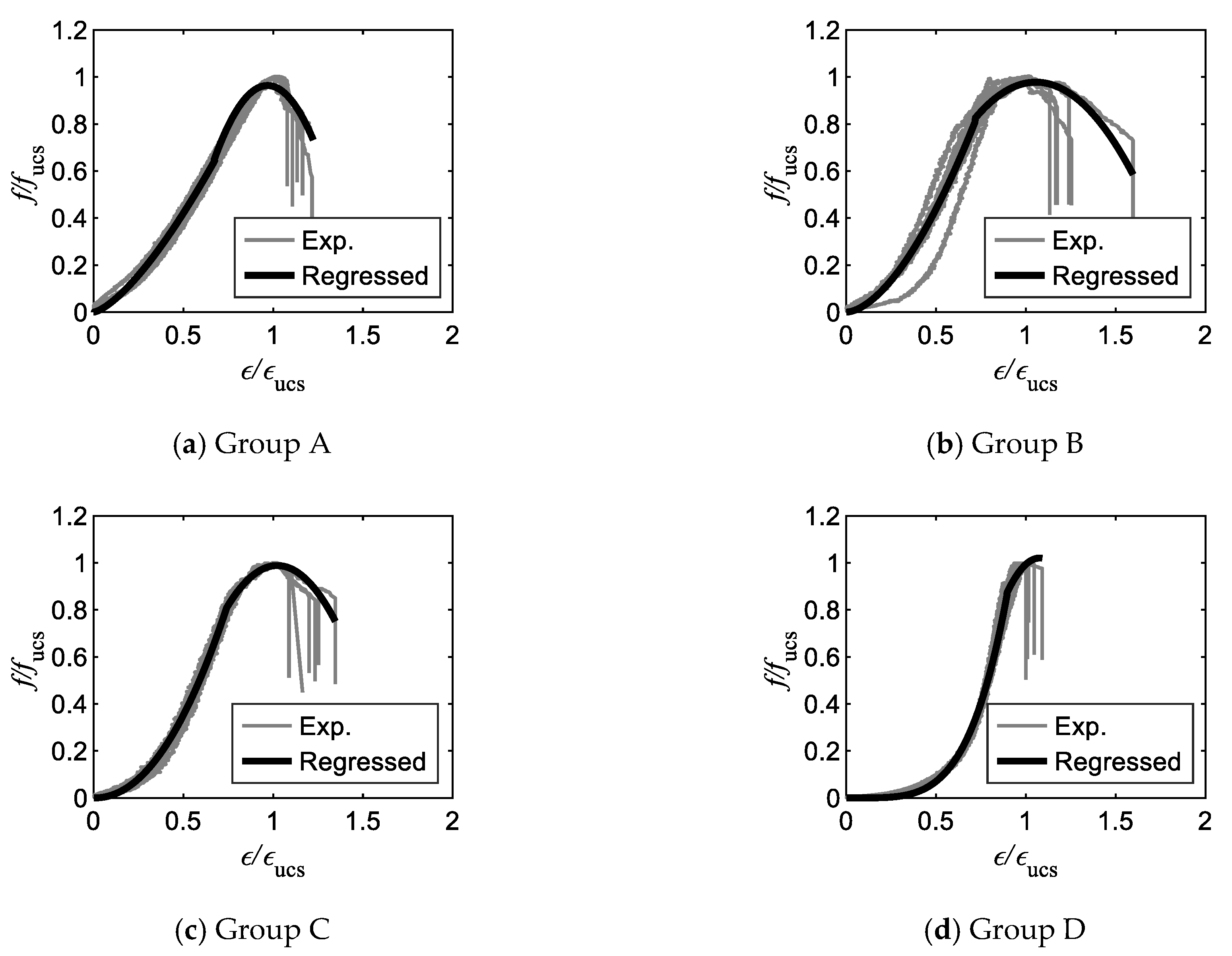

3.3. Compressive Strength and Porosity
| References | Expression | R2 | # Samples |
|---|---|---|---|
| Qi et al., 2022 [16] | fucs = 110.5 exp(−0.08 n) | 0.72 | 17 sandstone samples |
| Farrokhrouz and Asef, 2017 [29] | fucs = −3.03 n + 107.1 | 0.28 | 299 sandstone samples |
| Mishra and Basu, 2013 [38] | fucs = −55.7 ln(n) + 172.1 | 0.88 | 20 sandstone samples |
| Ludovico-Marques et al., 2012 [39] | fucs = 206.7 exp(−0.129 n) | — | 13 sandstone samples |
| Yasar et al., 2010 [74] | fucs = −2.27 n2 + 33.88 n − 16.30 | 0.96 | 11 sandstone samples |
| Kılıç and Teymen, 2008 [79] | fucs = 147.16 exp(−0.0835 n) | 0.93 | 19 rock types, including sandstone |
| Sabatakakis et al., 2008 [72] | fucs = 123.0 exp(−0.12 n) | 0.63 | 95 sandstone samples |
| Tugrul, 2004 [78] | fucs = 195.0 exp(−0.21 n) | 0.79 | 16 different sedimentary rocks, including sandstone |
| Chatterjee and Mukhopadhyay, 2002 [80] | fucs = 64.23 exp(−0.085 n) | 0.92 | 22 samples, including sandstone |
| Palchik, 1999 [73] | fucs = 74.4 exp(−0.04 n) | 0.78 | 16 samples of soft brittle porous sandstone |
| Plumb, 1994 [75] | fucs = 357 (1 − 0.028 n)2 | — | 784 sedimentary rocks, mainly sandstone and shale |

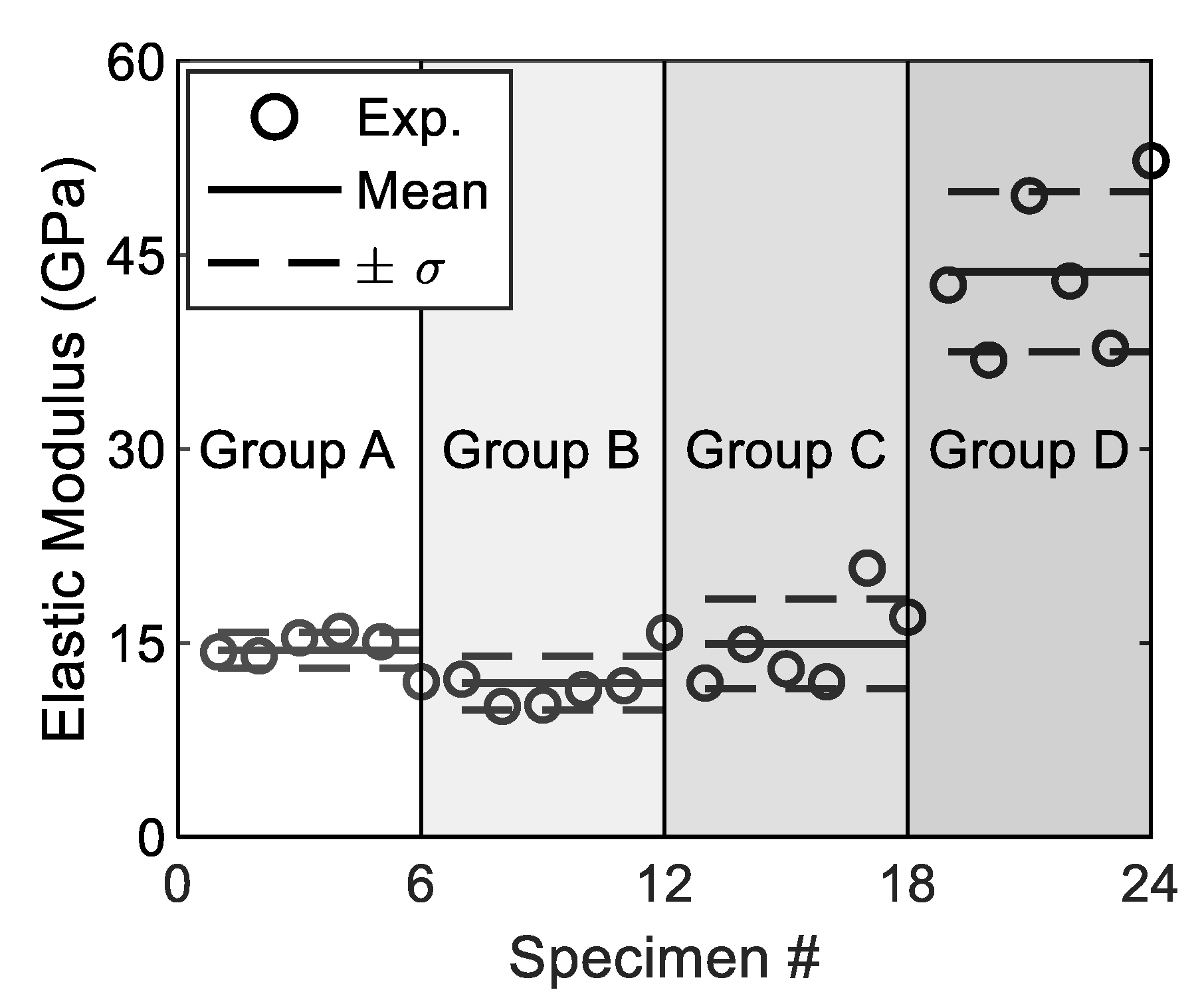
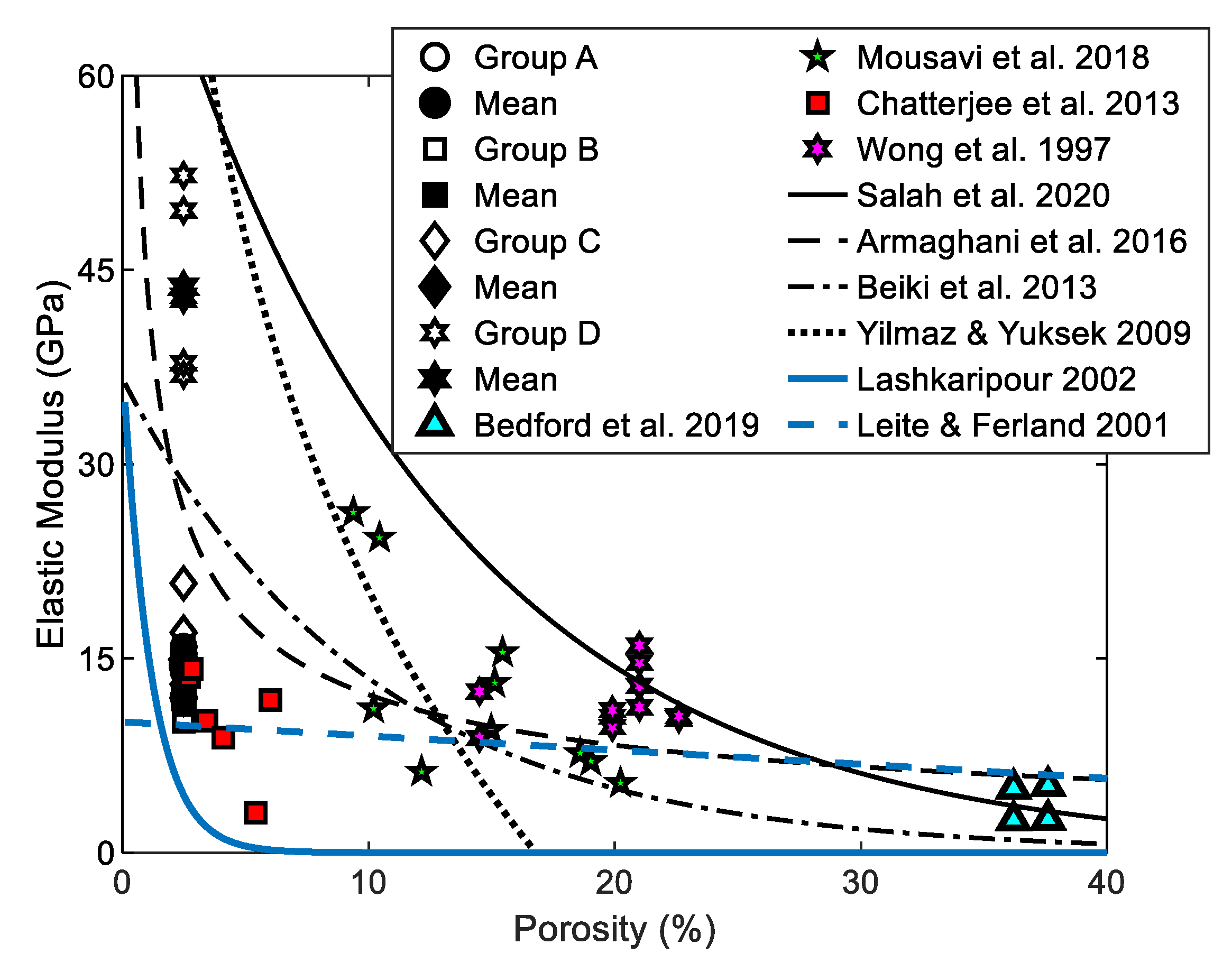
3.4. Elastic Modulus and Porosity
3.5. Compressive Strength and Elastic Modulus
| References | Expression | Units | R2 | # Sample |
|---|---|---|---|---|
| Farrokhrouz snd Asef, 2017 [29] | fucs = 5.49 E0.423/φ0.546 | fucs in MPa, E in GPa, φ is the porosity ratio | 0.8272 | 299 samples of sandstone |
| Sabatakakis et al. 2008 [72] | fucs = E/303 | fucs and E in MPa | 0.65 | 36 samples of sandstone |
| Chatterjee and Mukhopdahyay, 2002 [80] | fucs = (E − 0.17)/0.73 | fucs in MPa, E in GPa | 0.93 | 8 samples, including sandstone |
| Bell and Lindsay, 1999 [41] | fucs = (E − 5.6)/0.358 | fucs in MPa, E in GPa | — | 27 samples of sandstone |
| Bradford et al., 1998 [94] | fucs= 2.28 + 4.1089 E | E in GPa | — | Sandstone sample |
| Lacy, 1997 [95] | fucs = 0.2787 E2 + 2.4582 E | fucs in kpsi and E in Mpsi | 0.84 | 36 samples of weakly consolidated rocks |
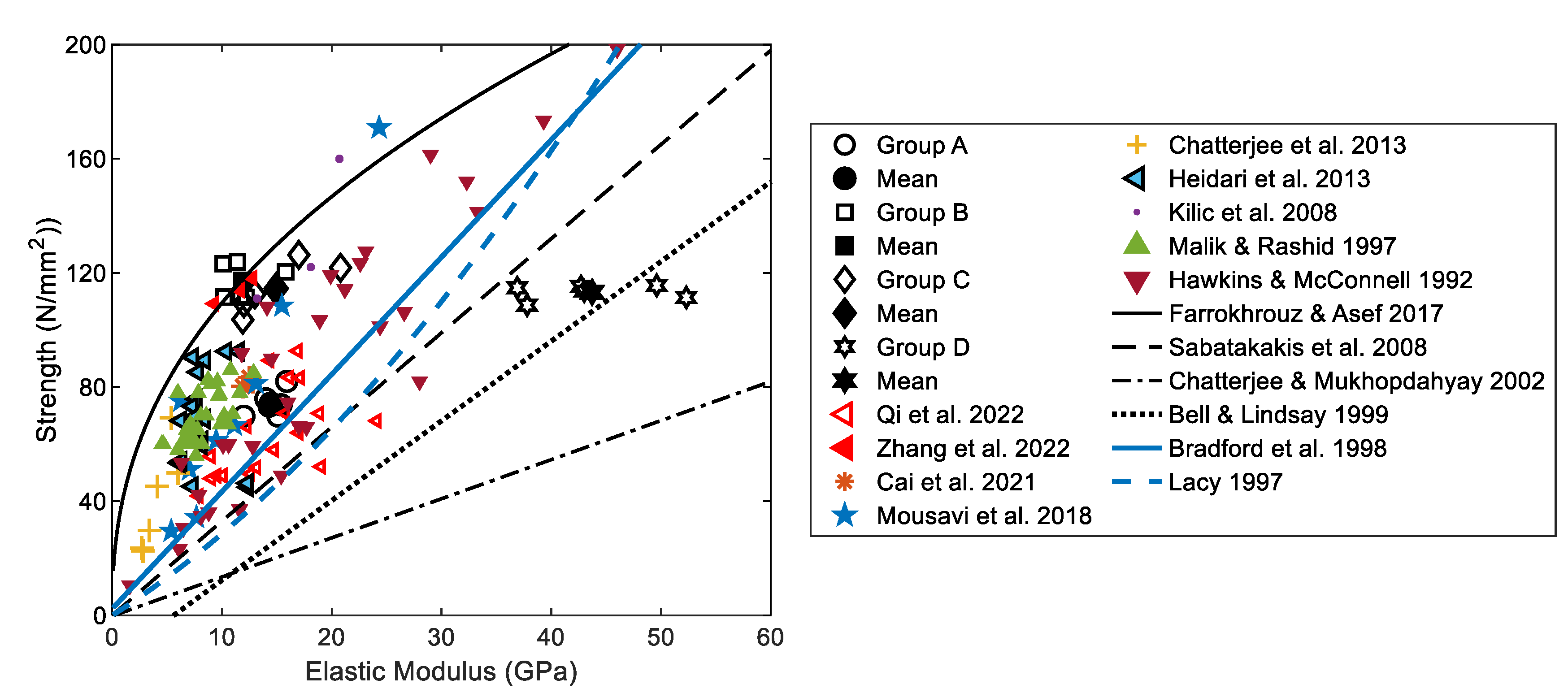
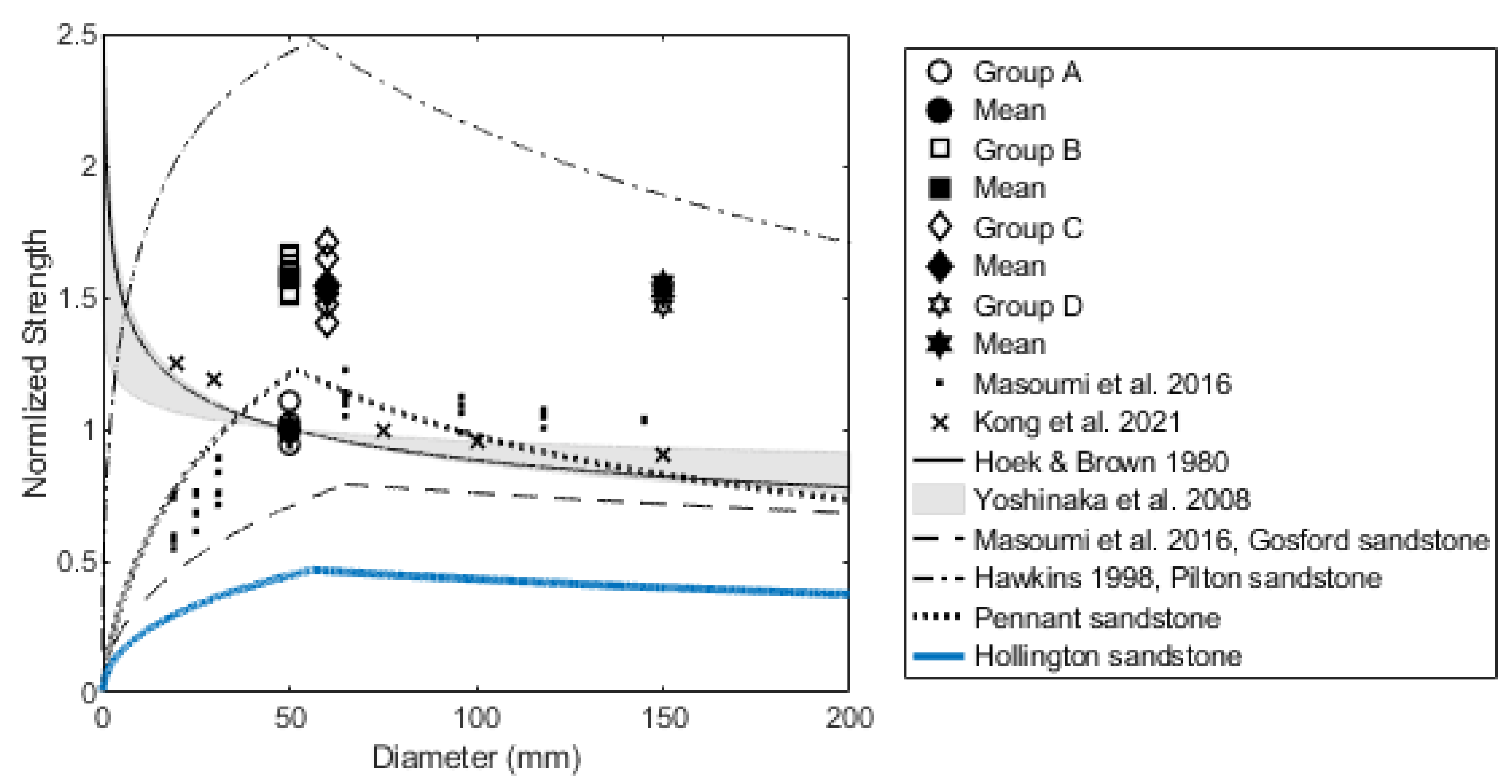
4. Size Effect
4.1. Size Effect on Diameter
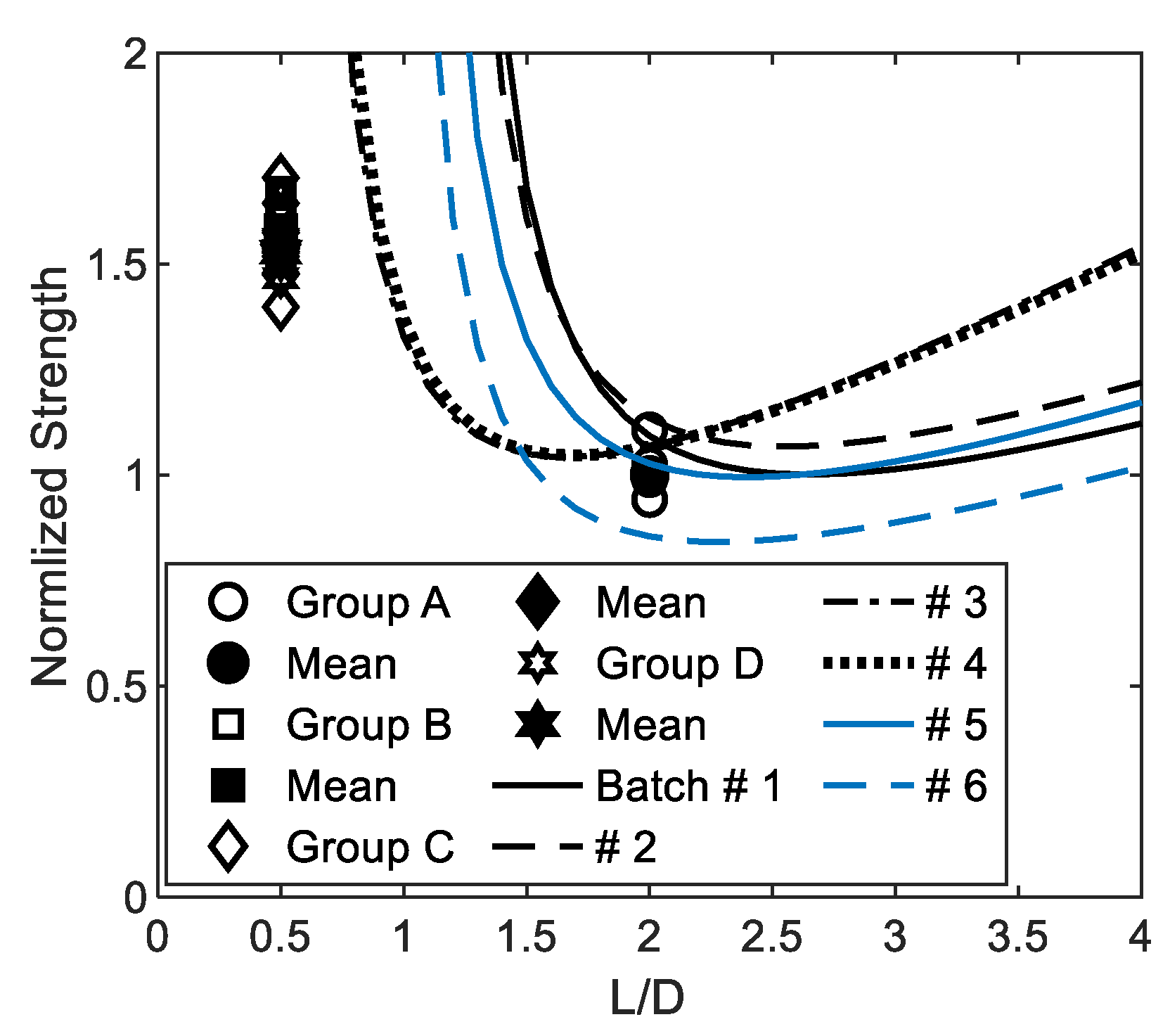
4.2. Size Effect on Length/Diameter Ratio (L/D)
| a | b | RSS | R2 |
|---|---|---|---|
| 0.835 | 0.361 | 0.142 | 0.936 |

5. Conclusions
- (1)
- A columnar vertical fracture was the dominant failure pattern. The stress–strain diagrams of group A converged more than those of the other groups, demonstrating stable mechanical behavior in the standard specimen. The geometry of the diagrams varied among the four groups. The critical strain generally increased with a decrease in the height of the cylinder, whereas the compressive strength exhibited an inverse trend.
- (2)
- The magnitudes of the crack closure stresses of the thin cylinders in groups B, C, and D were identical. A similar trend was observed for the crack initiation stress, crack damage stress, and peak stress. To obtain a representative stress–strain diagram for each group of cylinders, the experimental diagrams were normalized with the peak stress and corresponding critical strain. The normalized stress–strain diagram demonstrated the specific loading behavior of each group of cylinders. To obtain a representative mathematical expression of the diagram, a formula consisting of two parabolas divided by the crack initiation stress was employed for regression.
- (3)
- The correlations between porosity, UCS, and elastic modulus were evaluated based on empirical expressions. The expressions suggested by Lashkaripour [85] and Leite and Ferland [86] provided a reasonably accurate prediction of the UCS of thin cylinders with respect to porosity. However, none of the expressions in the literature achieved a good prediction of the elastic modulus.
- (4)
- The normalized strength was employed to evaluate the size effect on the diameter and L/D ratio of the cylinders. The UCS of group A with standard dimensions was correctly predicted using the expression suggested by Hoek and Brown [106]. However, for thin cylinders, none of the expressions in the literature provided a good prediction. A new expression in terms of L/D was proposed based on the regression analysis of the experimental results.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- John, M. The Influence of Length to Diameter Ratio on Rock Properties in Uniaxial Compression: A Contribution to Standardization in Rock Mechanics Testing; Report South African CSIR No ME1083/5; Council for Scientific and Industrial Research: Pretoria, South Africa, 1972. [Google Scholar]
- Baeza, J.R.; Compán, V.; Castillo, G.; Cámara, M.; Pachón, P. Determining static elastic modulus of weak sandstone in Andalusian historical constructions from non-destructive tests: San Cristóbal’s stone. J. Build. Eng. 2022, 57, 104864. [Google Scholar]
- Bellopede, R.; Zichella, L.; Marini, P. Stone roofing in the Aosta Valley, Italy: Technical properties and durability of traditional Lithotypes. J. Build. Eng. 2021, 35, 102068. [Google Scholar]
- Laila, L.R.; Gurupatham, B.G.A.; Roy, K.; Lim, J.B.P. Effect of super absorbent polymer on microstructural and mechanical properties of concrete blends using granite pulver. Struct. Concr. 2021, 22 (Suppl. S1), E898–E915. [Google Scholar]
- Hatır, M.E.; Ince, I.; Korkanç, M. Intelligent detection of deterioration in cultural stone heritage. J. Build. Eng. 2021, 44, 102690. [Google Scholar]
- Ferreira, C.; Dias, I.S.; Silva, A.; de Brito, J.; Flores-Colen, I. Criteria for selection of cladding systems based on their maintainability. J. Build. Eng. 2021, 39, 102260. [Google Scholar]
- Baek, J.-W.; Kang, S.-M.; Park, H.-G. Direct shear test and cyclic loading test for seismic capacity of dimension stone panel cladding with dowel pin connection. J. Build. Eng. 2021, 38, 102205. [Google Scholar]
- López, A.; Pozo-Antonio, J.; Moreno, A.; Rivas, T.; Pereira, D.; Ramil, A. Femtosecond laser texturing as a tool to increase the hydrophobicity of ornamental stone: The influence of lithology and texture. J. Build. Eng. 2022, 51, 104176. [Google Scholar]
- Pozo-Antonio, J.S.; Dionísio, A.; Vázquez, C. Effects of staining agents on an ornamental granite. J. Build. Eng. 2021, 44, 102700. [Google Scholar]
- Giaretton, M.; Dizhur, D.; da Porto, F.; Ingham, J. Constituent material properties of New Zealand unreinforced stone masonry buildings. J. Build. Eng. 2015, 4, 75–85. [Google Scholar]
- Zhang, Y.; Ta, X.; Qin, S. Effect of heat treatment on physico-mechanical behaviour of a natural building stone: Laizhou dolomite marble. J. Build. Eng. 2022, 47, 103885. [Google Scholar]
- Mousavi, S.H.; Silva, A.; de Brito, J.; Ekhlassi, A.; Hosseini, S.B. Influence of Design on the Service Life of Indirectly Fastened Natural Stone Cladding. J. Perform. Constr. Facil. 2019, 33, 04019021. [Google Scholar]
- Pimienta, L.; Fortin, J.; Guéguen, Y. Investigation of elastic weakening in limestone and sandstone samples from moisture adsorption. Geophys. J. Int. 2014, 199, 335–347. [Google Scholar]
- Elert, K.; Baños, E.G.; Velasco, A.I.; Bel-Anzué, P. Traditional roofing with sandstone slabs: Implications for the safeguarding of vernacular architecture. J. Build. Eng. 2021, 33, 101857. [Google Scholar]
- Feng, X.; Jimenez, R. Bayesian prediction of elastic modulus of intact rocks using their uniaxial compressive strength. Eng. Geol. 2014, 173, 32–40. [Google Scholar]
- Deere, D.U. Rock Mechanics in Engineering Practice; Wiley & Sons: London, UK, 1968. [Google Scholar]
- Qi, Y.; Ju, Y.; Yu, K.; Meng, S.; Qiao, P. The effect of grain size, porosity and mineralogy on the compressive strength of tight sandstones: A case study from the eastern Ordos Basin, China. J. Pet. Sci. Eng. 2022, 208, 109461. [Google Scholar]
- Garrido, M.E.; Petnga, F.B.; Martínez-Ibáñez, V.; Serón, J.B.; Hidalgo-Signes, C.; Tomás, R. Predicting the Uniaxial Compressive Strength of a Limestone Exposed to High Temperatures by Point Load and Leeb Rebound Hardness Testing. Rock Mech. Rock Eng. 2022, 55, 1–17. [Google Scholar]
- Mousavi, E.; Cheshomi, A.; Ashtari, M. Estimating elasticity modulus and uniaxial compressive strength of sandstone using indentation test. J. Pet. Sci. Eng. 2018, 169, 157–166. [Google Scholar]
- Lu, J.; Yin, G.; Zhang, D.; Gao, H.; Li, C.; Li, M. True triaxial strength and failure characteristics of cubic coal and sandstone under different loading paths. Int. J. Rock Mech. Min. Sci. 2020, 135, 104439. [Google Scholar]
- Rice-Birchall, E.; Faulkner, D.R.; Bedford, J.D. The manufacture, mechanical properties, and microstructural analysis of synthetic quartz-cemented sandstones. Int. J. Rock Mech. Min. Sci. 2021, 146, 104869. [Google Scholar]
- Potysz, A.; Bartz, W. Bioweathering of minerals and dissolution assessment by experimental simulations—Implications for sandstone rocks: A review. Constr. Build. Mater. 2022, 316, 125862. [Google Scholar]
- Wang, W.; Liu, T.G.; Shao, J.F. Effects of Acid Solution on the Mechanical Behavior of Sandstone. J. Mater. Civ. Eng. 2016, 28, 04015089. [Google Scholar]
- Pires, V.; Rosa, L.G.; Amaral, P.M.; Dionísio, A.; Simão, J.A.R. Experimental Studies of the Effect of SO2 on the Mechanical Properties of Selected Cladding Natural Stones. J. Mater. Civ. Eng. 2022, 34, 04022084. [Google Scholar]
- Eberhardt, E.; Stead, D.; Stimpson, B. Quantifying progressive pre-peak brittle fracture damage in rock during uniaxial compression. Int. J. Rock Mech. Min. Sci. 1999, 36, 361–380. [Google Scholar]
- Peng, K.; Liu, Z.; Zou, Q.; Zhang, Z.; Zhou, J. Static and Dynamic Mechanical Properties of Granite from Various Burial Depths. Rock Mech. Rock Eng. 2019, 52, 3545–3566. [Google Scholar]
- Lanaro, F.; Sato, T.; Nakama, S. Depth Variability of Compressive Strength Test Results of Toki Granite from Shobasama and Mizunami Construction Sites, Japan. Rock Mech. Rock Eng. 2009, 42, 611–629. [Google Scholar]
- Liu, J.-b.; Zhang, Z.-j.; Li, B. Microscopic & macroscopic characterizations of Beijing marble as a building material for UNESCO heritage sites: New insights into physico-mechanical property estimation and weathering resistance. Constr. Build. Mater. 2019, 225, 510–525. [Google Scholar]
- Huang, B.; Lu, W. Experimental investigation of the uniaxial compressive behavior of thin building granite. Constr. Build. Mater. 2021, 267, 120967. [Google Scholar]
- Farrokhrouz, M.; Asef, M.R. Experimental investigation for predicting compressive strength of sandstone. J. Nat. Gas Sci. Eng. 2017, 43, 222–229. [Google Scholar]
- Zorlu, K.; Gokceoglu, C.; Ocakoglu, F.; Nefeslioglu, H.A.; Acikalin, S. Prediction of uniaxial compressive strength of sandstones using petrography-based models. Eng. Geol. 2008, 96, 141–158. [Google Scholar]
- Armaghani, D.J.; Amin, M.F.M.; Yagiz, S.; Faradonbeh, R.S.; Abdullah, R.A. Prediction of the uniaxial compressive strength of sandstone using various modeling techniques. Int. J. Rock Mech. Min. Sci. 2016, 85, 174–186. [Google Scholar]
- Yurdakul, M.; Akdas, H. Modeling uniaxial compressive strength of building stones using non-destructive test results as neural networks input parameters. Constr. Build. Mater. 2013, 47, 1010–1019. [Google Scholar]
- Wang, Y.; Aladejare, A.E. Selection of site-specific regression model for characterization of uniaxial compressive strength of rock. Int. J. Rock Mech. Min. Sci. 2015, 75, 73–81. [Google Scholar]
- Çalışkan, A.; Demirhan, S.; Tekin, R. Comparison of different machine learning methods for estimating compressive strength of mortars. Constr. Build. Mater. 2022, 335, 127490. [Google Scholar]
- Li, S.; Wu, Y.; Huo, R.; Song, Z.; Fujii, Y.; Shen, Y. Mechanical Properties of Acid-corroded Sandstone Under Uniaxial Compression. Rock Mech. Rock Eng. 2021, 54, 289–302. [Google Scholar]
- Huang, S.; Xia, K. Effect of heat-treatment on the dynamic compressive strength of Longyou sandstone. Eng. Geol. 2015, 191, 1–7. [Google Scholar]
- Liu, B.; Sun, Y.; Wang, J.; Zhang, G. Characteristic Analysis of Crack Initiation and Crack Damage Stress of Sandstone and Mudstone under Low-Temperature Condition. J. Cold Reg. Eng. 2020, 34, 04020020. [Google Scholar]
- Mishra, D.A.; Basu, A. Estimation of uniaxial compressive strength of rock materials by index tests using regression analysis and fuzzy inference system. Eng. Geol. 2013, 160, 54–68. [Google Scholar]
- Ludovico-Marques, M.; Chastre, C.; Vasconcelos, G. Modelling the compressive mechanical behaviour of granite and sandstone historical building stones. Constr. Build. Mater. 2012, 28, 372–381. [Google Scholar]
- Shakoor, A.; Barefield, E.H. Relationship between Unconfined Compressive Strength and Degree of Saturation for Selected Sandstones. Environ. Eng. Geosci. 2009, 15, 29–40. [Google Scholar]
- Bell, F.G.; Lindsay, P. The petrographic and geomechanical properties of some sandstones from the Newspaper Member of the Natal Group near Durban, South Africa. Eng. Geol. 1999, 53, 57–81. [Google Scholar]
- BS EN 1926:2006; Natural Stone Test Methods—Determination of Uniaxial Compressive Strength. European Committee for Standardization: Brussels, Belgium, 2006.
- GB/T 50266-2013; CEC, Standard for Tests Method of Engineering Rock Masses. China Plan Press: Beijing, China, 2013.
- Bieniawski, Z.T. The effect of specimen size on compressive strength of coal. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1968, 5, 325–335. [Google Scholar]
- Cvitanović, N.Š.; Nikolić, M.; Ibrahimbegović, A. Influence of specimen shape deviations on uniaxial compressive strength of limestone and similar rocks. Int. J. Rock Mech. Min. Sci. 2015, 80, 357–372. [Google Scholar]
- ASTM D7012-14e1; Standard Test Methods for Compressive Strength and Elastic Moduli of Intact Rock Core Specimens under Varying States of Stress and Temperatures. ASTM International: West Conshohocken, PA, USA, 2014.
- ASTM C170/C170M-17; Standard Test Method for Compressive Strength of Dimension Stone. ASTM International: West Conshohocken, PA, USA, 2017.
- Fairhurst, C.E.; Hudson, J.A. Draft ISRM suggested method for the complete stress-strain curve for intact rock in uniaxial compression. Int. J. Rock Mech. Min. Sci. 1999, 36, 279–289. [Google Scholar]
- Hudson, J.A.; Crouch, S.L.; Fairhurst, C. Soft, stiff and servo-controlled testing machines: A review with reference to rock failure. Eng. Geol. 1972, 6, 155–189. [Google Scholar]
- Okubo, S.; Nishimatsu, Y. Uniaxial compression testing using a linear combination of stress and strain as the control variable. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1985, 22, 323–330. [Google Scholar]
- Vasconcelos, G.; Lourenço, P.B.; Alves, C.A.S.; Pamplona, J. Compressive Behavior of Granite: Experimental Approach. J. Mater. Civ. Eng. 2009, 21, 502–511. [Google Scholar]
- Cai, M.; Hou, P.Y.; Zhang, X.W.; Feng, X.T. Post-peak Stress–Strain curves of brittle hard rocks under axial-strain-controlled loading. Int. J. Rock Mech. Min. Sci. 2021, 147, 104921. [Google Scholar]
- Wong, L.N.Y.; Meng, F.; Guo, T.; Shi, X. The Role of Load Control Modes in Determination of Mechanical Properties of Granite. Rock Mech. Rock Eng. 2020, 53, 539–552. [Google Scholar]
- Ray, S.K.; Sarkar, M.; Singh, T.N. Effect of cyclic loading and strain rate on the mechanical behaviour of sandstone. Int. J. Rock Mech. Min. Sci. 1999, 36, 543–549. [Google Scholar]
- Song, Z.; Konietzky, H.; Wu, Y.; Du, K.; Cai, X. Mechanical behaviour of medium-grained sandstones exposed to differential cyclic loading with distinct loading and unloading rates. J. Rock Mech. Geotech. Eng. 2022, in press. [Google Scholar] [CrossRef]
- Tschegg, E.K.; Elser, M.; Stanzl-Tschegg, S.E. Biaxial fracture tests on concrete—Development and experience. Cem. Concr. Compos. 1995, 17, 57–75. [Google Scholar]
- Hoek, E.; Martin, C.D. Fracture initiation and propagation in intact rock—A review. J. Rock Mech. Geotech. Eng. 2014, 6, 287–300. [Google Scholar]
- Basu, A.; Mishra, D.A.; Roychowdhury, K. Rock failure modes under uniaxial compression, Brazilian, and point load tests. Bull. Eng. Geol. Environ. 2013, 72, 457–475. [Google Scholar]
- ASTM C39/C39M-14; Standard Test Method for Compressive Strength of Cylindrical Concrete Specimens. ASTM International: West Conshohocken, PA, USA, 2014.
- Vaneghi, R.G.; Thoeni, K.; Dyskin, A.V.; Sharifzadeh, M.; Sarmadivaleh, M. Strength and Damage Response of Sandstone and Granodiorite under Different Loading Conditions of Multistage Uniaxial Cyclic Compression. Int. J. Geomech. 2020, 20, 04020159. [Google Scholar]
- Fakhimi, A.; Hemami, B. Axial splitting of rocks under uniaxial compression. Int. J. Rock Mech. Min. Sci. 2015, 79, 124–134. [Google Scholar]
- Wasantha, P.L.P.; Ranjith, P.G.; Zhao, J.; Shao, S.S.; Permata, G. Strain Rate Effect on the Mechanical Behaviour of Sandstones with Different Grain Sizes. Rock Mech. Rock Eng. 2015, 48, 1883–1895. [Google Scholar]
- Jansen, D.C.; Shah, S.P. Effect of Length on Compressive Strain Softening of Concrete. J. Eng. Mech. 1997, 123, 25–35. [Google Scholar]
- Martin, C.D.; Chandler, N.A. The progressive fracture of Lac du Bonnet granite. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1994, 31, 643–659. [Google Scholar]
- Bieniawski, Z.T. Mechanism of brittle fracture of rock: Part I—Theory of the fracture process. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1967, 4, 395–406. [Google Scholar]
- MathWorks. Matlab (2012a); The MathWorks, Inc.: Natick, MA, USA, 2012. [Google Scholar]
- Liu, Z.; Yao, Q.; Kong, B.; Yin, J. Macro-micro mechanical properties of building sandstone under different thermal damage conditions and thermal stability evaluation using acoustic emission technology. Constr. Build. Mater. 2020, 246, 118485. [Google Scholar]
- Zhang, H.; Lu, K.; Zhang, W.; Li, D.; Yang, G. Quantification and acoustic emission characteristics of sandstone damage evolution under dry–wet cycles. J. Build. Eng. 2022, 48, 103996. [Google Scholar]
- Kong, F.; Xue, Y.; Qiu, D.; Gong, H.; Ning, Z. Effect of grain size or anisotropy on the correlation between uniaxial compressive strength and Schmidt hammer test for building stones. Constr. Build. Mater. 2021, 299, 123941. [Google Scholar]
- Plumb, R.A. Influence of composition and texture on failure properties of clastic rocks. In Proceedings of the Europec SPE/ISRM Rock Mechanics in Petroleum Engineering Conference, Delft, The Netherlands, 29–31 August 1994. [Google Scholar]
- Kılıç, A.; Teymen, A. Determination of mechanical properties of rocks using simple methods. Bull. Eng. Geol. Environ. 2008, 67, 237. [Google Scholar]
- Chatterjee, R.; Mukhopadhyay, M. Petrophysical and geomechanical properties of rocks from the oilfields of the Krishna-Godavari and Cauvery Basins, India. Bull. Eng. Geol. Environ. 2002, 61, 169–178. [Google Scholar]
- Ghasemi, E.; Kalhori, H.; Bagherpour, R.; Yagiz, S. Model tree approach for predicting uniaxial compressive strength and Young’s modulus of carbonate rocks. Bull. Eng. Geol. Environ. 2018, 77, 331–343. [Google Scholar]
- Palchik, V. Influence of Porosity and Elastic Modulus on Uniaxial Compressive Strength in Soft Brittle Porous Sandstones. Rock Mech. Rock Eng. 1999, 32, 303–309. [Google Scholar]
- Wang, Y.; Aladejare, A.E. Bayesian characterization of correlation between uniaxial compressive strength and Young’s modulus of rock. Int. J. Rock Mech. Min. Sci. 2016, 85, 10–19. [Google Scholar]
- Baud, P.; Wong, T.-f.; Zhu, W. Effects of porosity and crack density on the compressive strength of rocks. Int. J. Rock Mech. Min. Sci. 2014, 67, 202–211. [Google Scholar]
- Sabatakakis, N.; Koukis, G.; Tsiambaos, G.; Papanakli, S. Index properties and strength variation controlled by microstructure for sedimentary rocks. Eng. Geol. 2008, 97, 80–90. [Google Scholar]
- Yaşar, E.; Ranjith, P.G.; Perera, M.S.A. Physico-mechanical behaviour of southeastern Melbourne sedimentary rocks. Int. J. Rock Mech. Min. Sci. 2010, 47, 481–487. [Google Scholar]
- Tuǧrul, A. The effect of weathering on pore geometry and compressive strength of selected rock types from Turkey. Eng. Geol. 2004, 75, 215–227. [Google Scholar]
- Shi, X.; Cai, W.; Meng, Y.; Li, G.; Wen, K.; Zhang, Y. Weakening laws of rock uniaxial compressive strength with consideration of water content and rock porosity. Arab. J. Geosci. 2016, 9, 369. [Google Scholar]
- Chatterjee, R.; Manoharan, K.; Mukhopadhyay, M. Petrophysical and mechanical properties of cretaceous sedimentary rocks of Cauvery basin, Eastern continental Margin of India. J. Indian Geophys. Union 2013, 17, 349–359. [Google Scholar]
- Bedford, J.D.; Faulkner, D.R.; Wheeler, J.; Leclère, H. High-Resolution Mapping of Yield Curve Shape and Evolution for High-Porosity Sandstone. J. Geophys. Res. Solid Earth 2019, 124, 5450–5468. [Google Scholar]
- Wong, T.-F.; David, C.; Zhu, W. The transition from brittle faulting to cataclastic flow in porous sandstones: Mechanical deformation. J. Geophys. Res. Solid Earth 1997, 102, 3009–3025. [Google Scholar]
- Salah, M.K.; Alqudah, M.; Monzer, A.J.; David, C. Petrophysical and acoustic characteristics of Jurassic and Cretaceous rocks from Central Lebanon. Carbonates Evaporites 2020, 35, 12. [Google Scholar]
- Lashkaripour, G.R. Predicting mechanical properties of mudrock from index parameters. Bull. Eng. Geol. Environ. 2002, 61, 73–77. [Google Scholar]
- Leite, M.H.; Ferland, F. Determination of unconfined compressive strength and Young’s modulus of porous materials by indentation tests. Eng. Geol. 2001, 59, 267–280. [Google Scholar]
- Beiki, M.; Majdi, A.; Givshad, A.D. Application of genetic programming to predict the uniaxial compressive strength and elastic modulus of carbonate rocks. Int. J. Rock Mech. Min. Sci. 2013, 63, 159–169. [Google Scholar]
- Yilmaz, I.; Yuksek, G. Prediction of the strength and elasticity modulus of gypsum using multiple regression, ANN, and ANFIS models. Int. J. Rock Mech. Min. Sci. 2009, 46, 803–810. [Google Scholar]
- Hoek, E.; Diederichs, M.S. Empirical estimation of rock mass modulus. Int. J. Rock Mech. Min. Sci. 2006, 43, 203–215. [Google Scholar]
- Sonmez, H.; Tuncay, E.; Gokceoglu, C. Models to predict the uniaxial compressive strength and the Modulus of elasticity for Ankara Agglomerate. Int. J. Rock Mech. Min. Sci. 2004, 41, 717–729. [Google Scholar]
- Heidari, M.; Momeni, A.A.; Rafiei, B.; Khodabakhsh, S.; Torabi-Kaveh, M. Relationship Between Petrographic Characteristics and the Engineering Properties of Jurassic Sandstones, Hamedan, Iran. Rock Mech. Rock Eng. 2013, 46, 1091–1101. [Google Scholar]
- Malik, M.H.; Rashid, S. Correlation of some engineering geological properties of the Murree formation at lower Topa (Murree district), Pakistan. Geol. Bull. Univ. Peshawar 1997, 30, 69–81. [Google Scholar]
- Hawkins, A.B.; McConnell, B.J. Sensitivity of sandstone strength and deformability to changes in moisture content. Q. J. Eng. Geol. Hydrogeol. 1992, 25, 115–130. [Google Scholar]
- Bradford, I.D.R.; Fuller, J.; Thompson, P.J.; Walsgrove, T.R. Benefits of Assessing the Solids Production Risk in a North Sea Reservoir using Elastoplastic Modelling. In Proceedings of the SPE/ISRM Rock Mechanics in Petroleum Engineering, Trondheim, Norway, 8–10 July 1998. [Google Scholar]
- Lacy, L.L. Dynamic Rock Mechanics Testing for Optimized Fracture Designs. In Proceedings of the SPE Annual Technical Conference and Exhibition, San Antonio, TX, USA, 5–8 October 1997. [Google Scholar]
- Pratt, H.R.; Black, A.D.; Brown, W.S.; Brace, W.F. The effect of speciment size on the mechanical properties of unjointed diorite. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1972, 9, 513–516. [Google Scholar]
- Bažant, Z.P. Size Effect in Blunt Fracture: Concrete, Rock, Metal. J. Eng. Mech. 1984, 110, 518–535. [Google Scholar]
- Gonnermann, H.F. Effect of size and shape of test specimen on compressive strength of concrete. Proc. ASTM 1925, 25, 237–250. [Google Scholar]
- De la Fuente, A.; Aguado, A.; Molins, C.; Armengou, J. Innovations on components and testing for precast panels to be used in reinforced earth retaining walls. Constr. Build. Mater. 2011, 25, 2198–2205. [Google Scholar]
- Naeimi, N.; Moustafa, M.A. Compressive behavior and stress–strain relationships of confined and unconfined UHPC. Constr. Build. Mater. 2021, 272, 121844. [Google Scholar]
- Muciaccia, G.; Rosati, G.; di Luzio, G. Compressive failure and size effect in plain concrete cylindrical specimens. Constr. Build. Mater. 2017, 137, 185–194. [Google Scholar]
- Bažant, Z.P.; Planas, J. Fracture and Size Effect in Concrete and Other Quasibrittle Materials; CRC Press: London, UK, 1998. [Google Scholar]
- Mier, J.G.M. Fracture Processes of Concrete; CRC Press: Boca Raton, FL, USA, 1996. [Google Scholar]
- Hoek, E.; Brown, E.T. Practical estimates of rock mass strength. Int. J. Rock Mech. Min. Sci. 1997, 34, 1165–1186. [Google Scholar]
- Weibull, W. A statistical distribution of function of wide applicability. J. Appl. Mech. 1951, 18, 03112318. [Google Scholar]
- Hoek, E.; Brown, E.T. Underground Excavation in Rock; IMM: London, UK, 1980. [Google Scholar]
- Yoshinaka, R.; Osada, M.; Park, H.; Sasaki, T.; Sasaki, K. Practical determination of mechanical design parameters of intact rock considering scale effect. Eng. Geol. 2008, 96, 173–186. [Google Scholar]
- Griffith, A.A. The theory of rupture. In Proceedings of the 1st International Congress of Applied Mechanics, Delft, The Netherlands, 22–26 April 1924; pp. 55–63. [Google Scholar]
- Mandelbort, B.B. The Fractal Geometry of Nature; Freeman: San Francisco, CA, USA, 1982. [Google Scholar]
- Carpinteri, A.; Chiaia, B.; Ferro, G. Size effects on nominal tensile strength of concrete structures: Multifractality of material ligaments and dimensional transition from order to disorder. Mater. Struct. 1995, 28, 311. [Google Scholar]
- Bažant, Z.P. Scaling of quasibrittle fracture: Hypotheses of invasive and lacunar fractality, their critique and Weibull connection. Int. J. Fract. 1997, 83, 41. [Google Scholar]
- Hawkins, A.B. Aspects of rock strength. Bull. Eng. Geol. Environ. 1998, 57, 17–30. [Google Scholar]
- Darlington, W.J.; Ranjith, P.G.; Choi, S.K. The Effect of Specimen Size on Strength and Other Properties in Laboratory Testing of Rock and Rock-Like Cementitious Brittle Materials. Rock Mech. Rock Eng. 2011, 44, 513. [Google Scholar]
- Masoumi, H.; Saydam, S.; Hagan, P.C. Unified Size-Effect Law for Intact Rock. Int. J. Geomech. 2016, 16, 04015059. [Google Scholar]
- Mogi, K. The influence of the dimensions of specimens on the rock fracture strength of rocks. Bull. Earthq. Res. Inst. Tokyo Univ. 1962, 40, 175–185. [Google Scholar]
- Chen, J.; Yang, R.; Kang, Y. Influence of the Rock Length-to-Diameter Ratio and Failure Modes on Uniaxial Compression Strength. Geotech. Geol. Eng. 2020, 38, 2551–2557. [Google Scholar]
- Obert, L.; Duvall, W.I. Rock Mechanics and the Design of Structures in Rock; Wiley: London, UK, 1967. [Google Scholar]
- Kahraman, S.; Alber, M. Estimating unconfined compressive strength and elastic modulus of a fault breccia mixture of weak blocks and strong matrix. Int. J. Rock Mech. Min. Sci. 2006, 43, 1277–1287. [Google Scholar]
- Tuncay, E.; Hasancebi, N. The effect of length to diameter ratio of test specimens on the uniaxial compressive strength of rock. Bull. Eng. Geol. Environ. 2009, 68, 491. [Google Scholar]
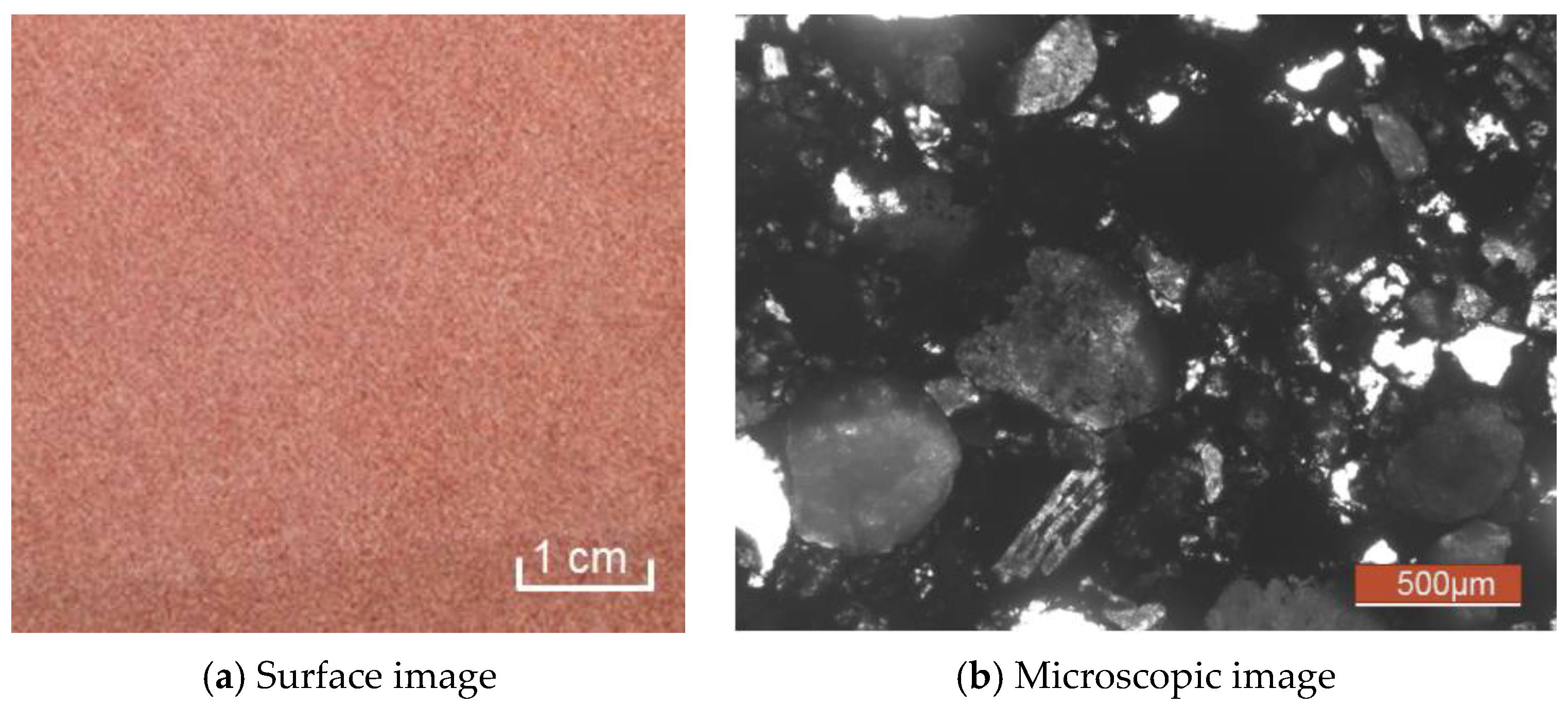





| Mineral | Quartz | Plagioclase | Calcite | Zeolite | Potash Feldspar | Others |
|---|---|---|---|---|---|---|
| Percentage (%) | 42.4 | 34.9 | 9.2 | 7.3 | 5.2 | 1.0 |
| References | Porosity (%) | Density (g/cm3) |
|---|---|---|
| Current study | 2.38 | 2.46 |
| Li et al., 2021 [35] | 5.91 | 2.48 |
| Liu et al., 2020 [37] | 20.48 | 1.85 |
| Mousavi et al., 2018 [18] | 9.38–20.23 | 1.99–2.88 |
| Huang and Xia, 2015 [36] | 17 | 2.15 |
| Mishra and Basu, 2013 [38] | 2.89–15.54 | 2.17–2.49 |
| Ludovico-Marques et al., 2012 [39] | 3.6–18.6 | 2.18–2.59 |
| Shakoor and Barefield, 2009 [40] | 4.12–12.72 | 2.07–2.52 |
| Bell and Lindsay, 1999 [41] | 5.6–10.1 | 2.43–2.57 |
| O | Si | AL | Ca | K | Fe | Na | Mg |
|---|---|---|---|---|---|---|---|
| 60.1 | 24.2 | 4.6 | 2.8 | 1.2 | 1.2 | 0.9 | 0.9 |
| Group # | L (mm) | D (mm) | L/D | Number |
|---|---|---|---|---|
| A | 100 | 50 | 2.0 | 6 |
| B | 25 | 50 | 0.5 | 6 |
| C | 30 | 60 | 0.5 | 6 |
| D | 75 | 150 | 0.5 | 6 |
| Group | a1 | b1 | a2 | b2 | c2 |
|---|---|---|---|---|---|
| A | 1.13 | 1.42 | −3.57 | 6.92 | −2.39 |
| B | 1.42 | 1.70 | −1.34 | 2.83 | −0.52 |
| C | 1.52 | 2.11 | −2.26 | 4.63 | −1.38 |
| D | 1.39 | 4.30 | −4.42 | 9.54 | −4.12 |
| Group | 1st Portion | 2nd Portion | ||
|---|---|---|---|---|
| RSS | R2 | RSS | R2 | |
| A | 6.699 | 0.965 | 8.634 | 0.433 |
| B | 14.61 | 0.880 | 5.933 | 0.595 |
| C | 1.788 | 0.979 | 0.359 | 0.903 |
| D | 6.425 | 0.982 | 2.908 | 0.712 |
| References | Expression | R2 | # Samples |
|---|---|---|---|
| Salah et al., 2020 [84] | E = 78.926 exp(−0.0852 n) | 0.96 | 49 samples, including sandstone |
| Armaghani et al., 2016 [31] | E = 43.899 n(−0.556) | 0.28 | 71 granite samples |
| Beiki et al., 2013 [87] | E = exp(−0.10 n + 3.6) | 0.23 | 72 different carbonate rock types |
| Beiki et al., 2013 [87] | E = 36.6 (0.91)n | 0.23 | 72 different carbonate rock types |
| Yilmaz and Yuksek, 2009 [88] | E = −39.1 ln(n) + 110.31 | 0.83 | 121 samples of gypsum |
| Lashkaripour, 2002 [85] | E = 37.9 exp(−0.863 n) | 0.68 | Claystone, clay shale, mudstone, mud shale |
| Leite and Ferland, 2001 [86] | E = 10.10 − 0.109 n | 0.74 | Artificial rock |
| Batch # | Sandstone Type | c (N/mm2) | φ (Degree) | Mean UCS (N/mm2) | L/D Limit |
|---|---|---|---|---|---|
| 1 | Fine | 18.75 | 48.6 | 99.13 | 1.2 |
| 2 | Fine | 19.47 | 47.1 | 92.87 | 1.1 |
| 3 | Fine | 31.96 | 27.7 | 101.44 | 0.6 |
| 4 | Argillaceous | 9.80 | 28.7 | 31.62 | 0.6 |
| 5 | Medium-coarse | 11.72 | 44.7 | 56.48 | 1.0 |
| 6 | Fine | 18.11 | 42.6 | 98.08 | 1.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, B.; Xu, Y.; Zhang, G. Experimental Investigation on Uniaxial Compressive Strength of Thin Building Sandstone. Buildings 2022, 12, 1945. https://doi.org/10.3390/buildings12111945
Huang B, Xu Y, Zhang G. Experimental Investigation on Uniaxial Compressive Strength of Thin Building Sandstone. Buildings. 2022; 12(11):1945. https://doi.org/10.3390/buildings12111945
Chicago/Turabian StyleHuang, Baofeng, Yixian Xu, and Guojun Zhang. 2022. "Experimental Investigation on Uniaxial Compressive Strength of Thin Building Sandstone" Buildings 12, no. 11: 1945. https://doi.org/10.3390/buildings12111945
APA StyleHuang, B., Xu, Y., & Zhang, G. (2022). Experimental Investigation on Uniaxial Compressive Strength of Thin Building Sandstone. Buildings, 12(11), 1945. https://doi.org/10.3390/buildings12111945







