A More Comprehensive Habitable Zone for Finding Life on Other Planets
Abstract
:1. Introduction
2. The Classical Habitable Zone
2.1. Additional Assumptions
2.2. Effective Stellar Flux
2.3. The Inner Edges of the Classical Habitable Zone
2.4. The Carbonate–Silicate Cycle and the Outer Edges of the Classical Habitable Zone
2.5. The Classical Habitable Zone with Empirical Limits
3. Habitability case studies: Venus and Mars
3.1. The Fate of Venus
3.2. The Fate of Mars
4. Limit Cycles
5. The Role of Oxygen in Habitability
5.1. The Importance of Oxygen-Poor Planets in the Search for Extraterrestrial Life
5.2. Is Oxygen Really a Good Biosignature?
6. Expanding the Spectral Range of the Classical HZ
7. Planetary Habitability: Extensions in Space
7.1. Extending the Habitable Zone with Hydrogen
7.2. Extending the Habitable Zone with Methane
7.3. Methane Daisyworld for Hotter Stars
8. Planetary Habitability: Extensions in Time
8.1. Habitability during the Pre-Main-Sequence
8.2. The Ultimate Fate of Worlds during the Post-Main-Sequence
9. Habitability of Ocean Worlds
10. Habitability of Desert Worlds
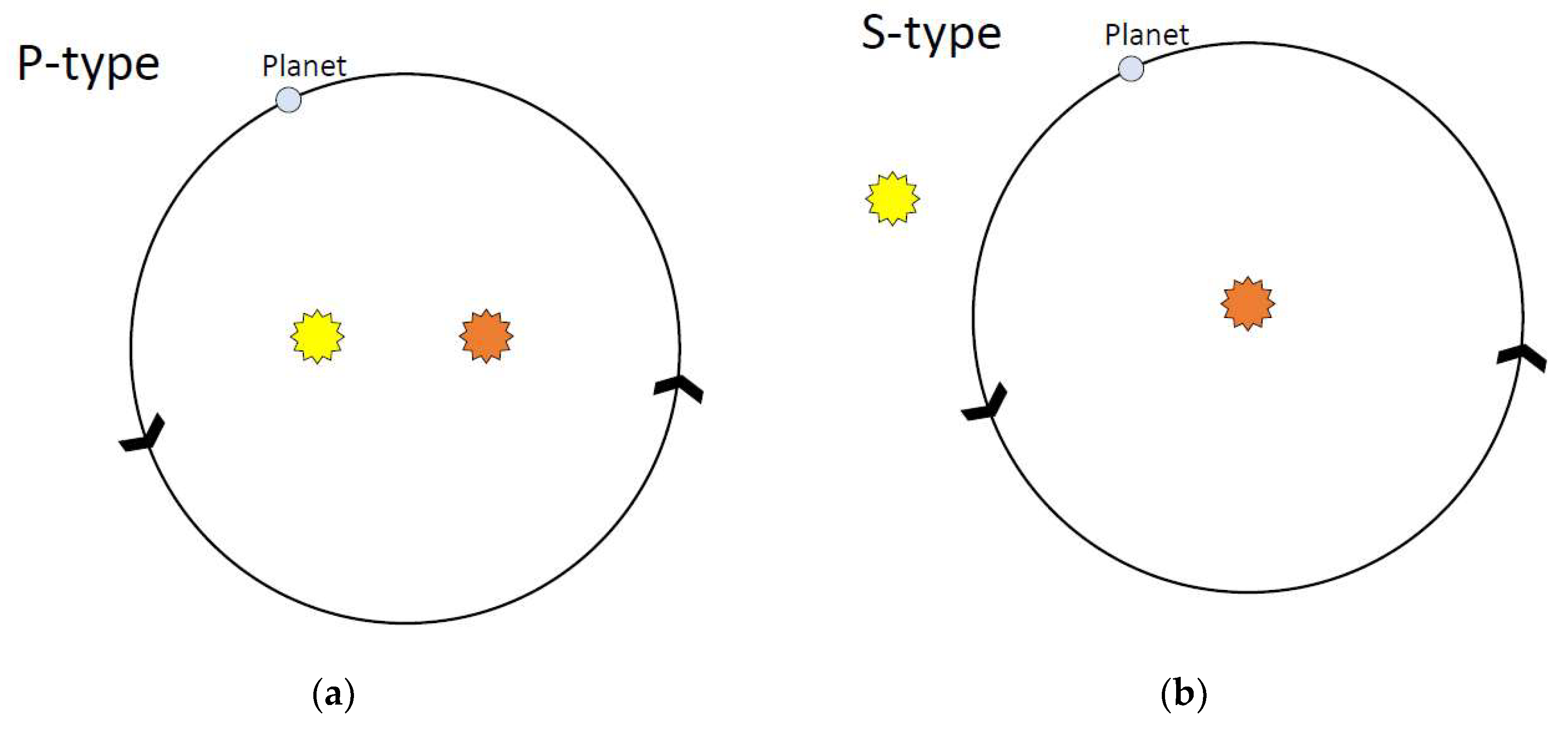
11. Binary Star Habitable Zones
12. Other Factors that May Influence the Inner and Outer Edges of the Habitable Zone
12.1. The Effects of Rotation Rate
12.2. The Effects of CO2 and CO2 Clouds on the Outer Edge
13. One-dimensional and 3D Models as Planetary Habitability Assessment Tools
14. An Appraisal of Superhabitability
15. Rethinking the Habitable Zone
15.1. Does the Habitable Zone Really Only Assess “Earth-like” Life?
15.2. The Classical HZ Should be Complemented with Other HZ Definitions
15.3. Recommendations for Using the HZ to Size Telescopes and Find Life
15.4. The HZ as a Navigational Filter
16. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Kasting, J.; Whitmire, D.; Raynolds, R. Habitable Zones around Main Sequence Stars. Icarus 1993, 101, 108–128. [Google Scholar] [CrossRef] [PubMed]
- Ramirez, R.M.; Kaltenegger, L. A Methane Extension to the Classical Habitable Zone. Astrophys. J. 2018, 858, 72. [Google Scholar] [CrossRef] [Green Version]
- Heng, K. The Imprecise Search for Extraterrestrial Habitability. Am. Sci. 2016, 104, 146–149. [Google Scholar]
- Benner, S.A.; Ricardo, A.; Carrigan, M.A. Is there a common chemical model for life in the universe? Curr. Opin. Chem. Biol. 2004, 8, 672–689. [Google Scholar] [CrossRef] [PubMed]
- Schulze-Makuch, D.; Irwin, L.N. The prospect of alien life in exotic forms on other worlds. Naturwissenschaften 2006, 93, 155–172. [Google Scholar] [CrossRef] [PubMed]
- Bains, W. Many chemistries could be used to build living systems. Astrobiology 2004, 4, 137–167. [Google Scholar] [CrossRef] [PubMed]
- Abe, Y.; Abe-Ouchi, A.; Sleep, N.H.; Zahnle, K.J. Habitable zone limits for dry planets. Astrobiology 2011, 11, 443–460. [Google Scholar] [CrossRef] [PubMed]
- Zsom, A.; Seager, S.; de Wit, J.; Stamenković, V. Toward the Minimum Inner Edge Distance of the Habitable Zone. Astrophys. J. 2013, 778, 109. [Google Scholar] [CrossRef]
- Sagan, C.; Salpeter, E.E. Particles, Environments and Possible Ecologies in the Jovian Atmosphere. Astrophys. J. Suppl. Ser. 1976, 32, 737–755. [Google Scholar] [CrossRef]
- Sagan, C. Cosmos, 1st ed.; Random House: New York City, NY, USA, 1980; ISBN 0-394-50294-9. [Google Scholar]
- Clarke, A. Is there a Universal Temperature Dependence of metabolism? Funct. Ecol. 2004, 18, 252–256. [Google Scholar] [CrossRef] [Green Version]
- Bains, W.; Xiao, Y.; Yu, C. Prediction of the Maximum Temperature for Life Based on the Stability of Metabolites to Decomposition in Water. Life 2015, 5, 1054–1100. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Goldblatt, C.; Robinson, T.D.; Zahnle, K.J.; Crisp, D. Low simulated radiation limit for runaway greenhouse climates. Nat. Geisci. 2013, 6, 661–667. [Google Scholar] [CrossRef]
- Gonzalez, G.; Brownlee, D.; Ward, P. The Galactic Habitable Zone: Galactic Chemical Evolution. Icarus 2001, 152, 185–200. [Google Scholar] [CrossRef] [Green Version]
- Lineweaver, C.H.; Fenner, Y.; Gibson, B.K. The galactic habitable zone and the age distribution of complex life in the Milky Way. Science 2004, 303, 59–62. [Google Scholar] [CrossRef] [PubMed]
- Heller, R. Exomoon habitability constrained by energy flux and orbital stability. Astron. Astrophys. 2012, 545. [Google Scholar] [CrossRef] [Green Version]
- Mcmahon, S.; Malley-james, J.O.; Parnell, J. Circumstellar habitable zones for deep terrestrial biospheres. Planet. Space Sci. 2013, 85, 312–318. [Google Scholar] [CrossRef] [Green Version]
- Watson, A.; Donahue, T.; Walker, J. The dynamics of a rapidly escaping atmosphere: Applications to the evolution of Earth and Venus. Icarus 1981, 48, 150–166. [Google Scholar] [CrossRef]
- Bains, W.; Seager, S.; Zsom, A. Photosynthesis in Hydrogen-Dominated Atmospheres. Life 2014, 4, 716–744. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kan, S.J.; Lewis, R.D.; Chen, K.; Arnold, F.H. Directed evolution of cytochrome c for carbon-silicon bond formation: Bringing silicon to life. Science 2016, 354, 1048–1051. [Google Scholar] [CrossRef] [PubMed]
- Ingersoll, A.P. The Runaway Greenhouse: A History of Water on Venus1. J. Atmos. Sci. 1969, 26, 1191–1198. [Google Scholar] [CrossRef]
- Ramirez, R.M. Terrestrial Planets under Extreme Radiative Forcings: Applications to Habitable Zones, Early Mars, and a High-CO2 Earth. Ph.D. Thesis, Pennsylvania State University, State College, PA, USA, 2014. [Google Scholar]
- Zahnle, K.J.; Kasting, J.F. Mass fractionation during transonic escape and implications for loss of water from Mars and Venus. Icarus 1986, 68, 462–480. [Google Scholar] [CrossRef]
- Wordsworth, R.; Pierrehumbert, R. Abiotic Oxygen-Dominated Atmospheres on Terrestrial Habitable Zone Planets. Astrophys. J. 2014, 785, L20. [Google Scholar] [CrossRef]
- Leconte, J.; Forget, F.; Charnay, B.; Wordsworth, R.; Pottier, A. Increased insolation threshold for runaway greenhouse processes on Earth-like planets. Nature 2013, 504, 268–271. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kopparapu, R.K.; Ramirez, R.; Kasting, J.F.; Eymet, V.; Robinson, T.D.; Mahadevan, S.; Terrien, R.C.; Domagal-Goldman, S.; Meadows, V.; Deshpande, R. Habitable Zones around Main-Sequence Stars: New Estimates. Astrophys. J. 2013, 765, 131. [Google Scholar] [CrossRef]
- Kopparapu, R.; Wolf, E.T.; Arney, G.; Batalha, N.E.; Haqq-Misra, J.; Grimm, S.L.; Heng, K. Habitable moist atmospheres on terrestrial planets near the inner edge of the habitable zone around M dwarfs. Astrophys. J. 2017, 845, 1. [Google Scholar] [CrossRef]
- Wolf, E.T.; Toon, O.B. Journal of Geophysical Research: Atmospheres the brightening Sun. J. Geophys. Res. Atmos. 2015, 120, 5775–5794. [Google Scholar] [CrossRef]
- Kasting, J.F.; Chen, H.; Kopparapu, R. Stratospheric temperatures and water loss from moist greenhouse atmospheres of Earth-like planets. Astrophys. J. Lett. 2015, 813. [Google Scholar] [CrossRef]
- Fujii, Y.; Del Genio, A.D.; Amundsen, D.S. NIR-driven Moist Upper Atmospheres of Synchronously Rotating Temperate Terrestrial Exoplanets. Astrophys. J. 2017, 848. [Google Scholar] [CrossRef]
- Ramirez, R.M. The moist greenhouse is sensitive to stratospheric temperature. Res. Notes AAS 2018, 2, 1. [Google Scholar] [CrossRef]
- Korenaga, J. Initiation and Evolution of Plate Tectonics on Earth: Theories and Observations. Annu. Rev. Earth Planet. Sci. 2013, 41, 117–151. [Google Scholar] [CrossRef]
- Walker, J.; Hays, P.; Kasting, J. A negative feedback mechanism for the long-term stabilization of Earth’s surface temperature. J. Geophys. Res. Oceans Banner 1981, 86, 9776–9782. [Google Scholar] [CrossRef]
- Kasting, J.F.; Catling, D. Evolution of a habitable planet. Annu. Rev. Astron. Astrophys. 2003, 41, 429–463. [Google Scholar] [CrossRef]
- Noack, L.; Godolt, M.; von Paris, P.; Plesa, A.-C.; Stracke, B.; Dreur, D.; Rauer, H. Can the interior structure influence the habitability of a rocky planet? Planet. Space Sci. 2014, 98, 14–29. [Google Scholar] [CrossRef]
- Hart, M.H. Habitable zones about main-sequence stars. Icarus 1979, 1, 351–357. [Google Scholar] [CrossRef]
- Kasting, J. Runaway and Moist Greenhouse Atmospheres and the Evolution of Earth and Venus. Icarus 1988, 74, 472–494. [Google Scholar] [CrossRef]
- Forget, F.; Pierrehumbert, R.T. Warming early Mars with carbon dioxide clouds that scatter infrared radiation. Science 1997, 287. [Google Scholar] [CrossRef]
- Forget, F.; Wordsworth, R.; Millour, E.; Madeleine, J.-B.; Kerber, L.; Leconte, J.; Marcq, E.; Haberle, R.M. 3D modelling of the early martian climate under a denser CO2 atmosphere: Temperatures and CO2 ice clouds. Icarus 2013, 222, 81–99. [Google Scholar] [CrossRef]
- Kitzmann, D. Clouds in the atmospheres of extrasolar planets. V. The impact of CO2 ice clouds on the outer boundary of the habitable zone. Astron. Astrophys. 2017, 600. [Google Scholar] [CrossRef]
- Feulner, G. The faint young sun problem. Rev. Geophys. 2012, 50. [Google Scholar] [CrossRef]
- Sheldon, N.D. Precambrian paleosols and atmospheric CO2 levels. Precambrian Res. 2006, 147, 148–155. [Google Scholar] [CrossRef]
- Rosing, M.T.; Bird, D.K.; Sleep, N.H.; Bjerrum, C.J. No climate paradox under the faint early Sun. Nature 2010, 464, 744–747. [Google Scholar] [CrossRef] [PubMed]
- Wolf, E.T.; Toon, O.B. Fractal organic hazes provided an ultraviolet shield for early Earth. Science 2010, 328, 1266–1268. [Google Scholar] [CrossRef] [PubMed]
- Kasting, J.F. Atmospheric science. How was early Earth kept warm? Science 2013, 339, 44–45. [Google Scholar] [CrossRef] [PubMed]
- Wordsworth, R.; Pierrehumbert, R. Hydrogen-nitrogen greenhouse warming in Earth’s early atmosphere. Science 2013, 339, 64–67. [Google Scholar] [CrossRef] [PubMed]
- Kanzaki, Y.; Murakami, T. Estimates of atmospheric CO2 in the Neoarchean–Paleoproterozoic from paleosols. Geochim. Cosmochim. Acta 2015, 159, 190–219. [Google Scholar] [CrossRef]
- Gough, D. Solar interior structure and luminosity variations. Sol. Phys. 1981, 74, 21–34. [Google Scholar] [CrossRef]
- Kopparapu, R.K.; Ramirez, R.M.; Schottelkotte, J.; Kasting, J.F.; Domagal-Goldman, S.; Eymet, V. Habitable zones around main-sequence stars: Dependence on planetary mass. Astrophys. J. Lett. 2014, 787. [Google Scholar] [CrossRef]
- Hamano, K.; Abe, Y.; Genda, H. Emergence of two types of terrestrial planet on solidification of magma ocean. Nature 2013, 497, 607–610. [Google Scholar] [CrossRef] [PubMed]
- Ramirez, R.M.; Kaltenegger, L. The habitable zones of pre-main-sequence stars. Astrophys. J. Lett. 2014, 797. [Google Scholar] [CrossRef]
- Donahue, T.M.; Hoffman, J.H.; Hodges, R.R.; Watson, A.J. Venus Was Wet: A Measurement of the Ratio of Deuterium to Hydrogen. Science 1982, 216, 630–633. [Google Scholar] [CrossRef] [PubMed]
- Elkins-Tanton, L.T. Linked magma ocean solidification and atmospheric growth for Earth and Mars. Earth Planet. Sci. Lett. 2008, 271, 181–191. [Google Scholar] [CrossRef]
- Lebrun, T.; Massol, H.; Chassefière, E.; Davaille, A.; Marcq, E.; Sarda, P.; Leblanc, F.; Brandeis, G. Thermal evolution of an early magma ocean in interaction with the atmosphere. J. Geophys. Res. Planets 2013, 118, 1155–1176. [Google Scholar] [CrossRef] [Green Version]
- Schaefer, L.; Wordsworth, R.D.; Berta-Thompson, Z.; Sasselov, D. Predictions of the atmospheric composition of GJ 1132b. Astrophys. J. 2016, 829, 63. [Google Scholar] [CrossRef]
- Carr, M.H. Volcanism on Mars. J. Geophys. Res. 1973, 78, 4049–4062. [Google Scholar] [CrossRef]
- Tian, F.; Kasting, J.F.; Solomon, S.C. Thermal escape of carbon from the early Martian atmosphere. Geophys. Res. Lett. 2009, 36. [Google Scholar] [CrossRef] [Green Version]
- Acuña, M.H.; Connerney, J.E.P.; Ness, N.F.; Lin, R.P.; Mitchell, D.; Carlson, C.W.; McFadden, K.A.; Anderson, H.; Mazelle, C.; Vignes, D.; et al. Global Distribution of Crustal Magnetization Discovered by the Mars Global Surveyor MAG/ER Experiment. Science 1999, 284, 790–793. [Google Scholar] [PubMed]
- Lillis, R.J.; Robbins, S.; Manga, M.; Halekas, J.S.; Frey, H.V. Time history of the Martian dynamo from crater magnetic field analysis. J. Geophys. Res. Planets 2013, 118, 1488–1511. [Google Scholar] [CrossRef] [Green Version]
- Milbury, C.; Schubert, G.; Raymond, C.A.; Smrekar, S.E.; Langlais, B. The history of Mars’ dynamo as revealed by modeling magnetic anomalies near Tyrrhenus Mons and Syrtis Major. J. Geophys. Res. 2012, 117. [Google Scholar] [CrossRef]
- Connerney, J.E. Magnetic Lineations in the Ancient Crust of Mars. Science 1999, 284, 794–798. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Connerney, J.E.P.; Acuna, M.; Ness, N.; Kletetschka, G.; Mitchel, D.; Lin, R.; Reme, H. Tectonic implications of Mars crustal magnetism. Proc. Natl. Acad. Sci. USA 2005, 102, 14970–14975. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Noack, L.; Breur, D. Plate tectonics on rocky exoplanets: Influence of initial conditions and mantle rheology. Planet. Space Sci. 2014, 98, 41–419. [Google Scholar] [CrossRef]
- Stamenković, V.; Höink, T.; Lenardic, A. The importance of temporal stress variation and dynamic disequilibrium for the initiation of plate tectonics. J. Geophys. Res. Planets 2016, 121, 896–915. [Google Scholar] [CrossRef] [Green Version]
- O’Neill, C.; Lenardic, A. Geological consequences of super-sized Earths. Geophys. Res. Lett. 2007, 34. [Google Scholar] [CrossRef] [Green Version]
- Valencia, D.; O’Connell, R.J.; Sasselov, D.D. Inevitability of Plate Tectonics on Super-Earths. Astrophys. J. 2007, 670, L45–L48. [Google Scholar] [CrossRef]
- Craddock, R.A. The case for rainfall on a warm, wet early Mars. J. Geophys. Res. 2002, 107, 5111. [Google Scholar] [CrossRef]
- Ramirez, R.M.; Craddock, R.A. The geological and climatological case for a warmer and wetter early Mars. Nat. Geosci. 2018, 11, 230–237. [Google Scholar] [CrossRef]
- Hynek, B.M.; Beach, M.; Hoke, M.R.T. Updated global map of Martian valley networks and implications for climate and hydrologic processes. J. Geophys. Res. 2010, 115, E09008. [Google Scholar] [CrossRef]
- Rodriguez, J.A.P.; Fairén, A.G.; Tanaka, K.L.; Zarroca, M.; Linares, R.; Platz, T.; Komatsu, G.; Miyamoto, H.; Kargel, J.S.; Jianguo, Y.; et al. Tsunami waves extensively resurfaced the shorelines of an early Martian ocean. Nat. Sci. Rep. 2016, 6. [Google Scholar] [CrossRef] [PubMed]
- Luo, W.; Cang, X.; Howard, A.D. New Martian valley network volume estimate climate consistent with ancient ocean and warm and wet climate. Nat. Commun. 2017, 8, 1–7. [Google Scholar] [CrossRef] [PubMed]
- Pollack, J.B.; Kasting, J.F.; Richardson, S.M.; Poliakoff, K. The case for a wet, warm climate on early Mars. Icarus 1987, 71, 203–224. [Google Scholar] [CrossRef] [Green Version]
- Kranendonk, M.J. Van Two types of arcean continental crust: Plume and plate tectonics on early Earth. Am. J. Sci. 2010, 310, 1187–1209. [Google Scholar] [CrossRef]
- Valley, J.W.; Peck, W.H.; King, E.M.; Wilde, S.A. A cool early Earth. Geology 2002, 30. [Google Scholar] [CrossRef]
- Foley, B.J.; Smye, A.J. Carbon cycling and habitability of Earth-size stagnant lid planets. arXiv 2017, arXiv:1712.03614. [Google Scholar]
- Kadoya, S.; Tajika, E. Conditions for Oceans on Earth-like Planets Orbiting within the Habitable Zone: Importance of Volcanic CO2 Degassing. Astrophys. J. 2014, 790. [Google Scholar] [CrossRef]
- Menou, K. Climate stability of habitable Earth-like planets. Earth Planet. Sci. Lett. 2015, 429, 20–24. [Google Scholar] [CrossRef] [Green Version]
- Batalha, N.E.; Kopparapu, R.K.; Haqq-Misra, J.; Kasting, J.F. Climate cycling on early Mars caused by the carbonate-silicate cycle. Earth Planet. Sci. Lett. 2016, 455, 7–13. [Google Scholar] [CrossRef]
- Haqq-Misra, J.; Kopparapu, R.K.; Batalha, N.E.; Harman, C.E.; Kasting, J.F. Limit Cycles Can Reduce the Width of the Habitable Zone. Astrophys. J. 2016, 827. [Google Scholar] [CrossRef]
- Paradise, A.; Menou, K. GCM Simulations of Unstable Climates in the Habitable Zone. Astrophys. J. 2017, 848, 33. [Google Scholar] [CrossRef] [Green Version]
- Berner, R.A.; Kothvala, Z. Geocarb III: A Revised Model of Atmospheric CO2 over Phanerozoic Time. Am. J. Sci. 2001, 301, 182–204. [Google Scholar] [CrossRef]
- Lasaga, A. Chemical kinetics of water-rock interactions. J. Geophys. Res. 1984, 89, 4009–4025. [Google Scholar] [CrossRef]
- Schwartzman, D.W.; Volk, T. Biotic enhancement of weathering and the habitability of Earth. Nature 1989, 340, 457–460. [Google Scholar] [CrossRef]
- Asolekar, S.R.; Valentine, R.L.; Schnoor, J.L. Kinetics of chemical weathering in B horizon spodosol fraction. Water Resour. Res. 1964, 27, 527–532. [Google Scholar] [CrossRef]
- Hoffman, P.F.; Kaufman, A.J.; Halverson, G.P.; Schrag, D.P. A Neoproterozoic Snowball Earth. Science 1998, 281. [Google Scholar] [CrossRef]
- Hoffman, P.F.; Schrag, D.P. The snowball Earth hypothesis: Testing the limits of global change. Terra Nova 2002, 14, 129–155. [Google Scholar] [CrossRef]
- Kasting, J.F. When methane made climate. Sci. Am. 2004, 291, 78–85. [Google Scholar] [CrossRef] [PubMed]
- Davis, J.M.; Balme, M.; Grindrod, P.M.; Williams, R.M.E.; Gupta, S. Extensive Noachian fluvial systems in Arabia Terra: Implications for early Martian climate. Geology 2016, 44, 847–850. [Google Scholar] [CrossRef] [Green Version]
- Hynek, B. Research Focus: The great climate paradox of ancient Mars. Geology 2016, 44, 879–880. [Google Scholar] [CrossRef]
- Kite, E.S.; Halevy, I.; Kahre, M.A.; Wolff, M.J.; Manga, M. Seasonal melting and the formation of sedimentary rocks on Mars, with predictions for the Gale Crater mound. Icarus 2013, 223, 181–210. [Google Scholar] [CrossRef] [Green Version]
- Hu, R.; Kass, D.M.; Ehlmann, B.L.; Yung, Y.L. Tracing the fate of carbon and the atmospheric evolution of Mars. Nat. Commun. 2015, 6, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Kurokawa, H.; Kurosawa, K.; Usui, T. A Lower Limit of Atmospheric Pressure on Early Mars Inferred from Nitrogen and Argon Isotopic Compositions. Icarus 2018, 299, 443–459. [Google Scholar] [CrossRef]
- Ramirez, R.M. A warmer and wetter solution for early Mars and the challenges with transient warming. Icarus 2017, 297. [Google Scholar] [CrossRef]
- Turbet, M.; Forget, F.; Leconte, J.; Charnay, B.; Tobie, G. CO2 condensation is a serious limit to the deglaciation of Earth-like planets. Earth Planet. Sci. Lett. 2017, 476, 11–21. [Google Scholar] [CrossRef]
- Morris, S.C.; Darwin, C. The Cambrian “explosion”: Slow-fuse or megatonnage? Proc. Natl. Acad. Sci. USA 2000, 97, 4426–4429. [Google Scholar] [CrossRef]
- Catling, D.C.; Glein, C.R.; Zahnle, K.J.; Mckay, C.P. Why O2 is required by complex life on habitable planets and the concept of planetary “oxygenation time”. Astrobiology 2005, 5, 415–438. [Google Scholar] [CrossRef] [PubMed]
- Danovaro, R.; Dell’Anno, A.; Pusceddu, A.; Gambi, C.; Heiner, I.; Kristensen, R.M. The first metazoa living in permanently anoxic conditions. BMC Biol. 2010, 8. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Luo, G.; Ono, S.; Beukes, N.J.; Wang, D.T.; Xie, S.; Summons, R.E. Rapid oxygenation of Earth’s atmosphere 2.33 billion years ago. Sci. Adv. 2016, 2. [Google Scholar] [CrossRef] [PubMed]
- Mills, D.B.; Canfield, D.E. Oxygen and animal evolution: Did a rise of atmospheric oxygen “trigger” the origin of animals? Bioessays 2014, 36, 1145–1155. [Google Scholar] [CrossRef] [PubMed]
- Lyons, T.W.; Reinhard, C.; Planavsky, N.J. The rise of oxygen in Earth’s early ocean and atmosphere. Nature 2014, 506. [Google Scholar] [CrossRef] [PubMed]
- Narbonne, G.M. THE EDIACARA BIOTA: Neoproterozoic Origin of Animals and Their Ecosystems. Annu. Rev. Earth Planet. Sci. 2005, 33, 421–442. [Google Scholar] [CrossRef]
- Müller, W.E.G.; Wang, X.; Schröder, H.C. Paleoclimate and Evolution: Emergence of Sponges during the Neoproterozoic. In Biosilica in Evolution, Morphogenesis, and Nanobiotechnology; Springer: Berlin/Heidelberg, Germany, 2009; pp. 55–77. [Google Scholar]
- Wray, G.A.; Levinton, J.S.; Shapiro, L.H. Molecular evidence for deep Precambrian divergences among metazoan phyla. Science 1996, 274, 568–573. [Google Scholar] [CrossRef]
- Bromhom, L.; Rambaut, A.; Fortey, R.; Cooper, A.; Penny, D. Testing the Cambrian explosion hypothesis by using a molecular dating technique. Proc. Natl. Acad. Sci. USA 1998, 95, 12386–12389. [Google Scholar] [CrossRef] [Green Version]
- Bains, W.; Schulze-Makuch, D. The Cosmic Zoo: The (Near) Inevitability of the Evolution of Complex, Macroscopic Life. Life 2016, 6, 1–27. [Google Scholar] [CrossRef] [PubMed]
- Meadows, V.S. Reflections on O2 as a Biosignature in Exoplanetary Atmospheres. Astrobiology 2017, 17, 1022–1052. [Google Scholar] [CrossRef] [PubMed]
- Meadows, V.S.; Reinhard, C.T.; Arney, G.N.; Parenteau, M.N.; Schwieterman, E.W.; Domagal-Goldman, S.D.; Lincowski, A.P.; Stapelfeldt, K.R.; Rauer, H.; DasSarma, S.; et al. Exoplanet Biosignatures: Understanding Oxygen as a Biosignature in the Context of Its Environment. arXiv 2017, arXiv:1705.07560. [Google Scholar] [CrossRef] [PubMed]
- Kaltenegger, L.; Traub, W.A.; Jucks, K.W. Spectral Evolution of an Earth-like Planet. Astrophys. J. 2007, 658, 598–616. [Google Scholar] [CrossRef]
- Pavlov, A.; Kasting, J.; Brown, L.; Freedman, R. Greenhouse warming by CH4 in the atmosphere of early Earth. J. Geophys. Res. 2000, 105, 11981–11990. [Google Scholar] [CrossRef] [PubMed]
- Ward, P.D.; Brownlee, D. Rare Earth: Why Complex Life Is Rare in the Universe; Copernicus, Springer: New York, NY, USA, 2000. [Google Scholar]
- Mckay, C.P. Requirements and limits for life in the context of exoplanets. Proc. Natl. Acad. Sci. USA 2014, 111, 12628–12633. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Krissansen-Totton, J.; Olson, S.; Catling, D.C. Disequilibrium biosignatures over Earth history and implications for detecting exoplanet life. Sci. Adv. 2018, 4. [Google Scholar] [CrossRef] [PubMed]
- Chassefiere, E. Hydrodynamic escape of hydrogen from a hot water-rich atmosphere: The case of Venus At low. J. Geophys. Res. 1996, 101, 26039–26056. [Google Scholar] [CrossRef]
- Luger, R.; Barnes, R. Extreme water loss and abiotic O2 buildup on planets throughout the habitable zones of M dwarfs. Astrobiology 2015, 15, 119–143. [Google Scholar] [CrossRef] [PubMed]
- Tian, F. History of water loss and atmospheric O2 buildup on rocky exoplanets near M dwarfs. Earth Planet. Sci. Lett. 2015, 432, 126–132. [Google Scholar] [CrossRef]
- Wordsworth, R.D.; Schaefer, L.K.; Fischer, R.A. Redox Evolution via Gravitational Differentiation on Low-mass Planets: Implications for Abiotic Oxygen, Water Loss, and Habitability. Astron. J. 2018, 155. [Google Scholar] [CrossRef]
- Odert, P.; Lammer, H.; Erkaev, N.V.; Nikolaou, A.; Lichtenegger, H.I.M.; Johnstone, C.P.; Kislyakova, K.G.; Leitzinger, M.; Tosi, N. Escape and fractionation of volatiles and noble gases from Mars-sized planetary embryos and growing protoplanets. Icarus 2018, 307, 327–346. [Google Scholar] [CrossRef] [Green Version]
- Rosenqvist, J.; Chassefière, E. Inorganic chemistry of O2 in a dense primitive atmosphere. Planet. Space Sci. 1995, 43, 3–10. [Google Scholar] [CrossRef]
- Hu, R.; Seager, S.; Bains, W. Photochemistry in Terrestrial Exoplanet Atmospheres. I. Photochemistry Model and Benchmark Cases. Astrophys. J. 2012, 761. [Google Scholar] [CrossRef]
- Domagal-Goldman, S.D.; Segura, A.; Claire, M.W.; Robinson, T.D.; Meadows, V.S. Abiotic Ozone and Oxygen in Atmospheres Similar to Prebiotic Earth. Astrophys. J. 2014, 792. [Google Scholar] [CrossRef]
- Tian, F.; France, K.; Linsky, J.L.; Mauas, P.J.D.; Vieytes, M.C. High stellar FUV/NUV ratio and oxygen contents in the atmospheres of potentially habitable planets. Earth Planet. Sci. Lett. 2014, 385, 22–27. [Google Scholar] [CrossRef] [Green Version]
- Harman, C.E.; Schwieterman, E.W.; Schottelkotte, J.C.; Kasting, J.F. Abiotic O2 Levels On Planets Around F, G, K, And M Stars: Possible False Positives For Life? Astrophys. J. 2015, 812, 2. [Google Scholar] [CrossRef]
- Gao, P.; Hu, R.; Robinson, T.D.; Li, C.; Yung, Y.L. Stability of CO2 Atmospheres on Desiccated M Dwarf Exoplanets. Astrophys. J. 2015, 806. [Google Scholar] [CrossRef]
- Halliwell, B.; Gutteridge, J. Oxygen toxicity, oxygen radicals, transition metals and disease. Biochem. J. 1984, 219, 1–14. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chawla, A.; Lavania, A.K. Oxygen toxicity. Med. J. Armed Forces India 2001, 57, 131–133. [Google Scholar] [CrossRef]
- Fogg, M.J. Dynamics of a terraformed Martian biosphere. Br. Interplanet. Soc. 1993, 46, 293–304. [Google Scholar]
- Belcher, C.M.; McElwain, J.C. Limits for combustion in low O2 redefine paleoatmospheric predictions for the Mesozoic. Science 2008, 321, 1197–1200. [Google Scholar] [CrossRef] [PubMed]
- Gerardi, M.H. The Microbiology of Anaerobic Digesters; Wiley-Interscience: Hoboken, NJ, USA, 2003. [Google Scholar]
- Pierrehumbert, R.; Gaidos, E. Hydrogen greenhouse planets beyond the habitable zone. Astrophys. J. Lett. 2011, 734, 1–5. [Google Scholar] [CrossRef]
- Danchi, W.C.; Lopez, B. Effect of Metallicity on the Evolution of the Habitable Zone from the Pre-Main Sequence To the Asymptotic Giant Branch and the Search for Life. Astrophys. J. 2013, 769, 27. [Google Scholar] [CrossRef]
- Ramirez, R.M.; Kaltenegger, L. Habitable zones of post-main sequence stars. Astrophys. J. 2016, 823. [Google Scholar] [CrossRef]
- Ramirez, R.M.; Kaltenegger, L. A Volcanic Hydrogen Habitable Zone. Astrophys. J. Lett. 2017, 837. [Google Scholar] [CrossRef] [Green Version]
- Mojzsis, S.J.; Arrhenius, G.; McKeegan, K.D.; Harrison, T.M.; Nutman, A.P.; Friend, C.R.L. Evidence for life on Earth before 3,800 million years ago. Nature 1997, 386. [Google Scholar] [CrossRef]
- Ramirez, R.M.; Abbot, D.S.; Airapetian, V.; Fujii, Y.; Hamano, K.; Levi, A.; Robinson, T.D.; Schaefer, L.; Wolf, E.; Wordsworth, R.D. The continued importance of habitability studies. arXiv 2018, arXiv:1803.00215. [Google Scholar]
- Stark, C.C.; Roberge, A.; Mandell, A.; Clampin, M.; Domagal-Goldman, S.D.; McElwain, M.W.; Stapelfeldt, K.R. Lower Limits on Aperture Size for an ExoEarth Detecting Coronagraphic Mission. Astrophys. J. 2015, 808. [Google Scholar] [CrossRef]
- Stevenson, D.J. Life-sustaining planets in interstellar space? Nature 1999, 400. [Google Scholar] [CrossRef] [PubMed]
- Sagan, C.; Mullen, G. Earth and Mars: Evolution of Atmospheres and Surface Temperatures. Science 1972, 177, 52–56. [Google Scholar] [CrossRef] [PubMed]
- Wordsworth, R. Transient conditions for biogenesis on low-mass exoplanets with escaping hydrogen atmospheres. Icarus 2012, 219, 267–273. [Google Scholar] [CrossRef] [Green Version]
- Abbot, D.S. A Proposal for Climate Stability on H2-greenhouse Planets. Astrophys. J. Lett. 2015, 815. [Google Scholar] [CrossRef]
- Ramirez, R.M.; Kopparapu, R.; Zugger, M.E.; Robinson, T.D.; Freedman, R.; Kasting, J.F. Warming early Mars with CO2 and H2. Nat. Geosci. 2014, 7, 59–63. [Google Scholar] [CrossRef]
- Wordsworth, R.; Kalugina, Y.; Lokshtanov, S.; Vigasin, A.; Ehlmann, B.; Head, J.; Sanders, C.; Wang, H. Transient reducing greenhouse warming on early Mars. Geophys. Res. Lett. 2017, 44, 665–671. [Google Scholar] [CrossRef] [Green Version]
- Gruszka, M.; Borysow, A. Roto-Translational Collision-Induced Absorption of CO2 for the Atmosphere of Venus at Frequencies from 0 to 250 cm−1, at Temperatures from 200 to 800 K. Icarus 1997, 129, 172–177. [Google Scholar] [CrossRef]
- Gruszka, M.; Borysow, A. Computer simulation of the far infrared collision induced absorption spectra of gaseous CO2. Mol. Phys. 1998, 93, 1007–1016. [Google Scholar] [CrossRef]
- Baranov, Y.I.; Lafferty, W.J.; Fraser, G.T. Infrared spectrum of the continuum and dimer absorption in the vicinity of the O2 vibrational fundamental in O2/CO2 mixtures. J. Mol. Spectrosc. 2004, 228, 432–440. [Google Scholar] [CrossRef]
- Trail, D.; Watson, E.B.; Tailby, N.D. The oxidation state of Hadean magmas and implications for early Earth’s atmosphere. Nature 2011, 480, 79–82. [Google Scholar] [CrossRef] [PubMed]
- Grott, M.; Morschhauser, A.; Breuer, D.; Hauber, E. Volcanic outgassing of CO2 and H2O on Mars. Earth Planet. Sci. Lett. 2011, 308, 391–400. [Google Scholar] [CrossRef]
- Wade, J.; Wood, B.J. Core formation and the oxidation state of the Earth. Earth Planet. Sci. Lett. 2005, 236, 78–95. [Google Scholar] [CrossRef]
- Wordsworth, R.D.; Pierrehumbert, R.T. Water Loss from Terrestrial Planets with CO2-Rich Atmospheres. Astrophys. J. 2013, 778, 154. [Google Scholar] [CrossRef]
- Luger, R.; Sestovic, M.; Kruse, E.; Grimm, S.L.; Demory, B.-O.; Agol, E.; Bolmont, E.; Fabrycky, D.; Fernandes, C.S.; Van Grootel, V.; et al. A seven-planet resonant chain in TRAPPIST-1. Nat. Astron. 2017, 1. [Google Scholar] [CrossRef]
- Catling, D.C.; Krissansen-Totton, J.; Kiang, N.Y.; Crisp, D.; Robinson, T.D.; DasSarma, S.; Rushby, A.; Del Genio, A.; Bains, W.; Domagal-Goldman, S. Exoplanet Biosignatures: A Framework for Their Assessment. Astrobiology 2018, 18. [Google Scholar] [CrossRef] [PubMed]
- Mumma, M.J.; Villanueva, G.L.; Novak, R.E.; Hewagama, T.; Bonev, B.P.; Disanti, M.A; Mandell, A.M.; Smith, M.D. Strong release of methane on Mars in northern summer 2003. Science 2009, 323, 1041–1045. [Google Scholar] [CrossRef] [PubMed]
- Seager, S.; Bains, W.; Hu, R. Biosignature Gases in H2-dominated Atmospheres on Rocky Exoplanets. Astrophys. J. 2013, 777. [Google Scholar] [CrossRef]
- Kasting, J.F.; Kopparapu, R.; Ramirez, R.M.; Harman, C.E. Remote life-detection criteria, habitable zone boundaries, and the frequency of Earth-like planets around M and late K stars. Proc. Natl. Acad. Sci. USA 2014, 111, 12641–12646. [Google Scholar] [CrossRef] [PubMed]
- von Paris, P.; Gebauer, S.; Godolt, M.; Grenfell, J.; Hedelt, P.; Kitzmann, D.; Patzer, A.; Rauer, H.; Stracke, B. The extrasolar planet GL 581 d: A potentially habitable planet? Astron. Astrophys. 2010, 522, 1–11. [Google Scholar] [CrossRef]
- Hu, Y.; Ding, F. Radiative constraints on the habitability of exoplanets Gliese 581 c and Gliese 581 d. Astron. Astrophys. 2011, 526, 1–8. [Google Scholar] [CrossRef]
- Wordsworth, R.D.; Selsis, F.; Millour, E.; Charnay, B.; Madeleine, J.; Pierre, I.; Laplace, S. Gliese 581d is the first discovered terrestrial-mass exoplanet in the habitable zone. Astrophys. J. Lett. 2011, 733, 2. [Google Scholar] [CrossRef]
- Kharecha, P.; Kasting, J.F.; Siefert, J. A coupled atmosphere–ecosystem model of the early Archean Earth. Geobiology 2005, 3, 53–76. [Google Scholar] [CrossRef]
- Haqq-Misra, J.D.; Domagal-Goldman, S.D.; Kasting, P.J.; Kasting, J.F. A revised, hazy methane greenhouse for the Archean Earth. Astrobiology 2008, 8, 1127–1137. [Google Scholar] [CrossRef] [PubMed]
- Pierrehumbert, R.T. Principles of Planetary Climate; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar]
- Arney, G.N.; Meadows, V.S.; Domagal-Goldman, S.D.; Deming, D.; Robinson, T.D.; Tovar, G.; Wolf, E.T.; Schwieterman, E. Pale Orange Dots: The Impact of Organic Haze on the Habitability and Detectability of Earthlike Exoplanets. Astrophys. J. 2017, 836. [Google Scholar] [CrossRef]
- Turbet, M.; Bolmont, E.; Leconte, J.; Forget, F.; Selsis, F.; Tobie, G.; Caldas, A.; Naar, J.; Gillon, M. Modeling climate diversity, tidal dynamics and the fate of volatiles on TRAPPIST-1 planets. Astron. Astrophys. 2017, 612. [Google Scholar] [CrossRef]
- Schaefer, L.; Fegley, B., Jr. Chemistry of atmospheres formed during accretion of the Earth and other terrestrial planets. Icarus 2010, 208, 434–448. [Google Scholar] [CrossRef]
- Shaw, G.H. Earth’s atmosphere—Hadean to early Proterozoic. Chemie der Erde 2008, 68, 235–364. [Google Scholar] [CrossRef]
- Guzmán-Marmolejo, A.; Segura, A.; Escobar-Briones, E. Abiotic production of methane in terrestrial planets. Astrobiology 2013, 13, 550–559. [Google Scholar] [CrossRef] [PubMed]
- Chassefière, E.; Langlais, B.; Quesnel, Y.; Leblanc, F. The fate of early Mars’ lost water: The role of serpentinization. J. Geophys. Res. Planets 2013, 118, 1123–1134. [Google Scholar] [CrossRef] [Green Version]
- Shaw, G.H. Earth’s Early Atmosphere and Surface Environment; Geological Society of America: Boulder, CO, USA, 2014. [Google Scholar]
- Etiope, G.; Lollar, B.S. Abiotic Methane on Earth. Rev. Geophys. 2013, 51, 276–299. [Google Scholar] [CrossRef]
- Bohm-Vitense, E.; Woods, J. An attempt to determine stellar Lyman-alpha emission-line fluxes for F stars with different metal abundances. Astrophys. J. 1983, 265, 331–337. [Google Scholar] [CrossRef]
- Landsman, W.; Simon, T. A catalog of stellar Lyman-alpha fluxes. Astrophys. J. 1993, 408, 305–322. [Google Scholar] [CrossRef]
- Segura, A.; Krelove, K.; Kasting, J.F.; Sommerlatt, D.; Meadows, V.; Crisp, D.; Cohen, M.; Mlawer, E. Ozone concentrations and ultraviolet fluxes on Earth-like planets around other stars. Astrobiology 2003, 3, 689–708. [Google Scholar] [CrossRef] [PubMed]
- Rugheimer, S.; Kaltenegger, L.; Zsom, A.; Segura, A.; Sasselov, D. Spectral Fingerprints of Earth-like Planets Around FGK Stars. Astrobiology 2013, 13, 251–269. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Watson, A.J.; Lovelock, J.E. Biological homeostasis of the global environment: The parable of Daisyworld. Tellus B 1983, 35. [Google Scholar] [CrossRef]
- Domagal-Goldman, S.D.; Kasting, J.F.; Johnston, D.T.; Farquhar, J. Organic haze, glaciations and multiple sulfur isotopes in the Mid-Archean Era. Earth Planet. Sci. Lett. 2008, 269, 29–40. [Google Scholar] [CrossRef]
- Toon, O.B.; Pollack, J.B.; Ward, W.; Burns, J.A.; Bilski, K. The astronomical theory of climatic change on Mars. Icarus 1980, 44, 552–607. [Google Scholar] [CrossRef]
- Haberle, R.M.; McKay, C.P.; Tyler, D.; Reynolds, R.T. Can synchronously rotating planets support an atmosphere? In Circumstellar Habitable Zones, Proceedings of the First International Conference; Doyle, L.R., Ed.; Travis House Publications: Menlo Park, CA, USA, 1996; pp. 29–33. [Google Scholar]
- Joshi, M.M.; Haberle, R.M.; Reynolds, R.T. Simulations of the Atmospheres of Synchronously Rotating Terrestrial Planets Orbiting M Dwarfs: Conditions for Atmospheric Collapse and the Implications for Habitability. Icarus 1997, 129, 450–465. [Google Scholar] [CrossRef] [Green Version]
- Leconte, J.; Wu, H.; Menou, K.; Murray, N. Asynchronous rotation of Earth-mass planets in the habitable zone of lower-mass stars. Science 2015, 347, 632–635. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lissauer, J.J. Planets Formed in Habitable Zones of M Dwarf Stars Probably Are Deficient in Volatiles. Astrophys. J. 2007, 660, L149–L152. [Google Scholar] [CrossRef]
- Raymond, S.N.; Scalo, J.; Meadows, V.S. A Decreased Probability of Habitable Planet Formation Around Low-Mass Stars. Astrophys. J. 2007, 669, 606–614. [Google Scholar] [CrossRef]
- Garraffo, C.; Drake, J.J.; Cohen, O. The Space Weather of Proxima Centauri b. Astrophys. J. Lett. 2016, 833. [Google Scholar] [CrossRef]
- Airapetian, V.S.; Glocer, A.; Khazanov, G.V.; Loyd, R.O.P.; France, K.; Sojka, J.; Danchi, W.C.; Liemohn, M.W.; Airapetian, V.S.; Glocer, A.; et al. How Hospitable Are Space Weather Affected Habitable Zones? The Role of Ion Escape. Astrophys. J. Lett. 2017, 836. [Google Scholar] [CrossRef]
- Pavlov, A.K.; Blinov, A.V.; Konstantinov, A.N. Sterilization of Martian surface by cosmic radiation. Planet. Space Sci. 2002, 50, 669–673. [Google Scholar] [CrossRef]
- Tian, F.; Ida, S. Water contents of Earth-mass planets around M dwarfs. Nat. Geosci. 2015, 8, 177–180. [Google Scholar] [CrossRef]
- Baraffe, I.; Homeier, D.; Allard, F.; Chabrier, G. New evolutionary models for pre-main sequence and main sequence low-mass stars down to the hydrogen-burning limit. Astron. Astrophys. 2015, 577. [Google Scholar] [CrossRef] [Green Version]
- Kasting, J.; Pollack, J. Loss of water from Venus. I. Hydrodynamic escape of hydrogen. Icarus 1983, 53, 479–508. [Google Scholar] [CrossRef]
- Ribas, I.; Guinan, E.; Gudel, M.; Audard, M. Evolution of the solar activity over time and effects on planetary atmospheres. I. High-energy irradiances (1-1700 Å). Astrophys. J. 2005, 622, 680–694. [Google Scholar] [CrossRef]
- Gillmann, C.; Chassefière, E.; Lognonné, P. A consistent picture of early hydrodynamic escape of Venus atmosphere explaining present Ne and Ar isotopic ratios and low oxygen atmospheric content. Earth Planet. Sci. Lett. 2009, 286, 503–513. [Google Scholar] [CrossRef]
- Lammer, H.; Odert, P.; Leitzinger, M.; Khodachenko, M.; Panchenko, M.; Kulikov, Y.; Zhang, T.; Lichtenegger, H.; Erkaev, N.; Wuchterl, G.; et al. Determining the mass loss limit for close-in exoplanets: What can we learn from transit observations? Astron. Astrophys. 2009, 506, 399–410. [Google Scholar] [CrossRef]
- Bolmont, E.; Selsis, F.; Owen, J.E.; Ribas, I.; Raymond, S.N.; Leconte, J.; Gillon, M. Water loss from terrestrial planets orbiting ultracool dwarfs: Implications for the planets of TRAPPIST-1. Mon. Not. R. Astron. Soc. 2017, 464, 3728–3741. [Google Scholar] [CrossRef]
- Bourrier, V.; Ehrenreich, D.; Wheatley, P.J.; Bolmont, E.; Gillon, M.; de Wit, J.; Burgasser, A.J.; Jehin, E.; Queloz, D.; Triaud, A.H.M.J. Reconnaissance of the TRAPPIST-1 exoplanet system in the Lyman-α line. Astron. Astrophys. 2017, 599. [Google Scholar] [CrossRef]
- Bourrier, V.; de Wit, J.; Bolmont, E.; Stamenković, V.; Wheatley, P.J.; Burgasser, A.J.; Delrez, L.; Demory, B.-O.; Ehrenreich, D.; Gillon, M.; et al. Temporal Evolution of the High-energy Irradiation and Water Content of TRAPPIST-1 Exoplanets. Astron. J. 2017, 154. [Google Scholar] [CrossRef]
- Lorenz, R.D.; Lunine, J.I.; McKay, C.P. Titan under a red giant sun: A new kind of “habitable” moon. Geophys. Res. Lett. 1997, 24, 2905. [Google Scholar] [CrossRef] [PubMed]
- Villaver, E.; Livio, M. Can Planets Survive Stellar Evolution? Astrophys. J. 2007, 661, 1192–1201. [Google Scholar] [CrossRef] [Green Version]
- Veras, D. Post-main-sequence planetary system evolution. R. Soc. Open Sci. 2016, 3. [Google Scholar] [CrossRef] [PubMed]
- Williams, D.M.; Kasting, J.F. Habitable moons around extrasolar giant planets. Nature 1997, 385, 234–236. [Google Scholar] [CrossRef] [PubMed]
- Shields, A.L.; Meadows, V.S.; Bitz, C.M.; Pierrehumbert, R.T.; Joshi, M.M.; Robinson, T.D. The effect of host star spectral energy distribution and ice-albedo feedback on the climate of extrasolar planets. Astrobiology 2013, 13, 715–739. [Google Scholar] [CrossRef] [PubMed]
- Yang, J.; Ding, F.; Ramirez, R.M.; Peltier, W.R.; Hu, Y.; Liu, Y. Abrupt climate transition of icy worlds from snowball to moist or runaway greenhouse. Nat. Geosci. 2017, 10, 556–560. [Google Scholar] [CrossRef]
- von Bloh, W.; Cuntz, M.; Schröder, K.-P.; Bounama, C.; Franck, S. Habitability of Super-Earth Planets Around Other Suns: Models Including Red Giant Branch Evolution. Astrobiology 2009, 9, 593–602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- He, M.Y.; Triaud, A.H.M.J.; Gillon, M. First limits on the occurrence rate of short-period planets orbiting brown dwarfs. Mon. Not. R. Astron. Soc. 2016, 464, 2687–2697. [Google Scholar] [CrossRef] [Green Version]
- Agol, E. Transit Surveys for Earths in the Habitable Zones of White Dwarfs. Astrophys. J. Lett. 2011, 731. [Google Scholar] [CrossRef]
- Fossati, L.; Bagnulo, S.; Haswell, C.A.; Patel, M.R.; Busuttil, R.; Kowalski, P.M.; Shulyak, D.V.; Sterzik, M.F.; Fossati, L.; Bagnulo, S.; et al. The Habitability and Detection of Earth-like Planets Orbiting Cool White Dwarfs. Astrophys. J. Lett. 2012, 757. [Google Scholar] [CrossRef]
- Heller, R.; Barnes, R. Exomoon Habitability Constrained by Illumination and Tidal Heating. Astrobiology 2013, 13, 18–46. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Nordhaus, J.; Spiegel, D.S. On the orbits of low-mass companions to white dwarfs and the fates of the known exoplanets. Mon. Not. R. Astron. Soc. 2013, 432, 500–505. [Google Scholar] [CrossRef] [Green Version]
- Alibert, Y.; Benz, W. Formation and composition of planets around very low mass stars. Astron. Astrophys. 2017, 598. [Google Scholar] [CrossRef] [Green Version]
- Hansen, B.M.S. In situ models for planet assembly around cool stars. Int. J. Astrobiol. 2015, 14, 267–278. [Google Scholar] [CrossRef]
- Unterborn, C.T.; Desch, S.J.; Hinkel, N.R.; Lorenzo, A. Inward migration of the TRAPPIST-1 planets as inferred from their water-rich compositions. Nat. Astron. 2018, 2, 297–302. [Google Scholar] [CrossRef] [Green Version]
- Morbidelli, A.; Bitsch, B.; Crida, A.; Gounelle, M.; Guillot, T.; Jacobson, S.; Johansen, A.; Lambrechts, M.; Lega, E. Fossilized condensation lines in the Solar System protoplanetary disk. Icarus 2016, 267, 368–376. [Google Scholar] [CrossRef] [Green Version]
- Gillon, M.; Triaud, A.H.M.J.; Demory, B.-O.; Jehin, E.; Agol, E.; Deck, K.M.; Lederer, S.M.; de Wit, J.; Burdanov, A.; Ingalls, J.G.; et al. Seven temperate terrestrial planets around the nearby ultracool dwarf star TRAPPIST-1. Nature 2017, 542, 456–460. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Grimm, S.L.; Demory, B.-O.; Gillon, M.; Dorn, C.; Agol, E.; Burdanov, A.; Delrez, L.; Sestovic, M.; Triaud, A.H.M.J.; Turbet, M.; et al. The nature of the TRAPPIST-1 exoplanets. Astron. Astrophys. 2018, 613. [Google Scholar] [CrossRef]
- Quarles, B.; Quintana, E.V.; Lopez, E.; Schlieder, J.E.; Barclay, T. Plausible Compositions of the Seven TRAPPIST-1 Planets Using Long-term Dynamical Simulations. Astrophys. J. Lett. 2017, 842. [Google Scholar] [CrossRef]
- Abbot, D.S.; Cowan, N.B.; Ciesla, F.J. Indication of Insensitivity of Planetary Weathering Behavior and Habitable Zone to Surface Land Fraction. Astrophys. J. 2012, 756. [Google Scholar] [CrossRef]
- Kite, E.S.; Manga, M.; Gaidos, E. Geodynamics and rate of volcanism on massive Earth-like planets. Astrophys. J. 2009, 700. [Google Scholar] [CrossRef]
- Noack, L.; Höning, D.; Rivoldini, A.; Heistracher, C.; Zimov, N.; Journaux, B.; Lammer, H.; Van Hoolst, T.; Bredehöft, J.H. Water-rich planets: How habitable is a water layer deeper than on Earth? Icarus 2016, 277, 215–236. [Google Scholar] [CrossRef]
- Levi, A.; Sasselov, D.; Podolak, M. The Abundance of Atmospheric CO2 in Ocean Exoplanets: A Novel CO2 Deposition Mechanism. Astrophys. J. 2017, 838. [Google Scholar] [CrossRef]
- Kaltenegger, L.; Sasselov, D.; Rugheimer, S. Water-Planets in The Habitable Zone: Atmospheric Chemistry, Observable Features, And The Case of KEPLER-62e and -62f. Astrophys. J. Lett. 2013, 775. [Google Scholar] [CrossRef]
- Ramirez, R.M.; Levi, A. The ice cap zone: A unique habitable zone for ocean worlds. Mon. Not. R. Astron. Soc. 2018, 477, 4627–4640. [Google Scholar] [CrossRef]
- Ramirez, R.M.; Kopparapu, R.K.; Lindner, V.; Kasting, J.F. Can increased atmospheric CO2 levels trigger a runaway greenhouse? Astrobiology 2014, 14, 714–731. [Google Scholar] [CrossRef] [PubMed]
- Lovelock, J.; Whitfield, M. Life span of the biosphere. Nature 1982, 296, 561–563. [Google Scholar] [CrossRef]
- Caldeira, K.; Kasting, J. Susceptibility of the early Earth to irreversible glaciation caused by carbon dioxide clouds. Nature 1992, 359, 226–228. [Google Scholar] [CrossRef] [PubMed]
- O’Malley-James, J.T.; Greaves, J.S.; Raven, J.A.; Cockell, C.S. Swansong biospheres: Refuges for life and novel microbial biospheres on terrestrial planets near the end of their habitable lifetimes. Int. J. Astrobiol. 2013, 12, 99–112. [Google Scholar] [CrossRef]
- Armstrong, D.J.; Osborn, H.P.; Brown, D.J.A.; Faedi, F.; Gómez Maqueo Chew, Y.; Martin, D.V.; Pollacco, D.; Udry, S. On the abundance of circumbinary planets. Mon. Not. R. Astron. Soc. 2014, 444, 1873–1883. [Google Scholar] [CrossRef] [Green Version]
- Quarles, B.; Musielak, Z.E.; Cuntz, M. Habitability of Earth-mass Planets and Moons in the Kepler-16 System. Astrophys. J. 2012, 750. [Google Scholar] [CrossRef]
- Orosz, J.A.; Welsh, W.F.; Carter, J.A.; Fabrycky, D.C.; Cochran, W.D.; Endl, M.; Ford, E.B.; Haghighipour, N.; MacQueen, P.J.; Mazeh, T.; et al. Kepler-47: A Transiting Circumbinary Multiplanet System. Science 2012, 337. [Google Scholar] [CrossRef] [PubMed]
- Eggl, S.; Pilat-Lohinger, E.; Georgakarakos, N.; Gyergyovits, M.; Funk, B. An Analytic Method to Determine Habitable Zones for S-Type Planetary Orbits in Binary Star Systems. Astrophys. J. 2012, 752. [Google Scholar] [CrossRef]
- Eggl, S.; Pilat-Lohinger, E.; Funk, B.; Georgakarakos, N.; Haghighipour, N. Circumstellar habitable zones of binary-star systems in the solar neighbourhood. Mon. Not. R. Astron. Soc. 2013, 428, 3104–3113. [Google Scholar] [CrossRef]
- Kane, S.R.; Hinkel, N.R. On the Habitable Zones of Circumbinary Planetary Systems. Astrophys. J. 2013, 762. [Google Scholar] [CrossRef]
- Kaltenegger, L.; Haghighipour, N. Calculating the Habitable Zone of Binary Star Systems. I. S-type Binaries. Astrophys. J. 2013, 777. [Google Scholar] [CrossRef]
- Haghighipour, N.; Kaltenegger, L. Calculating the Habitable Zone of Binary Star Systems. II. P-type Binaries. Astrophys. J. 2013, 777. [Google Scholar] [CrossRef]
- Forgan, D. Assessing circumbinary habitable zones using latitudinal energy balance modelling. Mon. Not. R. Astron. Soc. 2014, 437, 1352–1361. [Google Scholar] [CrossRef]
- Forgan, D. Milankovitch cycles of terrestrial planets in binary star systems. Mon. Not. R. Astron. Soc. 2016, 463, 2768–2780. [Google Scholar] [CrossRef] [Green Version]
- Raghavan, D.; McAlister, H.A.; Henry, T.J.; Latham, D.W.; Marcy, G.W.; Mason, B.D.; Gies, D.R.; White, R.J.; ten Brummelaar, T.A. A Survey of Stellar Families: Multiplicity of Solar-type Stars. Astrophys. J. Suppl. 2010, 190, 1–42. [Google Scholar] [CrossRef]
- Bate, M.R. Stellar, brown dwarf and multiple star properties from a radiation hydrodynamical simulation of star cluster formation. Mon. Not. R. Astron. Soc. 2012, 419, 3115–3146. [Google Scholar] [CrossRef]
- Duquennoy, A.; Mayor, M. Multiplicity among solar-type stars in the solar neighbourhood. II—Distribution of the orbital elements in an unbiased sample. Astron. Astophys. 1991, 248, 485–524. [Google Scholar]
- Dvorak, R. Critical orbits in the elliptic restricted three-body problem. Astron. Astrophys. 1986, 167, 379–386. [Google Scholar]
- Holman, M.J.; Wiegert, P.A. Long-Term Stability of Planets in Binary Systems. Astron. J. 1999, 117. [Google Scholar] [CrossRef]
- Quarles, B.; Satyal, S.; Kostov, V.; Kaib, N.; Haghighipour, N. Stability Limits of Circumbinary Planets: Is There a Pile-up in the Kepler CBPs? Astrophys. J. 2018, 856. [Google Scholar] [CrossRef]
- Yang, J.; Cowan, N.; Abbot, D. Stabilizing cloud feedback dramatically expands the habitable zone of tidally locked planets. Astrophys. J. Lett. 2013, 771, 2. [Google Scholar] [CrossRef]
- Yang, J.; Boue, G.; Fabrycky, D.C.; Abbot, D.S. Strong Dependence of the Inner Edge of the Habitable Zone on Planetary Rotation Rate. Astrophys. J. 2014, 787. [Google Scholar] [CrossRef]
- Kopparapu, R.; Wolf, E.T.; Haqq-Misra, J.; Yang, J.; Kasting, J.F.; Meadows, V.; Terrien, R.; Mahadevan, S. The Inner Edge of the Habitable Zone for Synchronously Rotating Planets around Low-mass Stars Using General Circulation Models. Astrophys. J. 2016, 819. [Google Scholar] [CrossRef]
- Bin, J.; Tian, F.; Liu, L. New inner boundaries of the habitable zones around M dwarfs. Earth Planet. Sci. Lett. 2018, 492, 121–129. [Google Scholar] [CrossRef]
- Cullum, J.; Stevens, D.; Joshi, M. The importance of planetary rotation period for ocean heat transport. Astrobiology 2014, 14, 645–650. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wolf, E.T.; Toon, O.B. Delayed onset of runaway and moist greenhouse climates for Earth. Geophys. Res. Lett. 2014, 41, 167–172. [Google Scholar] [CrossRef] [Green Version]
- Kopparapu, R.K.; Ramirez, R.M.; SchottelKotte, J.; Kasting, J.F.; Domagal-Goldman, S.; Eymet, V. Habitable Zones Around Main-Sequence Stars: Dependence on Planetary Mass. Astrophys. J. 2014, 787, L29. [Google Scholar] [CrossRef]
- Way, M.J.; Del Genio, A.D.; Kiang, N.Y.; Sohl, L.E.; Grinspoon, D.H.; Aleinov, I.; Kelley, M.; Clune, T. Was Venus the first habitable world of our solar system? Geophys. Res. Lett. 2016, 43, 8376–8383. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wolf, E.T. Assessing the Habitability of the TRAPPIST-1 System Using a 3D Climate Model. Astrophys. J. Lett. 2017, 839. [Google Scholar] [CrossRef]
- Wolf, E.T. Erratum: “Assessing the Habitability of the TRAPPIST-1 System Using a 3D Climate Model” (2017, ApJL, 839, L1). Astrophys. J. Lett. 2018, 855. [Google Scholar] [CrossRef]
- Edson, A.; Lee, S.; Bannon, P.; Kasting, J.F.; Pollard, D. Atmospheric circulations of terrestrial planets orbiting low-mass stars. Icarus 2011, 212, 1–13. [Google Scholar] [CrossRef]
- Mills, S.M.; Abbot, D.S. Utility of the Weak Temperature Gradient Approximation for Earth-like Tidally Locked Exoplanets. Astrophys. J. Lett. 2013, 774. [Google Scholar] [CrossRef]
- Yang, J.; Abbot, D.S. A Low-order Model of Water Vapor, Clouds, and Thermal Emission for Tidally Locked Terrestrial Planets. Astrophys. J. 2014, 784, 155. [Google Scholar] [CrossRef]
- Godolt, M.; Grenfell, J.L.; Kitzmann, D.; Kunze, M.; Langematz, U.; Patzer, A.B.C.; Rauer, H.; Stracke, B. Assessing the habitability of planets with Earth-like atmospheres with 1D and 3D climate modeling. Astron. Astrophys. 2016, 592. [Google Scholar] [CrossRef]
- Vladilo, G.; Murante, G.; Silva, L.; Provenzale, A.; Ferri, G.; Ragazzini, G. The habitable zone of Earth-like planets with different levels of atmospheric pressure. Astrophys. J. 2013, 767, 1–58. [Google Scholar] [CrossRef]
- Vladilo, G.; Silva, L.; Murante, G.; Filippi, L.; Provenzale, A. Modeling the Surface Temperature of Earth-like Planets. Astrophys. J. 2015, 804. [Google Scholar] [CrossRef]
- Marcq, E.; Salvador, A.; Massol, H.; Davaille, A. Thermal radiation of magma ocean planets using a 1-D radiative-convective model of H2O-CO2 atmospheres. J. Geophys. Res. Planets 2017, 122, 1539–1553. [Google Scholar] [CrossRef]
- Salvador, A.; Massol, H.; Davaille, A.; Marcq, E.; Sarda, P.; Chassefière, E. The relative influence of H2O and CO2 on the primitive surface conditions and evolution of rocky planets. J. Geophys. Res. Planets 2017, 122. [Google Scholar] [CrossRef]
- Engin, K.; Grenfell, J.L.; Godolt, M.; Barbara, S.; Heike, R. The Effect of Varying Atmospheric Pressure upon Habitability and Biosignatures of Earth-like Planets. Astrobiology 2018, 18. [Google Scholar] [CrossRef]
- Kaspi, Y.; Showman, A.P. Atmospheric Dynamics of Terrestrial Exoplanets over a Wide Range of Orbital and Atmospheric Parameters. Astrophys. J. 2015, 804. [Google Scholar] [CrossRef]
- Kodama, T.; Nitta, A.; Genda, H.; Takao, Y.; O’ishi, R.; Abe-Ouchi, A.; Abe, Y. Dependence of the Onset of the Runaway Greenhouse Effect on the Latitudinal Surface Water Distribution of Earth-Like Planets. J. Geophys. Res. Planets 2018, 123, 559–574. [Google Scholar] [CrossRef] [Green Version]
- Selsis, F.; Kasting, J.F.; Levrard, B.; Paillet, J.; Ribas, I.; Delfosse, X. Habitable planets around the star Gliese 581? Astron. Astrophys. 2007, 476, 1373–1387. [Google Scholar] [CrossRef] [Green Version]
- Segura, A.; Kasting, J.F.; Meadows, V.; Cohen, M.; Scalo, J.; Crisp, D.; Butler, R.A.H.; Tinetti, G. Biosignatures from Earth-Like Planets Around M Dwarfs. Astrobiology 2005, 5, 706–725. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- O’Malley-James, J.T.; Kaltenegger, L. UV surface habitability of the TRAPPIST-1 system. Mon. Not. R. Astron. Soc. Lett. 2017, 469. [Google Scholar] [CrossRef]
- Rugheimer, S.; Kaltenegger, L. Spectra of Earth-like Planets through Geological Evolution around FGKM Stars. Astrophys. J. 2018, 854. [Google Scholar] [CrossRef]
- Pierrehumbert, R.T.; Brogniez, H.; Roca, R. On the Relative Humidity of the Atmosphere. In The Global Circulation of the Atmosphere; Princeton University Press: Princeton, NJ, USA, 2007; pp. 143–185. [Google Scholar]
- Zsom, A.; Kaltenegger, L.; Goldblatt, C. A 1D microphysical cloud model for Earth, and Earth-like exoplanets: Liquid water and water ice clouds in the convective troposphere. Icarus 2012, 221, 603–616. [Google Scholar] [CrossRef] [Green Version]
- Silva, L.; Vladilo, G.; Schulte, P.M.; Murante, G.; Provenzale, A. From climate models to planetary habitability: Temperature constraints for complex life. Int. J. Astrobiol. 2017, 16, 244–265. [Google Scholar] [CrossRef]
- Heller, R.; Armstrong, J. Superhabitable worlds. Astrobiology 2014, 14, 50–66. [Google Scholar] [CrossRef] [PubMed]
- Berner, R.; Lasaga, A.; Garrels, R. The carbonate-silicate geochemical cycle and its effects on atmospheric carbon dioxide over the past 100 million years. Am. J. Sci. 1983, 283, 641–683. [Google Scholar] [CrossRef]
- Cuntz, M.; Guinan, E.F. About Exobiology: The Case for Dwarf K Stars. Astrophys. J. 2016, 827. [Google Scholar] [CrossRef]
- Coughlin, J.L.; Mullally, F.; Thompson, S.E.; Rowe, J.F.; Burke, C.J.; Latham, D.W.; Batalha, N.M.; Ofir, A.; Quarles, B.L.; Henze, C.E.; et al. Planetary Candidates Observed by Kepler. VII. The First Fully Uniform Catalog Based on the Entire 48-month Data Set (Q1-Q17 DR24). Astrophys. J. Suppl. Ser. 2016, 224. [Google Scholar] [CrossRef]
- Borucki, W.J.; Koch, D.; Basri, G.; Batalha, N.; Brown, T.; Caldwell, D.; Caldwell, J.; Christensen-Dalsgaard, J.; Cochran, W.D.; DeVore, E.; et al. Kepler Planet-Detection Mission: Introduction and First Results. Science 2010, 327. [Google Scholar] [CrossRef] [PubMed]
- Cabrol, N.A. Alien Mindscapes—A Perspective on the Search for Extraterrestrial Intelligence. Astrobiology 2016, 16, 661–676. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Tasker, E.; Tan, J.; Heng, K.; Kane, S.; Spiegel, D. The ELSI Origins Network Planetary Diversity Workshop. The language of exoplanet ranking metrics needs to change. Nature 2017, 1. [Google Scholar] [CrossRef]
- Kiang, N.Y.; Siefert, J.; Blackenship, R.E. Spectral Signatures of Photosynthesis. I. Review of Earth Organisms. Astrobiology 2007, 7, 222–251. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kiang, N.Y.; Segura, A.; Tinetti, G.; Blankenship, R.E.; Cohen, M.; Siefert, J.; Crisp, D.; Meadows, V.S. Spectral signatures of photosynthesis. II. Coevolution with other stars and the atmosphere on extrasolar worlds. Astrobiology 2007, 7, 252–274. [Google Scholar] [CrossRef] [PubMed]
- Bean, J.L.; Abbot, D.S.; Kempton, E.M.-R. A Statistical Comparative Planetology Approach to the Hunt for Habitable Exoplanets and Life beyond the Solar System. Astrophys. J. Lett. 2017, 841. [Google Scholar] [CrossRef]
- Siever, R. Sedimentological consequences of steady-state ocean-atmosphere. Sedimentology 1968, 11, 5–29. [Google Scholar] [CrossRef]
- Zahnle, K.J. Earth’s earliest atmosphere. Elements 2006, 2, 217–222. [Google Scholar] [CrossRef]
- Kasting, J.F.; Harman, C.E. Inner edge of the habitable zone. Nature 2013, 504, 221–223. [Google Scholar] [CrossRef] [PubMed]
- Dressing, C.D.; Charbonneau, D. The occurrence rate of small planets around small stars. Astrophys. J. 2013, 767. [Google Scholar] [CrossRef]
- Petigura, E.A.; Howard, A.W.; Marcy, G.W. Prevalence of Earth-size planets orbiting Sun-like stars. Proc. Natl. Acad. Sci. USA 2013, 110, 19273–19278. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Traub, W.A. Terrestrial, Habitable-zone Exoplanet Frequency from Kepler. Astrophys. J. 2012, 745. [Google Scholar] [CrossRef]
- Howell, S.B.; Sobeck, C.; Haas, M.; Still, M.; Barclay, T.; Mullally, F.; Troeltzsch, J.; Aigrain, S.; Bryson, S.T.; Caldwell, D.; et al. The K2 Mission: Characterization and Early Results. Publ. Astron. Soc. Pac. 2014, 126. [Google Scholar] [CrossRef]
- Ricker, G.R.; Vanderspek, R.K.; Latham, D.W.; Winn, J.N. The Transiting Exoplanet Survey Satellite Mission. In American Astronomical Society; American Astronomical Society Meeting #224, id.113.02: Boston, MA, USA, 2014. [Google Scholar]
- Seager, S.; Bains, W.; Petkowski, J.J. Toward a List of Molecules as Potential Biosignature Gases for the Search for Life on Exoplanets and Applications to Terrestrial Biochemistry. Astrobiology 2016, 16, 465–485. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Moore, W.B.; Lenardic, A.; Jellinek, A.M.; Johnson, C.L.; Goldblatt, C.; Lorenz, R.D. How habitable zones and super-Earths lead us astray. Nature 2017, 1. [Google Scholar] [CrossRef]















| Constant | Recent Venus | Leconte et al. | CO2 Maximum Greenhouse | Early Mars |
|---|---|---|---|---|
| SEFF(Sun) | 1.768 | 1.1105 | 0.3587 | 0.3246 |
| A | 1.3151 × 10−4 | 1.1921 × 10−4 | 5.8087 × 10−5 | 5.213 × 10−5 |
| B | 5.8695 × 10−10 | 9.5932 × 10−9 | 1.5393 × 10−9 | 4.5245 × 10−10 |
| C | −2.8895 × 10−12 | −2.6189 × 10−12 | −8.3547 × 10−13 | -1.0223 × 10−12 |
| D | 3.2174 × 10−16 | 1.3710 × 10−16 | 1.0319 × 10−16 | 9.6376 × 10−17 |
© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ramirez, R.M. A More Comprehensive Habitable Zone for Finding Life on Other Planets. Geosciences 2018, 8, 280. https://doi.org/10.3390/geosciences8080280
Ramirez RM. A More Comprehensive Habitable Zone for Finding Life on Other Planets. Geosciences. 2018; 8(8):280. https://doi.org/10.3390/geosciences8080280
Chicago/Turabian StyleRamirez, Ramses M. 2018. "A More Comprehensive Habitable Zone for Finding Life on Other Planets" Geosciences 8, no. 8: 280. https://doi.org/10.3390/geosciences8080280
APA StyleRamirez, R. M. (2018). A More Comprehensive Habitable Zone for Finding Life on Other Planets. Geosciences, 8(8), 280. https://doi.org/10.3390/geosciences8080280




