Experimentally Viable Techniques for Accessing Coexisting Attractors Correlated with Lyapunov Exponents
Abstract
:1. Introduction
2. Model and Methods
2.1. Laser with Injected Signal Model
2.2. Lyapunov Exponents
2.2.1. Generic Attractor Characteristics
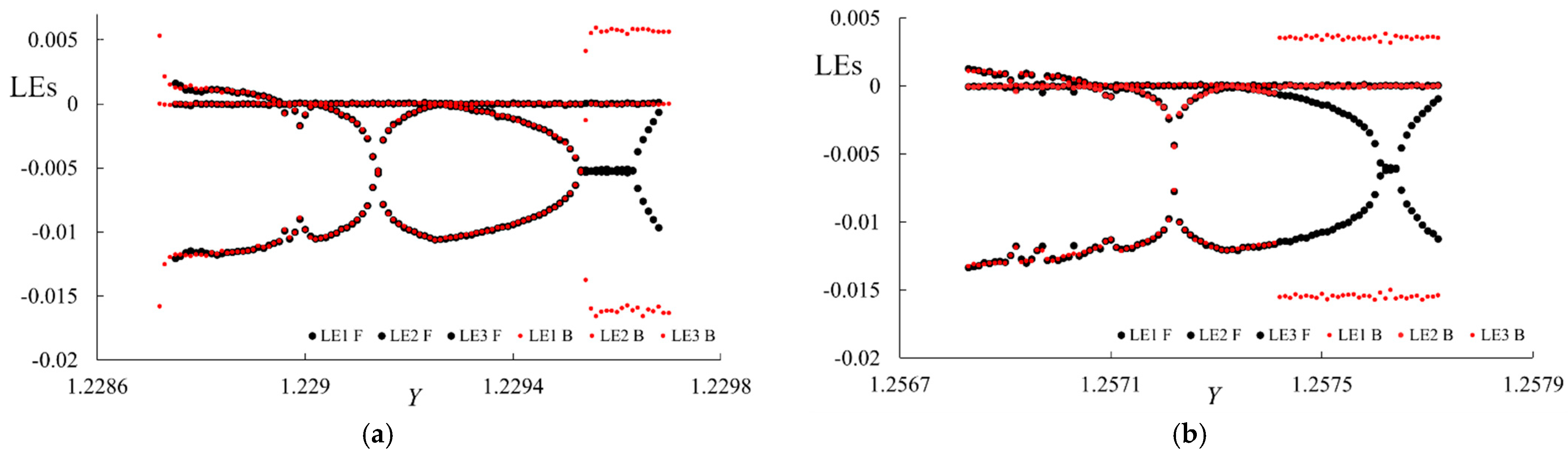
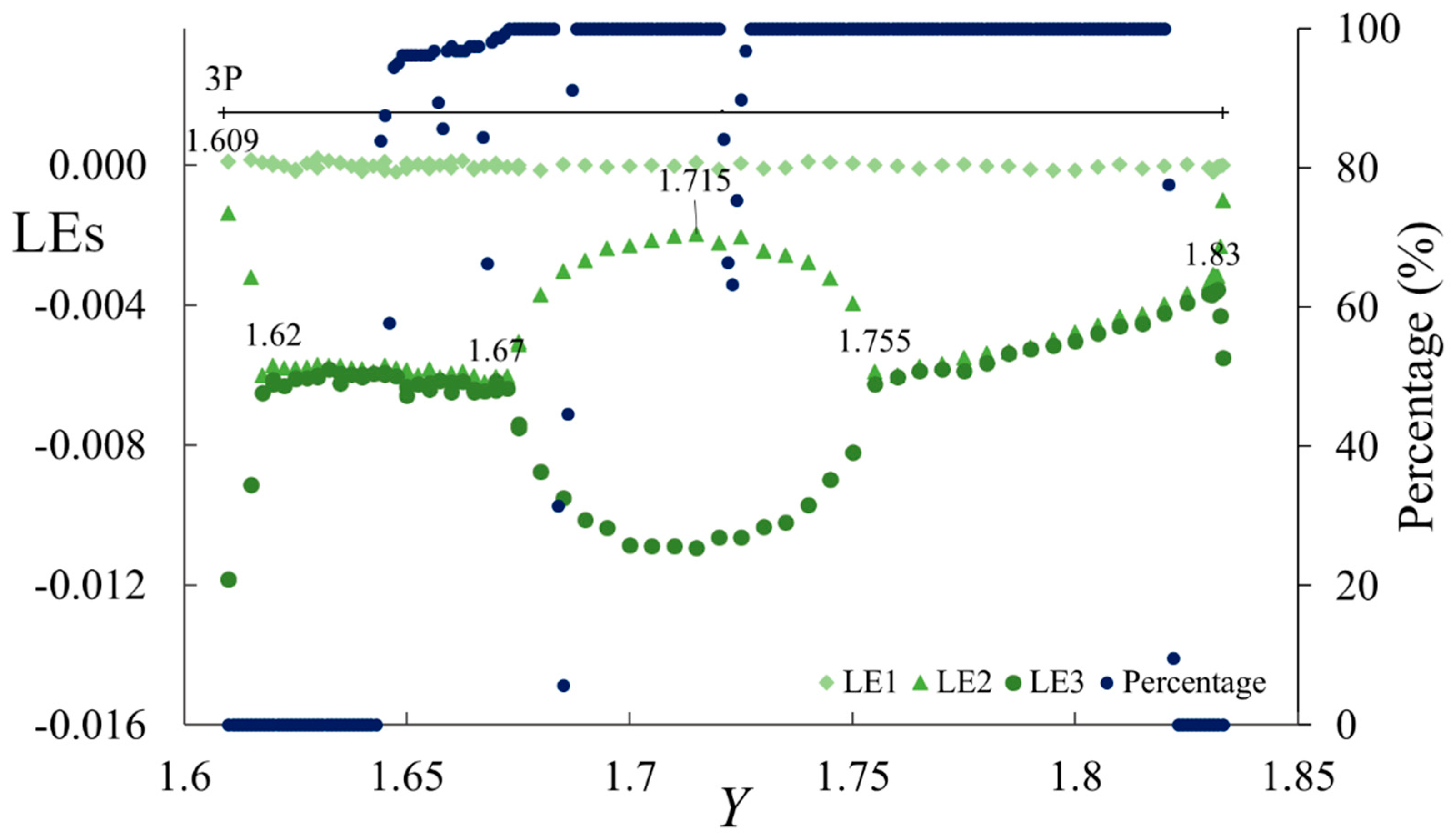
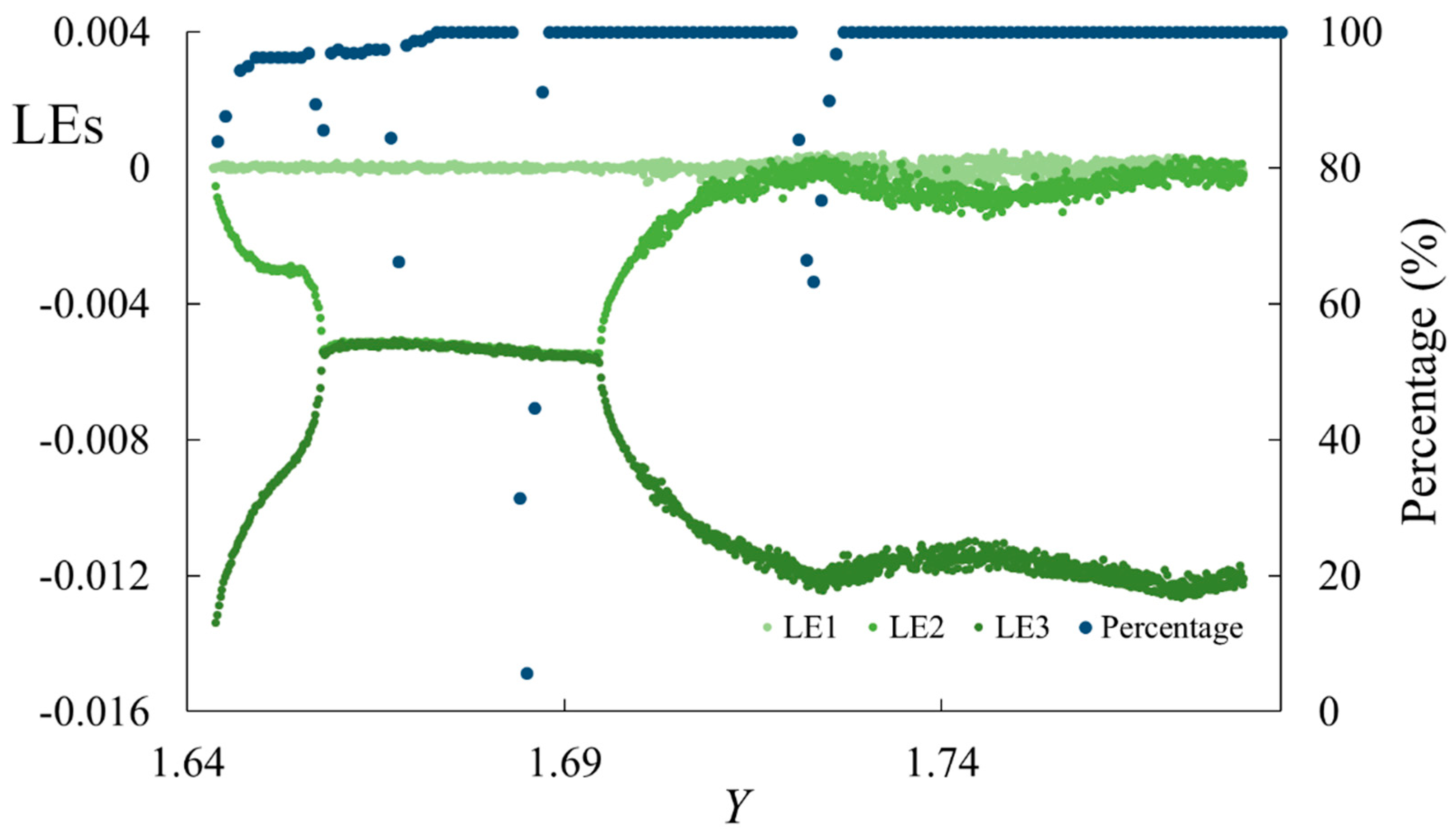
2.2.2. Symmetric-like LE Bubbles
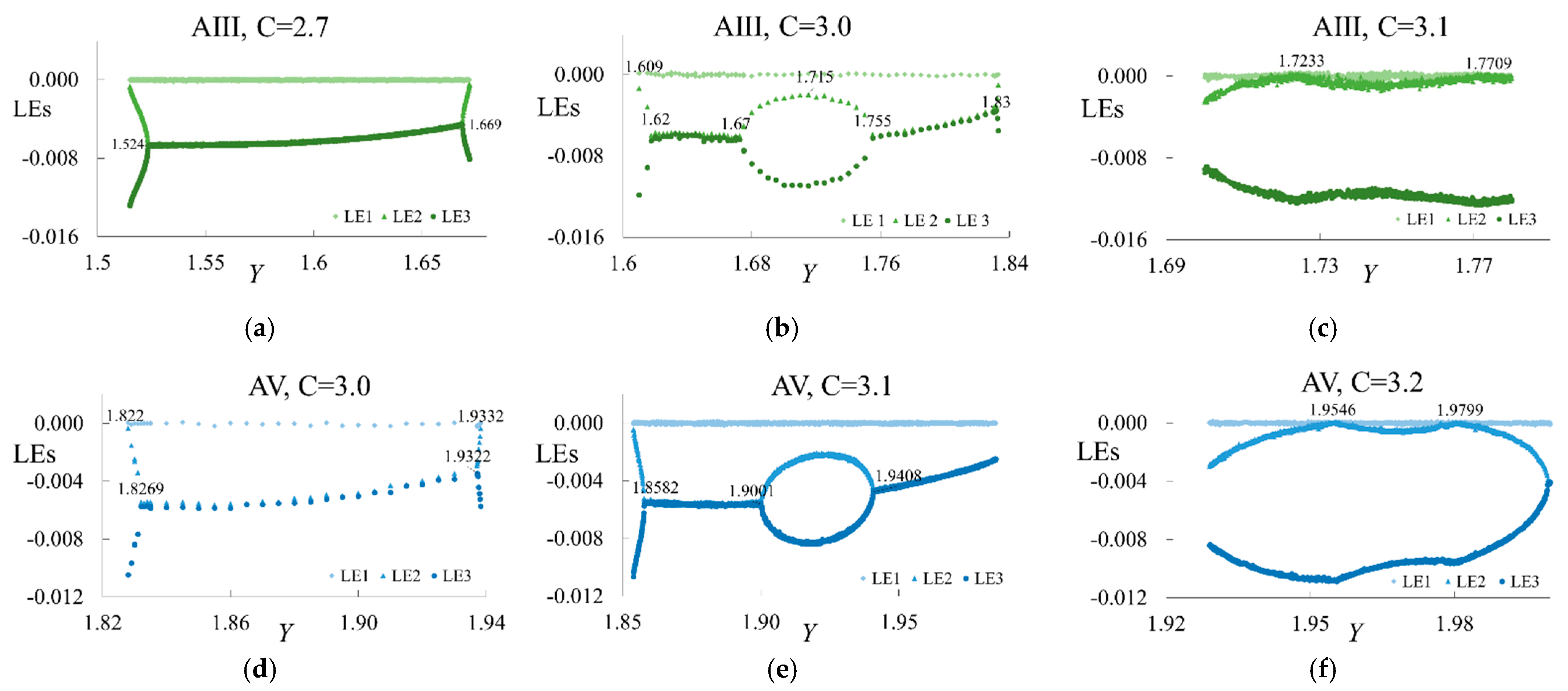
2.2.3. Window Characteristics Predicted by Lyapunov Exponents
2.3. Power Shifting to Access Attractors or Windows
2.3.1. A Theoretical Example of Power Shifting
2.3.2. Power-Shift Base Attractor Optimization
2.3.3. Power Shift with Noise and Ramped Injected Signal
2.3.4. Interim Parameter (Gain) Shift
3. Results
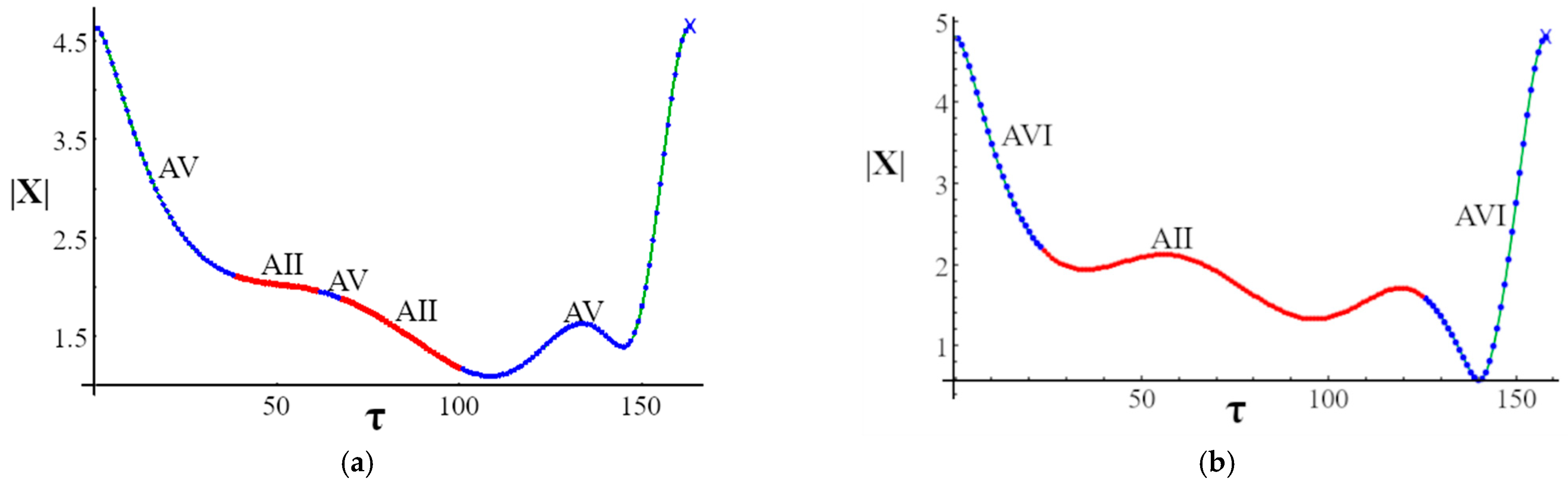
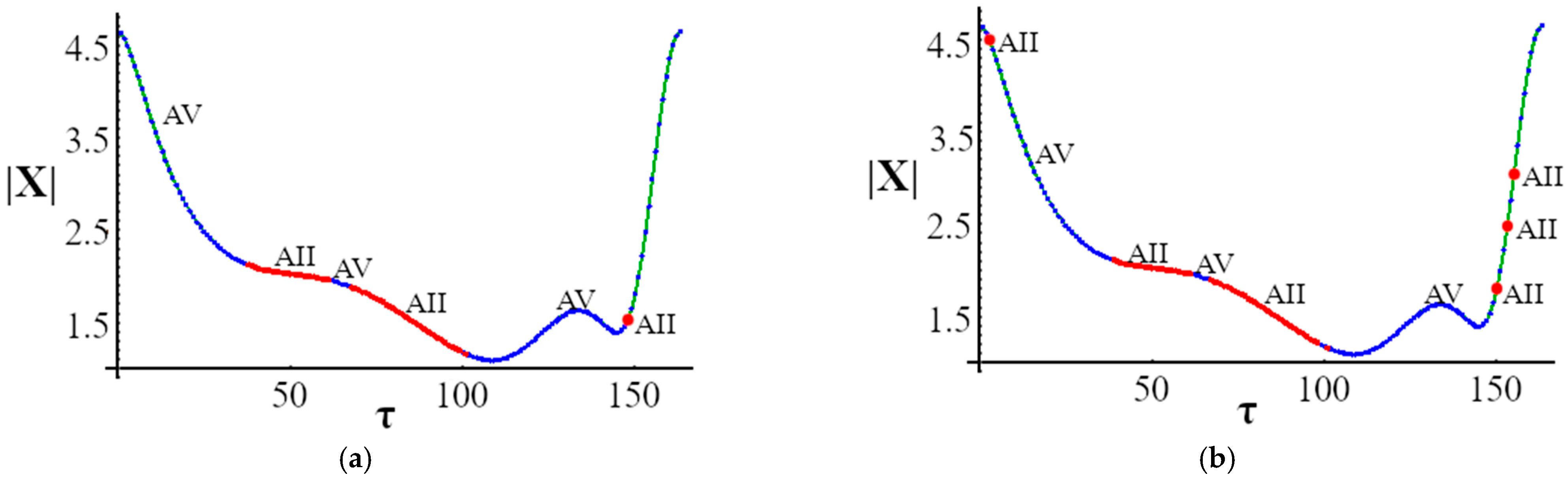
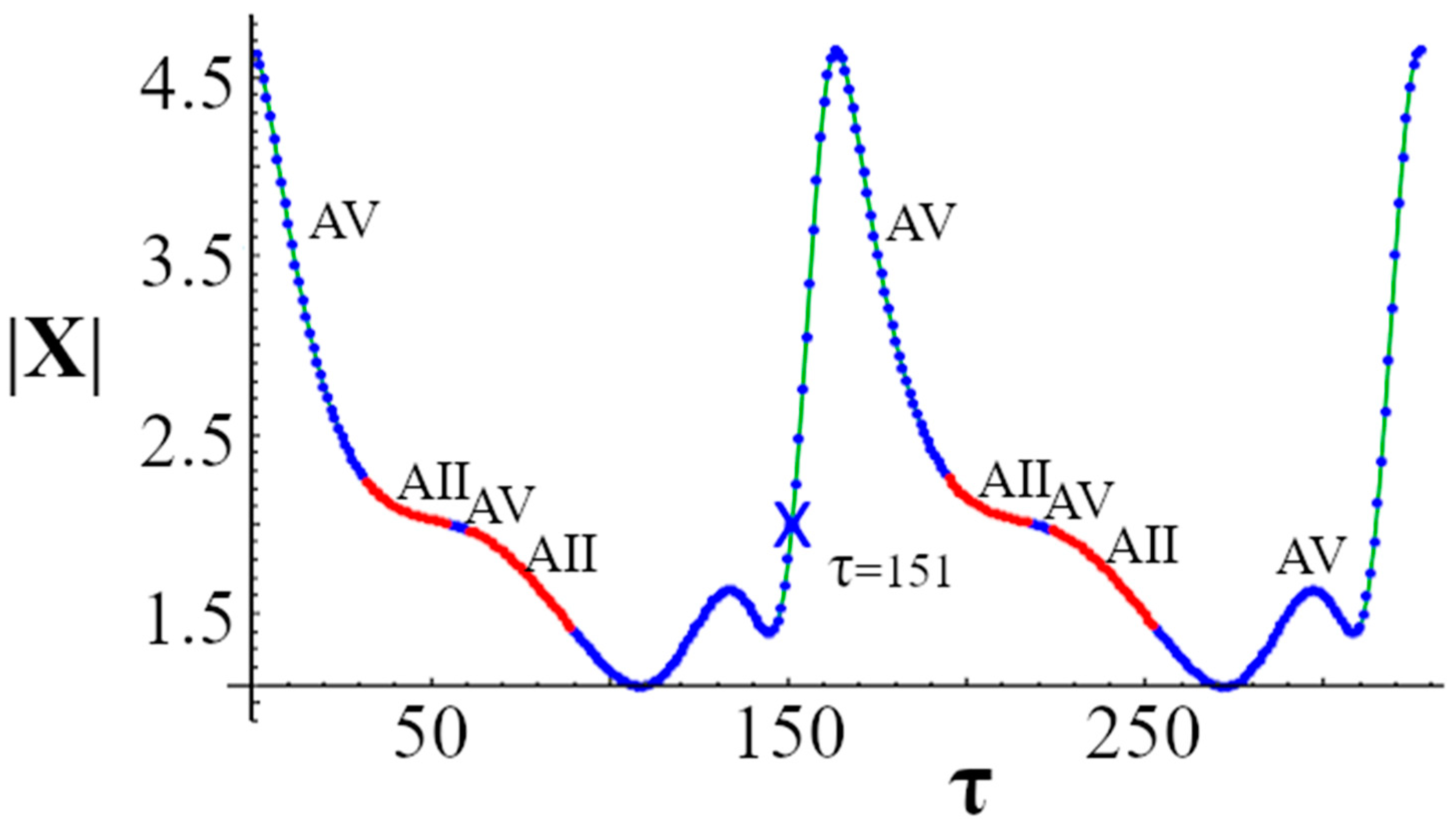
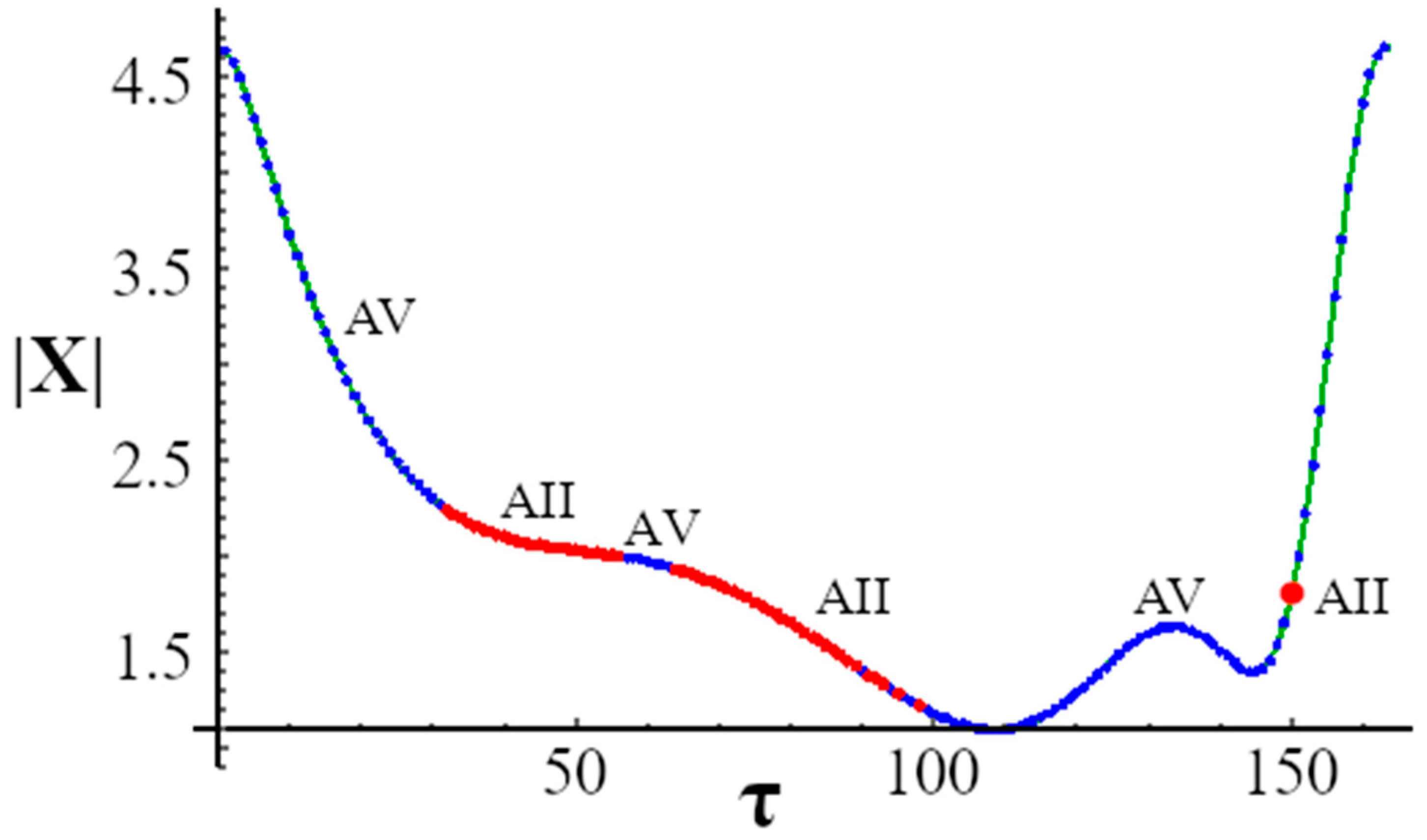
3.1. Gain Changes on Lyapunov Exponent Patterns
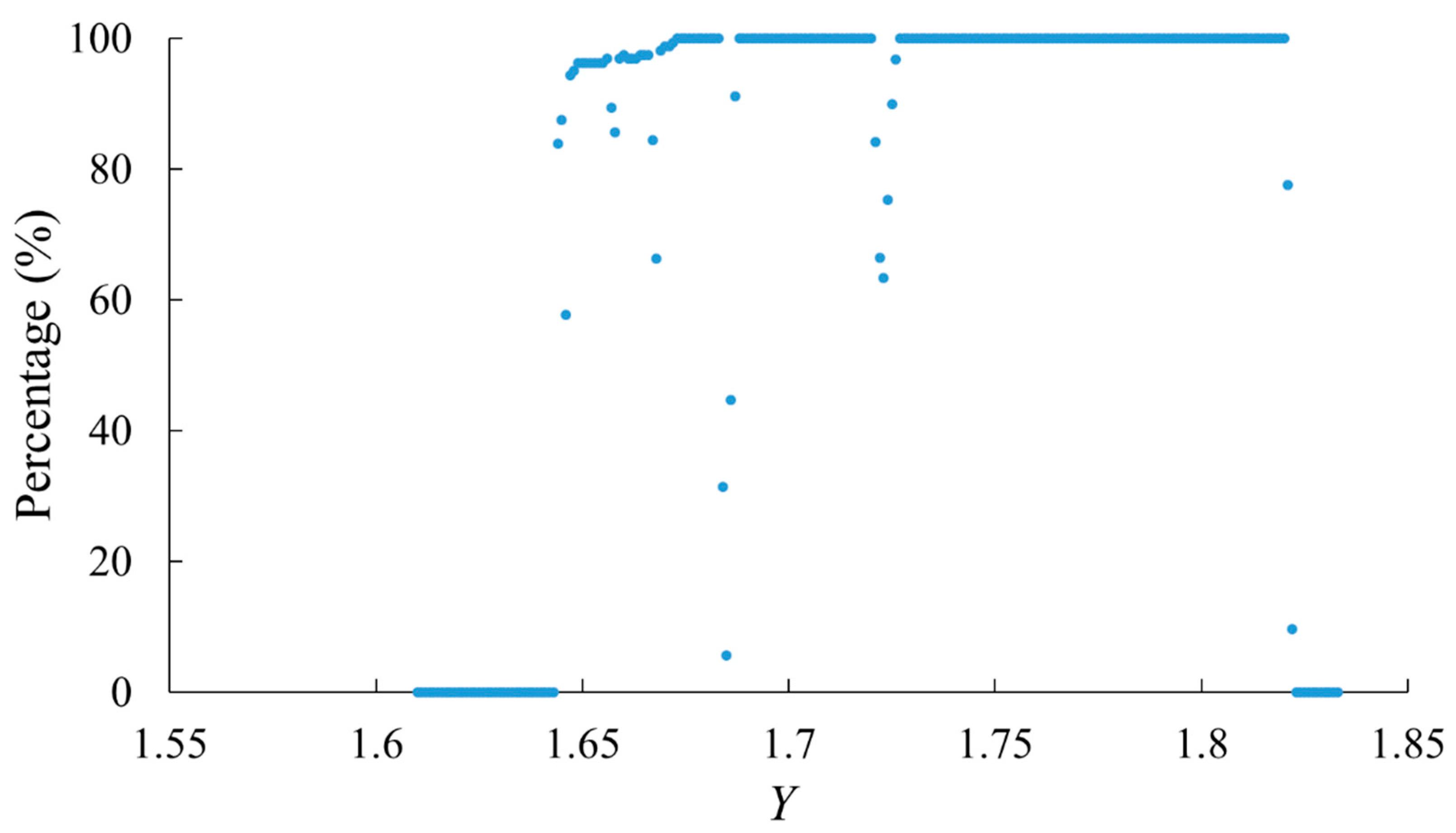
3.2. Power Shift
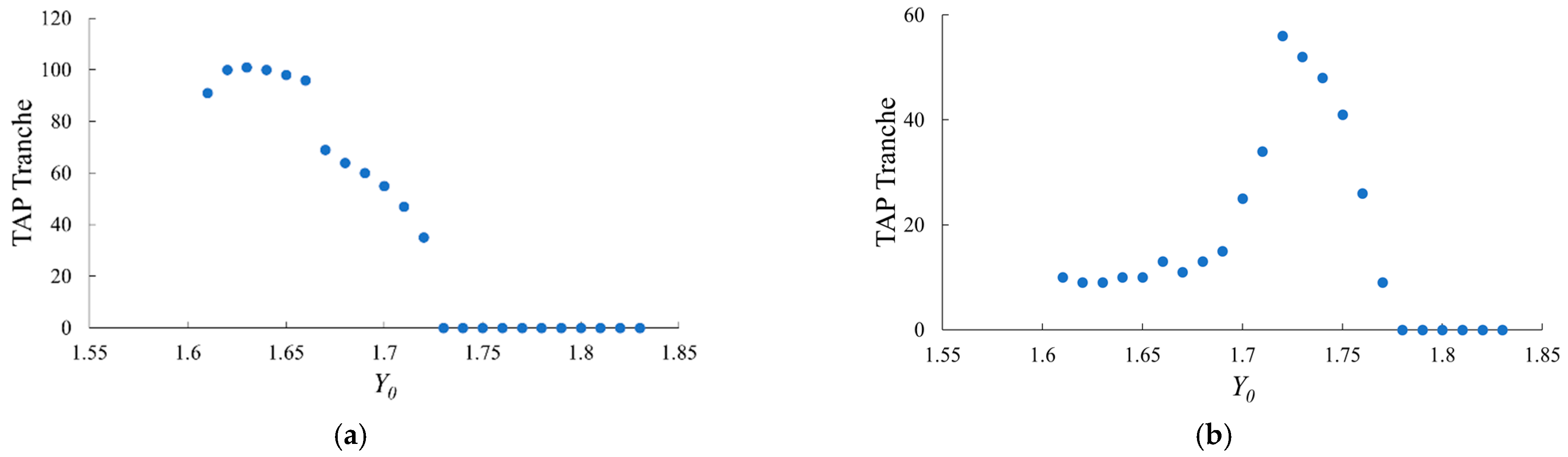
3.2.1. Optimized Base Attractor for Power Shift
3.2.2. Addition of Noise
3.2.3. Linear Ramp on Injected Signal
3.2.4. Linear Ramp and Noise Included
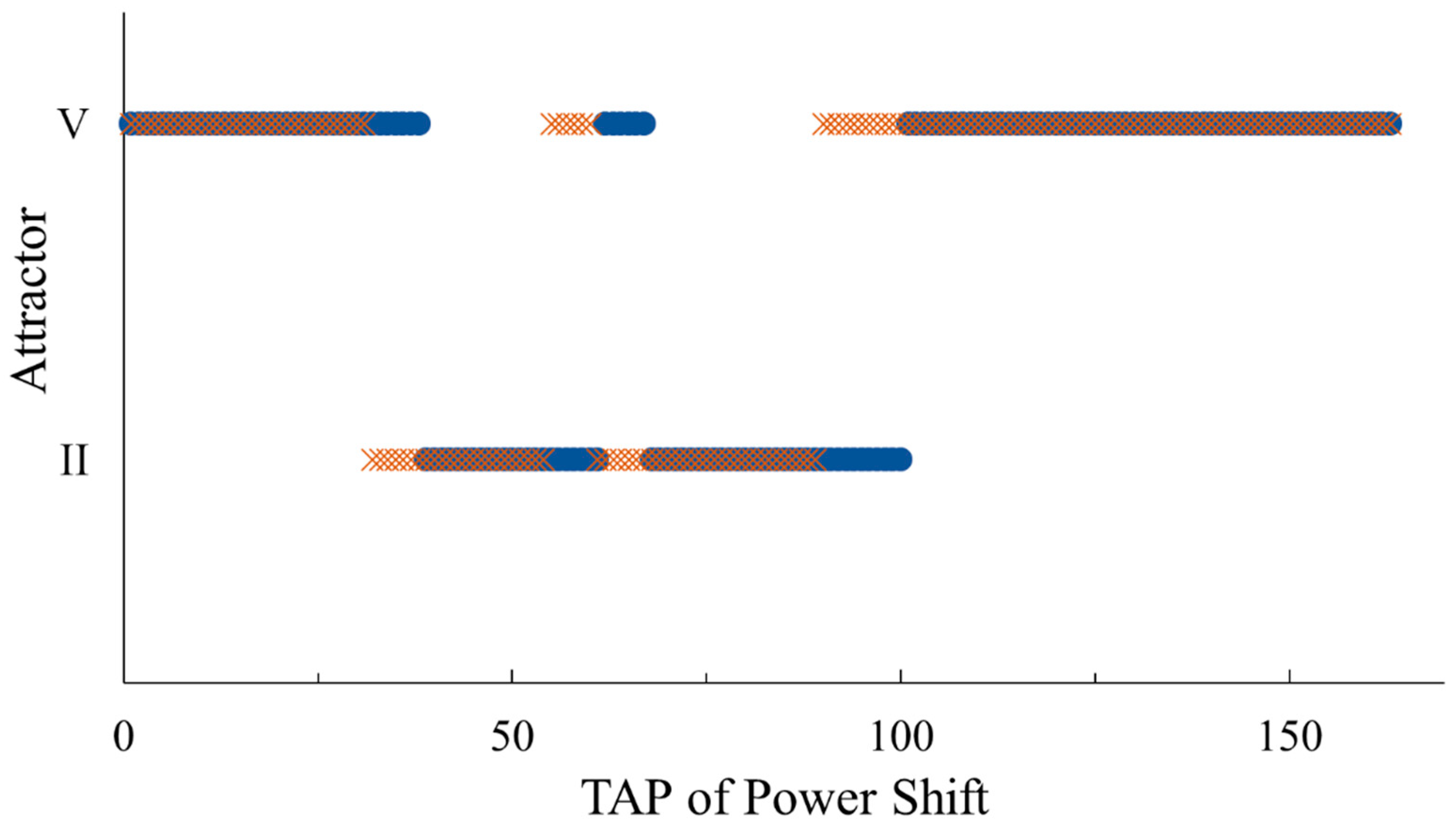
3.3. Interim Gain-Shift Results
4. Discussion
4.1. Gain Change Effects on LE Patterns
4.2. Power Shift
4.2.1. Optimized Power Shift
4.2.2. Effects of Noise
4.2.3. Effects of a Ramp on the Injected Signal
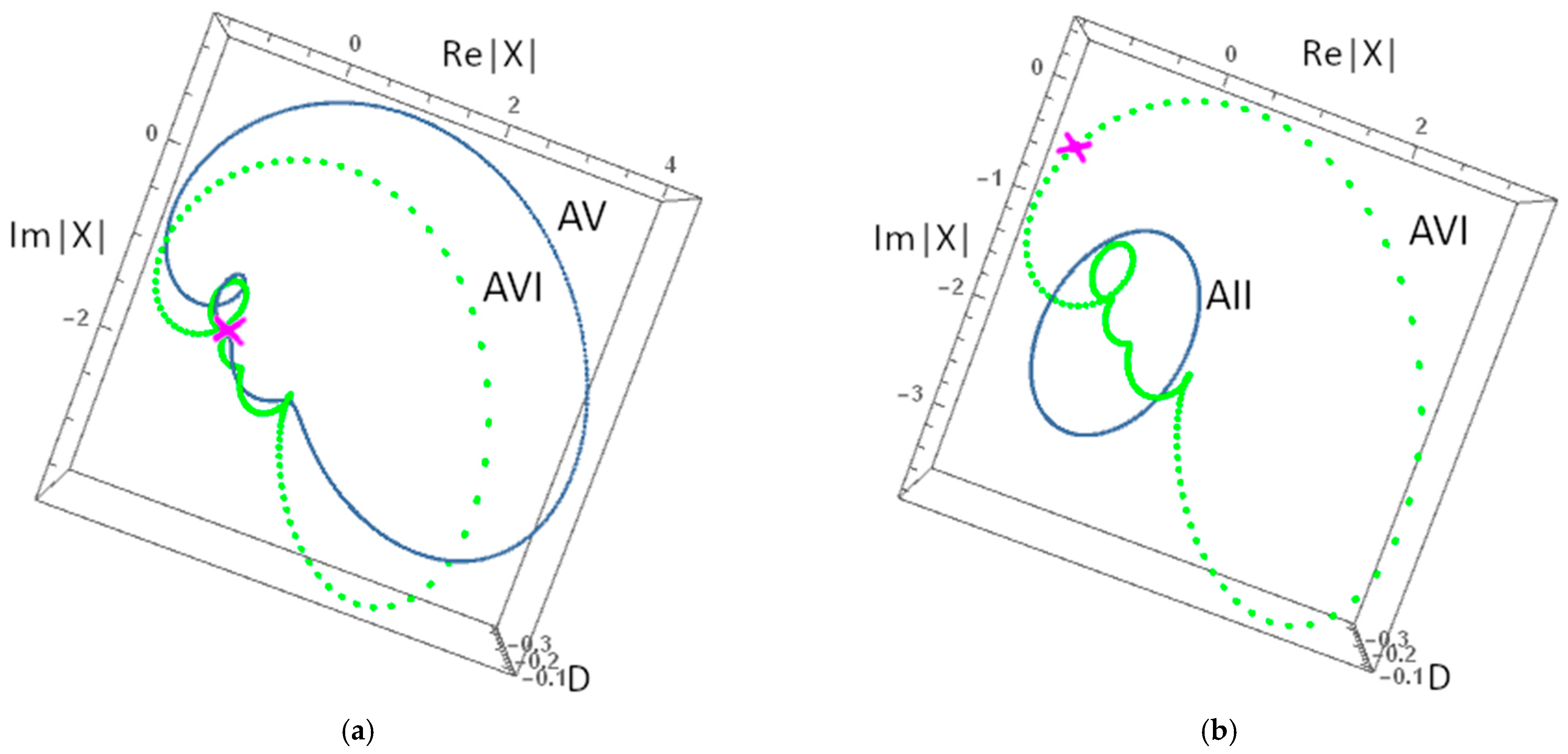
4.3. Gain Shift versus Symmetric-like Bubble
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
- Create m identical sequences of input signal amplitudes by dividing the domain ΔY into n equally spaced Y values and then randomize the order of each sequence. This creates multiple, completely arbitrary sequences of the same number set, Yji (I =1, 2, ..., n), (j = 1, 2, …, m);
- Power shift through the domain of the first random sequence of ΔY. To do this, begin the process at Y11, evolve the system for T units of time to stabilize the trajectory on a specific orbit (an attractor’s characteristic phase space);
- Record the final conditions of the laser variables and control parameter;
- Repeat steps 2 and 3 until all m sequences are recorded;
- Reorder all m sequences and compare the dynamics found at each input power, any discrepancies imply coexistence
Appendix B
| Goal Attractor | Base Attractor | Base Period (τ) | Y0 | YPS | TAP ± Leeway |
|---|---|---|---|---|---|
| AI | Any | Any | |||
| AII | Any | Any | 1.54 | Any | |
| AIII | AII | 108.025 | 1.51 * | 1.8 | 8 ± 52 |
| AIV | AI | 63.132 | 0.854 | 1.114 | 3 ± 4 |
| AV | AIII | 163.551 | 1.63 * | 1.84 | 151 ± 50 |
| AVI | AIII | 158.396 | 1.72 * | 1.96 | 154 ± 27 |
| AVII | AIII | 156.964 | 1.8 | 1.269 | 138 ± 2 |
References
- Bonifacio, R.; Lugiato, L.A. Cooperative effects and bistability for resonance fluorescence. Opt. Commun. 1976, 19, 172–176. [Google Scholar] [CrossRef]
- Bonifacio, R.; Lugiato, L.A. Mean field model for absorptive and dispersive bistability with inhomogeneous broadening. Lett. Nuovo Cimento 1978, 21, 517–521. [Google Scholar] [CrossRef]
- Erneux, T.; Gavrielides, A.; Kovanis, V. Low pump stability of an optically injected diode laser. Quantum Semiclassical Opt. J. Eur. Opt. Soc. Part B 1997, 9, 811–818. [Google Scholar] [CrossRef]
- Wieczorek, S.; Simpson, T.B.; Krauskopf, B.; Lenstra, D. Bifurcation transitions in an optically injected diode laser: Theory and experiment. Opt. Commun. 2003, 215, 125–134. [Google Scholar] [CrossRef] [Green Version]
- Tchinda, T.; Njitacke, Z.; Fozin Fonzin, T.; Fotsin, H. Dynamics of an optically injected diode laser subject to periodic perturbation: Occurrence of a large number of attractors, bistability and metastable chaos. CSSP 2019, 8, 66. [Google Scholar] [CrossRef]
- Lingnau, B.; Chow, W.W.; Schöll, E.; Lüdge, K. Feedback and injection locking instabilities in quantum-dot lasers: A microscopically based bifurcation analysis. New J. Phys. 2013, 15, 093031. [Google Scholar] [CrossRef]
- Jiang, Z.-F.; Wu, Z.-M.; Jayaprasath, E.; Yang, W.-Y.; Hu, C.-X.; Xia, G.-Q. Nonlinear dynamics of exclusive excited-state emission quantum dot lasers under optical injection. Photonics 2019, 6, 58. [Google Scholar] [CrossRef] [Green Version]
- Lugiato, L.A.; Narducci, L.M.; Bandy, D.K.; Pennise, C.A. Breathing, spiking and chaos in a laser with injected signal. Opt. 1983, 46, 64–68. [Google Scholar] [CrossRef]
- Bandy, D.K.; Narducci, L.M.; Lugiato, L.A. Coexisting attractors in a laser with an injected signal. JOSA B 1985, 2, 148–155. [Google Scholar] [CrossRef]
- Jones, D.J.; Bandy, D.K. Attractors and chaos in the laser with injected signal. J. Opt. Soc. Am. B 1990, 7, 2119. [Google Scholar] [CrossRef]
- Gallas, J.A.C. Structure of the parameter space of the Hénon map. Phys. Rev. Lett. 1993, 70, 2714–2717. [Google Scholar] [CrossRef] [PubMed]
- Bonatto, C.; Garreau, J.C.; Gallas, J.A.C. Self-similarities in the frequency-amplitude space of a loss-modulated CO2 laser. Phys. Rev. Lett. 2005, 95, 143905. [Google Scholar] [CrossRef] [Green Version]
- Bonatto, C.; Gallas, J.A.C. Accumulation horizons and period adding in optically injected semiconductor lasers. Phys. Rev. E 2007, 75, 055204. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Guckenheimer, J.; Holmes, P. Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields; Springer: New York, NY, USA, 1983. [Google Scholar]
- Haken, H. Synergetics: An Introduction; Springer: New York, NY, USA, 1983. [Google Scholar]
- Schuster, H.G.; Just, W. Deterministic Chaos: An Introduction, 4th ed.; Wiley-VCH: Berlin, Germany, 2005. [Google Scholar]
- Narducci, L.M.; Abraham, N.B. Laser Physics and Laser Instabilities; World Scientific: Singapore, 1980. [Google Scholar]
- Rössler, O.E. An equation for continuous chaos. Phys. Lett. A 1976, 57, 397–398. [Google Scholar] [CrossRef]
- Shaw, R. Strange attractors, chaotic behavior, and information flow. Z. Für Nat. A 1981, 36, 80–112. [Google Scholar] [CrossRef]
- Mandelbrot, B.B. The Fractal Geometry of Nature; Henry Holt and Company: New York, NY, USA, 1983; ISBN 978-0-7167-1186-5. [Google Scholar]
- Eckmann, J.-P.; Ruelle, D. Ergodic theory of chaos and strange attractors. In The Theory of Chaotic Attractors; Hunt, B.R., Li, T.-Y., Kennedy, J.A., Nusse, H.E., Eds.; Springer: New York, NY, USA, 2004; pp. 273–312. ISBN 978-0-387-21830-4. [Google Scholar]
- Thompson, J.M.T.; Stewart, H.B. Nonlinear Dynamics and Chaos: Geometrical Methods for Engineers and Scientists; Wiley: Hoboken, NJ, USA, 1986; ISBN 978-0-471-90960-6. [Google Scholar]
- Gibbs, H.M.; Hopf, F.A.; Kaplan, D.L.; Shoemaker, R.L. Observation of chaos in optical bistability. Phys. Rev. Lett. 1981, 46, 474–477. [Google Scholar] [CrossRef]
- Arecchi, F.T.; Meucci, R.; Puccioni, G.; Tredicce, J. Experimental evidence of subharmonic bifurcations, multistability, and turbulence in a Q-Switched gas laser. Phys. Rev. Lett. 1982, 49, 1217–1220. [Google Scholar] [CrossRef]
- Tredicce, J.R.; Arecchi, F.T.; Puccioni, G.P.; Poggi, A.; Gadomski, W. Dynamic behavior and onset of low-dimensional chaos in a modulated homogeneously broadened single-mode laser: Experiments and theory. Phys. Rev. A 1986, 34, 2073–2081. [Google Scholar] [CrossRef]
- Dangoisse, D.; Glorieux, P.; Hennequin, D. Laser chaotic attractors in crisis. Phys. Rev. Lett. 1986, 57, 2657–2660. [Google Scholar] [CrossRef]
- Dangoisse, D.; Glorieux, P.; Hennequin, D. Chaos in a CO2 laser with modulated parameters: Experiments and numerical simulations. Phys. Rev. A 1987, 36, 4775–4791. [Google Scholar] [CrossRef]
- Derozier, D.; Bielawski, S.; Glorieux, P. Dynamical behavior of a doped fiber laser under pump modulation. Opt. Commun. 1991, 83, 97–102. [Google Scholar] [CrossRef]
- Meucci, R.; Poggi, A.; Arecchi, F.T.; Tredicce, J.R. Dissipativity of an optical chaotic system characterized via generalized multistability. Opt. Commun. 1988, 65, 151–156. [Google Scholar] [CrossRef]
- Ott, E.; Grebogi, C.; Yorke, J.A. Controlling chaos. Phys. Rev. Lett. 1990, 64, 1196–1199. [Google Scholar] [CrossRef] [PubMed]
- Pyragas, K. Continuous control of chaos by self-controlling feedback. Phys. Lett. A 1992, 170, 421–428. [Google Scholar] [CrossRef]
- Li, C.; Sprott, J.C. Finding coexisting attractors using amplitude control. Nonlinear Dyn. 2014, 78, 2059–2064. [Google Scholar] [CrossRef]
- Li, C.; Wang, X.; Chen, G. Diagnosing multistability by offset boosting. Nonlinear Dyn. 2017, 90, 1335–1341. [Google Scholar] [CrossRef]
- Burton, E.K.T.; Hall, J.R.; Chapman, D.M.; Bandy, D.K. Shifts in control parameter dynamically access individual attractors in a multistable system. Nonlinear Dyn. 2021, 105, 1877–1883. [Google Scholar] [CrossRef]
- Gu, Y.; Bandy, D.K.; Yuan, J.-M.; Narducci, L.M. Bifurcation routes in a laser with injected signal. Phys. Rev. A 1985, 31, 354–360. [Google Scholar] [CrossRef]
- Bandy, D.K.; Hall, J.R.; Denker, M.E. Predicting the evolutionary dynamic behavior of a laser with injected signal using Lyapunov exponents. Phys. Rev. A 2015, 92, 013841. [Google Scholar] [CrossRef]
- Bandy, D.K.; Burton, E.K.T.; Hall, J.R.; Chapman, D.M.; Elrod, J.T. Predicting attractor characteristics using Lyapunov exponents in a laser with injected signal. Chaos Interdiscip. J. Nonlinear Sci. 2021, 31, 013120. [Google Scholar] [CrossRef]
- Weiss, C.O. Instabilities and Chaotic Emission of Far-Infrared NH3-Lasers; Abraham, N.B., Chrostowski, J., Eds.; American Institute of Physics: Quebec City, QC, Canada, 1986; Volume 0667, p. 26. [Google Scholar]
- Abraham, N.B.; Narducci, L.M. Laser Physics & Laser Instabilities; World Scientific Publishing Company: Singapore, 1988; ISBN 978-9971-5-0063-4. [Google Scholar]
- Abarbanel, H.D.I.; Brown, R.; Kadtke, J.B. Prediction and system identification in chaotic nonlinear systems: Time series with broadband spectra. Physics Letters A 1989, 138, 401–408. [Google Scholar] [CrossRef]
- Abarbanel, H.D.I.; Brown, R.; Kadtke, J.B. Prediction in chaotic nonlinear systems: Methods for time series with broadband Fourier spectra. Phys. Rev. A 1990, 41, 1782–1807. [Google Scholar] [CrossRef] [PubMed]
- Abarbanel, H.D.I.; Brown, R.; Kennel, M.B. Variation of Lyapunov exponents on a strange attractor. J. Nonlinear Sci. 1991, 1, 175–199. [Google Scholar] [CrossRef]
- Benettin, G.; Galgani, L.; Strelcyn, J.-M. Kolmogorov entropy and numerical experiments. Phys. Rev. A 1976, 14, 2338–2345. [Google Scholar] [CrossRef]
- Mandel, P.; Erneux, T. Laser Lorenz Equations with a time-dependent parameter. Phys. Rev. Lett. 1984, 53, 1818–1820. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hall, J.R.; Burton, E.K.T.; Chapman, D.M.; Bandy, D.K. Experimentally Viable Techniques for Accessing Coexisting Attractors Correlated with Lyapunov Exponents. Appl. Sci. 2021, 11, 9905. https://doi.org/10.3390/app11219905
Hall JR, Burton EKT, Chapman DM, Bandy DK. Experimentally Viable Techniques for Accessing Coexisting Attractors Correlated with Lyapunov Exponents. Applied Sciences. 2021; 11(21):9905. https://doi.org/10.3390/app11219905
Chicago/Turabian StyleHall, Joshua Ray, Erikk Kenneth Tilus Burton, Dylan Michael Chapman, and Donna Kay Bandy. 2021. "Experimentally Viable Techniques for Accessing Coexisting Attractors Correlated with Lyapunov Exponents" Applied Sciences 11, no. 21: 9905. https://doi.org/10.3390/app11219905






