A DEM Study on Bearing Behavior of Floating Geosynthetic-Encased Stone Column in Deep Soft Clays
Abstract
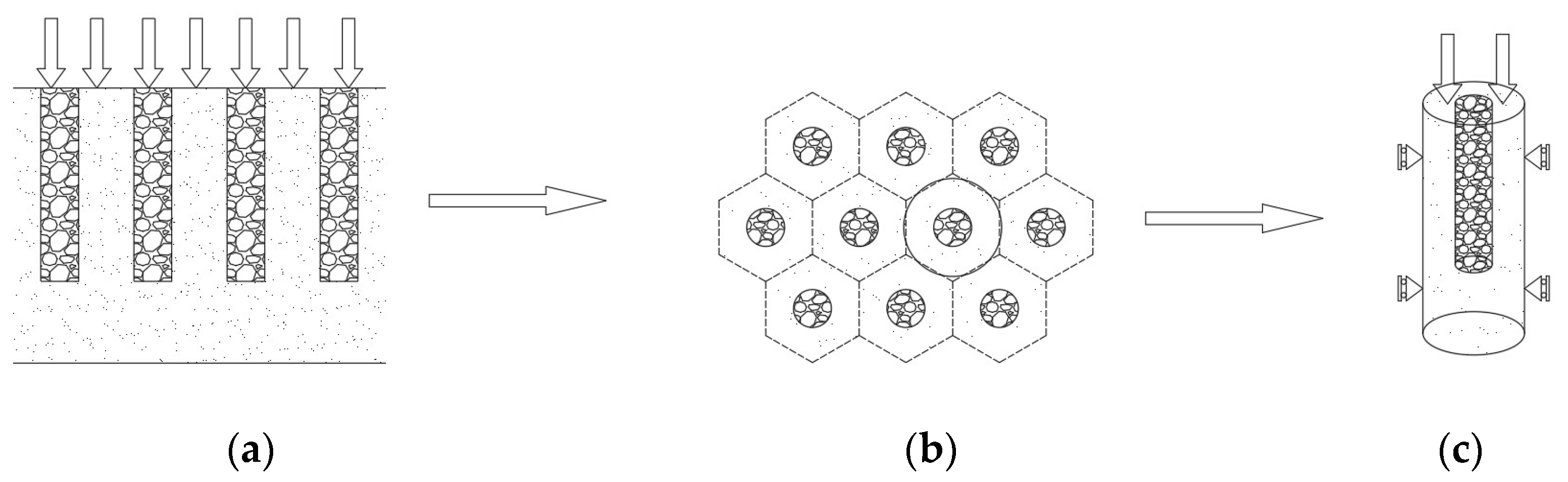
:1. Introduction
2. Numerical Simulation: Discrete Element Modeling
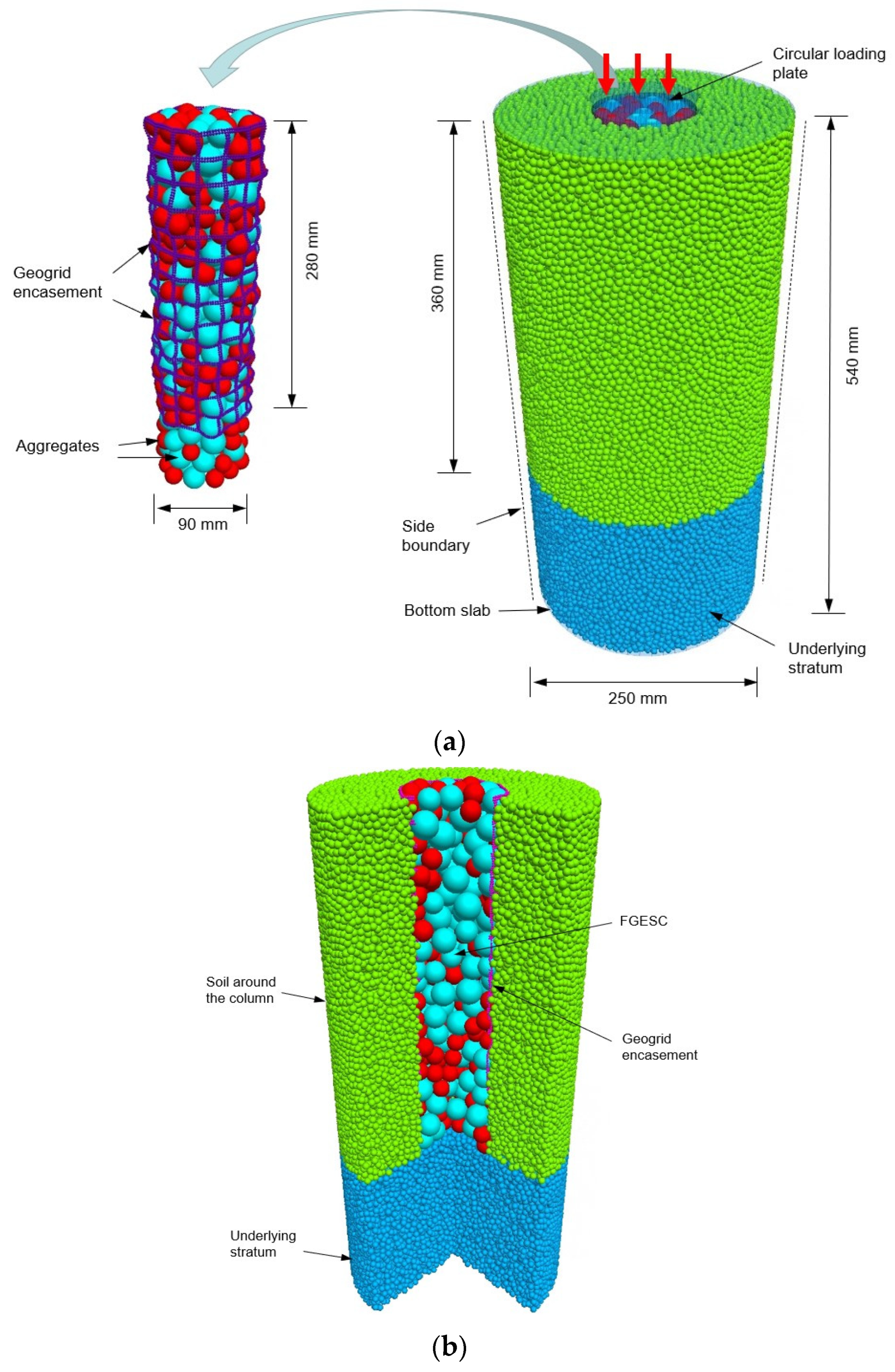
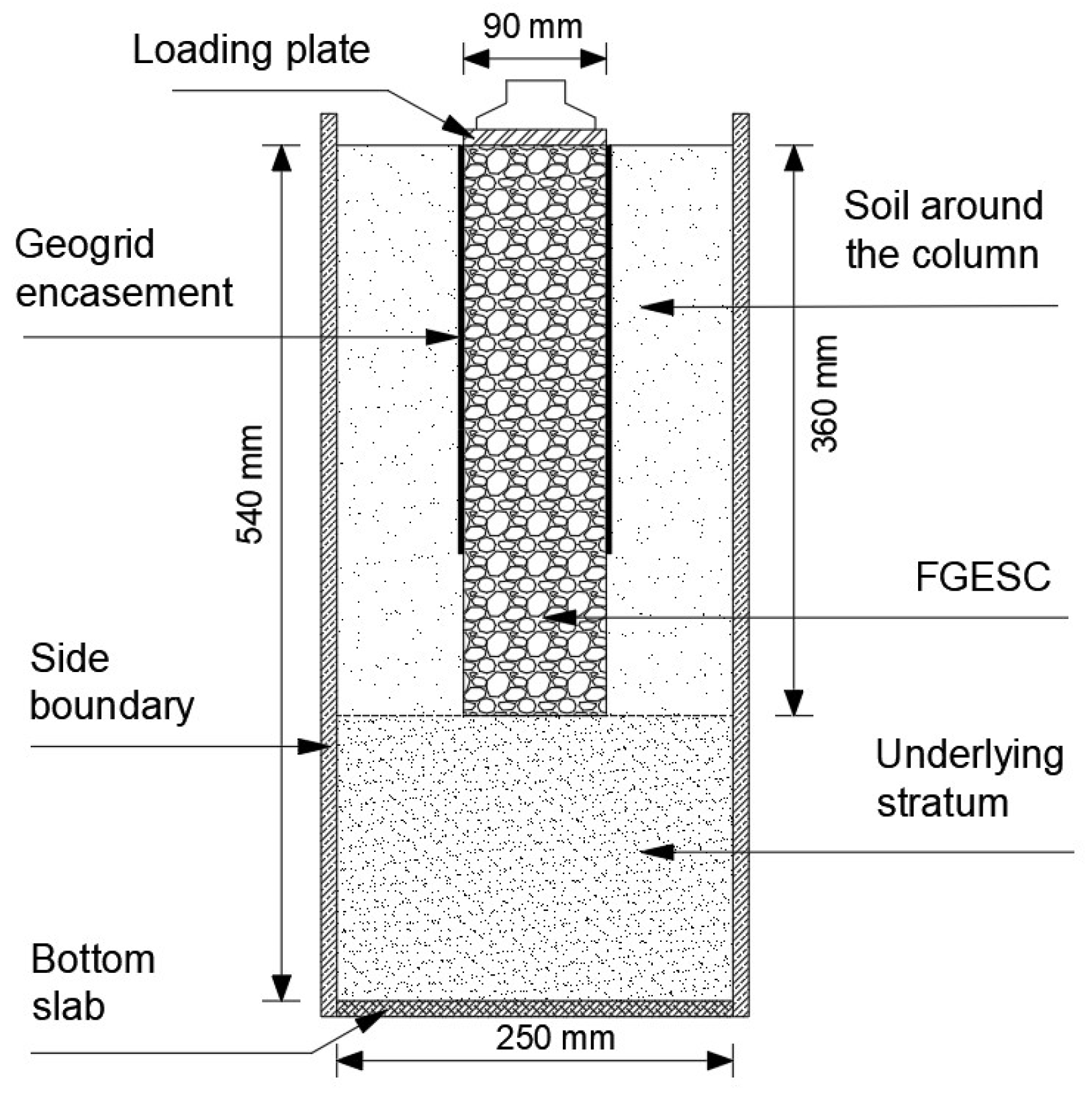
2.1. PFC Modeling of a FGESC in Soft Clay
2.2. Material Properties
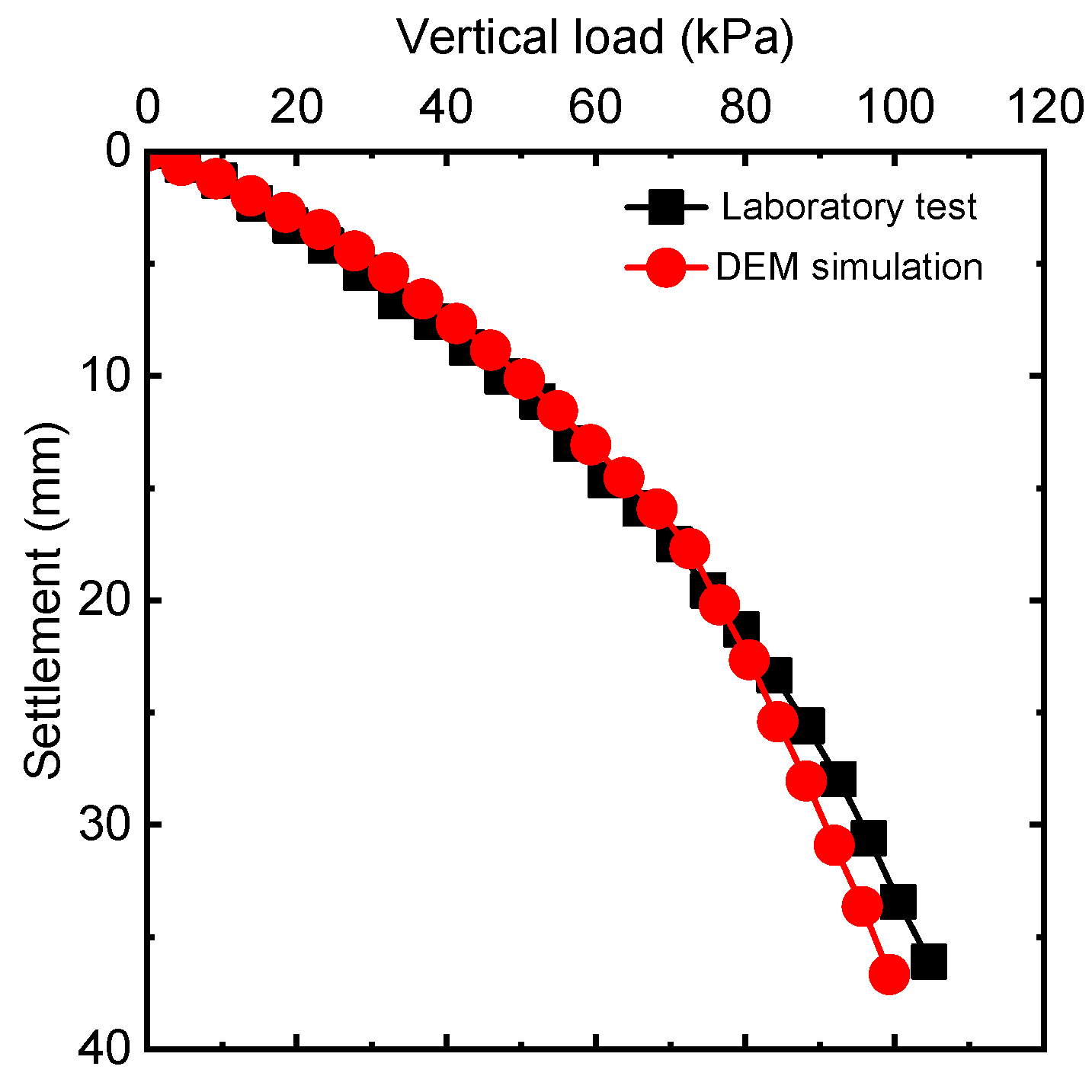
2.3. Validation of the DEM Model
3. Results and Discussion
3.1. Stresses and Deformation of the FGESC
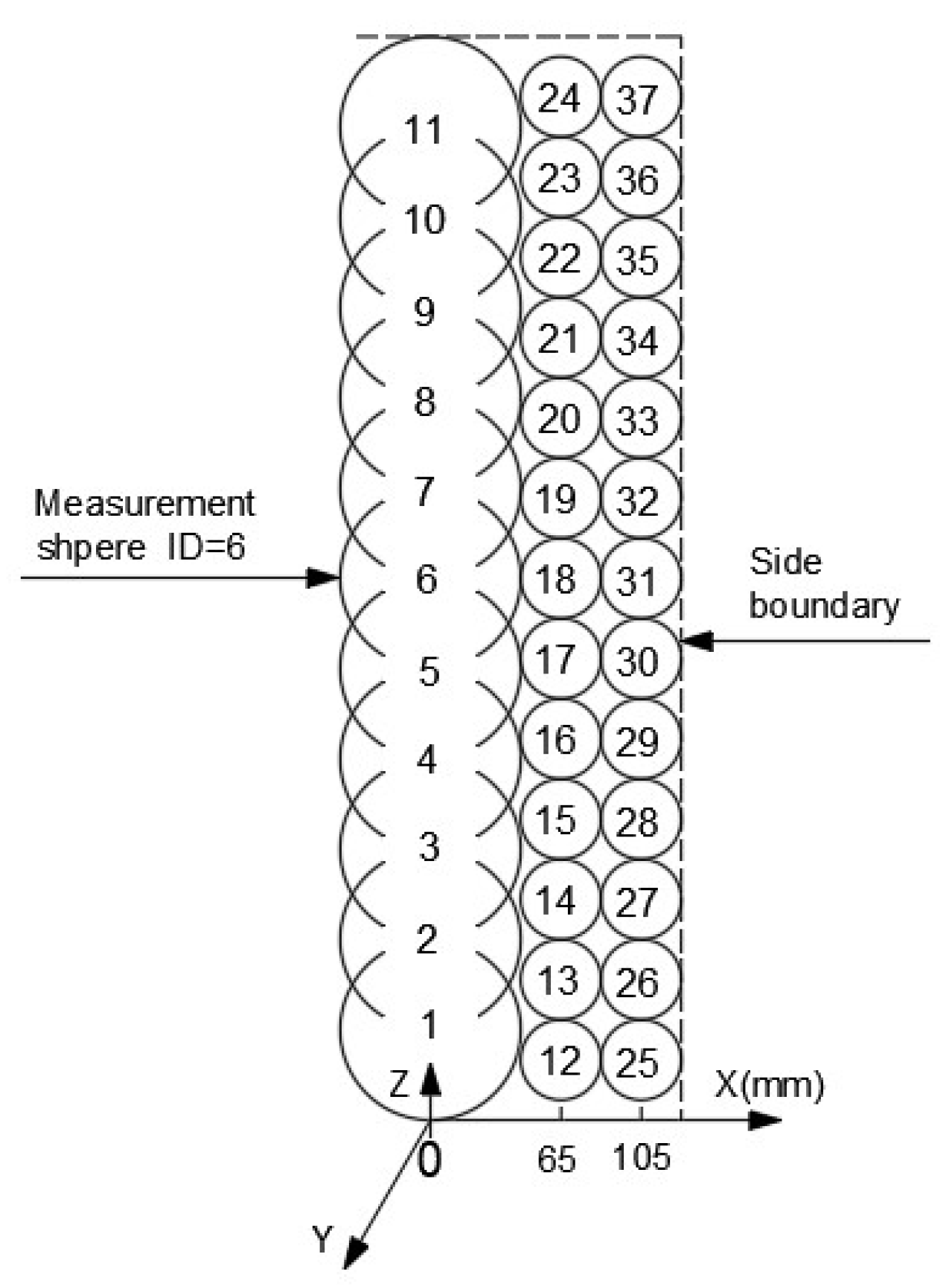
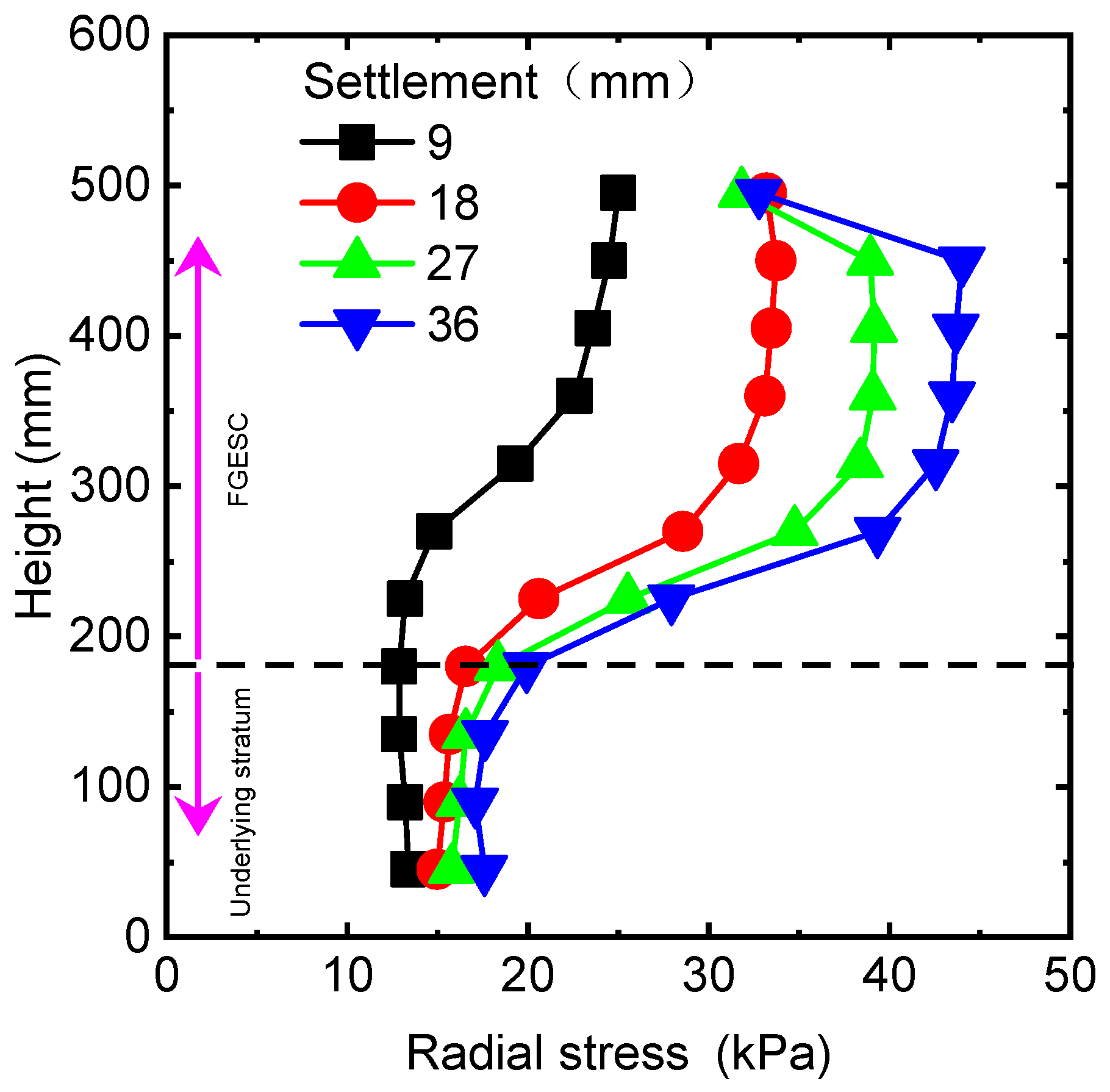
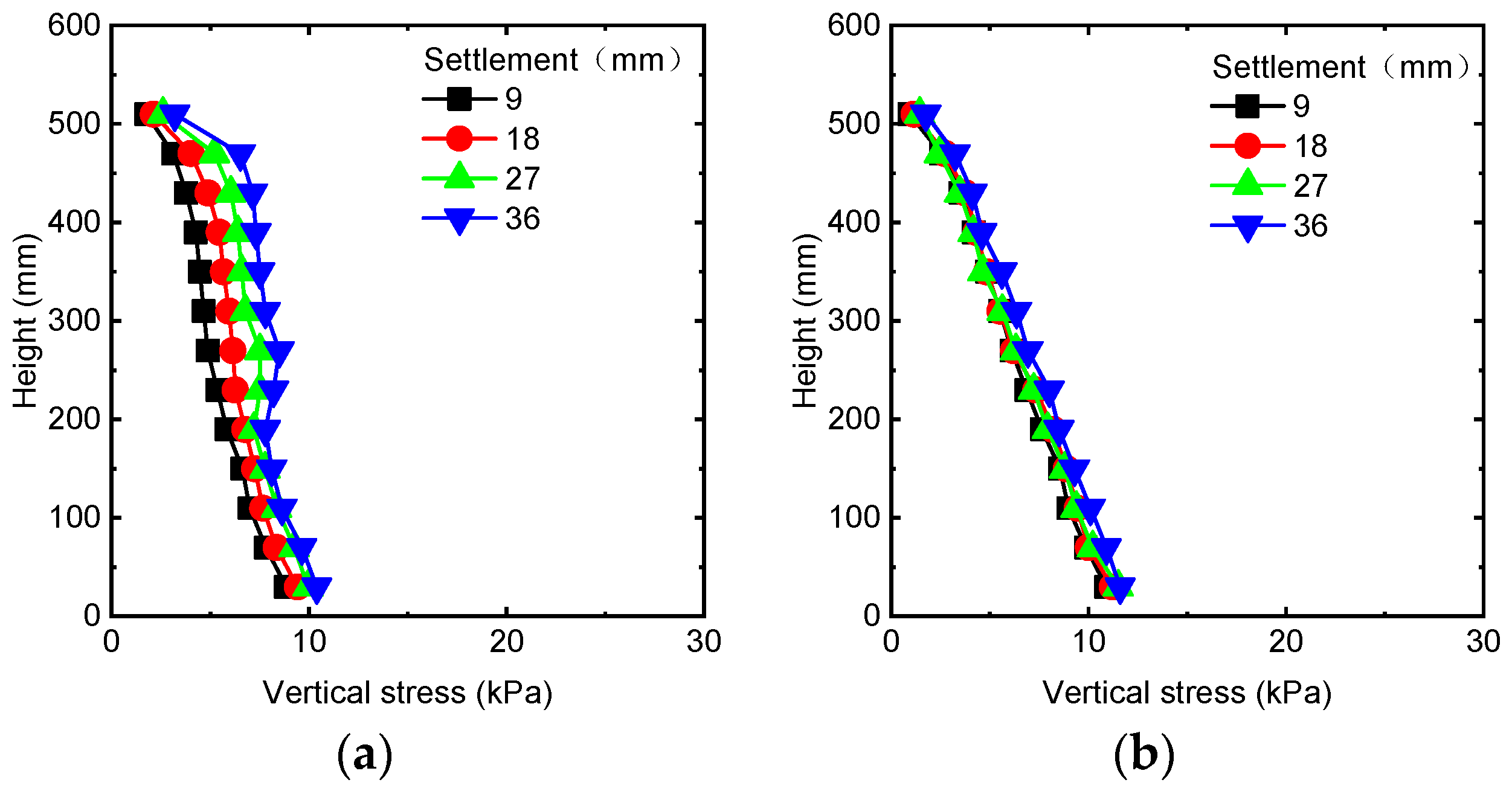
3.1.1. Vertical and Radial Stresses of FGESC and Underlying Soil
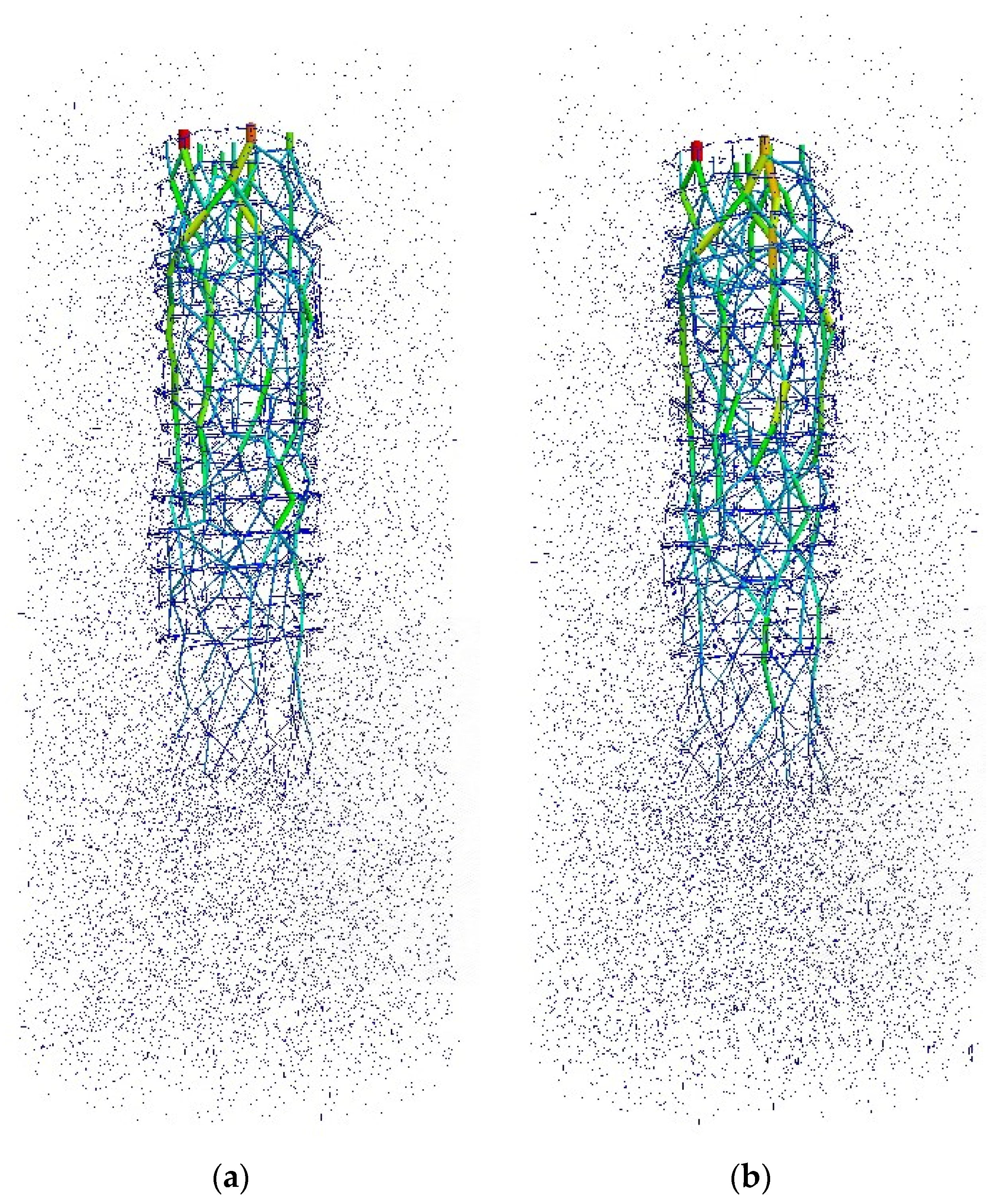
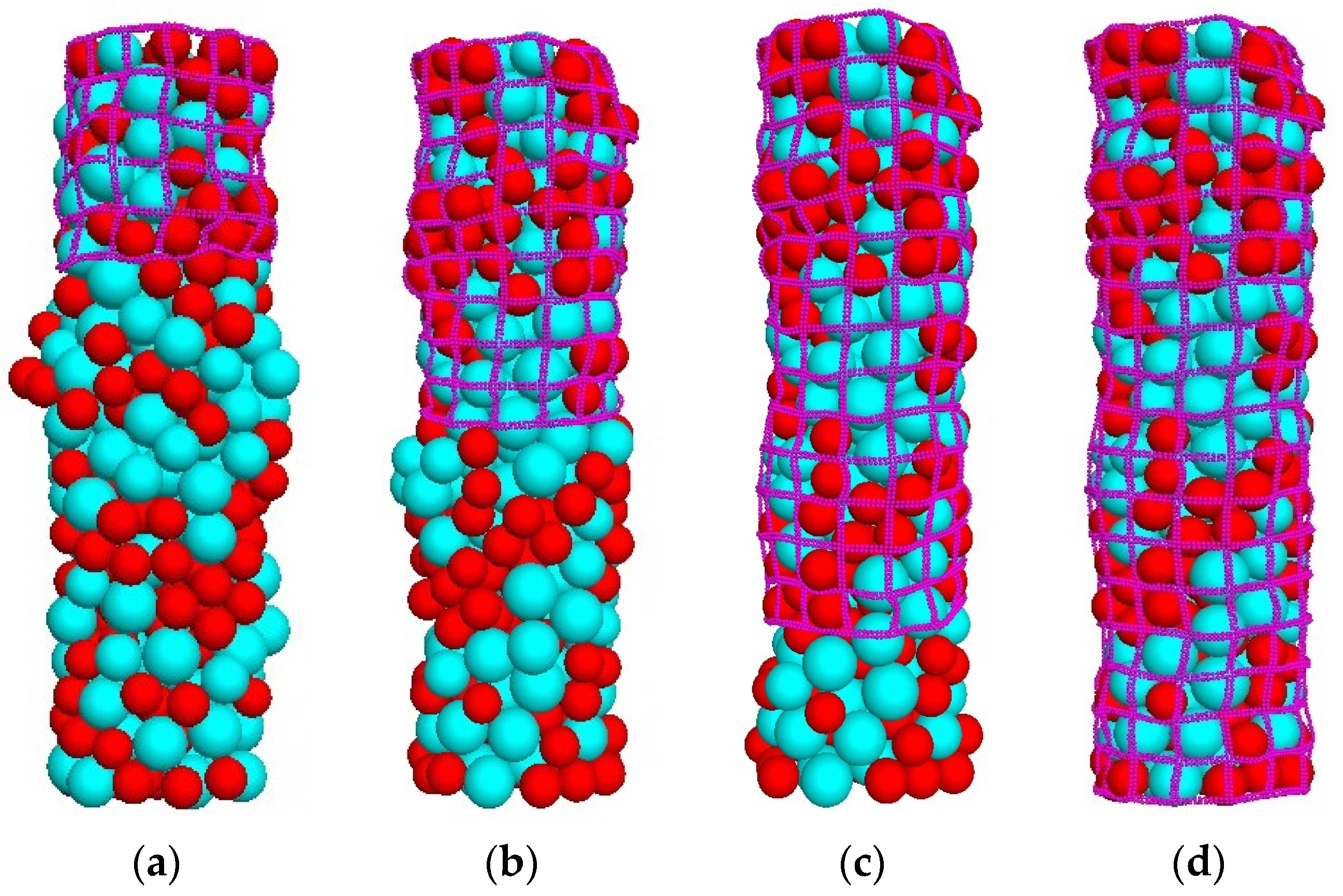
3.1.2. Force Chain of FGESC
3.1.3. Porosity of the FGESC
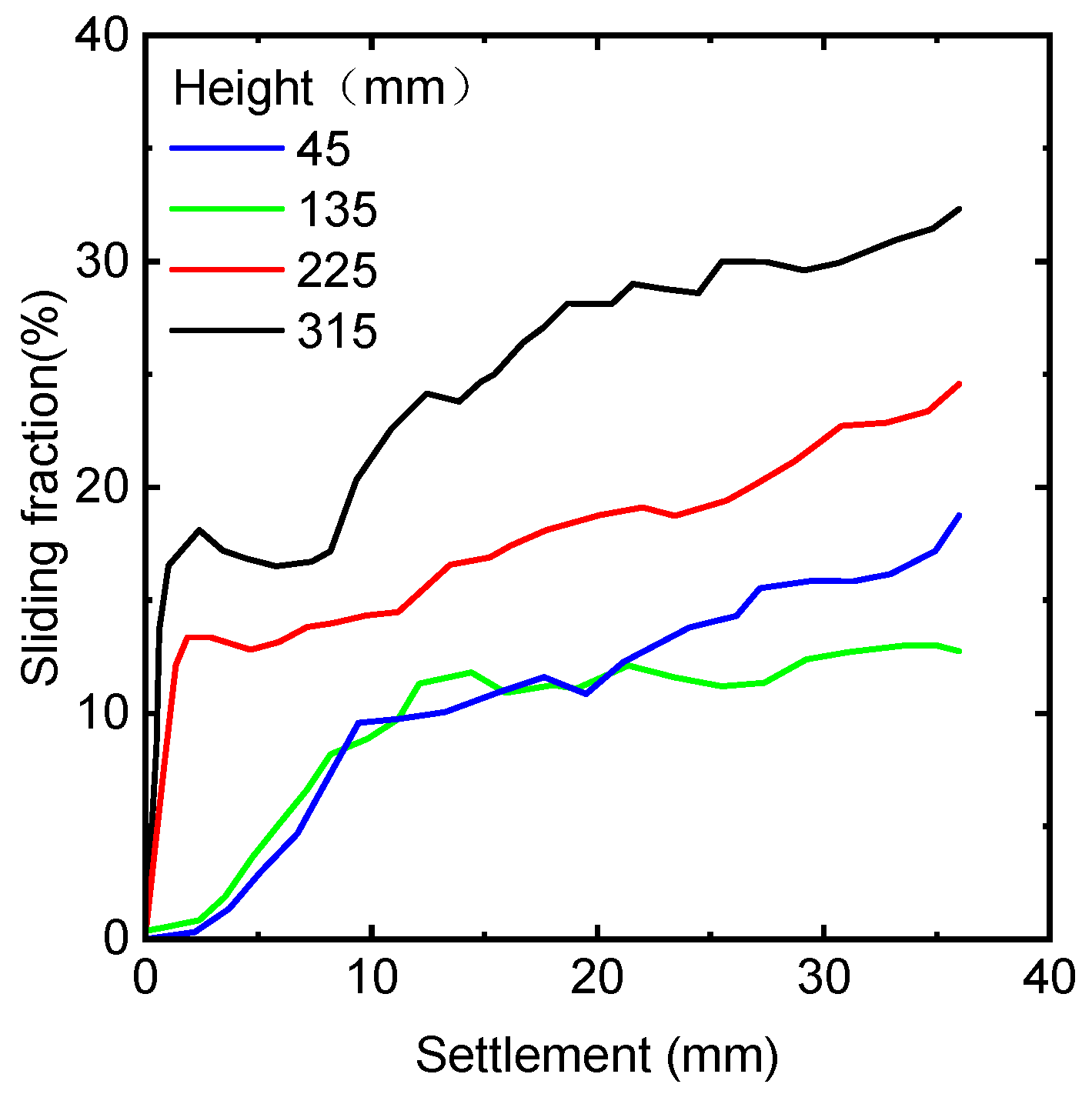
3.1.4. Particle Movement of the FGESC
3.2. Stresses of the Soil around the Column and the Underlying Stratum
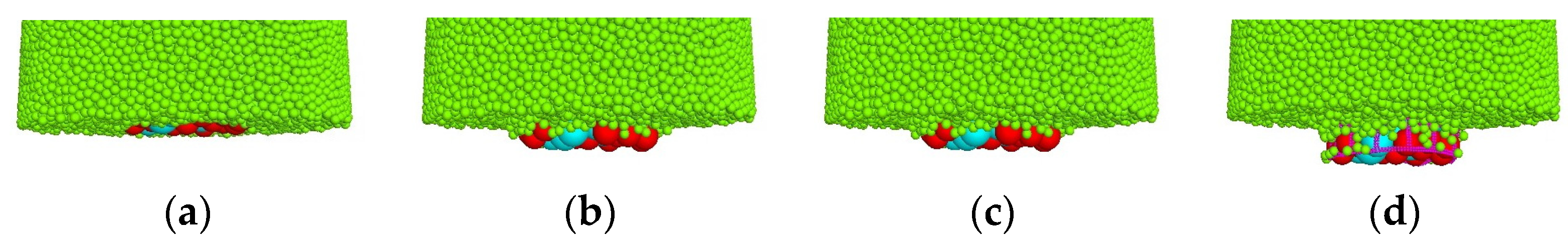
3.3. Failure Mode
3.4. Effect of Encasement Length
3.4.1. Effect on Load-Bearing Capacity of FGESC
3.4.2. Effect on Contact Force of the FGESC
3.4.3. Effect on Failure Mode of the FGESC
4. Conclusions
- (1)
- The FGESC with encasement depth of 280 mm has large vertical and radial stresses at the top region of the column under load. The aggregates in the column top region become loose under load as the porosity increases, the coordination number decreases, and the sliding ratio increases. The force chain of the column is relatively complete and can effectively transfer the upper load downward. When the encasement depth is 280 mm, the failure mode of the FGESC is mainly in the form of penetration failure.
- (2)
- When the encasement depth is 280 mm, the vertical and radial stresses of the soil near the column body are not affected much by the column body. It indicates that the presence of geogrids limits the movement of the aggregates in the column. Therefore, the large stresses of the column cannot be effectively transferred to the soil around the column.
- (3)
- As the encasement depth increases, the bearing capacity of the FGESC increases, the force chain of the column body becomes more complete, and the column can transfer the load downward more effectively. However, when the encasement depth exceeds 280 mm, the bearing capacity of FGESC does not increase much.
- (4)
- The encasement depth to a certain extent controls the failure mode of the FGESC. As the encasement depth increases, the failure mode of the FGESC gradually transitions from swelling deformation to penetration failure.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ali, G.; Iman, H.; Mehdi, S. Deformation and stability analysis of embankment over stone column-strengthened soft ground. KSCE J. Civ. Eng. 2021, 25, 404–416. [Google Scholar]
- Kousik, D.; Narendra, K.S.; Jagtap, B.N. Laboratory model studies on unreinforced and geogrid-reinforced sand bed over stone column-improved soft clay. Geotext. Geomembr. 2011, 29, 190–196. [Google Scholar]
- Lajevardi, S.H.; Shamsi, H.R.; Hamidi, M.; Enami, S. Numerical and experimental studies on single stone columns. Soil Mech. Found. Eng. 2018, 55, 340–345. [Google Scholar] [CrossRef]
- McCabe, B.A.; Nimmons, G.J.; Egan, D. A review of field performance of stone columns in soft soils. Geotech. Eng. 2009, 162, 323–334. [Google Scholar] [CrossRef] [Green Version]
- Gu, M.X.; Han, J.; Zhao, M.H. Three-dimensional DEM analysis of axially loaded geogrid-encased stone column in clay bed. Int. J. Geomech. 2020, 20, 11. [Google Scholar] [CrossRef]
- Almeida, M.S.S.; Hosseinpour, I.; Riccio, M. Performance of a geosynthetic-encased column (GEC) in soft ground: Numerical and analytical studies. Geosynth. Int. 2013, 20, 251–262. [Google Scholar] [CrossRef]
- Ali, K.; Shahu, J.T.; Sharma, K.G. Model tests on single and groups of stone columns with different geosynthetic reinforcement arrangement. Geosynth. Int. 2014, 21, 103–118. [Google Scholar] [CrossRef]
- Xu, Z.Y.; Zhang, L.; Zhou, S. Influence of encasement length and geosynthetic stiffness on the performance of stone column: 3D DEM-FDM coupled numerical investigation. Comput. Geotech. 2021, 132, 15. [Google Scholar] [CrossRef]
- Nagula, S.S.; Nguyen, D.M.; Grabe, J. Numerical modelling and validation of geosynthetic encased columns in soft soils with installation effect. Geotext. Geomembr. 2018, 46, 790–800. [Google Scholar] [CrossRef]
- Dash, S.K.; Bora, M.C. Influence of geosynthetic encasement on the performance of stone columns floating in soft clay. Can. Geotech. J. 2013, 50, 744–765. [Google Scholar] [CrossRef]
- Zhang, L.; Xu, Z.Y.; Zhou, S. Vertical cyclic loading response of geosynthetic-encased stone column in soft clay. Geotextile. Geomembr. 2020, 48, 897–911. [Google Scholar] [CrossRef]
- Yang, F.O.; Fan, G.; Wang, K.F.; Yang, C.; Lyu, W.Q.; Zhang, J.J. A large-scale shaking table model test for acceleration and deformation response of geosynthetic encased stone column composite ground. Geotextile. Geomembr. 2021, 49, 1407–1418. [Google Scholar] [CrossRef]
- Alexiew, D.; Raithel, M.; Küster, V.; Detert, O. 15 years of experience with geotextile encased granular columns as foundation system. In Proceedings of the ISSMGE Technical Committee TC 211 International Symposium on Ground Improvement (IS-GI BRUSSELS 2012), Brussels, Belgium, 31 May–1 June 2012. [Google Scholar]
- Hosseinpour, I.; Almeida, M.S.S.; Riccio, M. Ground improvement of soft soil by geotextile-encased columns. Ground Improv. 2016, 169, 297–305. [Google Scholar] [CrossRef] [Green Version]
- Xu, F.; Moayedi, H.; Foong, L.K.; Moghadam, M.J.; Zangeneh, M. Laboratory and numerical analysis of geogrid encased stone columns. Measurement 2021, 169, 14. [Google Scholar] [CrossRef]
- Deshpande, T.D.; Kumar, S.; Begum, G.; Basha, S.A.K.; Rao, B.H. Analysis of railway embankment supported with geosynthetic-encased stone columns in soft clays: A case study. Int. J. Geosynth. Ground Eng. 2021, 43, 16. [Google Scholar] [CrossRef]
- Farah, R.E.; Nalbantoglu, Z. Behavior of geotextile-encased single stone column in soft soils. Arab. J. Sci. Eng. 2020, 45, 3877–3890. [Google Scholar] [CrossRef]
- Wang, Q.D.; Michal, S.; Gu, X.F.; Miao, Z.P.; Du, W.C.; Zhang, J.; Gang, C. Lubricity and rheological properties of highly dispersed graphite in clay-water-based drilling fluids. Materials 2022, 15, 1083. [Google Scholar] [CrossRef]
- Gao, J.L.; Zhang, Y.P.; Wang, C.H.; Yuan, C. Behavior of characteristics of geosynthetic-encased stone column under cyclic loading. Transp. Geotech. 2021, 28, 100554. [Google Scholar] [CrossRef]
- Zhou, Y.; Kong, G.Q.; Yang, Q.; Li, H. Deformation analysis of geosynthetic-encased stone column using cavity expansion models with emphasis on boundary condition. Geotext. Geomembr. 2019, 47, 831–842. [Google Scholar] [CrossRef]
- Hong, Y.S.; Wu, C.S.; Kou, C.M.; Chang, C.H. A numerical analysis of a fully penetrated encased granular column. Geotext. Geomembr. 2017, 45, 391–405. [Google Scholar] [CrossRef]
- Hataf, N.; Nabipour, N.; Sadr, A. Experimental and numerical study on the bearing capacity of encased stone columns. Int. J. Geo-Eng. 2020, 11, 11. [Google Scholar] [CrossRef] [Green Version]
- Alexiew, D.; Moormann, C.; Jud, H. Foundation of a coal/coke stockyard on soft soil with geotextile encased columns and horizontal reinforcement. In Proceedings of the 17th International Conference on Soil Mechanics and Geotechnical Engineering, Alexandria, Egypt, 5–9 October 2009; pp. 2236–2239. [Google Scholar]
- Cimentada, A.; Costa, A.D.; Jorge, C.; Sagaseta, C. Laboratory study on radial consolidation and deformation in clay reinforced with stone columns. Can. Geotech. J. 2011, 48, 36–52. [Google Scholar] [CrossRef]
- Hasan, M.; Samadhiya, N.K. Experimental and numerical analysis of geosynthetic-reinforced floating granular piles in soft clays. Int. J. Geosynth. Ground Eng. 2016, 22, 13. [Google Scholar] [CrossRef] [Green Version]
- Hasan, M.; Samadhiya, N.K. Performance of geosynthetic-reinforced granular piles in soft clays: Model tests and numerical analysis. Comput. Geotech. 2017, 87, 178–187. [Google Scholar] [CrossRef]
- Najjar, S.S.; Sadek, S.; Lattouf, H.B.; Maalouf, Y. Drained triaxial response of clay reinforced with sand columns. Ground Improv. 2018, 173, 170–186. [Google Scholar] [CrossRef]
- Murugesan, S.; Rajagopal, K. Geosynthetic-encased stone columns: Numerical evaluation. Geotext. Geomembr. 2006, 24, 349–358. [Google Scholar] [CrossRef]
- Murugesan, S.; Rajagopal, K. Model tests on geosynthetic-encased stone columns. Geosynth. Int. 2007, 14, 346–354. [Google Scholar] [CrossRef]
- Murugesan, S.; Rajagopal, K. Studies on the Behavior of Single and Group of Geosynthetic Encased Stone Columns. J. Geotech. Geoenvironmental Eng. 2009, 136, 129–139. [Google Scholar] [CrossRef]
- Ghazavi, M.; Afshar, J.N. Bearing capacity of geosynthetic encased stone columns. Geotext. Geomembr. 2013, 38, 26–36. [Google Scholar] [CrossRef]
- Lateef, A.D.; Mohammad, Y.S. Three-dimensional numerical study on behavior of geosynthetic encased stone column placed in soft soil. Geotech. Geol. Eng. 2021, 39, 1901–1922. [Google Scholar]
- Hosseinpour, I.; Soriano, C.; Almeida, M.S.S. A comparative study for the performance of encased granular columns. J. Rock Mech. Geotech. Eng. 2019, 11, 379–388. [Google Scholar] [CrossRef]
- Chen, J.F.; Wang, X.T.; Xue, J.F.; Zeng, Y.; Feng, S.Z. Uniaxial compression behavior of geotextile encased stone columns. Geotext. Geomembr. 2018, 46, 277–283. [Google Scholar] [CrossRef]
- Gniel, J.; Bouazza, A. Improvement of soft soils using geogrid encased stone columns. Geotext. Geomembr. 2009, 27, 167–175. [Google Scholar] [CrossRef]
- Liu, S.C.; Zhang, D.W.; Song, T.; Zhang, G.L.; Fan, L.B. A method of settlement calculation of ground improved by floating deep mixed columns based on laboratory model tests and finite element analysis. Int. J. Civ. Eng. 2021, 16, 207–222. [Google Scholar] [CrossRef]
- Wang, K.F.; Liu, M.J.; Cao, J.; Niu, J.Y.; Zhuang, Y.X. Bearing characteristics of composite foundation reinforced by geosynthetic-encased stone column: Field tests and numerical analyses. Sustainability 2023, 15, 25. [Google Scholar] [CrossRef]
- Kang, B.; Wang, J.Q.; Zhou, Y.W.; Huang, S.B. Study on bearing capacity and failure mode of multi-layer-encased geosynthetic-encased stone column under dynamic and static loading. Sustainability 2023, 15, 18. [Google Scholar] [CrossRef]
- Liu, M.J.; Wang, K.F.; Niu, J.Y.; Ouyang, F. Static and dynamic load transfer behaviors of the composite foundation reinforced by the geosynthetic-encased stone column. Sustainability 2023, 15, 19. [Google Scholar] [CrossRef]
- Anita, A.; Karthika, S.; Divya, P.V. Construction and demolition waste as valuable resources for geosynthetic-encased stone columns. J. Hazard. Toxic Radioact. Waste 2023, 27, 13. [Google Scholar] [CrossRef]
- Almeida, M.S.S.; Hosseinpour, I.; Riccio, M.; Alexiew, D. Behavior of geotextile-encased granular columns supporting test embankment on soft deposit. J. Geotech. Geoenviron. Eng. 2015, 141, 9. [Google Scholar] [CrossRef]
- Debnath, P.; Dey, A.K. Bearing capacity of geogrid reinforced sand over encased stone columns in soft clay. Geotext. Geomembr. 2017, 45, 653–664. [Google Scholar] [CrossRef]
- Chen, J.F.; Zhang, X.; Yoo, C.; Gu, Z.A. Effect of basal reinforcement on performance of floating geosynthetic encased stone column-supported embankment. Geotext. Geomembr. 2022, 50, 566–580. [Google Scholar] [CrossRef]
- Hu, X.J.; Hu, H.B.; Xie, N.; Huang, Y.J.; Guo, P.P.; Gong, X.N. The effect of grain size heterogeneity on mechanical and microcracking behavior of pre-heated Lac du Bonnet granite using a grain-based model. Rock Mech. Rock Eng. 2023, 1–32. [Google Scholar] [CrossRef]
- Hu, X.J.; Guo, P.P.; Xie, N.; Hu, H.B.; Lei, G.; Ma, J.J.; Gong, X.N. Creep behavior and associated acoustic characteristics of heterogeneous granite containing a single pre-existing flaw using a grain-based parallel-bonded stress corrosion model. Rock Mech. Rock Eng. 2023, 1–34. [Google Scholar] [CrossRef]
- Hu, X.J.; Xie, N.; Zhu, Q.; Chen, L.; Li, P. Modeling damage evolution in heterogeneous granite using digital image-based grain-based model. Rock Mech. Rock Eng. 2020, 53, 4925–4945. [Google Scholar] [CrossRef]
- Hu, X.J.; Gong, X.N.; Hu, H.B.; Guo, P.P.; Ma, J.J. Cracking behavior and acoustic emission characteristics of heterogeneous granite with double pre-existing filled flaws and a circular hole under uniaxial compression: Insights from grain-based discrete element method modeling. Bull. Eng. Geol. Environ. 2022, 81, 162. [Google Scholar] [CrossRef]
- Liu, F.; Guo, P.P.; Hu, H.B.; Zhu, C.W.; Gong, X.N. Loading behavior and soil-structure interaction for a floating stone column under rigid foundation: A DEM study. Geofluids 2021, 13, 9508367. [Google Scholar] [CrossRef]
- Gu, M.X.; Cui, J.; Yuan, J.; Wu, Y.; Li, Y.D.; Mo, H.Z. The stress and deformation of stone column-improved soft clay by discrete element modelling. Eur. J. Environ. Civ. Eng. 2020, 18, 1544–1560. [Google Scholar] [CrossRef]









| Parameter | Value |
|---|---|
| Particle density (g/cm3) | 2.68 |
| Coefficient of particle friction | 0.80 |
| Particle normal stiffness (N/m) | 5.80 × 107 |
| Particle shear stiffness (N/m) | 1.00 × 107 |
| Parameter | Value |
|---|---|
| Particle density (g/cm3) | 0.96 |
| Particle normal stiffness (N/m) | 1.85 × 106 |
| Particle shear stiffness (N/m) | 1.85 × 106 |
| Parallel bond normal stiffness (N/m3) | 2.80 × 1011 |
| Parallel bond shear stiffness (N/m3) | 2.50 × 107 |
| Parallel bond normal strength (N/m2) | 3.00 × 108 |
| Parallel bond shear strength (N/m2) | 3.00 × 108 |
| Parameter | Value |
|---|---|
| Particle density (g/cm3) | 2.68 |
| Coefficient of particle friction | 0.30 |
| Particle normal stiffness (N/m) | 3.80 × 104 |
| Particle shear stiffness (N/m) | 3.80 × 104 |
| Contact bond normal strength (N) | 3.60 |
| Contact bond shear strength (N) | 3.60 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, F.; Guo, P.; Hu, X.; Li, B.; Hu, H.; Gong, X. A DEM Study on Bearing Behavior of Floating Geosynthetic-Encased Stone Column in Deep Soft Clays. Appl. Sci. 2023, 13, 6838. https://doi.org/10.3390/app13116838
Liu F, Guo P, Hu X, Li B, Hu H, Gong X. A DEM Study on Bearing Behavior of Floating Geosynthetic-Encased Stone Column in Deep Soft Clays. Applied Sciences. 2023; 13(11):6838. https://doi.org/10.3390/app13116838
Chicago/Turabian StyleLiu, Feng, Panpan Guo, Xunjian Hu, Baojian Li, Haibo Hu, and Xiaonan Gong. 2023. "A DEM Study on Bearing Behavior of Floating Geosynthetic-Encased Stone Column in Deep Soft Clays" Applied Sciences 13, no. 11: 6838. https://doi.org/10.3390/app13116838







