Resilient Modulus Characterization of Compacted Cohesive Subgrade Soil
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
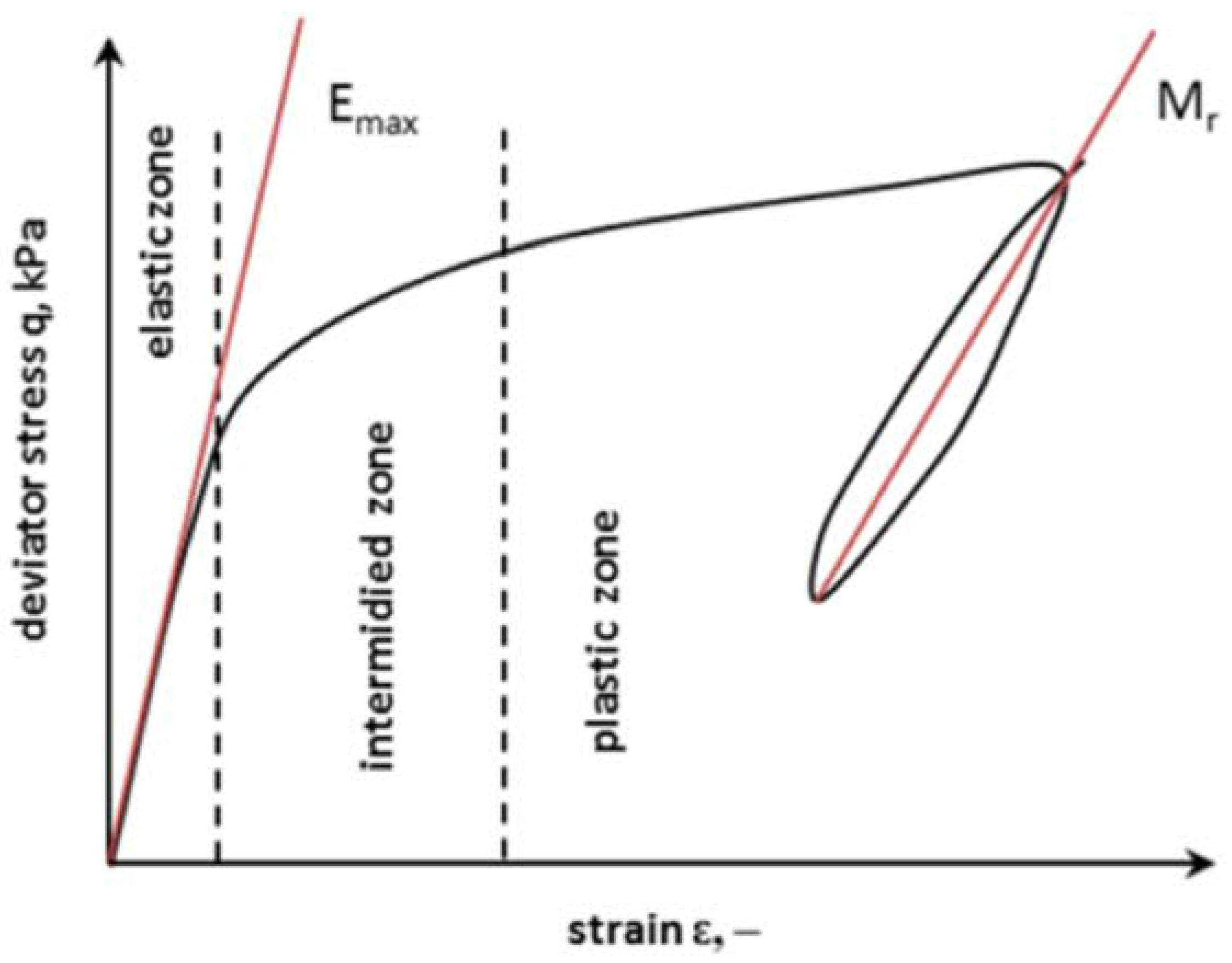
- -
- Plastic shakedown, characterized by a rapid decrease of the plastic strain rate, this phenomena is followed by an equilibrium state and fully resilient strains are observed.
- -
- Plastic creep is observed when plastic deformation are observed in each cycle or in a cumulative plot of plastic deformation against the number of cycles the increase of deformations are observed.
- -
- Incremental collapse, when plastic deformations are great and failure is achieved in a low number of cycles.
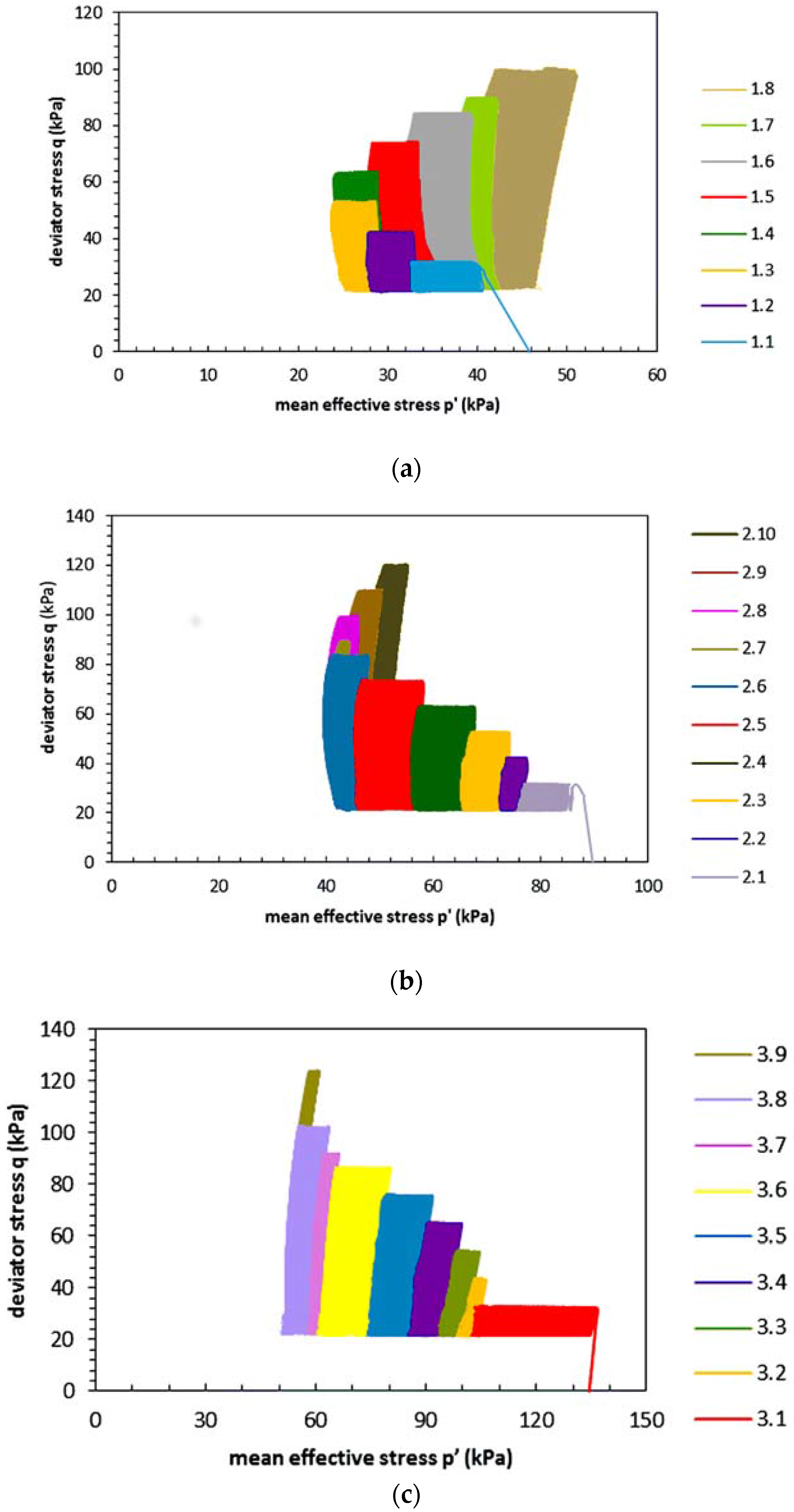
3.1. Pore Pressure Analysis
3.2. Resonant Column Test Results
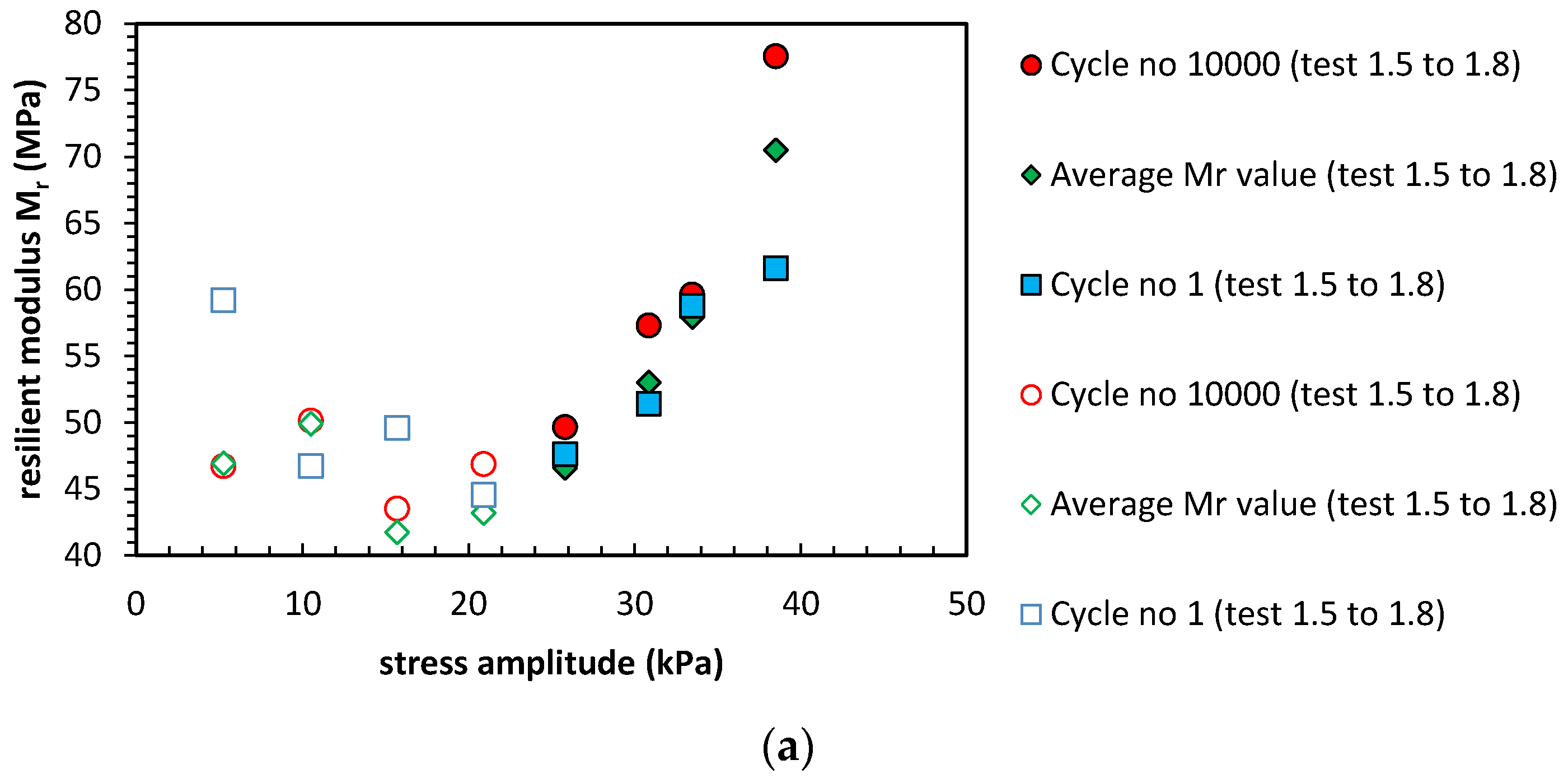
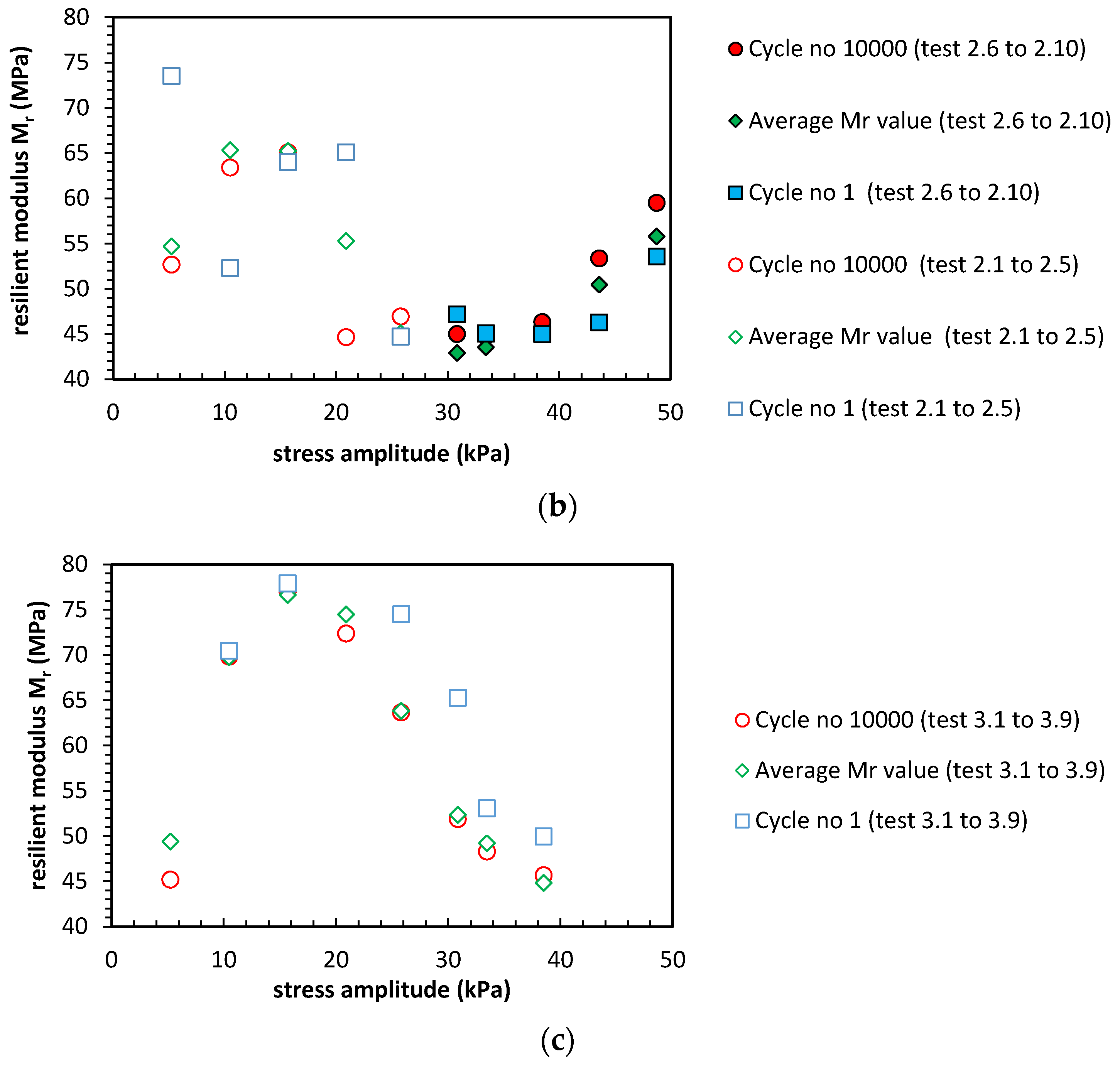
3.3. Analysis of Resilient Modulus Value
3.4. Analytical Model for Mr Calculation
3.5. Maximum Resilient Modulus Value Analysis
4. Conclusions
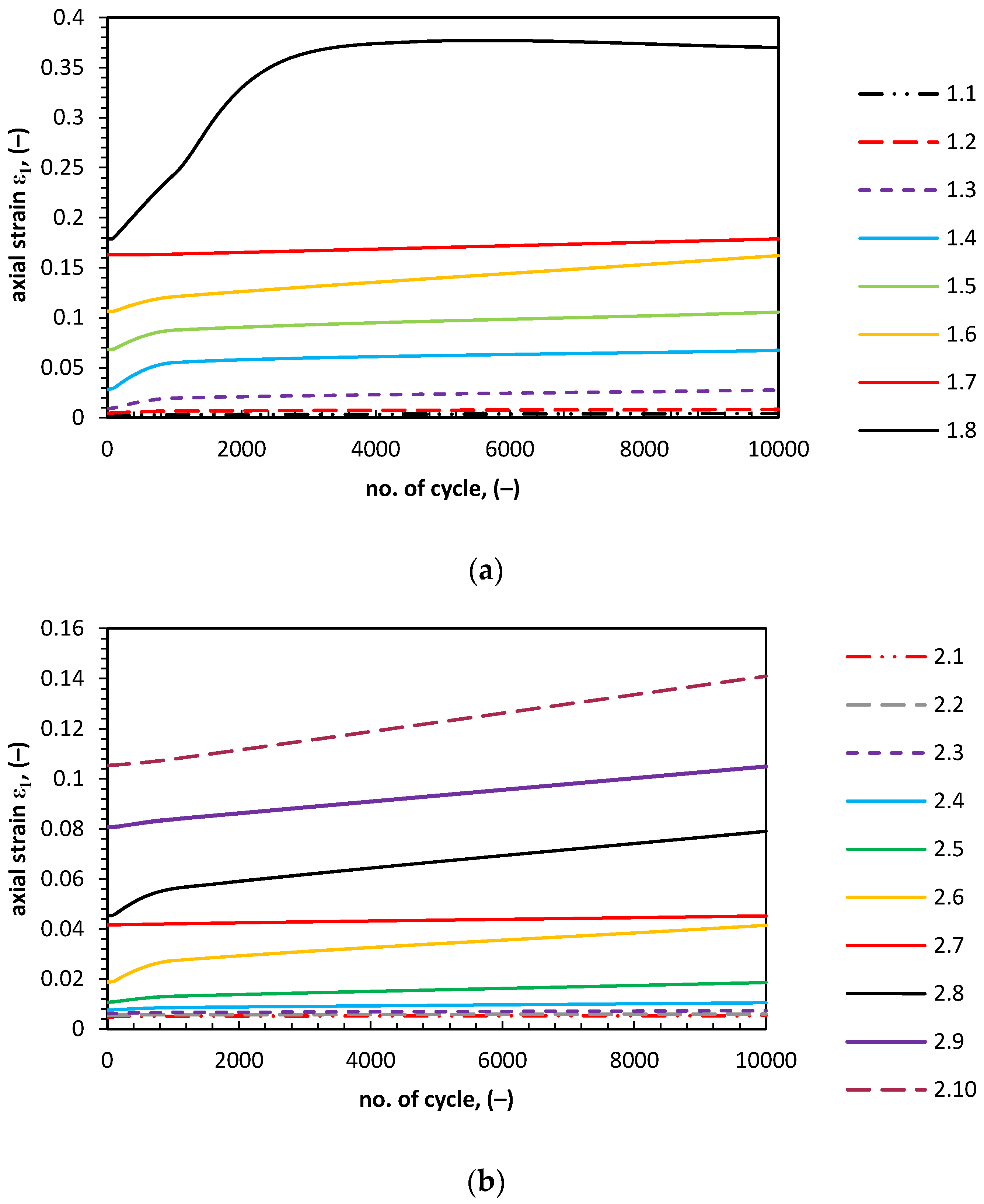
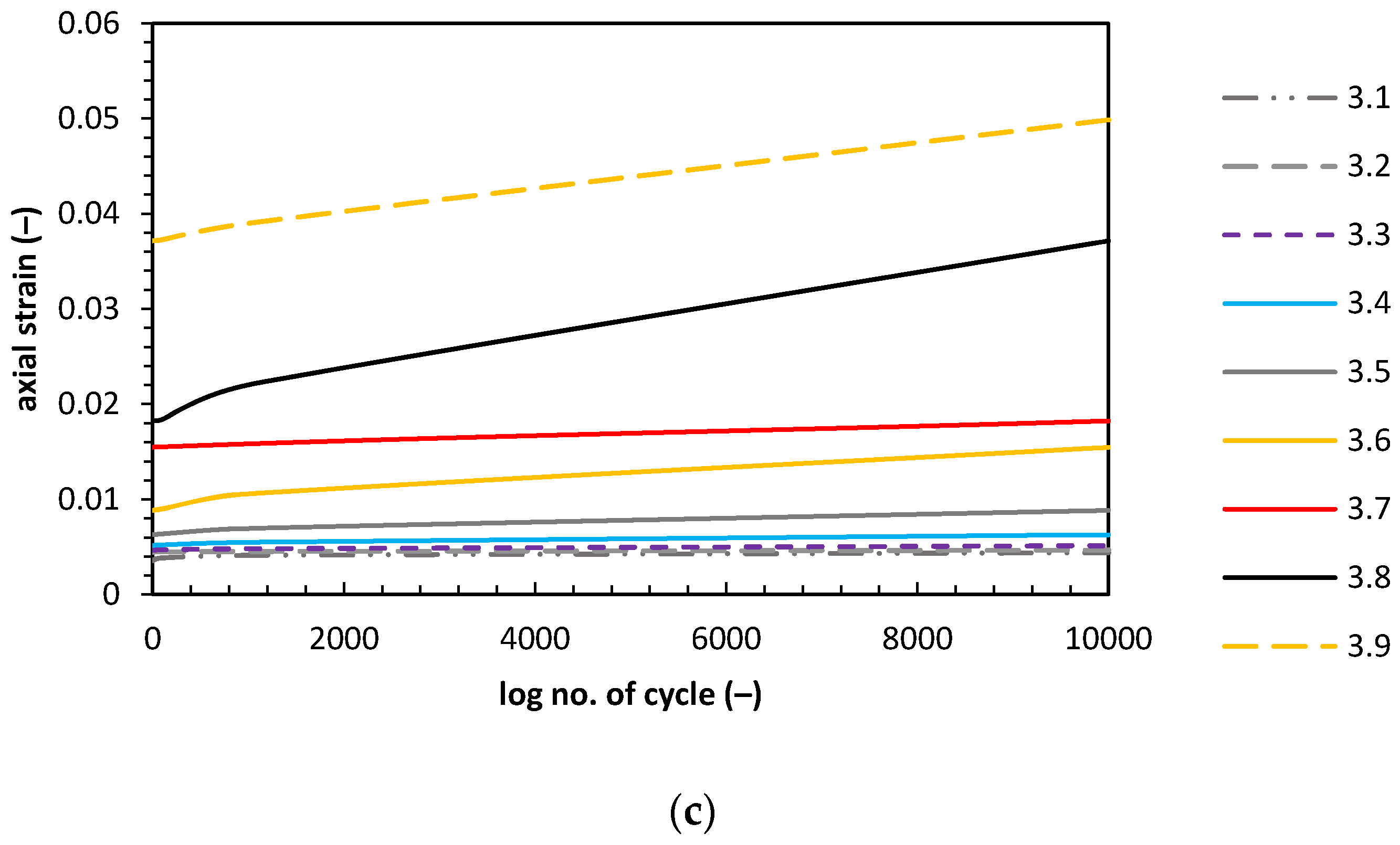
- The strain observed during this test indicates three possible ways of soil the respond to cyclic loading. Under the low deviator stress amplitude, low strain accumulation is observed. In intermediate deviator stress amplitude levels, the strain accumulation was higher, the growth of strain accumulation can be observed. The plastic strain accumulation presents a characteristic growth tendency, which is caused by pore pressure development.
- During the cyclic triaxial tests the stress path moves towards the deviator stress axis in the first few stages. When critical state of soil is achieved, the stress path starts to move in the opposite direction. The critical state did not last during the entire time of the test, which can be observed as plastic strain development during cycling. The strain rate decreases and, after numerous cycles, the purely resilient state can be observed.
- The pore water pressure develops in a similar scenario for three radial stress test conditions. At the first stages of the tests the pore water pressure rises due to the first load cycles, which cause the greatest pore pressure build up. Later, the pore water pressure develops through further stages of cyclic loading until the critical state is achieved. When pore water pressure reaches the equilibrium state, a hardening process begins.
- The maximum value of the Young’s modulus from RCA and TS tests for radial stress σ’3 equal to 45 kPa was 135.5 MPa, and for σ’3 equal to 90 kPa, Emax was 218.2 MPa.
- The recoverable strains characterized by the resilient modulus Mr value in the first cycle was between 44 and 59 MPa for confining pressure σ’3 equal to 45 kPa, between 48 and 78 MPa for σ’3 equal to 90 kPa, and from 44 to 81 MPa for σ’3 equal to 135 kPa. The resilient modulus Mr decrease was caused by plastic strain development.
- The analytical model presented in this article takes into consideration the actual values of effective stress p′ (parameter T), actual excess pore water pressure in reference to initial conditions before cyclic loading Δu1, the loading characteristics (qmax and χ), and the position of the effective stress path (the T parameter).
- The maximum resilient modulus for soil material was characterized. During cyclic loading, soil first degrades, then when Mr max reaches the plateau stage by around 1 × 103 to 2 × 103 cycles, pore pressure reaches equilibrium, and the maximal resilient modulus value starts to increase.
Author Contributions
Conflicts of Interest
References
- Habiballah, T.; Chazallon, C. An elastoplastic model based on the shakedown concept for flexible pavements unboung granular materials. Int. J. Numer. Anal. Methods Geomech. 2005, 29, 577–596. [Google Scholar] [CrossRef]
- Sun, L.; Gu, C.; Wang, P. Effects of cyclic confining pressure on the deformation characteristics of natural soft clay. Soil Dyn. Earthq. Eng. 2015, 78, 99–109. [Google Scholar] [CrossRef]
- Zhou, J.; Gong, X.-N. Strain degradation of saturated clay under cyclic loading. Can. Geotech. J. 2001, 38, 208–212. [Google Scholar] [CrossRef]
- Macijauskas, D.; Van Baars, S. A 3D shear material damping model for man-made vibrations of the ground. In Proceedings of the 13th Baltic Sea Geotechnical Conference, Vilnius, Lithuania, 22–24 September 2016; pp. 159–165. [Google Scholar]
- Guo, L.; Wang, J.; Cai, Y.; Liu, H.; Gao, Y.; Sun, H. Undrained deformation behavior of saturated soft clay under long-term cyclic loading. Soil Dyn. Earthq. Eng. 2013, 50, 28–37. [Google Scholar] [CrossRef]
- Chai, J.C.; Miura, N. Traffic-load-induced permanent deformation of road on subsoil. J. Mech. Geoenviron. Eng. 2002, 128, 907–916. [Google Scholar] [CrossRef]
- Sas, W.; Głuchowski, A. Effects of stabilization with cement on mechanical properties of cohesive soil-sandy-silty clay. Ann. Wars. Univ. Life Sci. SGGW Land Reclam. 2012, 45, 193–205. [Google Scholar] [CrossRef]
- Fall, M.; Sawangsuriya, A.; Benson, C.H.; Edil, T.B.; Bosscher, P.J. On the investigations of resilient modulus of residual tropical gravel lateritic soils from Senegal (West Africa). Geotech. Geol. Eng. 2008, 26, 13–35. [Google Scholar] [CrossRef]
- Chen, H.H.; Marshek, K.M.; Saraf, C.L. Effects of truck tire contact pressure distribution on the design of flexible pavements: A three-dimensional finite element approach. Transp. Res. Rec. 1986, 1095, 72–78. [Google Scholar]
- Brown, S.F. 36th Rankine lecture: Soil mechanics in pavement engineering. Geotechnique 1996, 46, 383–426. [Google Scholar] [CrossRef]
- García-Rojo, R.; Herrmann, H.J. Shakedown of unbound granular material. Granul. Matter 2005, 7, 109–118. [Google Scholar] [CrossRef]
- Głuchowski, A.; Sas, W.; Bąkowski, J.; Szymański, A. Obciążenia cykliczne gruntu spoistego w warunkach bez odpływu. Acta Sci. Pol. Ser. Arch. 2016, 15, 57–77. [Google Scholar]
- Ghadimi, B.; Nega, A.; Nikraz, H. Simulation of shakedown behavior in pavement’s granular layer. Int. J. Eng. Technol. 2015, 7, 198–203. [Google Scholar] [CrossRef]
- Bassani, M.; Khosravifar, S.; Goulias, D.G.; Schwartz, C.W. Long-term resilient and permanent deformation behaviour of Controlled Low-Strength Materials for pavement applications. Transp. Geotech. 2015, 2, 108–118. [Google Scholar] [CrossRef]
- Hardy, M.S.A.; Cebon, D. Response of continuous pavements to moving dynamic loads. J. Eng. Mech. 1993, 119, 1762–1780. [Google Scholar] [CrossRef]
- Kiersnowska, A.; Koda, E.; Fabianowski, W.; Kawalec, J. Effect of the Impact of Chemical and Environmental Factors on the Durability of the High Density Polyethylene (HDPE) Geogrid in a Sanitary Landfill. Appl. Sci. 2017, 7, 22. [Google Scholar] [CrossRef]
- Koda, E.; Szymański, A.; Wolski, W. Field and laboratory experience with the use of strip drains in organic soils. Can. Geotech. J. 1993, 30, 308–318. [Google Scholar] [CrossRef]
- Huhtala, M. COST 337—Unbound granular materials for road pavement. In Proceedings of the 6th International Conference on the Bearing Capacity of Roads and Airfields, Lisbon, Portugal, 24–26 June 2002; Correia, A.G., Branco, F.E., Eds.; ARRB Group Limited: Lisbon, Portugal, 2002. [Google Scholar]
- Andrei, D.; Witczak, M.; Schwartz, C.; Uzan, J. Harmonized resilient modulus test method for unbound pavement materials. Transp. Res. Rec. 2004, 1874, 29–37. [Google Scholar] [CrossRef]
- Lytton, R.L.; Uzan, J.; Fernando, E.G.; Roque, R.; Hiltunen, D.R.; Stoffels, S.M. Development and Validation of Performance Prediction Models and Specifications for Asphalt Binders and Paving Mixtures; Report No. SHRP-A-357, Transportation Research Board 1993; National Research Council: Washington, DC, USA, 1993. [Google Scholar]
- Witczak, M.W.; Uzan, J. The Universal Airport Pavement Design System; Report I of V: Granular Material Characterization; Department of Civil Engineering, University of Maryland: College Park, MD, USA, 1998. [Google Scholar]
- Wang, J.; Guo, L.; Cai, Y.; Xu, C.; Gu, C. Strain and pore pressure development on soft marine clay in triaxial tests with a large number of cycles. Ocean Eng. 2013, 74, 125–132. [Google Scholar] [CrossRef]
- Massarsch, K.R. Deformation properties of fine-grained soils from seismic tests. Keynote lecture. Presented at the International Conference on Site Characterization, ISC’2, Porto, Portugal, 19–22 September 2004. [Google Scholar]
- Sas, W.; Gabryś, K. Laboratory measurement of shear stiffness in resonant column apparatus. Acta Sci. Pol. Ser. Arch. 2012, 12, 39–50. [Google Scholar]
- Atkinson, J.H.; Sallfors, G. Exerimental determination of stress-strain-time characteristics in laboratory and in situ tests. In Proceedings of the Xth International Conference on Soil Mechanics and Foundation Engineering, Florence, Italy, 27–30 May 1991; Volume 3, pp. 915–956. [Google Scholar]
- Lai, C.G.; Rix, G.J. Solution of the rayleigh eigenproblem in viscoelastic materials. Bull. Seismol. Soc. Am. 2002, 92, 2297–2309. [Google Scholar] [CrossRef]
- Sas, W.; Głuchowski, A.; Gabryś, K.; Soból, E.; Szymański, A. Studies on Cyclic and Dynamic Loading on Cohesive Soil in Road Engineering. In Proceedings of the 13th Baltic Sea Geotechnical Conference, Vilnius, Lithuania, 22–24 September 2016; pp. 85–92. [Google Scholar]
- Davich, P.; Labuz, J.; Guzina, B.; Drescher, A. Small Strain and Resilient Modulus Testing of Granular Soils; A Final Report No. 2004-39; Minnesota Department of Transportation: Saint Paul, MN, USA, 2004. [Google Scholar]
- Ba, M.; Tinjum, J.M.; Fall, M. Prediction of permanent deformation model parameters of unbound base course aggregates under repeated loading. Road Mater. Pavement Des. 2015, 16, 854–869. [Google Scholar] [CrossRef]
- Lavasani, M.; Namin, M.L.; Fartash, H. Experimental investigation on mineral and organic fibers effect on resilient modulus and dynamic creep of stone matrix asphalt and continuous graded mixtures in three temperature levels. Constr. Build. Mater. 2015, 95, 232–242. [Google Scholar] [CrossRef]
- Huang, Y.H. Pavement Analysis and Design, 2nd ed.; Pearson Prentice Hall: Bergen County, NJ, USA, 2008. [Google Scholar]
- Sas, W.; Głuchowski, A.; Gabryś, K.; Soból, E.; Szymański, A. Deformation Behavior of Recycled Concrete Aggregate during Cyclic and Dynamic Loading Laboratory Tests. Materials 2016, 9, 780. [Google Scholar] [CrossRef]
- Mehrotra, A.; Abu-Farsakh, M.; Gaspard, K. Development of subgrade Mr constitutive models based on physical soil properties. Road Mater. Pavement Des. 2016, 17, 4. [Google Scholar] [CrossRef]
- Guo, L.; Chen, J.; Wang, J.; Cai, Y.; Deng, P. Influences of stress magnitude and loading frequency on cyclic behavior of K0-consolidated marine clay involving principal stress rotation. Soil Dyn. Earthq. Eng. 2016, 84, 94–107. [Google Scholar] [CrossRef]
- Seed, H.B.; Chan, C.K.; Monismith, C.L. Effects of repeated loading on the strength and deformation of compacted clay. Highw. Res. Board Proc. 1955, 34, 541–558. [Google Scholar]
- Han, Z.; Vanapalli, S.K. State-of-the-Art: Prediction of resilient modulus of unsaturated subgrade soils. Int. J. Geomech. 2016, 16, 04015104. [Google Scholar] [CrossRef]
- Tang, Y.Q.; Cui, Z.Q.; Zhang, X.; Zhao, S.K. Dynamic response and pore pressure model of the saturated soft clay around the tunnel under vibration loading of Shanghai subway. Eng. Geol. 2008, 98, 126–132. [Google Scholar] [CrossRef]
- Cai, Y.; Gu, C.; Wang, J.; Juang, C.H.; Xu, C.; Hu, X. One-way cyclic triaxial behavior of saturated clay: Comparison between constant and variable confining pressure. J. Geotech. Geoenviron. Eng. 2012, 139, 797–809. [Google Scholar] [CrossRef]
- Ba, M.; Fall, M.; Sall, O.; Samb, F. Effect of compaction moisture content on the resilient modulus of unbound aggregates from Senegal (West Africa). Geomaterials 2012, 2, 19–23. [Google Scholar] [CrossRef]
- Hopkins, T.C.; Beckham, T.L.; Sun, C. Resilient Modulus of Compacted Crushed Stone Aggregate Bases; Research Report KTC-05-27/SPR-229-01-1F; Transportation Center, College of Engineering, University of Kentucky: Lexington, KY, USA, 2007. [Google Scholar]
- Uzan, J. Permanent deformation in flexible pavements. J. Transp. Eng. 2004, 130, 6–13. [Google Scholar] [CrossRef]
- Yaghoubi, E.; Disfani, M.M.; Arulrajah, A.; Kodikara, J. Impact of Compaction Methods on Resilient Response of Unsaturated Granular Pavement Material. Procedia Eng. 2016, 143, 323–330. [Google Scholar] [CrossRef]
- Hicks, R.G.; Monismith, C.L. Factors influencing the resilient properties of granular materials. Highw. Res. Rec. 1971, 345, 15–31. [Google Scholar]
- Assimaki, D.; Kausel, E.; Whittle, A. Model for dynamic shear modulus and damping for granular soils. J. Geotech. Geoenviron. Eng. 2000, 126, 859–869. [Google Scholar] [CrossRef]
- Polish Committee for Standardization. Eurokod 7 Projektowanie Geotechniczne Część 2: Rozpoznawanie i Badanie Podłoża Gruntowego; PN-EN 14688-2:2004; Polish Committee for Standardization: Warsaw, Poland, 2004. [Google Scholar]
- Polish Committee for Standardization. Badania Geotechniczne-Badania Laboratoryjne Gruntów-Część 4: Oznaczanie Składu Granulometrycznego; Standards PKN-CEN ISO/TS 17892-4:2009; Polish Committee for Standardization: Warsaw, Poland, 2009. [Google Scholar]
- American Association of State Highway and Transportation Officials. Standard Method of Test for Moisture-Density Relations of Soils Using a 2.5-kg (5.5-lb) Rammer and a 305-mm (12-in.) Drop; AASHTO T99; American Association of State Highway and Transportation Officials: Washington, DC, USA, 2015. [Google Scholar]
- Sas, W.; Szymański, A.; Gabryś, K. The behaviour of natural cohesive soils under dynamic excitations. In Proceedings of the 18th International Conference on Soil Mechanics and Geotechnical Engineering, Paris, France, 2–6 September 2013; Volume 2, pp. 1587–1590. [Google Scholar]
- Gabryś, K.; Sas, W.; Szymański, A. Kolumna rezonansowa jako urządzenie do badań dynamicznych gruntów spoistych. Przegląd Naukowy Inżynieria i Kształtowanie Środowiska 2013, 22, 3–15. [Google Scholar]
- Sas, W.; Gabryś, K.; Soból, E.; Szymański, A. Dynamic Characterization of Cohesive Material Based on Wave Velocity Measurements. Appl. Sci. 2016, 6, 49. [Google Scholar] [CrossRef]
- Wichtmann, T.; Niemunis, A.; Triantafyllidis, T. Strain accumulation in sand due to cyclic loading: Drained triaxial tests. Soil Dyn. Earthq. Eng. 2005, 25, 967–979. [Google Scholar] [CrossRef]
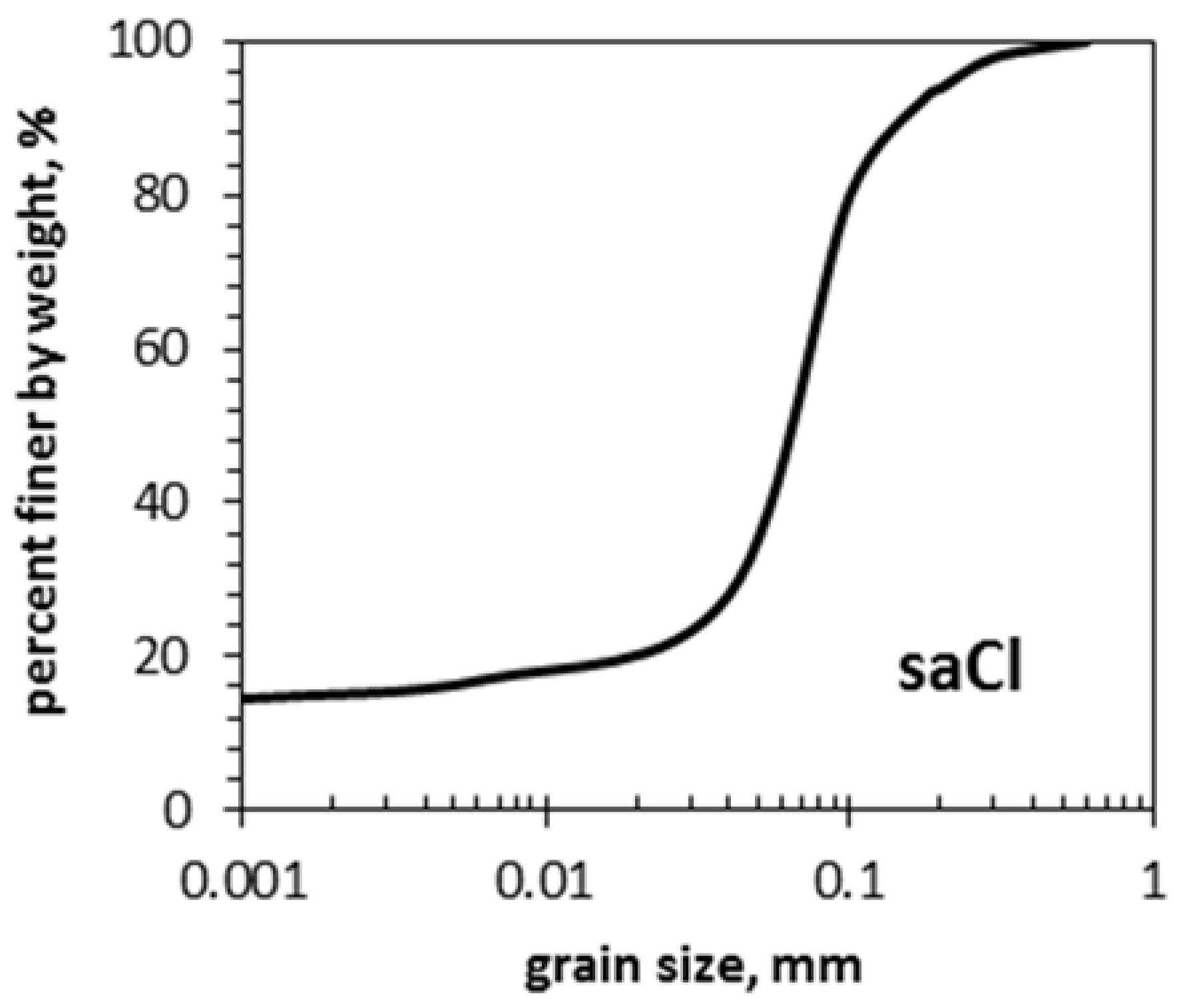








| Properties | Symbol | Value |
|---|---|---|
| Skeleton density | ρs (g∙m−3) | 2.64 |
| Volume density | ρd (g∙m−3) | 2.09 |
| Natural moisture | wn (%) | 12.82 |
| Liquid limit | LL (%) | 18.9 |
| Plasticity limit | PL (%) | 10.3 |
| Plasticity index | PI (-) | 8.6 |
| Void ratio | e0 (-) | 0.41 |
| Optimum moisture content | Wopt (%) | 10.2 |
| Maximum dry unit density | γd max (g/cm3) | 2.09 |
| Caption | σ3 (kPa) | Δq (kPa) | qm (kPa) | qmin (kPa) | qmax (kPa) | qa (kPa) |
|---|---|---|---|---|---|---|
| Test 1.1 | 45 | 10.60 | 47.90 | 21.30 | 31.90 | 5.30 |
| Test 1.2 | 45 | 21.20 | 53.20 | 21.30 | 42.50 | 10.60 |
| Test 1.3 | 45 | 31.40 | 58.70 | 21.50 | 52.90 | 15.70 |
| Test 1.4 | 45 | 41.70 | 64.45 | 21.80 | 63.50 | 20.85 |
| Test 1.5 | 45 | 52.10 | 70.25 | 22.10 | 74.20 | 26.05 |
| Test 1.6 | 45 | 61.90 | 75.55 | 22.30 | 84.20 | 30.95 |
| Test 1.7 | 45 | 67.50 | 78.35 | 22.30 | 89.80 | 33.75 |
| Test 1.8 | 45 | 77.90 | 83.55 | 22.30 | 100.20 | 38.95 |
| Test 1.9 | 45 | 88.70 | 88.75 | 22.20 | 110.90 | 44.35 |
| Test 2.1 | 90 | 10.50 | 47.45 | 21.10 | 31.60 | 5.25 |
| Test 2.2 | 90 | 21.00 | 52.70 | 21.10 | 42.10 | 10.50 |
| Test 2.3 | 90 | 31.40 | 58.30 | 21.30 | 52.70 | 15.70 |
| Test 2.4 | 90 | 41.80 | 63.70 | 21.40 | 63.20 | 20.90 |
| Test 2.5 | 90 | 51.60 | 69.00 | 21.60 | 73.20 | 25.80 |
| Test 2.6 | 90 | 61.70 | 74.45 | 21.80 | 83.50 | 30.85 |
| Test 2.7 | 90 | 66.90 | 77.65 | 22.10 | 89.00 | 33.45 |
| Test 2.8 | 90 | 77.00 | 83.10 | 22.30 | 99.30 | 38.50 |
| Test 2.9 | 90 | 87.20 | 88.40 | 22.40 | 109.60 | 43.60 |
| Test 2.10 | 90 | 97.50 | 93.95 | 22.60 | 120.10 | 48.75 |
| Test 3.1 | 135 | 10.50 | 47.45 | 21.10 | 31.60 | 5.25 |
| Test 3.2 | 135 | 21.00 | 52.70 | 21.10 | 42.10 | 10.50 |
| Test 3.3 | 135 | 31.40 | 58.30 | 21.30 | 52.70 | 15.70 |
| Test 3.4 | 135 | 41.80 | 63.70 | 21.40 | 63.20 | 20.90 |
| Test 3.5 | 135 | 51.60 | 69.00 | 21.60 | 73.20 | 25.80 |
| Test 3.6 | 135 | 61.70 | 74.45 | 21.80 | 83.50 | 30.85 |
| Test 3.7 | 135 | 66.90 | 77.65 | 22.10 | 89.00 | 33.45 |
| Test 3.8 | 135 | 77.00 | 83.10 | 22.30 | 99.30 | 38.50 |
| Test 3.9 | 135 | 97.50 | 93.95 | 22.60 | 120.10 | 48.75 |
| Average Value | Standard Deviation | Variance | Max Value | Min Value | |
|---|---|---|---|---|---|
| Dry soil mass (g) | 1092.0 | 19.8 | 392.3 | 1121.1 | 1067.6 |
| Height (cm) | 13.92 | 0.125 | 0.016 | 14.03 | 13.76 |
| Diameter (cm) | 6.92 | 0.051 | 0.003 | 6.98 | 6.86 |
| Porosity (–) | 0.22 | 0.005 | 0.000 | 0.23 | 0.21 |
| Water mass (g) | 112.28 | 4.707 | 22.155 | 119.60 | 106.70 |
| Full saturation soil mass (g) | 1204.25 | 24.178 | 584.558 | 1240.80 | 1174.30 |
| Soil dry density (g) | 2.09 | 0.003 | 0.000 | 2.09 | 2.08 |
| Moisture (%) | 0.10 | 0.003 | 0.000 | 0.11 | 0.10 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sas, W.; Głuchowski, A.; Gabryś, K.; Soból, E.; Szymański, A. Resilient Modulus Characterization of Compacted Cohesive Subgrade Soil. Appl. Sci. 2017, 7, 370. https://doi.org/10.3390/app7040370
Sas W, Głuchowski A, Gabryś K, Soból E, Szymański A. Resilient Modulus Characterization of Compacted Cohesive Subgrade Soil. Applied Sciences. 2017; 7(4):370. https://doi.org/10.3390/app7040370
Chicago/Turabian StyleSas, Wojciech, Andrzej Głuchowski, Katarzyna Gabryś, Emil Soból, and Alojzy Szymański. 2017. "Resilient Modulus Characterization of Compacted Cohesive Subgrade Soil" Applied Sciences 7, no. 4: 370. https://doi.org/10.3390/app7040370
APA StyleSas, W., Głuchowski, A., Gabryś, K., Soból, E., & Szymański, A. (2017). Resilient Modulus Characterization of Compacted Cohesive Subgrade Soil. Applied Sciences, 7(4), 370. https://doi.org/10.3390/app7040370








