A Jacket-Frame Mounted Oscillating Water Column with a Variable Aperture Skirt
Abstract
:1. Introduction
2. Materials and Methods
2.1. The Model
2.2. Experimental Set-Up and Testing Programme
3. Results
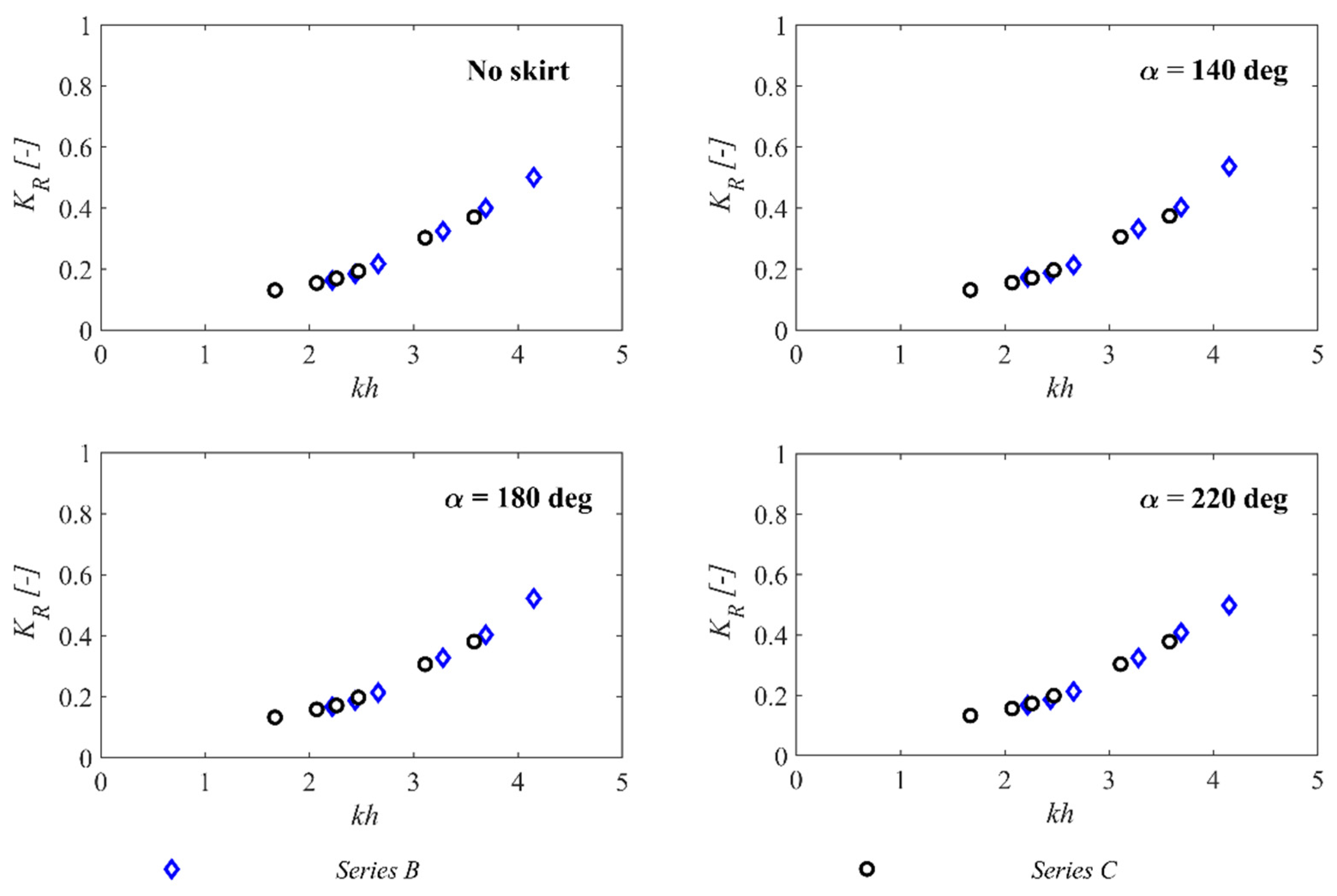
3.1. Reflection and Transmission Analysis
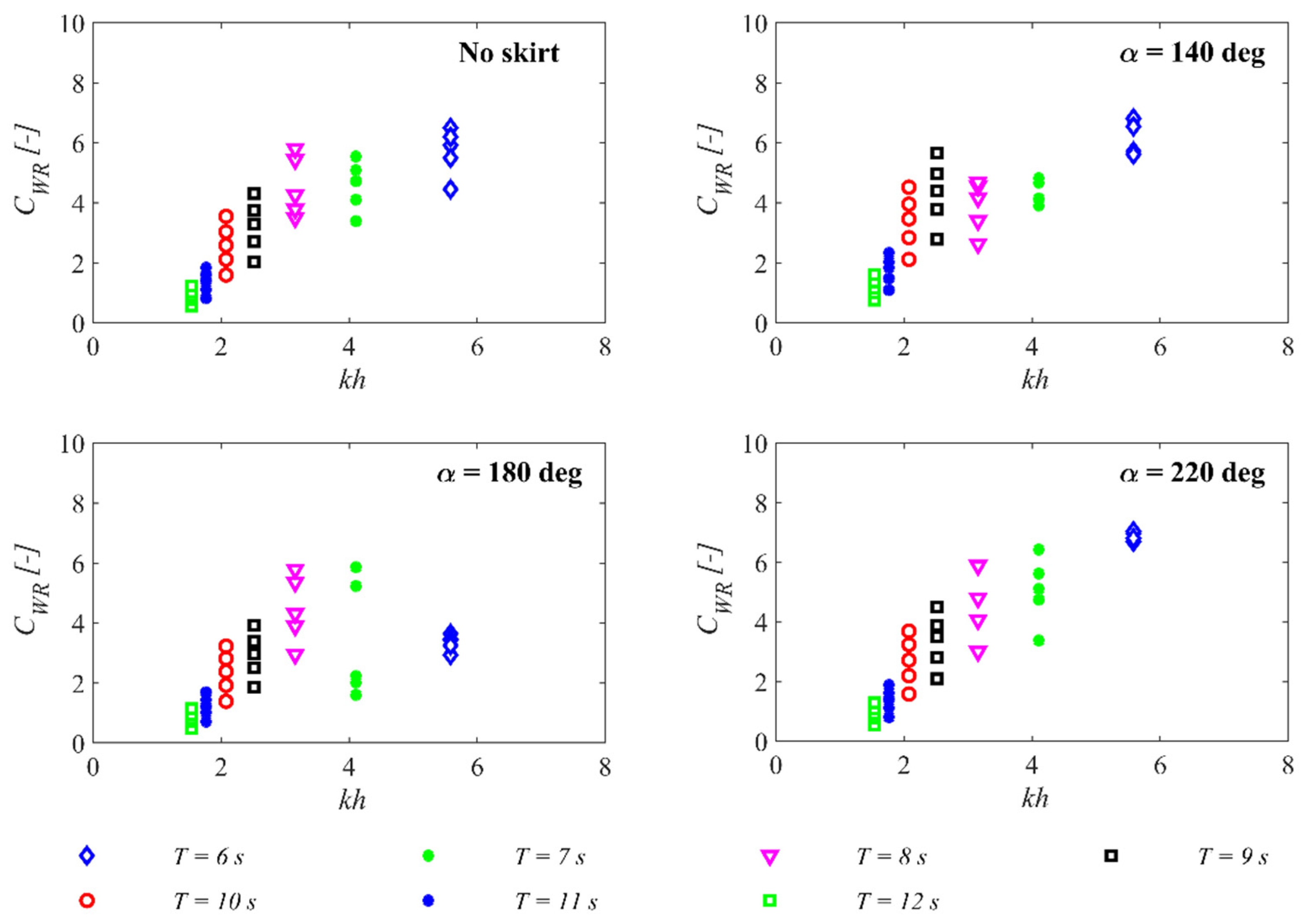
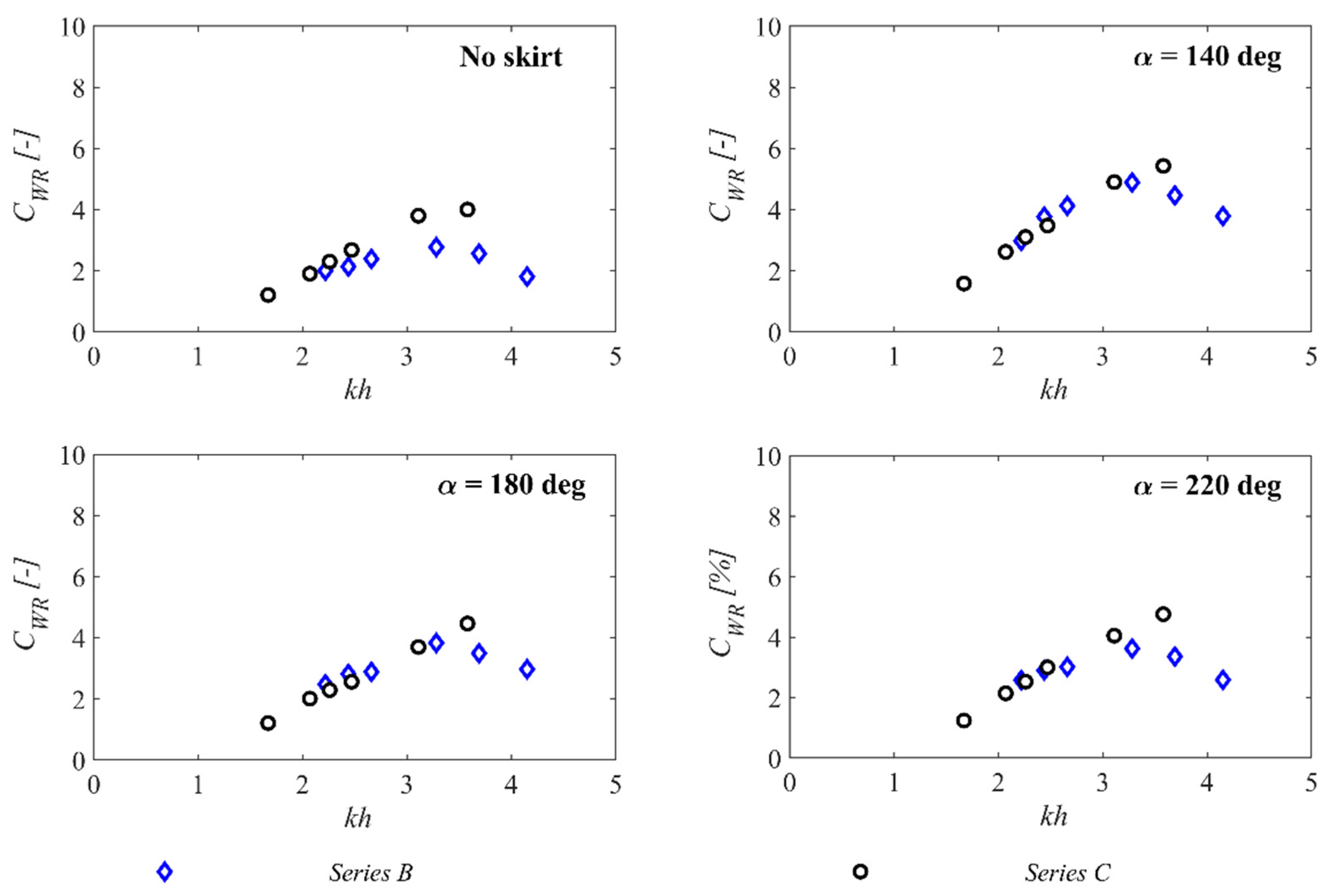
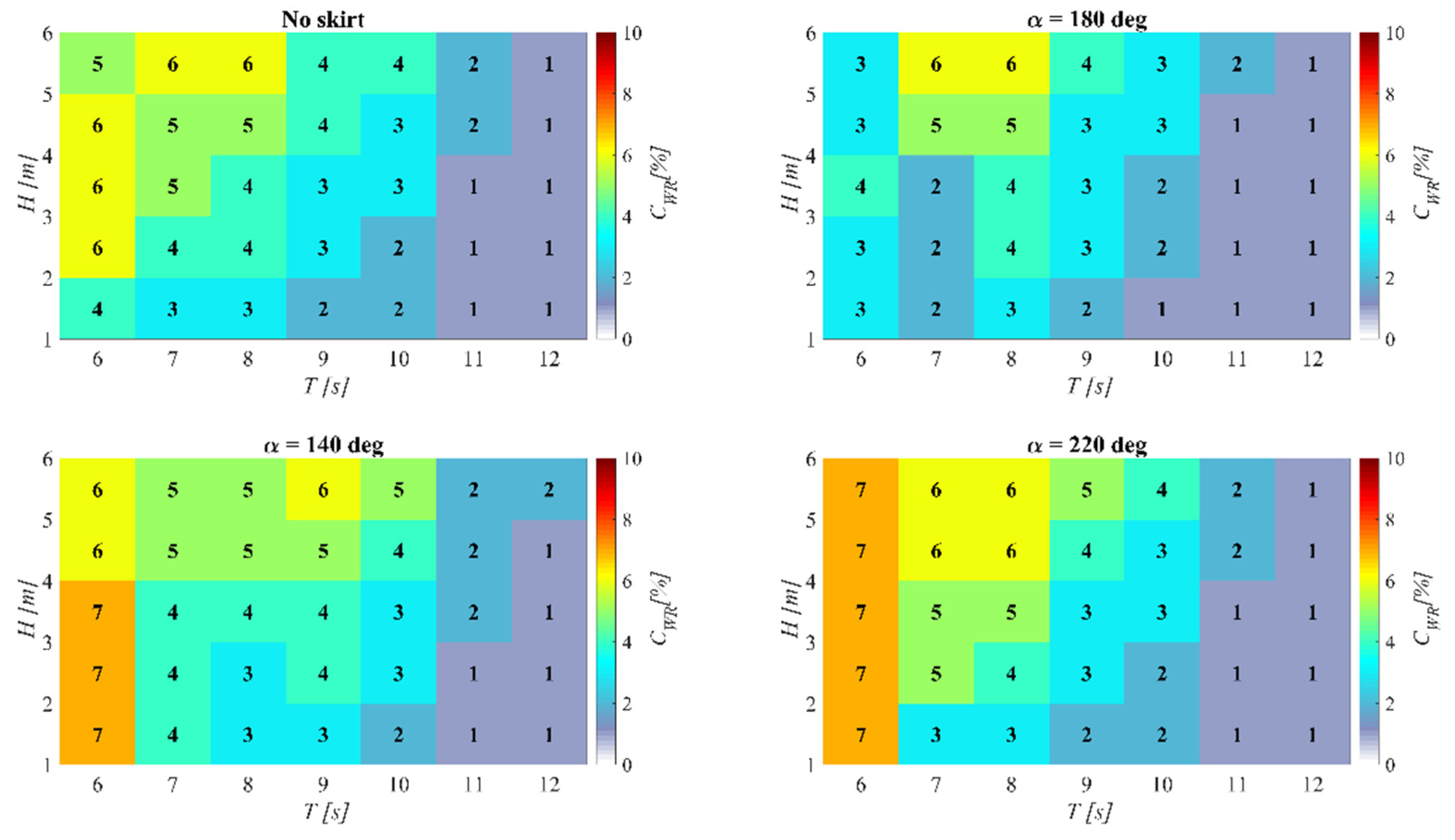
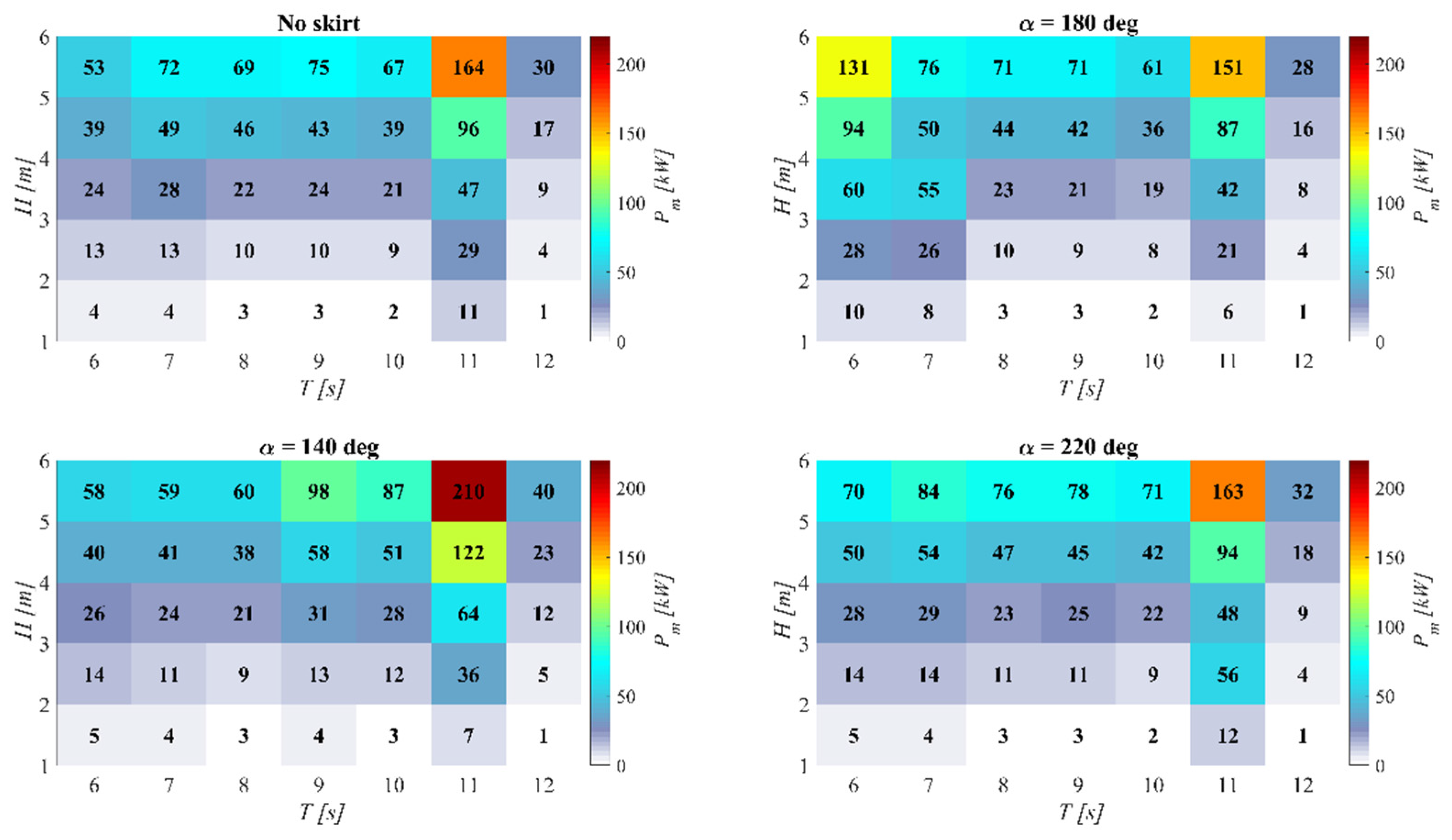
3.2. Hybrid Wind-Wave Device Performance
4. Discussion
5. Conclusions
- The OWC skirt aperture angle configuration was shown to not influence the wave field, meaning that the change in the skirt configuration would influence it.
- The hybrid device reflects between 7% and 50% of the incident waves, while between 6% and 41% is transmitted. It was also found that these values are similar to other WECs and hybrid devices.
- The energy conversion performance of the OWC was improved with a skirt in comparison with that of the device without a skirt.
- The best performance of the hybrid device in terms of capture width ratio occurs for the skirt aperture angle of 140-degrees.
- The region of best performance in the capture-width matrix varies in size significantly with the skirt aperture configuration, with the largest area observed for the 140-degrees aperture angle.
Supplementary Materials
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Weisse, R.; von Storch, H.; Callies, U.; Chrastansky, A.; Feser, F.; Grabemann, I.; Günther, H.; Pluess, A.; Stoye, T.; Tellkamp, J.; et al. Regional Meteorological–Marine Reanalyses and Climate Change Projections. Bull. Am. Meteorol. Soc. 2009, 90, 849–860. [Google Scholar] [CrossRef]
- Kang, J.-N.; Wei, Y.-M.; Liu, L.-C.; Han, R.; Yu, B.-Y.; Wang, J.-W. Energy Systems for Climate Change Mitigation: A Systematic Review. Appl. Energy 2020, 263, 114602. [Google Scholar] [CrossRef]
- Sun, X.; Huang, D.; Wu, G. The Current State of Offshore Wind Energy Technology Development. Energy 2012, 41, 298–312. [Google Scholar] [CrossRef]
- United Nations. Framework Convention on Climate Change Adoption of the Paris Agreement. In Proceedings of the 21st Conference of the Parties, Paris, France, 30 November–13 December 2015. [Google Scholar]
- Jeffrey, H.; Sedgwick, J. ORECCA European Offshore Renewable Energy Roadmap; ORECCA: Munchen, Germany, 2011; pp. 1–201. [Google Scholar]
- Astariz, S.; Perez-Collazo, C.; Abanades, J.; Iglesias, G. Co-Located Wave-Wind Farms: Economic Assessment as a Function of Layout. Renew. Energy 2015, 83, 837–849. [Google Scholar] [CrossRef]
- Stuiver, M.; Soma, K.; Koundouri, P.; van den Burg, S.; Gerritsen, A.; Harkamp, T.; Dalsgaard, N.; Zagonari, F.; Guanche, R.; Schouten, J.J.; et al. The Governance of Multi-Use Platforms at Sea for Energy Production and Aquaculture: Challenges for Policy Makers in European Seas. Sustainability 2016, 8, 333. [Google Scholar] [CrossRef]
- Quevedo, E.; Delory, M.; Castro, A.; Llinas, O.; de Lara, J.; Papandroulakis, N.; Anasrasiadis, P.; Bard, J.; Jeffrey, H.; Ingram, D.; et al. Multi-Use Offshore Platform Configurations in the Scope of the FP7 TROPOS Project. In Proceedings of the 2013 MTS/IEEE OCEANS, Bergen, Norway, 10–14 June 2013; pp. 1–7. [Google Scholar]
- Perez-Collazo, C.; Greaves, D.; Iglesias, G. A Review of Combined Wave and Offshore Wind Energy. Renew. Sustain. Energy Rev. 2015, 42, 141–153. [Google Scholar] [CrossRef]
- Perez-Collazo, C.; Jakobsen, M.M.; Buckland, H.; Fernandez Chozas, J. Synergies for a Wave-Wind Energy Concept. In Proceedings of the EWEA Offshore 2013, Frankfurt, Germany, 19–21 November 2013; pp. 1–10. [Google Scholar]
- Casale, C.; Serri, L.; Stolk, N.; Yildiz, I.; Cantù, M. Synergies, Innovative Designs and Concepts for Multipurpose Use of Conversion Platforms. In Results of ORECCA Project—WP4; ORECCA: Munchen, Germany, 2012; pp. 1–77. [Google Scholar]
- Perez-Collazo, C.; Greaves, D.; Iglesias, G. Proof of Concept of a Novel Hybrid Wind-Wave Energy Converter. In Proceedings of the OMAE2018, Madrid, Spain, 17–22 June 2018; Volume 10. Ocean Renewable Energy. [Google Scholar]
- Qu, Y.; Swales, J.K.; Hooper, T.; Austen, M.C.; Wang, X.; Papathanasopoulou, E.; Huang, J.; Yan, X. Economic Trade-Offs in Marine Resource Use between Offshore Wind Farms and Fisheries in Scottish Waters. Energy Econ. 2023, 125, 106811. [Google Scholar] [CrossRef]
- Cordis Marina Platform. Available online: https://cordis.europa.eu/project/id/241402/es (accessed on 23 September 2022).
- Cordis Mermaid. Available online: https://cordis.europa.eu/project/id/288710/reporting/es (accessed on 23 September 2022).
- Cordis Orecca. Available online: https://cordis.europa.eu/project/id/241421/es (accessed on 23 September 2022).
- Dong, X.; Li, Y.; Li, D.; Cao, F.; Jiang, X.; Shi, H. A State-of-the-Art Review of the Hybrid Wind-Wave Energy Converter. Prog. Energy 2022, 4, 042004. [Google Scholar] [CrossRef]
- Wei, Y.; Bechlenberg, A.; van Rooij, M.; Jayawardhana, B.; Vakis, A.I. Modelling of a Wave Energy Converter Array with a Nonlinear Power Take-off System in the Frequency Domain. Appl. Ocean Res. 2019, 90, 101824. [Google Scholar] [CrossRef]
- Baudry, V.; Babarit, A. Assessment of the Annual Energy Production of a Heaving Wave Energy Converter Sliding on the Mast of a Fixed Offshore Wind Turbine. In Proceedings of the Renewable Energy Congress XI (WREC XI), Abu Dhabi, United Arab Emirates, 25–30 September 2010. [Google Scholar]
- Wan, L.; Gao, Z.; Moan, T.; Lugni, C. Experimental and Numerical Comparisons of Hydrodynamic Responses for a Combined Wind and Wave Energy Converter Concept under Operational Conditions. Renew. Energy 2016, 93, 87–100. [Google Scholar] [CrossRef]
- Li, L.; Gao, Y.; Yuan, Z.; Day, S.; Hu, Z. Dynamic Response and Power Production of a Floating Integrated Wind, Wave and Tidal Energy System. Renew. Energy 2018, 116, 412–422. [Google Scholar] [CrossRef]
- Konispoliatis, D.N.; Katsaounis, G.M.; Manolas, D.I.; Soukissian, T.H.; Polyzos, S.; Mazarakos, T.P.; Voutsinas, S.G.; Mavrakos, S.A. REFOS: A Renewable Energy Multi-Purpose Floating Offshore System. Energies 2021, 14, 3126. [Google Scholar] [CrossRef]
- Iturrioz, A.; Sarmiento, J.; Ayllón, V.; Armesto, J.; Jurado, A.; Guanche, R.; Vidal, C.; Losada, I. Experimental and Numerical Design of a Combined Wind-Wave Concept; Taylor & Francis Books Ltd.: London, UK, 2016; pp. 667–673. [Google Scholar]
- Aubault, A.; Alves, M.; Sarmento, A.J.N.A.; Roddier, D.; Peiffer, A. Modeling of an Oscillating Water Column on the Floating Foundation Windfloat. In Proceedings of the International Conference on Offshore Mechanics and Arctic Engineering, Rotterdam, The Netherlands, 19–24 June 2011; pp. 235–246. [Google Scholar]
- Sarmiento, J.; Iturrioz, A.; Ayllón, V.; Guanche, R.; Losada, I.J. Experimental Modelling of a Multi-Use Floating Platform for Wave and Wind Energy Harvesting. Ocean Eng. 2019, 173, 761–773. [Google Scholar] [CrossRef]
- Zhu, H.; Hu, C.; Sueyoshi, M.; Yoshida, S. Integration of a Semisubmersible Floating Wind Turbine and Wave Energy Converters: An Experimental Study on Motion Reduction. J. Mar. Sci. Technol. 2020, 25, 667–674. [Google Scholar] [CrossRef]
- Zhou, Y.; Ning, D.; Shi, W.; Johanning, L.; Liang, D. Hydrodynamic Investigation on an OWC Wave Energy Converter Integrated into an Offshore Wind Turbine Monopile. Coast. Eng. 2020, 162, 103731. [Google Scholar] [CrossRef]
- Cong, P.; Teng, B.; Bai, W.; Ning, D.; Liu, Y. Wave Power Absorption by an Oscillating Water Column (OWC) Device of Annular Cross-Section in a Combined Wind-Wave Energy System. Appl. Ocean Res. 2021, 107, 102499. [Google Scholar] [CrossRef]
- Dang, T.D.; Phan, C.B.; Truong, H.V.A.; Le, C.D.; Nguyen, M.T.; Ahn, K.-K. A Study on Modeling of a Hybrid Wind Wave Energy Converter System. In Proceedings of the 2016 16th International Conference on Control, Automation and Systems (ICCAS), Gyeongju, Republic of Korea, 16–19 October 2016; pp. 182–187. [Google Scholar]
- Chuan, Q.; Ping, J.; Wen, D. Hybrid Offshore Wind, Wave and Tidal Turbine Energy Conversion System: Structure and Electrical Interface. Proc. CSEE 2014, 34, 2013–2021. [Google Scholar]
- Wang, X.; Zeng, X.; Li, J.; Yang, X.; Wang, H. A Review on Recent Advancements of Substructures for Offshore Wind Turbines. Energy Convers. Manag. 2018, 158, 103–119. [Google Scholar] [CrossRef]
- Lozano-Minguez, E.; Kolios, A.J.; Brennan, F.P. Multi-Criteria Assessment of Offshore Wind Turbine Support Structures. Renew. Energy 2011, 36, 2831–2837. [Google Scholar] [CrossRef]
- Falcão, A.F.O.; Henriques, J.C.C. Oscillating-Water-Column Wave Energy Converters and Air Turbines: A Review. Renew. Energy 2016, 85, 1391–1424. [Google Scholar] [CrossRef]
- Falcão, A.F.O.; Henriques, J.C.C.; Gato, L.M.C. Self-Rectifying Air Turbines for Wave Energy Conversion: A Comparative Analysis. Renew. Sustain. Energy Rev. 2018, 91, 1231–1241. [Google Scholar] [CrossRef]
- Simonetti, I.; Cappietti, L.; Elsafti, H.; Oumeraci, H. Optimization of the Geometry and the Turbine Induced Damping for Fixed Detached and Asymmetric OWC Devices: A Numerical Study. Energy 2017, 139, 1197–1209. [Google Scholar] [CrossRef]
- Hayati, M.; Nikseresht, A.H.; Haghighi, A.T. Sequential Optimization of the Geometrical Parameters of an OWC Device Based on the Specific Wave Characteristics. Renew. Energy 2020, 161, 386–394. [Google Scholar] [CrossRef]
- Boccotti, P. Comparison between a U-OWC and a Conventional OWC. Ocean Eng. 2007, 34, 799–805. [Google Scholar] [CrossRef]
- Rezanejad, K.; Bhattacharjee, J.; Guedes Soares, C. Stepped Sea Bottom Effects on the Efficiency of Nearshore Oscillating Water Column Device. Ocean Eng. 2013, 70, 25–38. [Google Scholar] [CrossRef]
- Howe, D.; Nader, J.-R. OWC WEC Integrated within a Breakwater versus Isolated: Experimental and Numerical Theoretical Study. Int. J. Mar. Energy 2017, 20, 165–182. [Google Scholar] [CrossRef]
- Rezanejad, K.; Souto-Iglesias, A.; Guedes Soares, C. Experimental Investigation on the Hydrodynamic Performance of an L-Shaped Duct Oscillating Water Column Wave Energy Converter. Ocean Eng. 2019, 173, 388–398. [Google Scholar] [CrossRef]
- Daniel Raj, D.; Sundar, V.; Sannasiraj, S.A. Enhancement of Hydrodynamic Performance of an Oscillating Water Column with Harbour Walls. Renew. Energy 2019, 132, 142–156. [Google Scholar] [CrossRef]
- Gaspar, L.A.; Teixeira, P.R.F.; Didier, E. Numerical Analysis of the Performance of Two Onshore Oscillating Water Column Wave Energy Converters at Different Chamber Wall Slopes. Ocean Eng. 2020, 201, 107119. [Google Scholar] [CrossRef]
- Zheng, S.; Zhu, G.; Simmonds, D.; Greaves, D.; Iglesias, G. Wave Power Extraction from a Tubular Structure Integrated Oscillating Water Column. Renew. Energy 2020, 150, 342–355. [Google Scholar] [CrossRef]
- Zhou, Y.; Zhang, C.; Ning, D. Hydrodynamic Investigation of a Concentric Cylindrical OWC Wave Energy Converter. Energies 2018, 11, 985. [Google Scholar] [CrossRef]
- Wan, C.; Yang, C.; Fang, Q.; You, Z.; Geng, J.; Wang, Y. Hydrodynamic Investigation of a Dual-Cylindrical OWC Wave Energy Converter Integrated into a Fixed Caisson Breakwater. Energies 2020, 13, 896. [Google Scholar] [CrossRef]
- Collazo, C.P.; Rodriguez, J.G.I.; Greaves, D.; University of Plymouth. Wave Energy Capture Device. International Patent Application No. PCT/GB2016/051403, 24 November 2016. [Google Scholar]
- Perez-Collazo, C.; Greaves, D.; Iglesias, G. A Novel Hybrid Wind-Wave Energy Converter for Jacket-Frame Substructures. Energies 2018, 11, 637. [Google Scholar] [CrossRef]
- Hughes, S.A. Physical Models and Laboratory Techniques in Coastal Engineering; World Scientific Publishing Co. Pte. Ltd.: Singapore, 1993; ISBN 981-02-1540-1. [Google Scholar]
- Sarmento, A.J.N.A.; Falcão, A.F.d.O. Wave Generation by an Oscillating Surface-Pressure and Its Applications in Wave Energy Extraction. J. Fluid Mech. 1985, 150, 467–485. [Google Scholar] [CrossRef]
- Weber, J.W. Representation of Non-Linear Aero-Thermodynamic Effects during Small Scale Physical Modelling of OWC WECs. In Proceedings of the 7th European Wave and Tidal Energy Conference, Porto, Portugal, 11–13 September 2007; pp. 11–14. [Google Scholar]
- Falcão, A.F.d.O.; Henriques, J.C.C. Model-Prototype Similarity of Oscillating-Water-Column Wave Energy Converters. Int. J. Mar. Energy 2014, 6, 18–34. [Google Scholar] [CrossRef]
- Elhanafi, A.; Macfarlane, G.; Fleming, A.; Leong, Z. Scaling and Air Compressibility Effects on a Three-Dimensional Offshore Stationary OWC Wave Energy Converter. Appl. Energy 2017, 189, 1–20. [Google Scholar] [CrossRef]
- Simonetti, I.; Cappietti, L.; Elsafti, H.; Oumeraci, H. Evaluation of Air Compressibility Effects on the Performance of Fixed OWC Wave Energy Converters Using CFD Modelling. Renew. Energy 2018, 119, 741–753. [Google Scholar] [CrossRef]
- Morris-Thomas, M.T.; Irvin, R.J.; Thiagarajan, K.P. An Investigation into the Hydrodynamic Efficiency of an Oscillating Water Column. J. Offshore Mech. Arct. Eng. 2007, 129, 273–278. [Google Scholar] [CrossRef]
- Dizadji, N.; Sajadian, S.E. Modeling and Optimization of the Chamber of OWC System. Energy 2011, 36, 2360–2366. [Google Scholar] [CrossRef]
- Sheng, W.; Lewis, A.; Alcorn, R. On Wave Energy Extraction of Oscillating Water Column Device. In Proceedings of the 4th International Conference on Ocean Energy, Dublin, Ireland, 17–19 October 2012. [Google Scholar]
- Hann, M.; Perez-Collazo, C. Chapter 7: Physical Modelling. In Wave and Tidal Energy; Greaves, D., Iglesias, G., Eds.; John Wiley & Sons Ltd.: West Sussex, UK, 2018; pp. 233–288. ISBN 978-1-119-01444-7. [Google Scholar]
- Nielsen, K. Annex II Report 2003. In Development of Recommended Practices for Testing and Evaluating Ocean Energy Systems; IEA—Ocean Energy Systems: Lisbon, Portugal, 2003; pp. 1–62. [Google Scholar]
- Mansard, E.P.; Funke, E.R. The Measurement of Incident and Reflected Spectra Using a Least Squares Method. In Proceedings of the International Conference on Coastal Engineering (ICCE), Sydney, Australia, 23–28 March 1980; Volume 1. [Google Scholar]
- Baquerizo, A.; Losada, M.A.; Smith, J.M. Wave Reflection from Beaches: A Predictive Model. J. Coast. Res. 1998, 14, 291–298. [Google Scholar]
- Fusco, F.; Nolan, G.; Ringwood, J.V. Variability Reduction through Optimal Combination of Wind/Wave Resources—An Irish Case Study. Energy 2010, 35, 314–325. [Google Scholar] [CrossRef]

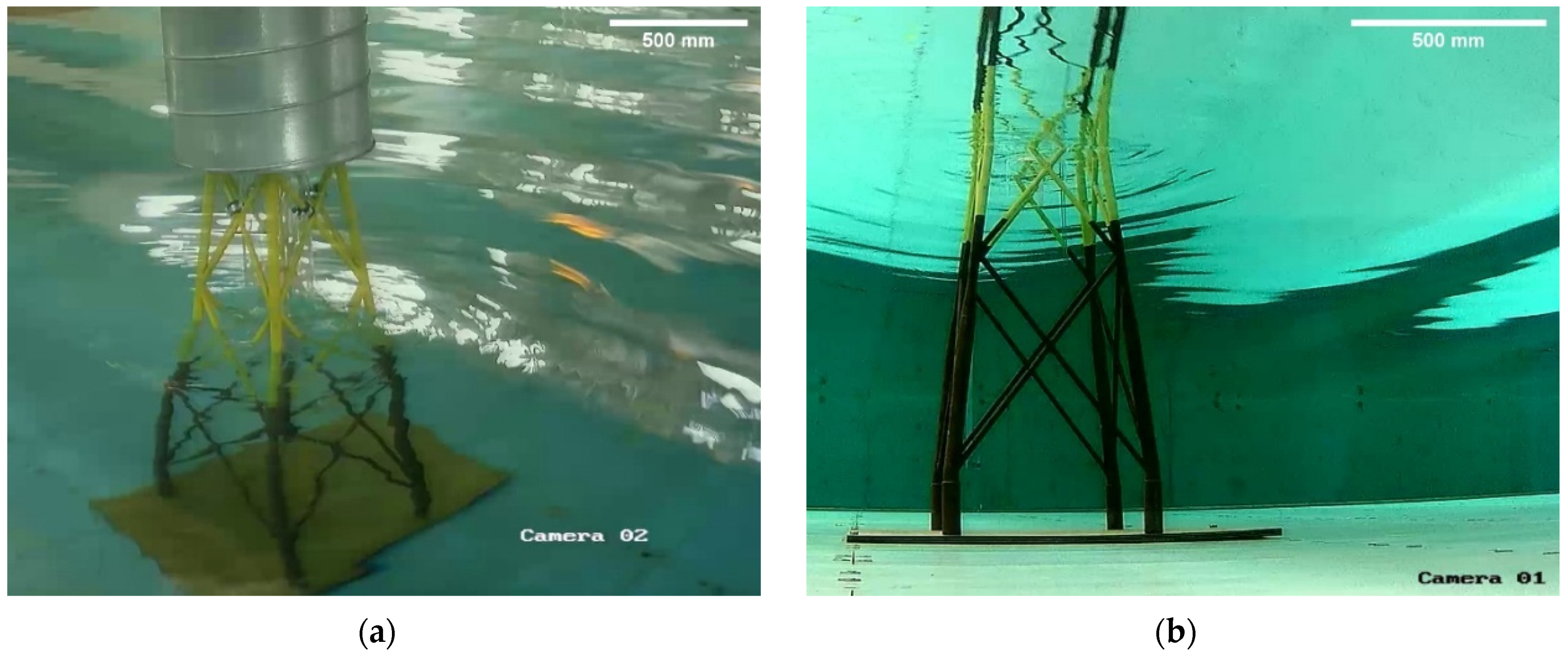
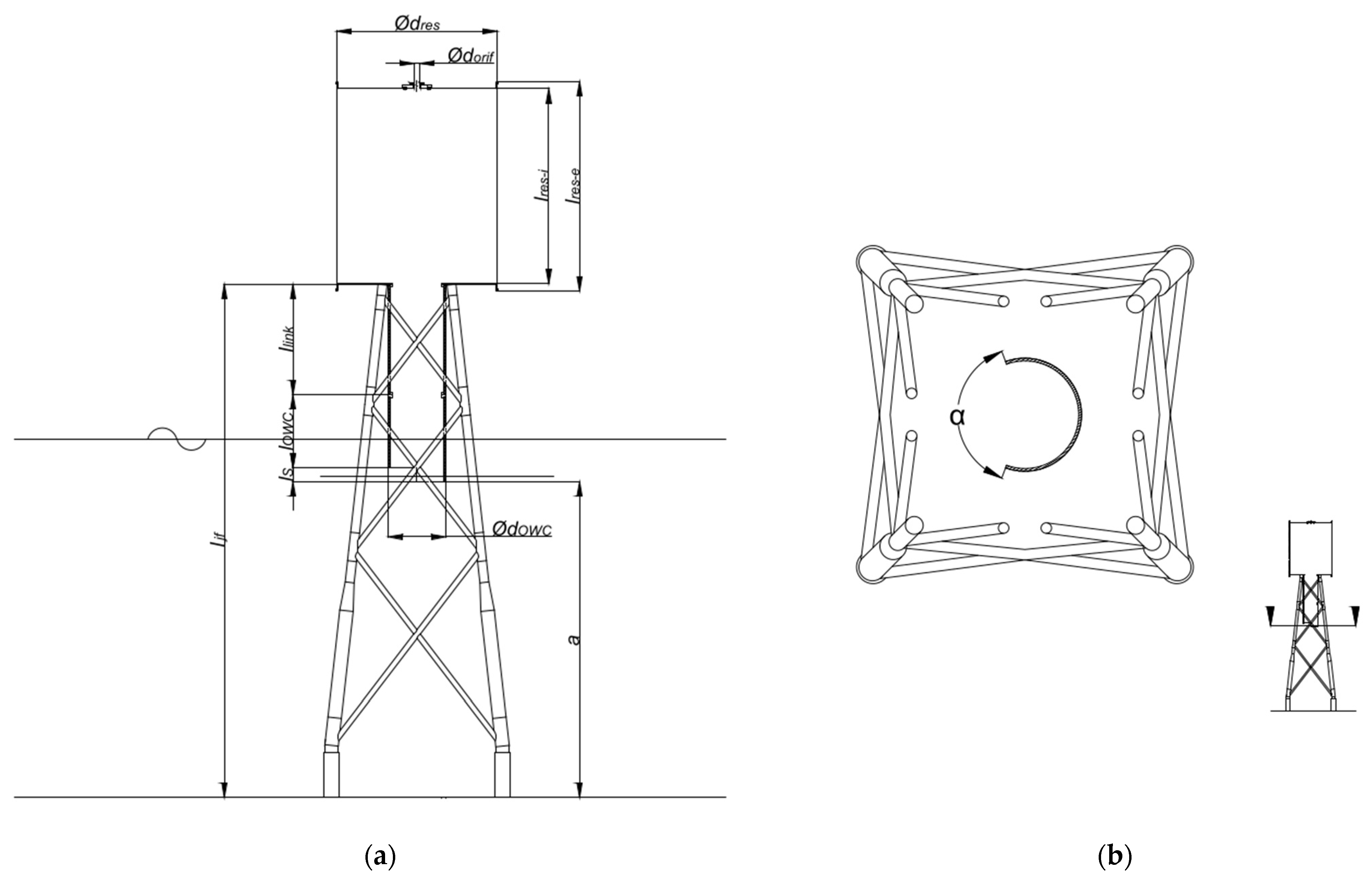
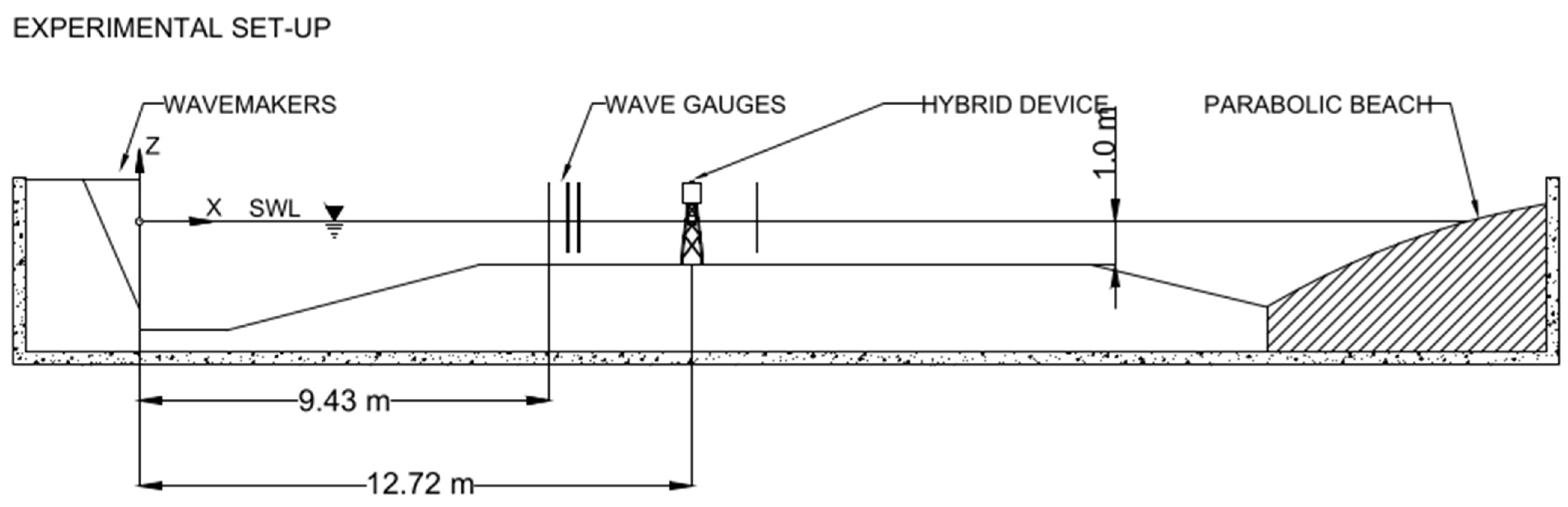


| Parameter | Symbol | Dimension |
|---|---|---|
| OWC air part external diameter | 450 mm | |
| OWC air part external length | 585 mm | |
| OWC air part internal length | 545 mm | |
| OWC air part wall-thickness | 1.5 mm | |
| OWC chamber draught | c | 80 mm |
| OWC chamber external diameter | 160 mm | |
| OWC chamber length | 200 mm | |
| OWC chamber-reservoir link length | 294 mm | |
| OWC chamber wall-thickness | 4 mm | |
| OWC orifice diameter | 15 mm | |
| Jacket-frame length | 1438 mm | |
| Jacket-frame base width | 525 mm | |
| Skirt length | 40 mm | |
| Skirt angle | 140-180-220 deg | |
| Distance to the floor | a | 884 mm |
| Water depth | H | 1000 mm |
| Parameter | X [m] |
|---|---|
| WG1 | 9.430 |
| WG2 | 9.870 |
| WG3 | 10.120 |
| WG4 | 14.100 |
| WG5 (OWC) | 12.590 |
| Centre of the model | 12.720 |
| Series A | T [s] | |||||||
|---|---|---|---|---|---|---|---|---|
| 6 | 7 | 8 | 9 | 10 | 11 | 12 | ||
| H [m] | 1.5 | SA01 | SA06 | SA11 | SA16 | SA21 | SA26 | SA31 |
| 2.5 | SA02 | SA07 | SA12 | SA17 | SA22 | SA27 | SA32 | |
| 3.5 | SA03 | SA08 | SA13 | SA18 | SA23 | SA28 | SA33 | |
| 4.5 | SA04 | SA09 | SA14 | SA19 | SA24 | SA29 | SA34 | |
| 5.5 | SA05 | SA10 | SA15 | SA20 | SA25 | SA30 | SA35 | |
| Series | Test Number | HS | TE | TZ | TP |
|---|---|---|---|---|---|
| Series B | SB01 | 0.5 m | 6.05 s | 5.04 s | 7.06 s |
| SB02 | 1.5 m | 6.49 s | 5.41 s | 7.57 s | |
| SB03 | 2.5 m | 6.98 s | 5.82 s | 8.14 s | |
| SB04 | 3.5 m | 8.00 s | 6.67 s | 9.33 s | |
| SB05 | 4.5 m | 8.48 s | 7.05 s | 9.87 s | |
| SB06 | 5.5 m | 9.10 s | 7.58 s | 10.62 s | |
| Series C | SC01 | 3.5 m | 6.60 s | 5.50 s | 7.70 s |
| SC02 | 7.20 s | 6.00 s | 8.40 s | ||
| SC03 | 8.40 s | 7.00 s | 9.80 s | ||
| SC04 | 9.00 s | 7.50 s | 10.50 s | ||
| SC05 | 9.60 s | 8.00 s | 11.20 s | ||
| SC06 | 11.40 s | 9.50 s | 13.30 s |
| WG1 | WG2 | WG3 | WG4 | OWC | ∆p | ||
|---|---|---|---|---|---|---|---|
| Regular waves | R2 | 0.996 | 0.996 | 0.996 | 0.995 | 0.996 | 0.965 |
| NRMSE | 3.30% | 3.04% | 2.96% | 3.27% | 3.68% | 5.10% | |
| Irregular waves | R2 | 0.972 | 0.971 | 0.971 | 0.957 | 0.957 | 0.951 |
| NRMSE | 3.28% | 3.69% | 3.38% | 4.55% | 4.18% | 3.70% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pérez-Collazo, C.; Greaves, D.M.; Iglesias, G. A Jacket-Frame Mounted Oscillating Water Column with a Variable Aperture Skirt. J. Mar. Sci. Eng. 2023, 11, 2383. https://doi.org/10.3390/jmse11122383
Pérez-Collazo C, Greaves DM, Iglesias G. A Jacket-Frame Mounted Oscillating Water Column with a Variable Aperture Skirt. Journal of Marine Science and Engineering. 2023; 11(12):2383. https://doi.org/10.3390/jmse11122383
Chicago/Turabian StylePérez-Collazo, Carlos, Deborah M. Greaves, and Gregorio Iglesias. 2023. "A Jacket-Frame Mounted Oscillating Water Column with a Variable Aperture Skirt" Journal of Marine Science and Engineering 11, no. 12: 2383. https://doi.org/10.3390/jmse11122383









