“Romeo and Juliet: A Love out of the Shell”: Using Storytelling to Address Students’ Misconceptions and Promote Modeling Competencies in Science
Abstract
1. Introduction
2. Theoretical Background
2.1. Students’ Misconceptions about the Atomic Models
2.2. The Role of Narrative and Storytelling
- -
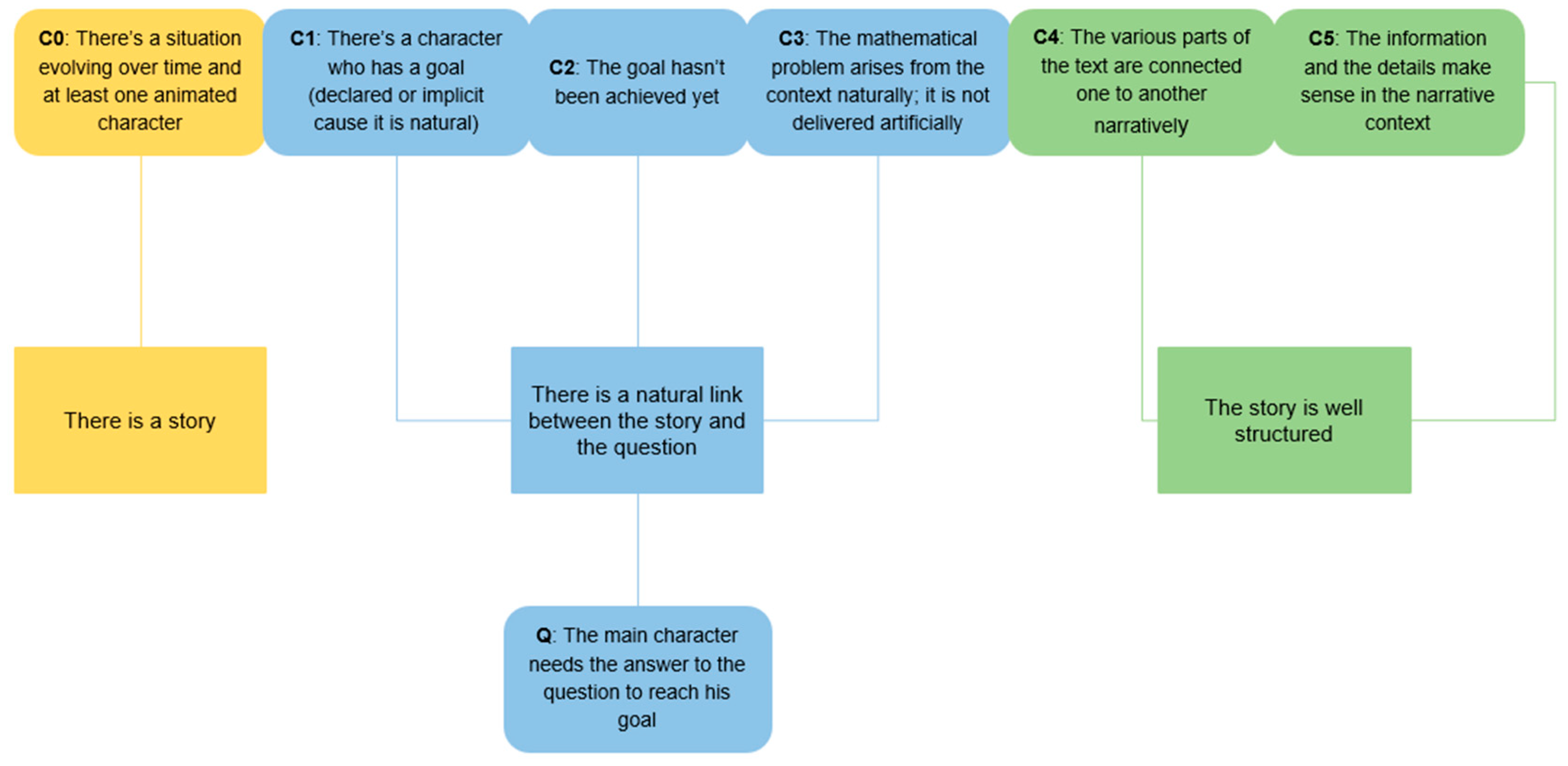
- narrative diachronicity: a story needs to be plunged into a temporal dimension as to be present in a problem;
- -
- normativeness: a story is about five key elements: at least one character, a goal to be achieved, an action, a useful tool to accomplish the action, and a specific situation as a set for the action.
2.3. Modeling Competency
2.4. Inquiry-Based Science Education
3. Method
3.1. The Story Problem
| PROLOGUE | |
| Prologue | Rationale of design principles |
| Romeo is a bold and dynamic electron found in an atom with seven energy levels. He is at the 4s energy level, together with the faithful Mercutio, his companion on raids. Always upside down compared to him, but then there is no place for two equal electrons in their crew. The two are part of the Montague family, known for being particularly lively. Juliet is an electron in 2s, she is more tied to her nucleus and in fact she is a Capulet, a rival family to that of the Montagues and decidedly more calm. Juliet is always accompanied by her nurse; they too are turned upside down with respect to each other. There is a grand ball to which everyone is invited, and, to better organize their arrangement, there is a need to schematize their position. Discuss with your classmates what should be the design of the atom where the two families «are» and build a model. | As highlighted in Section 2.1, some misconceptions can arise in students’ understanding of atomic models. To identify these misconceptions, the initial part of the story involves students in identifying the main characteristics of atoms and modeling them. Consequently, they are encouraged to connect their prior knowledge with the essential elements of the narrative. Notably, the story provides subtle hints, such as Romeo being depicted as a bold and dynamic electron (an idea students are familiar with due to their understanding of electrons moving around nuclei) or his friendship with Mercutio, with whom he exhibits a contrasting relationship (which relates to students’ knowledge of the Pauli Exclusion Principle from their background in chemistry). Meanwhile, Juliet is portrayed as more closely tied to the nucleus since she resides in a 2s orbital. In this context, the teacher’s objective is to pinpoint any misconceptions related to the atomic model that students may have developed from their previous studies. Following the C&Q model, the Prologue introduces the characters and immerses them in a plot, featuring a possible twist—the grand ball. The specific location of the grand ball remains implicit, as it is challenging to conceive of electrons dancing outside the metaphorical context of “moving swiftly”. However, all the other character details are essential for initiating the story and allowing mathematical and physical problems and situations to emerge. |
| CHAPTER 1 | |
| Chapter 1—part 1 | Rationale of design principles |
| At one point during the dance, Romeo notices Juliet in her orbital, and, even if he occasionally gets close to her, he is unable to stay there permanently: quivering with love, he asks who knows her and what her tastes are in terms of radiations (electrons are well known to be romantics). He discovers that Juliet is obsessed with color harmony and that the color she prefers is purple “486 nm”. To get noticed he wants to perform his famous photon—spectroscopic serenade and jump to emit a purple trail. Discuss with your teammates to help Romeo understand how far he will have to jump and whether or not he would have gotten closer to Juliet in this way. | In this chapter, we delve into the concept of interatomic orbital distances, exploring the inherent limitations imposed by electron position uncertainties. Much like the iconic star-crossed lovers, Romeo and Juliet, who yearn to be close but find themselves unable to maintain a permanent proximity, electrons face similar challenges in their spatial arrangements. To elucidate the intriguing connection between emission and electron transitions to different energy levels, we introduce a romantic–comedic twist, employing Juliet’s passion for color harmony as a plot device. Juliet’s preference for the color purple is strategically chosen to align with her energy level, prompting students to contemplate the intriguing relationship between spectroscopy lines and electron energy transitions. As students ponder the impossibility of Romeo and Juliet’s enduring closeness, they begin to discern potential disparities between their mental models and theoretical concepts. Through calculations of energy transitions and the resulting orbital distances, students gain insight into the quadratic proportionality that underlies these phenomena, prompting a gradual reshaping of their personal notions regarding orbital distances. |
| Chapter 1—part 2 | Rationale of design principles |
| The two are deeply in love and would like to spend the rest of their days together. But Juliet’s family hinders them, crying scandal: a Montague cannot be so tied to the nucleus! What to do? The nurse offers Romeo the chance to take her place, but, for her, this would mean losing her place next to Juliet. Romeo and Juliet, very hesitant, then decide to move towards the orbitals occupied by the Montagues. But how to get up there? While the couple is tormented by this problem, an enlightened friar, Lory, arrives to their rescue with two THz 457s, offering to give them a lift. Despite this help, Romeo and Juliet are unable to reach the Montague orbital, so they loudly invoke another friar, Enzo, asking for new help. Discuss with your teammates to understand how far they will jump thanks to the first photons and which photons Fra Enzo will have to carry for the two lovers to reach the Montague orbital. | In this section, students confront the challenges posed by the spatial separations between orbitals, compelling them to grasp two essential aspects. Firstly, they must discern the specific energy jump that a photon of a given frequency can facilitate, (the choice of Friar Lory in the plot is due to the role of Frair Lorenzo in “Romeo and Juliet”). Secondly, they need to determine the frequency of the photon required to reach the Montague orbital, so as to reflect on the inverse problem. In the framework of the C&Q model, it is strikingly evident that the characters are profoundly influenced by their individual roles and aspirations. Take, for instance, Romeo’s desire to gain Juliet’s attention and their joint pursuit of a life away from their feuding families. This narrative intricately parallels the fundamental interplay of orbitals within the model, establishing a direct and compelling link between the characters’ human drama and the pivotal role of orbitals in the model. Following the previous chapter, a deliberate choice was made to foster a collaborative learning environment among the students. The primary aim of this approach is to collectively cultivate a shared knowledge base within the entire class, enabling them to collaboratively synthesize the concepts explored. In this pedagogical strategy, students direct their attention towards specific facets of their engagement and exploratory experiences, thereby demonstrating their grasp of the concepts and their proficiency in employing related process skills. In this context, educators introduce a given concept, process, or skill, and learners are encouraged to articulate their understanding of these elements. Teachers play a pivotal role in guiding students towards achieving a deeper comprehension of the topic. Furthermore, students are tasked with constructing arguments that reflect their unique ideas, integrating various forms of representation such as verbal, graphic, and analytical. Simultaneously, they assess whether the atomic model they hold aligns with the theoretical framework under examination or if it necessitates adjustment. |
| CHAPTER 2 | |
| Chapter 2 and Epilogue | Rationale of design principles |
| Juliet’s escape has thrown the entire atomic balance into crisis, forcing some Montagues to change levels in order to maintain overall stability. Then, when the couple comes to the Montagues, they cry out for revenge, and the couple is then forced to flee again. The Montagues set out in search of Romeo and Juliet but fail because it is not possible to reconstruct the trajectory followed by the two lovers. The story unfortunately ends in tragedy: the two do manage to free themselves from the influence of their families, but they still understand that they cannot be together. Now condemned to separation, the two lovers decide to draw up a schema of the place (the atom) where they met to remember it forever. Discuss with your teammates why this trajectory cannot be reconstructed. End the story with a tragic ending, explaining the reasons for the separation sentence. EPILOGUE Construct with your teammates a possible model of the scheme realized by Romeo and Juliet. | In this second chapter, students begin to grapple with the consequences of the two electrons escaping and the inherent uncertainties that the uncertainty principle introduces. The tragic conclusion of the classic novel finds a reflection in the fate of these two electrons; once they are no longer bound to the atom, their paths are inevitably set on a collision course due to their opposite charges, leading them to be absorbed in a vast sea of electrons. At the outset, the consequences of Romeo and Juliet’s choices become apparent: the voids within the nucleus are replenished with new electrons, ultimately disturbing the equilibrium of the two feuding families. This disruption leads them to share orbits, not fueled by anger but by fate. The Montagues seek revenge, yet they grapple with the inability to reconstruct the electrons’ orbitals due to the uncertainty principle. As part of their learning journey, students are encouraged to collaboratively sketch their envisioned final atomic model following the narrative’s progression and to contemplate how they might populate it with electron positions. This final chapter is meticulously structured to allow students to solidify their acquired knowledge, particularly regarding the uncertainty principle and its relationship with trajectories. It seamlessly weaves this scientific understanding with the timeless drama of the story. In particular, a poignant concept is introduced towards the end, one that resonates deeply with the tragic essence of the narrative: the impossibility of two electrons remaining together outside the nucleus, mirroring Romeo and Juliet’s inability to coexist within their own narrative and drama. Instead, they find fulfillment in their tragic demise, existing beyond the scope of the other characters who continue to live. In this sense, the proposed narrative gains a profound, implicit connection with the dramatic elements, as the drama itself prompts us to question the fate of an electron outside the nucleus, without its nucleus as a reference point. Ultimately, even the identities of Romeo and Juliet lose their significance beyond their respective contexts, much like the indistinguishability of electrons except for the energy levels they occupy. While this concept may seem tangential to the primary objective of the story, it remains coherent with the underlying physics, providing a solid foundation for future developments in the atomic theory of nuclear physics, atoms, and electricity. |
3.2. The Teaching—Learning Sequence (TLS)
3.3. Participants and Setting
3.4. Data Collection and Analysis Criteria
- Recordings of student discussions, both within small groups and plenary sessions during the entire cycle;
- Drawings of the atomic models created by students in the “Prologue” phase and in the “Epilogue” phase;
- Written reports produced by students regarding the calculation of energy levels and radii in “Chapter 1”, and the conclusion of the story in “Chapter 2”;
- A diary maintained by the supervising teacher during the entire cycle;
- Interviews conducted with two chemistry teachers who participated in the final discussion during the “Epilogue” phase;
- A satisfaction questionnaire given to the students upon completing the activity.
- -
- answers to a satisfaction questionnaire administered to students at the end of the activity, to gauge how the students themselves experienced the entire activity;
- -
- the observations conducted by the teachers participating in the activity (the instructor who conducted the activity and two chemistry teachers who took part in the final discussion), to understand if the teacher’s perception was corroborated or not.
4. Preliminary Findings
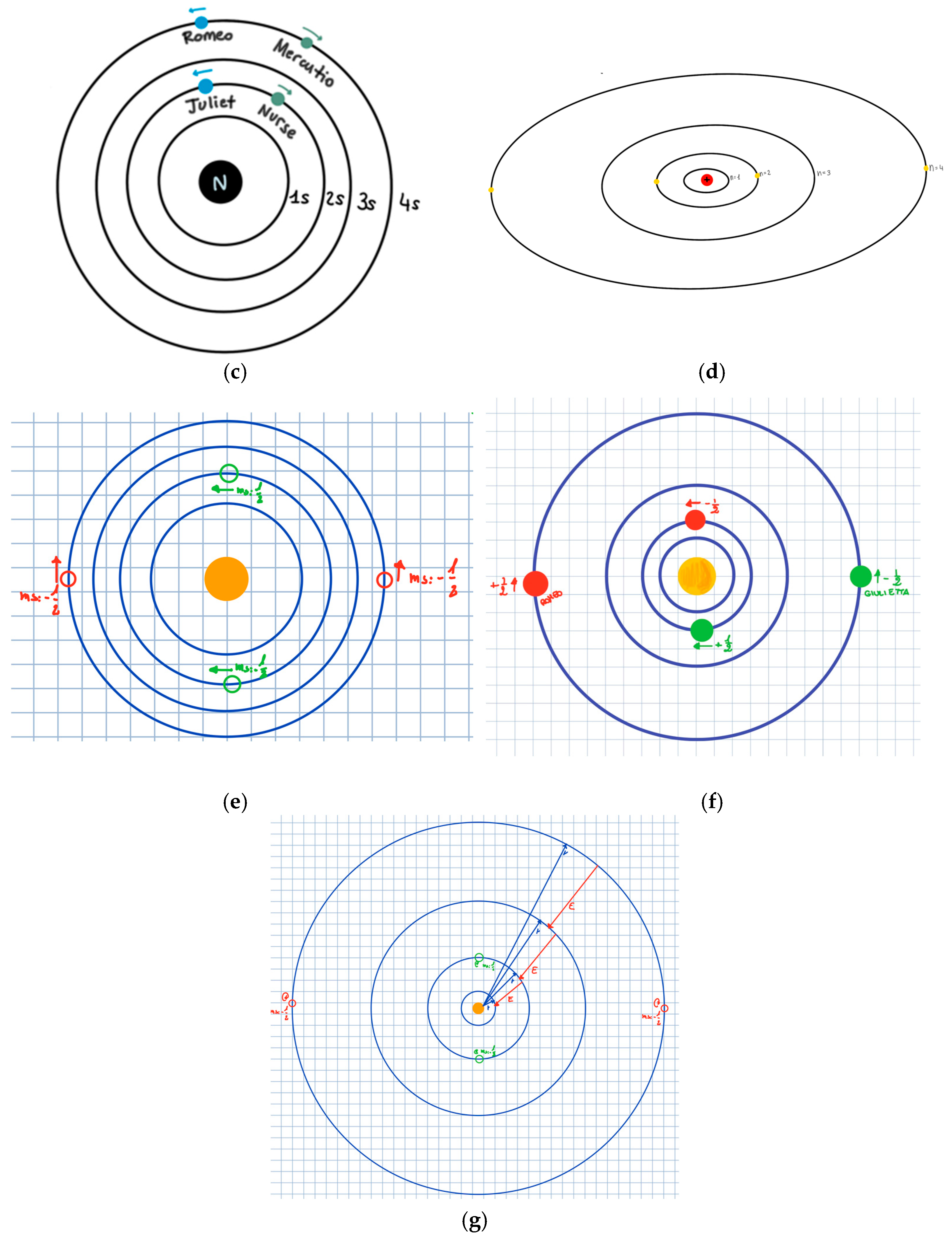
4.1. Students’ Models
- R1: orbits/orbitals represented at varying distances to convey the concept of energy levels more effectively;
- R2: orbits/orbitals represented at correct distances according to the radius;
- R3: attempt to depict the concept of orbitals and the correct distances between them.
4.1.1. Atom Representation (R1)
4.1.2. Atom Representation (R2)
4.1.3. Atom Representation (R3)
4.2. Students’ Engagement
- “The story”;
- A student with a specific learning disorder (SLD), mentioned, “The connection of a fairly complicated topic with such a simple story”;
- “Doing a lesson different from the usual, not sitting at desks in the classroom but collaborating in small groups”;
- “The organization of the work and the narrative we worked on”;
- “The originality of the story and the opportunity to engage in a suitable environment”;
- “The story and the location; the classroom we were in is very advanced and allowed us to interact effectively”.
- “The groups”;
- “The difficulty and challenges of working in groups”;
- “The inability to choose the groups”.
5. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Krijtenburg-Lewerissa, K.; Pol, H.J.; Brinkman, A.; Van Joolingen, W.R. Insights into teaching quantum mechanics in secondary and lower undergraduate education. Phys. Rev. Phys. Educ. 2017, 13, 010109. [Google Scholar] [CrossRef]
- Niss, M.; Blum, W. The learning and teaching of mathematical modelling. In The Learning and Teaching of Mathematical Modelling, 2nd ed.; Routledge: New York, NY, USA, 2020. [Google Scholar]
- Pellowsky, A. The World of Storytelling; R.R. Bowker Company: New York, NY, USA, 1977. [Google Scholar]
- Albano, G.; Coppola, C.; Dello Iacono, U. What Does ‘Inside Out’ Mean in Problem Solving? Learn. Math. 2021, 41, 32–36. [Google Scholar]
- Polo, M.; Dello Iacono, U.; Fiorentino, G.; Pierri, A. A social network analysis approach to digital interactive storytelling in mathematics. Int. J. Sci. Educ. 2019, 15, 239–250. [Google Scholar]
- Fuchs, H.U.; Contini, A.; Dumont, E.; Landini, A.; Corni, F. How metaphor and narrative interact in stories of forces of nature. In Narrative and Metaphor in Education. Look Both Ways; Hanne, M., Kaal, A., Eds.; Routledge: London, UK, 2018. [Google Scholar]
- Hadzigeorgiou, Y. Humanizing the teaching of physics through storytelling: The case of current electricity. Phys. Ed. 2006, 41, 42. [Google Scholar] [CrossRef]
- Collins, S.N. The importance of storytelling in chemical education. Nat. Chem. 2021, 13, 1–2. [Google Scholar] [CrossRef]
- Zabel, J.; Gropengiesser, H. Darwin’s Mental Landscape: Mapping Students’ Learning Progress in Evolution Theory. J. Biol. Educ. 2011, 45, 143–149. [Google Scholar] [CrossRef]
- Collins, S.; Steele, T.; Nelson, M. Storytelling as Pedagogy: The Power of Chemistry Stories as a Tool for Classroom Engagement. J. Chem. Educ. 2023, 100, 2664–2672. [Google Scholar] [CrossRef]
- Csikar, E.; Stefaniak, J.E. The utility of storytelling strategies in the biology classroom. Contemp. Educ. Technol. 2018, 9, 42–60. [Google Scholar]
- Ibarra-Herrera, C.C.; Carrizosa, A.; Yunes-Rojas, J.A.; Mata-Gómez, M.A. Design of an app based on gamification and storytelling as a tool for biology courses. Int. J. Interact. Des. Manuf. 2019, 13, 1271–1282. [Google Scholar] [CrossRef]
- Kapsala, N.; Mavrikaki, E. Storytelling as a pedagogical tool in nature of science instruction. In Nature of Science in Science Instruction: Rationales and Strategies; Springer Nature: Cham, Switzerland, 2020; pp. 485–512. [Google Scholar]
- Matamit, H.N.H.; Roslan, R.; Shahrill, M.; Said, H.M. Teaching Challenges on the Use of Storytelling in Elementary Science Lessons. IJER 2020, 9, 716–722. [Google Scholar] [CrossRef]
- Haven, K. Super Simple Storytelling: A Can-Do Guide for Every Classroom, Every Day; Teacher Ideas Press: Englewood, CO, USA, 2000. [Google Scholar]
- Ivanjek, L.; Shaffer, P.S.; McDermott, L.C.; Planinic, M.; Veza, D. Research as a guide for curriculum development: An example from introductory spectroscopy. I. Identifying student difficulties with atomic emission spectra. Am. J. Phys. 2015, 83, 85–90. [Google Scholar] [CrossRef]
- Taber, K.S. Learning quanta: Barriers to stimulating transitions in student understanding of orbital ideas. Sci. Educ. 2005, 89, 94–116. [Google Scholar] [CrossRef]
- Fuchs, H.U. From Stories to Scientific Models and Back: Narrative framing in modern macroscopic physics. Int. J. Sci. Educ. 2015, 37, 934–957. [Google Scholar] [CrossRef]
- Bruner, J. Actual Minds, Possible Worlds; Harvard University Press: Cambridge, MA, USA, 1986. [Google Scholar]
- Bruner, J. The narrative construction of reality. Crit. Inq. 1991, 18, 1–21. [Google Scholar] [CrossRef]
- Herman, D. Storytelling and the Sciences of Mind; MIT Press: Cambridge, MA, USA, 2013. [Google Scholar]
- Zan, R. The crucial role of narrative thought in understanding story problems. In Current State of Research on Mathematical Beliefs XVI; Institute of Mathematics and Natural Sciences, Tallinn University: Tallin, Estonia, 2011; pp. 287–305. [Google Scholar]
- Zan, R. La dimensione narrativa di un problema: Il modello C&D per l’analisi e la (ri) formulazione del testo. L’insegnamento della Matematica e delle Scienze Integrate 2012, 35, 107–126. [Google Scholar]
- Niss, M.; Højgaard, T. Mathematical competencies revisited. Educ. Stud. Math. 2019, 102, 9–28. [Google Scholar] [CrossRef]
- Niss, M.; Jensen, T.H. Kompetencer og Matematiklæring—Ideer og Inspiration til Udvikling af Matematikundervisning i Danmark; The Ministry of Education: Copenhagen, Denmark, 2002. (In Danish) [Google Scholar]
- Dewey, J. Science as subject-matter and as method. Science 1910, 31, 121–127. [Google Scholar] [CrossRef] [PubMed]
- Dewey, J. Unity of Science as a Social Problem; University of Chicago Press: Chicago, IL, USA, 1938. [Google Scholar]
- Bybee, R.W.; Taylor, J.A.; Gardner, A.; Van Scotter, P.; Powell, J.C.; Westbrook, A.; Landes, N. The BSCS 5E Instructional Model: Origins and Effectiveness. BSCS 2006, 5, 88–98. [Google Scholar]
- Areepattamannil, S.; Cairns, D.; Dickson, M. Teacher-Directed Versus Inquiry-Based Science Instruction: Investigating Links to Adolescent Students’ Science Dispositions Across 66 Countries. J. Sci. Teach. Educ. 2020, 31, 675–704. [Google Scholar] [CrossRef]
- Jerrim, J.; Oliver, M.; Sims, S. The relationship between inquiry-based teaching and students’ achievement. New evidence from a longitudinal PISA study in England. Learn. Instr. 2022, 80, 101310. [Google Scholar] [CrossRef]
- Albano, G.; Dello Iacono, U. Designing Digital Storytelling for Mathematics Special Education: An Experience in Support Teacher Education. Math. Enthus. 2019, 16, 263–288. [Google Scholar] [CrossRef]
- Albano, G.; Dello Iacono, U.; Fiorentino, G. A Technological Storytelling Approach to Nurture Mathematical Argumentation. In Proceedings of the 12th International Conference on Computer Supported Education (CSEDU), Prague, Czech Republic, 2–4 May 2020; Chad Lane, H., Zvacek, S., Uhomoibhi, J., Eds.; SCITEPRESS: Setúbal, Portugal, 2020; Volume 1, pp. 420–427. [Google Scholar]

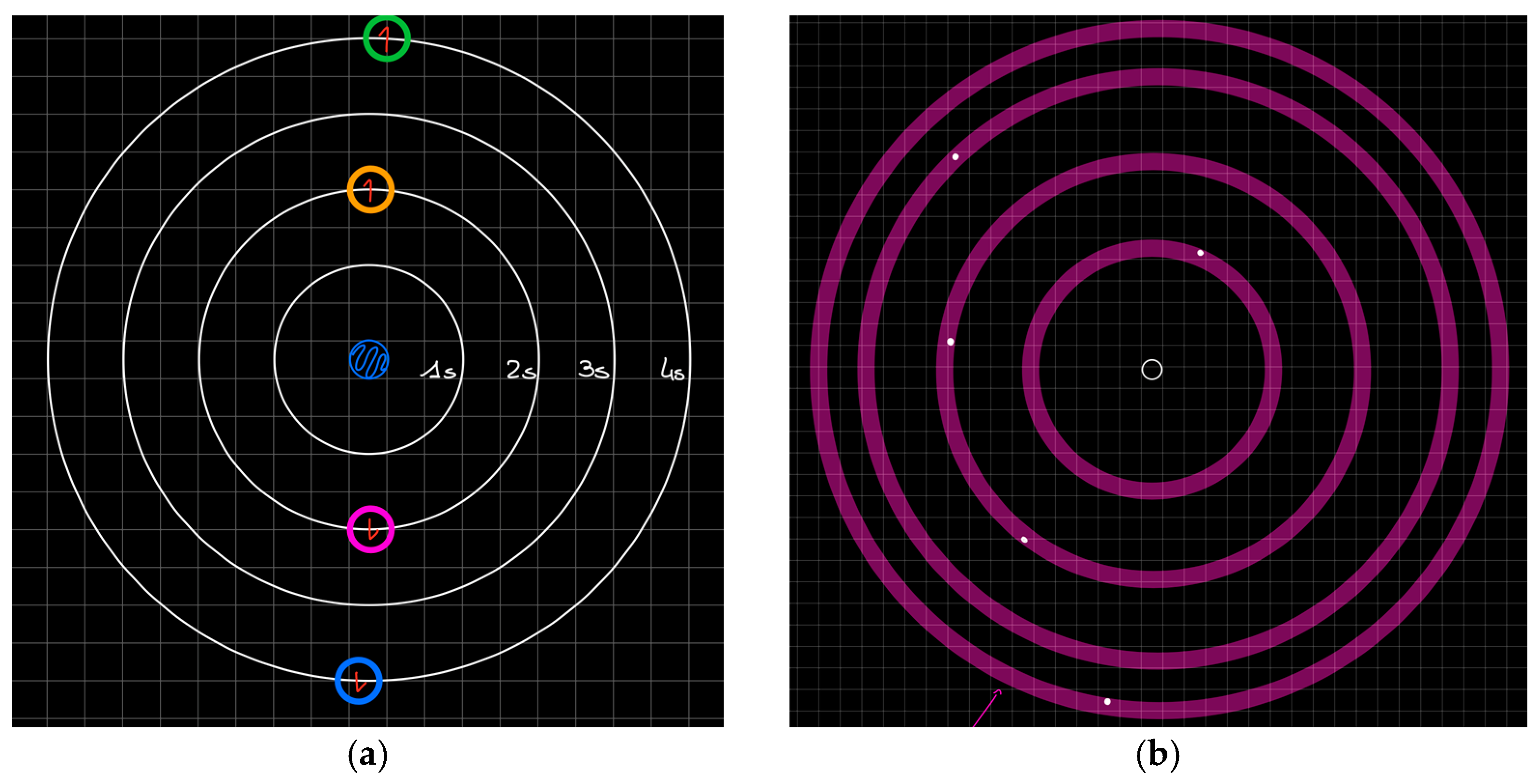

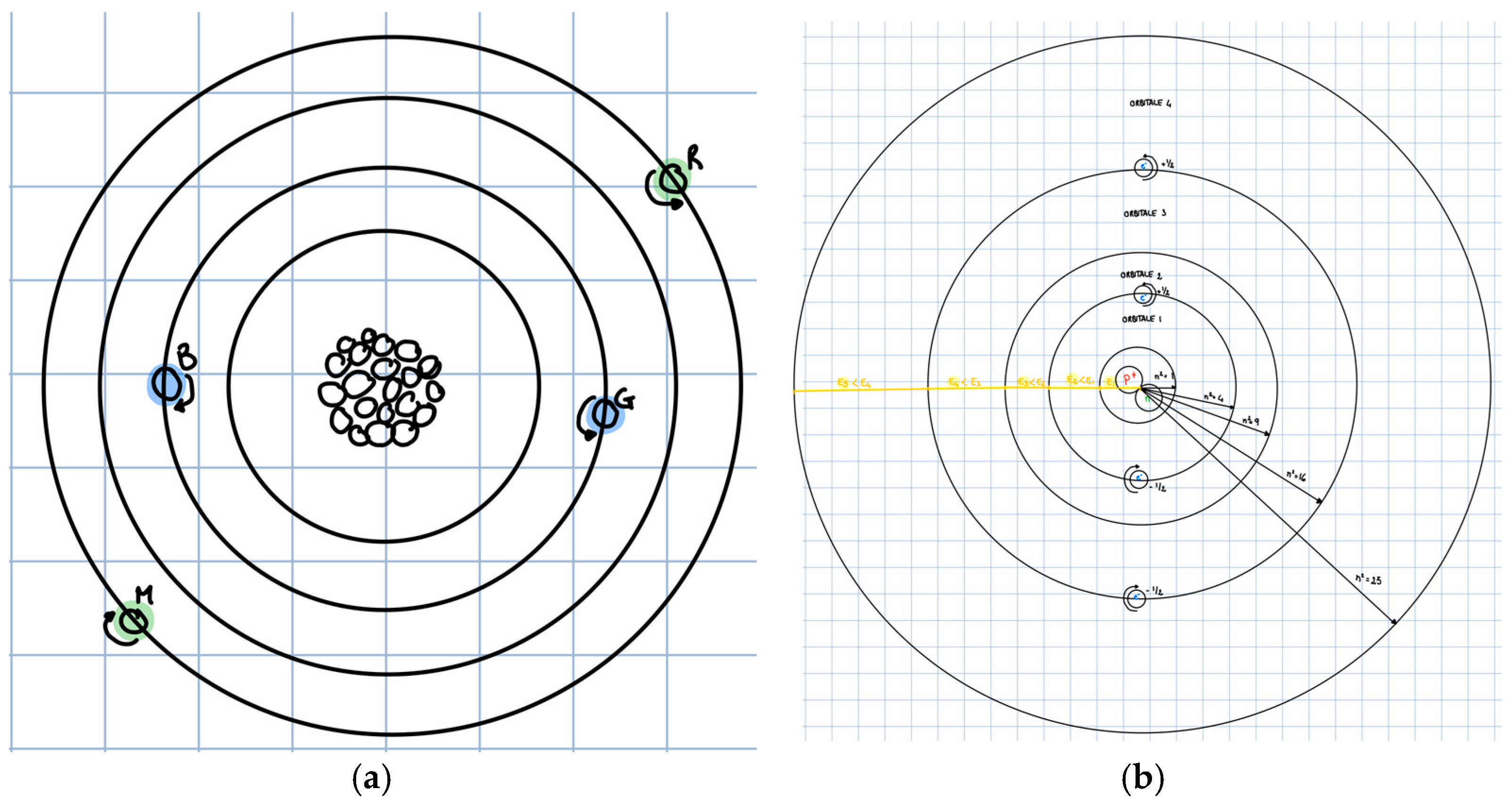

| On planetary and Bohr’s models [1] |
|
| On energetic levels [16] |
|
| On orbitals [17] |
|
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aquilina, G.; Dello Iacono, U.; Gabelli, L.; Picariello, L.; Scettri, G.; Termini, G. “Romeo and Juliet: A Love out of the Shell”: Using Storytelling to Address Students’ Misconceptions and Promote Modeling Competencies in Science. Educ. Sci. 2024, 14, 239. https://doi.org/10.3390/educsci14030239
Aquilina G, Dello Iacono U, Gabelli L, Picariello L, Scettri G, Termini G. “Romeo and Juliet: A Love out of the Shell”: Using Storytelling to Address Students’ Misconceptions and Promote Modeling Competencies in Science. Education Sciences. 2024; 14(3):239. https://doi.org/10.3390/educsci14030239
Chicago/Turabian StyleAquilina, Ginevra, Umberto Dello Iacono, Lucia Gabelli, Luca Picariello, Giacomo Scettri, and Giulia Termini. 2024. "“Romeo and Juliet: A Love out of the Shell”: Using Storytelling to Address Students’ Misconceptions and Promote Modeling Competencies in Science" Education Sciences 14, no. 3: 239. https://doi.org/10.3390/educsci14030239
APA StyleAquilina, G., Dello Iacono, U., Gabelli, L., Picariello, L., Scettri, G., & Termini, G. (2024). “Romeo and Juliet: A Love out of the Shell”: Using Storytelling to Address Students’ Misconceptions and Promote Modeling Competencies in Science. Education Sciences, 14(3), 239. https://doi.org/10.3390/educsci14030239







