Measuring and Calculating Current Atmospheric Phosphorous and Nitrogen Loadings to Utah Lake Using Field Samples and Geostatistical Analysis
Abstract
:1. Introduction
1.1. Study Goals and Importance
1.2. Utah Lake Water Quality Background
1.3. Deposition Clssification and Collection
1.4. Utah Lake Description
2. Materials and Methods
2.1. Sampling Methods and Locations
2.2. Sample Collection and Chemial Analysis Methods
2.3. Sample Analysis
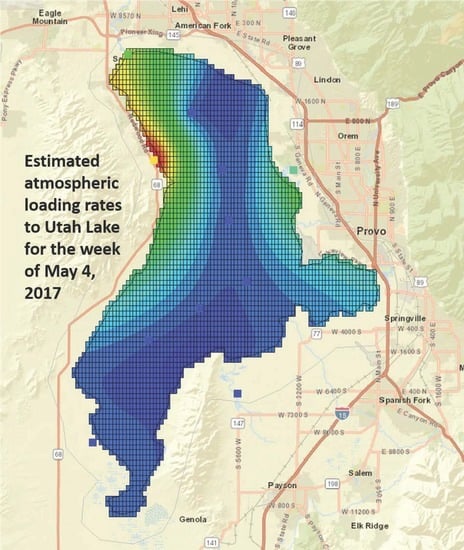
2.4. Atmospheric Load Calcualtions and Geostatisticl Methods
3. Results
3.1. Sampling Results
3.2. Phosphorous Load Calculation
3.3. Nitrogen Load Calculation
3.4. Site Comparison
3.5. Total Deposition
4. Discussion
4.1. Wet and Dry Deposition Rates
4.2. Local Sources versus Global Sources
4.3. Total Deposition
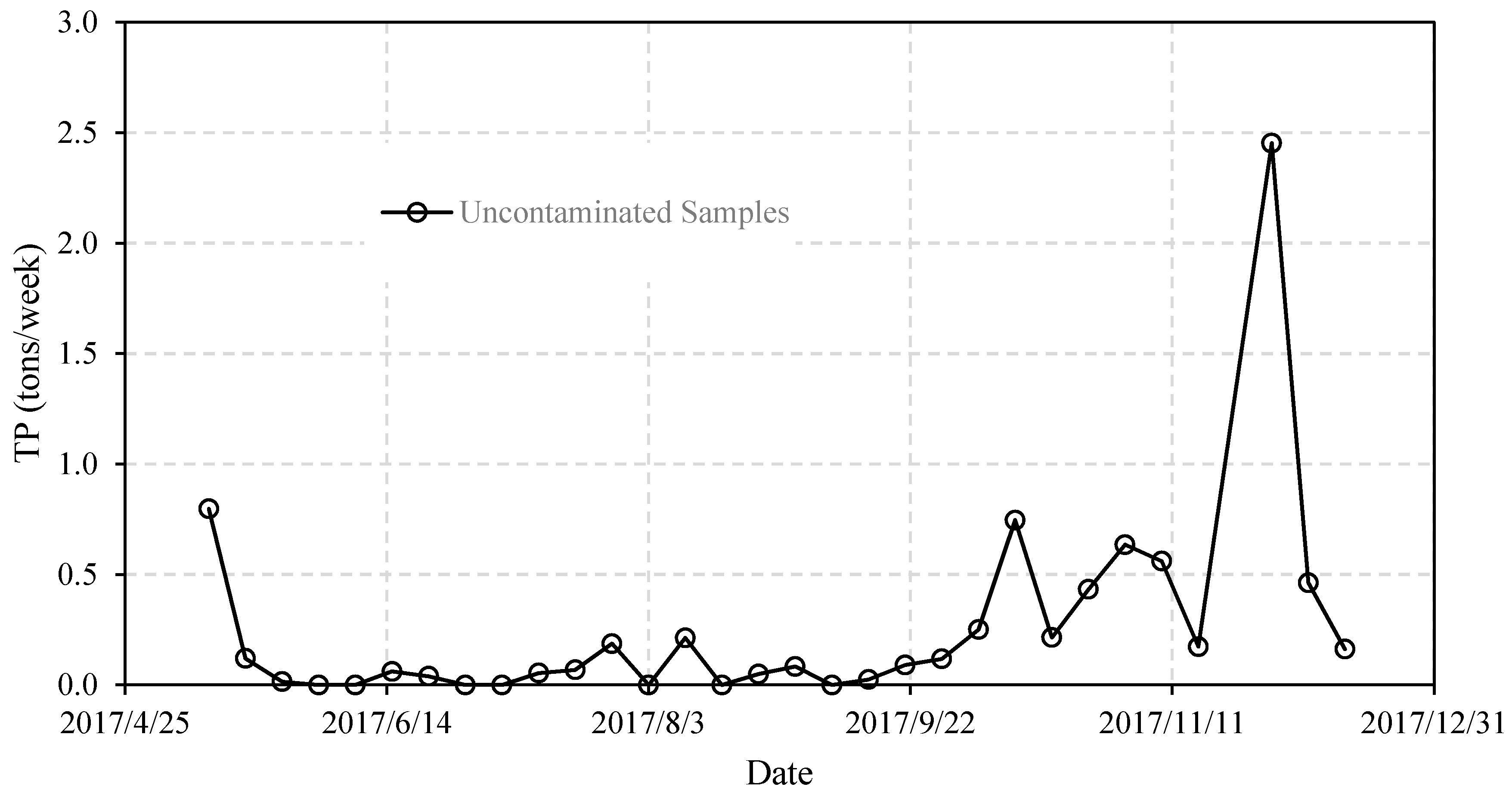
4.4. Contamination
4.5. Upper and Lower Bound Estimates
4.6. Future Work
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Anderson, D.M.; Glibert, P.M.; Burkholder, J.M. Harmful algal blooms and eutrophication: Nutrient sources, composition, and consequences. Estuaries 2002, 25, 704–726. [Google Scholar] [CrossRef]
- Heisler, J.; Glibert, P.M.; Burkholder, J.M.; Anderson, D.M.; Cochlan, W.; Dennison, W.C.; Dortch, Q.; Gobler, C.J.; Heil, C.A.; Humphries, E. Eutrophication and harmful algal blooms: A scientific consensus. Harmful Algae 2008, 8, 3–13. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cole, J.J.; Caraco, N.F.; Likens, G.E. Short-range atmospheric transport: A significant source of phosphorus to an oligotrophic lake. Limnol. Oceanogr. 1990, 35, 1230–1237. [Google Scholar] [CrossRef]
- Jassby, A.D.; Reuter, J.E.; Axler, R.P.; Goldman, C.R.; Hackley, S.H. Atmospheric deposition of nitrogen and phosphorus in the annual nutrient load of lake tahoe (california-nevada). Water Resour. Res. 1994, 30, 2207–2216. [Google Scholar] [CrossRef]
- Lewis, W.M. Precipitation chemistry and nutrient loading by precipitation in a tropical watershed. Water Resour. Res. 1981, 17, 169–181. [Google Scholar] [CrossRef]
- Schindler, D.; Newbury, R.; Beaty, K.; Campbell, P. Natural water and chemical budgets for a small precambrian lake basin in central canada. J. Fish. Board Can. 1976, 33, 2526–2543. [Google Scholar] [CrossRef]
- PSOMAS. Utah Lake Tmdl: Pollutant Loading Assessment & Designated Beneficial Use Impairment Assessment; PSOMAS: Salt Lake City, UT, USA, 2007. [Google Scholar]
- DWQ. Utah Lake Water Quality Work Plan 2015–2019; Utah State Division of Water Quality: Salt Lake City, UT, USA, 2016. [Google Scholar]
- Merritt, L.B.; Miller, A.W. Interim Report on Nutrient Loadings to Utah Lake; Report to the Utah Department of Water Quality: Salt Lake City, UT, USA, 2016. [Google Scholar]
- Sorokin, C.; Krauss, R.W. The effects of light intensity on the growth rates of green algae. Plant Physiol. 1958, 33, 109. [Google Scholar] [CrossRef] [PubMed]
- Brown, T.E.; Richardson, F.L. The effect of growth environment on the physiology of algae: Light intensity. J. Phycol. 1968, 4, 38–54. [Google Scholar] [CrossRef] [PubMed]
- Lee, G.F.; Rast, W.; Jones, R.A. Water report: Eutrophication of water bodies: Insights for an age old problem. Environ. Sci. Technol. 1978, 12, 900–908. [Google Scholar] [CrossRef]
- Hansen, C.; Swain, N.; Munson, K.; Adjei, Z.; Williams, G.P.; Miller, W. Development of sub-seasonal remote sensing chlorophyll-a detection models. Am. J. Plant Sci. 2013, 4, 21. [Google Scholar] [CrossRef]
- Hansen, C.H.; Dennison, P.; Burian, S.; Barber, M.; Williams, G. Hindcasting water quality in an optically complex system. WIT Trans. Ecol. Environ. 2016, 209, 35–44. [Google Scholar]
- Abu-Hmeidan, H.Y.; Williams, G.P.; Miller, A.W. Characterizing total phosphorus in current and geologic utah lake sediments: Implications for water quality management issues. Hydrology 2018, 5, 8. [Google Scholar] [CrossRef]
- Hansen, C.H.; Burian, S.J.; Dennison, P.E.; Williams, G.P. Spatiotemporal variability of lake water quality in the context of remote sensing models. Remote Sens. 2017, 9, 409. [Google Scholar] [CrossRef]
- Hansen, C.H.; Williams, G.P.; Adjei, Z.; Barlow, A.; Nelson, E.J.; Miller, A.W. Reservoir water quality monitoring using remote sensing with seasonal models: Case study of five central-utah reservoirs. Lake Reserv. Manag. 2015, 31, 225–240. [Google Scholar] [CrossRef]
- Carlson, R.E. A trophic state index for lakes. Limnol. Oceanogr. 1977, 22, 361–369. [Google Scholar] [CrossRef] [Green Version]
- Larsen, D.; Mercier, H. Lake phosphorus loading graphs: An alternative. In National Technical Information Service; NTIS: Springfield, VA, USA, 1975; Volume PB-243 869, p. 4. [Google Scholar]
- Nanus, L.; Campbell, D.H.; Ingersoll, G.P.; Clow, D.W.; Alisa Mast, M. Atmospheric deposition maps for the rocky mountains. Atmos. Environ. 2003, 37, 4881–4892. [Google Scholar] [CrossRef]
- Uttormark, P.D.; Chapin, J.D.; Green, K.M. Estimating Nutrient Loadings of Lakes from Non-Point Sources; Office of Research and Development, U.S. Environmental Protection Agency: Washington, DC, USA, 1974.
- Welch, H.E.; Legault, J.A. Precipitation chemistry and chemical limnology of fertilized and natural lakes at saqvaqjuac, nwt. Can. J. Fish. Aquat. Sci. 1986, 43, 1104–1134. [Google Scholar] [CrossRef]
- Ahn, H.; James, R.T. Variability, uncertainty, and sensitivity of phosphorus deposition load estimates in south florida. Water Air Soil Pollut. 2001, 126, 37–51. [Google Scholar] [CrossRef]
- NADP. Nadp Site Selection and Installation; National Atmospheric Deposition Program: Madison, WI, USA, 2014. [Google Scholar]
- Utah Agrc: Automated Geographic Reference Center. Available online: https://gis.utah.gov/data/ (accessed on 10 July 2017).
- NOAA. Climatological Data Publications. Available online: https://www.ncdc.noaa.gov/IPS/cd/cd.html (accessed on 5 March 2018).
- Anderson, K.A.; Downing, J.A. Dry and wet atmospheric deposition of nitrogen, phosphorus and silicon in an agricultural region. Water Air Soil Pollut. 2006, 176, 351–374. [Google Scholar] [CrossRef]
- Mahowald, N.; Jickells, T.D.; Baker, A.R.; Artaxo, P.; Benitez-Nelson, C.R.; Bergametti, G.; Bond, T.C.; Chen, Y.; Cohen, D.D.; Herut, B. Global distribution of atmospheric phosphorus sources, concentrations and deposition rates, and anthropogenic impacts. Glob. Biogeochem. Cycles 2008, 22. [Google Scholar] [CrossRef] [Green Version]
- Gomolka, R.E. An Investigation of Atmospheric Phosphorus as a Source of Lake Nutrient; University of Toronto: Toronto, ON, Canada, 1975. [Google Scholar]
- AQUAVEO. Ground Water Modeling System 10.2.4. Available online: https://www.aquaveo.com/software/gms-groundwater-modeling-system-introduction (accessed on 5 March 2018).
- Hendry, C.; Brezonik, P.; Edgerton, E. Atmospheric Deposition of Nitrogen and Phosphorus in Florida [Contaminants, Nutrients in Rainfall]; Food and Agriculture Organization: Rome, Italy, 1981. [Google Scholar]
- Linsey, G.; Schindler, D.; Stainton, M. Atmospheric deposition of nutrients and major ions at the experimental lakes area in northwestern ontario, 1970 to 1982. Can. J. Fish. Aquat. Sci. 1987, 44, s206–s214. [Google Scholar] [CrossRef]
- Shaw, R.; Trimbee, A.; Minty, A.; Fricker, H.; Prepas, E. Atmospheric deposition of phosphorus and nitrogen in central alberta with emphasis on narrow lake. Water Air Soil Pollut. 1989, 43, 119–134. [Google Scholar] [CrossRef]
- Delumyea, R.; Petel, R. Wet and dry deposition of phosphorus into lake huron. Water Air Soil Pollut. 1978, 10, 187–198. [Google Scholar] [CrossRef]
- Newman, E. Phosphorus inputs to terrestrial ecosystems. J. Ecol. 1995, 713–726. [Google Scholar] [CrossRef]
- Carling, G.T.; Randall, M.; Nelson, S.; Rey, K.; Hansen, N.; Bickmore, B.; Miller, T. Characterizing the Fate and Mobility of Phosphorus in Utah Lake Sediments. In Proceedings of the AGU Fall Meeting Abstracts, New Orleans, LA, USA, 11–15 December 2017. [Google Scholar]
- Merrell, P.D. Utah Lake Sediment Phosphorus Analysis; Brigham Young University: Provo, UT, USA, 2015. [Google Scholar]











| Issue | Lake Shore | Mosida | Saratoga Springs | Pump Station | Orem WWTP |
|---|---|---|---|---|---|
| Latitude | −111.787781 | −111.927626 | −111.868827 | −111.895347 | −111.735528 |
| Longitude | 40.11229 | 40.076452 | 40.283815 | 40.359414 | 40.276158 |
| Irrigation sources | Compliant | Central Pivot irrigation 380 m from collector | Compliant | Central Pivot irrigation 500 m from collector | Wheel line irrigation 500 m from site |
| ≥5 m from Equipment | Solar Panel | Solar Panel | Solar Panel | Solar Panel | Solar Panel |
| ≥5 m from Collector | 3 m from collector | Compliant | Compliant | Compliant | Compliant |
| ≥10 m from Collector | Access road is 7 m from collector | Compliant | Compliant | Compliant | Compliant |
| ≥20 m from Collector | Horse corral 10 m from collector | Compliant | Compliant | Compliant | Compliant |
| ≥30 m from Collector | Farm Shed 15 m from collector | Compliant | Small gravel driveway 25 m from collector | Compliant | Compliant |
| ≥100 m from Collector | Compliant | Compliant | Compliant | Compliant | Parking lot 60 m from collector |
| ≥500 m from Collector | Compliant | Compliant | Compliant | Compliant | Compliant |
| ≥1 km from Collector | Compliant | Compliant | Compliant | Compliant | Compliant |
| NAPD Site Classification | R | I | S | S | U |
| Number of Samples | |||
|---|---|---|---|
| Site | Dry | Wet | Total |
| Lake Shore | 29 | 12 | 41 |
| Mosida | 28 | 10 | 38 |
| Saratoga Springs | 30 | 14 | 44 |
| Pump Station | 28 | 10 | 38 |
| Orem WWTP | 22 | 5 | 27 |
| Site | No. of Data | Mean TP Concentrations (mg/L) | Rain | Total TP Load Rate (mg m−2 day−1) | |||
|---|---|---|---|---|---|---|---|
| Wet | Dry | cm(in)/Week | Mean | S.D. | Skewness | ||
| Lake Shore | 41 | 0.68 | 0.38 | 0.64(0.25) | 1.33 | 1.95 | 0.82 |
| Mosida | 38 | 0.22 | 1.10 | 0.30(0.12) | 2.77 | 5.63 | 2.55 |
| Saratoga Springs | 44 | 0.60 | 5.15 | 0.43(0.17) | 31.38 | 88.73 | 2.14 |
| Pump Station | 38 | 0.59 | 0.85 | 0.41(0.16) | 3.78 | 20.14 | 4.68 |
| Orem WWTP 1 | 27 | 1.62 | 0.39 | 0.28(0.11) | 1.26 | 2.65 | 3.33 |
| Average | 38 | 0.74 | 1.57 | 0.41(0.16) | 8.10 | 23.82 | 2.70 |
| Site | No. of Data | Mean DIN Concentrations (mg/L) | Rain | Total DIN Load Rate (mg m−2 day−1) | |||
|---|---|---|---|---|---|---|---|
| Wet | Dry | cm(in)/Week | Mean | S.D. | Skewness | ||
| Lake Shore | 41 | 4.30 | 1.15 | 0.64(0.25) | 4.09 | 4.06 | 0.47 |
| Mosida | 38 | 2.29 | 1.50 | 0.30(0.12) | 4.17 | 4.74 | 1.21 |
| Saratoga Springs | 44 | 4.86 | 6.00 | 0.43(0.17) | 36.06 | 124.62 | 3.31 |
| Pump Station | 38 | 4.29 | 0.38 | 0.41(0.16) | 1.59 | 2.33 | 2.31 |
| Orem WWTP | 27 | 7.55 | 1.33 | 0.28(0.11) | 5.23 | 4.60 | 3.04 |
| Average | 38 | 4.66 | 2.07 | 0.41(0.16) | 10.23 | 28.07 | 2.07 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Olsen, J.M.; Williams, G.P.; Miller, A.W.; Merritt, L. Measuring and Calculating Current Atmospheric Phosphorous and Nitrogen Loadings to Utah Lake Using Field Samples and Geostatistical Analysis. Hydrology 2018, 5, 45. https://doi.org/10.3390/hydrology5030045
Olsen JM, Williams GP, Miller AW, Merritt L. Measuring and Calculating Current Atmospheric Phosphorous and Nitrogen Loadings to Utah Lake Using Field Samples and Geostatistical Analysis. Hydrology. 2018; 5(3):45. https://doi.org/10.3390/hydrology5030045
Chicago/Turabian StyleOlsen, Jacob M., Gustavious P. Williams, A. Woodruff Miller, and LaVere Merritt. 2018. "Measuring and Calculating Current Atmospheric Phosphorous and Nitrogen Loadings to Utah Lake Using Field Samples and Geostatistical Analysis" Hydrology 5, no. 3: 45. https://doi.org/10.3390/hydrology5030045
APA StyleOlsen, J. M., Williams, G. P., Miller, A. W., & Merritt, L. (2018). Measuring and Calculating Current Atmospheric Phosphorous and Nitrogen Loadings to Utah Lake Using Field Samples and Geostatistical Analysis. Hydrology, 5(3), 45. https://doi.org/10.3390/hydrology5030045