Hydrogenation Thermodynamics of Ti16V60Cr24−xFex Alloys (x = 0, 4, 8, 12, 16, 20, 24)
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Microstructure, Crystal Structure and First Hydrogenation
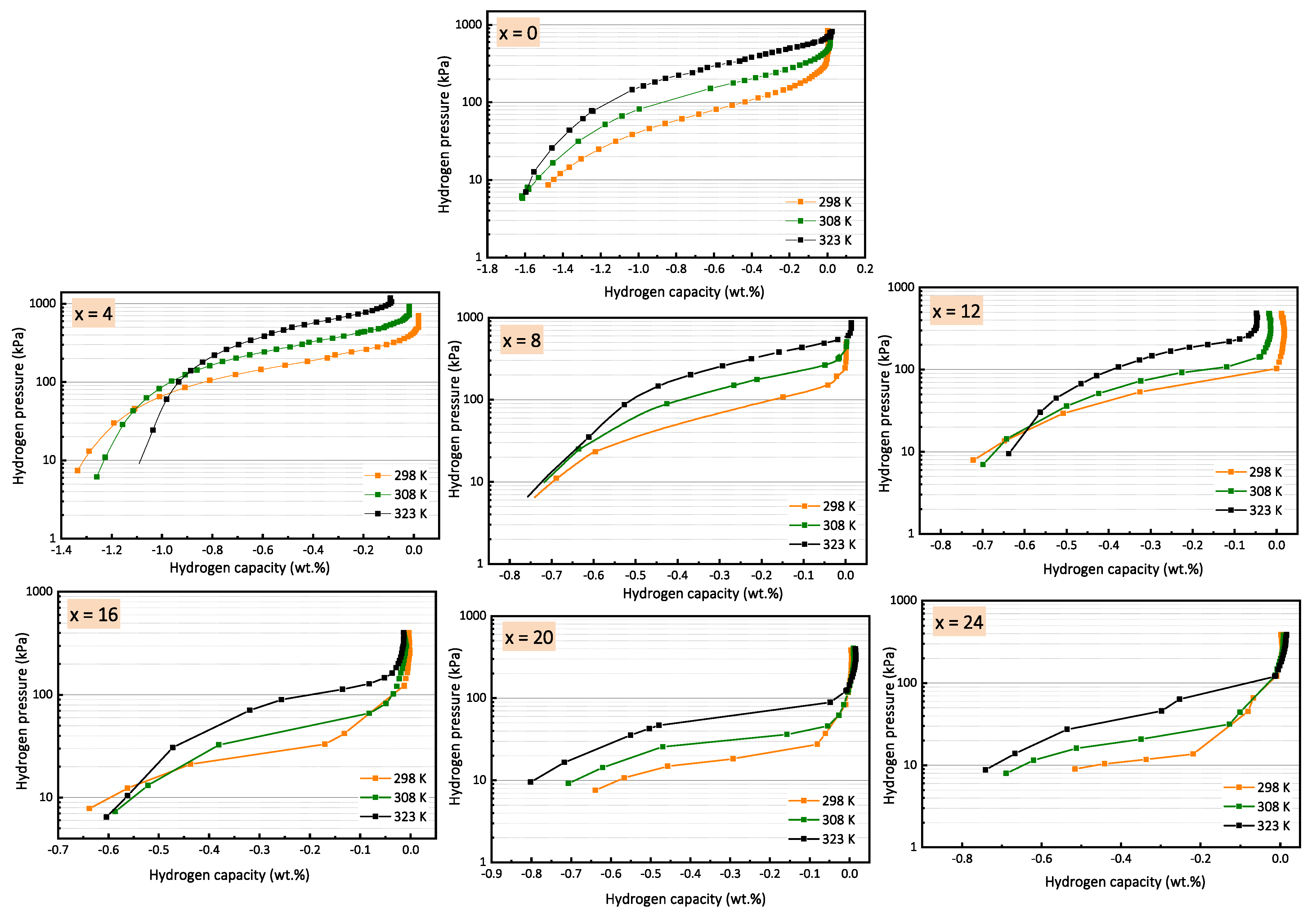
3.2. Pressure Composition Isotherms (PCIs)
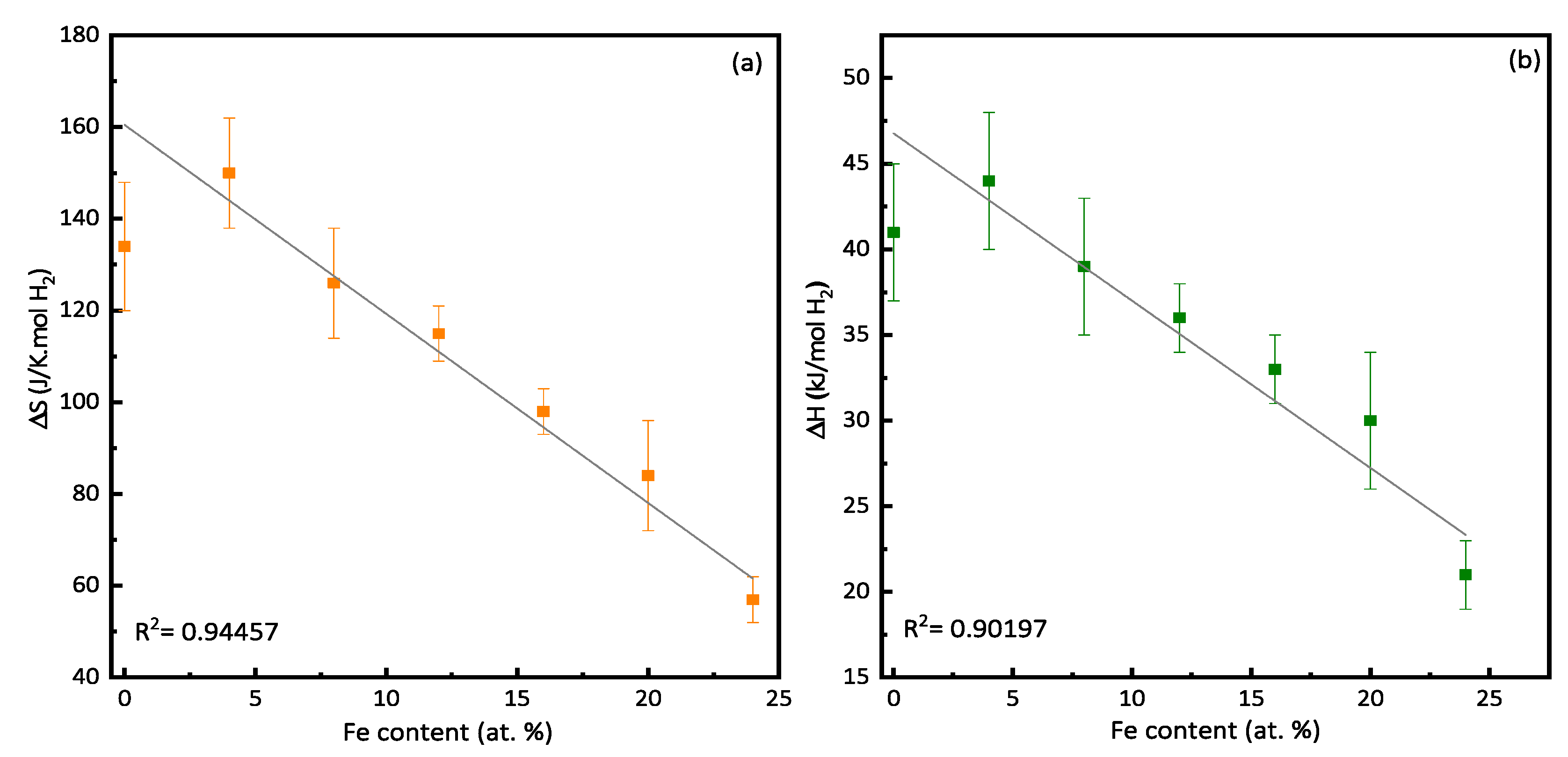
3.3. Enthalpy and Entropy
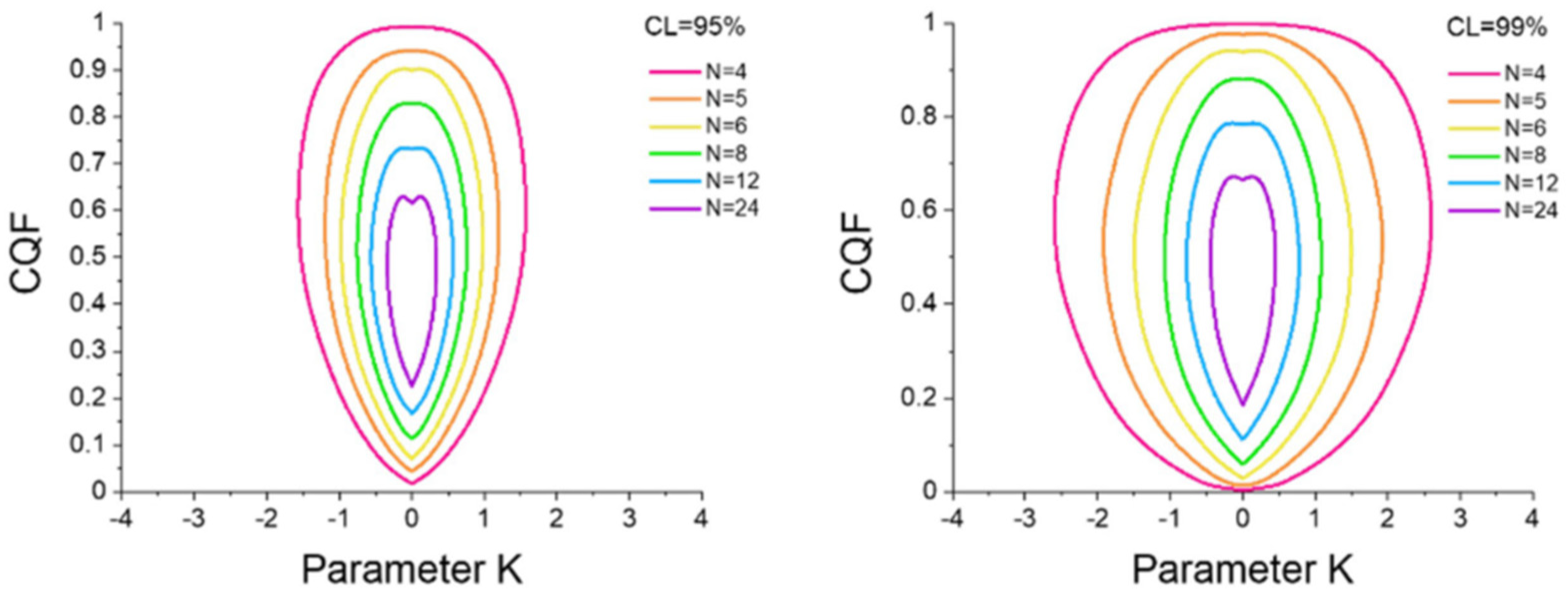
3.4. Enthalpy–Entropy Compensation
- Step 1
- Step 2
- Step 3
- Step 4
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tamura, T.; Kamegawa, A.; Takamura, H.; Okada, M. Hydrogen Isotope Effects on Absorption Properties of Ti-Cr-V Alloys. Mater. Trans. 2003, 44, 641–644. [Google Scholar] [CrossRef]
- Tamura, T.; Tominaga, Y.; Matsumoto, K.; Fuda, T.; Kuriiwa, T.; Kamegawa, A.; Takamura, H.; Okada, M. Protium Absorption Properties of Ti-V-Cr-Mn Alloys with a b.c.c. Structure. J. Alloys Compd. 2002, 330–332, 522–525. [Google Scholar] [CrossRef]
- Fuda, T.; Matsumoto, K.; Tominaga, Y.; Tamura, T.; Kuriiwa, T.; Kamegawa, A.; Okada, M. Effects of Additions of BCC Former Elements on Protium Absorbing Properties of Cr-Ti-V Alloys. Mater. Trans. JIM 2000, 41, 577–580. [Google Scholar] [CrossRef]
- Tsukahara, M.; Takahashi, K.; Mishima, T.; Isomura, A.; Sakai, T. Heat-Treatment Effects of V-Based Solid Solution Alloy with TiNi-Based Network Structure on Hydrogen Storage and Electrode Properties. J. Alloys Compd. 1996, 243, 133–138. [Google Scholar] [CrossRef]
- Itoh, H.; Arashima, H.; Kubo, K.; Kabutomori, T.; Ohnishi, K. Improvement of Cyclic Durability of BCC s Tructured Ti-Cr-V Alloys. J. Alloys Compd. 2005, 404–406, 417–420. [Google Scholar] [CrossRef]
- Lototsky, M.V.; Yartys, V.A.; Zavaliy, I.Y. Vanadium-Based BCC Alloys: Phase-Structural Characteristics and Hydrogen Sorption Properties. J. Alloys Compd. 2005, 404–406, 421–426. [Google Scholar] [CrossRef]
- Reilly, J.J.; Wiswall, R.H. The Higher Hydrides of Vanadium and Niobium. Inorg. Chem. 1970, 9, 1678–1682. [Google Scholar] [CrossRef]
- Seo, C.Y.; Kim, J.H.; Lee, P.S.; Lee, J.Y. Hydrogen Storage Properties of Vanadium-Based b.c.c. Solid Solution Metal Hydrides. J. Alloys Compd. 2003, 348, 252–257. [Google Scholar] [CrossRef]
- Kamble, A. Effect of Additives, Heat Treatment and Mechanical Deformations on Hydrogen Storage Properties of BCC Alloys. Ph.D. Thesis, Université du Québec à Trois, Rivières, QC, Canada, 2018. [Google Scholar]
- Sleiman, S.; Huot, J. Microstructure and First Hydrogenation Properties of TiHfZrNb1-XV1 + x Alloy for x = 0, 0.1, 0.2, 0.4, 0.6 and 1. Molecules 2022, 27, 1054. [Google Scholar] [CrossRef]
- Kamble, A.; Huot, J.; Sharma, P. Effect of Addition of Zr, Ni, and Zr-Ni Alloy on the Hydrogen Absorption of Body Centred Cubic 52Ti-12V-36Cr Alloy. Int. J. Hydrogen Energy 2018, 43, 7424–7429. [Google Scholar] [CrossRef]
- Ravalison, F.; Rabkin, E.; Huot, J. Methods to Improve the First Hydrogenation of the Vanadium-Rich BCC Alloy Ti 16 V 60 Cr 24. Hydrogen 2022, 3, 303–311. [Google Scholar] [CrossRef]
- Dixit, V.; Huot, J. Investigation of the Microstructure, Crystal Structure and Hydrogenation Kinetics of Ti-V-Cr Alloy with Zr Addition. J. Alloys Compd. 2019, 785, 1115–1120. [Google Scholar] [CrossRef]
- Khajavi, S.; Rajabi, M.; Huot, J. Effect of Cold Rolling and Ball Milling on First Hydrogenation of Ti0.5Zr0.5 (Mn1-XFex) Cr1, X = 0, 0.2, 0.4. J. Alloys Compd. 2019, 775, 912–920. [Google Scholar] [CrossRef]
- Sleiman, S.; Aliouat, A.; Huot, J. Materials Enhancement of First Hydrogenation of Ti 1 V 0.9 Cr 1.1 BCC Alloy by Cold Rolling and Ball Milling. Materials 2020, 13, 3106. [Google Scholar] [CrossRef] [PubMed]
- Liu, S.; Cui, C.; Wang, X.; Li, N.; Shi, J.; Cui, S.; Chen, P. Effect of Cooling Rate on Microstructure and Grain Refining Behavior of in Situ CeB6/Al Composite Inoculant in Aluminum. Metals 2017, 7, 204. [Google Scholar] [CrossRef]
- Lang, J.; Huot, J. A New Approach to the Processing of Metal Hydrides. J. Alloys Compd. 2011, 509, L18–L22. [Google Scholar] [CrossRef]
- Kumar, S.; Jain, A.; Ichikawa, T.; Kojima, Y.; Dey, G.K. Development of Vanadium Based Hydrogen Storage Material: A Review. Renew. Sustain. Energy Rev. 2017, 72, 791–800. [Google Scholar] [CrossRef]
- Strozi, R.B.; Silva, B.H.; Leiva, D.R.; Zlotea, C.; Botta, W.J.; Zepon, G. Tuning the Hydrogen Storage Properties of Ti-V-Nb-Cr Alloys by Controlling the Cr/(TiVNb) Ratio. J. Alloys Compd. 2023, 932, 167609. [Google Scholar] [CrossRef]
- Yukawa, H.; Teshima, A.; Yamashita, D.; Ito, S.; Morinaga, M.; Yamaguchi, S. Alloying Effects on the Hydriding Properties of Vanadium at Low Hydrogen Pressures. J. Alloys Compd. 2002, 337, 264–268. [Google Scholar] [CrossRef]
- Verbetsky, V.N.; Zotov, T.A.; Tatarintsev, A.V.; Movlaev, E.A. Hydrogen Sorption Properties of V1-x Crx (x = 0.1−0.5) Alloys. Inorg. Mater. 2013, 49, 149–152. [Google Scholar] [CrossRef]
- Kazumi, T.; Tamura, T.; Kamegawa, A.; Takamura, H.; Okada, M. Effect of Absorption-Desorption Cycles on Structure and Stability of Protides in Ti-Cr-V Alloys. Mater. Trans. 2002, 43, 2748–2752. [Google Scholar] [CrossRef]
- Asano, K.; Hayashi, S.; Nakamura, Y. Enhancement of Hydrogen Diffusion in the Body-Centered Tetragonal Monohydride Phase of the V-H System by Substitutional Al Studied by Proton Nuclear Magnetic Resonance. Acta Mater. 2015, 83, 479–487. [Google Scholar] [CrossRef]
- Kagawa, A.; Ono, E.; Kusakabe, T.; Sakamoto, Y. Absorption of Hydrogen by Vanadium-Rich VTi-Based Alloys. J. Less-Common Met. 1991, 172–174, 64–70. [Google Scholar] [CrossRef]
- Lynch, J.F.; Reilly, J.J.; Millot, F. The Absorption of Hydrogen by Binary Vanadium-Chromium Alloys. J. Phys. Chem. Solids 1978, 39, 883–890. [Google Scholar] [CrossRef]
- Wang, J.Y.; Jeng, R.R.; Nieh, J.K.; Lee, S.; Lee, S.L.; Bor, H.Y. Comparing the Hydrogen Storage Alloys-TiCrV and Vanadium-Rich TiCrMnV. Int. J. Hydrogen Energy 2007, 32, 3959–3964. [Google Scholar] [CrossRef]
- Tsukahara, M. Hydrogenation Properties of Vanadium-Based Alloys with Large Hydrogen Storage Capacity. Mater. Trans. 2011, 52, 68–72. [Google Scholar] [CrossRef]
- Yan, Y.; Li, Z.; Wu, Y.; Zhou, S. Hydrogen Absorption-Desorption Characteristic of (Ti0.85Zr0.15)1.1Cr1-XMoxMn Based Alloys with C14 Laves Phase. Prog. Nat. Sci. Mater. Int. 2022, 32, 143–149. [Google Scholar] [CrossRef]
- Kumar, A.; Muthukumar, P.; Kumar, E.A. Absorption Based Solid State Hydrogen Storage System: A Review. Sustain. Energy Technol. Assess. 2022, 52, 102204. [Google Scholar] [CrossRef]
- Aoki, M.; Noritake, T.; Ito, A.; Ishikiriyama, M.; Towata, S.I. Improvement of Cyclic Durability of Ti-Cr-V Alloy by Fe Substitution. Int. J. Hydrogen Energy 2011, 36, 12329–12332. [Google Scholar] [CrossRef]
- Towata, S.I.; Noritake, T.; Itoh, A.; Aoki, M.; Miwa, K. Effect of Partial Niobium and Iron Substitution on Short-Term Cycle Durability of Hydrogen Storage Ti-Cr-V Alloys. Int. J. Hydrogen Energy 2013, 38, 3024–3029. [Google Scholar] [CrossRef]
- Shashikala, K.; Banerjee, S.; Kumar, A.; Pai, M.R.; Pillai, C.G.S. Improvement of Hydrogen Storage Properties of TiCrV Alloy by Zr Substitution for Ti. Int. J. Hydrogen Energy 2009, 34, 6684–6689. [Google Scholar] [CrossRef]
- C. Julien, J.P.; Pereira-Ramos, A.M. (Eds.) New Trends in Intercalation Compounds for Energy Storage NATO Science Series; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2003; ISBN 9781402005954. [Google Scholar]
- Kohlmann, H. Metal Hydrides. Encycl. Phys. Sci. Technol. 2003, 441–458. [Google Scholar] [CrossRef]
- Schwarz, J.A.; Felton, L.E. Compensating Effects in Electromigration Kinetics. Solid State Electron. 1985, 28, 669–675. [Google Scholar] [CrossRef]
- Widenhorn, R.; Mündermann, L.; Rest, A.; Bodegom, E. Meyer-Neldel Rule for Dark Current in Charge-Coupled Devices. J. Appl. Phys. 2001, 89, 8179–8182. [Google Scholar] [CrossRef]
- Ullah, M.; Singh, T.B.; Sitter, H.; Sariciftci, N.S. Meyer-Neldel Rule in Fullerene Field-Effect Transistors. Appl. Phys. A Mater. Sci. Process. 2009, 97, 521–526. [Google Scholar] [CrossRef]
- Andreasen, A.; Vegge, T.; Pedersen, A.S. Compensation Effect in the Hydrogenation/Dehydrogenation Kinetics of Metal Hydrides. J. Phys. Chem. B 2005, 109, 3340–3344. [Google Scholar] [CrossRef]
- Griessen, R.; Boelsma, C.; Schreuders, H.; Broedersz, C.P.; Gremaud, R.; Dam, B. Single Quality Factor for Enthalpy-Entropy Compensation, Isoequilibrium and Isokinetic Relationships. ChemPhysChem 2020, 21, 1632–1643. [Google Scholar] [CrossRef]
- Cornish-Bowden, A. Enthalpy-Entropy Compensation: A Phantom Phenomenon. J. Biosci. 2002, 27, 121–126. [Google Scholar] [CrossRef]
- Patelli, N.; Calizzi, M.; Pasquini, L. Inorganics Interface Enthalpy-Entropy Competition in Nanoscale Metal Hydrides. Inorganics 2018, 6, 13. [Google Scholar] [CrossRef]
- Perez-Benito, J.F.; Mulero-Raichs, M. Enthalpy-Entropy Compensation Effect in Chemical Kinetics and Experimental Errors: A Numerical Simulation Approach. J. Phys. Chem. A 2016, 120, 7598–7609. [Google Scholar] [CrossRef]
- Griessen, R.; Dam, B. Simple Accurate Verification of Enthalpy-Entropy Compensation and Isoequilibrium Relationship. ChemPhysChem 2021, 22, 1774–1784. [Google Scholar] [CrossRef] [PubMed]
- Francia, R.; Huot, J. Microstructure and First Hydrogenation Properties of Ti16V60Cr24−xFex + 4 Wt.% Zr Alloy for x = 0, 4, 8, 12, 16, 20, 24. Energies 2023, 16, 5360. [Google Scholar] [CrossRef]
- Akiba, E.; Okada, M. Metallic Hydrides III: Cubic Solid- Solution Alloys. MRS Bull. 2002, 27, 699–703. [Google Scholar] [CrossRef]
- Lynch, J.F.; Maeland, A.J.; Libowitz, G.G. Lattice Parameter Variation and Thermodynamics of Dihydride Formation in the Vanadium-Rich V—Ti—Fe/H 2 System*. Z. FÜR Phys. Chem. 1985, 145, 51–59. [Google Scholar] [CrossRef]



| x | Maximum Hydrogen Capacity (wt.%) | Crystal Structure | |
|---|---|---|---|
| As-Cast | After Hydrogenation | ||
| 0 | 3.78 | BCC (100%) | BCT (100%) |
| 4 | 3.09 | BCC (100%) | BCT (80%) + C14 (20%) |
| 8 | 2.08 | BCC (100%) | BCT (78%) + C14 (22%) |
| 12 | 1.89 | BCC (79%) + C14 (21%) | BCT (76%) + C14 (24%) |
| 16 | 2.06 | BCC (77%) + C14 (23%) | BCT (58%) + C14 (24%) + C15 (18%) |
| 20 | 1.94 | BCC (74%) + C14 (26%) | BCT (64%) + C14 (23%) + C15 (13%) |
| 24 | 1.99 | BCC (72%) + C14 (28%) | BCT (53%) + C14 (37%) + C15 (11%) |
| x | ΔS (J/K·mol H2) | ΔH (kJ/mol H2) |
|---|---|---|
| 0 | −134 ± 14 | −41 ± 5 |
| 4 | −150 ± 12 | −44 ± 4 |
| 8 | −126 ± 12 | −39 ± 4 |
| 12 | −114 ± 6 | −36 ± 2 |
| 16 | −98 ± 5 | −33 ± 2 |
| 20 | −84 ± 12 | −30 ± 4 |
| 24 | −57 ± 5 | −21 ± 2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ravalison, F.; Huot, J. Hydrogenation Thermodynamics of Ti16V60Cr24−xFex Alloys (x = 0, 4, 8, 12, 16, 20, 24). Hydrogen 2024, 5, 29-38. https://doi.org/10.3390/hydrogen5010003
Ravalison F, Huot J. Hydrogenation Thermodynamics of Ti16V60Cr24−xFex Alloys (x = 0, 4, 8, 12, 16, 20, 24). Hydrogen. 2024; 5(1):29-38. https://doi.org/10.3390/hydrogen5010003
Chicago/Turabian StyleRavalison, Francia, and Jacques Huot. 2024. "Hydrogenation Thermodynamics of Ti16V60Cr24−xFex Alloys (x = 0, 4, 8, 12, 16, 20, 24)" Hydrogen 5, no. 1: 29-38. https://doi.org/10.3390/hydrogen5010003
APA StyleRavalison, F., & Huot, J. (2024). Hydrogenation Thermodynamics of Ti16V60Cr24−xFex Alloys (x = 0, 4, 8, 12, 16, 20, 24). Hydrogen, 5(1), 29-38. https://doi.org/10.3390/hydrogen5010003







