Heat Transfer in Nanomaterial Suspension (CuO and Al2O3) Using KKL Model
Abstract
:1. Introduction
2. Physical Situation
3. Numerical Procedure
Adam Predictor—Corrector Solver
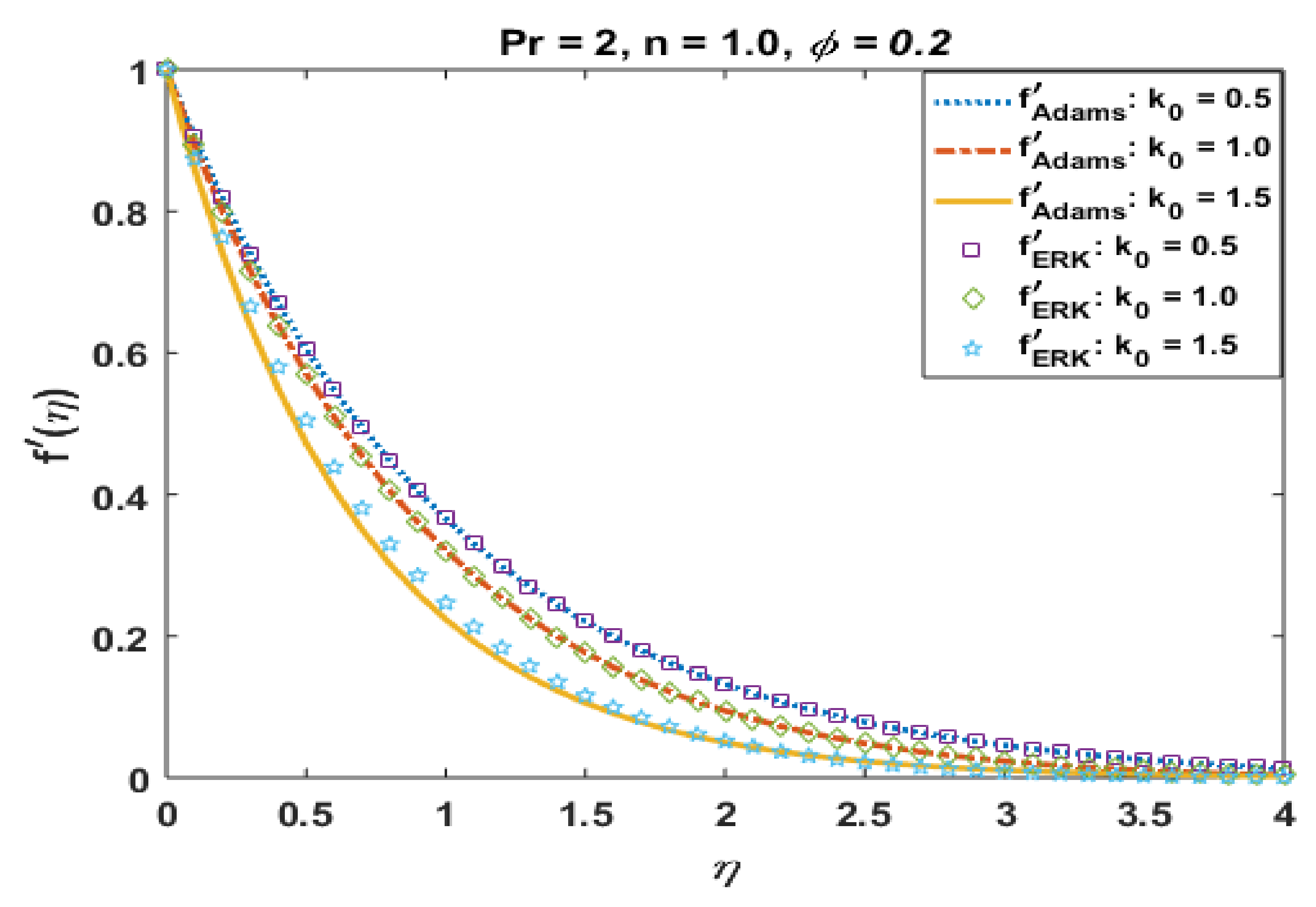
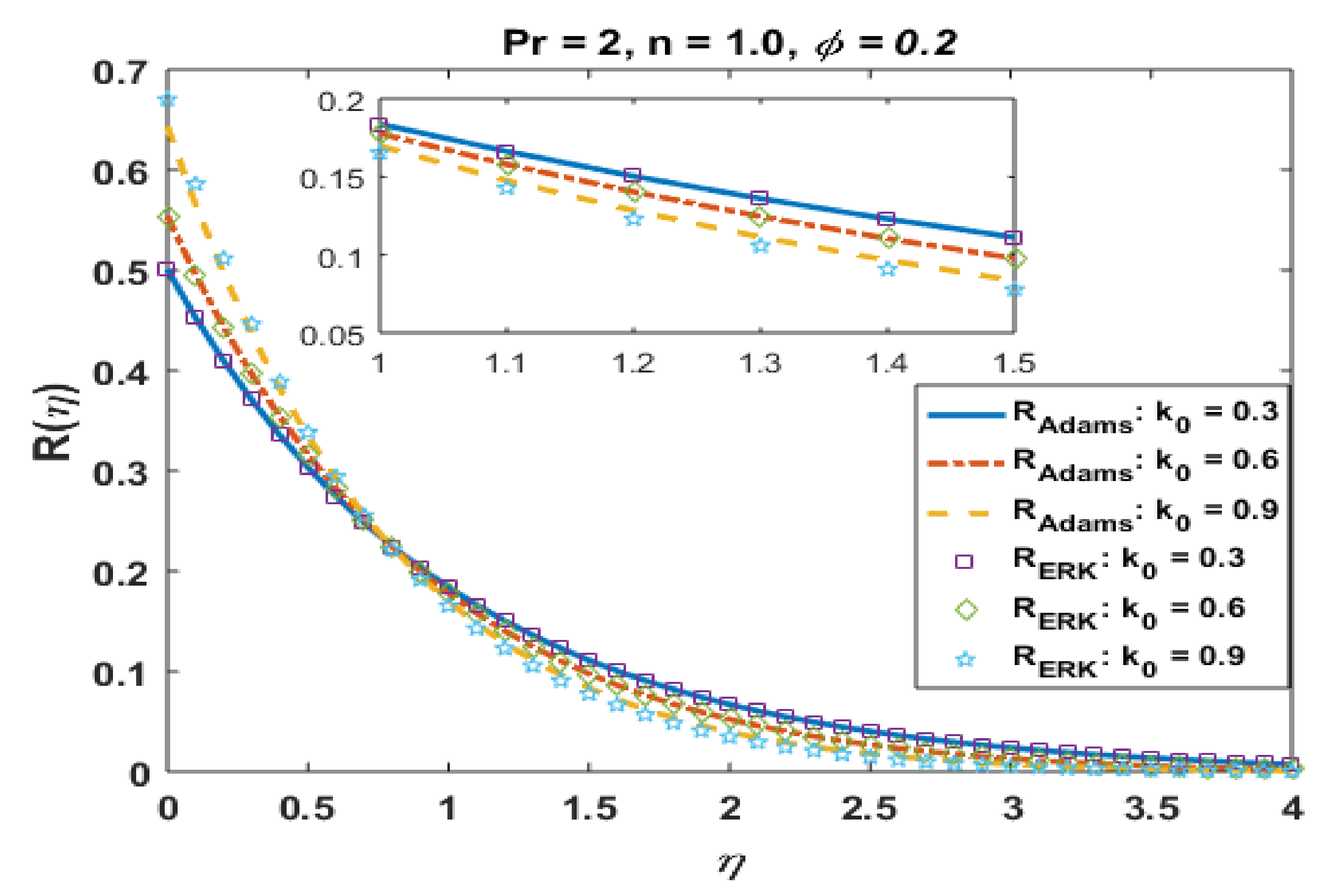
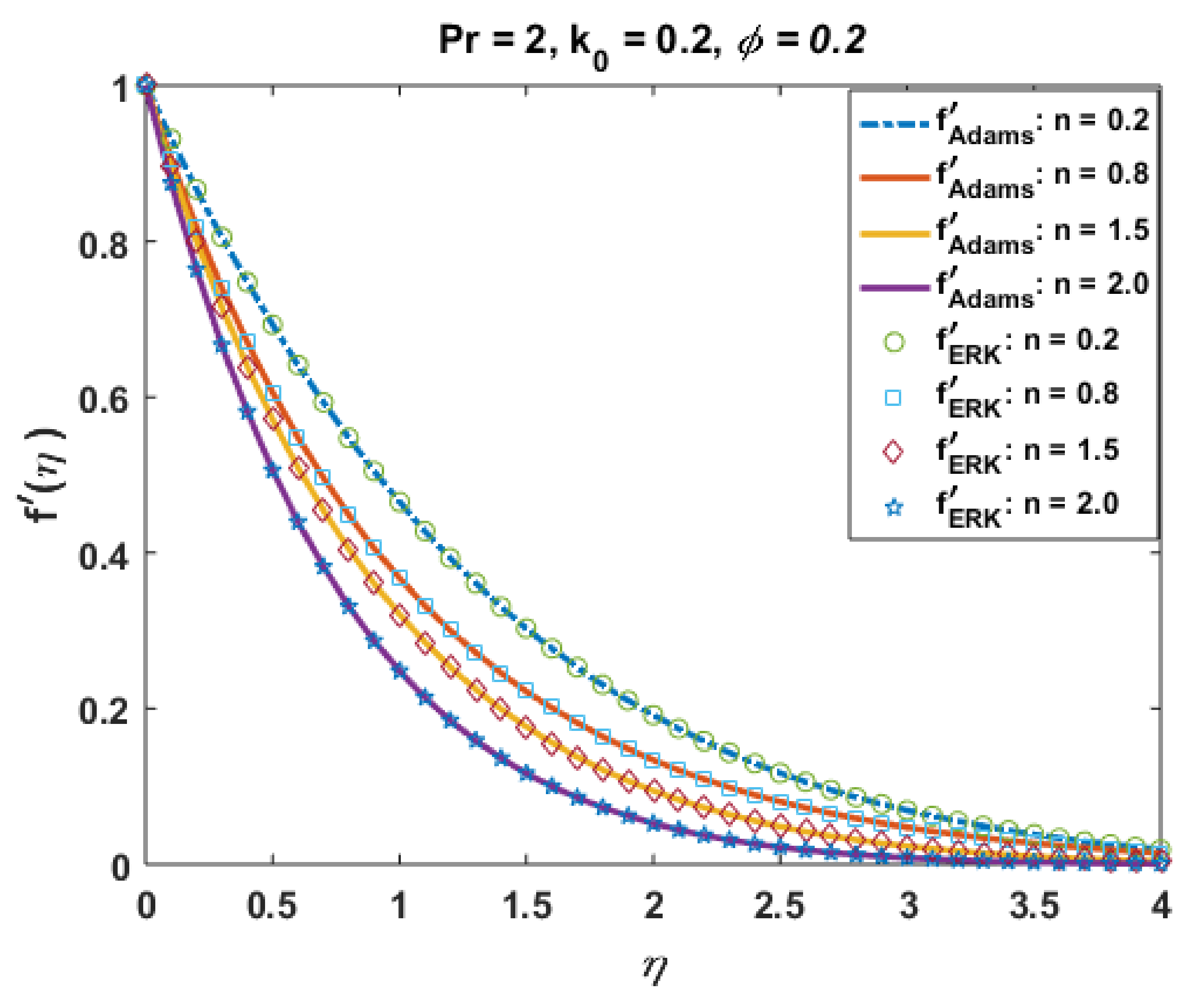
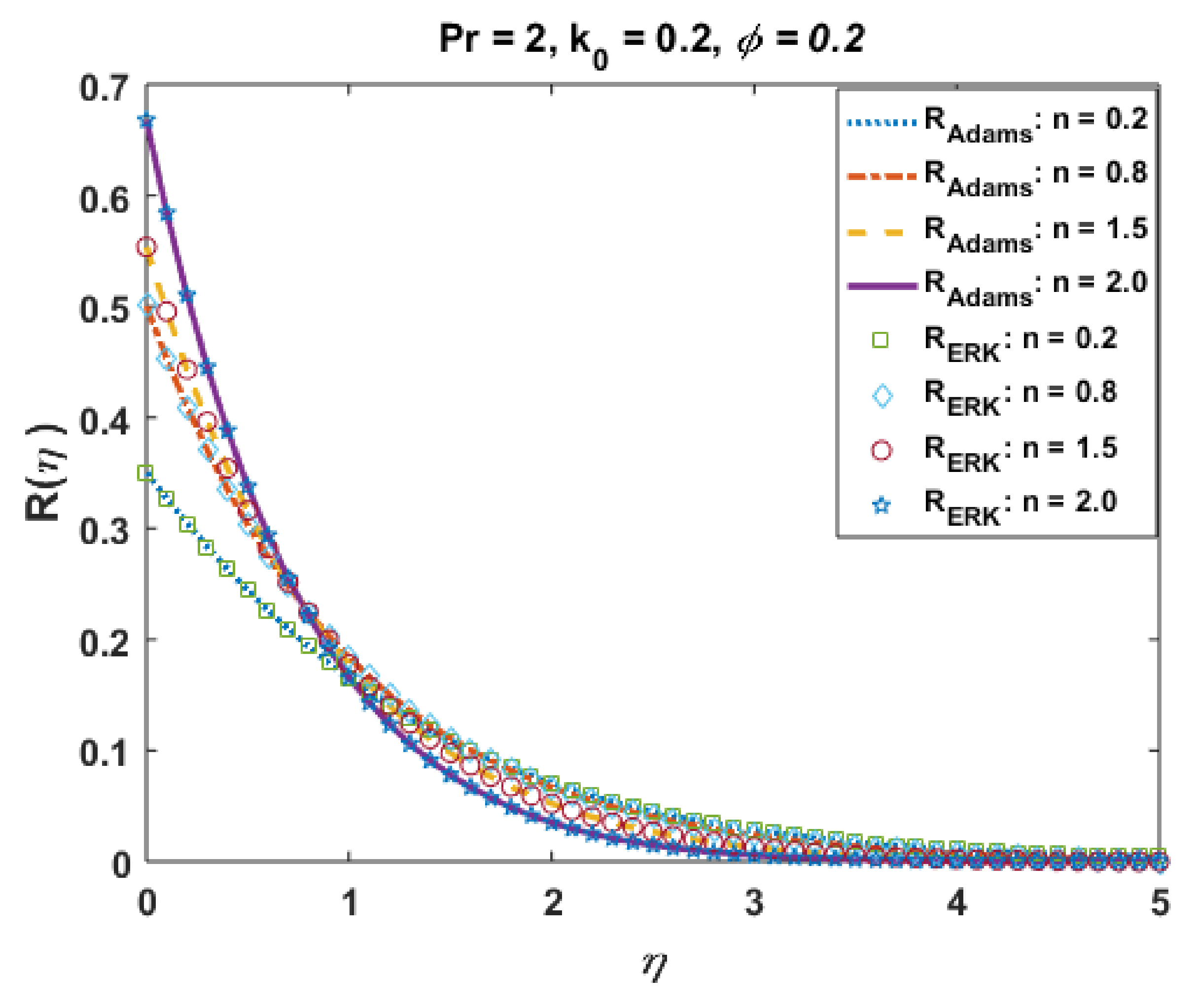
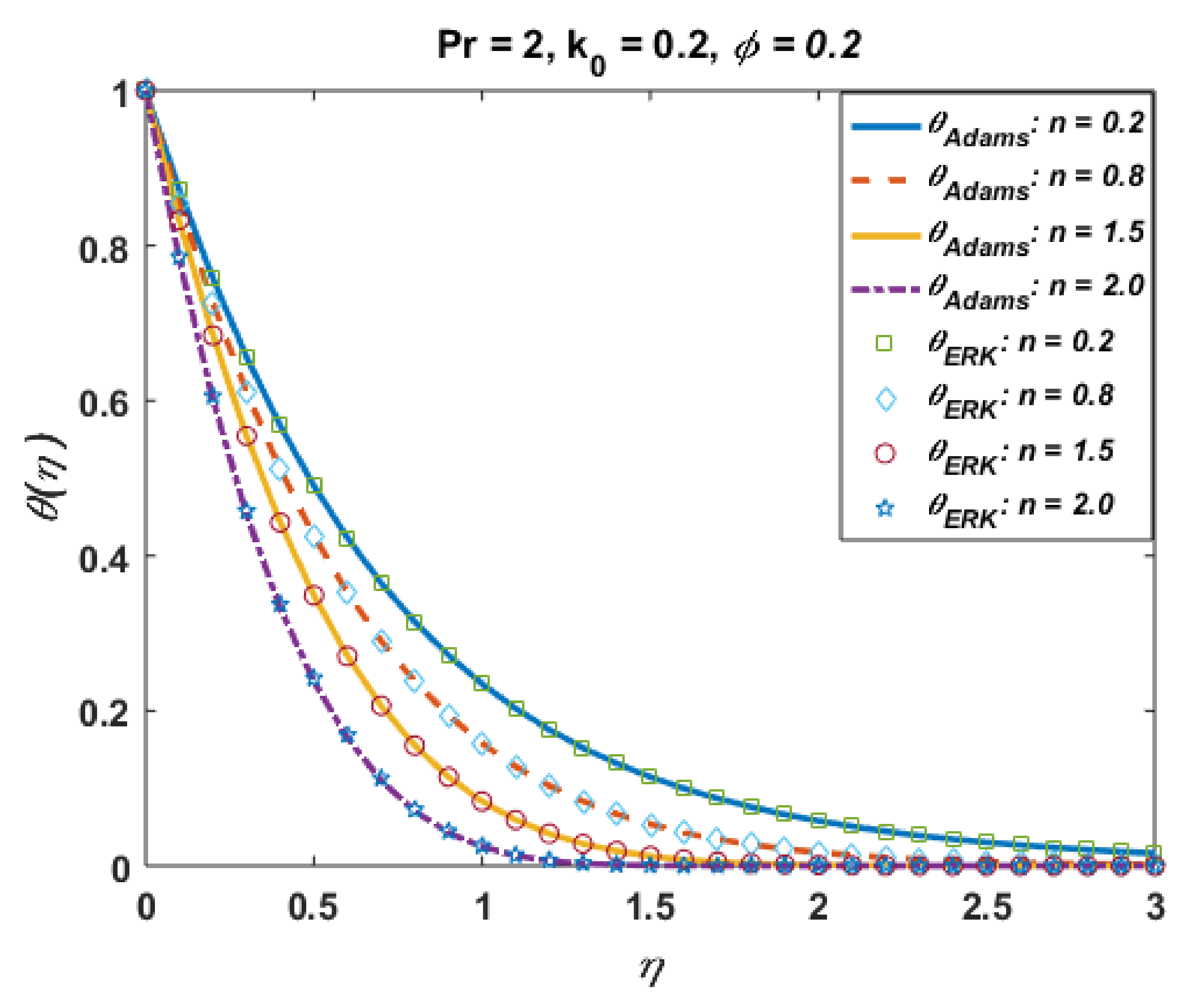
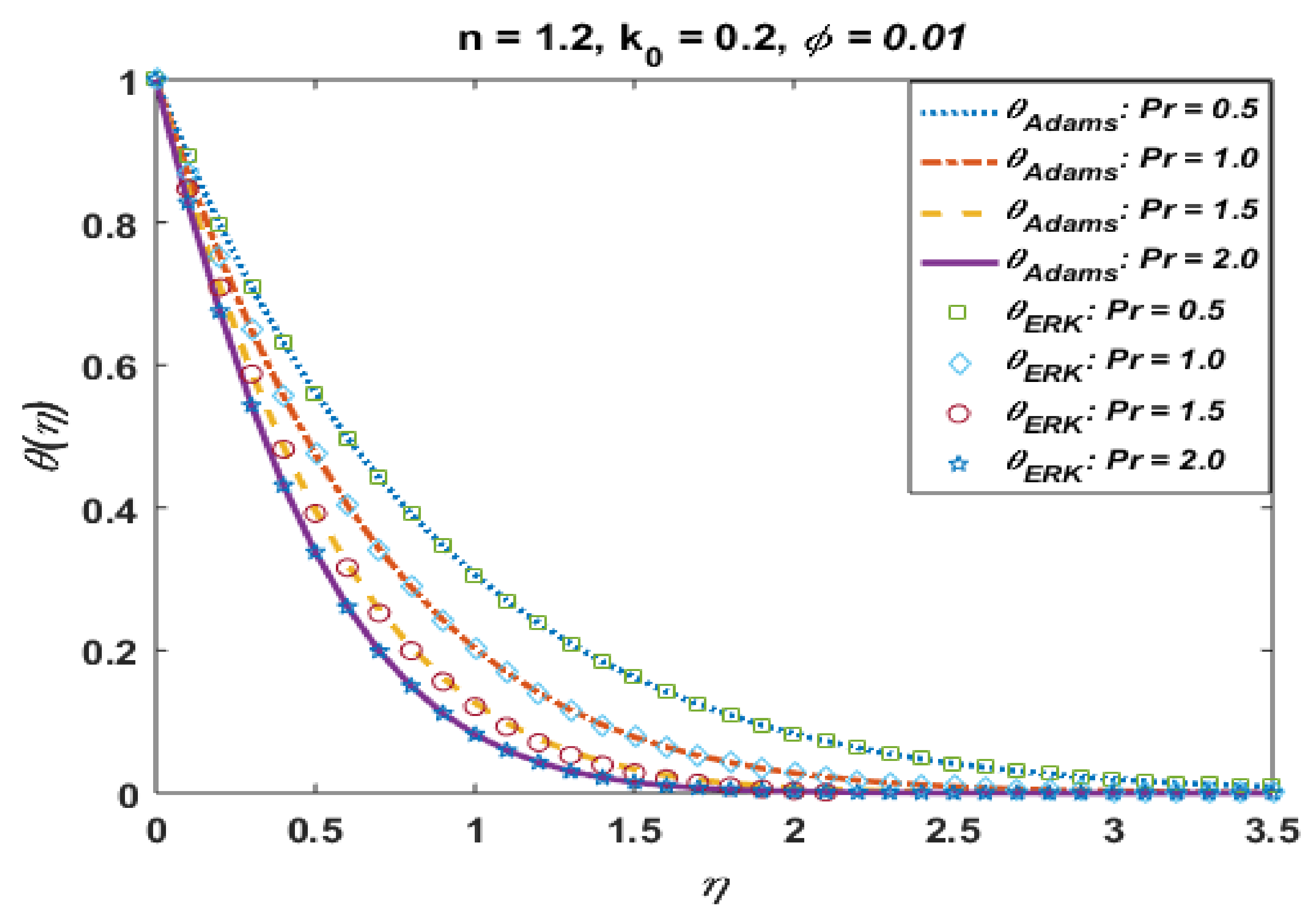
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Eringen, A.C. Theory of micropolar fluids. J. Math. Mech. 1966, 16, 1–18. [Google Scholar] [CrossRef]
- Mehmood, A.; Afsar, K.; Zameer, A.; Awan, S.E.; Raja, M.A.Z. Integrated intelligent computing paradigm for the dynamics of micropolar fluid flow with heat transfer in a permeable walled channel. Appl. Soft Comput. 2019, 79, 139–162. [Google Scholar] [CrossRef]
- Souayeh, B.; Alfannakh, H. Radiative melting heat transfer through a micropolar nanoliquid by using Koo and Kleinstreuer model. Eur. Phys. J. Plus 2021, 136, 1–15. [Google Scholar] [CrossRef]
- Baharifard, F.; Parand, K.; Rashidi, M.M. Novel solution for heat and mass transfer of a MHD micropolar fluid flow on a moving plate with suction and injection. Eng. Comput. 2020, 1–18. [Google Scholar] [CrossRef]
- Yasmin, A.; Ali, K.; Ashraf, M. Study of heat and mass transfer in MHD flow of micropolar fluid over a curved stretching sheet. Sci. Rep. 2020, 10, 1–11. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kumar, K.A.; Sugunamma, V.; Sandeep, N. Influence of viscous dissipation on MHD flow of micropolar fluid over a slendering stretching surface with modified heat flux model. J. Therm. Anal. Calorim. 2020, 139, 3661–3674. [Google Scholar] [CrossRef]
- Lund, L.A.; Omar, Z.; Khan, I.; Raza, J.; Sherif, E.-S.M.; Seikh, A.H. Magnetohydrodynamic (MHD) flow of micropolar fluid with effects of viscous dissipation and Joule heating over an exponential shrinking sheet: Triple solutions and stability analysis. Symmetry 2020, 12, 142. [Google Scholar] [CrossRef] [Green Version]
- Ghadikolaei, S.; Hosseinzadeh, K.; Ganji, D. Numerical study on magnetohydrodynic CNTs-water nanofluids as a micropolar dusty fluid influenced by non-linear thermal radiation and joule heating effect. Powder Technol. 2018, 340, 389–399. [Google Scholar] [CrossRef]
- Singh, K.; Pandey, A.K.; Kumar, M. Slip flow of micropolar fluid through a permeable wedge due to the effects of chemical reaction and heat source/sink with Hall and ion-slip currents: An analytic approach. Propuls. Power Res. 2020, 9, 289–303. [Google Scholar] [CrossRef]
- Rana, S.; Nawaz, M.; Saleem, S.; Alharbi, S.O. Numerical study on enhancement of heat transfer in hybrid nano-micropolar fluid. Phys. Scr. 2019, 95, 045201. [Google Scholar] [CrossRef]
- Ali, L.; Liu, X.; Ali, B.; Mujeed, S.; Abdal, S.; Khan, S.A. Analysis of magnetic properties of nano-particles due to a magnetic dipole in micropolar fluid flow over a stretching sheet. Coatings 2020, 10, 170. [Google Scholar] [CrossRef] [Green Version]
- Usman, A.H.; Shah, Z.; Humphries, U.W.; Kumam, P.; Thounthong, P. Soret, Dufour, and activation energy effects on double diffusive convective couple stress micropolar nanofluid flow in a Hall MHD generator system. AIP Adv. 2020, 10, 075010. [Google Scholar] [CrossRef]
- Tiwari, A.; Shah, P.D.; Chauhan, S.S. Analytical study of micropolar fluid flow through porous layered microvessels with heat transfer approach. Eur. Phys. J. Plus 2020, 135, 209. [Google Scholar] [CrossRef]
- Bhat, A.; Katagi, N.N. Micropolar fluid flow between a non-porous disk and a porous disk with slip: Keller-box solution. Ain Shams Eng. J. 2020, 11, 149–159. [Google Scholar] [CrossRef]
- Péters, F.; Lobry, L.; Lemaire, E. Pressure-driven flow of a micro-polar fluid: Measurement of the velocity profile. J. Rheol. 2010, 54, 311–325. [Google Scholar] [CrossRef] [Green Version]
- Sui, J.; Zheng, L.; Zhang, X.; Chen, G. Mixed convection heat transfer in power law fluids over a moving conveyor along an inclined plate. Int. J. Heat Mass Transf. 2015, 85, 1023–1033. [Google Scholar] [CrossRef]
- Zheng, L.; Zhang, X.; He, J. Transfer behavior of a class of generalized N-diffusion equations in a semi-infinite medium. Int. J. Therm. Sci. 2003, 42, 687–690. [Google Scholar] [CrossRef]
- Kandelousi, M.S. KKL correlation for simulation of nanofluid flow and heat transfer in a permeable channel. Phys. Lett. A 2014, 378, 3331–3339. [Google Scholar] [CrossRef]
- Haq, R.U.; Usman, M.; Algehyne, E.A. Natural convection of CuO–water nanofluid filled in a partially heated corrugated cavity: KKL model approach. Commun. Theor. Phys. 2020, 72, 085003. [Google Scholar] [CrossRef]
- AlSagri, A.S.; Moradi, R. Application of KKL model in studying of nanofluid heat transfer between two rotary tubes. Case Stud. Therm. Eng. 2019, 14, 100478. [Google Scholar] [CrossRef]
- Rana, S.; Nawaz, M. Investigation of enhancement of heat transfer in Sutterby nanofluid using Koo–Kleinstreuer and Li (KKL) correlations and Cattaneo–Christov heat flux model. Phys. Scr. 2019, 94, 115213. [Google Scholar] [CrossRef]
- Pourmehran, O.; Rahimi-Gorji, M.; Hatami, M.; Sahebi, S.; Domairry, G. Numerical optimization of microchannel heat sink (MCHS) performance cooled by KKL based nanofluids in saturated porous medium. J. Taiwan Inst. Chem. Eng. 2015, 55, 49–68. [Google Scholar] [CrossRef]
- Vijaybabu, T. Influence of permeable circular body and CuO–H2O nanofluid on buoyancy-driven flow and entropy generation. Int. J. Mech. Sci. 2020, 166, 105240. [Google Scholar] [CrossRef]
- Haq, R.U.; Aman, S. Water functionalized CuO nanoparticles filled in a partially heated trapezoidal cavity with inner heated obstacle: FEM approach. Int. J. Heat Mass Transf. 2019, 128, 401–417. [Google Scholar] [CrossRef]
- Rana, P.; Shukla, N.; Gupta, Y.; Pop, I. Analytical prediction of multiple solutions for MHD Jeffery–Hamel flow and heat transfer utilizing KKL nanofluid model. Phys. Lett. A 2019, 383, 176–185. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Mahian, O. Enhancement of PCM solidification using inorganic nanoparticles and an external magnetic field with application in energy storage systems. J. Clean. Prod. 2019, 215, 963–977. [Google Scholar] [CrossRef]
- Mehmood, K.; Hussain, S.; Sagheer, M. Numerical simulation of MHD mixed convection in alumina–water nanofluid filled square porous cavity using KKL model: Effects of non-linear thermal radiation and inclined magnetic field. J. Mol. Liq. 2017, 238, 485–498. [Google Scholar] [CrossRef]
- Li, Z.; Shehzad, S.; Sheikholeslami, M. An application of CVFEM for nanofluid heat transfer intensification in a porous sinusoidal cavity considering thermal non-equilibrium model. Comput. Methods Appl. Mech. Eng. 2018, 339, 663–680. [Google Scholar] [CrossRef]
- Gowda, R.J.P.; Munadel, S.A.; Kumar, R.N.; Prasannakumara, B.C.; Issakhov, A.; Gorji, M.R.; Turki, Y.A.A. Computational modelling of nanofluid flow over a curved stretching sheet using Koo–Kleinstreuer and Li (KKL) correlation and modified Fourier heat flux model. Chaos Solitons Fractals 2021, 145, 110774. [Google Scholar] [CrossRef]
- Sheikholeslami, M. Solidification of NEPCM under the effect of magnetic field in a porous thermal energy storage enclosure using CuO nanoparticles. J. Mol. Liq. 2018, 263, 303–315. [Google Scholar] [CrossRef]
- Awan, S.E.; Khan, Z.A.; Awais, M.; Rehman, S.U.; Raja, M.A.Z. Numerical treatment for hydro-magnetic unsteady channel flow of nanofluid with heat transfer. Results Phys. 2018, 9, 1543–1554. [Google Scholar] [CrossRef]
- Awan, S.E.; Awais, M.; Qayyum, A.; Rehman, S.U.; Khan, A.; Ali, H.; Raja, M.A.Z. Numerical computing paradigms for the dynamics of squeezing rheology of third grade fluid. Therm. Sci. 2020, 24, 4173–4182. [Google Scholar] [CrossRef]
- Awais, M.; Aqsa; Malik, M.; Awan, S.E. Generalized magnetic effects in a Sakiadis flow of polymeric nano-liquids: Analytic and numerical solutions. J. Mol. Liq. 2017, 241, 570–576. [Google Scholar] [CrossRef]
- Awan, S.E.; Raja, M.A.Z.; Mehmood, A.; Niazi, S.A.; Siddiqa, S. Numerical Treatments to Analyze the Nonlinear Radiative Heat Transfer in MHD Nanofluid Flow with Solar Energy. Arab. J. Sci. Eng. 2020, 45, 4975–4994. [Google Scholar] [CrossRef]
- Awan, S.E.; Raja, M.A.Z.; Gul, F.; Khan, Z.A.; Mehmood, A.; Shoaib, M. Numerical Computing Paradigm for Investigation of Micropolar Nanofluid Flow Between Parallel Plates System with Impact of Electrical MHD and Hall Current. Arab. J. Sci. Eng. 2021, 46, 645–662. [Google Scholar] [CrossRef]
- Awais, M.; Awan, S.E.; Raja, M.A.Z.; Parveen, N.; Khan, W.U.; Malik, M.Y.; He, Y. Effects of Variable Transport Properties on Heat and Mass Transfer in MHD Bioconvective Nanofluid Rheology with Gyrotactic Microorganisms: Numerical Approach. Coatings 2021, 11, 231. [Google Scholar] [CrossRef]
- Radhika, M.; Mahanthesh, B.; Thriveni, K. Solar radiative heat-driven Sakiadis flow of a dusty nanoliquid with Brownian motion and an exponential space-based heat source: Koo–Kleinstreuer–Li (KKL) model. Heat Transfer 2021, 50, 1232–1251. [Google Scholar] [CrossRef]
- Khan, J.A.; Raja, M.A.Z.; Syam, M.I.; Tanoli, S.A.K.; Awan, S.E. Design and application of nature inspired computing approach for nonlinear stiff oscillatory problems. Neural Comput. Appl. 2015, 26, 1763–1780. [Google Scholar] [CrossRef]
- Cheema, T.N.; Raja, M.A.Z.; Ahmad, I.; Naz, S.; Ilyas, H.; Shoaib, M. Intelligent computing with Levenberg–Marquardt artificial neural networks for nonlinear system of COVID-19 epidemic model for future generation disease control. Eur. Phys. J. Plus 2020, 135, 1–35. [Google Scholar]
- Parveen, N.; Awais, M.; Mumraz, S.; Ali, A.; Malik, M.Y. An estimation of pressure rise and heat transfer rate for hybrid nanofluid with endoscopic effects and induced magnetic field: Computational intelligence application. Eur. Phys. J. Plus 2020, 135, 1–41. [Google Scholar] [CrossRef]
- Ilyas, H.; Ahmad, I.; Raja, M.A.Z.; Tahir, M.B.; Shoaib, M. Intelligent computing for the dynamics of fluidic system of electrically conducting Ag/Cu nanoparticles with mixed convection for hydrogen possessions. Int. J. Hydrogen Energy 2021, 46, 4947–4980. [Google Scholar] [CrossRef]
- Sabir, Z.; Raja, M.A.Z.; Arbi, A.; Altamirano, G.C.; Cao, J. Neuro-swarms intelligent computing using Gudermannian kernel for solving a class of second order Lane-Emden singular nonlinear model. AIMS Math. 2021, 6, 2468–2485. [Google Scholar] [CrossRef]
- Awais, M.; Awan, S.E.; Iqbal, K.; Khan, Z.A.; Raja, M.A.Z. Hydromagnetic mixed convective flow over a wall with variable thickness and Cattaneo-Christov heat flux model: OHAM analysis. Results Phys. 2018, 8, 621–627. [Google Scholar] [CrossRef]
- Awais, M.; Awan, S.E.; Raja, M.A.Z.; Shoaib, M. Effects of Gyro-Tactic Organisms in Bio-convective Nano-material with Heat Immersion, Stratification, and Viscous Dissipation. Arab. J. Sci. Eng. 2020, 1–14. [Google Scholar] [CrossRef]
- Siddiqa, S.; Naqvi, S.; Begum, N.; Awan, S.; Hossain, M. Thermal radiation therapy of biomagnetic fluid flow in the presence of localized magnetic field. Int. J. Therm. Sci. 2018, 132, 457–465. [Google Scholar] [CrossRef]
- Awan, S.E.; Awais, M.; Rehman, S.U.; Niazi, S.A.; Raja, M.A.Z. Dynamical analysis for nanofluid slip rheology with thermal radiation, heat generation/absorption and convective wall properties. AIP Adv. 2018, 8, 075122. [Google Scholar] [CrossRef] [Green Version]
- Awais, M.; Raja, M.A.Z.; Awan, S.E.; Shoaib, M.; Ali, H.M. Heat and mass transfer phenomenon for the dynamics of Casson fluid through porous medium over shrinking wall subject to Lorentz force and heat source/sink. Alex. Eng. J. 2021, 60, 1355–1363. [Google Scholar] [CrossRef]

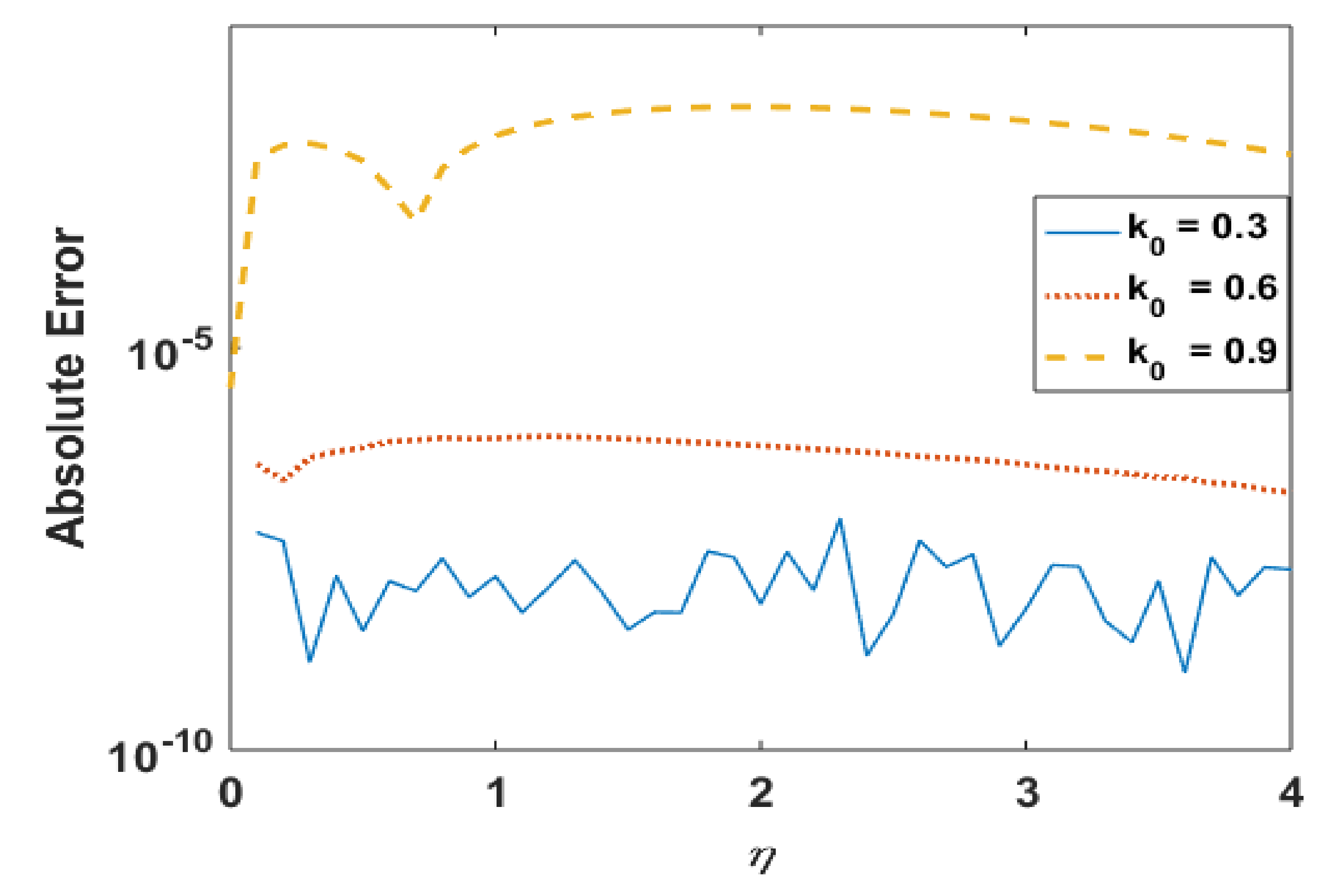
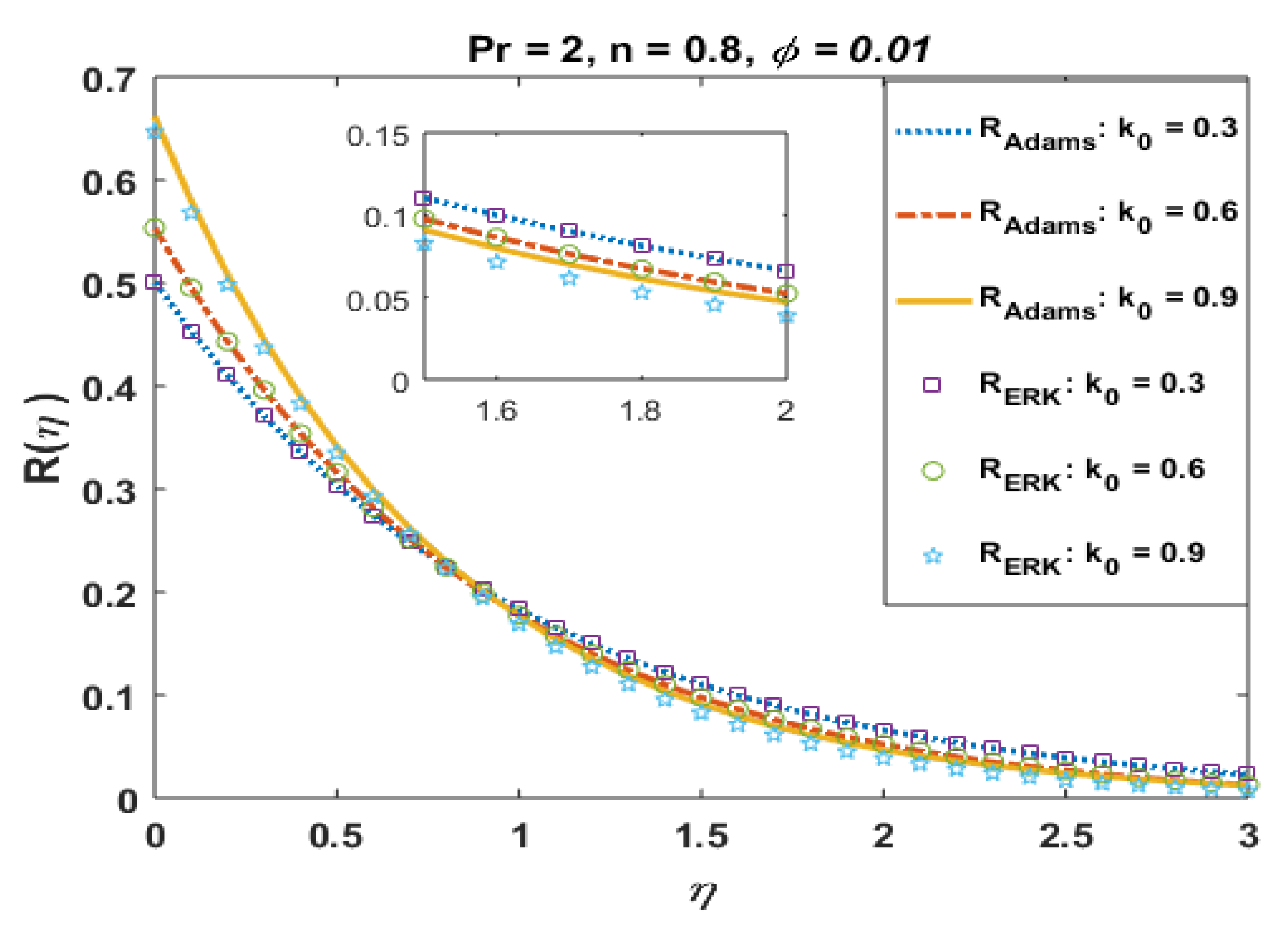
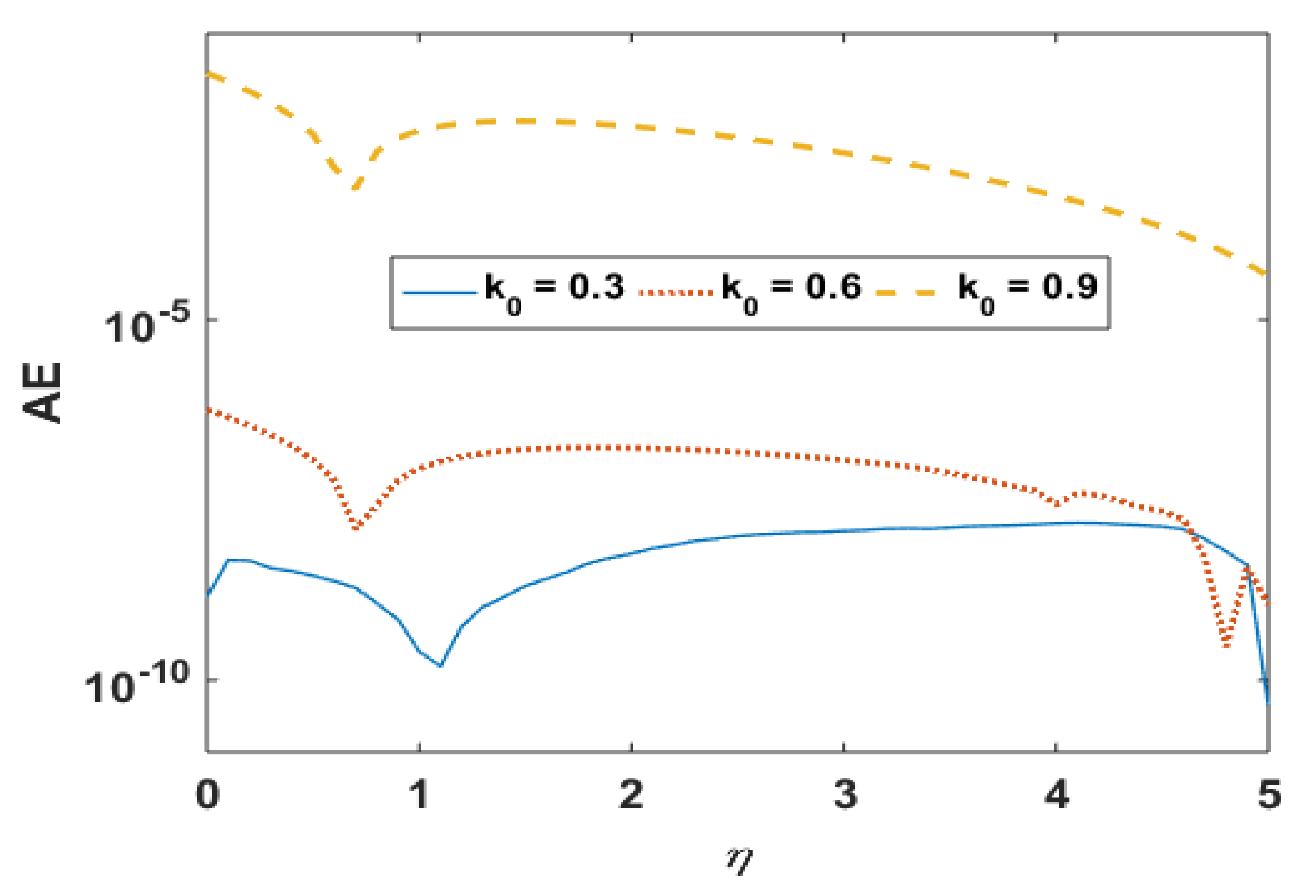
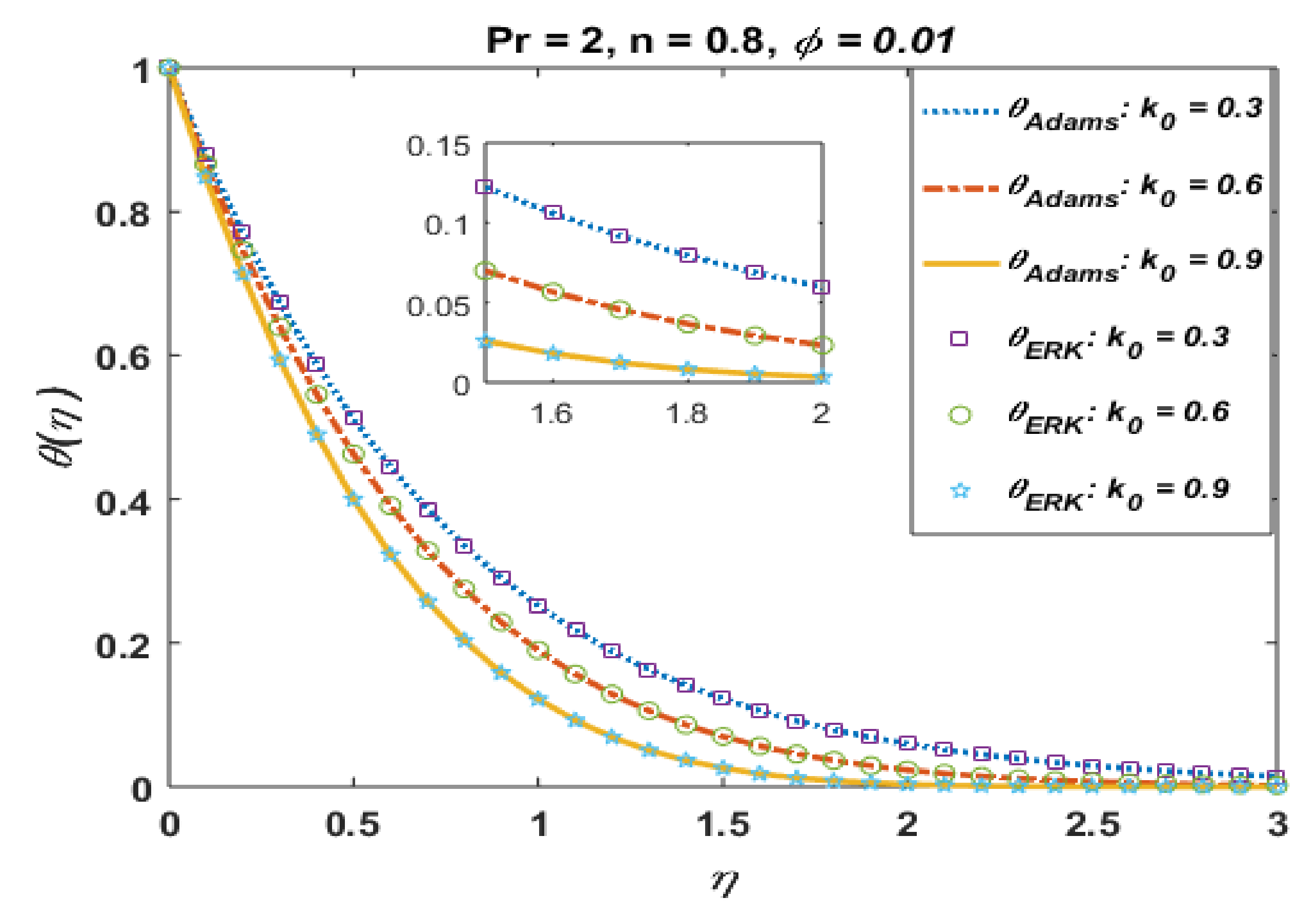

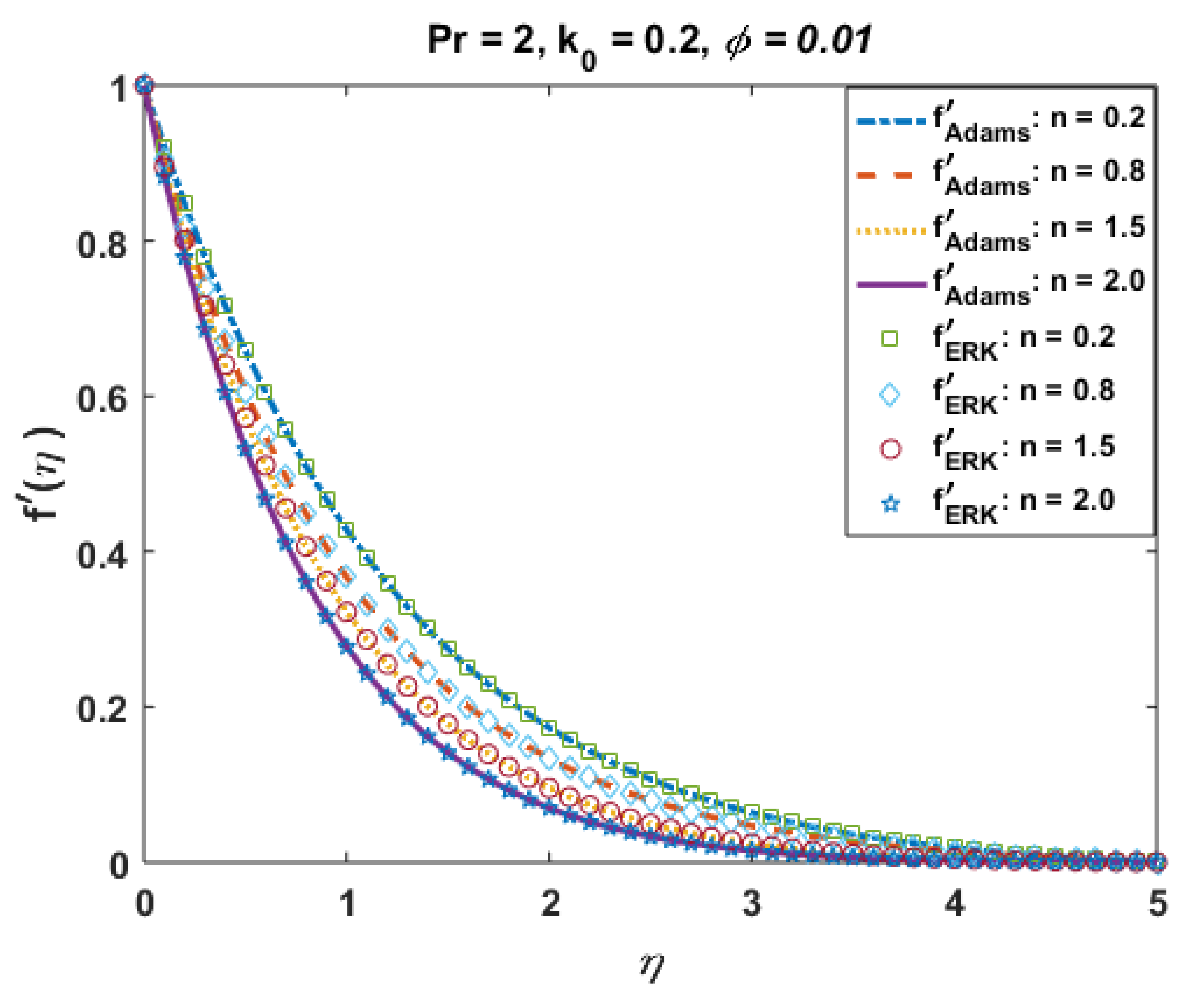
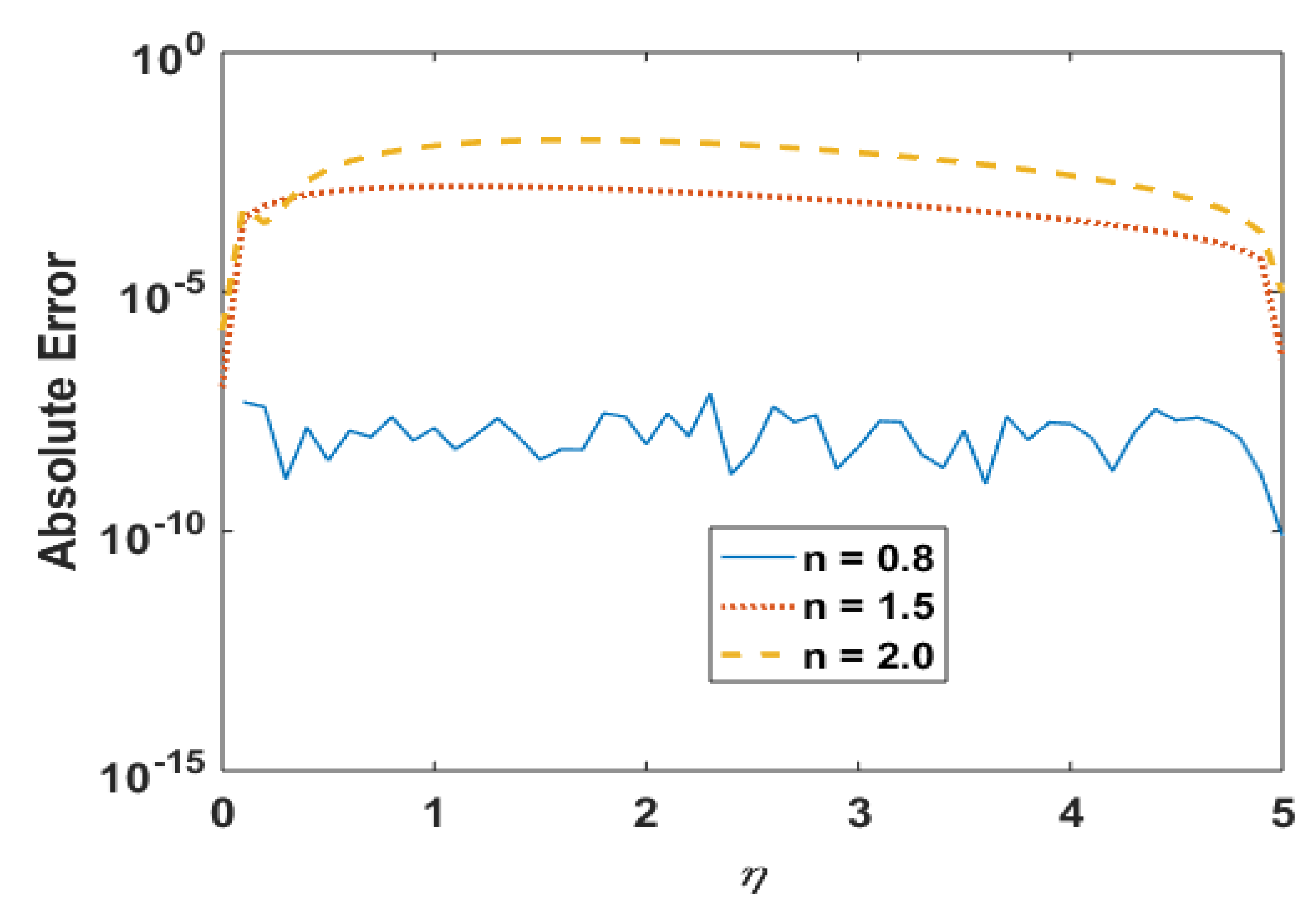
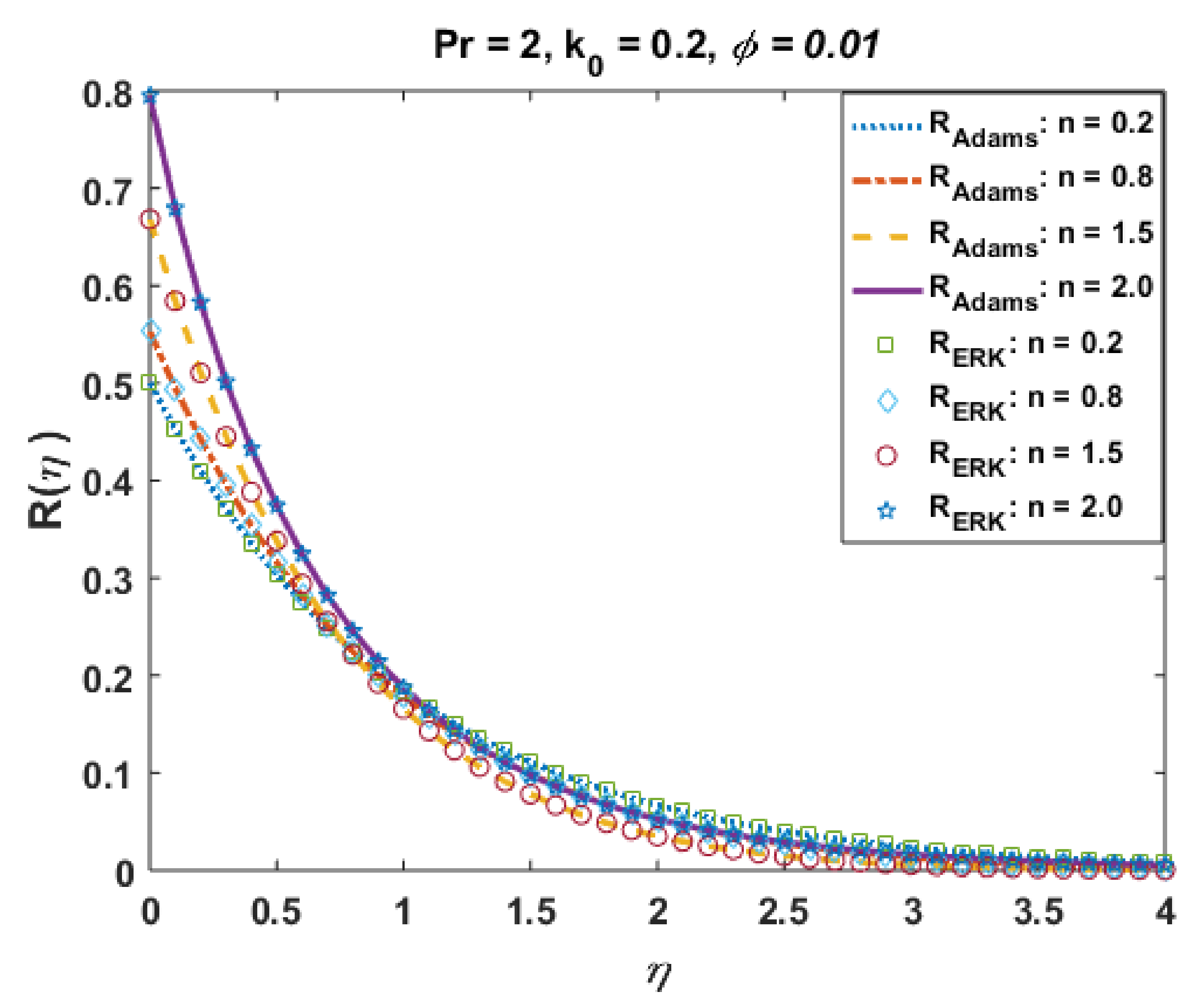



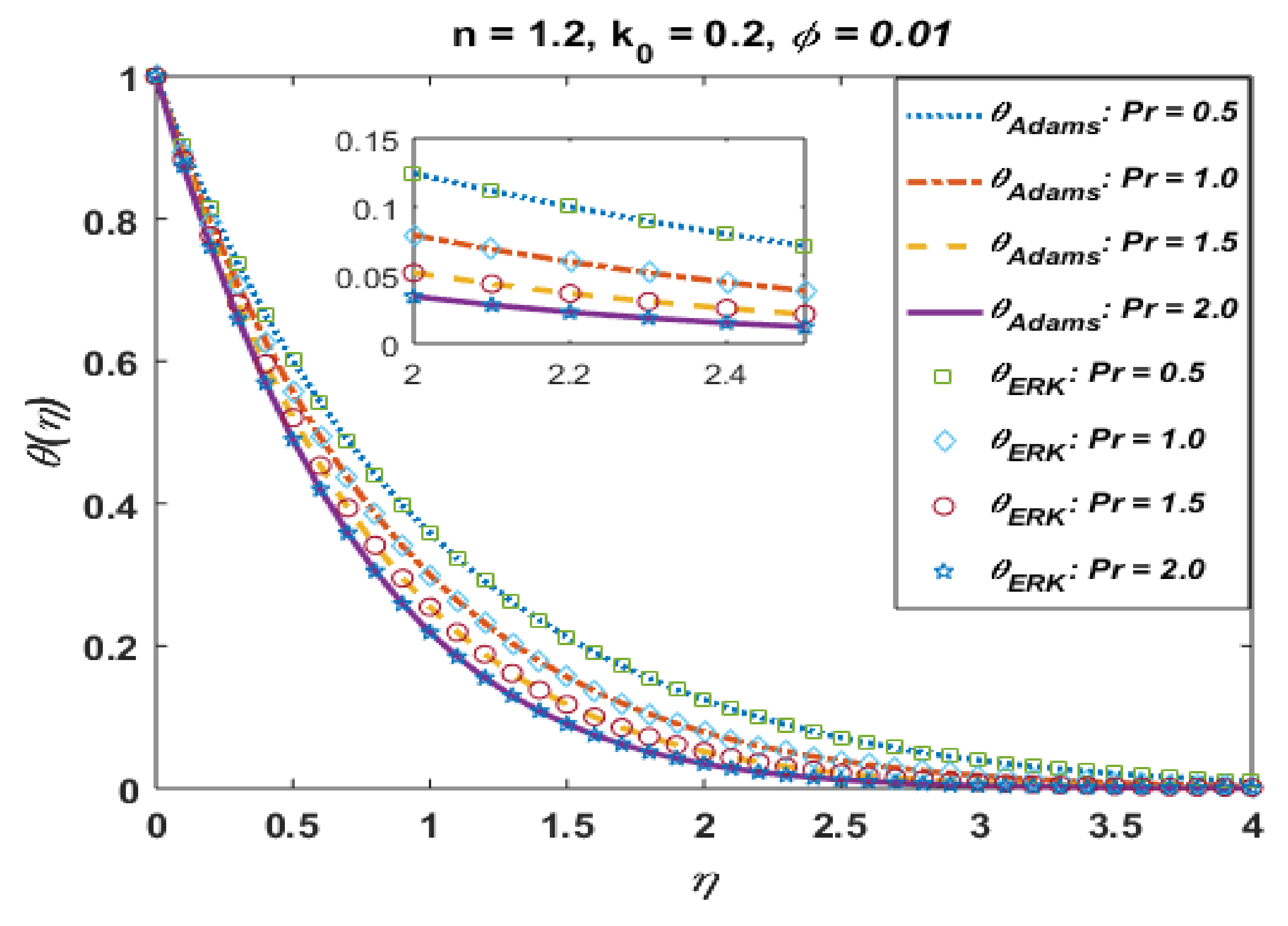


| Classical Models | Novel Models |
|---|---|
| classical Fourier law of heat flux | novel Fourier law of heat flux |
| classical stress–strain model | novel stress–strain model |
| Physical Property | Water/Base Fluid | ||
|---|---|---|---|
| 997.1 | 8933 | 3970 | |
| 4179 | 385 | 765 | |
| 0.613 | 401 | 25 | |
| - | 40 | 47 | |
| 0.05 | 5.96 × 1007 | 3.69 × 10 07 |
| Coefficient Values | CuO-Water [37] | Al2O3-Water |
|---|---|---|
| a1 | −26.593310846 | 52.813488759 |
| a2 | −0.403818333 | 6.115637295 |
| a3 | −33.3516805 | 0.6955715084 |
| a4 | −1.915825591 | 4.17455552786 × 10−2 |
| a5 | 6.42185846658 × 10−2 | 0.176919300241 |
| a6 | 48.40336955 | −298.19819084 |
| a7 | −9.787756683 | −34.532716906 |
| a8 | 190.245610009 | −3.9225289283 |
| a9 | 10.9285386565 | −0.2354329626 |
| a10 | −0.72009983664 | −0.999063481 |
| Index | Case | ||||
|---|---|---|---|---|---|
| 0 | 2.6727 | 1.7826 | 2.6976 | 1.7774 | |
| 0.1 | 3.3973 | 1.7291 | 3.4293 | 1.7233 | |
| 0.2 | 4.2556 | 1.6664 | 4.2897 | 1.6604 | |
| 0.3 | 5.2086 | 1.5909 | 5.2426 | 1.5847 | |
| 1.5 | 7.2436 | 1.1847 | 7.3631 | 1.1700 | |
| 2.73 | 7.3249 | 1.3146 | 7.3596 | 1.3038 | |
| 3.2 | 7.3394 | 1.3587 | 7.3590 | 1.3502 | |
| 4.0 | 7.3562 | 1.4205 | 7.3583 | 1.4179 | |
| 0.01 | 3.4186 | 1.7298 | 7.3515 | 1.3084 | |
| 0.04 | 3.3973 | 1.7291 | 7.3596 | 1.3038 | |
| 0.10 | 3.2506 | 1.7377 | 7.3582 | 1.2996 | |
| 0.15 | 2.9983 | 1.7575 | 7.3419 | 1.3001 | |
| Method | Accuracy Goal | ||||||
|---|---|---|---|---|---|---|---|
| Time | Steps | Evaluation | Time | Steps | Evaluation | ||
| Adams | 0.92875 | 177 | 395 | 1.675 | 184 | 398 | |
| 0.765625 | 162 | 374 | 1.37 | 172 | 384 | ||
| 0.8125 | 149 | 360 | 1.25 | 158 | 342 | ||
| 0.296875 | 64 | 145 | 0.39062 | 62 | 135 | ||
| BDF | 1.6875 | 243 | 657 | 1.95313 | 284 | 724 | |
| 1.60938 | 237 | 633 | 1.70313 | 249 | 681 | ||
| 1.32813 | 227 | 600 | 1.23438 | 219 | 575 | ||
| 0.4375 | 108 | 291 | 0.671875 | 105 | 291 | ||
| ERK | 3.45313 | 115 | 1837 | 3.9875 | 116 | 1853 | |
| 2.6875 | 84 | 1341 | 3.84375 | 84 | 1341 | ||
| 0.359375 | 67 | 675 | 0.359375 | 34 | 649 | ||
| 0.28125 | 21 | 212 | 0.28125 | 19 | 193 | ||
| IRK | 7.51563 | 145 | 2041 | 53.5 | 141 | 1968 | |
| 4.32813 | 113 | 1716 | 46.9375 | 97 | 1456 | ||
| 3.45313 | 96 | 1563 | 14.4688 | 89 | 1622 | ||
| 0.21875 | 39 | 641 | 12.5 | 41 | 670 | ||
| ET | 0.984375 | 199 | 420 | 1.88125 | 203 | 428 | |
| 0.87375 | 171 | 388 | 1.84375 | 183 | 387 | ||
| 0.828125 | 152 | 367 | 1.38264 | 174 | 363 | ||
| 0.467835 | 92 | 205 | 0.884375 | 98 | 183 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Awais, M.; Ehsan Awan, S.; Raja, M.A.Z.; Nawaz, M.; Khan, W.U.; Yousaf Malik, M.; He, Y. Heat Transfer in Nanomaterial Suspension (CuO and Al2O3) Using KKL Model. Coatings 2021, 11, 417. https://doi.org/10.3390/coatings11040417
Awais M, Ehsan Awan S, Raja MAZ, Nawaz M, Khan WU, Yousaf Malik M, He Y. Heat Transfer in Nanomaterial Suspension (CuO and Al2O3) Using KKL Model. Coatings. 2021; 11(4):417. https://doi.org/10.3390/coatings11040417
Chicago/Turabian StyleAwais, Muhammad, Saeed Ehsan Awan, Muhammad Asif Zahoor Raja, Muhammad Nawaz, Wasim Ullah Khan, Muhammad Yousaf Malik, and Yigang He. 2021. "Heat Transfer in Nanomaterial Suspension (CuO and Al2O3) Using KKL Model" Coatings 11, no. 4: 417. https://doi.org/10.3390/coatings11040417
APA StyleAwais, M., Ehsan Awan, S., Raja, M. A. Z., Nawaz, M., Khan, W. U., Yousaf Malik, M., & He, Y. (2021). Heat Transfer in Nanomaterial Suspension (CuO and Al2O3) Using KKL Model. Coatings, 11(4), 417. https://doi.org/10.3390/coatings11040417









