The Mathematics of a Successful Deconvolution: A Quantitative Assessment of Mixture-Based Combinatorial Libraries Screened Against Two Formylpeptide Receptors
Abstract
:1. Introduction

2. Results and Discussion
2.1. Comparison of Scaffold Ranking and Positional Scanning Using the Harmonic Mean


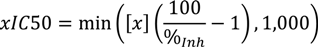
| Library | FPR1 | FPR2 | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Scaffold Ranking | Harmonic Means of Positional Scanning xIC50s | Scaffold Ranking | Harmonic Means of Positional Scanning xIC50s | |||||||||||
| xIC50 | SEM | P1 | P2 | P3 | P4 | AVG | xIC50 | SEM | P1 | P2 | P3 | P4 | AVG | |
| 1 | 708 | 175 | 489 | 540 | NA | NA | 514 | 609 | 226 | 349 | 353 | NA | NA | 351 |
| 2 | 1000 | 0 | 540 | 666 | 601 | NA | 602 | 563 | 252 | 259 | 381 | 402 | NA | 347 |
| 3 | 862 | 85 | 334 | 500 | 458 | NA | 431 | 720 | 200 | 437 | 257 | 260 | NA | 318 |
| 4 | 389 | 166 | 623 | 521 | 662 | NA | 602 | 123 | 37 | 494 | 393 | 324 | NA | 404 |
| 5 | 73 | 13 | 191 | 195 | 547 | 515 | 362 | 183 | 41 | 250 | 273 | 463 | 478 | 366 |
| 6 | 361 | 214 | 336 | 287 | 188 | 190 | 250 | 399 | 206 | 393 | 257 | 273 | 284 | 302 |
| 7 | 1000 | 0 | 583 | 451 | 575 | NA | 536 | 136 | 42 | 117 | 264 | 149 | NA | 177 |
| 8 | 308 | 232 | 145 | 160 | 200 | NA | 168 | 65 | 27 | 131 | 131 | 149 | NA | 137 |
| 9 | 268 | 165 | 456 | 666 | 491 | NA | 538 | 384 | 215 | 660 | 952 | 516 | NA | 709 |
| 10 | 786 | 214 | 707 | 820 | 551 | NA | 692 | 1000 | 0 | 977 | 901 | 440 | NA | 773 |
| 11 | 628 | 216 | 690 | 764 | 431 | NA | 628 | 291 | 236 | 365 | 315 | 392 | NA | 358 |
| 12 | 1000 | 0 | 725 | 666 | 470 | NA | 620 | 798 | 202 | 287 | 467 | 311 | NA | 355 |
| 13 | 744 | 165 | 406 | 344 | 417 | NA | 389 | 1000 | 0 | 276 | 288 | 234 | NA | 266 |
| 14 | 823 | 177 | 638 | 452 | 400 | NA | 497 | 1000 | 0 | 321 | 354 | 260 | NA | 312 |
| 15 | 1000 | 0 | 462 | 523 | 538 | NA | 508 | 781 | 219 | 555 | 652 | 672 | NA | 626 |
| 16 | 779 | 221 | 456 | 612 | 520 | NA | 529 | 1000 | 0 | 525 | 615 | 819 | NA | 653 |
| 17 | 1000 | 0 | 538 | 773 | 909 | NA | 740 | 1000 | 0 | 510 | 478 | 646 | NA | 545 |
| 18 | 803 | 197 | 581 | 799 | 1000 | 538 | 730 | 764 | 236 | 274 | 319 | 566 | 396 | 389 |
| 19 | 15 | 4 | 77 | 49 | 112 | 90 | 82 | 29 | 8 | 74 | 82 | 137 | 100 | 98 |
| 20 | 1000 | 0 | 206 | 368 | 545 | 431 | 387 | 640 | 218 | 184 | 319 | 424 | 331 | 315 |
| 21 | 1000 | 0 | 405 | 134 | 200 | 488 | 307 | 215 | 184 | 314 | 188 | 306 | 485 | 323 |
| 22 | 1000 | 0 | 197 | 500 | 487 | 640 | 456 | 1000 | 0 | 162 | 577 | 479 | 521 | 435 |
| 23 | 790 | 210 | 424 | 394 | 685 | NA | 501 | 1000 | 0 | 262 | 469 | 430 | NA | 387 |
| 24 | 44 | 12 | 174 | 273 | 188 | NA | 212 | 1000 | 0 | 433 | 627 | 512 | NA | 524 |
| 25 | 839 | 161 | 284 | 341 | 711 | NA | 445 | 771 | 229 | 424 | 508 | 579 | NA | 504 |
| 26 | 871 | 129 | 912 | 994 | 717 | NA | 874 | 332 | 223 | 424 | 397 | 316 | NA | 379 |
| 27 | 1000 | 0 | 705 | 772 | 389 | NA | 622 | 660 | 218 | 411 | 420 | 334 | NA | 388 |
| 28 | 588 | 242 | 593 | 361 | 684 | NA | 546 | 349 | 223 | 469 | 475 | 581 | NA | 508 |
| 29 | 1000 | 0 | 455 | 351 | 510 | NA | 439 | 1000 | 0 | 227 | 359 | 497 | NA | 361 |
| 30 | 1000 | 0 | 688 | 696 | 849 | NA | 744 | 866 | 134 | 606 | 509 | 421 | NA | 512 |
| 31 | 782 | 218 | 630 | 614 | 584 | NA | 609 | 1000 | 0 | 329 | 357 | 364 | NA | 350 |
| 32 | 144 | 12 | 97 | 70 | 141 | NA | 102 | 800 | 200 | 277 | 407 | 418 | NA | 367 |

2.2. Analysis of Positional Scanning Profiles
 be the rank-ordered activities of the mixtures in that position, so that x1 is the most active mixture’s activity, x2 is the second-most active mixture’s activity, etc. In this study, percentage inhibition values were used for the activities; since we are attempting to compare the differentiation of positional scanning profiles within a single study, absolute scaling issues are irrelevant so long as they are consistent, and so long as higher numbers correspond to greater activity. Next, the maximum drop in activity:
be the rank-ordered activities of the mixtures in that position, so that x1 is the most active mixture’s activity, x2 is the second-most active mixture’s activity, etc. In this study, percentage inhibition values were used for the activities; since we are attempting to compare the differentiation of positional scanning profiles within a single study, absolute scaling issues are irrelevant so long as they are consistent, and so long as higher numbers correspond to greater activity. Next, the maximum drop in activity:

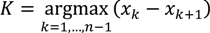
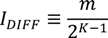
| Library | FPR1 | FPR2 | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| IDIFF | IDECON | IDIFF | IDECON | |||||||||
| P1 | P2 | P3 | P4 | AVG | P1 | P2 | P3 | P4 | AVG | |||
| 1 | 4.55 | 1.01 | NA | NA | 2.78 | 5.41 | 2.55 | 2.05 | NA | NA | 2.30 | 6.56 |
| 2 | 3.70 | 0.65 | 0.80 | NA | 1.72 | 2.85 | 10.60 | 1.00 | 1.40 | NA | 4.33 | 12.47 |
| 3 | 0.15 | 1.00 | 1.30 | NA | 0.82 | 1.90 | 1.80 | 4.10 | 0.55 | NA | 2.15 | 6.77 |
| 4 | 3.60 | 3.30 | 1.70 | NA | 2.87 | 4.76 | 4.50 | 1.40 | 0.09 | NA | 2.00 | 4.95 |
| 5 | 6.00 | 0.26 | 0.15 | 6.10 | 3.13 | 8.64 | 0.80 | 0.38 | 0.70 | 0.13 | 0.50 | 1.37 |
| 6 | 1.85 | 15.20 | 14.10 | 4.70 | 8.96 | 35.82 | 1.65 | 4.60 | 15.70 | 9.50 | 7.86 | 26.03 |
| 7 | 0.09 | 1.15 | 1.40 | NA | 0.88 | 1.64 | 9.10 | 0.26 | 10.95 | NA | 6.77 | 38.33 |
| 8 | 0.07 | 0.58 | 0.00 | NA | 0.21 | 1.27 | 8.30 | 0.68 | 0.44 | NA | 3.14 | 22.89 |
| 9 | 1.80 | 1.50 | 1.00 | NA | 1.43 | 2.67 | 1.05 | 0.15 | 2.20 | NA | 1.13 | 1.60 |
| 10 | 0.90 | 2.30 | 7.00 | NA | 3.40 | 4.91 | 0.60 | 3.70 | 10.50 | NA | 4.93 | 6.38 |
| 11 | 0.04 | 2.70 | 0.00 | NA | 0.91 | 1.45 | 0.03 | 0.43 | 0.70 | NA | 0.38 | 1.07 |
| 12 | 1.30 | 0.00 | 0.00 | NA | 0.43 | 0.70 | 0.17 | 0.12 | 3.80 | NA | 1.36 | 3.84 |
| 13 | 0.18 | 2.85 | 0.58 | NA | 1.20 | 3.09 | 1.45 | 0.00 | 5.05 | NA | 2.17 | 8.14 |
| 14 | 0.48 | 0.00 | 3.55 | NA | 1.34 | 2.70 | 4.85 | 4.80 | 0.23 | NA | 3.29 | 10.57 |
| 15 | 0.00 | 0.11 | 2.05 | NA | 0.72 | 1.42 | 0.03 | 0.53 | 0.31 | NA | 0.29 | 0.46 |
| 16 | 1.65 | 0.16 | 0.83 | NA | 0.88 | 1.66 | 2.60 | 0.95 | 0.20 | NA | 1.25 | 1.91 |
| 17 | 2.75 | 1.85 | 5.23 | NA | 3.28 | 4.43 | 3.60 | 1.80 | 0.00 | NA | 1.80 | 3.31 |
| 18 | 0.05 | 2.55 | 0.00 | 0.44 | 0.76 | 1.04 | 2.05 | 0.00 | 0.29 | 0.00 | 0.58 | 1.50 |
| 19 | 15.23 | 0.10 | 5.38 | 1.29 | 5.50 | 67.09 | 41.45 | 36.85 | 0.74 | 0.18 | 19.81 | 201.44 |
| 20 | 25.05 | 0.85 | 0.90 | 19.70 | 11.63 | 30.00 | 36.35 | 0.00 | 0.01 | 23.50 | 14.96 | 47.54 |
| 21 | 4.25 | 43.85 | 0.00 | 0.63 | 12.18 | 39.72 | 0.03 | 24.95 | 1.15 | 0.14 | 6.57 | 20.30 |
| 22 | 8.38 | 0.02 | 1.70 | 1.75 | 2.96 | 6.49 | 13.78 | 0.56 | 2.75 | 1.05 | 4.53 | 10.43 |
| 23 | 3.65 | 0.01 | 1.30 | NA | 1.65 | 3.30 | 0.01 | 0.80 | 2.70 | NA | 1.17 | 3.02 |
| 24 | 21.95 | 4.30 | 13.20 | NA | 13.15 | 62.15 | 2.45 | 0.02 | 1.25 | NA | 1.24 | 2.36 |
| 25 | 4.60 | 1.70 | 0.88 | NA | 2.39 | 5.37 | 2.10 | 0.00 | 0.01 | NA | 0.70 | 1.40 |
| 26 | 2.75 | 0.65 | 0.07 | NA | 1.16 | 1.32 | 0.93 | 0.04 | 1.50 | NA | 0.82 | 2.17 |
| 27 | 0.02 | 0.46 | 0.53 | NA | 0.34 | 0.54 | 0.40 | 0.00 | 0.02 | NA | 0.14 | 0.36 |
| 28 | 0.19 | 0.00 | 0.85 | NA | 0.35 | 0.64 | 1.70 | 1.60 | 0.00 | NA | 1.10 | 2.17 |
| 29 | 1.45 | 1.60 | 0.63 | NA | 1.23 | 2.79 | 41.00 | 1.45 | 7.35 | NA | 16.60 | 46.00 |
| 30 | 2.80 | 0.09 | 0.85 | NA | 1.25 | 1.67 | 0.04 | 0.68 | 4.50 | NA | 1.74 | 3.40 |
| 31 | 0.20 | 0.00 | 0.80 | NA | 0.33 | 0.55 | 2.30 | 0.01 | 2.85 | NA | 1.72 | 4.92 |
| 32 | 12.35 | 57.70 | 18.40 | NA | 29.48 | 287.89 | 3.00 | 0.74 | 0.60 | NA | 1.45 | 3.94 |


2.3. Selectivity in Scaffold Ranking and Positional Scanning

3. Conclusions
Supplementary Materials
Acknowledgments
Conflicts of Interest
References
- Houghten, R.A.; Pinilla, C.; Appel, J.R.; Blondelle, S.E.; Dooley, C.T.; Eichler, J.; Nefzi, A.; Ostresh, J.M. Mixture-based synthetic combinatorial libraries. J. Med. Chem. 1999, 42, 3743–3778. [Google Scholar] [CrossRef]
- Pinilla, C.; Appel, J.R.; Borras, E.; Houghten, R.A. Advances in the use of synthetic combinatorial chemistry: Mixture-based libraries. Nat. Med. 2003, 9, 118–122. [Google Scholar] [CrossRef]
- Houghten, R.A.; Pinilla, C.; Giulianotti, M.A.; Appel, J.R.; Dooley, C.T.; Nefzi, A.; Ostresh, J.M.; Yu, Y.; Maggiora, G.M.; Medina-Franco, J.L.; et al. Strategies for the use of mixture-based synthetic combinatorial libraries: Scaffold ranking, Direct testing in vivo, and enhanced deconvolution by computational methods. J. Comb. Chem. 2008, 10, 3–19. [Google Scholar] [CrossRef]
- Pinilla, C.; Appel, J.R.; Blanc, P.; Houghten, R.A. Rapid identification of high affinity peptide ligands using positional scanning synthetic peptide combinatorial libraries. Biotechniques 1992, 13, 901–905. [Google Scholar]
- Dooley, C.T.; Houghten, R.A. The use of positional scanning synthetic peptide combinatorial libraries for the rapid determination of opioid receptor ligands. Life Sci. 1993, 52, 1509–1517. [Google Scholar] [CrossRef]
- Santos, R.G.; Giulianotti, M.A.; Dooley, C.T.; Pinilla, C.; Appel, J.R.; Houghten, R.A. Use and implications of the harmonic mean model on mixtures for basic research and drug discovery. ACS Comb. Sci. 2011, 13, 337–344. [Google Scholar] [CrossRef]
- Giulianotti, M.A.; Debevec, G.; Santos, R.G.; Maida, L.E.; Chen, W.; Ou, L.; Yu, Y.; Dooley, C.T.; Houghten, R.A. A novel method for the determination of isokinetic ratios and its application in the synthesis of two new positional scanning libraries. ACS Comb. Sci. 2012, 14, 503–512. [Google Scholar] [CrossRef]
- Ranjit, D.K.; Rideout, M.C.; Nefzi, A.; Ostresh, J.M.; Pinilla, C.; Segall, A.M. Small molecule functional analogs of peptides that inhibit lambda site-specific recombination and bind Holliday junctions. Bioorg. Med. Chem. Lett. 2010, 20, 4531–4534. [Google Scholar] [CrossRef]
- Rideout, M.C.; Boldt, J.L.; Vahi-Ferguson, G.; Salamon, P.; Nefzi, A.; Ostresh, J.M.; Giulianotti, M.; Pinilla, C.; Segall, A.M. Potent antimicrobial small molecules screened as inhibitors of tyrosine recombinases and Holliday junction-resolving enzymes. Mol. Divers. 2011, 15, 989–1005. [Google Scholar] [CrossRef]
- Reilley, K.J.; Giulianotti, M.; Dooley, C.T.; Nefzi, A.; McLaughlin, J.P.; Houghten, R.A. Identification of two novel, potent, low-liability antinociceptive compounds from the direct in vivo screening of a large mixture-based combinatorial library. AAPS J. 2010, 12, 318–329. [Google Scholar] [CrossRef]
- Minond, D.; Cudic, M.; Bionda, N.; Giulianotti, M.; Maida, L.; Houghten, R.A.; Fields, G.B. Discovery of novel inhibitors of a disintegrin and metalloprotease 17 (ADAM17) using glycosylated and non-glycosylated substrates. J. Biol. Chem. 2012, 287, 36473–36487. [Google Scholar]
- Zhou, Y.; Bian, X.; Le, Y.; Gong, W.; Hu, J.; Zhang, X.; Wang, L.; Iribarren, P.; Salcedo, R.; Howard, O.M.; et al. Formylpeptide receptor FPR and the rapid growth of malignant human gliomas. J. Natl. Cancer. Inst. 2005, 97, 823–835. [Google Scholar] [CrossRef]
- Le, Y.; Yazawa, H.; Gong, W.; Yu, Z.; Ferrans, V.J.; Murphy, P.M.; Wang, J.M. The neurotoxic prion peptide fragment PrP(106-126) is a chemotactic agonist for the G protein-coupled receptor formyl peptide receptor-like 1. J. Immunol. 2001, 166, 1448–1451. [Google Scholar]
- Pinilla, C.; Edwards, B.S.; Appel, J.R.; Yates-Gibbins, T.; Giulianotti, M.; Medina-Franco, J.L.; Young, S.M.; Santos, R.G.; Sklar, L.A.; Houghten, R.A. Selective agonist and antagonist of formylpeptide receptors:duplex flow cytometry and mixture-based positional scanning libraries. Mol. Pharmacol. 2013. submitted. [Google Scholar]
- Medina-Franco, J.L.; Pinilla, C.; Appel, J.R.; Giulianotti, M.; Santos, R.G.; Yongye, A.B.; Edwards, B.S.; Sklar, L.A.; Houghten, R.A. Scanning structure-activity relantionships in combiatorial data sets: Rapid identification of activity-switches. J. Chem. Inf. Model. 2013. accepted. [Google Scholar]
- Sample Availability: All of the libraries are made available to the scientific community through various research agreements (http://www.tpims.org).
© 2013 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/3.0/).
Share and Cite
Santos, R.G.; Appel, J.R.; Giulianotti, M.A.; Edwards, B.S.; Sklar, L.A.; Houghten, R.A.; Pinilla, C. The Mathematics of a Successful Deconvolution: A Quantitative Assessment of Mixture-Based Combinatorial Libraries Screened Against Two Formylpeptide Receptors. Molecules 2013, 18, 6408-6424. https://doi.org/10.3390/molecules18066408
Santos RG, Appel JR, Giulianotti MA, Edwards BS, Sklar LA, Houghten RA, Pinilla C. The Mathematics of a Successful Deconvolution: A Quantitative Assessment of Mixture-Based Combinatorial Libraries Screened Against Two Formylpeptide Receptors. Molecules. 2013; 18(6):6408-6424. https://doi.org/10.3390/molecules18066408
Chicago/Turabian StyleSantos, Radleigh G., Jon R. Appel, Marc A. Giulianotti, Bruce S. Edwards, Larry A. Sklar, Richard A. Houghten, and Clemencia Pinilla. 2013. "The Mathematics of a Successful Deconvolution: A Quantitative Assessment of Mixture-Based Combinatorial Libraries Screened Against Two Formylpeptide Receptors" Molecules 18, no. 6: 6408-6424. https://doi.org/10.3390/molecules18066408
APA StyleSantos, R. G., Appel, J. R., Giulianotti, M. A., Edwards, B. S., Sklar, L. A., Houghten, R. A., & Pinilla, C. (2013). The Mathematics of a Successful Deconvolution: A Quantitative Assessment of Mixture-Based Combinatorial Libraries Screened Against Two Formylpeptide Receptors. Molecules, 18(6), 6408-6424. https://doi.org/10.3390/molecules18066408




