2.1. Weight Reduction
Weight reduction (WR) denotes the net difference in weight between the initial weight of the sample and the weight of the dehydrated fruit based on the initial sample weight [
16].
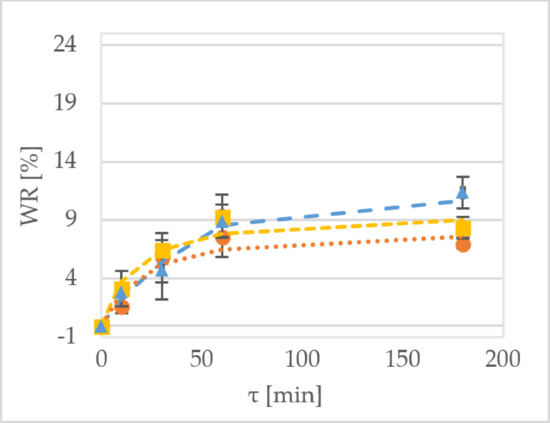
Figure 1 shows the WR of the samples during the OD of kiwiberry tissue. After 1 h of processing the ‘Geneva’ cultivar at 30 °C, the WR values were equal to 7.5%, 9.4% and 8.9% for the samples treated by sucrose, maltitol and xylitol, respectively (
Figure 1a). In comparison, the values obtained for the dehydration of the ‘Weiki’ cultivar were equal to 5.5%, 2.6% and 5.7% for the samples treated by sucrose, maltitol and xylitol, respectively (
Figure 1b). For the same process performed at 50 °C, the values obtained for the ‘Geneva’ cultivar were equal to 12.8%, 11.0% and 14.7% for sucrose, maltitol and xylitol, respectively (
Figure 1b). For the ‘Weiki’ cultivar, the WR values were 6.5%, 8.9% and 13.6% for the samples treated by sucrose, maltitol and xylitol, respectively (
Figure 1d). The ‘Geneva’ cultivar seemed to be more susceptible to OD, so it had higher WR values, which probably resulted from the thinner skin of the ‘Geneva’ fruits, whereas the ‘Weiki’ fruits have a higher endurance against environmental factors. In the case of this experiment, their resistance against mass transfer, which was represented by lower WR values, was diminished after the temperature was raised. The 50 °C tested samples showed similar and comparable results despite using different osmotic solutions (
Figure 1c,d). After 180 min of the process carried out at 30 °C, the ‘Geneva’ kiwiberry fruits reached the WR values of 7.0% and 11.3% for sucrose and xylitol, respectively (
Figure 1a). The same solutions at 50 °C provided WR values of 20% to 22% for sucrose and xylitol, respectively (
Figure 1b). For the ‘Weiki’ cultivar, after 60 min of OD at 30 °C, WR values were 4.7% for sucrose and 8.7% for xylitol, respectively (
Figure 1c). Applying high temperature resulted in a maximum WR value of 17.0% for maltitol and 16.8% for xylitol, respectively (
Figure 1d).
During the experiment, there was a noticeable slowing down of WR kinetics. Water leaving the tissue lowered osmotic pressure and influenced further processing. Occurrence of this phenomena could be diminished by increasing the ratio of solution to sample mass, which was used in the experiment performed by Kowalski and Mierzwa [
3] and Tiroutchelvame et al. [
17]. In the study by Arballo et al. [
18], researchers suggested that the optimum value for dehydrated cubes of fruits (pumpkin, kiwi, pear) resides between 112 min and 240 min of processing. For the kiwi cubes [
18], established by Arballo et al., the WR value equal to 27.8% was obtained when they were in 60% solute of sucrose for 145 min at 30 °C. Comparable observations can be made for kiwiberry halves at 30 °C. In addition, temperature strongly influenced obtained values, which was also confirmed by Alam et al. [
19], Yadav et al. [
20] and Cichowska et al. [
21]. During OD of anola slices researched by Alam et al. [
19] at temperatures between 30 °C and 60 °C, the optimum temperature was established at 51 °C.
In this experiment, xylitol was determined as the most effective osmotic reagent for reaching high WR values (
Figure 1). High efficiency of this polyol was confirmed by Mendonça et al. [
9,
18] and Cichowska et al. [
21]. Both authors agree that it is the result of xylitol’s low molecular weight. It is also worth noticing that all the investigated parameters of the OD had significant impacts on the WR values (
Table 1). Statistical analysis has also shown interactions between the tested variables (
Table 2); a significant for interaction between temperature–time–solution (
p < 0.05) and a significant for temperature–time (
p < 0.01) were identified. According to some researchers, WR is mostly influenced by the concentration of the osmotic solution and the temperature [
22]. The experiment performed on the kiwifruit slices by Cao et al. [
23], showed that the temperature of the process lessened the influence of the other factors or their combinations.
One of the aims of this study was to determine the best fitting model for OD kinetics. Two popular equations were used and modelled, i.e., Peleg’s [
24] and Ade-Omowaye et al. [
25]. The results of the statistical evaluation of experimental regression analysis for the WR are presented in
Table 3.
CRV values lower than 20% indicate usefulness of the tested equation for the prediction of the process [
26]. Mathematical modelling has shown higher usefulness of the Peleg’s model for the WR prediction. It was the only model that could calculate the expected values for process at 30 °C, although the resulting
CRV values were too high for practical use. On the other hand, fitting values for 50 °C have given high
R2 values, and low
CRV,
RMSE and
χ2 values (
Table 3). The Peleg’s equation has two independent variables representing initial mass transfer rate (
K1) and equilibrium moisture content (
K2). The
K1 and
K2 values for the 50 °C process indicated both high transfer and water removal rates (
Figure 2a,c). The
K1 values decreased with the increase of the temperature. There was no visible trend for the
K2 values. The usefulness of the Peleg’s model was also confirmed by Arballo et al. [
18] when researchers were using this model for predicting the OD of different fruits.
2.2. Water Loss
Water loss (WL) is a parameter that indicates amount of water removed during pre-treatment in relation to initial sample mass [
16].
Figure 1 shows water loss of samples during the OD of kiwiberry tissue. In most cases, the WL value for samples processed with xylitol, was higher when compared to the other osmotic solutions. For instance, after 10 min of immersion, the WR value at 30 °C of the ‘Geneva’ cultivar was 0.14 g/g initial dry matter (i.d.m.) and 0.25 g/g i.d.m. for sucrose and maltitol, respectively (
Figure 2a). In turn, for the ‘Weiki’ cultivar, the WL reached values of 0.33 g/g i.d.m. for sucrose and 0.37 g/g i.d.m. for xylitol, respectively (
Figure 2c).
After 60 min of the process, the highest WL values were obtained for the samples dehydrated at 50 °C, reaching values between 1.06 g/g i.d.m. for the ‘Geneva’ cultivar (
Figure 2b), and 0.87 g/g i.d.m. for the ‘Weiki’ cultivar (
Figure 2d), both dehydrated in xylitol. In the case of the 30 °C treatment in xylitol, the highest WL values were obtained equal to 0.53 g/g i.d.m. for the ‘Geneva’ cultivar (
Figure 2a) and 0.64 g/g i.d.m. for the ‘Weiki’ cultivar (
Figure 2c). Prolonging the process up to 180 min allowed further water removal. During this experiment, the highest WL values for both the ‘Geneva’ and ‘Weiki’ cultivars, were obtained from the samples processed with xylitol after 180 min of the OD at 50 °C, which reached up to 1.5 g/g i.d.m. (
Figure 2b,d). The lowest experimental values of the WL were achieved for materials kept at 30 °C in sucrose, which were 0.48 g/g i.d.m. and 0.57 g/g i.d.m. for the ‘Geneva’ and ‘Weiki’ cultivars, respectively (
Figure 2a,c).
The established WL values suggest that 60 min OD of kiwiberry at 30 °C allows to achieve results similar to 30 min immersion at 50 °C. As expected, the maximum WL values were reached in the case of the longest processing time (180 min) performed at the highest temperature (50 °C). Such high temperature, although it is beneficial for OD kinetics, can influence biochemical properties of the material [
27]. Statistical analysis showed significant differences in applied temperatures, osmotic solutions and process durations. There was no significant difference between the tested cultivars (
Table 4). There were a few significant (
p < 0.05) interactions between the tested variables (
Table 2) for cultivar–time, temperature–time–solution, and very strong interaction (
p < 0.01) for variables of temperature–time and time–solution.
Difference in osmotic pressure between solution and tissue is the driving force of the dehydration. Calculated ideal osmotic pressures, based on the equation given by Held et al. [
7] with the used solutes at 30 °C, were 4418.13 kPa, 4392.33 kPa and 9939.70 kPa for sucrose, maltitol and xylitol, respectively. At 50 °C, the established osmotic pressures were 4709.61 kPa, 4682.12 kPa and 10595.46 kPa for sucrose, maltitol and xylitol, respectively. During the process, water migrates to the solution, which lowers osmotic agents density and as a result, slows down OD [
1,
28]. High initial WL ratio after the first 2 h of OD, was also observed by Panagiotou et al
. [
29], who were dehydrating apples, bananas and kiwis using 40% sucrose at 40 °C. Similar observations were also made by Arballo et al. [
18], where scientists dehydrated pumpkins, kiwis and pears. They have noticed that the time of the process influences the outcome of the dehydration during the first 4 h of the process. In this experiment, similar observations can be made for samples immersed in samples at 30 °C. For kiwiberry treated at 50 °C, it should be taken into consideration that lengthening the process up to at least 4 h establishes plateau values. With the increased temperature, the viscosity of the osmotic reagent decreases, and as a result, the mass transfer improves [
20]. The influence of temperature on OD was confirmed by Ciurzyńska et al
. [
30], in the OD of apples at temperatures of 40 °C and 60 °C. In this experiment, xylitol was determined as the most effective osmotic reagent (
Figure 2). It is also worth noticing that difference in dehydration effectiveness between sucrose and maltitol turned out to be statistically insignificant (
Table 4). This was caused by the molecular weight of the solutes used. Lower molecular weight yields higher osmotic pressure (maltitol 344.31 g/mol, sucrose 342.3 g/mol and xylitol 152.15 g/mol).
The Peleg’s modelling of the WL was effective in almost all of the tested combinations of the used variables (
Table 5). Goodness of fit for this model has given high
R2 values, and low
CRV,
RMSE and
χ2, which is expected for applicable models. High goodness of fit of this model for the OD was also reported by Yadav and Singh [
1] and Cichowska et al
. [
21].
The Peleg’s equation has two independent variables, where
K1 represents initial mass transfer rate and
K2 describes equilibrium moisture content. As it was aforementioned, low
K1 and
K2 values indicate both high mass transfer and high water removal rates [
21,
31]. Measured WL values suggest lower effectiveness of sucrose and maltitol, in comparison to xylitol carried out at 30 °C (
Figure 1a,c). Statistical analysis has shown that these two osmotic agents belong to the same homogeneous group (
Table 3). This could be explained by similar and high molecular weight of these solutions; such observations were also reported by Mendonça et al
. [
11]. Molecular weight could have also influenced the
K1 values for the OD at 30 °C; such phenomena were also reported by other researchers [
28]. In addition, it could be explained by similar osmotic coefficient values, and should be further explored in the future experiments.
2.3. Solid Gain
Solid gain (SG) is a parameter which indicates amount of soluble solids that are incorporated into the sample during dehydration [
16].
Figure 3 shows SG of samples during the OD of kiwiberry halves. After 60 min OD of the ‘Geneva’ cultivar at 30 °C, the SG value were 0.08 g/g i.d.m., 0.07 g/g i.d.m. and 0.09 g/g i.d.m. for samples treated by sucrose, maltitol and xylitol, respectively (
Figure 3a). The ‘Weiki’ samples were more prone to display higher SG values, which were 0.31 g/g i.d.m., 0.32 g/g i.d.m. and 0.34 g/g i.d.m. for sucrose, maltitol and xylitol, respectively (
Figure 3c). When the process was performed at 50 °C, the SG values obtained for the ‘Geneva’ cultivar were 0.14 g/g i.d.m., 0.19 g/g i.d.m. and 0.3 g/g i.d.m. for sucrose, maltitol and xylitol, respectively (
Figure 3b). For the ‘Weiki’ cultivar treated at 50 °C, the SG values were 0.43 g/g i.d.m., 0.41 g/g i.d.m. and 0.31 g/g i.d.m. for sucrose, maltitol and xylitol, respectively (
Figure 3d). In both temperatures, the ‘Geneva’ cultivar seemed to be more efficient in the SG. As it was mentioned earlier, the dehydration at 50 °C displays the similar effectiveness despite using different osmotic solutions. On the other hand, the 30 °C processing shows larger SG differences between the used solutes. Comparable results for the 60 min dehydration at 50 °C were achieved after 180 min of the OD at 30 °C. Similar observations were also made for shorter processing time. For instance, after 10 min of the immersion at 30 °C, the SG values were similar to the 60 min process at 30 °C.
During the experiment performed by Chiu et al. [
32] and Lee et al
. [
33], researchers noticed that SG values are strongly influenced by temperature during the OD of Terung Asam and red algae, respectively. This was also the case during kiwiberry dehydration. The SG values after 180 min dehydration were 25–50% higher when the temperature was raised by 20 °C. According to Lazarides et al. [
34], higher temperature promotes faster water loss by plasticizing of cell membranes, increasing water diffusion and lowering viscosity of the osmotic medium. The difference in the SG ratios between the tested cultivars can be explained by the difference in strengths of cell membranes. SG is strongly influenced by ratios of solute to mass [
17,
32]. Although some researchers suggest the increase of this ratio [
3], results of different studies focused on effectiveness and quality of final product [
17] claim that a ratio of 5:1 is the optimized value from industrial and scientific perspectives. In this experiment, a ratio of 4:1 was used, which is a well-established experimental standard. Statistical analysis had shown that all the tested parameters had a statistically significant impact on the SG. However, it is worth mentioning that there was no statistical difference between 10 min and 30 min dehydration and between maltitol and sucrose (
Table 6).
There were a few significant (
p < 0.05) interactions between the tested variables (
Table 2) for cultivar-solution and very strong interaction (
p < 0.01) for cultivar-time, temperature-time, time-solution, cultivar-temperature-solution. In comparison to the other variables (WR and WL), SG interactions between variables were more often strongly influenced by osmotic solution.
The Peleg’s mathematical model has shown its high usefulness for the SG prediction (
Table 7). The
CRV values for 30 °C were often exceeding the critical value (20%), but they gives high goodness of fit at 50 °C OD. In most cases, fitting values for 50 °C have provided high
R2 values, and low
CRV,
RMSE and
χ2 values. The usefulness of this model was also confirmed by Arballo et al. [
15] and Cichowska et al. [
21]. No visible trend was established for the K
1 values of SG for the “Geneva” cultivar. For the “Weiki” cultivar, there was a noticeable reduction in the K
1 value when the temperature was increased. The impact of the applied temperature on K
1 values was also reported by Ganjloo et al. [
31]. This requires further analysis in future experiments.