Understanding the Molecule-Electrode Interface for Molecular Spintronic Devices: A Computational and Experimental Study
Abstract
:1. Introduction
2. Experimental
2.1. C28H20N2O2 (SYMLH2)
2.2. [(C28H18N2O2)3Dy2H2O] (1)
2.3. Iron Oxide Nanoparticles (IO-NPs)
2.4. Decoration of Iron Oxide Nanoparticles (NP-1)
2.5. Characterization
2.6. Computational Methods
3. Results and Discussion
3.1. Synthesis and Characterization
3.2. Magnetic Properties
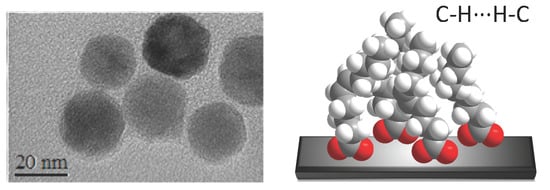
3.3. Nanostructuration of SYML-Dy2 on Iron Oxide Nanoparticles
3.4. Decoration of Nanoparticles
3.5. Magnetic Properties
3.6. Theoretical Study
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Sessoli, R.; Tsai, H.L.; Schake, A.R.; Wang, S.; Vincent, J.B.; Folting, K.; Gatteschi, D.; Christou, G.; Hendrickson, D.N. High-spin molecules: [Mn12O12(O2CR)16(H2O)4]. J. Am. Chem. Soc. 1993, 115, 1804–1816. [Google Scholar] [CrossRef]
- Bertaina, S.; Gambarelli, S.; Mitra, T.; Tsukerblat, B.; Müller, A.; Barbara, B. Quantum oscillations in a molecular magnet. Nature 2008, 453, 203–206. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Leuenberger, M.N.; Loss, D. Quantum computing in molecular magnets. Nature 2001, 410, 789–793. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Woodruff, D.N.; Winpenny, R.E.P.; Layfield, R.A. Lanthanide Single-Molecule Magnets. Chem. Rev. 2013, 113, 5110–5148. [Google Scholar] [CrossRef] [PubMed]
- Rinehart, J.D.; Long, J.R. Exploiting single-ion anisotropy in the design of f-element single-molecule magnets. Chem. Sci. 2011, 2, 2078–2085. [Google Scholar] [CrossRef]
- Gholizadeh Dogaheh, S.; Khanmohammadi, H.; Sanudo, E.C. Double-decker luminescent ytterbium and erbium SMMs with symmetric and asymmetric Schiff base ligands. New J. Chem. 2017, 41, 10101–10111. [Google Scholar] [CrossRef]
- Baibich, M.N.; Broto, J.M.; Fert, A.; Van Dau, F.N.; Petroff, F.; Etienne, P.; Creuzet, G.; Friederich, A.; Chazelas, J. Giant Magnetoresistance of (001)Fe/(001)Cr Magnetic Superlattices. Phys. Rev. Lett. 1988, 61, 2472–2475. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Binasch, G.; Grünberg, P.; Saurenbach, F.; Zinn, W. Enhanced magnetoresistance in layered magnetic structures with antiferromagnetic interlayer exchange. Phys. Rev. B 1989, 39, 4828–4830. [Google Scholar] [CrossRef]
- Tyagi, P. Multilayer edge molecular electronics devices: A review. J. Mater. Chem. 2011, 21, 4733–4742. [Google Scholar] [CrossRef]
- Rosado Piquer, L.; Sanudo, E.C. Heterometallic 3d-4f single-molecule magnets. Dalton Trans. 2015, 44, 8771–8780. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Deb, A.; Boron, T.T.; Itou, M.; Sakurai, Y.; Mallah, T.; Pecoraro, V.L.; Penner-Hahn, J.E. Understanding Spin Structure in Metallacrown Single-Molecule Magnets using Magnetic Compton Scattering. J. Am. Chem. Soc. 2014, 136, 4889–4892. [Google Scholar] [CrossRef] [PubMed]
- Bronstein, L.M.; Huang, X.; Retrum, J.; Schmucker, A.; Pink, M.; Stein, B.D.; Dragnea, B. Influence of Iron Oleate Complex Structure on Iron Oxide Nanoparticle Formation. Chem. Mater. 2007, 19, 3624–3632. [Google Scholar] [CrossRef]
- Donati, F.; Rusponi, S.; Stepanow, S.; Wäckerlin, C.; Singha, A.; Persichetti, L.; Baltic, R.; Diller, K.; Patthey, F.; Fernandes, E.; et al. Magnetic remanence in single atoms. Science 2016, 352, 318–321. [Google Scholar] [CrossRef] [PubMed]
- Wäckerlin, C.; Donati, F.; Singha, A.; Baltic, R.; Rusponi, S.; Diller, K.; Patthey, F.; Pivetta, M.; Lan, Y.; Klyatskaya, S.; et al. Single-Molecule Magnets: Giant Hysteresis of Single-Molecule Magnets Adsorbed on a Nonmagnetic Insulator. Adv. Mater. 2016, 28, 5142. [Google Scholar] [CrossRef] [PubMed]
- Prado, Y.; Daffé, N.; Michel, A.; Georgelin, T.; Yaacoub, N.; Grenèche, J.-M.; Choueikani, F.; Otero, E.; Ohresser, P.; Arrio, M.-A.; et al. Enhancing the magnetic anisotropy of maghemite nanoparticles via the surface coordination of molecular complexes. Nat. Commun. 2015, 6, 10139. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Grimme, S. Density functional theory with London dispersion corrections. WIREs Comput. Mol. Sci. 2011, 1, 211–228. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Gaussian 09, Revision D.01; Gaussian, Inc.: Wallingford, CT, USA, 2009. [Google Scholar]
- Stephens, P.J.; Devlin, F.J.; Chabalowski, C.F.; Frisch, M.J. Ab Initio Calculation of Vibrational Absorption and Circular Dichroism Spectra Using Density Functional Force Fields. J. Phys. Chem. 1994, 98, 11623–11627. [Google Scholar] [CrossRef]
- Ditchfield, R.; Hehre, W.J.; Pople, J.A. Self-Consistent Molecular-Orbital Methods. IX. An Extended Gaussian-Type Basis for Molecular-Orbital Studies of Organic Molecules. J. Chem. Phys. 1971, 54, 724–728. [Google Scholar] [CrossRef]
- Chai, J.-D.; Head-Gordon, M. Systematic optimization of long-range corrected hybrid density functionals. J. Chem. Phys. 2008, 128, 084106. [Google Scholar] [CrossRef] [PubMed]
- Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef] [PubMed]
- Boys, S.F.; Bernardi, F. The calculation of small molecular interactions by the differences of separate total energies. Some procedures with reduced errors. Mol. Phys. 1970, 19, 553–566. [Google Scholar] [CrossRef]
- Popović, Z.; Roje, V.; Pavlović, G.; Matković-Čalogović, D.; Giester, G. The first example of coexistence of the ketoamino–enolimino forms of diamine Schiff base naphthaldimine parts: the crystal and molecular structure of N,N′-bis(1-naphthaldimine)-o-phenylenediamine chloroform (1/1) solvate at 200 K. J. Mol. Struct. 2001, 597, 39–47. [Google Scholar] [CrossRef]
- Hardy, E.E.; Wyss, K.M.; Keller, R.J.; Gorden, J.D.; Gorden, A.E.V. Tunable ligand emission of napthylsalophen triple-decker dinuclear lanthanide(iii) sandwich complexes. Dalton Trans. 2018, 47, 1337–1346. [Google Scholar] [CrossRef] [PubMed]
- Ishikawa, N.; Sugita, M.; Ishikawa, T.; Koshihara, S.-y.; Kaizu, Y. Lanthanide Double-Decker Complexes Functioning as Magnets at the Single-Molecular Level. J. Am. Chem. Soc. 2003, 125, 8694–8695. [Google Scholar] [CrossRef] [PubMed]
- Benelli, C.; Gatteschi, D. Magnetism of Lanthanides in Molecular Materials with Transition-Metal Ions and Organic Radicals. Chem. Rev. 2002, 102, 2369–2388. [Google Scholar] [CrossRef] [PubMed]
- Miao, Y.-L.; Liu, J.-L.; Leng, J.-D.; Lin, Z.-J.; Tong, M.-L. Chloride templated formation of {Dy12(OH)16}20+ cluster core incorporating 1,10-phenanthroline-2,9-dicarboxylate. CrystEngComm 2011, 13, 3345–3348. [Google Scholar] [CrossRef]
- Campbell, V.E.; Tonelli, M.; Cimatti, I.; Moussy, J.-B.; Tortech, L.; Dappe, Y.J.; Rivière, E.; Guillot, R.; Delprat, S.; Mattana, R.; et al. Engineering the magnetic coupling and anisotropy at the molecule–magnetic surface interface in molecular spintronic devices. Nat. Commun. 2016, 7, 13646. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rosado Piquer, L.; Jimenez Romero, E.; Lan, Y.; Wernsdorfer, W.; Aromi, G.; Sanudo, E.C. Hybrid molecular-inorganic materials: A heterometallic [Ni4Tb] complex grafted on superparamagnetic iron oxide nanoparticles. Inorg. Chem. Front. 2017, 4, 595–603. [Google Scholar] [CrossRef]
- Goya, G.F.; Berquó, T.S.; Fonseca, F.C.; Morales, M.P. Static and dynamic magnetic properties of spherical magnetite nanoparticles. J. Appl. Phys. 2003, 94, 3520–3528. [Google Scholar] [CrossRef]
- Guardia, P.; Batlle-Brugal, B.; Roca, A.G.; Iglesias, O.; Morales, M.P.; Serna, C.J.; Labarta, A.; Batlle, X. Surfactant effects in magnetite nanoparticles of controlled size. J. Magn. Magn. Mater. 2007, 316, e756–e759. [Google Scholar] [CrossRef]
- Friedman, J.R.; Sarachik, M.P.; Tejada, J.; Ziolo, R. Macroscopic Measurement of Resonant Magnetization Tunneling in High-Spin Molecules. Phys. Rev. Lett. 1996, 76, 3830–3833. [Google Scholar] [CrossRef] [PubMed]
- Lodi Rizzini, A.; Krull, C.; Balashov, T.; Kavich, J.J.; Mugarza, A.; Miedema, P.S.; Thakur, P.K.; Sessi, V.; Klyatskaya, S.; Ruben, M.; et al. Coupling Single Molecule Magnets to Ferromagnetic Substrates. Phys. Rev. Lett. 2011, 107, 177205. [Google Scholar] [CrossRef] [PubMed]
- Estrader, M.; López-Ortega, A.; Estradé, S.; Golosovsky, I.V.; Salazar-Alvarez, G.; Vasilakaki, M.; Trohidou, K.N.; Varela, M.; Stanley, D.C.; Sinko, M.; et al. Robust antiferromagnetic coupling in hard-soft bi-magnetic core/shell nanoparticles. Nat. Commun. 2013, 4, 2960. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Moya, C.; Iglesias, O.; Batlle, X.; Labarta, A. Quantification of Dipolar Interactions in Fe3−XO4 Nanoparticles. J. Phys. Chem. C 2015, 119, 24142–24148. [Google Scholar] [CrossRef]
- Echeverría, J.; Aullón, G.; Danovich, D.; Shaik, S.; Alvarez, S. Dihydrogen contacts in alkanes are subtle but not faint. Nat. Chem. 2011, 3, 323–330. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Danovich, D.; Shaik, S.; Neese, F.; Echeverría, J.; Aullón, G.; Alvarez, S. Understanding the Nature of the CH···HC Interactions in Alkanes. J. Chem. Theory Comp. 2013, 9, 1977–1991. [Google Scholar] [CrossRef] [PubMed]
- Echeverría, J.; Aullón, G.; Alvarez, S. Dihydrogen intermolecular contacts in group 13 compounds: H···H or E···H (E = B, Al, Ga) interactions? Dalton Trans. 2017, 46, 2844–2854. [Google Scholar] [CrossRef] [PubMed]
- Echeverría, J.; Aullón, G.; Alvarez, S. Intermolecular interactions in group 14 hydrides: Beyond C-H···H-C contacts. Int. J. Quantum Chem. 2017, 117, e25432. [Google Scholar] [CrossRef]
- Echeverría, J. The silane-methane dimer revisited: more than a dispersion-bound system? Phys. Chem. Chem. Phys. 2017, 19, 32663–32669. [Google Scholar] [CrossRef] [PubMed]
- Echeverría, J. Abundance and Strength of M–H···H–C (M = Al, Ga, In) Dihydrogen Bonds. Cryst. Growth Des. 2017, 17, 2097–2103. [Google Scholar] [CrossRef]
- Wang, C.; Mo, Y.; Wagner, J.P.; Schreiner, P.R.; Jemmis, E.D.; Danovich, D.; Shaik, S. The Self-Association of Graphane Is Driven by London Dispersion and Enhanced Orbital Interactions. J. Chem. Theory Comput. 2015, 11, 1621–1630. [Google Scholar] [CrossRef] [PubMed]
- Burgess, J.A.J.; Malavolti, L.; Lanzilotto, V.; Mannini, M.; Yan, S.C.; Ninova, S.; Totti, F.; Rolf-Pissarczyk, S.; Cornia, A.; Sessoli, R.; et al. Magnetic fingerprint of individual Fe-4 molecular magnets under compression by a scanning tunnelling microscope. Nat. Commun. 2015, 6, 8216. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Malavolti, L.; Lanzilotto, V.; Ninova, S.; Poggini, L.; Cimatti, I.; Cortigiani, B.; Margheriti, L.; Chiappe, D.; Otero, E.; Sainctavit, P.; et al. Magnetic Bistability in a Submonolayer of Sublimated Fe-4 Single-Molecule Magnets. Nano Lett. 2015, 15, 535–541. [Google Scholar] [CrossRef] [PubMed]
- Caneschi, A.; Gatteschi, D.; Totti, F. Molecular magnets and surfaces: A promising marriage. A DFT insight. Coord. Chem. Rev. 2015, 289, 357–378. [Google Scholar] [CrossRef]
Sample Availability: Not available. |









| Compound | SYML-Dy2 |
|---|---|
| Crystal system | Triclinic |
| Space group | P-1 |
| a/Å | 10.5560(9) |
| b/Å | 18.0269(14) |
| c/Å | 18.4384(15) |
| 66.851(4) | |
| 73.702(4) | |
| 86.391(4) | |
| Volume/Å−3 | 3092.1(5) |
| Z | 2 |
| Final R | = 0.0271 wR2 = 0.1048 |
| T/K | 100 |
| Experimental (Å) | Simulation (Å) | Family of Planes |
|---|---|---|
| 2.646 | 2.648 | 0 1 3 |
| 2.097 | 2.031 | 3 2 2 |
| 1.559 | 1.555 | 2 3 4 |
| 1.522 | 1.529 | 1 2 5 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rosado Piquer, L.; Sánchez, R.R.; Sañudo, E.C.; Echeverría, J. Understanding the Molecule-Electrode Interface for Molecular Spintronic Devices: A Computational and Experimental Study. Molecules 2018, 23, 1441. https://doi.org/10.3390/molecules23061441
Rosado Piquer L, Sánchez RR, Sañudo EC, Echeverría J. Understanding the Molecule-Electrode Interface for Molecular Spintronic Devices: A Computational and Experimental Study. Molecules. 2018; 23(6):1441. https://doi.org/10.3390/molecules23061441
Chicago/Turabian StyleRosado Piquer, Lidia, Raquel Royo Sánchez, E. Carolina Sañudo, and Jorge Echeverría. 2018. "Understanding the Molecule-Electrode Interface for Molecular Spintronic Devices: A Computational and Experimental Study" Molecules 23, no. 6: 1441. https://doi.org/10.3390/molecules23061441
APA StyleRosado Piquer, L., Sánchez, R. R., Sañudo, E. C., & Echeverría, J. (2018). Understanding the Molecule-Electrode Interface for Molecular Spintronic Devices: A Computational and Experimental Study. Molecules, 23(6), 1441. https://doi.org/10.3390/molecules23061441