Review on Applications of 17O in Hydrological Cycle
Abstract
:1. Introduction
2. 17O Isotope Measurement in Water
2.1. 17O Definition and Isotopic Fractionation
2.2. 17O Measurement
2.2.1. Dual-Inlet Isotope Ratio Mass Spectrometry
2.2.2. Laser Absorption Spectroscopy
3. Use in Hydrological Studies
3.1. Low- and Mid-Latitude Hydrology
3.2. High-Latitude Hydrology
4. Summary and Future Direction
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Gat, J.R. Oxygen and hydrogen isotopes in the hydrologic cycle. Annu. Rev. Earth Planet. Sci. 1996, 24, 225–262. [Google Scholar] [CrossRef] [Green Version]
- Dansgaard, W. Stable isotopes in precipitation. Tellus 1964, 16, 436–468. [Google Scholar] [CrossRef]
- Kim, S.; Han, Y.; Do Hur, S.; Yoshimura, K.; Lee, J. Relating moisture transport to stablewater vapor isotopic variations of ambientwintertime along the western coast of Korea. Atmosphere (Basel) 2019, 10, 806. [Google Scholar] [CrossRef] [Green Version]
- Petit, J.R.; Jouzel, J.; Raynaud, D.; Barkov, N.I.; Barnola, J.-M.; Basile, I.; Bender, M.; Chappellaz, J.; Davisk, M.; Delaygue, G.; et al. Climate and atmospheric history of the past 420,000 years from the Vostok ice core, Antarctica The recent completion of drilling at Vostok station in East. Nature 1999, 399, 429–436. [Google Scholar] [CrossRef] [Green Version]
- Nyamgerel, Y.; Han, Y.; Kim, S.; Hong, S.B.; Lee, J.; Hur, S. Do Chronological characteristics for snow accumulation on Styx Glacier in northern Victoria Land, Antarctica. J. Glaciol. 2020, 66, 916–926. [Google Scholar] [CrossRef]
- Lee, J.; Worden, J.; Noone, D.; Bowman, K.; Eldering, A.; Legrande, A.; Li, J.L.F.; Schmidt, G.; Sodemann, H. Relating tropical ocean clouds to moist processes using water vapor isotope measurements. Atmos. Chem. Phys. 2011, 11, 741–752. [Google Scholar] [CrossRef] [Green Version]
- Craig, H. Isotopic Variations in Meteoric Waters. Science 1961, 133, 1702–1703. [Google Scholar] [CrossRef]
- Kern, Z.; Hatvani, I.G.; Czuppon, G.; Fórizs, I.; Erdélyi, D.; Kanduč, T.; Palcsu, L.; Vreča, P. Isotopic “altitude” and “continental” effects in modern precipitation across the Adriatic-Pannonian region. Water 2020, 12, 1791. [Google Scholar] [CrossRef]
- Kong, Y.; Wang, K.; Li, J.; Pang, Z. Stable isotopes of precipitation in China: A consideration of moisture sources. Water 2019, 11, 1239. [Google Scholar] [CrossRef] [Green Version]
- Bronić, I.K.; Barešić, J.; Borković, D.; Sironić, A.; Mikelić, I.L.; Vreča, P. Long-Term isotope records of precipitation in Zagreb, Croatia. Water 2020, 12, 226. [Google Scholar]
- Heydarizad, M.; Raeisi, E.; Sorí, R.; Gimeno, L. Developing meteoric water lines for Iran based on air masses and moisture sources. Water 2019, 11, 2359. [Google Scholar] [CrossRef] [Green Version]
- Lee, J.; Feng, X.; Faiia, A.M.; Posmentier, E.S.; Kirchner, J.W.; Osterhuber, R.; Taylor, S. Isotopic evolution of a seasonal snowcover and its melt by isotopic exchange between liquid water and ice. Chem. Geol. 2010, 270, 126–134. [Google Scholar] [CrossRef]
- Lee, J.; Feng, X.; Posmentier, E.S.; Faiia, A.M.; Taylor, S. Stable isotopic exchange rate constant between snow and liquid water. Chem. Geol. 2009, 260, 57–62. [Google Scholar] [CrossRef]
- Lee, J.; Feng, X.; Faiia, A.; Posmentier, E.; Osterhuber, R.; Kirchner, J. Isotopic evolution of snowmelt: A new model incorporating mobile and immobile water. Water Resour. Res. 2010, 46, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Kim, H.; Cho, S.H.; Lee, D.; Jung, Y.Y.; Kim, Y.H.; Koh, D.C.; Lee, J. Influence of pre-event water on streamflow in a granitic watershed using hydrograph separation. Environ. Earth Sci. 2017, 76. [Google Scholar] [CrossRef]
- Galewsky, J.; Steen-Larsen, H.C.; Field, R.D.; Worden, J.; Risi, C.; Schneider, M. Stable isotopes in atmospheric water vapor and applications to the hydrologic cycle. Rev. Geophys. 2016. [Google Scholar] [CrossRef]
- Stenni, B.; Scarchilli, C.; Masson-Delmotte, V.; Schlosser, E.; Ciardini, V.; Dreossi, G.; Grigioni, P.; Bonazza, M.; Cagnati, A.; Karlicek, D.; et al. Three-year monitoring of stable isotopes of precipitation at Concordia Station, East Antarctica. Cryosphere 2016, 10, 2415–2428. [Google Scholar] [CrossRef] [Green Version]
- Johnsen, S.J.; Dahl-Jensen, D.; Gundestrup, N.; Steffensen, J.P.; Clausen, H.B.; Miller, H.; Masson-Delmotte, V.; Sveinbjörnsdottir, A.E.; White, J. Oxygen isotope and palaeotemperature records from six Greenland ice-core stations: Camp Century, Dye-3, GRIP, GISP2, Renland and NorthGRIP. J. Quat. Sci. 2001. [Google Scholar] [CrossRef]
- Masson-Delmotte, V.; Hou, S.; Ekaykin, A.; Jouzel, J.; Aristarain, A.; Bernardo, R.T.; Bromwich, D.; Cattani, O.; Delmotte, M.M.; Falourd, S.; et al. A review of antarctic surface snow isotopic composition: Observations, atmospheric circulation, and isotopic modeling. J. Clim. 2008, 21, 3359–3387. [Google Scholar] [CrossRef] [Green Version]
- Goursaud, S.; Masson-Delmotte, V.; Favier, V.; Orsi, A.; Werner, M. Water stable isotopes spatio-temporal variability in Antarctica in 1960–2013: Observations and simulations from the ECHAM5-wiso atmospheric general circulation model. Clim. Past Discuss. 2017, 1957, 1–48. [Google Scholar] [CrossRef] [Green Version]
- Opel, T.; Meyer, H.; Wetterich, S.; Laepple, T.; Dereviagin, A.; Murton, J. Ice wedges as archives of winter paleoclimate: A review. Permafr. Periglac. Process. 2018, 29, 199–209. [Google Scholar] [CrossRef]
- Porter, T.J.; Opel, T. Recent advances in paleoclimatological studies of Arctic wedge- and pore-ice stable-water isotope records. Permafr. Periglac. Process. 2020, 31, 429–441. [Google Scholar] [CrossRef]
- Rosman, K.J.R.; Taylor, P.D.P. Isotopic compositions of the elements 1997. J. Phys. Chem. Ref. Data 1998, 27, 1275–1287. [Google Scholar] [CrossRef] [Green Version]
- Giauque, W.F.; Johnston, H.L. An isotope of oxygen, mass 17, in the Earth’s atmosphere. J. Am. Chem. Soc. 1929, 51, 3528–3534. [Google Scholar] [CrossRef]
- Masson-Delmotte, V.; Dreyfus, G.; Braconnot, P.; Johnsen, S.; Jouzel, J.; Kageyama, M.; Landais, A.; Loutre, M.-F.; Nouet, J.; Parrenin, F.; et al. Past temperature reconstructions from deep ice cores: Relevance for future climate change. Clim. Past Discuss. 2006, 2, 399–448. [Google Scholar]
- Luz, B.; Barkan, E. Variations of 17O/16O and 18O/16O in meteoric waters. Geochim. Cosmochim. Acta 2010, 74, 6276–6286. [Google Scholar] [CrossRef]
- Passey, B.H.; Levin, N.E. Triple Oxygen Isotopes in Meteoric Waters, Carbonates, and Biological Apatites: Implications for Continental Paleoclimate Reconstruction. Rev. Mineral. Geochem. 2021, 86, 429–462. [Google Scholar] [CrossRef]
- Landais, A.; Barkan, E.; Luz, B. Record of δ18O and 17O-excess in ice from Vostok Antarctica during the last 150,000 years. Geophys. Res. Lett. 2008, 35, 1–5. [Google Scholar]
- Barkan, E.; Luz, B. Diffusivity fractionations of H216O/H 217O and H216O/H218O in air and their implications for isotope hydrology. Rapid Commun. Mass Spectrom. 2007, 21, 2999–3005. [Google Scholar] [CrossRef] [PubMed]
- Schoenemann, S.W.; Steig, E.J.; Ding, Q.; Markle, B.R.; Schauer. Triple water-isotopologue record from WAIS Divide, Antarctica: Controls on glacial-interglacial changes in 17O-excess of precipitation. J. Geophys. Res. Atmos. 2014, 119, 8741–8763. [Google Scholar]
- Merlivat, L.; Jouzel, J.O. Global climatic interpretation of the deuterium-oxygen 18 relationship for precipitation. J. Geophys. Res. 1979, 84, 5029–5033. [Google Scholar] [CrossRef]
- Jouzel, J.; Froehlich, K.; Schotterer, U. Deutérium et oxygène-18 dans les précipitations contemporaines: Données et modélisation. Hydrol. Sci. J. 1997, 42, 747–763. [Google Scholar] [CrossRef]
- Angert, A.; Cappa, C.D.; DePaolo, D.J. Kinetic 17O effects in the hydrologic cycle: Indirect evidence and implications. Geochim. Cosmochim. Acta 2004, 68, 3487–3495. [Google Scholar] [CrossRef]
- Landais, A.; Ekaykin, A.; Barkan, E.; Winkler, R.; Luz, B. Seasonal variations of 17O-excess and d-excess in snow precipitation at Vostok station, East Antarctica. J. Glaciol. 2012, 58, 725–733. [Google Scholar] [CrossRef] [Green Version]
- Vreča, P.; Kern, Z. Use of water isotopes in hydrological processes. Water 2020, 12, 2227. [Google Scholar] [CrossRef]
- Aron, P.G.; Levin, N.E.; Beverly, E.J.; Huth, T.E.; Passey, B.H.; Pelletier, E.M.; Poulsen, C.J.; Winkelstern, I.Z.; Yarian, D.A. Triple oxygen isotopes in the water cycle. Chem. Geol. 2020, 565, 120026. [Google Scholar] [CrossRef]
- Tian, C.; Wang, L.; Tian, F.; Zhao, S.; Jiao, W. O-excess in China. Geochim. Cosmochim. Acta. 2019, 260, 1–14. [Google Scholar] [CrossRef]
- Surma, J.; Assonov, S.; Staubwasser, M. Triple Oxygen Isotope Systematics in the Hydrologic Cycle. Rev. Mineral. Geochem. 2021, 86, 401–428. [Google Scholar] [CrossRef]
- Thiemens, M.H.; Heidenreich, J.E. The mass-independent fractionation of oxygen: A novel isotope effect and its possible cosmochemical implications. Science 1983, 219, 1073–1075. [Google Scholar] [CrossRef]
- Barkan, E.; Luz, B. High precision measurements of and 18O/16O ratios in H2O. Rapid Commun. Mass Spectrom. 2005, 19, 3737–3742. [Google Scholar] [CrossRef]
- Meijer, H.A.J.; Li, W.J. The Use of Electrolysis for Accurate δO and δO Isotope Measurements in Water. Isot. Environ. Health Stud. 1998, 34, 349–369. [Google Scholar] [CrossRef]
- Uemura, R.; Barkan, E.; Abe, O.; Luz, B. Triple isotope composition of oxygen in atmospheric water vapor. Geophys. Res. Lett. 2010, 37, 1–4. [Google Scholar] [CrossRef] [Green Version]
- Bao, H.; Cao, X.; Hayles, J.A. Triple Oxygen Isotopes: Fundamental Relationships and Applications. Annu. Rev. Earth Planet. Sci. 2016, 44, 463–492. [Google Scholar] [CrossRef]
- Schoenemann, S.W.; Schauer, A.J.; Steig, E.J. Measurement of SLAP2 and GISP δ17O and proposed VSMOW-SLAP normalization for δ17O and 17O excess. Rapid Commun. Mass Spectrom. 2013, 27, 582–590. [Google Scholar] [CrossRef]
- Pang, H.; Hou, S.; Landais, A.; Masson-Delmotte, V.; Prie, F.; Steen-Larsen, H.C.; Risi, C.; Li, Y.; Jouzel, J.; Wang, Y.; et al. Spatial distribution of 17O-excess in surface snow along a traverse from Zhongshan station to Dome, East Antarctica. Earth Planet. Sci. Lett. 2015, 414, 126–133. [Google Scholar] [CrossRef]
- Li, S.; Levin, N.E.; Chesson, L.A. Continental scale variation in 17O-excess of meteoric waters in the United States. Geochim. Cosmochim. Acta 2015, 164, 110–126. [Google Scholar] [CrossRef]
- Li, S.; Levin, N.E.; Soderberg, K.; Dennis, K.J.; Caylor, K.K. Triple oxygen isotope composition of leaf waters in Mpala, central Kenya. Earth Planet. Sci. Lett. 2017, 468, 38–50. [Google Scholar] [CrossRef]
- Landais, A.; Steen-Larsen, H.C.; Guillevic, M.; Masson-Delmotte, V.; Vinther, B.; Winkler, R. Triple isotopic composition of oxygen in surface snow and water vapor at NEEM (Greenland). Geochim. Cosmochim. Acta. 2012, 77, 304–316. [Google Scholar] [CrossRef]
- Passey, B.H.; Ji, H. Triple oxygen isotope signatures of evaporation in lake waters and carbonates: A case study from the western United States. Earth Planet. Sci. Lett. 2019, 518, 1–12. [Google Scholar] [CrossRef]
- Martin, F.M. Isotopic fractionation and the quantification of 17O anomalies in the oxygen three-isotope system: An appraisal and geochemical significance. Geochim. Cosmochim. Acta. 2002, 66, 1881–1889. [Google Scholar]
- McKeegan, K.D.; Leshin, L.A. Stable isotope variations in extraterrestrial materials. Rev. Mineral. Geochem. 2001, 43, 278–318. [Google Scholar] [CrossRef] [Green Version]
- Baker, L.; Franchi, I.A.; Maynard, J.; Wright, I.P.; Pillinger, C.T. A Technique for the Determination of 18O/16O and O/16O isotopic Ratios in Water from Small Liquid and Solid Samples. Anal. Chem. 2002, 74, 1665–1673. [Google Scholar] [CrossRef]
- Jabeen, I.; Kusakabe, M. Determination of δ17O values of reference water samples VSMOW and SLAP. Chemical Geology 1997, 2541. [Google Scholar] [CrossRef]
- Surma, J.; Assonov, S.; Bolourchi, M.J.; Staubwasser, M. Triple oxygen isotope signatures in evaporated water bodies from the Sistan Oasis, Iran. Geophys. Res. Lett. 2015, 42, 8456–8462. [Google Scholar] [CrossRef] [Green Version]
- Affek, H.P.; Barkan, E. A new method for high-precision measurements of 17O/16O ratios in H2O. Rapid Commun. Mass Spectrom. 2018, 32, 2096–2097. [Google Scholar] [CrossRef] [PubMed]
- Barkan, E.; Musan, I.; Luz, B. High-precision measurements of δ17O and 17Oexcess of NBS19 and NBS18. Rapid Commun. Mass Spectrom. 2015, 29, 2219–2224. [Google Scholar] [CrossRef]
- Schauer, A.J.; Schoenemann, S.W.; Steig, E.J. Routine high-precision analysis of triple water-isotope ratios using cavity ring-down spectroscopy. Rapid Commun. Mass Spectrom. 2016, 30, 2059–2069. [Google Scholar] [CrossRef] [PubMed]
- Wassenaar, L.I.; Ahmad, M.; Aggarwal, P.; Van Duren, M.; Pöltenstein, L.; Araguas, L.; Kurttas, T. Worldwide proficiency test for routine analysis of δ2H and δ18O in water by isotope-ratio mass spectrometry and laser absorption spectroscopy. Rapid Commun. Mass Spectrom. 2012, 26, 1641–1648. [Google Scholar] [CrossRef]
- Pierchala, A.; Rozanski, K.; Dulinski, M.; Gorczyca, Z.; Marzec, M.; Czub, R. High-precision measurements of δ2H, δ18O and δ17O in water with the aid of cavity ring-down laser spectroscopy. Isot. Environ. Health Stud. 2019, 55, 290–307. [Google Scholar] [CrossRef] [PubMed]
- Berman, E.S.F.; Levin, N.E.; Landais, A.; Li, S.; Owano, T. Measurement of δ18O, δ17O, and 17O-excess in Water by Off-Axis Spectrometry, Integrated Cavity Output Spectroscopy and Isotope Ratio Mass. NIH Public Access 2013, 85, 10392–10398. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Steig, E.J.; Gkinis, V.; Schauer, A.J.; Schoenemann, S.W.; Samek, K.; Hoffnagle, J.; Dennis, K.J.; Tan, S.M. Calibrated high-precision 17O-excess measurements using cavity ring-down spectroscopy with laser-current-tuned cavity resonance. Atmos. Meas. Tech. 2014, 7, 2421–2435. [Google Scholar] [CrossRef] [Green Version]
- Sturm, P.; Knohl, A. Water vapor δ2H and δ18O measurements using off-axis integrated cavity output spectroscopy. Atmos. Meas. Tech. 2010, 3, 67–77. [Google Scholar] [CrossRef] [Green Version]
- Wassenaar, L.I.; Terzer-Wassmuth, S.; Douence, C.; Araguas-Araguas, L.; Aggarwal, P.K.; Coplen, T.B. Seeking excellence: An evaluation of 235 international laboratories conducting water isotope analyses by isotope-ratio and laser-absorption spectrometry. Rapid Commun. Mass Spectrom. 2018, 32, 393–406. [Google Scholar] [CrossRef] [PubMed]
- Skrzypek, G.; Ford, D. Stable isotope analysis of saline water samples on a cavity ring-down spectroscopy instrument. Environ. Sci. Technol. 2014, 48, 2827–2834. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hendry, M.J.; Richman, B.; Wassenaar, L.I. Correcting for methane interferences on δ2H and δ18O measurements in pore water using H2O (liquid)-H2O (vapor) equilibration laser spectroscopy. Anal. Chem. 2011, 83, 5789–5796. [Google Scholar] [CrossRef] [PubMed]
- Risi, C.; Landais, A.; Winkler, R.; Vimeux, F. Geoscientific Instrumentation Methods and Data Systems Can we determine what controls the spatio-temporal distribution of d-excess and O-excess in precipitation using the LMDZ general circulation model? Clim. Past. 2013, 9, 2173–2193. [Google Scholar] [CrossRef] [Green Version]
- Horita, J.; Wesolowski, D.J. Horita and Wesolowski 1994. Geochim. Cosmochim. Acta. 1994, 58, 1–13. [Google Scholar]
- Tian, C.; Jiao, W.; Beysens, D.; Kaseke, K.F.; Medici, M.-G.; Li, F.; Wang, L. Investigating the role of evaporation in dew formation under different climates using 17O-excess. J. Hydrol. 2021, 592, 125847, ISSN 0022-1694. [Google Scholar] [CrossRef]
- Affolter, S.; Häuselmann, A.D.; Fleitmann, D.; Häuselmann, P.; Leuenberger, M. Triple isotope (δD, δ17O, δ18O) study on precipitation, drip water and speleothem fluid inclusions for a Western Central European cave (NW Switzerland). Quat. Sci. Rev. 2015, 127, 73–89. [Google Scholar] [CrossRef]
- Landais, A.; Risi, C.; Bony, S.; Vimeux, F.; Descroix, L.; Falourd, S.; Bouygues, A. Combined measurements of 17O excess and d-excess in African monsoon precipitation: Implications for evaluating convective parameterizations. Earth Planet. Sci. Lett. 2010, 298, 104–112. [Google Scholar] [CrossRef]
- Uechi, Y.; Uemura, R. Dominant influence of the humidity in the moisture source region on the 17O-excess in precipitation on a subtropical island. Earth Planet. Sci. Lett. 2019, 513, 20–28. [Google Scholar] [CrossRef]
- Bershaw, J.; Hansen, D.D.; Schauer, A.J. Deuterium excess and 17O-excess variability in meteoric water across the Pacific Northwest, USA. Tellus B Chem. Phys. Meteorol. 2020, 72, 1–17. [Google Scholar] [CrossRef]
- Miller, M.F. Precipitation regime influence on oxygen triple-isotope distributions in Antarctic precipitation and ice cores. Earth Planet. Sci. Lett. 2018, 481, 316–327. [Google Scholar] [CrossRef]
- Winkler, R.; Landais, A.; Risi, C.; Baroni, M.; Ekaykin, A.; Jouzel, J.; Petit, J.R.; Prie, F.; Minster, B.; Falourd, S. Interannual variation of water isotopologues at Vostok indicates a contribution from stratospheric water vapor. Proc. Natl. Acad. Sci. USA 2013, 110, 17674–17679. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Schoenemann, S.W.; Steig, E.J. Seasonal and spatial variations of 17O excess and d-excess in Antarctic precipitation: Insights from an intermediate complexity isotope model. J. Geophys. Res. Atmos. 2016, 215–247. [Google Scholar]
- Winkler, R.; Landais, A.; Sodemann, H.; Dümbgen, L.; Prié, F.; Masson-Delmotte, V.; Stenni, B.; Jouzel, J. Deglaciation records of 17O-excess in East Antarctica: Reliable reconstruction of oceanic normalized relative humidity from coastal sites. Clim. Past. 2012, 8, 1–16. [Google Scholar] [CrossRef] [Green Version]
- Touzeau, A.; Landais, A.; Stenni, B.; Uemura, R.; Fukui, K.; Fujita, S.; Guilbaud, S.; Ekaykin, A.; Casado, M.; Barkan, E.; et al. Acquisition of isotopic composition for surface snow in East Antarctica and the links to climatic parameters. Cryosphere 2016, 10, 837–852. [Google Scholar] [CrossRef] [Green Version]
- Pang, H.; Hou, S.; Landais, A.; Delmotte, V.M.; Jouzel, J. Influence of Summer Sublimation on δD, δ18O, and δ17O in Precipitation, East Antarctica, and Implications for Climate Reconstruction From Ice Cores. J. Geophys. Res. Atmos. 2019, 124, 7339–7358. [Google Scholar]
- Zahn, A.; Franz, P.; Bechtel, C.; Grooß, J.U.; Röckmann, T. Modelling the budget of middle atmospheric water vapour isotopes. Atmos. Chem. Phys. 2006, 6, 2073–2090. [Google Scholar] [CrossRef] [Green Version]
- Tian, C.; Wang, L. Data Descriptor: Stable isotope variations of daily precipitation from 2014–2018 in the central United States. Nat. Publ. Gr. 2019, 9, 1–8. [Google Scholar]
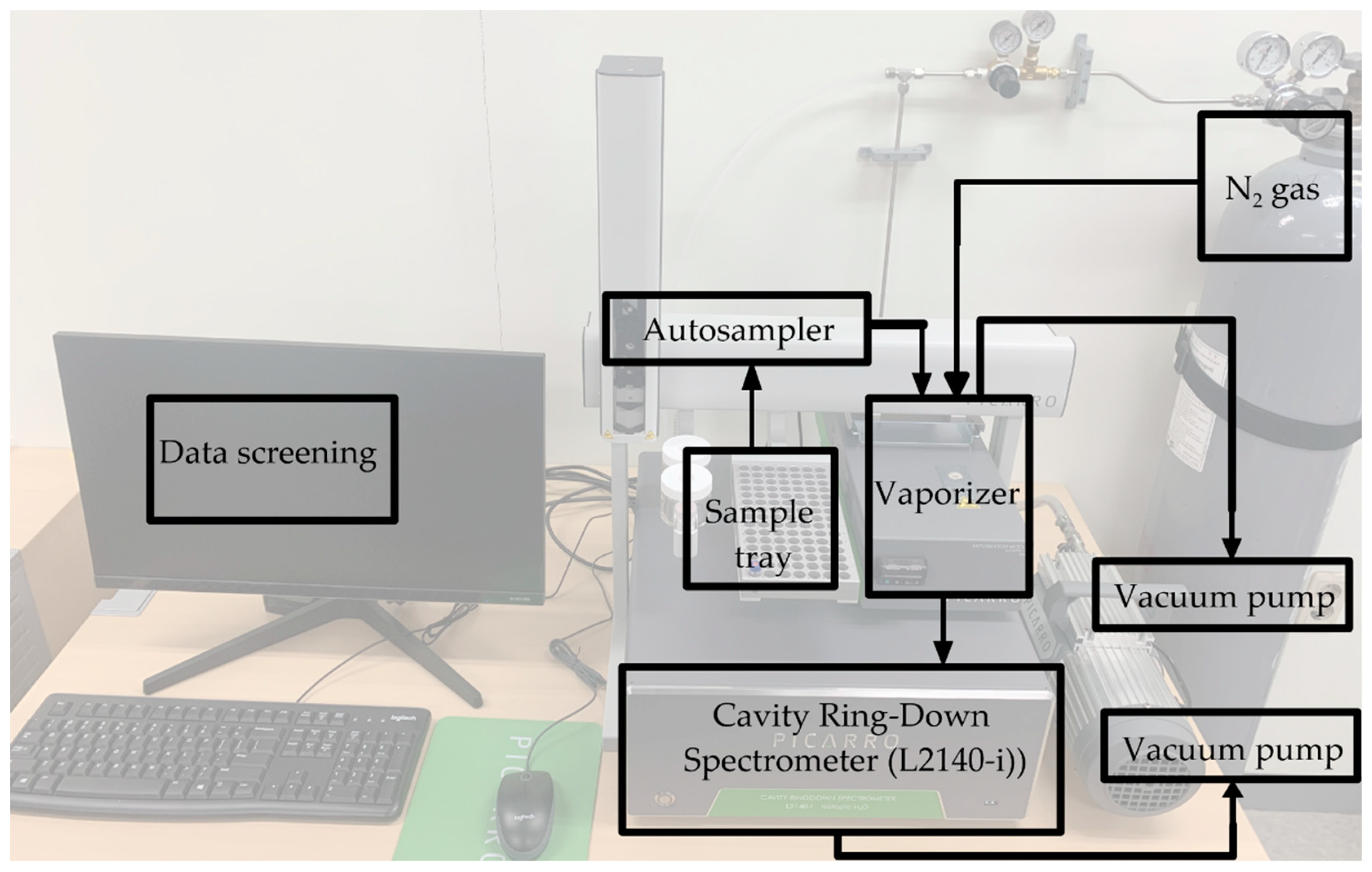
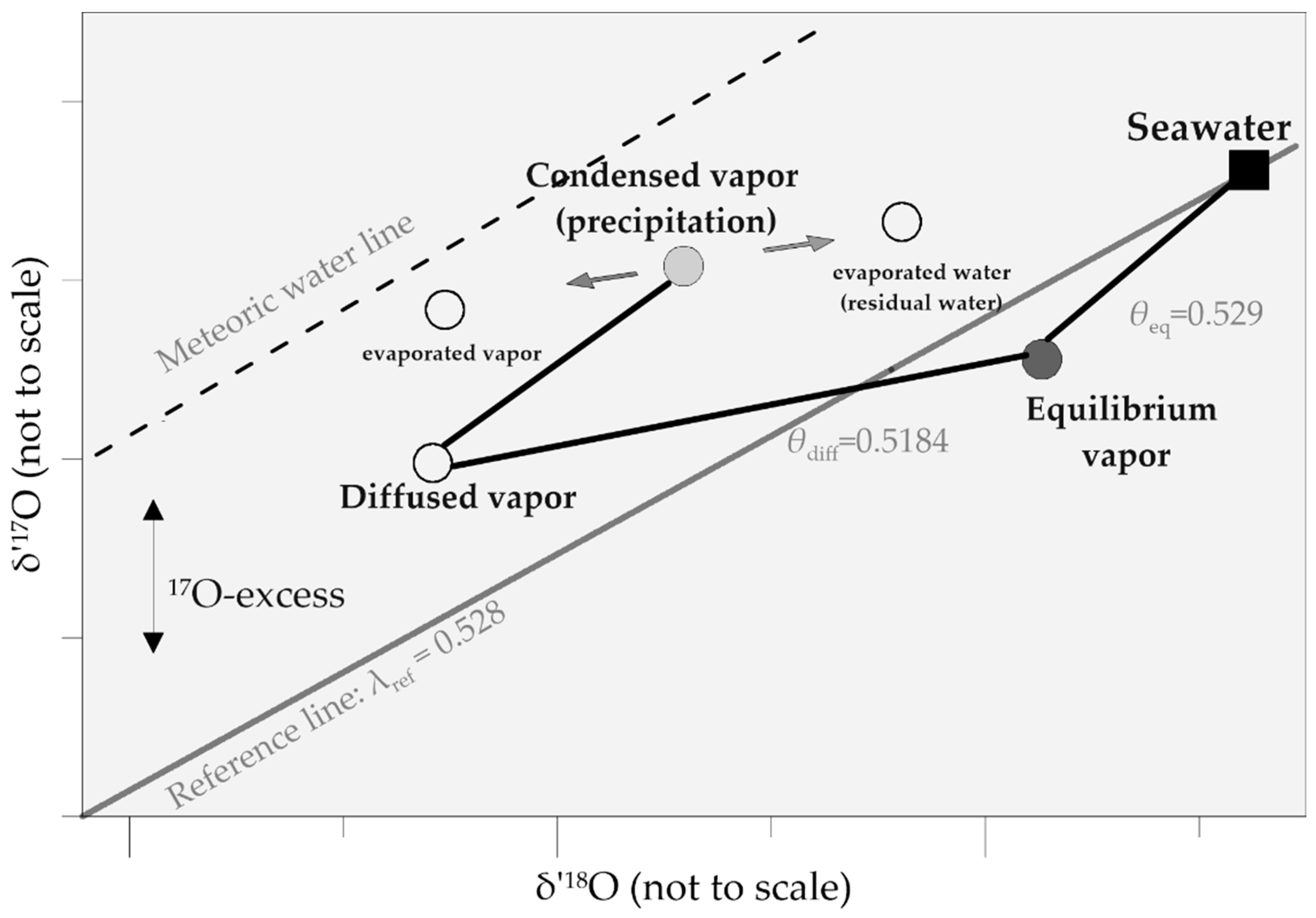
| Isotope | Abundance | δ Notation | δ Value of VSMOW2 | δ Value of SLAP2 | δ Value of GISP |
|---|---|---|---|---|---|
| 16O | 99.76% | – | |||
| 17O | 0.038% | δ17O | 0 | −29.6986‰ 1 | −13.16 ± 0.05 1 |
| 18O | 0.20% | δ18O | 0 | −55.5‰ 2 | −24.82 ± 0.08 1 |
| Sample Type | δ18O Range | 17O-Excess Mean or Range (Per meg) | λref | Analytical Method | Time Scale | Reference |
|---|---|---|---|---|---|---|
| Low- and mid-latitude Precipitation | ||||||
| Vapor (Mt.Zugspitze, Germany) | (−34.4 to –20.4) | (30 to 82) | 0.5265 | IRMS | 2016 (Feb to May) | [38] |
| Cave water (Canada and USA) | −9.69 (−17.67 to −6.39) | 0.05 (0.03 to 0.07) | 0.530 | IRMS | 2005 (Feb, Jul) | [26] |
| Cave drip waters (Northwest Switzerland) | −8.7 | 19 | – | Picarro CRDS | 2010 (Nov) to 2014 (Jun) | [69] |
| Fluid inclusions (Northwest Switzerland) | −8.3 | 10 | Picarro CRDS | 2012 (Mar) to 2014 (Jun) | [69] | |
| Leaf water (Mpala central Kenya) | 7.84 (−0.27 to 16.14) | −0.06 (−0.16 to 0.04) | IRMS | 2012 (Jun–Jul) | [47] | |
| Stem water (Mpala central Kenya) | −2.46 (−4.71 to 0.59) | 0.02 (0.01 to 0.03) | IRMS | 2012 (Jun–Jul) | [47] | |
| Pond (Sistan Oasis, Iran) | 25.83 (13.81 to 29.07) | −144.4 (−172 to –56) | IRMS | – | [54] | |
| Lake and pond | −8.06 (−16.03 to 4.19) | 0.02 (−0.02 to 0.04) | 0.528 | IRMS | 2002, 2008 | [26] |
| Surface water | −8.88 (−20.31 to 9.56) | −46.4 to 55.67 (mostly 14 to 33) | 0.528 | IRMS | Global scale | [36] |
| River, terminal lakes, well, spring, irrigation channel (Sistan Oasis, Iran) | −1.69 (−6.84 to 14.03) | −3.91 (−59 to 25) | – | IRMS | – | [54] |
| Lake and river (western USA) | −9.58 (−19.11 to −0.34) | 5.28 (−39 to 46) | IRMS | [49] | ||
| Dam water (Mpala central Kenya) | −1.08 (−3.98 to 2.26) | 0.01 (−0.004 to 0.02) | IRMS | 2012 (Jun–Jul) | [47] | |
| Tap, spring water (Israel) | −4.99 (−5.74 to –4.24) | 0.04 (0.03 to 0.05) | 0.515 | IRMS | 2008 | [26] |
| Tap water (continental USA) | −8 ± 4.7 (−20.7 to −0.7) | 17 ± 11 (−6 to 43) | 0.526 to 0.527 | IRMS | 2006 to 2011 | [46] |
| Tap and puddle water (Mpala central Kenya) | 3.36 (2.33 to 4.39) | −0.01 (−0.01 to −0.003) | – | IRMS | 2012 (Jun–Jul) | [47] |
| Precipitation (Northwest Switzerland) | −9.9 | 18 | Picarro CRDS | 2012 (Mar) to 2014 (Jun) | [69] | |
| Precipitation (Okinawa Island, Japan) | −4.81 (−9.88 to −1.05) | 25.26 (4 to 54) | Picarro CRDS | 2011 (Jan) to 2012 (Dec) | [71] | |
| Precipitation (central USA) | −6.25 | 31 | 0.5275 | OA-ICOS | 2014 (Jun) to 2018 (May) | [80] |
| Rain (Indonesia, India, and Israel) | −5.45 (−9.03 to –2.53) | 0.04 (0.02 to 0.06) | 0.522 | IRMS | 2005 (Mar to Nov) 2001 to 2008 2008 (Feb to Aug) | [26] |
| Water vapor (south Indian and Southern Ocean) | −15.45 (−23.41 to −11.65) | 13.51 (−6 to 46) | 0.532 | IRMS | 2005 (Dec) to 2006 (Jan) | [42] |
| Seawater (Atlantic, Pacific Ocean, Mediterranean, and Northern Red Sea) | 0.42 (−0.42 to 2.43) | −0.0045 (−0.01 to 0.0038) | 0.528 | IRMS | – | [26] |
| Snow (Mt.Zugspitze, Germany) | (−21.7 to −8.3) | (17 to 62) | – | IRMS | [38] | |
| Snow, ice (Canada, Montenegro) | −17.34 (−26.11 to −5.44) | 0.02 (−0.01 to 0.04) | 0.529 | IRMS | 2009, 2010 | [26] |
| High-latitude precipitation | ||||||
| Vapor (NEEM, Greenland) | −41.77 (−44.63 to −38.33) | 32.33 (15 to 48) | 0.528 | IRMS | 2008 (Aug) | [48] |
| Snow precipitation (NEEM, Greenland) | −29.60 (−35.53 to −24.33) | 35.67 (23 to 43) | IRMS | 2008 (Aug) | [48] | |
| Firn core (NEEM, Greenland) | −32.17 (−38.80 to −26.70) | 49.70 (30 to 73) | – | IRMS | 2003 (Jan) to 2005 (Aug) | [48] |
| Snow pit (Vostok) | −57.13 (−61.20 to −51.39) | 7.43 (−36 to 42) | IRMS | 1949 to 2008 | [74] | |
| Surface snow (traverse from Zhongshan station to Dome A) | −34.30 (−58.69 to −17.60) | 34.95 (9 to 51) | IRMS | 2009 (Dec) to 2010 (Jan) | [45] | |
| Snow (transect from Syowa to Dome Fuji) | −47.26 (−56.69 to −30.20) | 24.82 (6.1 to 43.8) | – | IRMS | – | [77] |
| Ice core (Vostok) | −58 (−50.74 to −62.05) | 24.31 (−6 to 54) | IRMS | 150ka years | [28] | |
| Surface snow (Vostok, Antarctica) | −41.27 (−28.23 to −51.03) | 44.5 (25 to 62) | 0.528 | IRMS | – | [28] |
| Snow or ice (Dome F, Antarctica) | −58.42 | −0.006 | – | IRMS | [26] | |
| Ice core (Talos Dome, Antarctica) | −41.67 to −36.52 | – | 0.5278 | IRMS | 9.15 to 33.78 ka age | [76] |
| Ice core (WAIS, Antarctica) | −35.44 (−42.68 to −31.61) | 25.22 (2.9 to 38.46) | – | IRMS | 25 ka | [30] |
| Ice core (Taylor Dome, Antarctica) | −39.56 (−42.92 to −37.36) | 14.28 (−1 to 28) | 0.5313 | IRMS | – | [30] |
| Ice core (Siple Dome Antarctica) | −31.31 (−36.21 to −24.02) | 19 (8 to 27) | – | IRMS | [30] | |
| Snow precipitation (Vostok, Antarctica) | −68.47 to −50.51 | −27 to 29 | 0.5308 | IRMS | 2000 (Feb–Oct) | [34] |
| Snow precipitation (Vostok, Antarctica) | −58.5 to −52.6 | – | 0.5269 | IRMS | 1999 (Dec) to 2000 (Jun) | [77] |
| Ice core (Dome C, Antarctica) | −56.48 to −46.639 | 0.5294 | IRMS | 7.61–24.8 ka age | [76] | |
| Snow precipitation (Dome C, Antarctica) | −55.58 (−69.63 to –38.89) | 18.43 (−11 to 47) | 0.5282 | IRMS | 2010 (Sep–Nov) | [77] |
| Snow (Dome C, Antarctica) | −54.25 (−61.35 to −48.19) | 31.64 (14 to 47) | 0.5287 | IRMS | 2010 (Dec) to 2011 (Dec) | [77] |
| Snow pit (Dome C, Antarctica) | −51.14 (−55.31 to −46.06) | 31.68 (17 to 51) | 0.528 | IRMS | – | [77] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nyamgerel, Y.; Han, Y.; Kim, M.; Koh, D.; Lee, J. Review on Applications of 17O in Hydrological Cycle. Molecules 2021, 26, 4468. https://doi.org/10.3390/molecules26154468
Nyamgerel Y, Han Y, Kim M, Koh D, Lee J. Review on Applications of 17O in Hydrological Cycle. Molecules. 2021; 26(15):4468. https://doi.org/10.3390/molecules26154468
Chicago/Turabian StyleNyamgerel, Yalalt, Yeongcheol Han, Minji Kim, Dongchan Koh, and Jeonghoon Lee. 2021. "Review on Applications of 17O in Hydrological Cycle" Molecules 26, no. 15: 4468. https://doi.org/10.3390/molecules26154468
APA StyleNyamgerel, Y., Han, Y., Kim, M., Koh, D., & Lee, J. (2021). Review on Applications of 17O in Hydrological Cycle. Molecules, 26(15), 4468. https://doi.org/10.3390/molecules26154468







